 Journal of Quantum Information Science, 2013, 3, 34-41 http://dx.doi.org/10.4236/jqis.2013.31008 Published Online March 2013 (http://www.scirp.org/journal/jqis) Measurement-Induced Nonlocality and Geometric Discord in the Spin-Boson Model Guoyou Wang, Zilong Fan, Haosheng Zeng* Key Laboratory of Low-Dimensional Quantum Structures and Quantum Control of Ministry of Education, Department of Physics, Hunan Normal University, Changsha, China Email: *hszeng@hunnu.edu.cn Received December 9, 2012; revised January 22, 2013; accepted February 1, 2013 ABSTRACT Dynamics of measurement-induced-nonlocality (MIN) and geometric measure of discord (GD) in the spin-boson model are studied. Analytical results show that for two large classes of initial states, MINs are equal but GDs are different. At the end of evolution, MIN and GD initially stored in the spin system transfer completely to reservoirs. The quantum beats for MIN and GD are also found, which are the results of quantum interference between two local non-Markovian dynamics via quantum correlation. Keywords: Measurement-Induced Nonlocality; Geometric Discord; Quantum Beat 1. Introduction Quantum correlation arises from noncommutativity of oper- ators representing states, observables, and measurements [1]. Quantum entanglement, as the earliest known quan- tum correlation, has acquired extensive research and is found to be an useful resource in quantum communica- tion and quantum computation [2]. However, entangle- ment is not the unique kind of quantum correlation. A more general concept, quantum discord [3], was found, which is regarded as the measure of all nonclassical cor- relations in a bipartite system, being the entanglement of a particular case of it. It was shown that there exists sepa- rable states with nonzero discord which can be used to perform quantum computation [4,5]. Quantum discord is also useful in the studies of quantum phase transition [6,7] and estimation of quantum correlations in Grover search algorithm [8]. Unfortunately, evaluation of quantum dis- cord in general requires considerable numerical minimi- zation and analytical results that are known only for cer- tain classes of states [9-11]. More recently, two measures out of geometric perspective in measurements, GD [12] and MIN [13], were proposed, which as the authors shown have the merit of easier evaluation. In fact, Luo and Fu [14,15] have evaluated the GD for some typical classes of states and found the tight lower bound. The dissecting about the meaning of GD was also done [16,17]. Realistic quantum systems cannot avoid interactions with their environments, leading to the change of quan- tum correlation. In the last decade, the influences of en- vironments to quantum entanglement [18] and quantum discord [19] have been investigated extensively. An in- teresting phenomenon, named as “entanglement sudden death” (ESD) [20,21] for a pair of entangled qubits ex- posed to local Markovian environments was found. In contrast, quantum discord in similar conditions decays only in asymptotic time [22], which signifies that quan- tum discord is more robust against Markovian noise than entanglement. There are also many works involved in the evolutions of quantum entanglement and discord in the non-markovian environments [23-29]. Especially, trap- ping [30] and quantum beats [31] for quantum entangle- ment and discord for a pair of qubits in local structured environments were found. In this paper, we investigate the dynamics of GD and MIN in a system that consists of two independent spins (qubits) coupled respectively to their local environments. Our motivation is to find the evolutional properties of GD and MIN and the difference between their evolutions and make a comparison between the evolutions of quantum entanglement or/and discord. The paper is constructed as follows. In Section 2, we introduce the original definitions of GD and MIN. In Section 3, we first introduce the inter- action model, and then study the evolution of GD and MIN of different partitions for two classes of initial states. Section 4 is devoted to the study of quantum beats for GD and MIN. And the conclusion is arranged in Section 5. 2. GD and MIN Let be a bipartite state shared by parties A and B. The GD of is defined as [12], *Corresponding author. C opyright © 2013 SciRes. JQIS  G. Y. WANG ET AL. 35 2 min , A A D (1) where the min is taken over all von Neumann measure- ments on subsystem A, and the Hilbert Schmidt A norm defined by 2† trX X. On the other hand, the MIN is defined as [13] 2 max A A N , (2) here the max is taken over the von Neumann measure- ments which do not disturb A locally, that is, AA AA kk k . For a two-qubit system, the general state may be writ- ten as, 3 1 33 1,1 1 4 . AB AB ii i AB AB ii ijij iij x yt 11 1 1 (3) Here i are the Pauli operators, and AB ii xTr 1 AB i y i Tr 1 ij tTr are the components of the local Bloch vectors, AB i j ij Tt are the components of the correlation tensor . For this state, we have [12,13] 22 max 1, 4 DxTk (4) and 2 3 11 if 0, 4 1if 0. 4 ttt t trTTx TTxx x N trTT x (5) where 22 i i x with 123 ,, t xxx t , is the max k t maximum eigenvalue of matrix xTT, and 3 the minimum eigenvalue of matrix . t TT 3. Model and Dynamics of MIN and GD The model we consider consists of two independent spins interacting respectively with their local boson reservoirs. The total Hamiltonian is, 2† 0 ,,, 1 2† ,, , 1 2 . z iikiki ik iki iki ik ik Hb gb b k b (6) Here i and ii are the Pauli and raising (low- ering) operators for the spin. is the crea- ith † ,,ikik bb ith tion (annihilation) operator of the mode in the reservoir with corresponding frequency ,ik ith . The cou- pling strength between spin and its reservoir mode is denoted by ,ik i k . For simplicity, we assume the two spins have equal Zeeman splitting 0 , and the reservoirs are initially in vacuum states and not correlated to the spins. Under these conditions, the model can be solved exactly for any initial state of the spins and any form of spectral reservoirs. In this paper, we will mainly discuss two types of reservoirs—unstructured flat reservoirs and structured Lorentzian reservoirs, and also two large types of initial states of spins—double-excitation and one-exci- tation states. 3.1. Double-Excitation Dynamics of MIN and GD Let us first study the double-excitation case, i.e., the joint initial state for the whole system is, 12 2 00 1100, 121 0 sssrr (7) where 22 1 , and the collective state 00 ii k rr k represents vacuum state of reservoir . i r Due to the conservation of excitation number under the evolution of Jaynes-Cummings model, the dynamical state of the whole system has the form, 11 12 2 10 10 sr sr ss t 11 22 22 12 11 2 01 01 0000. sr sr rr tt t t (8) Here we introduce the collective state 1 1 ii kk k rr i t t 1 to denote the one-excitation state of the reservoir i [19,32]. The coefficients r it and it , which are determined by the quantum dy- namical equation, satisfy 111 0d t ii ttfttt , (9) and 22 1. ii tt , (10) Here the kernel function is defined by 01 1de itt ii ftt J with spectral density 2 ,,iik k Jg ik . The reduced density matrix for the two spins reads Copyright © 2013 SciRes. JQIS 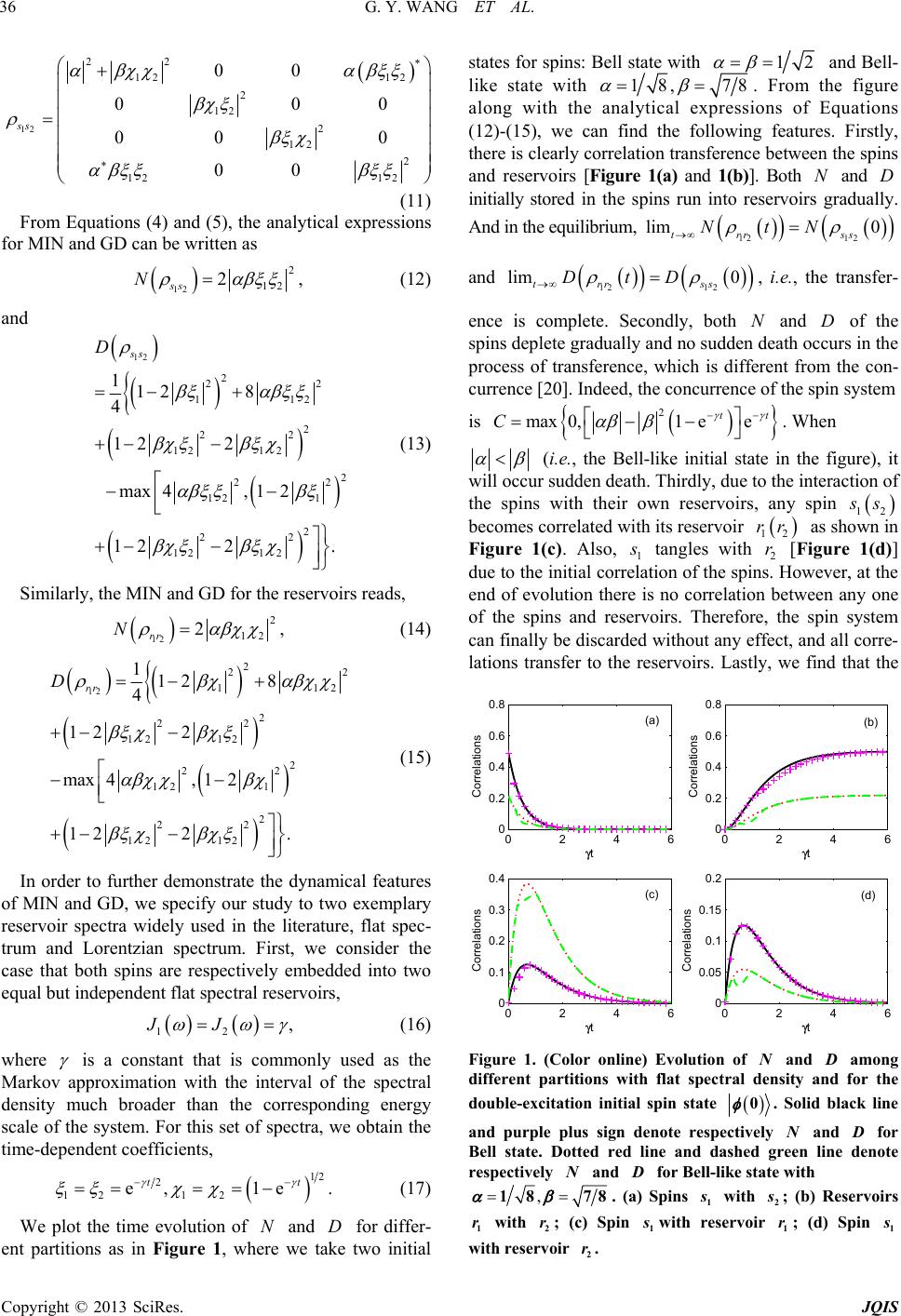 G. Y. WANG ET AL. 36 12 22 12 12 2 12 2 12 2 12 12 00 00 00 0 00 ss 0 (11) From Equations (4) and (5), the analytical expressions for MIN and GD can be written as 12 2 12 2 ss N , (12) and 12 2 22 112 2 22 121 2 2 22 12 1 2 22 121 2 1128 4 122 max4, 12 12 2. ss D (13) Similarly, the MIN and GD for the reservoirs reads, 12 2 12 2 rr N , (14) 12 2 22 11 2 22 12 12 2 22 12 1 2 22 12 12 112 8 4 12 2 max4, 12 12 2. rr D2 (15) In order to further demonstrate the dynamical features of MIN and GD, we specify our study to two exemplary reservoir spectra widely used in the literature, flat spec- trum and Lorentzian spectrum. First, we consider the case that both spins are respectively embedded into two equal but independent flat spectral reservoirs, 12,JJ (16) where is a constant that is commonly used as the Markov approximation with the interval of the spectral density much broader than the corresponding energy scale of the system. For this set of spectra, we obtain the time-dependent coefficients, 12 2 121 2 e,1e . (17) tt We plot the time evolution of and for differ- ent partitions as in Figure 1, where we take two initial states for spins: Bell state with ND 12 and Bell- like state with 18, 78 . From the figure along with the analytical expressions of Equations (12)-(15), we can find the following features. Firstly, there is clearly correlation transference between the spins and reservoirs [Figure 1(a) and 1(b)]. Both and initially stored in the spins run into reservoirs gradually. N D And in the equilibrium, 0 ss 121 2 limtrr Nt N and 1 20 ss D 12 limtrr Dt , i.e., the transfer- ence is complete. Secondly, both and of the spins deplete gradually and no sudden death occurs in the process of transference, which is different from the con- currence [20]. Indeed, the concurrence of the spin system ND is 2 max 0,1ee t C t . When (i.e., the Bell-like initial state in the figure), it will occur sudden death. Thirdly, due to the interaction of the spins with their own reservoirs, any spin 12 s becomes correlated with its reservoir 1 ras shown in Figure 1(c). Also, 1 2 r tangles with 2 r [Figure 1(d)] due to the initial correlation of the spins. However, at the end of evolution there is no correlation between any one of the spins and reservoirs. Therefore, the spin system can finally be discarded without any effect, and all corre- lations transfer to the reservoirs. Lastly, we find that the 0 2 4 6 0 0.2 0.4 0.6 0.8 γt Correlations 0 2 4 6 0 0.2 0.4 0.6 0.8 γt Correlations 0 2 4 6 0 0.1 0.2 0.3 0.4 γt Correlations 0 2 4 6 0 0.05 0.1 0.15 0.2 γt Correlations (b) (a) (c) (d) Figure 1. (Color online) Evolution of and N among different partitions with flat spectral density and for the double-excitation initial spin state 0 . Solid black line and purple plus sign denote respectively and N for Bell state. Dotted red line and dashed green line denote respectively and N for Bell-like state with ,18 78 . (a) Spins with ; (b) Reservoirs with ; (c) Spin with reservoir ; (d) Spin s1s2 r1 r1 with r2s1s1 reservoir r2. Copyright © 2013 SciRes. JQIS 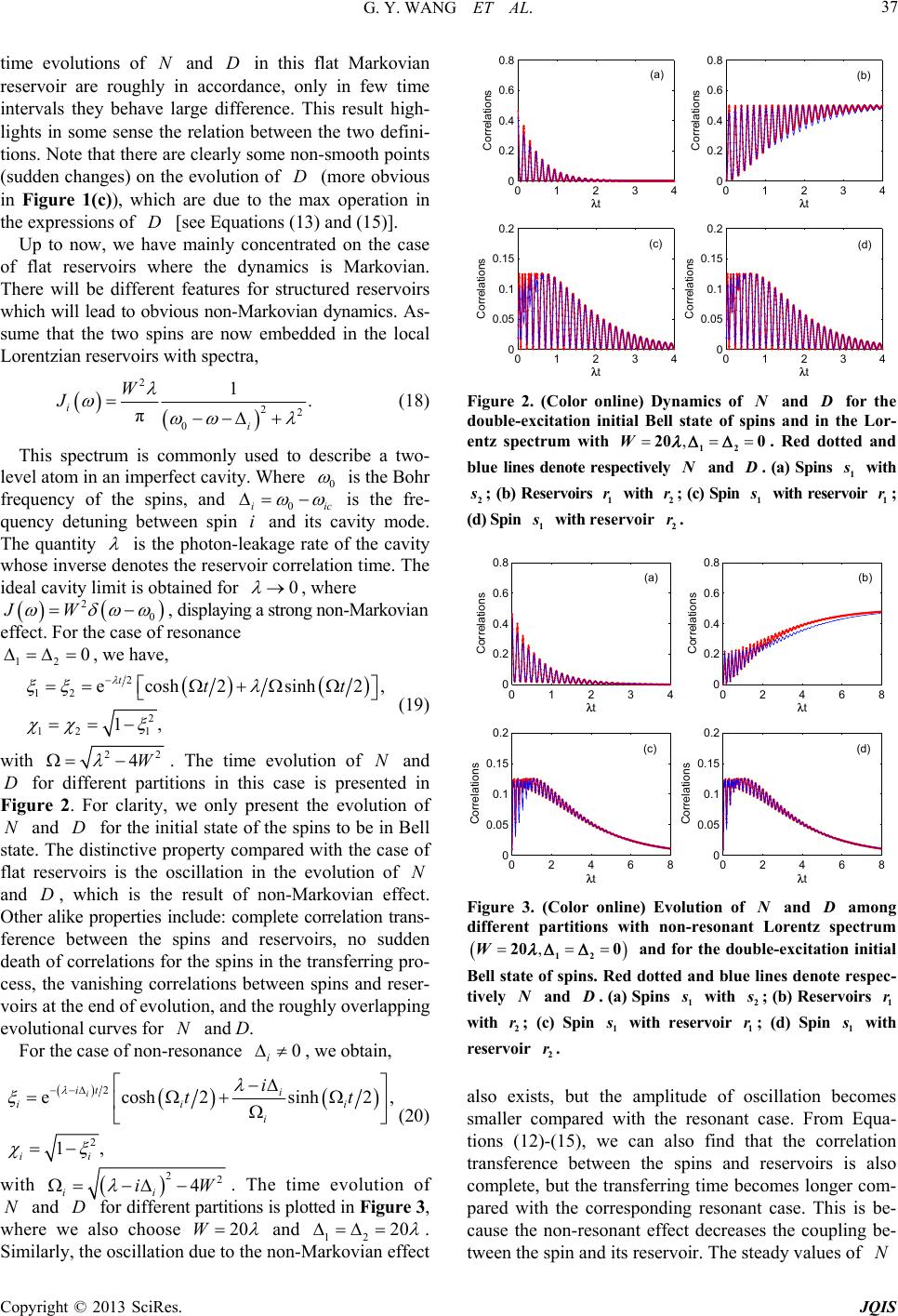 G. Y. WANG ET AL. 37 time evolution of s and in this flat Markovian se of N D dreservoir are roughly in accor ance, only in few time intervals they behave large difference. This result high- lights in some sense the relation between the two defini- tions. Note that there are clearly some non-smooth points (sudden changes) on the evolution of D (more obvious in Figure 1(c)), which are due to theax operation in the expressions of D [see Equations (13) and (15)]. Up to now, we have mainly concentrated on the ca m flat reservoirs where the dynamics is Markovian. There will be different features for structured reservoirs which will lead to obvious non-Markovian dynamics. As- sume that the two spins are now embedded in the local Lorentzian reservoirs with spectra, 21W 22 0 . π i i J (18) This spectrum is commonly used to describe a two- level atom in an imperfect cavity. Where 0 is the Bohr frequency of the spins, and 0iic ts c is the fre- quency detuning between spin avity mode. The quantity i and i is the photon-leakage rate of the cavity whose inversenotes the reservoir correlation time. The ideal cavity limit is obtained for 0 de , where 2 0 JW , displaying a strong non-M resonance 12 0 , we have, arkovian effect. For thof e case 2 ecosh t 12 2 12 1 2 sinh2, 1, tt (19) with 22 4W . The time evolution of and es , we obtain, N D for different partitions in this case is presented in ure 2. For clarity, we only present the evolution of N and D for the initial state of the spins to be in Bell te. Theistinctive property compared with the case of flat reservoirs is the oscillation in the evolution of N and D, which is the result of non-Markovian effe Other alike properties include: complete correlation trans- ference between the spins and reservoirs, no sudden death of correlations for the spins in the transferring pro- cess, the vanishing correlations between spins and reser- voirs at the end of evolution, and the roughly overlapping evolutional curves for N and D. For the case of non-ronance Fig sta d ct. 0 i 2 i it 2 ecosh2sinh2, 1, i iii i ii i tt (20) with 22 4 ii iW 01234 0 0.2 0.4 0.6 0.8 . The time evolution of dfor different parti N antions is plotted in Figure 3, ere we also choose 20W D wh and 12 20 . Similarly, the oscillation due to the non-Markovian effect λt Correlations 0.8 01234 0 0.2 0.4 0.6 λt Correlations 01234 0 0.05 0.1 0.15 0.2 λt Correlations 0 12 3 4 0 0.05 0.1 0.15 0.2 λt Correlations (a) (b) (c) (d) Figure 2. (Color online) Dynamics of and N for the double-excitation initial Bell state of s ande Lorpins in th- entz spectrum with , W12 20 0 . Red dotted and blue lines denote respectively N and . (a) Spins s1 with s2; (b) Reservoirs r1 with2ith reservoir r1; (d) Spin s1 with reservoir r2 r; (c) Spin . s1 w 01234 0 0.2 0.4 0.6 0.8 λt Correlations 0.8 0246 0 0.2 0.4 0.6 λt Correlations 02468 0 0.05 0.1 0.15 0.2 λt Correlations 0 2 4 6 0 0.05 0.1 0.15 0.2 λt Correlations (b) (a) (c) (d) Figure 3. (Color online) Evolution of and N among different partitions with non-resonanntectrut Lorez spm , W12 20 0 and for the doub-excitatn initial Bell state of spins. Red dotted and blue lines denote respec- le io tively N and . (a) ir Spins s1 with s2; (b) Reservoirs r1 with r2; (c) Spin s1 with reservoir r1; (d) Spin s1 with reservo r2. also exist, bsut the amplitude of oscillation becomes aller compared with the resonant case. From Equa- tween the spin and its reservoir. The steady values of sm tions (12)-(15), we can also find that the correlation transference between the spins and reservoirs is also complete, but the transferring time becomes longer com- pared with the corresponding resonant case. This is be- cause the non-resonant effect decreases the coupling be- N Copyright © 2013 SciRes. JQIS 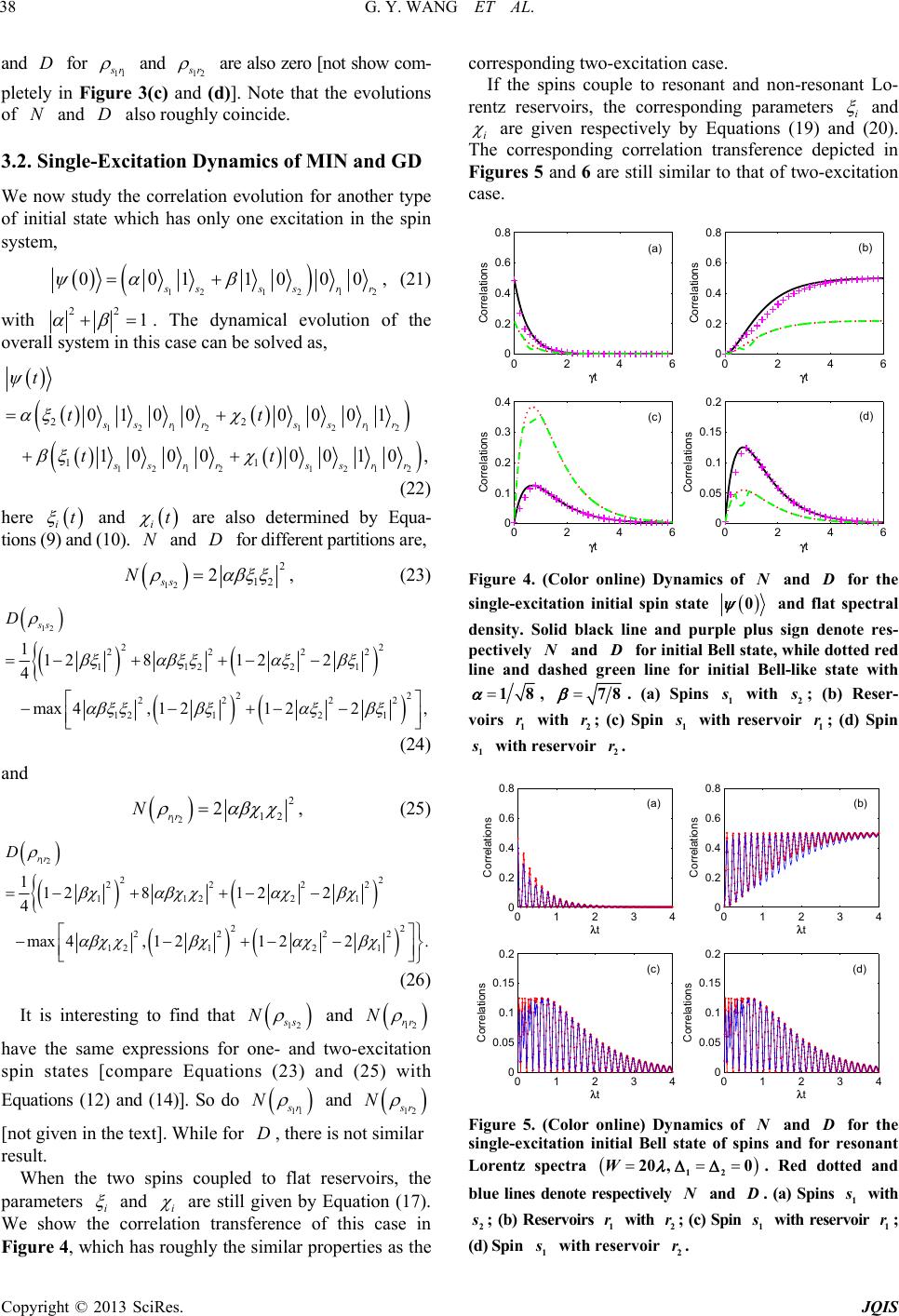 G. Y. WANG ET AL. 38 and D for 11 r and 12 r are also zero [not show com- pletely in Figure 3(c) and (d)]. Note that the evolutions of N and D also roughly coincide. 3.2. Single-Excitation Dynamics of MIN and GD Wew stud the correlation evolution noy for another type of initial state which has only one excitation in the spin system, 12121 2 0011000, sssrr (21) with 22 1 . The dynamical evolution of the overall system in this case can be solved as, 12 121212 12121212 11 01 10 000010, rr ssrrssrr tt (22 here and are also determined by Equa- 0). for different partitions 22 0100 00 ssrrss t tt ) are, it tions (9) and (1 it N and D 12 2 12 2,N (23) ss 12 2 22 112 21 2 22 22 1212 1 112812 2 4 max4, 12122, ss (24) and 2 2 22 D 12 2 12 2, rr N (25) 12 22 2222 2 11 2 21 22 22 22 1212 1 112 812 4 max4,12122 rr (26) It is interesting to find that and . D 12 ss N 12 rr N have the same expressions for one- and two-excit sp(23) 5) with ation in states [compare Equations and (2 Equations (12) and (14)]. So do 11 r N and 12 sr N [not given in the text]. While for D, there is not similar result. When the two spins coupled reserv to flatoirs, the parameters i and i are still gen by Equation (17). We sho iv w e corrlation transference of this case in Fi g th e gure 4, which has roughly the similar properties as the correspondin two-excitation case. If the spins couple to resonant and non-resonant Lo- rentz reservoirs, the corresponding parameters i and i are given respectively by Equations (19) an20). ca d ( The corresponding correlation transference depicted in Figures 5 and 6 are still similar to that of two-excitation se. 0 2 4 6 0 0.2 0.4 0.6 0.8 γt Correlations 0.8 0.6 0 2 4 6 0 0.2 0.4 γt Correlations 0 2 4 6 0 0.1 0.2 0.3 0.4 γt Correlations 0 2 4 6 0 0.05 0.1 0.15 0.2 γt Correlations (a) (b) (c) (d) Figure 4. (Color online) Dynamics of and N for the single-excitation initial spin state 0 andpectral density. Solid black line and purple plus sign denote re flat s s- pectively N and for initial Bell sta, while tted red line and dashed green line for inell-like state with te itial Bdo 18 , 78 . (a) Spins s1 with s2; (b) Reser- voirs r1 with r2; ( Spin s1 with reservoir r1; (d) Spin s1 with reservoir r2. c) 01234 0 0.2 0.4 0.6 0.8 λt Correlations 0.8 01234 0 0.2 0.4 0.6 λt Correlations 01234 0 0.05 0.1 0.15 0.2 λt 01234 0 0.05 0.1 0.15 0.2 λt Correlations (a) (b) (c) (d) Figure 5. (Color online) Dynamics of and N for the single-excitation initial Bell state of spdesonant Lorentz spectra ins an for r 12 20 ,W 0. Red and d dotte blue lines denote respectively N and . (a) Spins s1 with s2; (b) Reservoirs r1 with r2; (c) Spin s1 with reservoir r1; (d) Spin s with res 1ervoir r2. Copyright © 2013 SciRes. JQIS 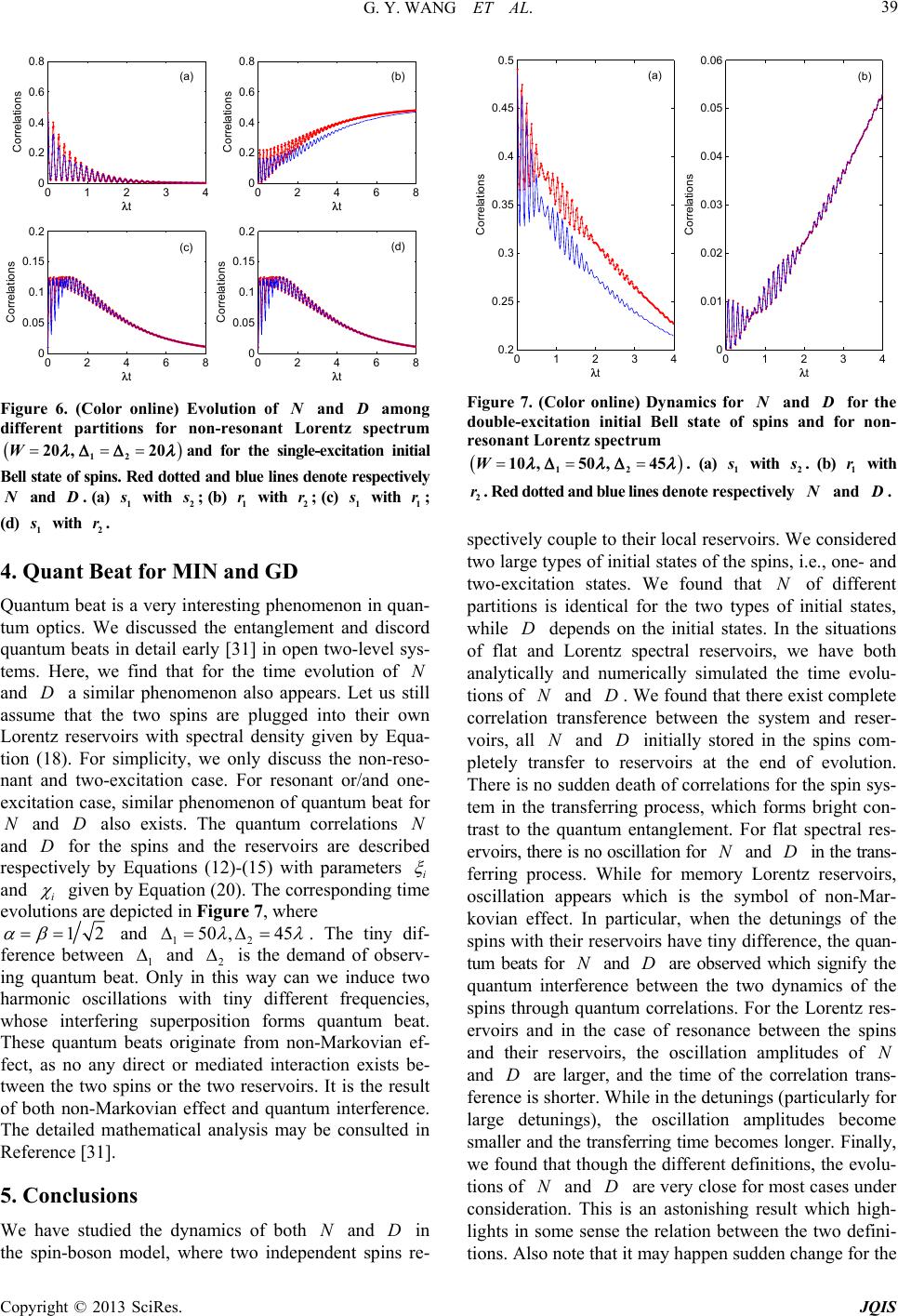 G. Y. WANG ET AL. 39 01234 0 0.2 0.4 0.6 0.8 λt Correlations 0 2 4 6 8 0 0.2 0.4 0.6 0.8 λt Correlations 02468 0 0.05 0.1 0.15 0.2 λt Correlations 0 2 4 6 8 0 0.05 0.1 0.15 0.2 λt Correlations (a) (b) (c) (d) Figure 6. (Color online) Evolution of and N among different partitions for non-resonanntectrum t Lorez sp 12 20 ,20W and for the sinle-exc initiag s d itation es l Bell state of spins. Red dotted and blue l ineenote rpec tively N and . (a) s1 with s2; (b) r1 with r2; (c) s1 with r1; 4. Quant Beat for MIN and GD Quantum beat is a very inte (d) s1 with r2. resting phenomenon in quan- tum optics. We discussed the entanglement and discord n two-level sys-quantum beats in detail early [31] in ope tems. Here, we find that for the time evolution of N and D a similar phenomenon also appears. Let us still assume that the two spins are plugged into their own Lorentz reservoirs with spectral density given by Equa- tion (18). For simplicity, we only discuss the non-reso- nant and two-excitation case. For resonant or/and one- excitation case, similar phenomenon of quantum beat for N and D also exists. The quantum correlations N and D for the spins and the reservoirs are described respectively by Equations (12)-(15) with parameters i and i given by Equation (20). The corresponding time evolutions are depicted in Figure 7, where 12 and 12 50 ,45 . The tiny dif- ference between 1 and 2 is the demand of observ- ing quantum beat. Only in this way can we induce two harmonic oscillations with tiny different frequencies, whose interfering superpoion forms quantum beat. These quantum beats originate from non-Markovian ef- fect, as no any direct or mediated interaction exists be- tween the two spins or the two reservoirs. It is the result of both non-Markovian effect and quantum interference. The detailed mathematical analysis may be consulted in Reference [31]. 5. Conclusions We have studied sit the dynamics of both and in the spin-boson model, where two independent spins re- N D 01234 0.2 0.25 0.3 0.35 0.4 0.45 0.5 λt Correlations 0.06 01234 0 0.01 0.02 0.03 0.04 0.05 λt Correlations (a) (b) Figure 7. (Color online) Dynamics for and N for the double-excitation initial Bell state of spi non- resonant Lorentz spectrum ns and for 10 ,50 ,45W 12 . (a) wits121 r2. Red dotted and blue lines denote respectively N and with s. (b) rh . al resevoirs. We cons dered o large types of initial states of the spins, i, one- d o-excitation states. We found that N of differt spectively couple to their locri tw .e. an entw partitions is identical for the two types of initial states, while D depends on the initial states. In the situations of flat and Lorentz spectral reservoirs, we have both analytically and numerically simulated the time evolu- tions of N and D. We found that there exist complete correlation transference between the system and reser- voirs, all N and D initially stored in the spins com- pletely transfer to reservoirs at the end of evolution. There is no sudden death of correlations for the spin sys- tem in the transferring process, which forms bright con- trast to the quantum entanglement. For flat spectral res- ervoirs, there is no oscillation for N and D in the trans- ferring process. While for memory Lorentz reservoirs, oscillation appears which is the symbol of non-Mar- kovian effect. In particular, when the detunings of the spins with their reservoirs have tiny difference, the quan- tum beats for N and D are observed which signify the quantum interference between the two dynamics of the spins through quantum correlations. For the Lorentz res- ervoirs and in the case of resonance between the spins and their reservoirs, the oscillation amplitudes of N and D are larger, and the time of the correlation trans- ference is shorter. While in the detunings (particularly for large detunings), the oscillation amplitudes become smaller and the transferring time becomes longer. Finally, we found that though the different definitions, the evolu- tions of N and D are very close for most cases under consideration. This is an astonishing result which high- lights in some sense the relation between the two defini- tions. Also note that it may happen sudden change for the Copyright © 2013 SciRes. JQIS  G. Y. WANG ET AL. 40 evolutionf D. Note that a similar work for the evolutions of the quan- tum and classical correlations was done [29]. The trans- ference of N and D studied here has some similar properties to at o of quantum and classical correlations. H of n d finding the dy or the Doctoral Program 20124306110003) the Cons- g Measurement-Induced Disturbance to racterizeations as Classical or Quantum,” Physica Review A01. doi:10.1103/Ph th in Correl , Vol. 7 owever it also appears many differences, for example, N is the same for one- and two-excitation cases, and D may happen sudd change in the evolutional proc- ess. Especially, N and D have very coincident evolu- tions in most cases under considerations. Quantum correlation is a kind of unique characteristics quantum system which could be a new resource in quantum information techology. Exploring the relation between various quantum correlations an en namical rules of them in practical environments can not only contribute to a better understanding of the con- cepts, but also offer possible references for applications. 6. Acknowledgements This work is supported by the National Natural Science Foundation of China (Grant Nos. 11275064, 11075050), Specialized Research Fund f Higher Education (Grant No. of truct Program of the National Key Discipline, the Pro- gram for Changjiang Scholars and Innovative Research Team in University under Grant No. IRT0964, and Hu- nan Provincial Natural Science Foundation under Grant No. 11JJ7001. REFERENCES [1] S. Luo, “UsCha- l 7, No. 2, 2008, Article ID: 0223 ysRevA.77.022301 [2] M. A. Nielsen and I. L. Chuang, “Quantum Computation and Quantum Information,” Cambridge University Press, Cambridge, 2000. [3] H. Ollivier and W. H. Zurek, “Quantum Discord: A Meas- evLett.88.017901 ure of the Quantumness of Correlations,” Physical Review Letters, Vol. 88, No. 1, 2001, Article ID: 017901. doi:10.1103/PhysR [4] A. Datta, A. Shaji and C. M. Caves, “Quantum Discord and the Power of One Qubit,” Physical Review Letters, Vol. 100, No. 5, 2008, Article ID: 050502. doi:10.1103/PhysRevLett.100.050502 [5] B. P. Lanyon, M. Barbieri, M. P. Almeida and A. G. White, “Experimental Quantum Computing without En- tanglement,” Physical Review Letters, Vol. 101, No. 20, 2008, Article ID: 200501. doi:10.1103/PhysRevLett.101.200501 [6] R. Dillenschneider, “Quantum Discord and Quantum Phase Transition in Spin Chains,” Physical Review B, Vol. 78, No. 22, 2008, Article ID: 224413. doi:10.1103/PhysRevB.78.224413 [7] M. S. Sarandy, “Classical Correlation and Quantum Dis- cord in Critical Systems,” Physical Review A, Vol. 80, No. 2, 2009, Article ID: 022108. doi:10.1103/PhysRevA.80.022108 [8] J. Cui and H. Fan, “Correlations in the Grover Search,” Journal of Physics A: Mathematical and Theoretical, Vol. 43, No. 4, 2010, Article ID: 045305. doi:10.1088/1751-8113/43/4/045305 [9] S. Luo, “Quantum Discord for Two-Qubit Systems,” Phy- sical Review A, Vol. 77, No. 4, 2008, Article ID: 042303. doi:10.1103/PhysRevA.77.042303 [10] G. Adesso and A. Datta, “Quantum versus Classical Cor- 1 relations in Gaussian States,” Physical Review Letters, Vol. 105, No. 3, 2010, Article ID: 030501. doi:10.1103/PhysRevLett.105.03050 [11] P. Giorda and M. G. A. Paris, “Gaussian Quantum Dis- cord,” Physical Review Letters, Vol. 105, No. 2, 2010, Article ID: 020503. doi:10.1103/PhysRevLett.105.020503 [12] B. Dakic, V. Vedral and C. Brukner, “Necessary and Suf- ficient Condition for Nonzero Quantum Discord,” Phy- sical Review Letters, Vol. 105, 190502. No. 19, 2010, Article ID: 190502doi:10.1103/PhysRevLett.105. [13] S. Luo and S. Fu, “Measurement-Induced Nonlocality,” Physical Review Letters, Vol. 106, No. 12, 2011, Article ID: 120401. doi:10.1103/PhysRevLett.106.120401 [14] S. Luo and S. Fu, “Geometric Measure of Quantum Dis- cord,” Physical Review A, Vol. 82, No. 3, 2010, Article ID: 034302. doi:10.1103/PhysRevA.82.034302 [15] S. Luo and S. Fu, “Evaluating the Geometric Measure of Quantum Discord,” Theoretical and Mathematical Phy- sics, Vol. 171, No. 3, 2012, pp. 870-878. doi:10.1007/s11232-012-0082-x [16] B. Bellomo, R. Lo Franco and G. Compagno, “Dynamics of Geometric and Entropic Quantifiers of Correlations in Open Quantum Systems,” Physical Revi No. 1, 2012, Article ID: 012312. ew A, Vol. 86, doi:10.1103/PhysRevA.86.012312 [17] B. Bellomo, G. L. Giorgi, F. Galve, R. Lo Franco, G. Com- pagno and R. Zambrini, “Unified View of Correlations Using the Square Norm Distance,” Vol. 85, No. 3, 2012, Article ID: 032 Physical Review A, 104. doi:10.1103/PhysRevA.85.032104 [18] K. Ann and G. Jaeger, “Finite-Time Destruction of En- tanglement and Non-Locality by Environmental Influ- ences,” Foundations of Physics, Vol. 39, N 790-828. o. 7, 2009, pp. 295-8doi:10.1007/s10701-009-9 7-8, 2011, pp. ysRevLett.93.140404 [19] B. Bellomo, G. Compagno, R. Lo Franco, A. Ridolfo and S. Savasta, “Dynamics and Extraction of Quantum Dis- cord in Multipartite Open Systems,” International Jour- nal of Quantum Information, Vol. 9, No. 1665-1676. [20] T. Yu and J. H. Eberly, “Finite-Time Disentanglement via Spontaneous Emission,” Physical Review Letters, Vol. 93, No. 14, 2004, Article ID: 140404. doi:10.1103/Ph [21] B. Bellomo, G. Compagno, A. D’Arrigo, G. Falci, R. Lo Franco and E. Paladino, “Entanglement Degradation in the Copyright © 2013 SciRes. JQIS  G. Y. WANG ET AL. Copyright © 2013 SciRes. JQIS 41 and Quantum Noise,” , Article ID: 062309. Solid State: Interplay of Adiabatic Physical R evi ew A, Vol. 81, No. 6, 2010 doi:10.1103/PhysRevA.81.062309 [22] T. Werlang, S. Souza, F. F. Fanchini and C. J. Villas Boas, “Robustness of Quantum Discord to Sudden Death,” Phy- sical Review A, Vol. 80, No. 2, 2009, Article ID: 024103. doi:10.1103/PhysRevA.80.024103 [23] B. Bellomo, R. Lo Franco and G. Compagno, “Non-Mar- kovian Effects on the Dynamics of Entanglement,” Phy- sical Review Letters, Vol. 99, No. 16, 2007, Article ID: 160502. doi:10.1103/PhysRevLett.99.160502 ol. 27, No. ID: 014019. [24] R. Lo Franco, B. Bellomo, S. Maniscalco and G. Com- pagno, “Dynamics of Quantum Correlations in Two-Qu- bit Systems within Non-Markovian Environments,” In- ternational Journal of Modern Physics B, V 1-3, 2013, Article ID: 1345053. [25] R. Lo Franco, A. D’Arrigo, G. Falci, G. Compagno and E. Paladino, “Entanglement Dynamics in Superconducting Qubits Affected by Local Bistable Impurities,” Physica Scripta, Vol. T147, 2012, Article doi:10.1088/0031-8949/2012/T147/014019 [26] B. Bellomo, G. Compagno, R. Lo Franco, A. Ridolfo and S. Savasta, “Entanglement Dynamics of Two Independent Cavity-Embedded Quantum Dots,” Physica Sc T143, 2011, Article ID: 014004. ripta, Vol. doi:10.1088/0031-8949/2011/T143/014004 [27] R. Lo Franco, B. Bellomo, E. Andersson and G. Com- pagno, “Revival of Quantum Correlations without Sys- tem-Environment Back-Action,” Physical Review A, Vol. 85, No. 3, 2012, Article ID: 032318. doi:10.1103/PhysRevA.85.032318 [28] Q. J. Tong, J. H. An, H. G. Luo and nism of Entanglement Preservation C. H. Oh, “Mecha- ,” Physical Review A, Vol. 81, No. 5, 2010, Article ID: 052330. doi:10.1103/PhysRevA.81.052330 [29] R. C. Ge, M. Gong, C. F. Li, J. S. Xu and G “Quantum Correlation and Classica . C. Guo, l Correlation Dynam- ics in the Spin-Boson Model,” Physical Review A, Vol. 81, No. 6, 2010, Article ID: 064103. doi:10.1103/PhysRevA.81.064103 [30] B. Bellomo, R. Lo Franco, S. Manis pagno, “Entanglement Trapping in calco and G. Com- Structured Environ- ments,” Physical Review A, Vol. 78, No. 6, 2008, Article ID: 060302(R). doi:10.1103/PhysRevA.78.060302 [31] H. S. Zeng, Y. P. Zheng, N. Tang and G. Y. Wang, “Cor- relation Quantum Beats Induced by Non-Markovian Ef- fect,” Quantu m Information Process, Vol. 12, No. 4, 2013, pp. 1637-1650. doi:10.1007/s11128-012-0437-0 [32] C. E. Lopez, G. Romero and J. C. Retamal, “Dynamics of Entanglement Transfer through Multipartite Dissipative Systems,” Physical Review A, Vol. 81, No. 6, 2010, Arti- cle ID: 062114. doi:10.1103/PhysRevA.81.062114
|