 Journal of Quantum Information Science, 2013, 3, 27-33 http://dx.doi.org/10.4236/jqis.2013.31007 Published Online March 2013 (http://www.scirp.org/journal/jqis) Non-Markovian D ynamics of an Open Two-Level System with Amplitude-Phase Damping Ning Tang, Guoyou Wang, Zilong Fan, Haosheng Zeng* Key Laboratory of Low-Dimensional Quantum Structures and Quantum Control of Ministry of Education, Department of Physics, Hunan Normal University, Changsha, China Email: *hszeng@hunnu.edu.cn Received February 6, 2013; revised March 10, 2013; accepted March 19, 2013 ABSTRACT By use of the measure, the backflow of information presented recently, we study the non-Markovianity of the dynamics for a two-level system interacting with a zero-temperature structured environment via amplitude-phase coupling. In the limit of weak coupling between the system and its reservoir, the time-local non-Markovian master equation for the re- duced state of the system is derived. Under the secular approximation, the exact analytic solution is obtained. Numerical simulations show that the amplitude and phase dampings can produce destructive interference to the backflow of infor- mation, leading to the weaker non-Markovianity of the compound dynamics compared with the dynamics of a single amplitude or phase damping model. We also study the characteristics of the initial-state pairs that maximize the back- flow of information. Keywords: Non-Markovianity; Amplitude-Phase Dampings; Destructive Interference 1. Introduction The evolution of open quantum systems can be divided into two basic types, i.e., Markovian and non-Markovian processes. For memoryless Markovian processes, the environment acts as a sink and the information that the system released into the environment during their inter- action no longer reflows to the system. However, this is not the case in the non-Markovian processes, where the lost information will return to the system at a later time, so that the later evolution of the system is affected by its past history, i.e., which appears memory effect. Although almost all early works are devoted to the study of Markovian processes [1], people recently found that Many relevant physical systems, such as the quan- tum optical system, quantum dot [2], superconductor sys- tem [3], quantum chemistry [4] and biological system [5] etc. can not be described simply by Markovian dynamics. Quantum non-Markovian processes can lead to distinctly different effects on decoherence and disentanglement [6, 7] of open systems compared with Markovian processes, which are important both for the enriching of the basic theory of quantum mechanics and for some practical ap- plications, such as the quantum metrology [8] and quan- tum key distribution [9]. Because of these distinct prop- erties and extensive applications, more and more atten- tions and interest have been devoted to the study of non-Markovian processes of open systems, including the measures of non-Markovianity [10-17], the positivity [18, 19], and some other dynamical properties [20-26] of non- Markovian processes. Experimentally, the simulation [27-30] of non-Markovian environment has been real- ized. The study of non-Markovian dynamics of open quan- tum systems is typically very involved and often requires some assumptions or approximations. In the previous re- searches of non-Markovian dynamics, only a single cou- pling way (amplitude-damping or dephase interaction) is assumed. Further, the rotating wave approximation, that is, neglecting the counter-rotating terms in the system-re- servoir interaction Hamiltonian, is employed. These as- sumptions and approximations limit the serviceable range of the model. In this paper, we consider a complex model which simultaneously consists of amplitude and phase dampings to the environment. We also reserve the counterrotating terms in the system-reservoir interaction Hamiltonian. Our motivation is to compare the non- Markovian features of the dynamics for amplitude and phase dampings, and to observe the interference be- tween the two non-Markovian dynamics induced by the two damping ways. The article is organized as follows. In Section 2, we introduce the microscopic Hamiltonian model and derive the non-Markovian time-local master equation for a two- level system weakly coupled to a vacuum reservoir. In *Corresponding author. C opyright © 2013 SciRes. JQIS  N. TANG ET AL. 28 Section 3, we solve the master equation under the so- called secular approximation and present the analytic expression for the calculation of non-Markovianity based on the measure proposed by Breuer, Laine and Piilo (BLP) [10] recently. In Section 4, we choose the Lor- entzian spectra reservoir as an exemplary example and simulate numerically the non-Markovianity of the system dynamics. The non-Markovian effects produced by am- plitude noise, phase noise and their combination are in- vestigated. And finally the conclusion is arranged in Sec- tion 5. 2. Microscopic Model Consider a two-level atom with Bohr frequency 0 in- teracting with a zero-temperature bosonic reservoir mod- eled by an infinite chain of quantum harmonic oscillators. The total Hamiltonian for this system in the Schrodinger picture is given by. 0 1 2 . kk kkzkk kk kkk k bbgb b gbb (1) where and are the Pauli and inversion opera- tors of the atom, , kk b and k b are respectively the frequency, annihilation and creation operators for the k-th harmonic oscillator of the reservoir. The atom cou- ples to its environment via both amplitude and phase interactions, k is the coupling strength which is as- sumed to be real for simplicity. The parameters and describe the relative strengthes of the two couplings which satisfy . Note that we include the counter-rotating terms, and 22 1 bk k b , in the interac- tion Hamiltonian. The time-convolutionless (TCL) projection operator technique [1] is most effective in dealing with the dy- namics of open quantum systems. In the limit of weak coupling between the system and its environment, by expanding the TCL generator to the second order with respect to coupling strength, the non-Markovian master equation describing the evolution of the reduced system, in the interaction picture, can be written as d,. dLS tiHtt Dt Dt t (2) where 2, LS Ht StSt (3) is the Lamb shift Hamiltonian which describes a small shift in the energy of the eigenstates of the two-level atom. In many theoretical researches [20], this term was neglected usually. But in this paper, we will take it into the consideration. The parameters and SS which corresponds to respectively the Lamb shifts of levels 0 and 1 may be written as 0 0dd sin, t St J (4) with 1, 1 and 2 kk k Jg the spectral distribution of the environment. The dissipator Dt that describes the secular motion of the system has the form 2 2 2 0 1, 2 1, 2 , zz Dtt tt tt t tt t (5) where the first term describes the dissipation of the atom to its environment with time-dependent decay rate t , and the second term denotes the heating with a rate t . The last term describes the purely dephasing with a rate 0t. These time-dependent rates can be written as, 0 0 2d dcos, t tJ (6) with 1,1, 0 . The dissipator Dt represents the contribution of the so-called nonsecular terms, that is, terms oscillat- ing rapidly with Bohr frequency 0 , 2 .., z z z z z z Dt ut ivtt tit t tit t ti tt ti tt ttitit t ttitit th c (7) here h.c. denotes the Hermitian conjugation, and the time-dependent coefficients are defined as 0 0 2d dcoscos, t utJ tt (8) 0 0 2d dcossin, t vtJ tt (9) 0 0dd cos, t tJt (10) 0 0dd cos, t tJ t (11) Copyright © 2013 SciRes. JQIS  N. TANG ET AL. 29 0 0dd sin, t tJt t (12) 0 0dd sin t tJ . (13) 3. Solution and Non-Markovian Measure We now consider the case where the nonsecular term Dt can be neglected. Just as pointed out by Maniscalco [31], this kind of secular approximation that used after tracing over the bath degrees of freedom is different from the rotating wave approximation before the tracing. It is a more precise approximation that con- sists in an average over rapidly oscillating terms, but does not wash out the effect of the counter-rotating terms present in the coupling Hamiltonian. Under the secular approximation, the master Equation (2) has the Lindblad- like form with time-dependant decay rates. It is straight- forward and easy to show that the corresponding Bloch equation may be written as 22 22 0 14 2, x bb y SSb(14) 22 22 0 14, 2 yx bbS Sb(15) 22 , zz bb (16) where the three components of the Bloch vector are defined as = j bt trt with and ,,jxyz the Pauli operators. For compactness we omit the argument of all the time-dependent coefficients. Employing the method proposed by Hall [32], the corresponding Bloch Equations (14)-(16) can be solved exactly which gives e0cos 0sin t xx y btbt bt , , , (17) e0sin 0cos t yx y btbt bt (18) 2 0 e0ede t tts zz btbss s (19) with 22 2 0 0 1d4, 2 t tss ss (20) 2 0d, t ts ss (21) and 2 0d. t tsSsSs (22) In this paper, we employ the (BLP) measure [10] to describe the non-Markovianity of the considered system. Note that Markovian processes always tend to continu- ously reduce the trace distance between any two states of a quantum system, thus an increase of the trace distance during any time interval implies the emergence of non- Markovianity. BLP further linked the change of the trace distance to the flow of information between the system and its environment, and concluded that the backflow of information from environment to the system is the key feature of a non-Markovian dynamics. In quantum in- formation science, the trace distance for quantum states 1 and 2 is defined as [33] 121 2 1 , 2 Dtr, (23) with trace norm defined as AA . For a given pair of initial states 1,2 0 of the system, the change of the dynamical trace-distance can be described by its time derivative 1, 212 d ,0 , d tDt t ,t (24) where 1,2 t are the dynamical states of the system with the initial states 1,2 0 . For Markovian processes, the monotonically reduction of the trace distance implies 1,2 ,0t 0 for any initial states 1,2 0 and at any time . If there exists a pair of initial states of the system such that for some evolutional timet, t 1,2 ,0t 0 , then the information takes backflow from environment to the system, and the process is non- Markovian. In order to describe the degrees of non-Mark- ovianity of the whole dynamical process, the quantity, 1,2 1,2 0 0 maxd,0,tt (25) is introduced. Where the time integration is extended over all intervals in which is positive, and the ma- ximum is taken over all initial-state pairs of the system. For any Markovian process, . The larger the quantity is, the higher the non-Markovianity of the process is. 0 For our considered open system and by use of the so- lution of Equations (17)-(22), Equations (24) becomes 222 2 0 2 22 22 1e4 4 2e , t t xy z Gt bb b (26) where 12 2 22 22 ee tt xy z Gtbbb and 12 0 jj j bb b 0 is the difference between the two Bloch components that correspond to the initial states 1,2 0 Copyright © 2013 SciRes. JQIS 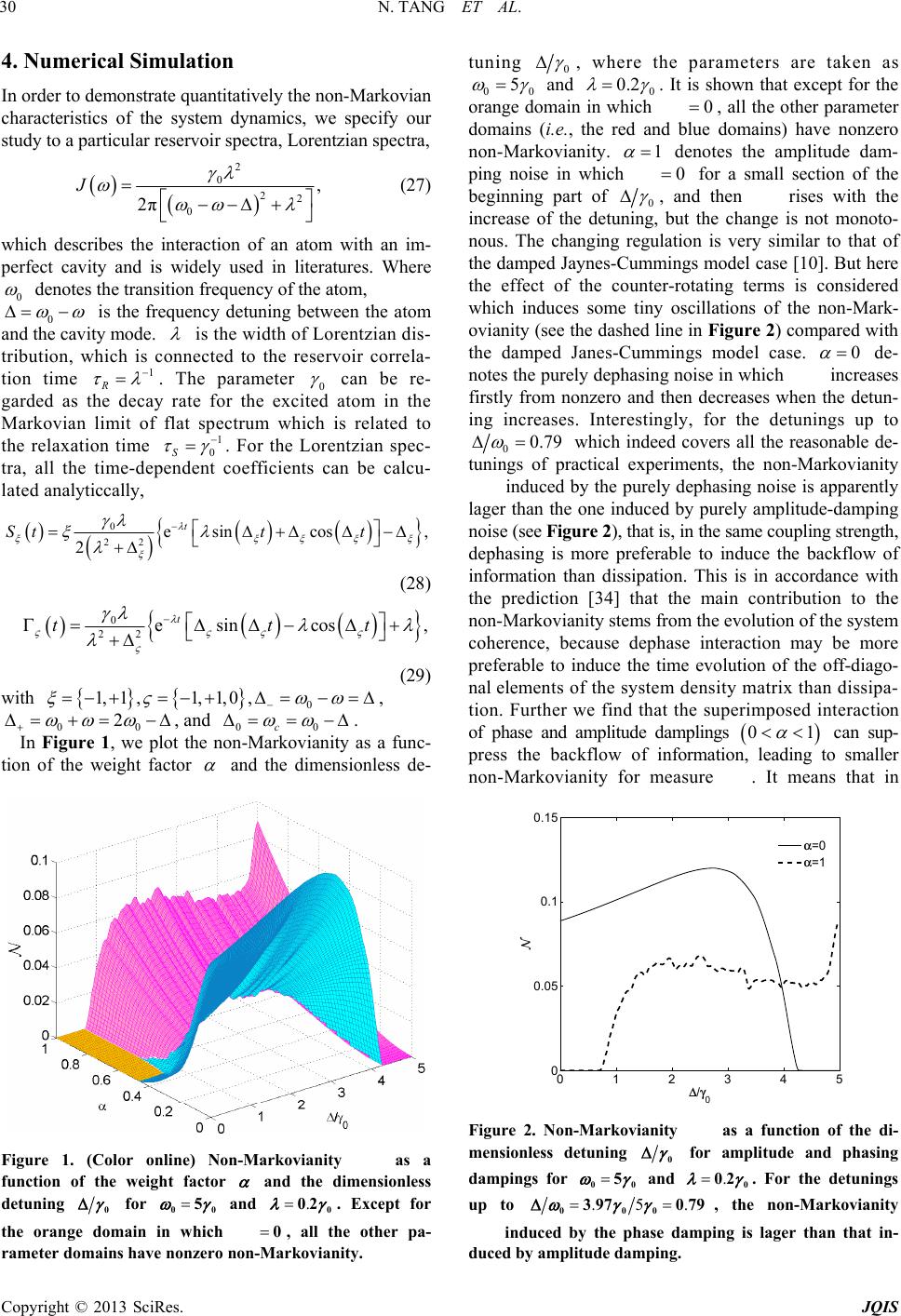 N. TANG ET AL. 30 4. Numerical Simulation In order to demonstrate quantitatively the non-Markovian characteristics of the system dynamics, we specify our study to a particular reservoir spectra, Lorentzian spectra, 2 0 22 0 , 2π J (27) which describes the interaction of an atom with an im- perfect cavity and is widely used in literatures. Where 0 denotes the transition frequency of the atom, 0 is the frequency detuning between the atom and the cavity mode. is the width of Lorentzian dis- tribution, which is connected to the reservoir correla- tion time 1 R . The parameter 0 can be re- garded as the decay rate for the excited atom in the Markovian limit of flat spectrum which is related to the relaxation time 1 0 S . For the Lorentzian spec- tra, all the time-dependent coefficients can be calcu- lated analyticcally, 0 22 esin cos 2 t Stt t , (28) 0 22 esin cos, t tt t (29) with , 0 1,1,1,1, 0, 2 00 00c , and . In Figure 1, we plot the non-Markovianity as a func- tion of the weight factor and the dimensionless de- Figure 1. (Color online) Non-Markovianity as a function of the weight factor and the dimensionless detuning 0 for 0 50 and . 0 02 0 . Except for the orange domain in which , all the other pa- rameter domains have nonzero non-Markovianity. tuning 0 , where the parameters are taken as 00 5 and 0 0.2 . It is shown that except for the orange domain in which , all the other parameter domains (i.e., the red and blue domains) have nonzero non-Markovianity. 0 1 denotes the amplitude dam- ping noise in which 0 for a small section of the beginning part of 0 , and then rises with the increase of the detuning, but the change is not monoto- nous. The changing regulation is very similar to that of the damped Jaynes-Cummings model case [10]. But here the effect of the counter-rotating terms is considered which induces some tiny oscillations of the non-Mark- ovianity (see the dashed line in Figure 2) compared with the damped Janes-Cummings model case. 0 de- notes the purely dephasing noise in which increases firstly from nonzero and then decreases when the detun- ing increases. Interestingly, for the detunings up to 00.79 which indeed covers all the reasonable de- tunings of practical experiments, the non-Markovianity induced by the purely dephasing noise is apparently lager than the one induced by purely amplitude-damping noise (see Figure 2), that is, in the same coupling strength, dephasing is more preferable to induce the backflow of information than dissipation. This is in accordance with the prediction [34] that the main contribution to the non-Markovianity stems from the evolution of the system coherence, because dephase interaction may be more preferable to induce the time evolution of the off-diago- nal elements of the system density matrix than dissipa- tion. Further we find that the superimposed interaction of phase and amplitude damplings can sup- press the backflow of information, leading to smaller non-Markovianity for measure . It means that in 10 0 1 2 3 4 0 0.05 0.1 0.15 Δ/γ0 N α=0 α=1 Figure 2. Non-Markovianity as a function of the di- mensionless detuning 0 for amplitude and phasing dampings for 0 50 and . 0 02 . For the detunings up to .5. 00 397 0 079 , the non-Markovianity induced by the phase damping is lager than that in- duced by amplitude damping. Copyright © 2013 SciRes. JQIS  N. TANG ET AL. 31 practice we may, not only by the engineering of envi- ronmental structure but also by the adjusting of the cou- pling ways between the system and its environment, ma- nipulate the non-Markovian dynamics of open quantum systems. In Figure 3, we plot the measure as a function of the weight factor and the dimensionless decay rate 0 , where the parameters is chosen as 025 and 5 . We find that for a given weight factor , the measure increases monotonously with decay rate 0 . This may be understood easily: The parameter is the inverse of the reservoir correlation time. The de- crease of indicates an increase of the reservoir cor- relation time. The parameter 0 is the inverse of the system relaxation time. Decreasing the system relaxation time is equivalent to increasing the reservoir correlation time. Both the two changes can enhance the non-Mark- ovianity of the system dynamics [35]. Note that for 1 , the result is similar to the one of damped Jaynes-cummings model with resonant interaction [36]. Further we also find that the dephase noise 0 can give rise to much more non-Markovianity than ampli- tude-damping noise , and their superposition can reduce the value of the measure . These results once again demonstrate the dominating rules of the quantum coherence to the non-Marko- vianity of open quantum system and the effect of de- structive interference between amplitude and phase dampings. 1 0 1 We also investigate the problem of what initial state pairs can maximize the measure . Interestingly, in our numerical simulations, we find that there exist only two types of such initial state pairs. One pair is 0 and 1 which in Figures 1 and 3 applies to the red parame- Figure 3. (Color online) Non-Markovianity as a func- tion of the weight factor and the dimensionless decay rate 0 for 025 and 5 . ter domain, the other pair is 101 2 and 101 2 which applies to the blue parameter domain. Both the initial state pairs have the largest trace distance. For the orange parameter domain in Figure 1, the measure 0 . Note that the initial state pair 0,1 agree with the result [10] for the damped Jaynes-Cummings model, though here we have con- sidered the effect of counter-rotating terms in the interac- tion Hamiltonian. However, the initial state pair , does not coincide with the result ,0 of reference [36]. The former is composed by two or- thogonal states with maximal coherence. While the later contains the ground state and a maximal coherence state which have no the largest trace distance. 5. Conclusions In conclusion, we have studied the non-Markovianity of the dynamics for a two-level system interacting with a zero-temperature structured environment via amplitude- phase damping. In the limit of weak coupling between the system and the reservoir, we have derived the time- local non-Markovian master equation for the reduced state of the system. The exact analytic solution under the secular approximation has been gained and the non- Markovian properties for the system dynamics based on the BLP measure have been studied. We found, in the same coupling strength between the system and its envi- ronment, that the dephasing interaction is more beneficial to induce the backflow of information from the environ- ment to the system compared with amplitude-damping mo- del, leading to more stronger non-Markovianity for the system dynamics. This result further proves the previous viewpoint that the main contribution to the non-Marko- vianity stems from the evolution of the system coherence. We also found that the information backflows induced by amplitude and phase damplings can take destructive in- terference so as to suppress the non-Markovianity of the system dynamics. For a given system-reservoir coupling model (a fixed ), the non-Markovian measure increases monotonously with 0 , but it is not monotonous with dimensionless detuning 0 . The physical explanation for the former case is clear, but for the later case the reason is not clear yet and requires further studying. In the definition of BLP non-Markovianity measure, a maximization over all possible initial state pairs is in- volved. This maximization process usually requires to spend much time or effort on the practical calculations. In our numerical simulations, we found that there are only two pairs of such initial states. One pair was found previously but another pair is new. For various different Copyright © 2013 SciRes. JQIS  N. TANG ET AL. 32 kinds of dynamical models, looking for the characteris- tics of such initial state pairs is perhaps a problem worthy of consideration [37,38]. The measure of non-Markovianity is a fundamental problem in the study of open quantum system dynamics. In the numerical simulations, we have only considered the Lorentzian environment. Actually, our analytic re- sults also adapt to other structured environments, such as the Ohmic reservoir, the photonic band-gap material [39], etc. By properly engineering the structure of the envi- ronment, one can selectively alter the non-Markovian dy- namical property of the open quantum system, so as to effectively control the evolution of some interesting phy- sical quantities, such as the quantum coherence, quan tum entanglement and discord. Therefore, our work will also be helpful for the researches of the related problems. 6. Acknowledgements This work is supported by the National Natural Science Foundation of China (Grant Nos. 11275064, 11075050), Specialized Research Fund for the Doctoral Program of Higher Education (Grant No. 20124306110003) the Cons- truct Program of the National Key Discipline, the Pro- gram for Changjiang Scholars and Innovative Research Team in University under Grant No. IRT0964, and Hu- nan Provincial Natural Science Foundation under Grant No. 11JJ7001. REFERENCES [1] H. P. Breuer and F. Petruccione, “The Theory of Open Quantum Systems,” Oxford University Press, Oxford, 2007. doi:10.1093/acprof:oso/9780199213900.001.0001 [2] Y. Kubota and K. Nobusada, “Applicability of Site-Basis Time-Evolution Equation for Thermalization of Exciton States in a Quantum Dot Array,” Journal of the Physical Societ y o f Ja p an , Vol. 78, No. 11, 2009, Article ID: 114603. doi:10.1143/JPSJ.78.114603 [3] Y. H. Ji and J. J. Hu, “Entanglement and Decoherence of Coupled Superconductor Qubits in a Non-Markovian En- vironments,” Chinese Physic s B, Vol. 19, No. 6, 2010, Ar- ticle ID: 060304. doi:10.1088/1674-1056/19/6/060304 [4] J. Shao, “Decoupling Quantum Dissipation Interaction via Stochastic Fields,” Journal of Chemical Physics, Vol. 120, No. 11, 2004, p. 5053. doi:10.1063/1.1647528 [5] A. W. Chin, A. Datta, F. Caruso, S. F. Huelga and M. B. Plenio, “Noise-Assisted Energy Transfer in Quantum Net- works and Light-Harvesting Complexes,” New Journal of Physics, Vol. 12, No. 6, 2010, Article ID: 065002. doi:10.1088/1367-2630/12/6/065002 [6] A. G. Dijkstra and Y. Tanimura, “Non-Markovian Entan- glement Dynamics in the Presence of System-Bath Co- herence,” Physical Review Letters, Vol. 104, No. 25, 2010, Article ID: 250401. doi:10.1103/PhysRevLett.104.250401 [7] B. Bellomo, R. Lo Franco and G. Compagno, “Non-Mar- kovian Effects on the Dynamics of Entanglement,” Phy- sical Review Letters, Vol. 99, No. 16, 2007, Article ID: 160502. doi:10.1103/PhysRevLett.99.160502 [8] A. W. Chin, S. F. Huelga and M. B. Plenio, “Quantum Me- trology in Non-Markovian Environments,” Physical Re- view Letters, Vol. 109, No. 23, 2012, Article ID: 233601. doi:10.1103/PhysRevLett.109.233601 [9] R. Vasile, S. Olivares, M. G. A. Paris and S. Maniscalco, “Continuous-Variable Quantum Key Distribution in Non- Markovian Channels,” Physical Review A, Vol. 83, No. 4, 2011, Article ID: 042321. doi:10.1103/PhysRevA.83.042321 [10] H. P. Breuer, E. M. Laine and J. Piilo, “Measure for the Degree of Non-Markovian Behavior of Quantum Pro- cesses in Open Systems,” Physical Review Letters, Vol. 103, No. 21, 2009, Article ID: 210401. doi:10.1103/PhysRevLett.103.210401 [11] A. Rivas, S. F. Huelga and M. B. Plenio, “Entanglement and Non-Markovianity of Quantum Evolutions,” Physical Review Letters, Vol. 105, No. 5, 2010, Article ID: 050403. doi:10.1103/PhysRevLett.105.050403 [12] M. M. Wolf, J. Eisert, T. S. Cubitt and J. I. Cirac, “As- sessing Non-Markovian Quantum Dynamics,” Physical Review Letters, Vol. 101, No. 15, 2008, Article ID: 150402. doi:10.1103/PhysRevLett.101.150402 [13] A. R. Usha Devi, A. K. Rajagopal and Sudha, “Open- System Quantum Dynamics with Correlated Initial States, Not Completely Positive Maps, and Non-Markovianity,” Physical Review A, Vol. 83, No. 2, 2011, Article ID: 022109. doi:10.1103/PhysRevA.83.022109 [14] X. M. Lu, X. G. Wang and C. P. Sun, “Quantum Fisher Information Flow and Non-Markovian Processes of Open Systems,” Physical Review A, Vol. 82, No. 4, 2010, Ar- ticle ID: 042103. doi:10.1103/PhysRevA.82.042103 [15] S. Luo, S. Fu and H. Song, “Quantifying Non-Marko- vianity via Correlations,” Physical Review A, Vol. 86, No. 4, 2012, Article ID: 044101. doi:10.1103/PhysRevA.86.044101 [16] D. Chruscinski, A. Kossakowski and A. Rivas, “Measures of Non-Markovianity: Divisibility versus Backflow of Information,” Physical Review A, Vol. 83, No. 5, 2011, Ar- ticle ID: 052128. doi:10.1103/PhysRevA.83.052128 [17] H. S. Zeng, N. Tang, Y. P. Zheng and G. Y. Wang, “Equi- valence of the Measures of Non-Markovianity for Open Two-Level,” Physical Review A, Vol. 84, No. 3, 2011, Article ID: 032118. doi:10.1103/PhysRevA.84.032118 [18] A. Shabani and D. A. Lidar, “Vanishing Quantum Discord is Necessary and Sufficient for Completely Positive Maps,” Physical Review Lette rs, Vol. 102, No. 10, 2009, Article ID: 100402. doi:10.1103/PhysRevLett.102.100402 [19] H. P. Breuer and B. Vacchini, “Structure of Completely Positive Quantum Master Equations with Memory Ker- nel,” Physical Review E, Vol. 79, No. 4, 2009, Article ID: 041147. doi:10.1103/PhysRevE.79.041147 [20] P. Haikka and S. Maniscalco, “Non-Markovian Dynamics of a Damped Driven Two-State System,” Physical Review A, Vol. 81, No. 5, 2010, Article ID: 052103. doi:10.1103/PhysRevA.81.052103 Copyright © 2013 SciRes. JQIS  N. TANG ET AL. Copyright © 2013 SciRes. JQIS 33 [21] K. W. Chang and C. K. Law, “Non-Markovian Master Equa- tion for a Damped Oscillator with Time-Varying Parame- ters,” Physical Review A, Vol. 81, No. 5, 2010, Article ID: 052105. doi:10.1103/PhysRevA.81.052105 [22] W. J. Gu and G. Y. Li, “Non-Markovian Behavior for Spon- taneous Decay of a V-Type Three-Level Atom with Quan- tum Interference,” Physical Review A, Vol. 85, No. 1, 2012, Article ID: 014101. doi:10.1103/PhysRevA.85.014101 [23] D. Chruscinski, A. Kossakowski and S. Pascazio, “Long- Time Memory in Non-Markovian Evolutions,” Physical Review A, Vol. 81, No. 3, 2010, Article ID: 032101. doi:10.1103/PhysRevA.81.032101 [24] P. Haikka, J. D. Cresser and S. Maniscalco, “Comparing Different Non-Markovianity Measures in a Driven Qubit System,” Physical Review A, Vol. 83, No. 1, 2011, Article ID: 012112. doi:10.1103/PhysRevA.83.012112 [25] X. Yin, J. Ma, X. Wang and F. Nori, “Spin Squeezing under Non-Markovian Channels by the Hierarchy Equa- tion Method,” Physical Review A, Vol. 86, No. 1, 2012, Article ID: 012308. doi:10.1103/PhysRevA.86.012308 [26] J. Li, G. McKeown, F. L. Semiao and M. Paternostro, “Non- Markovian Effects on the Nonlocality of a Qubit-Oscil- lator System,” Physical Review A, Vol. 85, No. 2, 2012, Article ID: 022116. doi:10.1103/PhysRevA.85.022116 [27] J. S. Xu, C. F. Li, M. Gong, X. B. Zou, C. H. Shi, G. Chen and G. C. Guo, “Experimental Demonstration of Photonic Entanglement Collapse and Revival,” Physical Review Letters, Vol. 104, No. 10, 2010, Article ID: 100502. doi:10.1103/PhysRevLett.104.100502 [28] J. S. Xu, C. F. Li, C. J. Zhang, X. Y. Xu, Y. S. Zhang and G. C. Guo, “Experimental Investigation of the Non-Mar- kovian Dynamics of Classical and Quantum Correlations,” Physical Review A, Vol. 82, No. 4, 2010, Article ID: 042328. doi:10.1103/PhysRevA.82.042328 [29] B. H. Liu, L. Li, Y. F. Huang, C. F. Li, G. C. Guo, E. M. Laine, H. P. Breuer and J. Piilo, “Experimental Control of the Transition from Markovian to Non-Markovian Dyna- mics of Open Quantum Systems,” Nature Physics, Vol. 7, No. 12, 2011, pp. 931-934. doi:10.1038/nphys2085 [30] J. S. Tang, C. F. Li, Y. L. Li, X. B. Zou, G. C. Gou, H. P. Breuer, E. M. Laine and J. Piilo, “Measuring Non-Mar- kovianity of Processes with Controllable System-Envi- ronment Interaction,” Europhysics Letters, Vol. 97, No. 1, 2012, Article ID: 10002. doi:10.1209/0295-5075/97/10002 [31] S. Maniscalco, J. Piilo, F. Intravaia, F. Petruccione and A. Messina, “Lindblad- and Non-Lindblad-Type Dynamics of a Quantum Brownian Particle,” Physical Review A, Vol. 70, No. 3, 2004, Article ID: 032113. doi:10.1103/PhysRevA.70.032113 [32] M. J. W. Hall, “Complete Positivity for Time-Dependent Qubit Master Equations,” Journal of Physics A: Mathe- mat ical and The oretical, Vol. 41, No. 20, 2008, Article ID: 205302. doi:10.1088/1751-8113/41/20/205302 [33] M. A. Nielsen and I. L. Chuang, “Quantum Computation and Quantum Information,” Cambridge University Press, Cambridge, 2000. [34] G. Clos and H. P. Breuer, “Quantification of Memory Effects in the Spin-Boson Model,” Physical Review A, Vol. 86, No. 1, 2012, Article ID: 012115. doi:10.1103/PhysRevA.86.012115 [35] J.-G. Li, J. Zou and B. Shao, “Non-Markovianity of the Damped Jaynes-Cummings Model with Detuning,” Phy- sical Review A, Vol. 81, No. 6, 2010, Article ID: 062124. doi:10.1103/PhysRevA.81.062124 [36] E. M. Laine, J. Piilo and H. P. Breuer, “Measure for the Non-Markovianity of Quantum Processes,” Physical Re- view A, Vol. 81, No. 6, 2010, Article ID: 062115. doi:10.1103/PhysRevA.81.062115 [37] Z. Y. Xu, W. L. Yang and M. Feng, “Proposed Method for Direct Measurement of the Non-Markovian Character of the Qubits Coupled to Bosonic Reservoirs,” Physical Review A, Vol. 81, No. 4, 2010, Article ID: 044105. doi:10.1103/PhysRevA.81.044105 [38] Z. He, J. Zou, L. Li and B. Shao, “Effective Method of Calculating the Non-Markovianity N for Single-Channel Open Systems,” Physical Review A, Vol. 83, No. 1, 2011, Article ID: 012108. doi:10.1103/PhysRevA.83.012108 [39] M. Woldeyohannes and S. John, “Coherent Control of Spontaneous Emission near a Photonic Band Edge,” Jour- nal of Optics B: Quantum and Semiclassical Optics, Vol. 5, No. 2, 2003, p. 43. doi:10.1088/1464-4266/5/2/201
|