Engineering, 2010, 2, 720-726
doi:10.4236/eng.2010.29093 Published Online September 2010 (http://www.SciRP.org/journal/eng)
Finite Element Analysis of Contact Pressures between Seat
Cushion and Human Buttock-Thigh Tissue
Chak Yin Tang, Wai Chan, Chi Pont Tsui
1Department of Industrial and Systems Engineering, Hong Kong Polytechnic University,
Hong Kong, China
Received May 19, 2010; revised July 21, 2010; accepted August 6, 2010
Abstract
Unrelieved pressure on load-bearing muscle tissues of humans can produce pressure ulcers. In a seated up-
right posture, the highest pressures occur inferior to the ischial tuberosities (ITs). Moreover, the vibration can
initiate the development of pressure ulcer. Therefore, the seat cushion is not only used to lower the maximum
seating pressure on buttocks but also minimize the transmission of vibration to human body. The purpose of
this study was to investigate the effects of varying vertical vibration frequencies on seat-interface contact
pressure during sitting on three different seat cushions by using a finite element modeling approach. A sim-
plified two-dimensional human buttock-thigh model was developed to simulate the mechanical response of
the muscle of buttocks and thigh under vertical vibration. Static and vibrational loads with five different fre-
quencies of 0.1, 1, 10, 30 and 50 Hz and the same amplitude of 3 mm were applied to different seat cushions.
The result showed that the “SAF 6060” seat cushion with both hyperelastic and viscoelastic behaviors could
be effective in reducing the amplitude of varying maximum contact pressure, especially for the frequency of
10-20 Hz. This method could help in design of seat cushions with appropriate material properties and shape
so as to reduce vibrations transmitted to human body at a certain frequency range.
Keywords: Finite Element Analysis, Seat Cushion, Vibration, Buttock-Thigh
1. Introduction
Static seating comfort is important for drivers and wheel-
chair users. Seat-interface pressure distribution has been
used as an objective measure for discomfort prediction
[1]. Experimental methods could find the interface pres-
sure between human body and seat. However, it could
not provide the information about subcutaneous stress
and deformations of soft tissues. For drivers and wheel-
chair users who have sat for a long time, they could be
associated with an increased risk of pressure ulcers [2].
Moreover, severe pressure ulcer initiates in muscle tissue
overlying a bony prominence (ischial tuberosity) and
progresses outwards through fat and skin, giving rise to
the subcutaneous stress [3,4]. Many researchers con-
ducted two-dimensional (2D) and three-dimensional (3D)
finite element analyses to investigate the subcutaneous
stress [1,5-12]. Ragan et al. determined the effects of the
thickness of polymer foam wheelchair cushions on sub-
cutaneous pressures during seating by using a finite ele-
ment approach [8]. It was found that seat-interface pres-
sures were a good indicator for reducing the subcutane-
ous stress.
Shocks normal to the seat cushions and shear stresses
could initiate the development of pressure ulcer [13,14].
Following the directive 2002/44/EC of the European
Parliament, manufacturers of seat cushions were required
to limit vibration exposure to users. Wu et al., [15] found
that the maximum ischium pressure and the effective
contact area on a soft seat occur near resonant frequency
of the coupled human-seated system (2.5-3.0 Hz), and
generally increased considerably with increase in the
magnitude of vibration excitation. DiGiovine et al., [16]
discussed that the application of the appropriate seating
system may reduce the amount of whole-body vibration
experienced by an individual during manual wheelchair
propulsion, They also suggested the manufacturers should
concentrate on designing seat cushions that shift the
resonant frequency away from the range of frequencies
most sensitive to humans during whole-body vibration
(4-12.5 Hz) as defined in ISO 2631-1:1997 [17].
Owing to these reasons, many researchers developed a

C. Y. TANG ET AL.
721
biomechanical model of human body for investigating
the dynamic response to vertical vibrations. Verver et al.,
[18] developed a finite element model for the human
body using MADYMO while Siefert et al. [9] used
CASIMIR for model creation. However, for their models,
only hyperelastic behavior was used to describe the seat
cushion and human soft tissue but their viscoelastic
properties were not considered. Kitazaki and Griffin [19]
used beam, spring and mass elements to model a 2D hu-
man model including the spine, viscera, head, pelvis and
buttocks tissue. This model was good for estimating the
transmission capability from vertical seat motion to ver-
tical spinal motion. Other methods such as lumped pa-
rameter model [20,21] and finite segment model [22]
were also used to evaluate the whole-body vibration .
However, these two methods could not present realistic
human geometry. Other researchers experimentally de-
termined if seat cushions of a selected wheelchair could
minimize the transmission of vibrations to users [16,23,
24].
The main goal of our study was to investigate the ef-
fects of vertical vibration on subcutaneous stress of but-
tocks sitting on three different seat cushions by using a
finite element modeling approach. The simplified but-
tock-thigh model was developed for the analysis. The
hyperelastic and viscoelastic properties were used to de-
scribe the mechanical behaviors of the human soft tissue
and seat cushion.
2. Methodology
2.1. Buttock-Thigh Model
Figure 1 shows the buttock-thigh model consisting of a
femur and ischial tuberosity (IT) for representing humans
in a sitting posture. It was assumed that a human sit up-
rightly on the cushion and there was no support on the
feet. The total length of the buttock-thigh model was 60
cm for the male at the fifty-percentile [25]. A circle with
a radius of 1cm represents the cross section of the IT
where supports the body’s weight in a sitting position.
The distance between the bottom of the IT and the skin
was 4 cm [8]. The thickness of the cushion was set to 8
cm because Chow and Odell [26] found that the cushion
thickness beyond 8cm was ineffective in further reducing
subcutaneous stress. The distance between the buttock-
thigh model and the cushion was set to 1 cm at the be-
ginning step because the buttocks were assumed not to
sit on the cushion initially. With the finite element tool –
ABAQUS, the buttock-thigh model and seat cushion
were meshed using 2D plane strain elements as shown in
Figure 2.
Figure 1. The buttock-thigh model and the cushion.
Figure 2. Finite element model of the buttock-thigh model
and the cushion.
2.2. Material Models
The muscle and seat cushions were assigned different
material properties. All bones in the model were defined
as rigid bodies, because their stiffness is very high as
compared to the muscle and cushion.
2.2.1. Seat Cushions
Three different material properties of seat cushions were
considered in this study. One of the seat cushions was
assumed to be made of SAF 6060 polymer foam with
rate-independent hyperelastic and viscoelastic behaviors,
which was produced by Foam Partner Fritz Nauer AG.
Another two seat cushions were similar to the above-
mentioned SAF6060 cushion but with a linear elastic
response instead of the hyperelastic behavior. The Young
moduli of these two cushions were separately set to 15
kPa (Elastic 15000) and 20 kPa (Elastic 20000), while
their Poisson’s ratio was 0.3 [27].
The hyperealstic behavior of the seat cushion SAF6060
was represented using a non-linear isotropic compressi-
ble hyperelastic soft foam material model or a so-called
hyperfoam. The hyperelastic behavior of the foam mate-
rial is described by the strain energy potential, U in the
following form:



N
123
2 1
21
ˆˆˆ
31
ii
iii el
i
i
i i
UJ


 










 (1)
where N is the order of the strain energy potential, i

,
i

and i

are the temperature-dependent material pa-
rameters; 1
3 ˆ()
th
ii
J



, 123
ˆˆˆ el
J
 and i

are the prin-
cipal stretches. el Jis the ratio of elastic-deformation to

C. Y. TANG ET AL.
722
volume-change and th
J
is the ratio of the thermal-strain
to volume-change. The coefficients i

are related to the
initial shear modulus while the coefficient i

deter-
mines the degree of compressibility and is related to the
Poisson’s ratio [28]. In the present work, polyurethane
soft foam parameters determined by Schrodt et al. [29]
from uniaxial compression tests were used to define the
above-mentioned material parameters for SAF 6060
cushion as listed in Table 1. Moreover, its density was
set to 60 kg/m3 and a second order (N = 2) of the strain
energy potential was used.
The viscoelastic behavior of the SAF 6060 cushion
was defined by using a time-based Prony-series model
for the shear modulus only because the time-dependency
of bulk modulus is generally not significant [5]. Time-
dependent shear relaxation modulus G(t) is given by

 011G
i
Nt
i
i
Gt GGe
 

 
 (2)
where G
i

is the relaxation time and N is the order of the
Prony series. G0 and Gi are the instantaneous shear
modulus and relative shear modulus, respectively. With
reference to the findings by the work of Grujicic et al.
[5], the viscoelastic material parameters for the SAF
6060 cushion were defined such that N = 2, G1 = 0.3003
and τ1 = 0.010014 s, and G2 = 0.1997 and τ2 = 0.1002 s.
2.2.2. Muscle
The muscular portion of the buttock-thigh model was
modeled using a non-linear visco-hyperelastic isotropic
material model to describe the human soft tissue. The
density of soft tissue is 1000 kg/m3 [30] and hyperelastic
portion of the soft tissue is expressed in the form of the
polynomial strain energy potential, W:

2
12
11
1
33 (1)
ij
NN
el i
ij
ij i
i
WCII J
D
 


(3)
where ij
Cand i
D are the material parameters. A higher
N value may provide a better fit to the exact solution.
However, it may cause numerical difficulty in fitting the
material constants and require enough data to cover the
entire range of interest of deformation. Therefore a very
higher N value is not usually recommended. In the pre-
sent study, N = 2 was chosen and the hyperelastic pa-
rameters for the muscle model obtained from a URL,
http://lyle.smu.edu/~hyao/academics.html#Research are
listed in Table 2. Referring to the work of Tang and Tsui
[31], the viscoelastic parameters for the muscle model
were set as G1 = 0.5, K1 = 0.5 and τ1 = 0.8 s.
2.3. Simulation Set-Up
In the present study, ABAQUS explicit dynamics analy-
sis has been performed to deal with the dynamic problem.
As this type of analysis uses a consistent large-deforma-
tion theory, the cushion and the muscle model could un-
dergo large rotations and deformation. The analysis pro-
cedure was implemented by using an explicit integration
rule and diagonal element mass matrices. The equations
of motion for the body were integrated using the follow-
ing explicit central-difference integration rule:
(1)
11
22 ()
2
() ()
ii
tt
NN N
i
ii
uu u




 
and
11
22
()( 1)
() ()
NN N
ii
ii
uutu



where
N
u is a degree of freedom (a displacement or
rotation component) and the subscript i refers to the in-
crement number in an explicit dynamics step. The cen-
tral-difference integration operator is explicit such that
the kinematic state is advanced using known values of
(1/2)
N
i
u
 and ()
N
i
u
 from the previous increment [28].
2.4. Interaction between the Buttock-Thigh
Model and Cushion
In this work, it was assumed that the human sit on the
cushion and then the vertical vibration was applied onto
the bottom of the cushion. The loading was applied to the
model due to the weight of the human, such that a grav-
ity-based body force was used to prescribe the loading.
The interaction between the buttock-thigh model and the
cushion was analyzed in ABAQUS/Explicit using a pen-
alty contact method with finite-sliding. Surface-to-sur-
face contact with a coefficient of friction of 0.5 [5] was
used to define the contact pair between the buttock-thigh
model and cushion. The outer surface of the buttock-
thigh model was defined as the “master surface” which
has a larger surface and higher stiffness, while the sur-
face of the cushion was defined as the “slave surface”
which is softer and has smaller surface than that of the
buttock-thigh model. The surfaces between the bone and
muscle were tied together so that there was no relative
motion between them.
Table 1. Hyperelastic material parameters for the SAF6060
cushion.

1
[MPa] 
1

1

2
[MPa] 
2

2
0.481
× 10-2
0.198
× 1020.145 × 10-10.36 × 10-2 0.198 × 102 0.65 × 10-2
Table 2. Hyperelastic material parameters for the muscle
model (All values in MPa).
C10 C01 C20 C11 C02 D1 D2
0.08556 −0.05841 0.039 −0.02319 0.00851 3.652730

C. Y. TANG ET AL.
723
2.5. Loading and Boundary Conditions
According to fifty-percentile male body segment masses
[25], the total weight is 80.4 kg, including 54.5 kg of the
upper body and 25.9 kg of the lower body. The current
model represented only one side of the body so that the
mass assigned to the model should be halved. Thus, the
weight of the upper body on the iscahial tuberosity was
set as 27.3 kg while the weight of lower leg on the femur
was set as 4.5 kg. Figure 3 shows the positions of the
assigned masses. It was assumed that the cushion was
placed on a rigid surface so that the bottom surface of the
cushion was restricted to move in all directions. More-
over, the upper surface of the buttock-thigh model was
limited to move in X-direction only.
The analyses were performed in two steps. In the first
step, the buttock-thigh model moved to sit on the seat
cushion due to the gravity load (9.81 ms-2). After 15
seconds, there followed a second step in which a vertical
vibration in the form of harmonic motion was applied
onto the bottom of the cushion. The periodic definition
method was used to define the amplitude. The frequen-
cies of the vibration were chosen to be 0.1, 1, 10, 20 and
50 Hz which are the frequencies generated mostly in our
life according to Table 3 [32]. A vibration amplitude of
3 mm was applied to the bottom of the cushion. Each
vibration case was conducted for 50 cycles (see Table
4).
Figure 3. Loading and boundary conditions on the buttock-
thigh model and cushion.
Table 3. Sensitivity of human systems to vibration.
Frequency
Level
Frequency
range Sensitivity Vibration Generator
Low 0 to 1-2 Hz Vestibular system
Ships,
cranes, air-
crafts
Middle 2 to 20-30
Hz
Biomechanics: body
resonances
Vehicles,
aircrafts
High > 20 Hz
Somesthetic recep-
tors in muscles,
tendons, skins
Tools, ma-
chinery
Table 4. Different loading conditions for the simulation.
Loading
Grav
ity
Vertical
vibration
of 0.1
Hz
Vertical
vibration
of 1 Hz
Vertical
vibration
of 10 Hz
Vertical
vibration
of 20 Hz
Vertical
vibration
of 50 Hz
Duration
(Second) 15500 50 5 2.5 1
3. Results and Discussions
As the distribution of the contact pressure at the seated
human/cushion contact interface is important for deter-
mining the seating comfort [5], the maximum contact
pressure at three different locations of the three cushions
at the frequency of 20 Hz have been identified as shown
in Figure 4. Among them, the SAF 6060 cushion shows
the lowest contact pressure at the locations of A and B.
As the location B is under the ichial tuberosities (IT),
minimizing the contact pressure at this location means
minimizing the stress under IT. It can also be observed
from Figure 5 that the maximum von Mises stress near
IT using the SAF 6060 cushion is lower than the other
two cushions by about 30~37% at the same frequency of
20 Hz. Moreover, the SAF 6060 cushion shows lower
stress within the buttock especially at the region near the
cushion as shown in Figure 6. Therefore, the SAF 6060
cushion is effective in reducing the stress below IT, and
also has better pressure distributions at three distinct lo-
cations than the other two.
(a)
(b)
(c)
Figure 4. Maximum contact pressure at the three different
locations of the three cushions at the frequency of 20 Hz. (a)
Elastic 15000; (b) Elastic 20000 and (c) SAF 6060.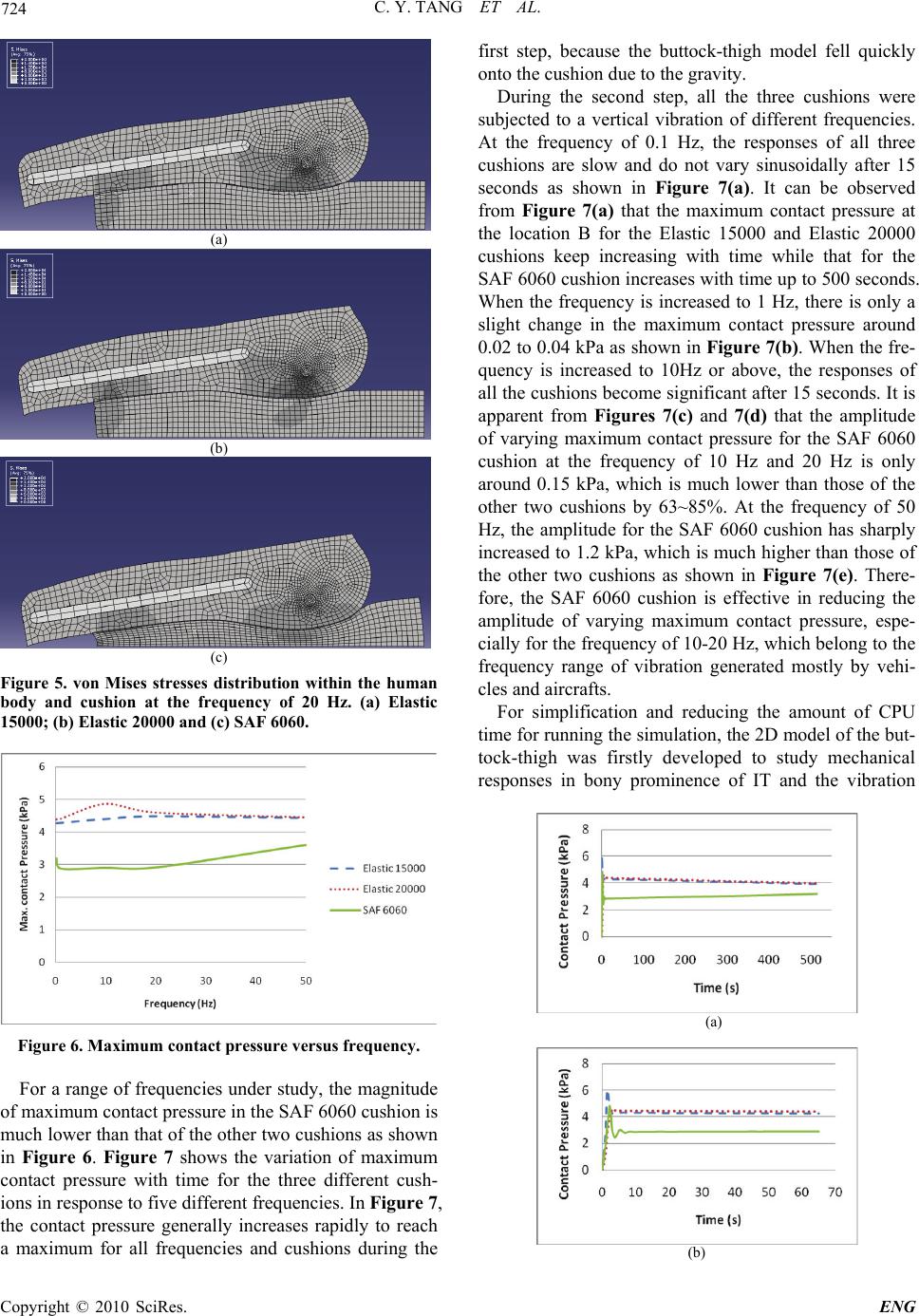

C. Y. TANG ET AL.
724
(a)
(b)
(c)
Figure 5. von Mises stresses distribution within the human
body and cushion at the frequency of 20 Hz. (a) Elastic
15000; (b) Elastic 20000 and (c) SAF 6060.
Figure 6. Maximum contact pressure versus frequency.
For a range of frequencies under study, the magnitude
of maximum contact pressure in the SAF 6060 cushion is
much lower than that of the other two cushions as shown
in Figure 6. Figure 7 shows the variation of maximum
contact pressure with time for the three different cush-
ions in response to five different frequencies. In Figure 7,
the contact pressure generally increases rapidly to reach
a maximum for all frequencies and cushions during the
first step, because the buttock-thigh model fell quickly
onto the cushion due to the gravity.
During the second step, all the three cushions were
subjected to a vertical vibration of different frequencies.
At the frequency of 0.1 Hz, the responses of all three
cushions are slow and do not vary sinusoidally after 15
seconds as shown in Figure 7(a). It can be observed
from Figure 7(a) that the maximum contact pressure at
the location B for the Elastic 15000 and Elastic 20000
cushions keep increasing with time while that for the
SAF 6060 cushion increases with time up to 500 seconds.
When the frequency is increased to 1 Hz, there is only a
slight change in the maximum contact pressure around
0.02 to 0.04 kPa as shown in Figure 7(b). When the fre-
quency is increased to 10Hz or above, the responses of
all the cushions become significant after 15 seconds. It is
apparent from Figures 7(c) and 7(d) that the amplitude
of varying maximum contact pressure for the SAF 6060
cushion at the frequency of 10 Hz and 20 Hz is only
around 0.15 kPa, which is much lower than those of the
other two cushions by 63~85%. At the frequency of 50
Hz, the amplitude for the SAF 6060 cushion has sharply
increased to 1.2 kPa, which is much higher than those of
the other two cushions as shown in Figure 7(e). There-
fore, the SAF 6060 cushion is effective in reducing the
amplitude of varying maximum contact pressure, espe-
cially for the frequency of 10-20 Hz, which belong to the
frequency range of vibration generated mostly by vehi-
cles and aircrafts.
For simplification and reducing the amount of CPU
time for running the simulation, the 2D model of the but-
tock-thigh was firstly developed to study mechanical
responses in bony prominence of IT and the vibration
(a)
(b)

C. Y. TANG ET AL.
725
(c)
(d)
(e)
Figure 7. Variation of maximum contact pressure at loca-
tion B with time at various frequencies. (a) 0.1 Hz; (b) 1 Hz;
(c) 10 Hz; (d) 20 Hz and (e) 50 Hz.
transmissibility of different cushions under vertical vi-
bration at different frequencies. As 3D finite element
model has the advantages of an accurate anatomical ge-
ometry and accuracy in performance, a 3D model repre-
senting more complicated geometry may be developed in
future work.
4. Conclusions
This simplified simulation was designed to mimic the
response of the human under the vertical vibration. Finite
element analysis could evaluate the transmission of vi-
brations onto different seat cushions subjected to varying
frequencies. From this analysis, the SAF 6060 seat cush-
ion was found to be effective in reducing the amplitude
of varying maximum contact pressure, especially for the
frequency of 10-20 Hz, belonging to the frequency range
of vibration generated mostly by vehicles and aircraft.
Thus, this method is useful for designing the material
properties and the shape of the seat cushion for reducing
the transmission of vibrations to users at a certain fre-
quency range.
5. Acknowledgements
The authors would like to thank the Research Grants
Council of the Hong Kong Special Administrative Re-
gion for its support of the project (PolyU 5273/07E).
6. References
[1] M. M. Verver, J. van Hoof, C. W. J. Oomens, J. S. H. M.
Wismans and F. P. T. Baaijens, “A Finite Element Model
of the Human Buttocks for Prediction of Seat Pressure
Distributions,” Computer Methods in Biomechanics and
Biomedical Engineering, Vol. 7, No. 4, 2004, pp. 193-
203.
[2] D. M. Brienza, P. E. Karg, M. J. Geyer, S. Kelsey and E.
Trefler, “The Relationship between Pressure Ulcer Inci-
dence and Buttock-Seat Cushion Interface Pressure in
At-Risk Elderly Wheelchair Users,” Archives of Physical
Medicine and Rehabilitation, Vol. 82, No. 4, 2002, pp.
529-533.
[3] J. M. Black, “Moving toward Consensus on Deep Tissue
Injury and Pressure Ulcer Staging,” Advances in Skin &
Wound Care: The Journal for Prevention and Healing,”
Vol. 18, No. 8, 2005, pp. 415-421.
[4] M. R. Bliss, “Acute Pressure Area Care: Sir James
Paget’s Legacy,” Lancet, Vol. 339, No. 8792, 1992, pp.
221-223.
[5] M. Grujicic, B. Pandurangan, G. Arakere, W. C. Bell, T.
He and X. Xie, “Seat-Cushion and Soft-Tissue Material
Modeling and a Finite Element Investigation of the Seat-
ing Comfort for Passenger-Vehicle Occupants,” Materi-
als & Design, Vol. 30, No. 10, 2009, pp. 4273-4285.
[6] E. Linder-Ganz, N. Shabshin, Y. Itzchak, Z. Yizhar, I.
Siev-Ner and A. Gefen, “Strains and Stresses in Sub-
Dermal Tissues of the Buttocks are Greater in Paraplegics
than in Healthy During Sitting,” Journal of Biomechanics,
Vol. 41, No. 3, 2008, pp. 567-580.
[7] M. Makhsous, D. Lim, R. Hendrix, J. Bankard, W. Z.
Rymer and L. Fang, “Finite Element Analysis for Evalua-
tion of Pressure Ulcer on the Buttock: Development and
Vvalidation,” IEEE Transactions on Neural Systems and
Rehabilitation Engineering, Vol. 15, No. 4, 2007, pp.
517-525.
[8] R. Ragan, T. W. Kernozek, M. Bidar and J. W. Matheson,
“Seat-Interface Pressures on Various Thicknesses of
Foam Wheelchair Cushions: A Finite Modeling Ap-
proach,” Archives of Physical Medicine and Rehabilita-
tion, Vol. 83, No. 6, 2002, pp. 872-875.
[9] A. Siefert, S. Pankoke and H. P. Wölfel, “Virtual Opti-
misation of Car Passenger Seats: Simulation of Static and

C. Y. TANG ET AL.
726
Dynamic Effects on Drivers’ Seating Comfort,” Interna-
tional Journal of Industrial Ergonomics, Vol. 38, No. 5-6,
2008, pp. 410-424.
[10] Q. Sun, F. Lin, L. Ruberte, E. Nam, R. Hendrix and M.
Makhsous, “FE Modeling and Analysis of Compressed
Human Buttock-Thigh Tissue,” Proceeding of ASB 29th
Annual Meeting, Georgia, 2005.
[11] B. A. Todd and J. G. Thacker, “Three-Dimensional Com-
puter Model of the Human Buttocks, In Vivo,” Journal of
Rehabilitation Research, Vol. 31, No. 2, 1984, pp. 111-
118.
[12] E. Wagnac, C. Aubin and J. Dansereau, “A New Method
to Generate a Patient-Specific Finite Element Model of
the Human Buttocks,” IEEE transactions on Biomedical
Engineering, Vol. 55, No. 2, 2008, pp. 774-782.
[13] R. A. Cooper, “Wheelchair Selection and Configuration,”
Demos Medical, New York, 1998.
[14] J. L. Minkel, “Seating and Mobility Considerations for
People with Spinal Cord Injury,” Physical Therapy, Vol.
80, No. 7, 2000, pp. 701-709.
[15] X. Wu, S. Rakheja and P. É. Boileau, “Distribution of
Human-Seat Interface Pressure on a Soft Automotive
Seat under Vertical Vibration,” International Journal of
Industrial Ergonomics, Vol. 24, No. 5, 1999, pp. 545-
557.
[16] C. P. DiGiovine, R. A. Cooper, S. G. Fitzgerald, M. L.
Boninger, E. J. Wolf and S. Guo, “Whole-Body Vibration
During Manual Wheelchair Propulsion with Selected Seat
Cushions and Back Supports,” IEEE Transactions on
Neural Systems and Rehabilitation Engineering, Vol. 11,
No. 3, 2003, pp. 311-322.
[17] International Organization for Standardization, “ISO
2631-1: 1997, Mechanical Vibration and Shock - Evalua-
tion of Human Exposure to Whole-Body Vibration,”
Switzerland, 1997.
[18] M. M. Verver, J. van Hoof, C. W. J. Oomens, N. van de
Wouw and J. S. H. M. Wismans, “Estimation of Spinal
Loading in Vertical Vibrations by Numerical Simula-
tion,” Clinical Biomechanics, Vol. 18, No. 9, 2003, pp.
800-811.
[19] S. Kitazaki and M. J. Griffin, “A Modal Analysis of
Whole-Body Vertical Vibration Using a Finite Element
Model of the Human Body,” Journal of Sound and Vi-
bration, Vol. 200, No. 1, 1997, pp. 83-103.
[20] T.-H. Kim, Y.-T. Kim and Y.-S. Yoon, “Development of
a Biomechanical Model of the Human Body in a Sitting
Posture with Vibration Transmissibility in the Vertical
Direction,” International Journal of Industrial Ergonom-
ics, Vol. 35, No. 9, 2005, pp. 817-829.
[21] J. Rosen and M. Arcan, “Modeling the Human Body/Seat
System in a Vibration Environment,” Journal of Biome-
chanical Engineering, Vol. 125, No. 2, 2003, pp. 223-
231.
[22] F. M. L. Amirouche, “Modeling of Human Reactions to
Whole-body Vibration,” Journal of Biomechanical Engi-
neering, Vol. 109, No. 3, 1987, pp. 210-217.
[23] D. R. Huston, C. C. Johnson, M. A. Wood and X. Zhao,
“Vibration Attenuating Characteristics of Air Filled Seat
Cushions,” Journal of Sound and Vibration, Vol. 222, No.
2, 1999, pp. 333-340.
[24] E. J. Wolf, M. S. R. A. Cooper, C. P. DiGiovine, M. L.
Boninger and S. Guo, “Using the Absorbed Power
Method to Evaluate Effectiveness of Vibration Absorp-
tion of Selected Seat Cushions During Manual Wheel-
chair Propulsion,” Medical Engineering & Physics, Vol.
26, No. 9, 2004, pp. 799-806.
[25] R. L. Huston, “Principles of Biomechanics,” CRC Press,
Boca Raton, 2009.
[26] W. Chow and E. Odell, “Deformations and Stresses in
Soft Body Tissues of a Sitting Person,” Journal of Bio-
mechanical Engineering, Vol. 100, No. 2, 1978, pp. 79-
87.
[27] A. Lowe and R. S. Lakes, “Negative Poisson’s Ratio
Foam as Seat Cushion Material,” Cellular Polymers, Vol.
19, No. 3, 2000, pp. 157-167.
[28] ABAQUS Version 6.9-1, “User Documentation,” Das-
sault Systems, 2009.
[29] M. Schrodt, G. Benderoth and A. Kuhhorn, “Hyperelastic
Description of Polymer Soft Foams at Finite Deforma-
tions,” Technische Mechanik, Vol. 25, No. 3-4, 2005, pp.
162-173.
[30] E. Pennestrì, P. P. Valentini and L. Vita, “Comfort
Analysis of Car Occupants: Comparison between Multi-
body and Finite Element Models,” International Journal
of Vehicle Systems Modelling and Testing, Vol. 1, No.
1-3, 2005, pp. 68-78.
[31] C. Y. Tang and C. P. Tsui, “Method of Modeling Muscu-
lar Tissue with Active Finite Elements,” U. S. Patent, The
Hong Kong Polytechnic University, Kowloon (HK),
2006.
[32] D. B. Chaffin, G. B. J. Andersson and B. J. Martin, “Oc-
cupational Biomechanics,” Wiley-Interscience, Hoboken,
2006.