Advances in Ma terials Physics and Che mist ry, 2012, 2, 233-236
doi:10.4236/ampc.2012.24B059 Published Online December 2012 (htt p://www.SciRP.org/journal/ampc)
Optimization of Draft Tube Position in a Spouted Bed Reactor
using Re s ponse Surface Me t hod ology
Elaheh Baghban, A r jomand Mehrabani-Zeinabad
Ch emical Engineering D epartmen t, Isfahan University of Technology, Isfahan, Iran
Received 2012
ABSTRACT
Optimization of draft tube position in a spouted bed reactor used for treatment of wastewater containing low concentration of heavy
metals is investigated in this paper. Response surface methodology is used to optimize the draft tube height, the draft tube width and
the gap between the bottom of the draft tube and the inlet nozzle. It is observed that the draft tube with a height of 60 millimeter,
width of 12 millimeter and the gap of 13 millimeter between its bottom and inlet nozzle, results in optimum value of minimum
spouting velocity, measured 45 cubic centimeter per second (2.7 Liter per minute).
Keywords: Spouted Be d; Draft Tube; Minimum Spouting Velocity; Response Surface Methodology
1. Introduction
Low concentration of heavy metals in contaminated wastewater
result s in lo w reaction r ates over electro de surface area an d thus
special considerations are necessary for reactor selection and
design. Some of the most important requirements of these
reactors are [1]:
• Large active surface area per unit reactor volume
• High mass transfer rate
• High curr ent efficiency
• Hig h cur rent densit y
• Low cell voltage
• Uniform distr i bution of ele c t rode potent ia l
• Low maintenance cos t
The spouted bed electrode studied at Berkeley in a collabora-
tive effort with PASMINCO, the Australian zinc company, may
significantly improve the electrodeposition of heavy metals.
The spouted bed consists of a vessel filled with relatively
coarse particles. A jet of fluid is injected vertically through a
small opening located centrally at the base of the vessel. If the
jet veloci ty is hi gh enough , it causes a stream of part icles to rise
rapidly in a central core within the bed. As the jet expands
above the bed, the fluid velocity drops and the particles fall out
onto the top of an annular region surrounding the central jet.
The particles then move slowly down in the bed until they are
again swept up in the central jet. A spouted bed may incorpo-
rate a ‘‘draft tube’’ to confine the spread of the central jet of
fluid; in this way, spouted beds of large height-to-width ratio
can also be operated. A spouting bed of conducting particles
can then be made into an electrode by incorporating a current
feeder and a diaphragm beyond which lies the counter electrode
[2].
At low flo w rates of electrol yt e, there ar e no p articles passing
through the top of the draft tube and, therefore, no recirculation
of particles. This is the ‘‘fixed bed zone’’; the particles in the
annular region are motionless. At higher flow rates, beyond a
minimum spouting flow rate, p articles issue fro m th e top of the
draft tube and recirculation occurs. This is the ‘‘stable spouted
bed zone’’. The particles descend smoothly in the annular re-
gion. At a yet higher flow rate, the bed starts to behave irregu-
larly, particularly in its upper regions, and the movement of
particles in the annular region is no longer uniformly downward.
It is conjectured that this ‘‘unstable spouted bed zone’’ is inci-
pient fluidization of the particles in the annular region [3].
Hydrodynamics of the spouted bed was investigated by
Verma et al. [3], Piskova and Mörl [4], Duarte et al. [5], Shir-
vanian et al. [6 ,7] and Kazd obin et al. [8] . The posit ive effect o f
draft tube existence on the performance of the spouted bed
reactor u sed for waste water tre atment is obvious. In this paper
the draft tube position and height of the spouted bed of figure 1
is optimized via response surface methodology.
2. Methodology
2.1. Experimental Set-up and P r ocedure
The dimensions of the spouted bed reactor of this study are
shown in Figure 1. The draft tube (with rectangular cross sec-
tion) was formed by vertical aluminum curved strips of differ-
ent height in order to optimize the draft tube height (h), the
draft tube width (d) and the gap dimension between the inlet
nozzl e and the bottom of the draft tube (g). The curvature of the
bottom of the draft tube was designed due to gained results of
the previous runs which confirm the positive effect of this cur-
vature on decreasing the minimum spouting velocity. The inlet
nozzle diameter was set to 4 mm based on previous runs in
order to minimize the minimum spouting velocity as well as to
create the s table spouting.
The reactor inlet flow enters fro m th e inlet bottom no zzle af-
ter passing through a rotameter and exits from an opening in-
serted beside the reactor. The pressure drop was measured us-
ing a manometer. The Plexiglas construction and ‘‘flat’’ geo-
metry of the reactor provided the observation of the spouted
bed, including the interior of the draft tube.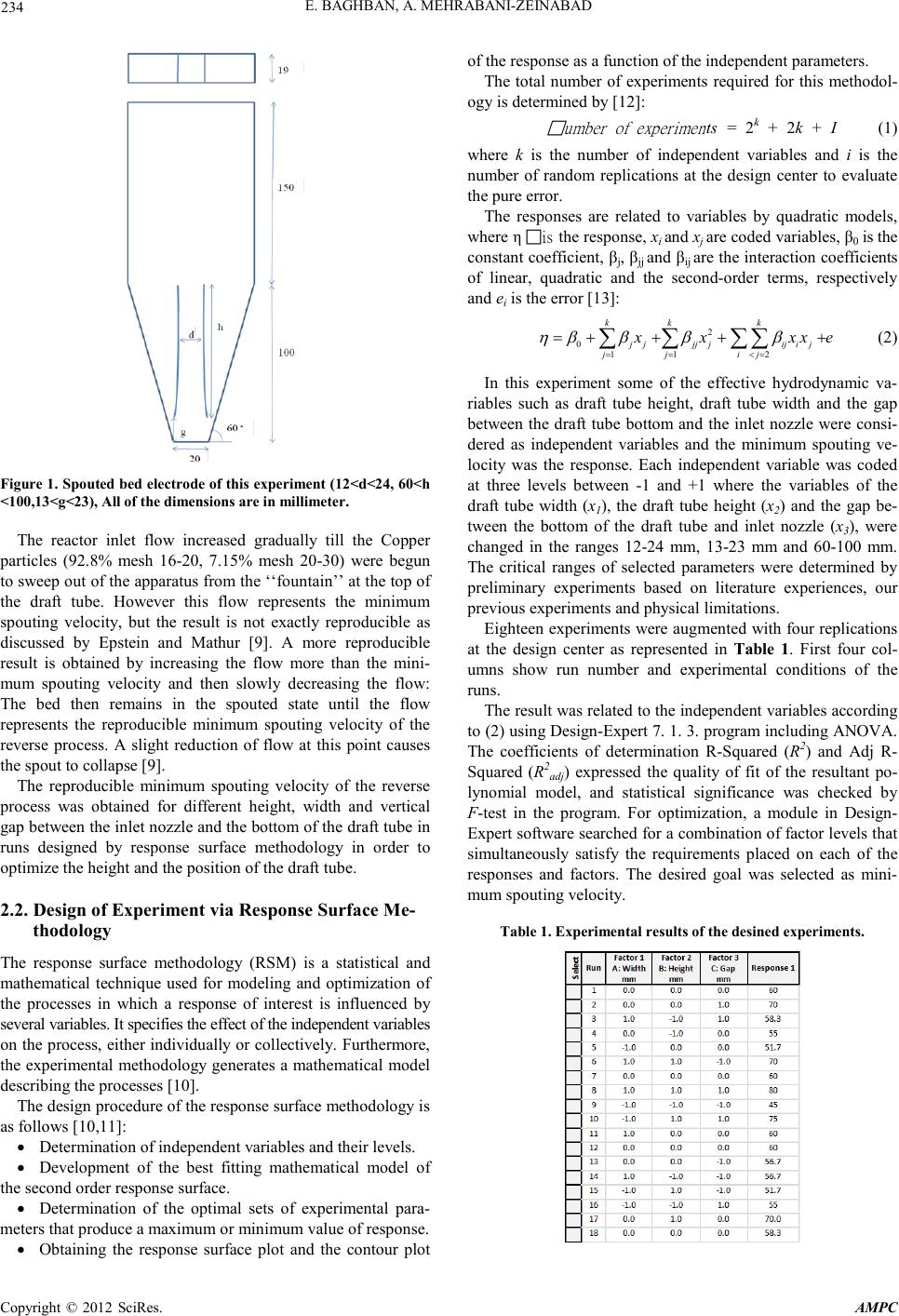

E. BAGH BAN , A. MEH R AB ANI-Z EIN ABAD
234
Figure 1. Spouted bed electrode of this experiment (12<d<24, 60<h
<100,13<g<23), All of the dimensions are in millimeter.
The reactor inlet flow increased gradually till the Copper
particles (92.8% mesh 16-20, 7.15% mesh 20-30) were begun
to sweep out of the apparatus from the ‘‘fountain’’ at the top of
the draft tube. However this flow represents the minimum
spouting velocity, but the result is not exactly reproducible as
discussed by Epstein and Mathur [9]. A more reproducible
result is obtained by increasing the flow more than the mini-
mum spouting velocity and then slowly decreasing the flow:
The bed then remains in the spouted state until the flow
represents the reproducible minimum spouting velocity of the
reverse process. A slight reduction of flow at this point causes
the spout to collapse [9].
The reproducible minimum spouting velocity of the reverse
process was obtained for different height, width and vertical
gap between the inlet nozzle and the bottom of the draft tube in
runs designed by response surface methodology in order to
optimize the height and the position of the draft tube.
2.2. Design of Experiment via Response Surface Me-
thodology
The response surface methodology (RSM) is a statistical and
mathematical technique used for modeling and optimization of
the processes in which a response of interest is influenced by
several variables. It specifies the effect of the independent variables
on the process, either individually or collectively. Furthermore,
the experimental method ology generates a mathematical model
describing the processes [10].
The design procedure of the response surface methodology is
as follows [10,11]:
• Determinat ion of in depend ent variables and thei r levels.
• Development of the best fitting mathematical model of
the seco nd order res ponse surface.
• Determination of the optimal sets of experimental para-
meters th at produce a maximum o r minimum value of response.
• Obtaining the response surface plot and the contour plot
of the response as a function of the independ ent parameters.
The total number of experiments required for this methodol-
ogy is determined by [12]:
umber of experimen ts = 2k + 2k + I (1)
where k is the number of independent variables and i is the
number of random replications at the design center to evaluate
the pure error.
The responses are related to variables by quadratic models,
where η is the response, xi and xj are coded variables, β0 is the
constant coefficient, βj, βjj and βij are t he interact ion coefficients
of linear, quadratic and the second-order terms, respectively
and ei is the error [13]:
2
011 2
kk k
j jjj jiji j
j jij
xxxx e ηβ βββ
= =<=
=+++ +
∑ ∑∑∑
(2)
In this experiment some of the effective hydrodynamic va-
riables such as draft tube height, draft tube width and the gap
between the draft tube bottom and the inlet nozzle were consi-
dered as independent variables and the minimum spouting ve-
locity was the response. Each independent variable was coded
at three levels between -1 and +1 where the variables of the
draft tube width (x1), the draft tube height (x2) and the gap be-
tween the bottom of the draft tube and inlet nozzle (x3), were
changed in the ranges 12-24 mm, 13-23 mm and 60-100 mm.
The critical ranges of selected parameters were determined by
preliminary experiments based on literature experiences, our
previous experiments and physical limitations.
Eighteen experiments were augmented with four repli cations
at the design center as represented in Table 1. First four col-
umns show run number and experimental conditions of the
runs.
The result was related to th e independ ent variabl es accord ing
to (2) using Design-Expert 7. 1. 3. program including ANOVA.
The coefficients of determination R-Squared (R2) and Adj R-
Squ ared (R2adj) expressed the quality of fit of the resultant po-
lynomial model, and statistical significance was checked by
F-test in the program. For optimization, a module in Design -
Expert so ftware searched for a combination of factor levels that
simultaneously satisfy the requirements placed on each of the
responses and factors. The desired goal was selected as mini-
mum spouting velocity.
Tabl e 1. Experimental results of the desined experiments.

E. BAGH BAN , A. MEHRABANI-Z EIN ABAD
235
3. Results
3.1. Optimization of Draft Tube Position via RSM
The experi mental result s of the design ed experiments sh own in
Table 1 were related to the independent variables as shown in
(3):
η=59.78+4.66A+7.67B+5.82C+1.04AB-2.71AC
+2.71BC-4.14A2+2.51B2+3. 36C2 (3)
ANOVA results of this quadratic model are presented in Ta-
ble 2. In the table, model F-value of 148.51 implies that the
model is significant. Prob > F is less than 0.05 for all terms,
indicating that terms are significant for the equation. Adeq Pre-
cision of 48.508, which indicates an adequate signal to noise
ratio, also confirms the model validity. The Pred R-Squared of
0.9298 is also in reasonable agreement with the Adj R-Squared
of 0.9874.
The result s were op timized by Design-Expert soft ware using
the approximated function in (3). Optimized conditions under
specified constraints were obtained for minimum height (6o
mm), minimum width (12 mm) and minimum vertical gap
(13mm) of the designed draft tube. Under these optimized con-
ditions, observed minimum spouting velocity was 45cm3/s.
Equation (3) has been used to visualize the effects of experi-
mental factors on responses in 3D graphs of Figure 2 and Fig-
ure 3.
Tabl e 2. ANOVA results of the predicted quadretic model.
Source Sum of squres Mea n s qure F-Value Prob>F
Model 1347.30 149.70 148.51 <0.0001
Residual 8.06 1.01
Lack of F i t 5.90 1.18 1.63 0.3644
Pure Error 2.17 0.72
R2=0.9941, R2adj=0.9874, R2Pr e =0.9298, Adeq Precision =48.508
A: Width
Figure 2. The eff ect of t he draft tube height and width on minimum
spouting veloci ty when the gap between inlet nozzle and draft tube
is optimum.
The expected dependence of the draft tube height and mini-
mum spouting velocity is shown in Figure 2. When the draft
tube height is increased, the minimum energy required by the
particles t o sweep out of the draft tube is in creased due to high-
er vertical distance. Consequently, the minimum required ve-
locity for creating spout is increased. However decrease in the
draft tube height has a positive effect on minimum spouting
velocity and thus total energy requirements, buts it is limited by
the filled bed height, which is six centimeter in this experiment.
Minimum spouting velocity is increased via increase of the
draft tube width when the gap between the i nlet nozzle and the
draft tube is constant in its optimum value (x3= -1). The impor-
tant role of the draft tube in the spouted bed reactor is to sepa-
rate inner fluidized bed zone and outer packed bed zone. When
the d raft tube width i s increased, the fluidization zone expands,
but has no effect on the spouting zone created by the fluid jet.
This means the bed operation approaches fluidized bed which
requires more fluidization velocity to be fluidized. Despite the
positive effect of decreasing the draft tube width on minimum
spouting velocity, more decrease of the draft tube width, causes
some of the agglomerated particles to stick in the tube and the
bed stability to dissipate.
The gap between the inlet nozzl e and the draft tube als o has a
not iceable effect on minimum spouting velocity as shown in
Figure 3. Upon increasing the gap between inlet nozzle and
draft tube, the fraction of the inflowing liquid diverted from the
draft tube by passing up through the annular region is increased.
Consequently, the internal jet power is deceased that must be
compensated by higher spouting velocity.
4. Conclusion
In this paper, the dependence of minimum spouting ve-
locity and the draft tube height, the draft tube width and
the gap between the bottom of the draft tube and t he i nle t
nozz le wa s in ves ti ga ted t hro u gh exp e ri ments desi gne d b y
response surface methodology. The mathematical model
fitted by Design Expert software and validated by
ANOVA, was used in order to optimize the mentioned
variables.
Figure 3. The effect of the draft tube height and the gap between
inlet nozzl e and the draft tube on minimum spouting velocity when
the draft tube width is optimum.

E. BAGH BAN , A. MEH R AB ANI-Z EIN ABAD
236
It was observed that the optimized draft tube height, draft
tube width and the gap between the draft tube and the inlet
nozzle are 60 mm, 12 mm and 13 mm, respectively which
represents the minimum spouting velocity of 45 cubic centime-
ter per second (2.7 Liter p er min ut e).
REFERENCES
[1] K. Scott, “ Journal Review. Electrochemical Methods for the
Treatment of Industrial Process Streams and Effluents. Part 1:
Cell Design and the Recovery of Dissolved Metals by Electro-
deposition”, Developments in Chemical Engineering and Mineral
Processing, Vol. 1, pp. 185-197, October 1992.
[2] V. Jiricny, A. Roy, J. W. Evans, “Electrodepositi on of Zin k from
Sodium Zincate/Hydroxide Electrolytes in a Spouted Bed Elec-
trode”, Metallurgical and Materials Transactions, Vol. 31B, pp.
755-766, Aug, 2000
[3] A. Verma, J. C. Salas-Mora les, J.W. Evans , “Spout ed Bed Elec-
trowinning of Zinc: Part II. Investigations of the Dynamics of
Particles in Large Thin Spouted Beds”, Metallurgical and Mate-
rials Transactions, Vol. 28B, pp . 69-79, Feb 1997
[4] E. Piskova and L. Mörl, “Fluidization regimes in different
spouted bed apparatus constructions”, Chemical Engineering and
Processin g, Vol. 46 , pp.695-702, 2007.
[5] C. R. Duarte, V. V. Murata, A. S. Barrozo, “ Experimental and
Numerical Study of Spouted Bed Fluid Dynamics”, Brazilian
Journal of Chemical Engineering, Vol. 25, pp.
95-107,January-Marc h 2 008.
[6] P. A. Shirvanian, J. M. Calo, G. Hradil, “ Numerical Simulation
of Fluid-Particle Hydrodynamics in a Rectangular Spouted Ves-
sel”; Interrnational Journal of Multiphase Flow, Vol. 32, pp.
739-753, 2006.
[7] P.A. Shirvanian, J. M. Calo, “Hydrodynamic scaling of a rec-
tangular spouted vessel with a draft duct”, Chemical Engineering
Journ al, Vol. 103, pp. 29-34, 2004
[8] K. Kazdobin, N. Shvab, S. Tsapakh, “ Scaling-up of Fluidized
Bed Electrochemical Reactors”, Chemical Engineering Journal,
Vol. 79 , pp. 20 3-209, October 2000
[9] K. B. Mathur., N. Epstein, “Spouted Beds”,Academic Press. Inc.,
1974, pp. 15-25.
[10] R. Saravanathamizhan, N. Mohan, N. Balasubramanian, V.
Ramamurthi , C. Ahmed Basha, “ Evaluation of Elec-
tro-Oxidation of Textile Effluent Using Response Surface Me-
thod”, Clean , Vol. 35, pp. 355-361, July 2007
[11] D. Granato, G. F. Branco, V. M. de Araűjo Calado, “ Experi-
mental Design and Application of Response Surface Methodol-
ogy for Process Modelling and Optimization: A Review”, Food
Resear ch Internatio nal , 20 0 1
[12] B. K. Körbahti, A. Tanyolaç. “Electrochemical Treatment of
Simulated Textile Wastewater with Industrial Components and
Levafix B lue CA Reacti ve Dye: Opt imizati on th rough Response
Surface Methodology”. Journal of Hazardous Materials, Vol. 151,
pp. 422-431, 2008
[13] B. K. Körbahti, N. Aktaş, A. Tanyolaç.,” Optimization of Elec-
trochemical Treatment of Industrial Paint Wasteater with Re-
sponse Surface Methodology’, Journal of Hazardous Materials,
Vol. 148, pp. 83-90, 2008.