Cross-kink multi-sol i ton solutions for th e (3+ 1)-D
Jimbo-Miwa equat ion
Zhenhui Xu1, Hanlin Chen
2
1Applied Technology College, Southwest University of Science and Technology, Mianyang
621010,PR C hina,e- mail: xuz he nhui@swu s t . e du. cn,xuzhenhui19@16 3.com
2
School of Science, Southwest University of Science and Technology, Mianyang 621010, China.
Abstract - In this paper, by using bilinear form and extended three-wave type of ans¨atz approach, we obtain new
cross-kink mul ti -soliton solutions of the (3+1)-dimensional J imbo -Miwa equati on, including the periodic b reather-type of
kink three-soliton solutions, the cross-kink four-soliton solutions, the doubly periodic breathertype of soliton solutions
and the doubly periodic breather-type of cross-kink two-soliton solutions. It is shown that the generalized three-wave
method, with the help of symbolic computation, provides an effective and powerful mathematical tool for solving high
dimensional nonlinear evolution equations in mathematical physics.
Keyword s -Jimbo-Mi wa equation; Extended three-wave method; Cross-kin k mult i -soli ton.
1 Introduction
It is well known that many important phenomena in
physics and other fields are described by nonlinear partial
differential equations. As mathematical models of these
phenomena, the investigation of exact solutions is
important in mathematical physics. Many methods are
availabl e to look for exact solutions of nonlinear evolution
equations, such as the inverse scattering method, the Lie
group method, the mappi ng method, Exp-function method,
ans¨atz technique, three-wave tape of ansatz approach
and so on [1-3]. In this paper, we consider the following
Jimbo-Miwa equation:
3 3230
xxxy xxy xxyyt xz
uuuu uuu+ ++−=
(1)
which comes from the second member of a KP-hierarchy
called Jimbo-Miwa equation firstly introduced by
Jimbo-Mi wa [ 4 ]. By means o f the two-solito n method and
bilinear methods, the the two-soliton solutions, three-wave
solutions of the Jimbo-Miwa were found as well as [5-6].
In this paper, we discuss further the (3 + 1)-dimensional
Jimbo-Miwa equation, by using bilinear form and extend
thr ee-wave type of ansatz approach, respectively[7-9],
Some new cross-kink multi-soliton solutions are obtained.
2 The multi-soliton solutions
We assume
2(ln )
x
uf=
(2)
Where
(, ,,)ff xyzt=
is unknown real function.
Substituting Eq.(2) into Eq.(1), we can reduce Eq.(1) into
the following Hirota bilinear equation
3
(23)0
x yytxz
DDDDDD ff+ −⋅=
(3)
where the Hirota bilinear operator D is defined by
(
,0mn≥
)
''
''
'' ,
(,) (,)
()() [(,)(,)]
mn
xt
mn
x xt t
D D fxtgxt
fxtgx t
xx tt= =
⋅
∂∂∂∂
=−− ⋅
∂∂∂∂
(4)
Now we suppose the solution of Eq.(3) as
12 34
cos( )sinh( )cosh( )fe e
ξξ
δ ηδγδθδ
−
=++ ++
(5)
Where
1111
ax by czdt ξ = +++
,
2222
a xbyczdt η = +++
,
3333
ax byczdt γ = +++
4444
a xbyczdt θ = +++
and
, , ,(1,2,3,4)
iii i
abcdi=
are some constants to be
determined later. Substituting Eq.(5) into Eq.(3)and
equating all the coefficients of different powers of
,, sin(), cos(), sinh(), cosh(), sinh(), cosh()ee
ξξ
ηη γγθθ
−
and consta nt term to zero, we can obtain a set of algebraic
equations for
, , ,,(1,2,3,4;
iii ij
abcd i δ =
.
1,2,3,4)j=
,
Solving the system with the aid of Maple, we get the
following results:
Case (I):
Open Journal of Applied Sciences
Supplement：2012 world Congress on Engineering and Technology
215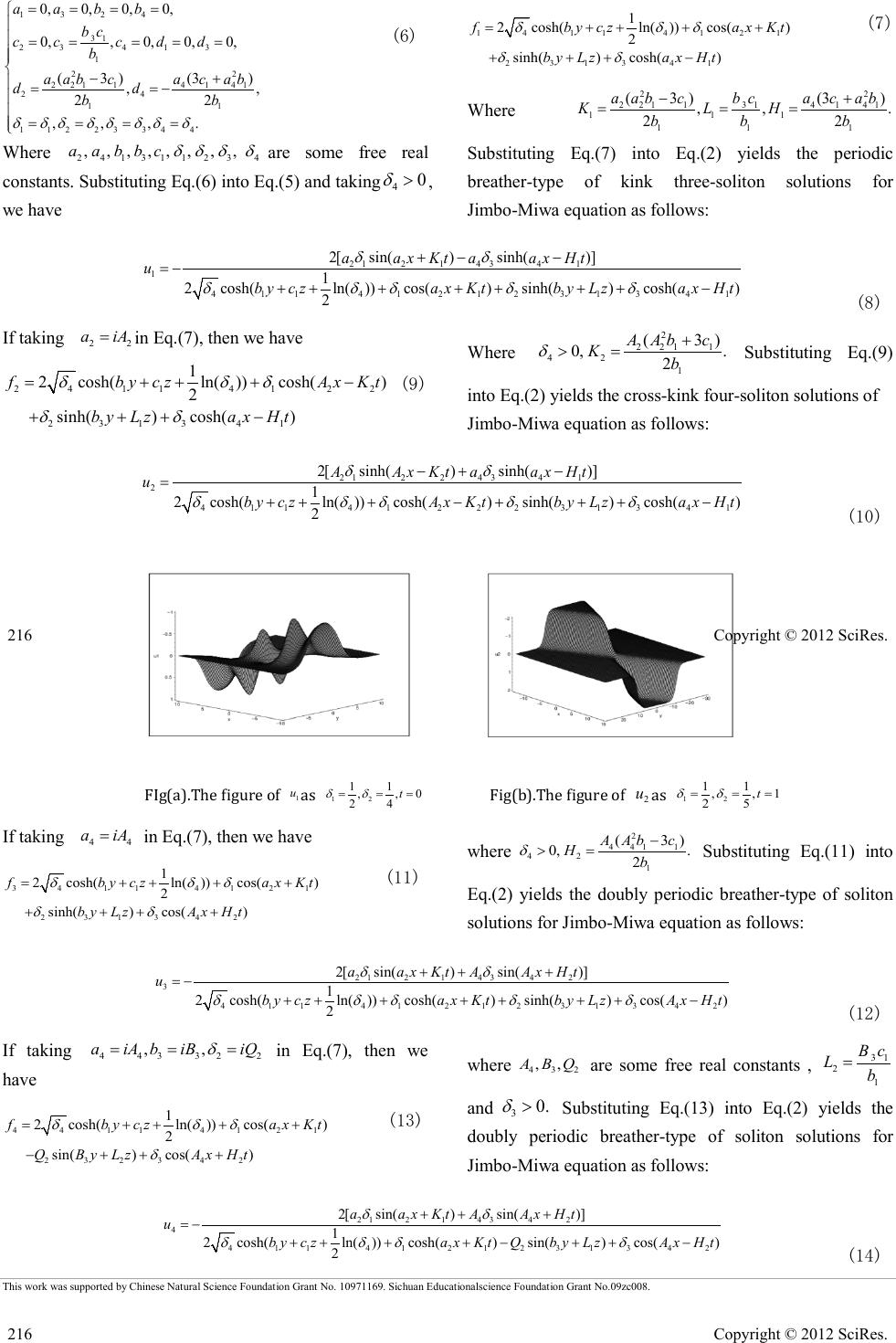

This work was supported by Chinese Natural Science Foundation Grant No. 10971169. Sichuan Educationalscience Foundation Grant No.09zc008.
1 324
31
23413
1
22
2 2114141
24
11
1122334 4
0,0, 0, 0,
0,, 0, 0,0,
(3 )(3)
,,
22
,,,.
aab b
bc
cc cdd
b
a abcacab
dd
bb
δδδδδδδδ
== = =


= ====



−+
== −

== = =


(6)
Where
241311 2 34
,,, ,, ,,,a abbc δδδ δ
are some free real
constant s . Substit uting Eq.(6) into Eq.(5) and ta ki ng
40
δ >
,
we have
14114 12 1
23134 1
1
2cosh(ln( ))cos()
2
sinh() cosh()
fby czaxKt
by LzaxHt
δ δδ
δδ
=++ ++
+ ++−
（7）
Where
22
31
2 2114141
1 11
11 1
(3 )(3)
,, .
22
bc
a abcacab
K LH
bb b
−+
== =
Substituting Eq.(7) into Eq.(2) yields the periodic
breather-type of kink three-soliton solutions for
Jimbo-Miwa equation as follows:
2121 4341
1
4114121 231341
2[ sin()sinh()]
1
2cosh(ln( ))cos()sinh()cosh()
2
aaxKtaax Ht
uby czax Ktby Lzax Ht
δδ
δδδ δδ
+− −
= −
+++++++−
(8)
If taking
22
a iA=
in Eq.(7), then we ha ve
24114122
231 34 1
1
2cosh(ln( ))cosh()
2
sinh() cosh()
fby czAx Kt
byLzax Ht
δ δδ
δδ
=++ +−
+ ++−
(9)
Where
2
2 211
42
1
( 3)
0, .
2
A Abc
Kb
δ
+
>=
Substituting Eq.(9)
into Eq.(2) yields the cross-kink four-soliton solutions of
Jimbo-Miwa equation as follows:
21224341
2
411412223 1341
2[ sinh()sinh()]
1
2cosh(ln( ))cosh()sinh()cosh()
2
AAxKt aaxHt
ubyczAxKt byLzaxHt
δδ
δδδδ δ
−+ −
=
+++−++ +−
(10)
FIg(a).The figure of
1
u
as
12
11
, ,0
24
t δδ == =
Fig(b).The figure of
2
u
as
12
11
, ,1
25
t δδ == =
If taking
44
a iA=
in Eq.(7), then we have
34114 121
231 34 2
1
2cosh(ln( ))cos()
2
sinh() cos()
fby czaxKt
byLzAx Ht
δ δδ
δδ
=++ ++
+++ +
(11)
where
2
4 411
42
1
( 3)
0, .
2
A Abc
Hb δ
−
>=
Substituting Eq.(11) into
Eq.(2) yields the doubly periodic breather-type of soliton
solu t ions for Jimbo-Miwa equation as follows:
21 2 1 4342
3
4114121231342
2[sin()sin()]
1
2cosh(ln( ))cosh()sinh()cos()
2
aaxKt AAxHt
uby czax KtbyLzAx Ht
δδ
δδδδ δ
++ +
= −
++++++ +−
(12)
If taking
4 43 322
,,aiAbiBiQ δ = ==
in Eq.(7), then we
have
44114121
232 34 2
1
2cosh(ln( ))cos()
2
sin() cos()
fby czaxKt
QBy LzAxHt
δ δδ
δ
=++ ++
− +++
(13)
where
432
,,ABQ
are some free real constants ,
31
2
1
Bc
Lb =
and
3
0. δ >
Substituting Eq.(13) into Eq.(2) yields the
doubly periodic breather-type of soliton solutions for
Jimbo-Miwa equation as follows:
212 1 4342
4
411412123 1342
2[ sin()sin()]
1
2cosh(ln( ))cosh()sin()cos()
2
aaxKt AAxHt
uby czaxKtQbyLzAx Ht
δδ
δ δδδ
+++
= −
++++ −+ +−
(14)
216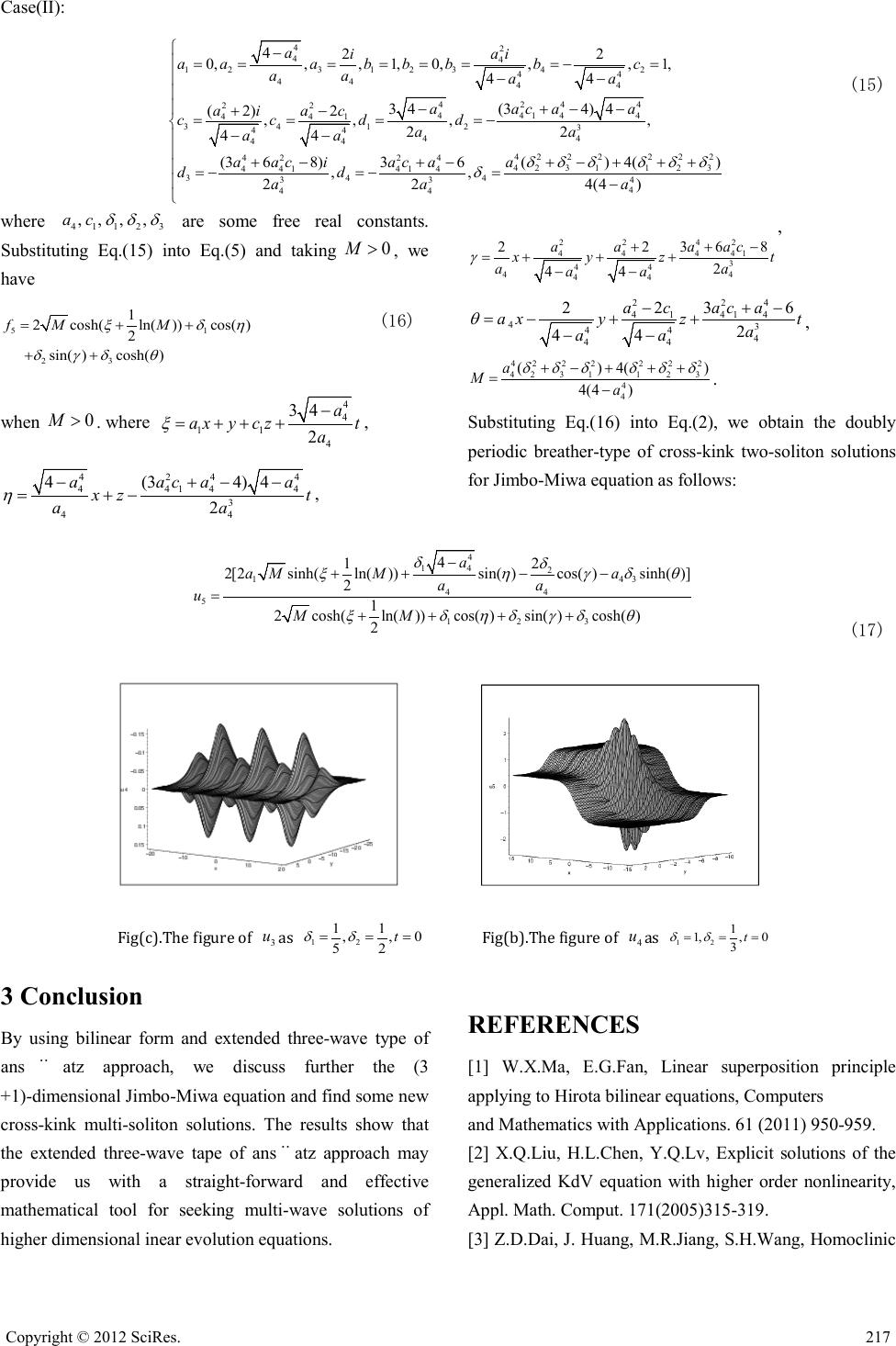
216

Case (II):
42
44
1 2312 342
44
44 44
424 4
22 441 44
4 41
3 4123
44
44
44
42 2 422 442 3 1
44141 4
3 44
33
44
422
0,,, 1,0,,,1,
44
3 4(34) 4
( 2)2
,, ,,
22
44
( (3 68)36
,,
22
aai
i
a aabb bbc
aa aa
aac aa
a iac
c cdd
aa
aa
a aaciaca
dd
aa
δδδ
δ
−
=======−=
−−
−+− −
+−
==== −
−−
+− + −+−
=− =−=
2 222
123
4
4
) 4()
4(4 )a
δδδ







+ ++

−

(15)
where
411 2 3
,,, ,ac δδδ
are some free real constants.
Substituting Eq.(15) into Eq.(5) and taking
0M>
, we
have
51
23
1
2cosh(ln())cos( )
2
sin( )cosh( )
fMM ξ δη
δ γδθ
= ++
++
(16)
when
0M>
. where
4
4
11
4
34
2
a
axy czt
a
ξ
−
=+++
,
4244
44144
3
44
4(34)4
2
aacaa
xz t
aa
η
−+− −
= +−
,
2 242
444 41
3
44
44
44
2 368
2
2
44
aaa ac
xyz t
aa
aa
γ
+ +−
=+++
−−
,
2 24
4141 4
43
44 4
44
23 6 2
2
44
acac a
ax y zt
a
aa
θ
− +−
=−++
−−
,
4222 222
4231123
4
4
() 4()
4(4 )
a
Ma
δδδδδδ +− +++
=−
.
Substituting Eq.(16) into Eq.(2), we obtain the doubly
periodic breather-type of cross-kink two-soliton solutions
for Jimbo-Miwa equation as follows:
4
14 2
1 43
44
5
1 23
42
1
2[2sinh(ln())sin( )cos()sinh( )]
21
2cosh(ln())cos( )sin( )cosh( )
2
a
aM Ma
aa
uMM
δδ
ξηγδθ
ξδηδγδθ
−
++− −
=
+ +++
(17)
Fig(c).The figure of
3
u
as
12
11
, ,0
52
t δδ == =
Fig(b).The figure of
4
u
as
12
1
1,, 0
3t
δδ ===
3 Conclusion
By using bilinear form and extended three-wave type of
ans ¨atz approach, we discuss further the (3
+1)-dimensional Jimbo-Miwa equation and find some new
cros s -kink multi-soliton solutions. The results show that
the extended three-wave tape of ans¨atz approach may
provide us with a str aight -forward and effective
mathematical tool for seeking multi-wave solutions of
higher dimensional inear evolution equations.
REFERENCES
[1] W.X.Ma, E.G.Fan, Linear superposition principle
applying to Hirota bilinear equa tions, Computers
and Mathematics with Applications. 61 (2011) 950-959.
[2] X.Q.Liu, H.L.Chen, Y.Q.Lv, Explicit solutions of the
generalized KdV equation with higher order nonlinearity,
Appl. Math. Comput. 171(2005)315-319 .
[3] Z.D.Dai , J. Huang, M.R.Jiang, S.H.Wang, Ho moclinic
217

orbits and periodic solitons for Boussinesq equation with
even constraint, Chaos Soliton and Fractals. 26 (2005)
1189-1194 .
[4] M. Jimbo, T. Miwa, Publ. Res. Inst. Math. Sci. 19
(1983) 943; MathSciNet.
[5] Z.D.Dai, Z.T.Li, Z.J.Liu, D.L.Li, Exact cross
kink-wave solutions and resonance for the JimboCMiwa
equation, Physica A 384 (2007) 285C290.
[6] Z.T.Li, Z.D.Dai, J.Liu, Exact three-wave solutions for
the (3 + 1)-dimensional Jimbo-Miwa equation,
Computers and Mathematics with Applications 61 (2011)
2062C2 066.
[7] Z.H.Xu, D.Q.Xian, New periodic solitary-wave
solutions for the Benjiamin Ono equation, Applied
Mathematics and Computation. 215 (2010) 4439-4442.
[8] K.W.Chow, A class of doubly periodic waves for
nonlinear evolution equations, Wave Motion. 35 (2002)
71-90.
[9] Z.H.Xu, X.Q. Liu, Explicit Peaked Wave Solution to
the Generalized Camassa-Holm Equation, Acta
Mathematicae Applica tae Sinica. 26(2)(20 10)277-282.
218
218