Effect of user fee on patient’s welfare and efficiency in a two tier health care market

doi:10.4236/health.2010.29164

Paper Menu >>

Journal Menu >>

Vol.2, No.9, 1110-1119 (2010) Health

doi:10.4236/health.2010.29164

Effect of user fee on patient’s welfare and efficiency in a

two tier health care market

Eugenia Amporfu

Kwame Nkrumah University of Science and Technology, Kumasi, Ghana; eamporfu@gmail.com

Received 26 March 2010; revised 27 May 2010; accepted 29 May 2010.

ABSTRACT

This is a theoretical paper examining the effect

of user fee on patients’ welfare and social wel-

fare under three forms of provider reimburse-

ments: full cost, prospective payment and cost

sharing. The paper extends Rickman and

McGuire (1999) by introducing user fee to the

public sector and maintaining the assumption

that providers can work in both the private and

public health sectors. Contrary to previous

studies, this study shows that efficiency is

possible under the full cost reimbursement. The

paper also shows the conditions under which

efficiency is possible under each reimburse-

ment scheme. Patient’s welfare can improve

with the introduction of user fee when services

in the public and private sector are comple-

mentary.

Keywords: User Fee; Two Tier Healthcare; Mixed

Financing; Prospective Payment; Cost Sharing

1. INTRODUCTION

Many countries have the two tier health care system: the

coexistence of public and private health care sectors.

And over the years, public health care reform in many

countries has involved a switch from fully financed sys-

tem to mixed ones [1,2]. Such a switch is observed in

both industrialized and developing countries. The mixed

financing system involves making the patient bear at

least part of the cost of care provided in the public sector.

The intention is to partly relieve the government of the

burden of funding public health care and at the same

time reduce excessive use of care that might exist under

the fully funded system. Various payment schemes are

also used in the public health sector to pay health care

providers. The purpose of this paper is to find the impact

of patient direct payment at the point of purchase, in the

public health sector, on the efficiency of three payment

schemes: full cost reimbursement, prospective payment

and cost sharing in a two tier health care sector.

Example of mixed financing systems in industrialised

countries consists of a combination of compulsory social

security system covering a package of essential services

and private insurance policy to cover the rest. The pa-

tient then has to pay premium and co-payment [3]. Some

of the industrialised countries that have adopted this

include Australia, Italy, and the United States (in the

Medicare plan) [3]. In developing countries the mixed

financing system in general involves introduction of user

fee. Many Sub-Saharan countries such as Cote d’Ivoire,

Kenya, and Nigeria have adopted this system.

There is considerable theoretical literature on the ef-

fect of private insurance (with co-payment) on quality of

care and the efficient provision of services (e.g., [4-8].

While these analyses centred more on the effect of in-

surance on quality and efficiency of services than on the

reimbursement schemes, others examined the role of

different reimbursement schemes in the efficient provi-

sion of services [9-17]. The question then is does the

effect of mixed financing system on efficiency depend

on the type of reimbursement scheme to providers.

To answer the question, the current paper extended a

model on a two tier health care system in [16] that ex-

amined the effect of the private health care sector on the

efficiency of provider reimbursement schemes. The ex-

tension involves the introduction of a user fee to the

public sector. Reference [16] in turn, was an extension of

[18], which examined the effect of reimbursement

scheme on the supply of services in the public sector. In

their study, the public health care was a fully funded

system (e.g., the National Health Services in the Britain)

and so the patient did not pay for the services received.

Reference [16] extended [18] by including a private

sector while maintaining the full funding system in the

public sector. The specific interest in the paper then is to

examine how their results of the two previous papers

would be affected when a user fee is included in the

public sector.

E. Amporfu / HEALTH 2 (2010) 1110-1119

1111

Earlier studies that examined health production in

private and public sector with user fee in the public sec-

tor include [18-20]. Reference [18] presented an empiri-

cal model in which effort was not observable and pa-

tients had to choose medical contracts for health care

provision from government and mission hospitals in the

Cameroun. Reference [20] also presented an empirical

study, which examined how the introduction of user fee

to the public sector affects quality and accessibility of

services. In a theoretical model in which public sector

services are covered partly by compulsory social insur-

ance, partly by private insurance and partly by

out-of-pocket, [21] examined the conditions for optimal

rates of social insurance and private coinsurance. Fol-

lowing [16] the current paper focuses on three forms of

provider-reimbursement schemes: full-cost reimburse-

ment, prospective payment and cost sharing.

2. METHOD

This is a model of mixed health care market, public and

private, that allows physicians to work in both sectors.

Let q represent publicly provided health care received by

a representative patient and s represent privately pro-

vided health care. The patient receives benefit, B(q,s),

from treatment and pays for it according to the marginal

benefit it provides: Bs(.)s, for treatment in the private

sector and (1-



)Bq(.)q for treatment in the public sector,

where 0 <



< 1 is the fraction of the fee paid by the

government. These fees do not have to equal the full cost

of treatment. The patient could have partial insurance

and bear only part of the cost of treatment. What is im-

portant is that the patient bears some cost for treatment

in both sectors. B(.) has positive marginal products, with

unique maxima in q and s, Bqq < 0, Bss < 0. Treatments, q

and s, can be complements, Bsq(.) > 0, substitutes, Bsq(.)

< 0 or unrelated Bsq(.) = 0. Treatments are complements

if, for example, the physician uses the public sector to

request the patient to do some tests in the private sector

to help with diagnoses in the public sector. When the

physician uses, for example, the private sector to treat an

illness that can be treated in the public sector then q and

s are substitutes. The patient’s net benefit is:

(,)(,)(,)(1)(,)

NqsBqsB qssBqsq





 (1)

With the exception of the last term on the RHS, which

represents the fee in the public sector, (1) is identical to

the patient’s net benefit in [16]. The marginal net bene-

fits are:

(,)(,)(1)(,)(,) 0

qq qqsq

NqsB qsqBqssBqs





 

(2)

(,)(,) (1)(,)0

sss qs

NqssB qsBqsq





 

(3)

Physicians are regulated and are often expected to

follow a code of ethics with the purpose of taking the

patient’s interest into account when choosing treatment.

Following [22] it is assumed that the physician cares

about the ethics of treatment2. Thus, the physician cares

about the well-being of his patient, N(q,s), as well as the

profit of his public hospital,



h and private sector profit,



() (1) (,)()

RqB qsqcq



  (4)

where R(q) is the revenue that the public hospital re-

ceives from the government. Again with the exception of

(1 –



)Bq(q,s)q (4) is identical to the public hospital

profit in [16]. The private profit, however, is the same as

that in [16]:

(,) ()

Bqss cs





(5)

where c’(i) > 0, c’’(i) > 0 (i = q, s),3 and



h and



p have

unique maximum in q and s respectively, the marginal

profits are:

(1)(,) or < 0

ssq

Bqsq



 (6)

'() (1)(,)

(,)'() or > 0

RqB qs

qBqscq







(7)

where R’(q) > 0.

(,) 0

qsq

Bqss







 (8)

(,)(,)'() 0

ss ss

BqssB qscs







 (9)

The physician’s utility function is U(



p, N), with

UN > 0, 0



, 0



, UNN < 0, 0





, and





. The physician chooses q and s to maximize

his utility. The first order conditions are:

qqNq

UU UN





 (10)

ssNs

UU UN







(11)

1Note that Nq can be negative when services are complements and sis

very large. This case is not examined.

2I do not use the assumption in [16] that patients do not search among

alternative physicians but because physicians care about the welfare o

atients they do not charge a monopolist price. In the current model, the

hysician’s care for the patient represents his care for ethics of treat-

ment .This prevents the physician from charging monopolist price. As

shown in (11) the physician would charge a monopolist price if UN =

0. This is consistent with [23], where the physician is constrained by

atient information. In [23] even though, patients cannot evaluate the

marginal benefit from treatment from a given physician they can evalu-

ate the absolute utility upon treatment. Patients can observe the utility

of other patients after treatment. If patients of one physician end up

with a lower utility on average than others then the physician looses

atients.

3In order to avoid the implied requirement that the marginal cost in the

two sectors be equal, I do not assume constant marginal costs for the

rovision of treatment as in [16].

E. Amporfu / HEALTH 2 (2010) 1110-1119

1112

UUN





 (12)

By substituting (12) into (10) and rearranging pro-

duces:

qqsq

MRS NN















(13)

where MRS N



h = UN /U



h > 0. Eq.13 defines a locus {q,s}

that maximize the physician’s utility. With the exception

of the second term on the RHS, (13) is identical to what

[16] obtained. This second term appears here because the

inclusion of a fee in the public sector makes



h, which is

zero in [16], positive, negative or zero, in the current

model, depending on whether q and s are complements,

substitutes, or unrelated respectively. The terms in bra-

ckets are the magnitudes of the slopes of the private

sector iso-profit (



p) and the patient’s indifference

curve (Nq/Ns).

The welfare function, W(q, s) = B(q, s) – c(q) – c(s), is

used to find efficiency under the various reimbursement

schemes. It is assumed that the welfare function is con-

cave and has unique maxima in q and s. Efficiency re-

quires that the following first order conditions for the

maximization of the welfare function are satisfied:

(,) 0

Bqs c (14)

(,) 0

Bqsc (15)

The three physician reimbursement rules in the public

sector and their effect on equilibrium q and s provided

by the physician are now examined. The reimbursement

rules are full-cost reimbursement, prospective payment,

and cost sharing. The patients in [16] did not have to pay

fees in the public sector and so the full-cost reimburse-

ment involved the government providing enough reve-

nue to cover the cost of production. In the current model,

however, the full-cost reimbursement, involves the pub-

lic hospital receiving R(q) from the government to cover

part of the cost not covered by the user fee. Prospective

payment involves the government giving a fixed amount

of revenue, G, to the hospital regardless of the total cost

of production and of the total fees collected. The

cost-sharing rule is a combination of prospective pay-

ment and cost reimbursement. The government gives

fixed revenue, G, and then pays for a fraction of the cost

of production. Under each rule, (13) is used to examine

how the q and s chosen by the physician affect optimal-

ity from the points of view of the patient and society.

The patient’s indifference curves are downward slop-

ing for both complements and substitutes5. This is be-

cause the slope of the indifference curve is –Nq/Ns and

with Nq > 0, Ns > 0 regardless of the relationship be-

tween q and s. Figures 1(a) and 1(b) shows the iso-profits

for the physician’s private profit. The slope of the

iso-profit (-



p) depends on whether treatments are

substitutes or complements. As shown in (8) and (9),



is positive when treatments are complements and nega-

tive when they are substitutes;



p is positive when s is

very small and becomes negative as it is increases. Thus,

when treatments are substitutes, the iso-profit slopes up-

wards when s is small and downwards when s increases

with profit increasing as q falls. The opposite occurs

when treatments are complements. When treatments are

complements the iso-profit slopes downwards when s is

small and upwards as s increases with profit increasing

in q. These are shown in Figure 1.

3. RESULTS AND DISCUSSION

3.1. Full Cost Reimbursement

Under this rule, the government provides the revenue

required to cover part of the cost of production that the

user fee could not cover. Hence



h = 0 and so



h = 0; i.e.,

the physician chooses q to maximize public hospital

profit. Equation (13), then, becomes:

qqsq

spp

MRS NN













(16)

Rearranging (16) produces:

Ns s

MRS NN

RS NN







 (17)

Notice that when



h = 0, the two slopes in brackets in

(16) are equal, and the results are identical to those in

[16], i.e., the physician chooses q and s to equate the

slopes of the iso-profit and the patient’s indifference

curves. Such result is possible in the current model if q

and s are unrelated, i.e., if Bqs(q, s) = 0 implying that



= 0. It is of interest to examine the optimality of equilib-

rium q and s from the patient’s and society’s perspective

when services are complements,



h > 0, and when they

are substitutes,



h < 0. Eq.17 shows that in equilibrium

the difference between the slopes of the iso-

profit and indifference curves depends on the sign of



When treatments are substitutes,



h < 0, the iso-profit

curve is steeper than the patient’s indifference curve. The

resulting q lowers the patient’s welfare compared to [15]

as well as [16]. Reference [15] showed that in the ab-

4where ch and cp represent the marginal cost of q and s respectively.

5Because the marginal net benefits in this model differ from those in

Rickman and McGuire the patient’s indifference curves in this model

are also different. The indifference curves in Rickman and McGuire

slope downward when q and s are substitutes and upward when they are

complements (U-shaped indifference curves). In Rickman and McGuire

s >0, whether q and s are substitutes or complements; Nq> 0 when

they are substitutes and

< 0 when they are complements.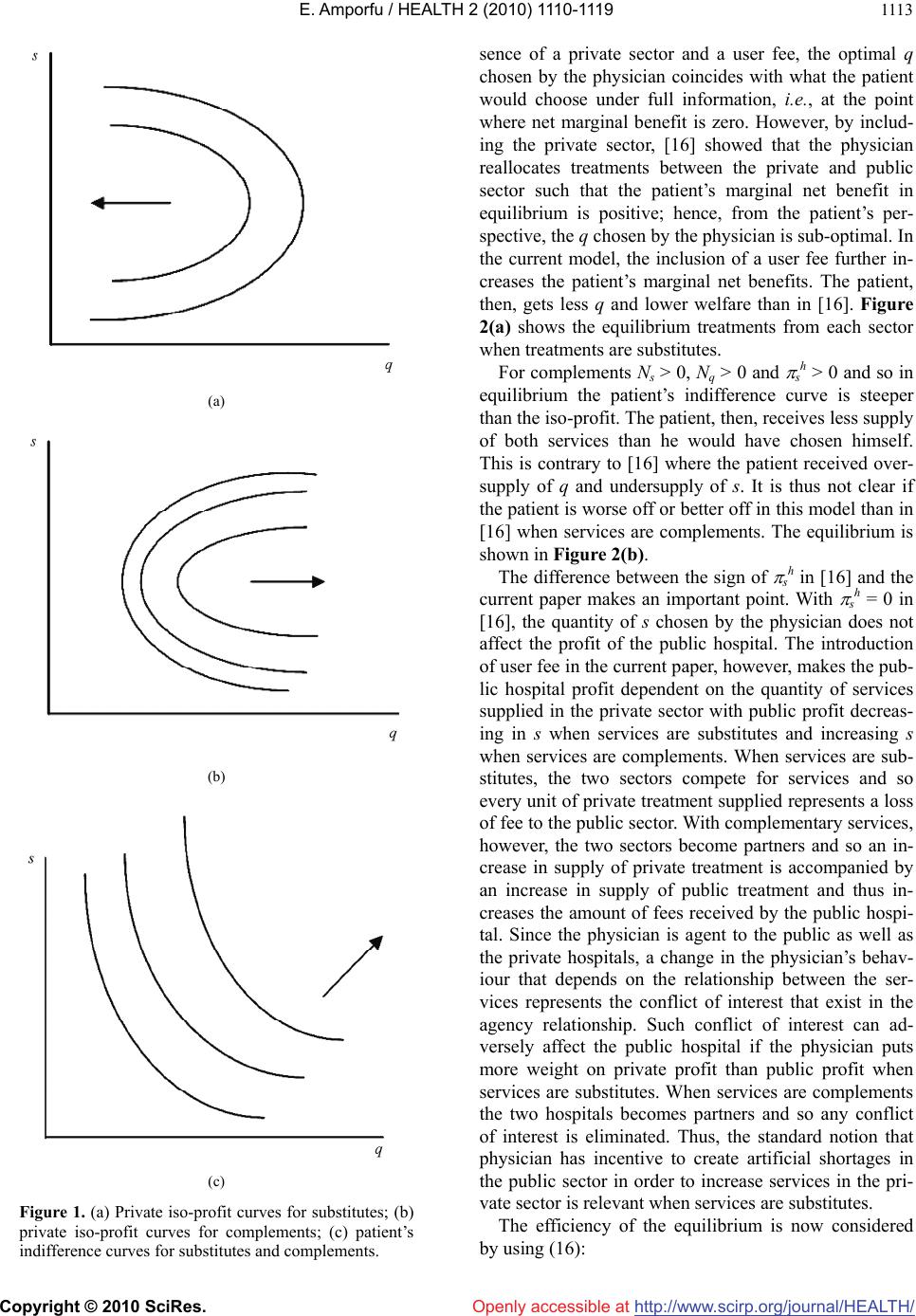

E. Amporfu / HEALTH 2 (2010) 1110-1119

1113

(a)

(b)

(c)

Figure 1. (a) Private iso-profit curves for substitutes; (b)

private iso-profit curves for complements; (c) patient’s

indifference curves for substitutes and complements.

sence of a private sector and a user fee, the optimal q

chosen by the physician coincides with what the patient

would choose under full information, i.e., at the point

where net marginal benefit is zero. However, by includ-

ing the private sector, [16] showed that the physician

reallocates treatments between the private and public

sector such that the patient’s marginal net benefit in

equilibrium is positive; hence, from the patient’s per-

spective, the q chosen by the physician is sub-optimal. In

the current model, the inclusion of a user fee further in-

creases the patient’s marginal net benefits. The patient,

then, gets less q and lower welfare than in [16]. Figure

2(a) shows the equilibrium treatments from each sector

when treatments are substitutes.

For complements Ns > 0, Nq > 0 and



h > 0 and so in

equilibrium the patient’s indifference curve is steeper

than the iso-profit. The patient, then, receives less supply

of both services than he would have chosen himself.

This is contrary to [16] where the patient received over-

supply of q and undersupply of s. It is thus not clear if

the patient is worse off or better off in this model than in

[16] when services are complements. The equilibrium is

shown in Figure 2(b).

The difference between the sign of



h in [16] and the

current paper makes an important point. With



h = 0 in

[16], the quantity of s chosen by the physician does not

affect the profit of the public hospital. The introduction

of user fee in the current paper, however, makes the pub-

lic hospital profit dependent on the quantity of services

supplied in the private sector with public profit decreas-

ing in s when services are substitutes and increasing s

when services are complements. When services are sub-

stitutes, the two sectors compete for services and so

every unit of private treatment supplied represents a loss

of fee to the public sector. With complementary services,

however, the two sectors become partners and so an in-

crease in supply of private treatment is accompanied by

an increase in supply of public treatment and thus in-

creases the amount of fees received by the public hospi-

tal. Since the physician is agent to the public as well as

the private hospitals, a change in the physician’s behav-

iour that depends on the relationship between the ser-

vices represents the conflict of interest that exist in the

agency relationship. Such conflict of interest can ad-

versely affect the public hospital if the physician puts

more weight on private profit than public profit when

services are substitutes. When services are complements

the two hospitals becomes partners and so any conflict

of interest is eliminated. Thus, the standard notion that

physician has incentive to create artificial shortages in

the public sector in order to increase services in the pri-

vate sector is relevant when services are substitutes.

The efficiency of the equilibrium is now considered

by using (16):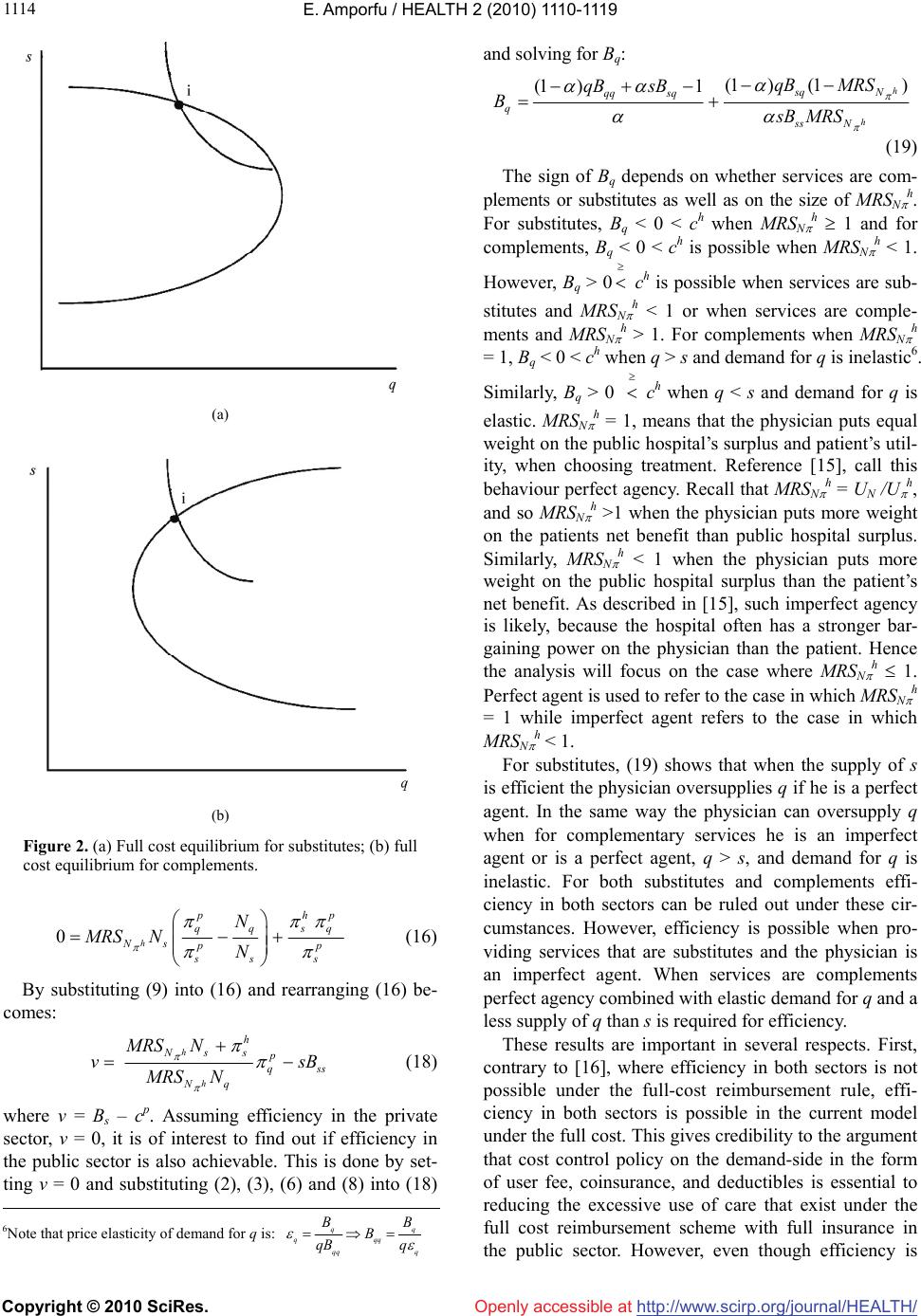

E. Amporfu / HEALTH 2 (2010) 1110-1119

1114

(a)

(b)

Figure 2. (a) Full cost equilibrium for substitutes; (b) full

cost equilibrium for complements.

qqsq

MRS NN













(16)

By substituting (9) into (16) and rearranging (16) be-

comes:

Ns s

qss

MRS N

vsB

MRS N









(18)

where v = Bs – cp. Assuming efficiency in the private

sector, v = 0, it is of interest to find out if efficiency in

the public sector is also achievable. This is done by set-

ting v = 0 and substituting (2), (3), (6) and (8) into (18)

and solving for Bq:

(1 )(1)

(1 )1h

sq N

qq sq

ss N

qB MRS

qB sB

BsB MRS













(19)

The sign of Bq depends on whether services are com-

plements or substitutes as well as on the size of MRS N



For substitutes, Bq < 0 < ch when MRSN



h  1 and for

complements, Bq < 0 < ch is possible when MRSN



h < 1.

However, Bq > 0





ch is possible when services are sub-

stitutes and MRSN



h < 1 or when services are comple-

ments and MRSN



h > 1. For complements when MRSN



= 1, Bq < 0 < ch when q > s and demand for q is inelastic6.

Similarly, Bq > 0





ch when q < s and demand for q is

elastic. MRSN



h = 1, means that the physician puts equal

weight on the public hospital’s surplus and patient’s util-

ity, when choosing treatment. Reference [15], call this

behaviour perfect agency. Recall that MRSN



h = UN /U



and so MRSN



h >1 when the physician puts more weight

on the patients net benefit than public hospital surplus.

Similarly, MRSN



h < 1 when the physician puts more

weight on the public hospital surplus than the patient’s

net benefit. As described in [15], such imperfect agency

is likely, because the hospital often has a stronger bar-

gaining power on the physician than the patient. Hence

the analysis will focus on the case where MRSN



h  1.

Perfect agent is used to refer to the case in which MRSN



= 1 while imperfect agent refers to the case in which

MRSN



h < 1.

For substitutes, (19) shows that when the supply of s

is efficient the physician oversupplies q if he is a perfect

agent. In the same way the physician can oversupply q

when for complementary services he is an imperfect

agent or is a perfect agent, q > s, and demand for q is

inelastic. For both substitutes and complements effi-

ciency in both sectors can be ruled out under these cir-

cumstances. However, efficiency is possible when pro-

viding services that are substitutes and the physician is

an imperfect agent. When services are complements

perfect agency combined with elastic demand for q and a

less supply of q than s is required for efficiency.

These results are important in several respects. First,

contrary to [16], where efficiency in both sectors is not

possible under the full-cost reimbursement rule, effi-

ciency in both sectors is possible in the current model

under the full cost. This gives credibility to the argument

that cost control policy on the demand-side in the form

of user fee, coinsurance, and deductibles is essential to

reducing the excessive use of care that exist under the

full cost reimbursement scheme with full insurance in

the public sector. However, even though efficiency is

6Note that price elasticity of demand for q is: qq

qqq

qq q

qB q





E. Amporfu / HEALTH 2 (2010) 1110-1119

1115

possible it is achieved at the expense of the patient’s

welfare. This is not surprising because the user fee forces

the patient rather than the provider to internalize any

externality that existed in the market. As already ex-

plained, the user fee reduces the equilibrium supply of

services and this makes the patient worse off. Secondly,

imperfect agency is required for efficiency. Unlike [16]

as well as [15] where the agency role is not able to

achieve efficiency under full cost reimbursement, im-

perfect agency is crucial for efficiency in the current

model. In [16], efficiency in the private sector is only

achievable at the expense of oversupply of services in

the public sector. The possibility of efficiency in the

current model then implies that when services are sub-

stitutes, the introduction of user fee in the public sector

constraints the physician from oversupplying services to

the extent of supplying the amount that maximizes social

surplus as long as the physician is an imperfect agent.

The user fee then transfers any cost caused by the im-

perfection of the agency unto the patient. Thirdly, the

elasticity of demand for public service is important for

efficiency when services are complements. For comple-

mentary services, the user fee maximizes social surplus

by reducing oversupply as long as the physician is a

perfect agent when demand for q is elastic and the equi-

librium supply of q is less than s. When services are

complements and there are no close substitutes the re-

sulting inelastic demand for q makes even perfect agen-

cy unable to achieve efficiency in the public sector. This

weakens the ability of the user fee to achieve the effi-

ciency and so weakens the argument for cost control on

the demand side when services are complements.

The results are also contrary to what [24] and [25]

found in comparing full cost reimbursement with pro-

spective payment. They show that cost-reimbursement

like fee-for-service is characterized by oversupply be-

cause it does not provide the provider incentive to

economize on the quantity of services. Like [15] they did

not have user fee in their models.

3.2. Prospective Payment

Under this rule, the government gives a fixed amount of

revenue, G, to the public hospital regardless of cost of

the production and revenue collected from user fee. Thus,

(4) becomes:

(1)( , )( )

GBqsqcq



 (20)

The resulting marginal profit with respect to q is neg-

ative:

(1)( , )(1)(, )'( )0

qq qq

BqsqB qscq

 



  (21)

Eq.13, then, becomes:

(1)( , )(1)(, )'( )

qqq

php

qqsq

BqsqB qscq

MRS NN









 









(22)

Rearrange to get:

'()(1)(,)(1)(,)

qq q

Nss

Ns s

cqqBqsB qs

MRS N

MRS NN









 





(23)

With the exception of the first term on the left hand

side (LHS), (23) is identical to (13). This (positive) term

determines the difference between the equilibrium q and

s under the full-cost payment scheme and the prospec-

tive payment scheme. Again, the relationship between

the slopes of the indifference curve and the iso-profit

depends on whether services are substitutes or comple-

ments. For substitutes, the coefficient of the iso-profit’s

slope is less than one. Thus, (23) shows that, under the

prospective payment scheme, the equilibrium q and s

occurs at a point where the iso-profit is steeper than the

indifference curve but not as steep as under the full-cost

scheme. This is shown in Figure 3(a) as (ii) which has

less q and more s than the full cost equilibrium7. This

result is equivalent to [16]. When services are comple-

ments, the coefficient of the iso-profit’s slope in (23) is

greater than one implying that in equilibrium the iso-

profit is flatter than the indifference curve but not as flat

as under the full cost. As shown in Figure 3(b) as ii, the

equilibrium q and s are both less than those of the full

cost and for a given level of private profit, the patient

ends up on a lower indifference curve than the full cost

equilibrium. This again is consistent with the results of

[16].

To determine the efficiency of this equilibrium (2), (3),

(6), (8), and (9) are substituted into (23) set Bs = cp (i.e.,

efficiency in the private sector), and rearranged to pro-

duce:

(1)(1 )

sqqq ss

BBB

kMRS BqB























(24)

where k = Bq – ch = 0 is required for efficiency in the

public sector. Eq.24 shows that k = 0 when MRSN



h = 1.

However, k > 0 when MRSN



h < 1 and k < 0 when

MRSN



h > 18. Efficiency in the public sector depends

7Note Note that a fall in q causes an increase in private profit when

services are substitutes. By using the positive sloped portion of the

iso-profit it is possible to obtain similar results if the private iso-profit

curve is allowed to shift to the left and indifference curve remains un-

changed.

8The underlying assumption is that

sqssqq

BBB

, i.e., the q and s

maximize patient benefit if cost were zero, making the term in the

uare bracket

ositive.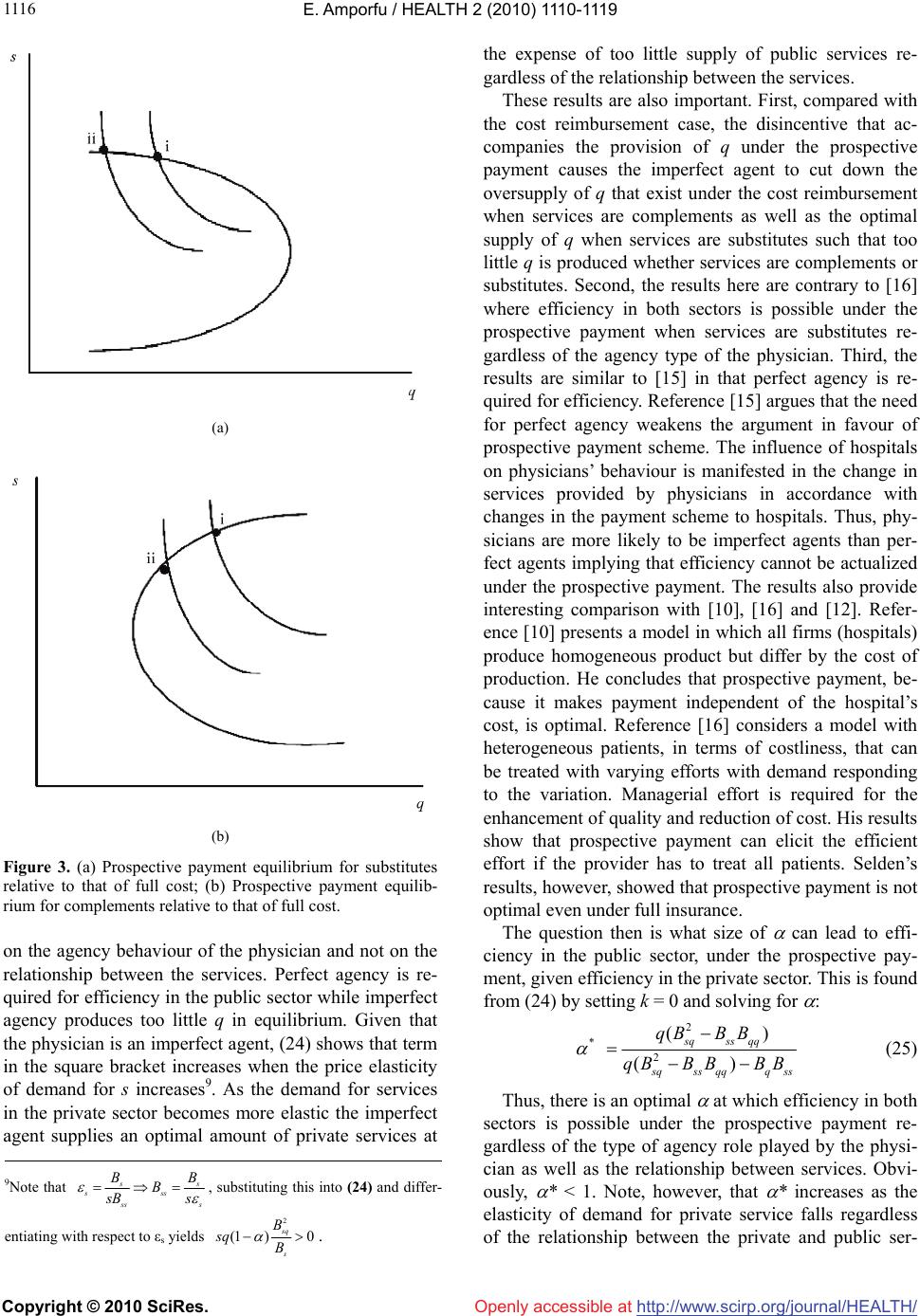

E. Amporfu / HEALTH 2 (2010) 1110-1119

1116

(a)

(b)

Figure 3. (a) Prospective payment equilibrium for substitutes

relative to that of full cost; (b) Prospective payment equilib-

rium for complements relative to that of full cost.

on the agency behaviour of the physician and not on the

relationship between the services. Perfect agency is re-

quired for efficiency in the public sector while imperfect

agency produces too little q in equilibrium. Given that

the physician is an imperfect agent, (24) shows that term

in the square bracket increases when the price elasticity

of demand for s increases9. As the demand for services

in the private sector becomes more elastic the imperfect

agent supplies an optimal amount of private services at

the expense of too little supply of public services re-

gardless of the relationship between the services.

These results are also important. First, compared with

the cost reimbursement case, the disincentive that ac-

companies the provision of q under the prospective

payment causes the imperfect agent to cut down the

oversupply of q that exist under the cost reimbursement

when services are complements as well as the optimal

supply of q when services are substitutes such that too

little q is produced whether services are complements or

substitutes. Second, the results here are contrary to [16]

where efficiency in both sectors is possible under the

prospective payment when services are substitutes re-

gardless of the agency type of the physician. Third, the

results are similar to [15] in that perfect agency is re-

quired for efficiency. Reference [15] argues that the need

for perfect agency weakens the argument in favour of

prospective payment scheme. The influence of hospitals

on physicians’ behaviour is manifested in the change in

services provided by physicians in accordance with

changes in the payment scheme to hospitals. Thus, phy-

sicians are more likely to be imperfect agents than per-

fect agents implying that efficiency cannot be actualized

under the prospective payment. The results also provide

interesting comparison with [10], [16] and [12]. Refer-

ence [10] presents a model in which all firms (hospitals)

produce homogeneous product but differ by the cost of

production. He concludes that prospective payment, be-

cause it makes payment independent of the hospital’s

cost, is optimal. Reference [16] considers a model with

heterogeneous patients, in terms of costliness, that can

be treated with varying efforts with demand responding

to the variation. Managerial effort is required for the

enhancement of quality and reduction of cost. His results

show that prospective payment can elicit the efficient

effort if the provider has to treat all patients. Selden’s

results, however, showed that prospective payment is not

optimal even under full insurance.

The question then is what size of



can lead to effi-

ciency in the public sector, under the prospective pay-

ment, given efficiency in the private sector. This is found

from (24) by setting k = 0 and solving for



()

sqss qq

qssqqqss

qBBB

qBBBBB







(25)

Thus, there is an optimal



at which efficiency in both

sectors is possible under the prospective payment re-

gardless of the type of agency role played by the physi-

cian as well as the relationship between services. Obvi-

ously,



* < 1. Note, however, that



* increases as the

elasticity of demand for private service falls regardless

of the relationship between the private and public ser-

9Note that

sss



, substituting this into (24) and differ-

entiating with respect to εs yields

(1 )0

sq B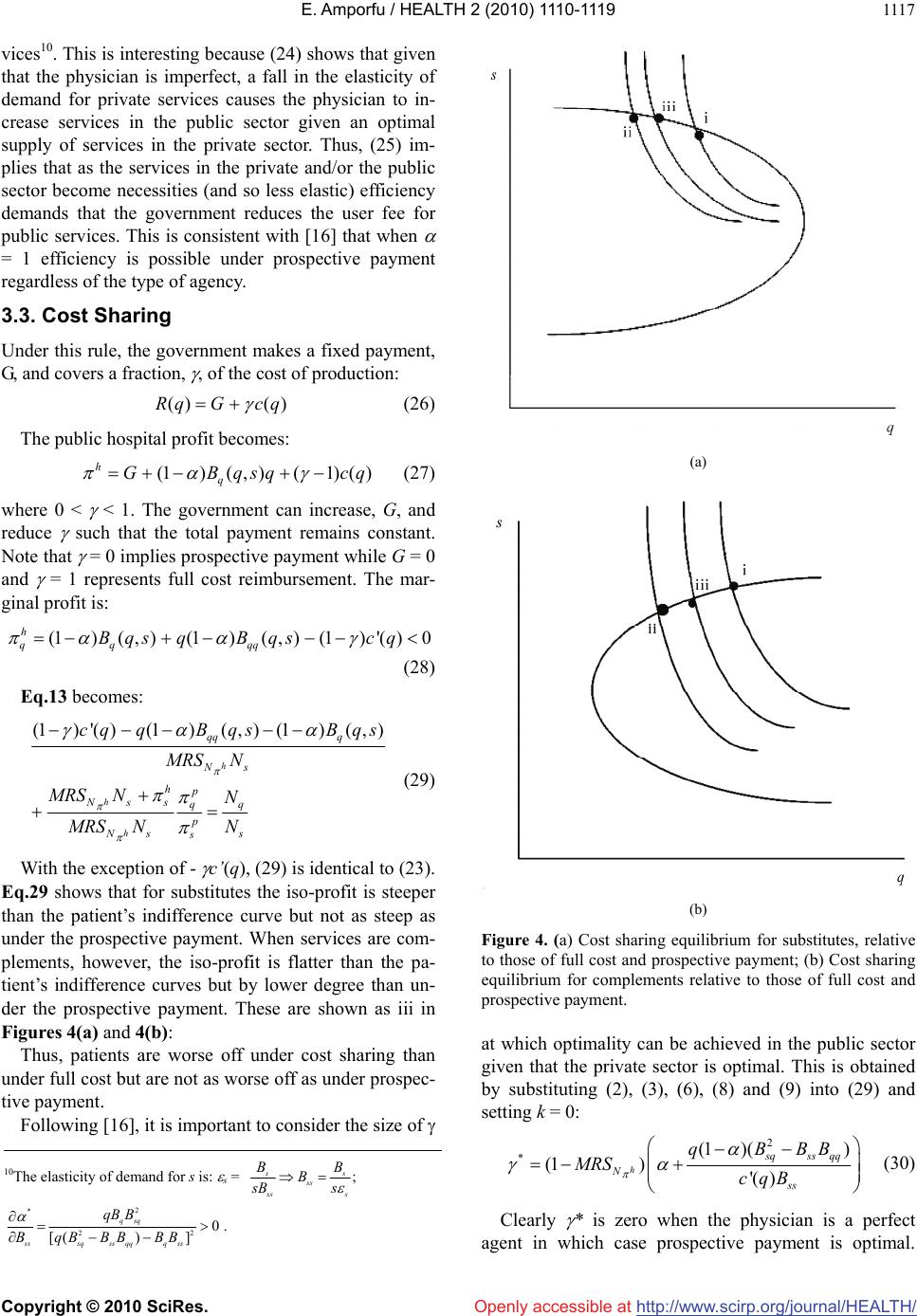





.

E. Amporfu / HEALTH 2 (2010) 1110-1119

1117

vices10. This is interesting because (24) shows that given

that the physician is imperfect, a fall in the elasticity of

demand for private services causes the physician to in-

crease services in the public sector given an optimal

supply of services in the private sector. Thus, (25) im-

plies that as the services in the private and/or the public

sector become necessities (and so less elastic) efficiency

demands that the government reduces the user fee for

public services. This is consistent with [16] that when



= 1 efficiency is possible under prospective payment

regardless of the type of agency.

3.3. Cost Sharing

Under this rule, the government makes a fixed payment,

G, and covers a fraction,



, of the cost of production:

() ()Rq Gcq



 (26)

The public hospital profit becomes:

(1)(,)(1) ()

GBqsqcq

 

 (27)

where 0 <



< 1. The government can increase, G, and

reduce



such that the total payment remains constant.

Note that



= 0 implies prospective payment while G = 0

and



= 1 represents full cost reimbursement. The mar-

ginal profit is:

(1)(,)(1)(,) (1)'()0

qq qq

BqsqB qscq

 

 

(28)

Eq.13 becomes:

(1)'()(1)(,)(1)(, )

qq q

Nss

Ns s

cqqBqsB qs

MRS N

MRS NN



 





 





(29)

With the exception of -



c’(q), (29) is identical to (23).

Eq.29 shows that for substitutes the iso-profit is steeper

than the patient’s indifference curve but not as steep as

under the prospective payment. When services are com-

plements, however, the iso-profit is flatter than the pa-

tient’s indifference curves but by lower degree than un-

der the prospective payment. These are shown as iii in

Figures 4(a) and 4(b):

Thus, patients are worse off under cost sharing than

under full cost but are not as worse off as under prospec-

tive payment.

Following [16], it is important to consider the size of 

(a)

(b)

Figure 4. (a) Cost sharing equilibrium for substitutes, relative

to those of full cost and prospective payment; (b) Cost sharing

equilibrium for complements relative to those of full cost and

prospective payment.

at which optimality can be achieved in the public sector

given that the private sector is optimal. This is obtained

by substituting (2), (3), (6), (8) and (9) into (29) and

setting k = 0:

*(1 )()

(1 )'( )

sqss qq

qBBB

MRS cqB











 





(30)

Clearly



* is zero when the physician is a perfect

agent in which case prospective payment is optimal.

10The elasticity of demand for s is:



s = ;

ss s





[() ]

qsq

sssqssqqqss

qB B

BqBBBBB





.

E. Amporfu / HEALTH 2 (2010) 1110-1119

1118

However,



* cannot be one11, i.e., cost sharing can be

used to ensure optimal supply of services. It is optimal

for the government to use a combination of fixed fee and

incentive payment to reward the hospital because this

forces providers to internalize the externalities that leads

to undersupply under prospective payment (Selden 1990;

[15] 1986) and oversupply under full cost reimburse-

ment. Equation (30) shows that the size of * does not

depend on the relationship between services i.e., it is

optimal for the government to pass on the same fraction

of cost for substitutes and complements. In [16] effi-

ciency in both sectors requires that



* = (1- MRSN



h).

This is identical to what [15] recommend for efficiency

in the public sector when there is no private sector. Thus,

in Rickman & McGuire, the optimal fraction of cost that

is passed on to providers does not depend on the rela-

tionship between the services.

The term in the square bracket in (30) represents the

effect of the user fee in the public sector on the size of *.

Note that when



= 1 (30) becomes



* = (1 - MRSN



h),

which is the same as in [16] as well as in [15]. With the

term in the square bracket less than one,



* in the current

model is less than that in [16]. Thus, when there is a user

fee in the public sector, efficiency requires that less rev-

enue be retained to induce optimal provision of public

services for both substitutes and complements than when

there is no user fee. Note that the second term in the

square bracket increases in the elasticity of services in

both sectors12. The



* here is subject to the same setback

as in [15] as well as [16] in that information on an un-

observable variable, the physician’s utility, is required.

However, the effect of elasticity on



* in the current

model reduces the dependence on information on the

physician’s utility. The presence of the positive term in

the bracket which depends on elasticity reduces the

range within which



* falls. Thus, under the user fee

system in the public sector in a two-tier system, cost

sharing can ensure efficiency in both the public and pri-

vate sector regardless of the relationship between ser-

vices in the two sectors. This is also intuitive. Compared

to the full cost reimbursement system (where G = 0 and



= 1), the fall in marginal revenue resulting from letting



* < 1 gives the imperfect agent the incentive to reduce

the oversupply of complementary services while main-

taining supply of substitutes at the efficient level. For

comparison with prospective payment, (where G > 0 and



= 0) the increase in marginal revenue from setting



* <

1 induces the imperfect agent to increase services.

4. CONCLUSIONS

Many recent health care reforms introduce user fees to

the public sector. This paper examined patient’s welfare

and efficiency under different provider reimbursement

schemes in the public sector in a mixed health care sys-

tem where the patient bears cost for treatment in both the

public and private sectors. The paper extended previous

studies by introducing user fee in the public sector. The

provider reimbursement schemes examined are full-cost

reimbursement, prospective payment and cost sharing.

The results show that efficiency is possible under the

full cost reimbursement scheme if the physician trades

off public hospital surplus for patient net benefit when

services are substitutes and trading off patient’s net ben-

efit for public hospital surplus or being a perfect agent

when services are complements. This is contrary to the

results in previous studies where there is oversupply of

services in equilibrium in the public sector under the full

cost reimbursement. Under the prospective payment

efficiency is only possible when the fraction of cost not

covered by the user fee is at its optimal level. Similarly,

under the cost-sharing scheme, efficiency occurs only if

the fraction of cost that the government passes on to

providers is at its optimal level. This is similar to the

results in previous studies; however, the optimal fraction

in this paper is less than that in previous studies. Of the

three reimbursements schemes, the patient is worst off

under the prospective payment and has the highest utility

under the full-cost reimbursement. In general the intro-

duction of user fee in the public sector makes the patient

worse off when services are substitutes. However, it is

not clear whether the user fee makes patients worse off

or better off when services are complements.

REFERENCES

[1] Besley, T. and Gouveia, B. (1994) Alternative systems of

health care provision. Economic Policy, 203-258.

[2] Zweifel, P. and Breyer, F. (1997) Health economics. Ox-

ford University Press, Oxford.

[3] Petretto, A. (1999) Optimal social health insurance with

supplementary private insurance. Journal of Health

Economics, 18(1), 727-745.

[4] Holmer, M. (1984) Tax policy and the demand for health

insurance. Journal of Health Economics, 3, 203-221.

[5] Zuckerman, S. (1987) Commercial insurers and all-payer

regulation evidence on hospitals’ responses to financial

need. Journal of Health Economics, 6(3), 165-187.

[6] Gravelle, H. (1999) Capitation contracts: access and

quality. Journal of Health Economics, 18(1), 315-340.

[7] Chernick, H.A., Holmer, M.R. and Weinberg, D.H. (1987)

Tax policy toward health insurance and the demand for

medical services. Journal of Health Economics, 61-25.

[8] Selden, T.M. (1999) Premium subsidies for health insur-

11This is based on the assumption that

()

'( )

sqssqq

qB BB

cqB





(1 )0

ss ss





 

and





(1) 0

'( )

Bcq





 



E. Amporfu / HEALTH 2 (2010) 1110-1119

1119

ance: Excessive coverage vs. adverse selection. Journal

of Health Economics, 18, 709-725.

[9] Pope, G. (1990) Hospital nonprice competition and med-

icare reimbursement policy. Journal of Health Econom-

ics, 8(2), 147-172

[10] Schleifer, A. (1985) A theory of yardstick competition.

The RAND Journal of Economics, 16(3), 319-327.

[11] Keeler, E.B. (1990) What proportion of hospital cost

differences is justifiable? Journal of Health Economics

9(3), 359-365.

[12] Selden, T.M. (1990) A model of capitation. Journal of

Health Economics, 9, 397-409.

[13] Ellis, R.P. and McGuire, T.G., (1993) Supply-side and

demand-side cost sharing in health care. Journal of Eco-

nomic Perspectives, 7(4), 135-151.

[14] Ma, C. A. (1994) Health care payment systems: Cost and

quality incentives. Journal of Economics & Management

Strategy, 3(1), 93-112.

[15] Ellis, R.P., McGuire, T.G., (1986) Provider behavior un-

der prospective reimbursement, cost sharing and supply,

Journal of Health Economics, 5(1), 129-151.

[16] McGuire, A. and Rickman, N. (1999) Regulating pro-

vider’s reimbursement in a mixed market for health care.

Scottish Journal of Political Economy, 46(1), 53-71.

[17] Leger, P.T. (2000) Quality control mechanisms under

capitation payment for medical services. Canadian

Journal of Economics, 33(2), 565-586.

[18] Leonard, K.L. (1998) Institutional structure of health

care in rural cameroun: Structural estimation of produc-

tion in teams with unobservable effort. Discussion Paper

Series N, 9798-9816.

[19] Bloom, G. and Segall, M. (1994) Expenditure and fi-

nancing of the health sector inkenya, abridged report of a

study performed for the ministry of health and the world

bank. IDS Commissioned Study, 9.

[20] Lavy, V. and Germain, J. (1995) Tradeoffs in cost, quality

and accessibility in utilization of health facilities: In-

sights from Ghana. In: Shaw, R.P. and Ainsworth, Eds.,

Financing Health Services through User Fees and In-

surance Case Studies from Sub-Saharan Africa, World

Bank Discussion Papers, 103-121.

[21] Petretto, A. (1999) Optimal social health insurance with

supplementary private insurance. Journal of Health

Economics, 18(3), 727-745.

[22] Evans, R.G. (1974) Supplier-induced demand: Some

empirical evidence and implications. In: Perlman, M.,

Ed., The Economics of Health and Medical Care, Wiley,

New York.

[23] Farley, P. (1986) Theories of the price and quantity of

physician services: A synthesis and critique. Journal of

Health Economics, 5(1), 315-333.

[24] Laffont, J.J. and Tirole, J. (1986) Optimal payment sys-

tems for health services. Journal of Health Economics,

9(4), 375-396.

[25] Laffont, J.-J. and Tirole, J. (1993) A theory of incentives

in procurement and regulation. MIT Press, Cambridge.