The integral equations of Compton Scatter Tomography

doi:10.4236/ojapps.2012.24B013

Paper Menu >>

Journal Menu >>

The integral equations of Compton Scatter Tomography

M. K. Nguyen

Equipes Traitement de l’Information et Systèmes (ETIS)

UMR CNRS 8051/ ENSEA / University of Cergy-Pontoise

F-95014 Cergy-Pontoise, France

mai.nguyen-verger@u-cergy.fr

T. T. Truong

Laboratoire de Physique Théorique et Modélisation (LPTM)

UMR CNRS 8089 / University of Cergy-Pontoise

F-95302 Cergy-Pontoise

truong@u-cergy.fr

Abstract — Two new Compton Scatter Tomography modalities, which are aimed at imaging hidden structures in bulk

matter for industrial non-destructive control (or testing) and for medical diagnostics are shown to be based on the solutions

of a special class of Chebyshev integral transforms. Besides their remarkable analytic properties, they can be inverted by

existing methods which lend themselves nicely to numerical treatment and provide convergent, stable and fast computation

algorithms. The existence of explicit inversion formulas implies that viable new imaging techniques can be developed, which

may take over the current ones in a near future.

Keywords-Integral transforms; Radon transforms; Compton scatter imaging; Non-destructive testing; Medical imaging.

1. Introduction

Integral equations arise naturally in a wide range of

fields as the proper way to represent a relevant phenomena

to be exploited for applications. Examples are numerous in

wave propagation, transport theory, optics,

electromagnetism, acoustics, etc. Quite often, the

conversion of differential processes into integral processes

turns out to be beneficial in the sense that a solution can be

worked out by integral operator techniques. This is in

particular the case of aerodynamics, in which, for the first

time an integral equation of the first kind appears with a

Chebyshev integral kernel. Ta Li [1], who discovered this

class of integral equations, has also realized that the

equation he had discovered belongs to a much larger family

with a hypergeometric function kernel, including numerous

special cases involving Legendre, Jacobi, Gegenbauer

polynomial kernels. What should be pointed out is the fact

that their inversion, essential for applications, relies on a

very peculiar property of the Gauss hypergeometric

function, which was most elegantly derived by R.

Buschman in [2].

In this paper, we show how the process of measuring

energy flux densities of scattered gamma rays by bulk

matter leads to the reconstruction its structure and

composition without having to dismember it or take it apart.

The image is called tomographic when such operation is

done in a two-dimensional slice, perpendicular to some

main axis of the object. A three-dimensional image can be

then obtained by juxtaposition of a large number of

tomographic images. To this end, in the next section, we

recall the basic principle of Compton Scatter Tomography

and introduce two recently suggested operating modalities.

Section 3 handles the resulting integral transforms by

describing the derivation of their inversion. The paper ends

with a short conclusion and some perspectives for

applications.

2. Compton Scatter Tomography

One of the most efficient way of probing the inner

structure of an objects the use of penetrating radiation (X-

or gamma-rays). Originally one exploits the phenomena of

radiation attenuation inside matter from emission to

detection along a straight line path. This has given rise to

the widely known X-ray scanner, which is nowadays

commonly used in hospitals and in industrial protocols.

However as radiation propagates inside matter, scattering

with distributed electric charges - or Compton effect - is the

main cause for its attenuation. So a smart idea would be to

collect information carried by scattered radiation to try to

image the internal structure of matter. Of course there are

many ways to put this idea to work. One of them is called

Compton Scatter Tomography (or CST).

In CST the object is illuminated by a monochromatic

source of penetrating radiation S, placed at some spatial

position. An energy sensitive detector D will measure the

radiation flux density for a given scattered energy at all

accessible sites outside the object. If the totality of such

measurements allows to reconstruct the electric charge

density inside the object, a corresponding image of this

object is therefore obtained. This is the principle of CST,

when this is done in a plane containing the line SD. As the

object electric charge density is a smooth real-valued

function f(x,y) with compact support in R2, the set of

measurements is required to have two independent variables.

One natural variable is the radiation scattered energy, which

is directly related to the scattering angle Ȧ by the well-

known Compton relation. So when D registers scattered

Open Journal of Applied Sciences

Supplement：2012 world Congress on Engineering and Technology

Cop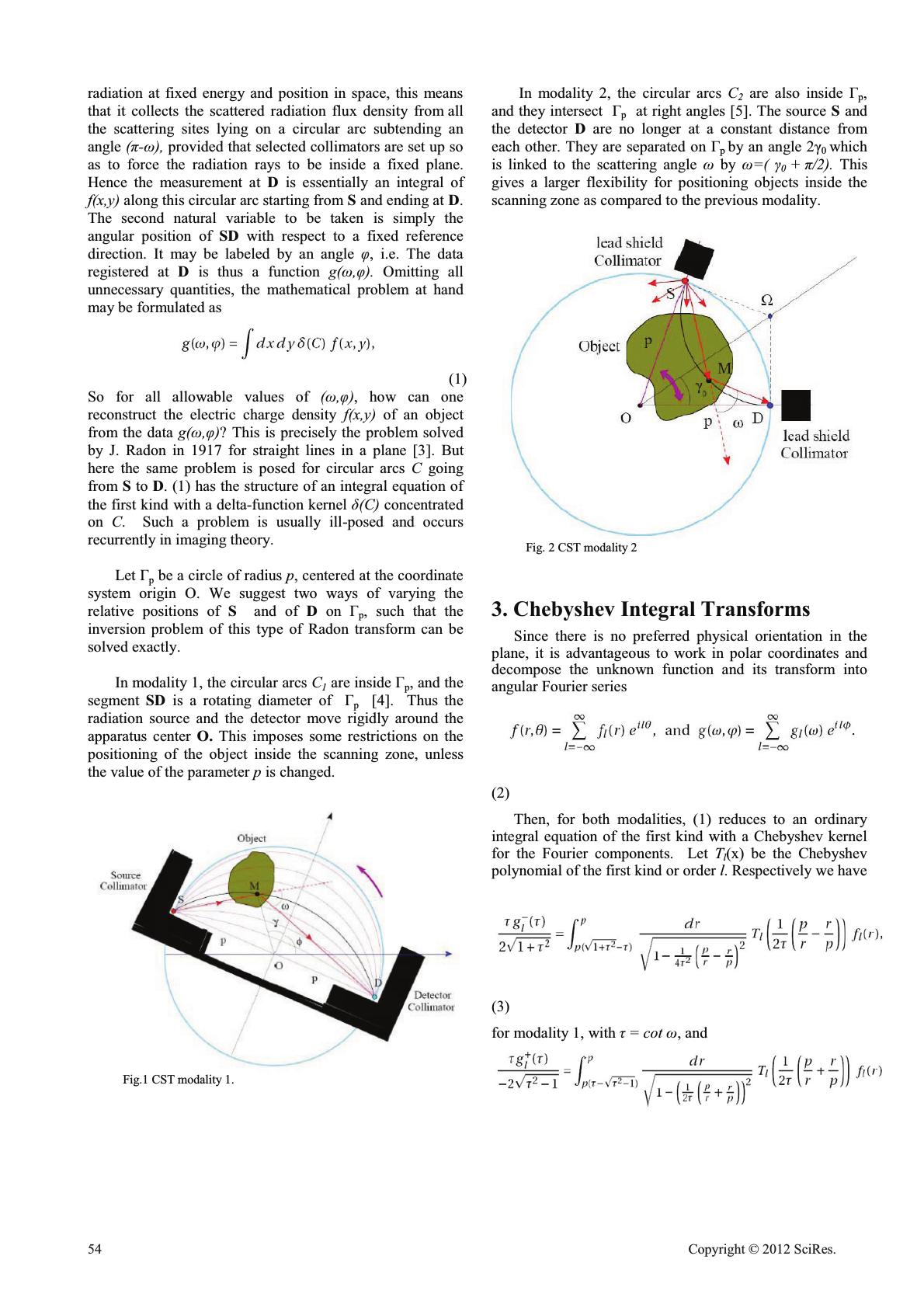

radiation at fixed energy and position in space, this means

that it collects the scattered radiation flux density from all

the scattering sites lying on a circular arc subtending an

angle ʌ-Ȧ provided that selected collimators are set up so

as to force the radiation rays to be inside a fixed plane.

Hence the measurement at Dis essentially an integral of

f(x,y) along this circular arc starting from Sand ending at D.

The second natural variable to be taken is simply the

angular position of SD with respect to a fixed reference

direction. It may be labeled by an angle ĳ, i.e. The data

registered at Dis thus a function JȦĳ Omitting all

unnecessary quantities, the mathematical problem at hand

may be formulated as

(1)

So for all allowable values of Ȧĳ, how can one

reconstruct the electric charge density f(x,y) of an object

from the data JȦĳ? This is precisely the problem solved

by J. Radon in 1917 for straight lines in a plane [3]. But

here the same problem is posed for circular arcs C going

from S to D. (1) has the structure of an integral equation of

the first kind with a delta-function kernel į& concentrated

on C. Such a problem is usually ill-posed and occurs

recurrently in imaging theory.

p be a circle of radius p, centered at the coordinate

system origin O. We suggest two ways of varying the

relative positions of S and of D 

p, such that the

inversion problem of this type of Radon transform can be

solved exactly.

In modality 1, the circular arcs C1 p, and the

segment SD       p [4]. Thus the

radiation source and the detector move rigidly around the

apparatus center O. This imposes some restrictions on the

positioning of the object inside the scanning zone, unless

the value of the parameter pis changed.

Fig.1 CST modality 1.

In modality 2, the circular arcs C2 p,

 p at right angles [5]. The source Sand

the detector D are no longer at a constant distance from

each other. They are sepap    0 which

is linked to the scattering angle Ȧ by Ȧ Ȗ0+ʌ This

gives a larger flexibility for positioning objects inside the

scanning zone as compared to the previous modality.

Fig. 2 CST modality 2

3. Chebyshev Integral Transforms

Since there is no preferred physical orientation in the

plane, it is advantageous to work in polar coordinates and

decompose the unknown function and its transform into

angular Fourier series

(2)

Then, for both modalities, (1) reduces to an ordinary

integral equation of the first kind with a Chebyshev kernel

for the Fourier components. Let Tl(x) be the Chebyshev

polynomial of the first kind or order l. Respectively we have

(3)

for modality 1, with Ĳ FRWȦ, and

54 Cop

(4)

for modality 2, with Ĳ FRVHFȦ.

To get their inverses we bring these equations to the

form of the Chebyshev transform of A. M. Cormack [6]

through the following change of variables

(5)

and functions

(6)

for modality 1,

(7)

for modality 2.

Then (3,4) are rewritten as

(8)

which has the form of the Chebyshev transform in [6]. Here



(9)

the inversion of (8) can be achieved by a clever application

of (9). The resulting explicit inversion formula reads

(10)

However (10), as such is still improper for setting up a

computational algorithm. It needs to be regularized as

indicated in [7]. This is so because the transforms GlĲhave

to verify the co-called consistency conditions, which consist

of the vanishing of a finite number of moments of the data.

Then the proper form of (10) is made up of two parts

(11)

where Ul-1(x) is the Chebyshev polynomial of the second

kind and of order (l-1) 

Using the Fourier series

(12)

we reconstruct the functions

(13)

Then (11) may be used to derive a close form for the

inversion formula first for the intermediate functions given

in (12)

(14)

A final form of the inversion formula can be given in terms

of the original functions (IUș the object electric charge

density and JĲĳ the measured radiation flux density on

the detector) by “extracting” IUșand JĲĳ from (13)

Cop

(15)

–integral should be understood as a Cauchy

principal value. The structure of this formula is analogous

to that of the classical Radon transform. Yet it belongs to

two types of Radon transforms on finite circular arcs which

have appeared for the first time in the literature of integral

geometry in the sense of I. M. Gelfand. This is in contrast to

other types of Radon transforms which are defined on

closed (or unbounded open) curves in the plane.

Remark:

In practice a computing algorithm can be set up from

(11) following the approach of Chapman and Carey [9]. It

-integration range into a finite

number of integrals and then making the proper change of

variables so that each term is represented by an exactly

calculated indefinite integral. The derivative of GlĲ is

simply calculated by linear interpolation. Of course the

discretization steps must be optimized to save computing

time. This way of reconstructing the unknown function

turns out to be efficient, consistent with the data and give

satisfactory results [10].

4. Conclusion and Perspectives

The idea of CST goes back to the early 50’s. However

the proposed scanning processes operate either point by

point or line by line. Much later, to improve sensitivity,

wide angle radiation collimators have been introduced. This

has led naturally to the concept of integral measurements

along arcs of circles. But no true inversion method was then

at hand.

In the mid 90’s, a first true CST modality was

introduced by S. J. Norton [8]. It has a fixed source of

radiation and a moving detector running on a straight line

containing the source site. Such a CST scanner is, in

particular appropriate for large objects which can only be

studied from one side (such as a wall or a long metal beam).

Here one may also formulate the problem in terms of

circular harmonic components of the unknown electron

density and end up with an integral equation with a

Chebyshev kernel as shown independently by A. M.

Cormack in [6]. The inversion procedure is thus simpler

because one actually ends up with (14), in which q+or q- is

replaced by q.

Consequently the two CST scanning modalities

proposed here appear to be complementary to Norton’s CST

modality. All three use Compton scattered radiation as

imaging agent to probe the hidden parts of objects of

interest in non-destructive control or in medical diagnostics.

All three are based on the inversion of a Chebyshev integral

transform with different degrees of complexity but share

many common features which usher them into a near future

as tomorrow imaging scanners for medical as well as

industrial applications.

REFERENCES

[1] Ta. Li, “A new class of integral transforms,” Proc.

Amer. Math. Soc., vol. 11, pp. 290–298, 1960.

[2] R.G. Buschman, “Integrals of hypergeometric

functions,” Math. Zeitschr. vol 89, pp. 74-76, 1965.

[3] J. Radon, “Über die Bestimmung von Funktionnen

durch ihre Integralwerte längst gewisser

Mannigfaltigkeiten,” Ber.Verh.Sachs.Akad.Wiss.

Leipzig-Math.-Natur.Kl. vol. 69, pp. 262-277,

1917.

[4] M. K. Nguyen and T. T. Truong, “Inversion of a

new circular arc Radon transform for Compton

scattering tomography,” Inverse Problems vol. 26,

pp. 065005, 2010.

[5] T. T. Truong and M. K. Nguyen, “Radon transforms

on generalized Cormack’s curves and a new

Compton scatter tomography modality,” Inverse

Problems, vol. 27, pp. 125001, 2011.

[6] A. M. Cormack, “The Radon transform on a family

of curves in the plane ,” Proc. Amer. Math. Soc.,

vol. 83, pp. 325-330, October 1981.

[7] A. M. Cormack, “Radon’s problem – Old and new”,

SIAM Proceedings, vol. 14, pp. 33-39, 1984.

[8] S. J; Norton, “Compton scattering tomography,” J.

Appl. Phys., vol. 70, pp. 2007-2015, 1994.

[9] C. H. Chapman and P. W. Carey, “The circular 

harmonic Radon transform,” Inverse Problems, vol.

2, pp. 23-79, 1986.

[10] M. K. Nguyen, C. Faye, G. Rigaud and T. T.

Truong, “A novel technological

imaging process using ionizing radiation

properties,”

Proceedings of the 9th IEEE - RVIF International

Conference on Computing and Communications

Technologies (RIVF 12), Ho-chi-Minh City,

Vietnam, February 2012.

56 Cop