J. Serv. Sci. & Management, 2009, 2: 47-55
Published Online March 2009 in SciRes (www.SciRP.org/journal/jssm)
Polluting Productions and Sustainable Economic
Growth: A Local Stability Analysis
Giovanni Bella
1
1
University of Cagliari, Italy.
Received January 25
th
, 2009; revised February 24
th
, 2009; accepted March 10
th
, 2009.
ABSTRACT
The aim of this paper is to analyze the link between natural capital and economic growth, in a Romer-type economy
characterized by dirty emissions in the production process, and to examine the conditions under which a sustainable
growth, which implies a decreasing level of dirty emissions, might be both feasible and optimal. This work is close to
Aghion-Howitt (1998) with some more general specifications, in particular regarding the structure of preferences and
the technological sector. We also deeply study the transitional dynamics of this economy towards the steady state, and
conclude that a determinate saddle path sustainable equilibrium can be reached even in presence of a long run positive
level of polluting emissions, thanks to a growing level of new home-made inventories, without whom some indetermi-
nacy problems are likely to emerge.
Keywords: local stability, sustainable growth, aghion-howitt model
1. Introduction
It is commonly believed that economic development
might lead to overexploitation of natural resources and
intensification of environmental damages, as for example
the augment of carbon dioxide concentrations in the at-
mosphere due to an increase in transportation services.
On the contrary, empirical evidence suggests that rich
societies seek a less polluted environment to live in, so
they are more willing to invest in abatement technologies
and enforce environmental regulations. The logical conse-
quence must be that economic activity will then also lower
the dirtiness of any existing production technique, which
leaves the door open, for example, to those supporting the
so-called Environmental Kuznets Curve hypothesis [1].
During the last three decades, this counterposition en-
couraged many economists to develop models in which
economic growth depends on the extractive use of the
environment. Inspired by the work of the Club of Rome
and its pessimistic view on the possibility to attain long-
run growth under environmental constraints, these mod-
els tried to depict the conflict between growth and the
environment. Over time the variety of models grew rap-
idly, differing not only with respect to the basic frame-
work adopted but also with respect to the type of envi-
ronmental resource being considered and the problem
analyzed, mainly because each model has specific prop-
erties that become useful for the analysis of either spe-
cific economic concerns.
More recently, research on endogenous growth and the
environment turned more and more attention from one- to
multi-sector models, where knowledge accumulation
might have the potential of lowering environmental
damages through an increase in technological progress
[2,3,4,5]. Likewise, in the seminal paper of Aghion and
Howitt [6], to whom we will be referring to as AH from
now on, it is shown that an unlimited growth can indeed
be sustained when account is taken of both environmental
resource use and innovation in abatement activities [7,8].
Broadly speaking, the properties of endogenous technical
change have been widely investigated in the existing eco-
nomic literature, with some indeterminacy problems and
Hopf bifurcating outcomes being of particular concern [9].
On the contrary, we want to show in this paper that the in-
troduction of the environmental issue can drive the econ-
omy back to a unique, locally stable, equilibrium solution,
where sustainability of consumption is finally reached.
However, this occurs only if a specific sustainability rule,
stating that consumption and natural capital grow at the
same rate, is to be followed, which is also consistent with a
forward looking individual behavior and no myopic state-
ment of the adopted social policy. Therefore, the basic
question we want to address is whether a sustainable path
can be reached even if some dirty production processes,
assumed here to be necessary for any economic activity are
adopted.
To this bulk of literature this paper devotes particular
attention, aimed at developing a model close to AH, that
considers pollution as a choice variable entering the pro-
duction function as a measure of dirtiness, whose exter-

48 GIOVANNI BELLA
nal effects allow to increase the level of output [10]. It
seems then to be interpreted as pollution is a necessary
part of production and economic growth. Moving a step
forward from AH, we also deeply concentrate on the
study of the transitional dynamics of the model, and pro-
vide the whole necessary and sufficient conditions for the
existence of a feasible steady- state equilibrium path as-
sociated with a positive long-run growth. Moreover, we
conclude that a determinate saddle path sustainable equi-
librium can be reached even in presence of a long run
positive level of polluting emissions, thanks to a growing
level of new home-made inventories, without whom some
indeterminacy problems are likely to emerge [11,12].
The rest of the paper is organized as follows. In section
2, we derive the formal structure of the model, with par-
ticular attention to the set of preferences, the level of
technology, and the introduction of pollution as a crucial
variable for the system to grow. In Section 3, we concen-
trate on the solution of the optimization problem, and
deeply investigate the stability properties of the associ-
ated steady state solution. The transitional dynamics of
this economy will provide some interesting results and
policy suggestions on the way to drive an economy along
a sustainable growth path. A final section concludes, and
a subsequent Appendix provides all the necessary proofs.
2. A Model with Dirtiness
Before we enter the algebraic version of the model, we
ought to provide some detailed explanations related to the
production function, the dynamics of the environment,
and the set of preferences used to characterize the econ-
omy, whose properties we want to investigate in the rest
of the paper.
Following Romer, 1990, let S
0
represent the fixed
amount of skilled labour, which can be devoted to pro-
duction of the final good, S
Y
, or to improvement of tech-
nology, S
A
. Henceforth, we will normalize the problem
by assuming
0
1
Y A
SS S
= +=
(1)
In particular, technology (A) is not fixed. It can be cre-
ated by engaging human capital in research, growing over
time according to
A
S
A
A
γϕ +=
&
(2)
γ
indicates the research success parameter. Let us then
assume that technology
A
be partly the result of en-
dogenous (home-made) R&D efforts,
A
S γ , whilst the
remaining part depends on some exogenous new invento-
ries, whose spill-over effects can be synthesized through
a constant catch-up parameter,
ϕ
[13]. We assume
0/ >+=
A
SAA γϕ
&
, as long as either
ϕ
or
γ
and
A
S
are set positive.
1
Thus, technology can grow without
bound. We will show afterwards that, if we relax the
positiveness assumption on
γ
, the economy will face the
emergence of some undesired and indeterminate equilib-
rium problems.
Moreover, although technology is not directly linked to
pollution here, we basically consider the discovery of
new goods, or new (i.e. less polluting) production proc-
esses, as the implicit way societies follow to broadly re-
duce their dependence from environmental resources.
Basically, we are saying that each new inventory due to
technological advance is also assumed to be cleaner than
the previous one. This is also consistent with the empiri-
cal evidence that developed societies seek a less polluted
environment to live in.
Note also that research activity is assumed to be hu-
man-capital-intensive and technology-intensive, with no
capital (
K
) and ordinary unskilled labour (
L
) engaged
in that activity. To produce the final good
Y
, however,
K
does enter as an input along with human capital
Y
S
and technology
A
.
2
According to the assumptions made
in AH, the main feature of this economy is that produc-
tion is also affected by another variable indicating the
intensity of pollution,
(
)
[
]
1,0∈tz , such that higher values
of
z
yield more of the good but also more pollution
3
(
)
zKSAY
A
α
α
α −
−=
1
1 (3)
We may also consider
z
as a measure of dirtiness of
the existing production technique [10]. For example, fo-
cus on cheese manufacturing. Only a fraction of the raw
milk processed gives rise to white cheese (or other diary
products), the remaining is called whey, a liquid
by-product, only partially recyclable, which constitutes
the greater part of the resulting pollution loads. In other
words, we are assuming that production of output arises
at the expenses of the environment, with some polluting
emissions being necessarily needed.
Moreover, it is assumed that the flow of pollution
P
is proportional to the level of production, and that the use
of cleaner technologies (which means low values of
z
)
reduces the pollution/output ratio.
4
Formally,
γ
Yz
P
=
(4)
1
It is assumed that technology does not depreciate
2
Remember that in Romer, 1990, technology is assumed to be made up
of an infinite set of designs for capital, which (for simplici
ty) enter the
production function in an additively separable manner, given by
(
)
(
)
1,0
11
<<=
−−−+
βαη
βα
βα
βα
KLAASY
Y
where
η
represents the units of capital goods to produce one unit of
any type of design. Here we assume, for simplicity, that there is no
unskilled labour; that is all workers are supposed to be specialized. Let
us then consider
Y
SL = to derive E
quation (3), and normalize the
scale parameter to unity, for simplicity (1
1
=
−+ βα
η ).
3
This production function exhibits constant returns to scale at a disag-
gregate level because each firm takes z
as given. On the contrary, a
social planner can internalize this kind of externality, due to pollution
intensity, thus obtaining increasing returns.
4
The extractive use of the environment in production can either be
modeled as an input to production or, like here, as a by-product of pro-
duction; that is, pollution influences output indirectly.

GIOVANNI BELLA 49
To clarify the utility of using both variables, P and
z
,
as two sides of the same coin (the damages to the envi-
ronment), let us make another example that can be drawn
from current industrially advanced economies. Basically,
oil combustion is being needed either to feed the engine
of our cars or to stoke the furnaces of our firms, with CO
2
emissions being an unavoidable consequence. Referring
to our model it would imply that only a fraction of the oil
burnt (
z
) serves to produce final output (
Y
), the rest
being pushed into the atmosphere as a resulting emis-
sions' burden. Nevertheless, it is indeed true that not all
of these emissions are damaging, since carbon sequestra-
tion due to forests allows, for example, to reduce the total
pollution loads.
What distinguishes this economy from the one defined
in AH is that we let pollution,
P
, depend also on the
parameter expressing research success in technological
advances,
γ
; that is like assuming that the bigger
γ
-values the smaller the impact of dirty techniques on
pollution, and then the cleaner the ecosystem.
5
On the other hand, the level of investment in physical
capital is given by the usual functional form CYK −=
&
.
2.1 Dynamics of the Environment
Commonly, the environmental sector can be represented
by the dynamics of the stock of natural capital available
to the economy,
E
:
PENE −= )(
&
(5)
where )(EN determines the speed at which nature re-
generates, while
P
measures the negative effect due to
polluting emission.
6
The former is constantly reduced not
only by economic activities, but also by non-anthropo-
genic processes, such that ecosystems have to devote part
of their regeneration capacity to the maintenance of their
own structure.
7
If the capacity for regeneration exceeds the require-
ments for maintenance, )(EN becomes positive. )(EN
can therefore be interpreted as the difference between
natural resource reproduction and resource use for main-
tenance [14] that determines nature's capacity to recover
from pollution and resource extraction [4,5].
Some authors [15] propose a linear representation of
the regeneration function
EEN θ =)( (6)
where
θ
denotes the constant rate of regeneration.
8
Following this approach, if we substitute both Equa-
tions (4) and (6) into (5), we explicitly end up with
γ
θ YzEE −=
&
(7)
which represents the environmental constraint to be used
in the subsequent maximization problem.
2.2 The Set of Preferences
Let the preferences of the representative agent depend
either on the level of consumption,
t
C , or the stock of
natural capital available to the economy,
t
E .
9
The in-
tertemporal utility function is then given by
dteECU
t
tt
ρ −
∞
∫
),(
0
(8)
where
ρ
is the social discount rate.
10
)(⋅U is continuous, twice differentiable, and possesses
the following properties: 0>
C
U , 0>
E
U , 0≤
CC
U .
Also suppose that )(⋅U is concave with respect to its
two arguments:
(
)
0
2
≥−⋅
CEEECC
UUU .
11
Theoretically, sustainable development usually com-
prises two conditions. Firstly, a non-decreasing level of
consumption or utility levels, and secondly a constant or
improving state of the environment. Whether sustainable
development in this sense can be optimal, depends on the
functional form of the utility function [4].
12
A specific utility function is assumed here to have the
following CES structure
(
)
σ
σ
−
−
=⋅
−
1
1
)(
1
CE
U
where 0
>
σ
represents the inverse of the intertemporal
elasticity of substitution.
This functional form guarantees that both C and
E
grow at the same rate, so that the EC / ratio is
constant in equilibrium [16].
13
We show in the next
9
One interpretation would be forests, which contri
bute to welfare both
as sources of timber and also as stocks which provide many ecosystem
services to society (for example, carbon's sequestration, preservation of
bio-diversity)
10
For simplicity, time subscripts will be omitted in the rest of the paper
11
Constraints to the optimization problem could, for example, be intro-
duced by defining critical minimum levels for natural capital (Barbier
and Markandya, 1990) or by excluding decreasing utility paths (Pezzey,
1992). But as these restrictions usually invol
ve inequality constraints,
they may complicate the optimization problem considerably
12
While AH deal (to simplify the analysis) with a loga
rithmic, thus
separable, utility function, we prefer to introduce a non-separable func-
tion instead (as in Musu, 1995)
, that allows to compare consumption
and environ
mental quality as two substitutes, according to agents' tastes
towards them. Nonetheless, it will be shown that both assumptions can
be finally reconciled
13
We show that an improvement in natural capital is c
onductive to
growth only if we assume that consumption and natural capital are
substitutes, which implies U
CE
＜ 0. Therefore, households will be will-
ing to postpone part of their consumption opportunities only if the ex-
pected stock of natural capital is improved
5
Conversely, a negative value of
γ
reduces abatement programs, thus
finally increasing the amount of pollution realized.
6
We follow here the broad definition of natural capital given by Co-
stanza and Daly, 1992.
7
Conventional wisdom holds that plants will purify the air, helping to
reduce concentration of harmful gases. But, recently, it has been shown
that when temperatures exceed a threshold, trees and ot
her plants emit
chemicals that encourage toxic ozone production (Science, 2004).
8
Although several criticisms have been raised against the algebraic
sim
plicity of this specification (e.g., Rosendahl, 1996), it remains still
widely used in the literature of
the field, as for example in our reference
model of Aghion and Howitt, 1998.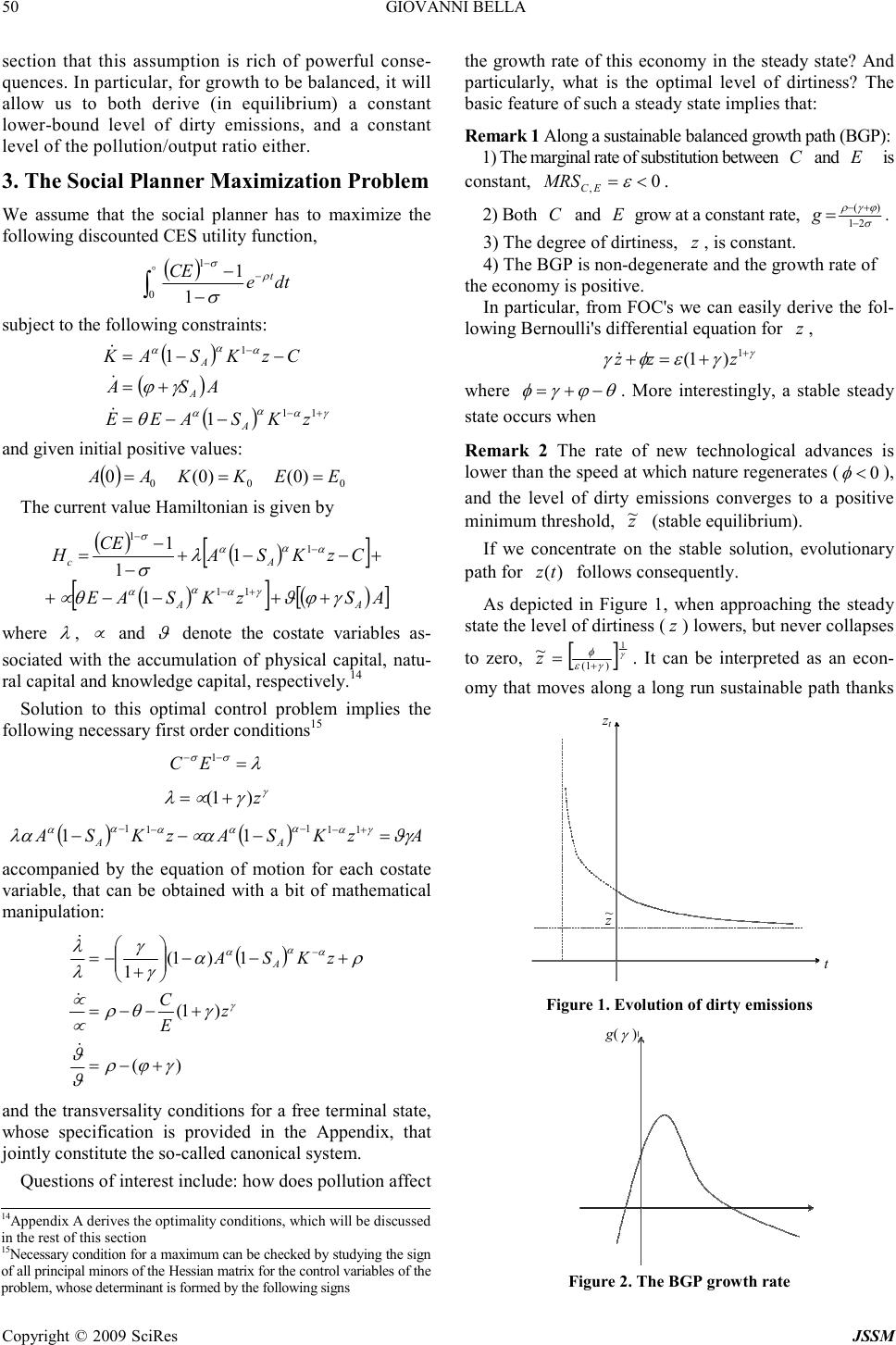

50 GIOVANNI BELLA
section that this assumption is rich of powerful conse-
quences. In particular, for growth to be balanced, it will
allow us to both derive (in equilibrium) a constant
lower-bound level of dirty emissions, and a constant
level of the pollution/output ratio either.
3. The Social Planner Maximization Problem
We assume that the social planner has to maximize the
following discounted CES utility function,
(
)
dte
CE
t ρ
σ
σ
−
−
∞
−
−
∫
1
1
1
0
subject to the following constraints:
(
)
( )
( )
γα
α
α
α
α
α
θ
γϕ
+−
−
−−=
+=
−−=
11
1
1
1
zKSAEE
ASA
CzKSAK
A
A
A
&
&
&
and given initial positive values:
(
)
000
)0()0(0 EEKKAA ===
The current value Hamiltonian is given by
(
)
( ) [ ]
( ) [ ] ( ) [ ] ASzKSAE
CzKSA
CE
H
AA
Ac
γϕϑθµ
λ
σ
γα
α
α
α
α
α
σ
++−−+
+−−+
−
−
=
+−
−
−
11
1
1
1
1
1
1
where
λ
,
µ
and
ϑ
denote the costate variables as-
sociated with the accumulation of physical capital, natu-
ral capital and knowledge capital, respectively.
14
Solution to this optimal control problem implies the
following necessary first order conditions
15
λ
σσ
=
−− 1
EC
γ
γµλ z)1(+=
(
)
(
)
AzKSAzKSA
AA
ϑγµαλα
γα
α
αα
α
α
=−−−
+−
−
−
−11 1
1
1
11
accompanied by the equation of motion for each costate
variable, that can be obtained with a bit of mathematical
manipulation:
( )
)(
)1(
1)1(
1
γϕρ
ϑ
ϑ
γθρ
µ
µ
ρα
γ
γ
λ
λ
γ
α
α
α
+−=
+−−=
+−−








+
−=
−
&
&
&
z
E
C
zKSA
A
and the transversality conditions for a free terminal state,
whose specification is provided in the Appendix, that
jointly constitute the so-called canonical system.
Questions of interest include: how does pollution affect
the growth rate of this economy in the steady state? And
particularly, what is the optimal level of dirtiness? The
basic feature of such a steady state implies that:
Remark 1 Along a sustainable balanced growth path (BGP):
1) The marginal rate of substitution between C and
E
is
constant, 0
,
<= ε
EC
MRS .
2) Both C and
E
grow at a constant rate,
σ
ϕγρ
21
)(
−
+−
=g .
3) The degree of dirtiness,
z
, is constant.
4) The BGP is non-degenerate and the growth rate of
the economy is positive.
In particular, from FOC's we can easily derive the fol-
lowing Bernoulli's differential equation for
z
,
γ
γεφγ
+
+=+
1
)1( zzz
&
where
θ
ϕ
γ
φ
−
+
=
. More interestingly, a stable steady
state occurs when
Remark 2 The rate of new technological advances is
lower than the speed at which nature regenerates ( 0
<
φ
),
and the level of dirty emissions converges to a positive
minimum threshold,
z
~
(stable equilibrium).
If we concentrate on the stable solution, evolutionary
path for )( tz follows consequently.
As depicted in Figure 1, when approaching the steady
state the level of dirtiness (
z
) lowers, but never collapses
to zero,
[
]
γ
γε φ
1
)1(
~
+
=z . It can be interpreted as an econ-
omy that moves along a long run sustainable path thanks
Figure 1. Evolution of dirty emissions
Figure 2. The BGP growth rate
14
Appendix A derives the optimality conditions, which will be dis
cussed
in the rest of this section
15
Necessary condition for a maximum can be checked by studying the sign
of all principal minors of the Hessian matrix for the control vari
ables of the
problem, whose determinant is formed by the following signs
z
t
z
~
t
g (
γ
)

GIOVANNI BELLA 51
to a positive value of dirty emissions, unless we admit a
stop in the economic development. This is consistent with
the behavior of an advanced economy where, despite the
presence of a high demand for environmental protection
and a rise in technological innovations, it can be noted
nonetheless a substitution amongst pollutants, whose
pressure on the ecosystem is far away from disappearing.
This economy seems to mimic one where, to achieve
balanced growth, pollution grows at the same level of
output. However, we conclude that this economy behaves
in a sustainable way only if natural capital grows more
than technological sector. To summarize, it can be thought
as a simple parable to explain why rich economies, despite
their preferences towards clean air, and the presence of a
technological sector that permits to substitute inputs in
production, still achieve high levels of output, though as-
sociated with higher levels of emissions (
2
CO , for exam-
ple) to the atmosphere of the environment they live.
3.1 The Reduced Model
We can reduce the dimension of the canonical system given
so far through the following convenient variable substitution
qz
E
C
m
K
Y
x
K
C
=
=
=
γ
and consequently end up with a new system in three di-
mensions,
x
,
m
, and
q
:
mqq
x
mq
qq
mqmmm
xmxmqxx
)1()1(
21
1
)1(
1
2
2
2
2
σβγ
σ
σ
ξ
βσ
δη
β
σ
σ
ξ
−+++





−
−=
+−=
+−+ 




−
−=
&
&
&
where
γ
αγ
δ
αγ
γϕαγθ
η
σ
α
γ
γ
β
σ
ρθσ
ξ
+
+
=
−
++
=





−








+
=
−−
=
1
1
)1(
)(
1
1
)1(
The associated Jacobian matrix at the steady state (
∗
x ,
∗
m ,
∗
q ) is then
( )( )( )
( )( )( ) 











−+−−
−
−−−+
=
∗
∗∗
∗
∗
∗
∗∗
∗
∗∗
−
∗
−
∗
−
∗∗
∗
−
∗
−
∗
−
∗
∗
x
qm
x
q
x
qm
x
qm
qq
mm
mqxx
J
σ
σ
σ
σ
σ
σ
βσ
σ
σ
σ
σ
σ
σ
γσβ
δ
β
212121
1
111
)1()1(
0
)1(
2
2
2
Studying the behavior of this economy while converg-
ing to the steady state needs particular attention, espe-
cially if we want to control for the presence of undesired
outcomes due to the rise of indeterminacy problems. To
this end, we apply the neat Routh-Hurwitz criterion to the
structure of eigenvalues associated with J
*
, and easily verify
that 0 >
∗
trJ , and 0 <
∗
DetJ . In this case, the sequence of
signs becomes ( - , +, ?, - ), the only possibility is thus two
positive and one negative eigenvalues. The interior steady
state is therefore determinate, or saddle path stable.
16
This is quite a piece of news when dealing with a
Romer-type economy, whose uniqueness of the equi-
librium trajectory, largely studied in several papers,
showed the need for some parameters of the model to
belong to a particular defined set. For example, Asada
et al . [17] study the stability properties of a social plan-
ner version of the Romer model and several modifica-
tions of it, including the complementarity of different
intermediate goods introduced by Benhabib et al . [18],
and find the emergence of Hopf bifurcation points and
stable periodic solutions [19,20].
More recently, Slobodyan [9] reconsiders a slightly
simpler version of Benhabib et al . [18], and derives the
restrictions on the parameter values necessary to obtain
an interior steady state solution. He shows that Hopf bi-
furcation leading from determinate steady state to a com-
pletely stable one does not exist, but that indeterminate
steady state can become absolutely unstable (explosive)
through Hopf bifurcation.
In this light, we ought to make a deep investigation, by
relaxing the assumption made upon the research success
parameter,
γ
, and show that in case we allow it to be-
come negative, some indeterminacy problems may finally
arise, and thus complicate the possibility to attain a sus-
tainable equilibrium solution either. The next section is
devoted to this end.
3.2 A Numerical Analysis
Without any loss of generality, and for the sake of sim-
plicity, in this section we analyze a simpler version of
the model set above, where we constrain 1
=
σ
. It is in-
deed like moving back to the AH model, where the struc-
ture of preferences implies a logarithmic utility function.
Firstly, let us consider the case 0
>
γ
, then the Jaco-
bian matrix easily reduces to












−
−
−
=
∗
∗
∗
∗∗
∗∗
∗∗
∗
ρ
δ
δ
β
x
q
x
qm
mm
xx
J
2
2
2
1
0
0
16
Since the eigenvalues of J
*
are the solutions of its characteristic equa-
tion

52 GIOVANNI BELLA
with
02
*
>= ρ trJ
[ ] 0
22*
<+−=
∗∗
∗
∗
xq
x
m
DetJ βδ
β
ρ
the system is still characterized by a two-change of sign
( - , +, ?, - ), which implies the local stability of the steady
state solution.
In particular, stability of system ( S ) needs
[ ] 0)1(
1
=+−+
=+
+=
∗∗∗∗
∗∗
∗∗
qmxq
mq
mx
ργ
δ
β
η
δρ
which implies, solving for
∗
m , the following quadratic
equation
0)(
2
=−−=
∗∗
cbmammG
where
[ ]
( ) ( )
( )()( )
( )
2
2
2
]11[
1
1
1
2
)1(
)2)(1)(1(
)1)(1(1
αγθρρ
αγ
γ
αγρ
γ
αγθαγ
γ
αγ
αγ
α
γ
γββδ
++=
−−
+
+
+
+
++
=
+
+
+
−
=+−−=
c
b
a
and given 0
>
a , and 0
>
c , this allows us to understand
why there is only one possible positive solution for
∗
m
in steady state, and the system is therefore locally stable,
whatever the sign of b , as shown in Figure 3.
On the contrary, if we allow the research success pa-
rameter (i.e. the degree of pollution abatement), to fall
below zero, 0
<
γ
, then some unexpected economic
outcomes may arise. In particular, whenever 1
1
−<<− γ
α
,
we conclude that either 0,
<
ba or 0
>
c, whose
graphic representation in Figure 4 clearly shows the
Figure 3. Unique equilibrium
presence of two positive solutions for 0)(=
∗
mG , and
thus consequently signal the emergence of a multiplicity
of equilibria.
To conclude, the new home-made inventories become
a key indicator to achieve a long run sustainable equilib-
rium. On the one hand, in fact, we have shown so far that
as long as an increase in the stock of knowledge is real-
ized, i.e.
γ
is positive, the economy converges to a sad-
dle path stable steady state. On the other hand, when the
home-made research sector experiences a decreasing
level of new inventories, which means a negative value
for
γ
, the economy is likely to manifest some indeter-
minacy problems. In this case, a multiplicity of equilibria
is therefore possible to arise, and consequently generate a
situation where the economy might be trapped in a lower
equilibrium solution. Other non-economic factors are
thus possibly acting as a means for equilibria to differ
along the transition path towards the steady state.
4. Concluding Remarks
A clear connection between growth and the quality of the
environment is complex. Some elements of environ-
mental quality appear to improve with growth; others
worsen; still others exhibit deterioration followed by
amelioration. Despite this evidence, most studies dealing
with the impact of environmental policy on growth
ignore the adverse effect of pollution on productivity.
The state of the environment may worsen with time if
concentrations of pollutants accumulate or if consumer
tastes shift towards pollution-intensive goods. The op-
posite does occur if technological innovations make
abatement less costly or if increasing awareness causes
an autonomous shift in public demands for environ-
mental safeguards. To this end, only if technological
progress has to provide the means to reducing the
over-exploitation of natural resources, a sustainable
growth can be possible.
To bridge the existing gap we set up a model close to
Aghion and Howitt [6] and examine the problem of sus-
tainable growth in presence of dirty (i.e. polluting)
Figure 4. Multiple equilibria
G
(
m
*
)
(
b
＜
0)
(
b
＜
0)
m
*
G
(
m
*
)
m
*

GIOVANNI BELLA 53
production processes. We show that under certain condi-
tions a sustainable growth is always attainable. The main
difference with respect to our analysis regards the defini-
tion of a non-separable utility function (where both con-
sumption and the environment are seen as two substi-
tutes), and a particular technological sector where both
home-made and outsourcing research activities are con-
sidered.
Particular attention has been devoted to the transitional
dynamics of the model around the steady state, where the
role of home-made research has turned out as a key de-
vice for stability and uniqueness of equilibrium solutions.
Indeed, if the home-made research parameter is allowed
to be negative, some indeterminacy problems arise, and
multiple equilibria are likely to emerge. In this latter case,
some non-economic factors become crucial in the solu-
tion of our decision making problem. This is consistent to
how real economies nowadays behave, whenever their
different cultural backgrounds impinge on the approach
used to tackle the problem of a sustainable allocation of
the available natural resources amongst generations. That
is also likely to influence the development path of these
economies towards the steady state, and eventually trap
the systems into an unavoidable low equilibrium level.
REFERENCES
[1] D. I. Stern, “The rise and fall of the environmental kuznets
curve,” World Development 32, pp. 1419-1439, 2004.
[2] S. Smulders, “Environmental policy and sustainable eco-
nomic growth: An endogenous growth perspective,” De
Economist 143, pp. 163-195, 1995.
[3] A. L. Bovenberg and S. Smulders, “Environmental quality
and pollution-augmenting technological change in a
two-sector endogenous growth model,” Journal of Public
Economics 57, pp. 369-391, 1995.
[4] A. L. Bovenberg and S. Smulders, “Transitional impacts
of environmental policy in an endogenous growth model,”
International Economic Review 37, pp. 861-893, 1996.
[5] K. Pittel, “Sustainability and endogenous growth,” Ed-
ward Elgar, Cheltenham, 2003.
[6] P. Aghion and P. Howitt, “Endogenous growth theory (2nd
edition),” MIT Press, Cambridge, Massachusetts, 1998.
[7] A. Grimaud and F. Ricci, “The growth-environment
trade-off: horizontal vs vertical innovations,” Fondazione
ENI Enrico Mattei Working Paper n, pp. 34-99, 1999.
[8] P. Schou, “Polluting non renewable resources and
growth,” Environmental and Resource Economics 16 (2),
pp. 211-227, 2000.
[9] S. Slobodyan, “Indeterminacy and stability in a modified
Romer model,” Journal of Macroeconomics 29, pp.
169-177, 2007.
[10] A. Grimaud, “Pollution permits and sustainable growth in
a schumpeterian model,” Journal of Environmental Eco-
nomics and Management 38, pp. 249-266, 1999.
[11] S. I. Restrepo-Ochoa and J. Vazquez, “Cyclical features
of the Uzawa--Lucas endogenous growth model,” Eco-
nomic Modelling 21, pp. 285-322, 2004.
[12] M. A. Gomez, “Transitional dynamics in an endogenous
growth model with physical capital,” Human Capital and
R&D. Studies in Nonlinear Dynamics & Econometrics 9
(1), article 5, 2005.
[13] S. Smulders, “Economic growth and environmental qual-
ity,” In: Folmer, H., Gabel, L. (Eds), Principles of Envi-
ronmental and Resource Economics, Edward Elgar,
Chapter 20, pp. 602-664, 2000.
[14] S. Smulders, “Entropy, environment, and endogenous
economic growth,” International Tax and Public Finance
2, pp. 319-340, 1995.
[15] I. Musu, “Transitional dynamics to optimal sustainable
growth,” Fondazione ENI Enrico Mattei Working Paper n.
50.95, 1995.
[16] R. J. Barro and X. Salai-Martin, “Economic growth,”
McGraw-Hill, New York, 1995.
[17] T. Asada, W. Semmler, and A. J. Novak, “Endogenous
growth and the balanced growth equilibrium,” Research in
Economics 52, pp. 189-212, 1998.
[18] J. Benhabib, R. Perli, and D. Xie, Monopolistic competi-
tion, indeterminacy and growth. Ricerche Economiche 48,
pp. 279-298, 1994.
[19] L. G. Arnold, “Endogenous technological change: A note
on stability,” Economic Theory 16, pp. 219-226, 2000.
[20] L. G. Arnold, “Stability of the market equilibrium in Ro-
mer's model of endogenous technological change: A com-
plete characterization,” Journal of Macroeconomics 22, pp.
69-84, 2000.
(Edited by Vivian and Ann)

54 GIOVANNI BELLA
Appendix A
The current value Hamiltonian for the maximization
problem is given by
(
)
( )
[
]
( )
[ ]
[ ] ASzKSAE
CzKSA
CE
H
AA
Ac
)(1
1
1
1
11
1
1
γϕϑθµ
λ
σ
γα
α
α
α
α
α
σ
++−−+
+−−+
−
−
=
+−
−
−
(1)
where
λ
,
µ
and
ϑ
denote the costate variables as-
sociated with the accumulation of physical capital, natu-
ral capital and knowledge capital, respectively.
First order conditions can be written as:
1.a
[
]
0=
∂
∂
C
H
c:
λλ
σσσσ
=⇒=−=
∂
∂−−−− 11 0
ECEC
C
H
c
(2)
1.b
[
]
0=
∂
∂
z
H
c
:
( )( ) 01)1(1
11 =−+−−=
∂
∂−−
γα
α
αα
α
α
γµλ zKSAKSA
z
H
AA
c
(3)
that is simply
γ
γµλ z)1( += (4)
1.c
[
]
0=
∂
∂
A
c
S
H
:
( )( )
0
11
11
1
1
1
=+
−+−−=
∂
∂
+−
−
−
−
AzK
SAzKSA
S
H
AA
A
c
ϑγ
µαλα
γα
α
αα
α
α
(5)
or rather, using (A.3a) it becomes
(
)
AzKSA
A
ϑµγα
γα
α
α
=−
+−
−11 1
1 (6)
Equation of motion for each costate variable is given by
λρλ +
∂
∂
−=K
H
c
&
(7)
µρµ +
∂
∂
−= E
H
c
&
(8)
ϑρϑ +
∂
∂
−= A
H
c
&
(9)
and we can simply derive, by means of the conditions
obtained above:
( )
( ) ργγα
ϑ
µ
ϑ
ϑ
ρθ
µµ
µ
ρα
γ
γ
λ
λ
γα
α
α
σσ
α
α
α
+−−−=
+−−=
+−−








+
−=
+−−
−−
−
AA
A
SzKSA
EC
zKSA
111
1
1
1)1(
1
&
&
&
(10)
by substituting out condition (A.4a) into the law of mo-
tion of
ϑ
, it follows
)( γϕρ
ϑ
ϑ
+−=
&
(11)
whereas, taking logs in (A.2) and differentiating, we have
E
E
C
C&
&& )1(
σσ
λ
λ
−+−= (12)
From condition (A.6) derives
µρµθµ
σσ
+−−=
−−
EC
1
&
(13)
or, alternatively,
ρθ
µµ
µ
+−−=
E
U
&
(14)
given that
E
E
U
UEC ==
−−
∂
⋅∂ σσ 1 )(
. But substituting out
µ
in the RHS, by means of (A.3a), we obtain
ρθγ
λµ
µ
γ
+−+−= z
U
E
)1(
&
(15)
Since
C
U= λ , from FOC, we have
ρθγ
µ
µ
γ
+−+−= z
U
U
C
E
)1(
&
(16)
and finally, since equilibrium requires that
E
C
U
U
C
E
=, it
follows
γ
γθρ
µ
µ
z
E
C)1( +−−=
&
(17)
· Arrow sufficiency theorem holds since the maxi-
mized Hamiltonian, evaluated along the optimal control
variables, is concave in all the state variables, as we can
simply check through the sing of the minors of the Hessian
matrix, whose determinant implies the following sings
−+
−
+−
=
0
00
0
H (18)
· hence, 0
1
<H, 0
2
>H, and 0
3
<H iff
1
>
A
,
that is the number of designs must necessarily be greater
that one.
· Transversality conditions for a free terminal state
hold for all shadow prices, and are given by
0
~~~~
lim
0
~
~
~
~
lim
0
~
~
~
~
lim
)()(
)2()21(
)2()21(
===
===
===
−−−−−−
∞→
+−−−−
∞→
+−−−−
∞→
tgtgttt
t
tgtgtgtt
t
tgtgtgtt
t
eAeeAeAe
eEeeEeEe
eKeeKeKe
γϕργϕρρ
ρσρσρ
ρσρσρ
ϑϑϑ
µµµ
λλλ
(19)
· Where λ
~
, µ
~
, ϑ
~
, and
K
~
,
E
~
,
A
~
, are the
shadow prices and the state-values on the balanced
growth path;

GIOVANNI BELLA 55
· Moreover, for free time
t
, we need to show that
0lim =
∞→
H
t
, which is always verified due to convergence
towards zero of both the discounted utility function,
0)(lim =⋅
−
∞→
t
t
eU
ρ
, and all the multipliers, as proved above.
Transitional dynamics of the problem can be studied
by applying the Routh-Hurwitz criterion to the autono-
mous system
mqmmm
xmxmqxx
βσ
δη
β
σ
σ
ξ
1
)1(
1
2
2
+−=
+−+





−
−=
&
&
mqq
x
mq
qq )1()1(
21
2
2
σβγ
σ
σ
ξ −+++





−
−=
&
and the associated Jacobian matrix, evaluated along the
steady state










=
∗∗∗
∗∗∗
∗∗∗
∗
333231
232221
131211
JJJ
JJJ
JJJ
J
where
(
)
*
**
1
*
11
x
qm
xJ
σ
σ −
∗
+=
(
)
*
1
*
12
)1( qxJ
σ
σ
β
−
∗
−−=
(
)
*
1
13
mJ
σ
σ −
∗
−=
0
21
=
∗
J
*
22
mJ δ −=
∗
*
1
23
mJ
βσ
=
∗
( )
2
*
2
**
21
31 x
qm
J
σ
σ −
∗
=
( )
*
2
*
21
*
32
)1(
x
q
qJ
σ
σ
σβ
−
∗
−−=
(
)
*
**
21
*
33
)1(
x
qm
qJ
σ
σ
γ
−
∗
−+=
which implies consequently
0)1(22
*
*
**
*
>++= q
x
qm
trJ γ
and
0
11121
21121
)1(
1
2
***
2
**
2
*
2
**
*
2
**
2
**
*
*
2
*
**
*
**
**
<










−
−







−
−







−





−
+

















−
+





−
−





−
++−










−
+=
mqmxm
x
qm
x
qm
qm
x
qm
qm
x
qm
xDetJ
σ
σ
δ
βσ
σ
βσ
β
σ
σ
βσ
σ
σ
σ
σ
σ
δγδ
σ
σ
which implies also










−
−







−
−










−
++












−
+
<










−
−







−
−







−





−
2
***
2
**
2
*
2
**
*
2
***
2
**
2
*
2
**
21211
)1(
1
11121
mqmxm
x
qm
q
mqmxm
x
qm
σ
σ
δ
βσ
σ
σ
σ
γδ
σ
σ
σ
σ
δ
βσ
σ
βσ
β
σ
σ
since it is always verifiable that
σ
σ
σ
σ
211
−
>
−





−
++<
−
σ
σ
γδ
βσ
β
1
)1(
1
or rather
[
]
0)1()1(
2
>++− σγγαα