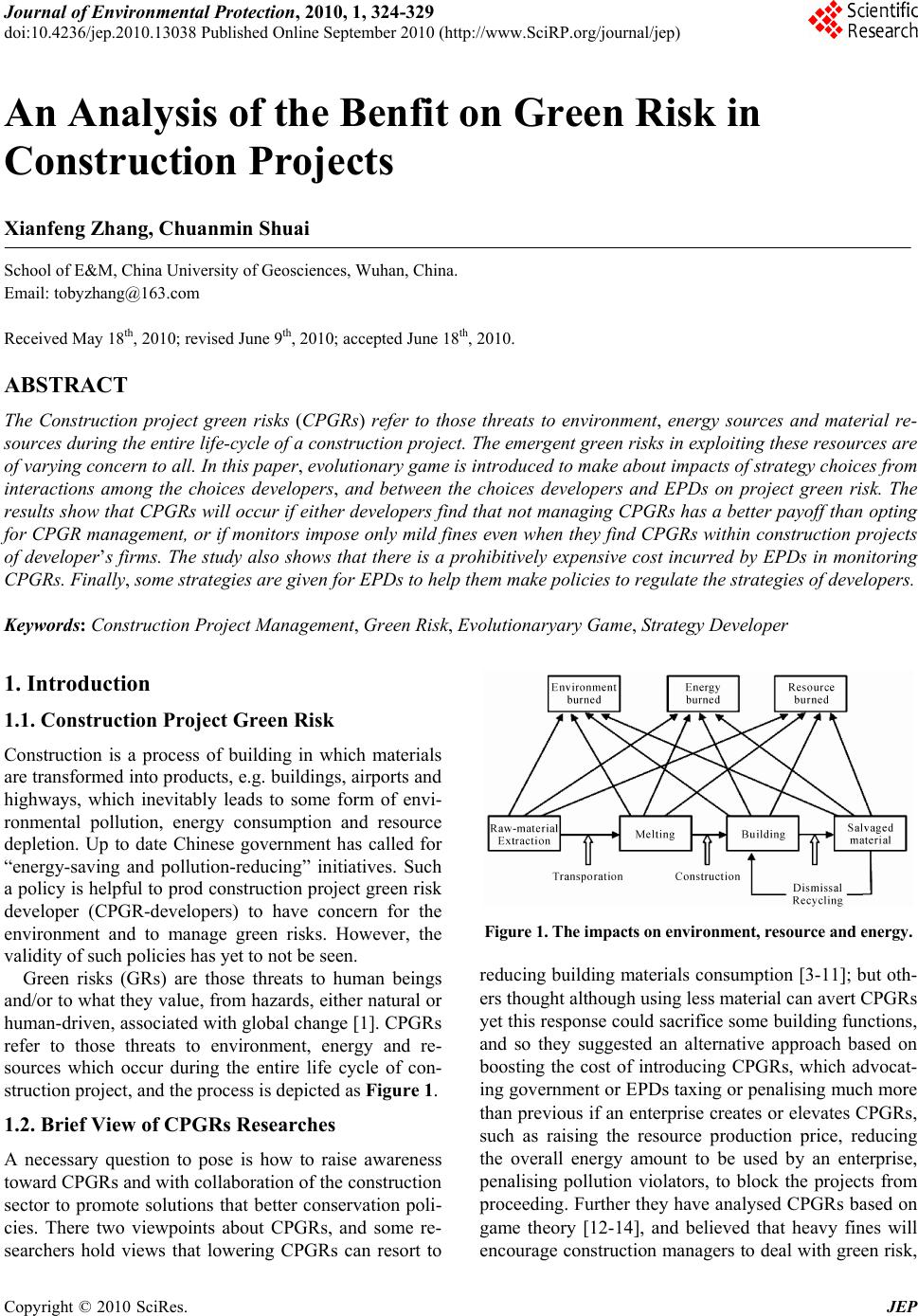Journal of Environmental Protection, 2010, 1, 324-329
doi:10.4236/jep.2010.13038 Published Online September 2010 (http://www.SciRP.org/journal/jep)
An Analysis of the Benfit on Green Risk in
Construction Projects
Xianfeng Zhang, Chuanmin Shuai
School of E&M, China University of Geosciences, Wuhan, China.
Received May 18th, 2010; revised June 9th, 2010; accepted June 18th, 2010.
ABSTRACT
The Construction project green risks (CPGRs) refer to those threats to environment, energy sources and material re-
sources during the entire life-cycle of a construction project. The emergent green risks in exploiting these resources are
of varying concern to all. In this paper, evolutionary game is introduced to make about impacts of strategy choices from
interactions among the choices developers, and between the choices developers and EPDs on project green risk. The
results show that CPGRs will occur if either developers find that not managing CPGRs has a better payoff than opting
for CPGR management, or if monitors impose only mild fines even when they find CPGRs within construction projects
of developer’s firms. The study also shows that there is a prohibitively expensive cost incurred by EPDs in monitoring
CPGRs. Finally, some strategies are given for EPDs to help them make policies to regulate the strategies of developers.
Keywords: Construction Project Management, Green Risk, Evolutionaryary Game, Strategy Developer
1. Introduction
1.1. Construction Project Green Risk
Construction is a process of building in which materials
are transformed into products, e.g. buildings, airports and
highways, which inevitably leads to some form of envi-
ronmental pollution, energy consumption and resource
depletion. Up to date Chinese government has called for
“energy-saving and pollution-reducing” initiatives. Such
a policy is helpful to prod construction project green risk
developer (CPGR-developers) to have concern for the
environment and to manage green risks. However, the
validity of such policies has yet to not be seen.
Green risks (GRs) are those threats to human beings
and/or to what they value, from hazards, either natural or
human-driven, associated with global change [1]. CPGRs
refer to those threats to environment, energy and re-
sources which occur during the entire life cycle of con-
struction project, and the process is depicted as Figure 1.
1.2. Brief View of CPGRs Researches
A necessary question to pose is how to raise awareness
toward CPGRs and with collaboration of the construction
sector to promote solutions that better conservation poli-
cies. There two viewpoints about CPGRs, and some re-
searchers hold views that lowering CPGRs can resort to
Figure 1. The impacts on environment, resource and energy.
reducing building materials consumption [3-11]; but oth-
ers thought although using less material can avert CPGRs
yet this response could sacrifice some building functions,
and so they suggested an alternative approach based on
boosting the cost of introducing CPGRs, which advocat-
ing government or EPDs taxing or penalising much more
than previous if an enterprise creates or elevates CPGRs,
such as raising the resource production price, reducing
the overall energy amount to be used by an enterprise,
penalising pollution violators, to block the projects from
proceeding. Further they have analysed CPGRs based on
game theory [12-14], and believed that heavy fines will
encourage construction managers to deal with green risk,

An Analysis of the Benfit on Green Risk in Construction Projects 325
compel them to make a decision either to comply with
environmental policy decreed by government to better
management of CPGRs, or to face penalties from EPDs
and incur financial cost or some other such punishment.
Unfortunately traditional game theory is usually lim-
ited to exploring rational agents’ interactions. However,
the rational assumption is not valid for CPGR-developers
in that these developers will find it difficult to know
competitors’ strategies and hence their own decisions
will not be treated rationally due to incomplete knowl-
edge. Thus bounded rationality, as it is called, is the
norm, and over time CPGR-developers will learn and
develop their own strategies, or imitate other players’
strategies. Indeed, they may change them constantly and
hence develop an evolutionary stable strategy (ESS). In
this article, evolutionary game is introduced to analyse
the choice behaviours, by considering decision-makings
of CPGR developers and their interactions among each
other and with EPDs, in order to find motives and rea-
sons to behaviours towards CPGRs.
2. The Evolutionary Game Based Model of
CPGR Developers
Confronted with CPGRs, developers (those firms who by
their activity in the construction area produce the CPGRs)
have two choices. One choice, which here is called manag,
is using new technology to reduce green risk. The tech-
nology may take the form of utilizing low-energy con-
sumption machines, providing low-energy steel, or in-
creasing the use of recycled metals. The other choice is
non-manag, i.e., in plain speak, shirking responsibility
for green risk and simply doing nothing. Now we assume
that there exists a construction project for which there are
two vying construction firms that have presented project
tenders. Within the game theory context, we introduce
outcomes determined by the attitude of the developers to
CPGRs. If a developer elects to deal with them the gain
is A; if the other does nothing, the developer gets B, oth-
erwise C. Of course, the two developers will both get D
if they show no concern toward CPGRs. The payoff ma-
trix that game theory ascribes to the two players of this
game is as follows Table 1.
For the two players, the problem is which strategy
should be employed; the decision depends on the action
Table 1. Payoff matrix of CPGR-developers.
CPGR-Developer II
Manag. Non-manag.
Manag. A, A B, C
CPGR-Developer I
Non-manag. C, B D, D
of the other; such a situation illustrates a game of imper-
fect information. As a consequence, we rank the pay-offs
as A ≠ B ≠ C ≠ D. If two players are rational economic
agents, the result of the game depends on the relative
values assigned to A, B, C and D. However, the develop-
ers are in fact bounded rational agents, so after playing
the game, the values A, B, C and D must have some
relevance to reality, so that evolutionary game theory is
applicable.
In this game, the two developers choose between two
strategies with a certain payoff. However, how will a
population of developers that repeatedly play this game
evolve? In what follows, it will be assumed that the pay-
offs will be the same for every developer. We cannot
answer the above question without introducing some
assumptions concerning the nature of the population. Let
us being by assuming the number of developers is large,
so that we can represent the state of the population by
keeping tract of what proportion of developers follow the
strategy managem en t and those following non-manage-
ment. We further assume that the proportions following a
particular strategy at the next generation of play is pro-
portional to that of the current generation. Thus the
strategies themselves are now playing each other. This
then provides us with differential equations and hence
continuous dynamics known as replicator dynamics for
an evolutionary gaming theory. Finally we assume that
strategies are uncorrelated, i.e. that the probability with
which every strategy meets every other strategy depends
only on the relative frequencies within the population.
Thus the games between developers are randomly played.
We denote the frequency of population in management
strategy in the CPGR decision game as x, which will vary
with time t, and consequently the population ratio of
non-management strategies with 1 − x, and then We have:
(1 )
(1 )
(1 )
t
f
tf
uxA xB
uxC xD
uxu xu







(1)
where ut is the expected gain for the management strat-
egy, uf is the expected gain for the non-management
strategy, uis the average gain for all construction firms
for the project at that generation.
Based on 2 × 2 symmetric game model, we can get
dynamic Equation (2), then obtain Equation (3) if substi-
tuting (1) into, and further induce Equation (4) and three
possible stationary states are eventually obtained if set-
ting ()/ 0F xdxdt

. According to evolutionaryary
stable strategy (ESS) of the differential equation, we ob-
tain the optimal solution xi* is ESS, if F′(x*
i) =0. The
equations listed as follows:

An Analysis of the Benfit on Green Risk in Construction Projects 326
/()(1)( )
ttf
dxdtxu uxxuu (2)
() /(1)[()(1)()]
F
xdxdtx xxACxBD (3)
12
3
0, 1,
01
xx
DB DB
xif
ABCD ABCD






 

(4)
In the model there are four aspects needed to be fo-
cused as follows:
The circumstance A = C and B = D implies one player,
who is playing management strategy, will receive more
gain, irrespective of whether the other players are exe-
cuting management strategy or not. This situation will
occur if government can strictly enforce laws concerning
CPGR-developers. If this is the case, the risks can be
determined and developers will be punished. The loss
resulting from punishment is higher than the cost of
managing the risks. Thus, F′(x*
1) = 0, F′(x* 2) ＜ 0, x* 3 is
not ESS, x*
2 = 1 is the only ESS. Therefore, we can de-
scribe the game result as one in which a bounded rational
developer, after playing repeatedly, will change to man-
agement strategy. This selection will encourage manage-
ment strategy as the cleverer scheme for developers.
It is possible that one player who opts for non-manage-
ment strategy will gain more whether or not it is played
by the other player. This situation requires A ＜ C and B
＜ D. Normally, this state arises when the EPDs are
completely in breach of their duty and hence the ignored
CPGR-developers will find that there would be more
gain if they played non-management strategy rather than
management strategy. In this case x*
1 = 0 is the only ESS
because F′(x*
1) ＜ 0 and F′(x* 2) = 0. Playing the game
repeatedly, bounded rational developers will think it is
foolish to elect management strategy.
If one player chooses management strategy in this
CPGR decision game, the other player then finds man-
agement strategy is a smart choice for himself, as in this
situation if A = C and B ＜ D is satisfied then one
player receives less gain from management strategy than
from the non-management strategy when the other player
does nothing about CPGRs. These cases will occur re-
peatedly if the non-management action earns more bene-
fit than the loss incurred in the punishment from EPDs.
Of course, F′(x*
1) ＜ 0, F′(x* 2) ＜ 0, F′(x* 3) = 0, x* 1 = 0
and x*
2 = 1 are ESSes. Thus the game result will decided
on the original population level of x. When x ∈ (0, x*
3),
after repeated play, the developer will give up manage-
ment strategy. However, when x ∈ (x*
3, 1), the contrary
situation will be taken up. Especially, if D = B, A ≠ C,
thus x*
3 = 0, all the developers will chose non-management
plan and the management strategy is used when D ≠ B
and A = C because of x*
3 = 1.
Of course, if A ＜ C and B = D then this situation
implies that management strategy has less gain than non-
management when the other developer executes man-
agement strategy, or means the contrary strategy when
the others abandon management strategy while the player
finds that he will get severely punished due to increasing
CPGRs. This means the player’s strategy of choice de-
pends on the other players’ actions according to the seri-
ousness of CPGRs. For example, after using non-mana-
gement strategy, developers find themselves receiving
less fines and the others playing management to the risk
resource at the same time. Which choices will they make?
Clearly, non-management strategy! In contrast, when
they find they are receiving higher fines, the only right
choice is to take up management strategy because if
they give up management the nasty risk resources
strategy within the others doing the same things. Thus
123
()0,()0,()0Fx FxFx
 

 , 3
x
 is the only ESS.
The result tells us the frequency 3
x
 of developers out
of all developers will management the CPGRs after re-
peatedly playing the game, and the frequency playing
non-management strategy will be (*
3
1
x
). Obviously,
the frequency of management strategy players is rising
with “B - D” increasing, but increasing “C - A” attracts
some bad implications.
From the above analysis, we know that projects re-
ceiving little CPGR is possible if we reduce the expected
benefit of non-management strategy over management
strategy. Therefore, serious policy is necessary from
government. In the next section, we discuss game strate-
gies between monitors from environment protection de-
partments (EPD) and CPGR-developers.
3. The Evolutionary Game Based Model of
CPGR-Developers and EPDs
The developers have two choices: implementing either a
management or non-management strategy in confronting
CPGRs; meanwhile the EPDs can also chose between a
monitoring or non-monitoring attitude. Through envi-
ronmental monitoring, EPDs will provide judgment on
whether CPGRs are acceptable, in which case no action
is taken, or unacceptable, in which case a fine will be
adjudicated. However monitoring needs funding and may
even cost more than is bargained, thus creating in itself a
problem for EPDs. In contrasting the comparative gain
between various strategies, we assume the benefit to two
players is 0 (in reality, the benefit is not 0) under the cri-
terion that developers will execute a management strat-
egy toward CPGRs and the EPD carries out non–monitor
strategy. We then let 10C refer to the EPD’s moni-
toring costs of CPGRs, 20C the punishment to EPDs
for breach of duty from government, 0S the fine to
be imposed by EPDs on non-management behaviour of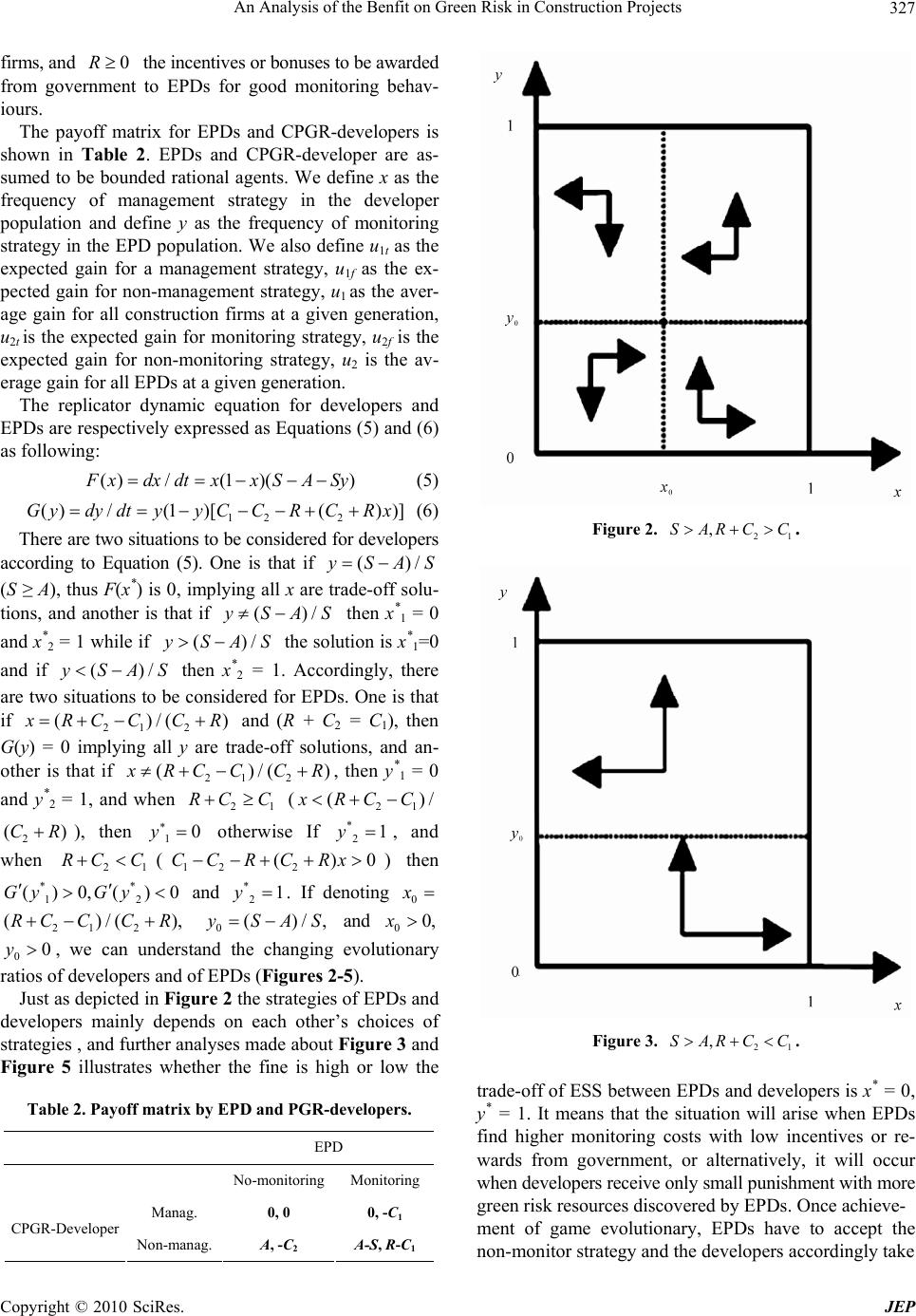

An Analysis of the Benfit on Green Risk in Construction Projects 327
firms, and 0R the incentives or bonuses to be awarded
from government to EPDs for good monitoring behav-
iours.
The payoff matrix for EPDs and CPGR-developers is
shown in Table 2. EPDs and CPGR-developer are as-
sumed to be bounded rational agents. We define x as the
frequency of management strategy in the developer
population and define y as the frequency of monitoring
strategy in the EPD population. We also define u1t as the
expected gain for a management strategy, u1f as the ex-
pected gain for non-management strategy, u1 as the aver-
age gain for all construction firms at a given generation,
u2t is the expected gain for monitoring strategy, u2f is the
expected gain for non-monitoring strategy, u2 is the av-
erage gain for all EPDs at a given generation.
The replicator dynamic equation for developers and
EPDs are respectively expressed as Equations (5) and (6)
as following:
()/(1 )()
F
xdxdtx xSASy (5)
12 2
()/(1)[())]Gydy dtyy CCRCRx (6)
There are two situations to be considered for developers
according to Equation (5). One is that if ()/ySAS
(S ≥ A), thus F(x*) is 0, implying all x are trade-off solu-
tions, and another is that if ()/ySAS

 then x*
1 = 0
and x*
2 = 1 while if ()/ySAS the solution is x* 1=0
and if ()/ySAS then x*
2 = 1. Accordingly, there
are two situations to be considered for EPDs. One is that
if 21 2 ()/()
x
RC CCR  and (R + C2 = C1), then
G(y) = 0 implying all y are trade-off solutions, and an-
other is that if 21 2 ()/()
x
RC CCR, then y*
1 = 0
and y*
2 = 1, and when 21
RC C (21 ()/xRCC
2
()CR), then 10y otherwise If *
21y

, and
when 21 RC C(12 2 ()0CC RCRx ) then
**
12
()0,()0Gy Gy

 and *
21y. If denoting 0
x

21 2
()/(),RC CCR 0()/,ySAS

 and 00,x
00y, we can understand the changing evolutionary
ratios of developers and of EPDs (Figures 2-5).
Just as depicted in Figure 2 the strategies of EPDs and
developers mainly depends on each other’s choices of
strategies , and further analyses made about Figure 3 and
Figure 5 illustrates whether the fine is high or low the
Table 2. Payoff matrix by EPD and PGR-developers.
EPD
No-monitoring Monitoring
Manag. 0, 0 0, -C1
CPGR-Developer
Non-manag. A, -C2 A-S, R-C1
Figure 2. 21 ,SARC C.
Figure 3. 21 ,SARCC.
trade-off of ESS between EPDs and developers is x* = 0,
y* = 1. It means that the situation will arise when EPDs
find higher monitoring costs with low incentives or re-
wards from government, or alternatively, it will occur
when developers receive only small punishment with more
green risk resources discovered by EPDs. Once achieve-
ment of game evolutionary, EPDs have to accept the
non-monitor strategy and the developers accordingly take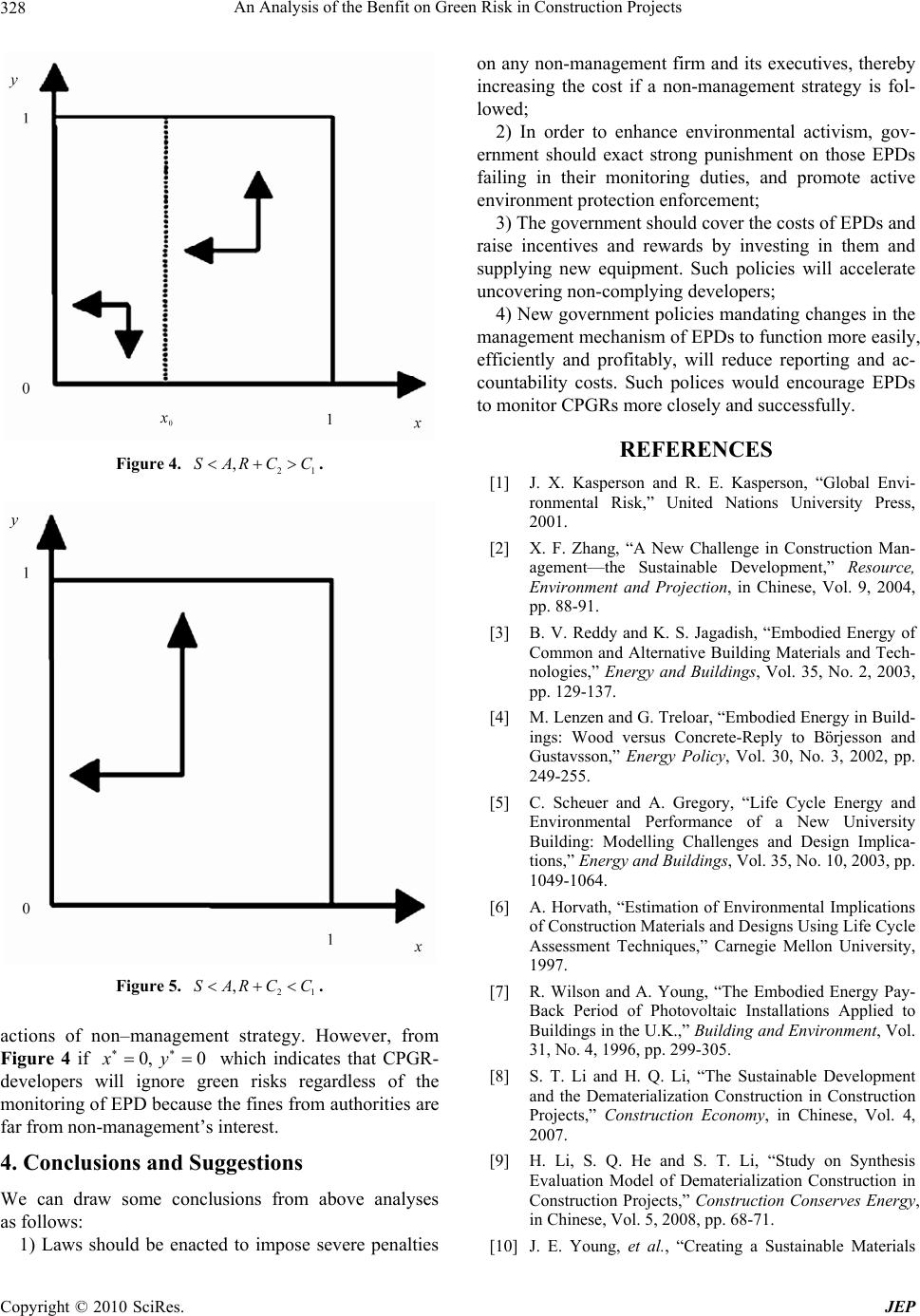

An Analysis of the Benfit on Green Risk in Construction Projects 328
Figure 4. 21 ,SARC C.
Figure 5. 21
,SARC C
.
actions of non–management strategy. However, from
Figure 4 if 0, 0xy

 which indicates that CPGR-
developers will ignore green risks regardless of the
monitoring of EPD because the fines from authorities are
far from non-management’s interest.
4. Conclusions and Suggestions
We can draw some conclusions from above analyses
as follows:
1) Laws should be enacted to impose severe penalties
on any non-management firm and its executives, thereby
increasing the cost if a non-management strategy is fol-
lowed;
2) In order to enhance environmental activism, gov-
ernment should exact strong punishment on those EPDs
failing in their monitoring duties, and promote active
environment protection enforcement;
3) The government should cover the costs of EPDs and
raise incentives and rewards by investing in them and
supplying new equipment. Such policies will accelerate
uncovering non-complying developers;
4) New government policies mandating changes in the
management mechanism of EPDs to function more easily,
efficiently and profitably, will reduce reporting and ac-
countability costs. Such polices would encourage EPDs
to monitor CPGRs more closely and successfully.
REFERENCES
[1] J. X. Kasperson and R. E. Kasperson, “Global Envi-
ronmental Risk,” United Nations University Press,
2001.
[2] X. F. Zhang, “A New Challenge in Construction Man-
agement—the Sustainable Development,” Resource,
Environment and Projection, in Chinese, Vol. 9, 2004,
pp. 88-91.
[3] B. V. Reddy and K. S. Jagadish, “Embodied Energy of
Common and Alternative Building Materials and Tech-
nologies,” Energy and Buildings, Vol. 35, No. 2, 2003,
pp. 129-137.
[4] M. Lenzen and G. Treloar, “Embodied Energy in Build-
ings: Wood versus Concrete-Reply to Börjesson and
Gustavsson,” Energy Policy, Vol. 30, No. 3, 2002, pp.
249-255.
[5] C. Scheuer and A. Gregory, “Life Cycle Energy and
Environmental Performance of a New University
Building: Modelling Challenges and Design Implica-
tions,” Energy and Buildings, Vol. 35, No. 10, 2003, pp.
1049-1064.
[6] A. Horvath, “Estimation of Environmental Implications
of Construction Materials and Designs Using Life Cycle
Assessment Techniques,” Carnegie Mellon University,
1997.
[7] R. Wilson and A. Young, “The Embodied Energy Pay-
Back Period of Photovoltaic Installations Applied to
Buildings in the U.K.,” Building and Environment, Vol.
31, No. 4, 1996, pp. 299-305.
[8] S. T. Li and H. Q. Li, “The Sustainable Development
and the Dematerialization Construction in Construction
Projects,” Construction Economy, in Chinese, Vol. 4,
2007.
[9] H. Li, S. Q. He and S. T. Li, “Study on Synthesis
Evaluation Model of Dematerialization Construction in
Construction Projects,” Construction Conserves Energy,
in Chinese, Vol. 5, 2008, pp. 68-71.
[10] J. E. Young, et al., “Creating a Sustainable Materials

An Analysis of the Benfit on Green Risk in Construction Projects 329
Economy,” State of the World, in Chinese, Vol. 6, 1995,
pp. 88-92.
[11] Federal Minister for the Environment, “Towards Sus-
tainable Development in Germany,” Government of the
Federal Republic of Germany, 1997.
[12] Y. H. Feng and Y. Q. Li, “Game Analyses of Environ-
ment Protection,” Journal of Xi’an University of Sci-
ence & Technology, in Chinese, Vol. 23, No. 4, 2003,
pp. 408- 410.
[13] W. Wu, G. Y. Cheng, H. C. Wang, et al., “The Gamble
Analysis on Environmental Pollution Problem,” Sys-
tems Engineering-Theory & Practice, in Chinese, Vol.
21, No. 10, 2001, pp. 115-119.
[14] D. M. Wang and W. Q. Li, “Application of Game The-
ory to Environmental Protection,” Urban Environment
& Urban Ecology, in Chinese, Vol. 17, No. 5, 2004, pp.
45-47.
[15] H. Gintis, “Game Theory Evolving,” Princeton Univer-
sity Press, Princeton, 2000.
[16] S. Y. Xie, “Economic Game Theory,” in Chinese, Fu-
dan University Press, Shanghai, 2002.