American Journal of Computational Mathematics, 2012, 2, 341-344
http://dx.doi.org/10.4236/ajcm.2012.24047 Published Online December 2012 (http://www.SciRP.org/journal/ajcm)
A New Block-Predictor Corrector Algorithm for the
Solution of


,, ,

 
yf
x
yy y

Adetola O. Adesanya1, Mfon O. Udo2, Adam M. Alkali1
1Department of Mathematics, Modibbo Adama University of Technology, Yola, Nigeria
2Department of Mathematics and Statistics, Cross River University of Technology, Calabar, Nigeria
Received May 10, 2012; revised September 16, 2012; accepted September 25, 2012
ABSTRACT
We consider direct solution to third order ordinary differential equations in this paper. Method of collection and inter-
polation of the power series approximant of single variable is considered to derive a linear multistep method (LMM)
with continuous coefficient. Block method was later adopted to generate the independent solution at selected grid points.
The properties of the block viz: order, zero stability and stability region are investigated. Our method was tested on
third order ordinary differential equation and found to give better result when compared with existing methods.
Keywords: Collection; Interpolation; Power Series; Approximant; Linear Multistep; Continuous Coefficient; Block
Method
1. Introduction
This paper considers the general third order initial value
problems of the form


 
00
00 00
,, ,,,
,.
yfxyyyyx y
yx yyx y
 

 
 (1)
Conventionally, higher order ordinary differential equ-
ations are solved directly by the predictor-corrector me-
thod where separate predictors are developed to im-
plement the correct and Taylor series expansion adopted
to provide the starting values. Predictor-corrector me-
thods are extensively studied by [1-5]. These authors
proposed linear multistep methods with continuous co-
efficient, which have advantage of evaluation at all
points within the grid over the proposed method in [6]
The major setbacks of predictor-corrector method are
extensively discussed by [7].
Lately, many authors have adopted block method to
solve ordinary differential equations because it addresses
some of the setbacks of predictor-corrector method
discussed by [6]. Among these authors are [8-10].
According to [6], the general block formula is given
by


.
mn nm
yhyhy

 Y edfbF

(2)
where is e
s
s vector, is r-vector and is
vector,
d b
rr 
s
is the interpolation points and is the
collection points.
r
F
is a k-vector whose entry is thj


,, njnj nj
ffty



is the order of the differential
equation.
Given a predictor equation in the form

0

mn n
yh y

df. (3) Ye
Putting (3) in (2) gives



.
nn n
y hyhy
 
 bFe df
mn
yhYe df

(4)
Equation (4) is called a self starting block-predictor-
corrector method because the prediction equation is
gotten directly from the block formula as claimed by
[11,12].
In this paper, we propose an order six block method
with step length of four using the method proposed by
[11] for the solution of third order ordinary differential
equation.
2. Methodology
2.1. Derivation of the Continuous Coefficient
We consider monomial power series as our basis fun-
ction in the form

1
0
.
sr
j
j
j
y
xa


x
.
(5)
The third derivative of (5) gives

yx

 
1
3
3
12
j
j
jjj ax
sr
j



  (6)
C
opyright © 2012 SciRes. AJCM

A. O. ADESANYA ET AL. 342
The solution to (1) is soughted on the partition π
N
:
012 1nnN within the
integration interval [a,b] with constant step length
given as
axx xxxxb



,01.
ni n
hx xiN

 h
Substituting (6) into (1) gives


 

 
1
3
=3
,, ,
12
sr j
j
j
fxyxyx y x
jjj ax
 


.
;
,
(7)
Interpolating (5) at collocating (7)
gives a system of equations

,113
ni
xj


1sr

,014
ni
xj

0
.
j
j
ns
j
ax y




(8)

 
1
3
3
12
sr j.
j
nr
j
jjj axf
 


 
 (9)
Solving (8) and (9) for
j
a’s and substituting back into
(5) gives a LMM with continuous coefficients of the
form
 
34
3
1
10
.
tt
j
nj
jj
yh f 




nj
(10)
where
j
a’s and
j

’s are given as






22
12
2
3
1
=;=
2
1
=32;
2
tttttt
ttt






76 542
0
12 71135 49 26;
10080
t tttttt  


7654 2
1
1
=421141012652 ;
5040
tttttt    t


765 42
2
12147105532432 ;
1680
tttttt   t


3
765 432
1
5040
435701758401078452 ;
t
ttt tttt
 
  


765 4 3
4
1221 77 105105 58.
10080
tttttt   t
where 3.
n
xx
th



2.2. Derivation of the Block Method
The general block formula proposed by [6] in the nor-
malized form is given by

 
0.
mn nm
yhy hy
  
 AY edfbF (11)
2;
Evaluating (10) at 1,0,4
n
xx i
;



,014;
ni
xi

the first and
second derivative at and substituting
into (10) gives the coefficient of (11) as
T
113331 14312483675314756251292514,
1120 63011201051440 90160 45 720 90 80 45



d
T
111100000000
123411110000 ,
9 12 812341111
22







e
012 12
A
 identity matrix,
107332 1863 21763811764232625164
10083155603158540153604540 45
1038243 3247271698 1114
1680215601052403 801530 1510 15
435245128 398364 5364 221
168 31511210536045 845360454045
47 1981871
10080 63011206348030
b  

T
.
9197
11
0
30 160720 12045
 
 
 
 
 
 
 
 

 
 
4
3. Analysis of the Properties of the Block
3.1. Order of the Method
We define a linear operator on the block (11) to give
  


:mm mm yx hyhyhy
  
 Ye dfbF(12)
Expanding

n

y
xih and

n

f
xjh in Taylor
series , (12) gives






 
01
2
2
:
pp
p
yxhCyxChyx
ChyxCh yx





(13)
The block (11) and associated linear operator are said
to have order if p011 2 0, 0
pp
CCC C


 .

A. O. ADESANYA ET AL. 343
The term 2p is called the error constant and implies
that the local truncation error for the block is given by
C
nk



22 3
20
pp p
p n
tChyxh
 




(14)
Hence the block (11) has order 6, with error
constant

62
0h
2
13
40
224



, 

zz
91243 321078
320 45 448031510080 315
916313 8
315 16090 160945
p
C



N
E
3.2. Zero Stability of the Block
The block (11) is said to be zero stable if the roots
of the characteristic polynomial
satisfies
1, 2,
s
z

det 

,A1z and the root
1z has multiplicity not exceeding the order of the
differential equation. moreover as

hz
 
0,1 ,
r
z


 


 where

is the order of
the differential equation,


0
dimrA
For the block (11), 12,3r


 
3
91z
 
Hence our method is zero stable.
3.3. Convergence
A method is said to be convergent if it is zero stable and
has order .
1p
From the theorem above, our method is convergent.
4. Numerical Experiments
4.1. Test Problem
We test our schemes with third order initial value pro-
blems:
Problem 1. Consider a special third order initial value
problem
3sinyx







01, 0 0,0201
y
yy x



Exact solution:

2
3cos2
2
x
yxx 




This problem was solved by [13] using self-starting
predictor-corrector method for special third order diffe-
rential equations where a scheme of order six was pro-
posed.
Problem 2. Consider a linear third order initial value
problem
0yy

 





 

01, 01,01,0,1yy yx
 
 
Exact solution:


21 cossin
y
xx x
This problem was solved by [14] where a method of
order six was proposed. They adopted predictor corrector
method in their implementation. Our result is shown in
Table 1.
4.2. Numerical Results
The following notations are used in the table.
XVAL: Value of the independent variable where nu-
merical value is taken;
ERC: Exact result at XVAL;
NRC: Numerical result of the new result at XVAL;
ERR: Magnitude of error of the new result at XVAL.
5. Discussion
We have proposed a new block method for solving third
order initial value problem in this paper. It should be
noted that the method performs better when the step-size
is chosen within the stability interval. The Tabl e s 1 and 2
had shown our new method is more efficient in terms of
accuracy when compared with the self starting predictor
Table 1. Showing result of pr oble m 1, h = 0.01.
XVAL ERC NRC NRC ERR IN [13]
0.1 0.990012495834077 0.990012495834077 0.0000+00 9.992007(–16)
0.2 0.960199733523725 0.960199733523724 9.99200(–16) 7.660538(–15)
0.3 0.911009467376818 0.911009467376816 1.55431(–15) 2.287059(–14)
0.4 0.843182982008655 0.843182982008652 3.10862(–15) 5.906386(–14)
0.5 0.757747685671118 0.757747685671113 4.66293(–15) 1.153521(–13)
0.6 0.656006844729035 0.656006844729028 6.88338(–15) 1.982858(–13)
0.7 0.539526561853465 0.539526561853456 9.10382(–15) 3.127498(–13)
0.8 0.410120128041496 0.410120128041484 1.14908(–14) 4.635736(–13)
0.9 0.269829904811992 0.269829904811978 1.42108(–14) 6.542544(–13)
1.0 0.120906917604418 0.120906917604401 1.74582(–14) 8.885253(–13)

A. O. ADESANYA ET AL. 344
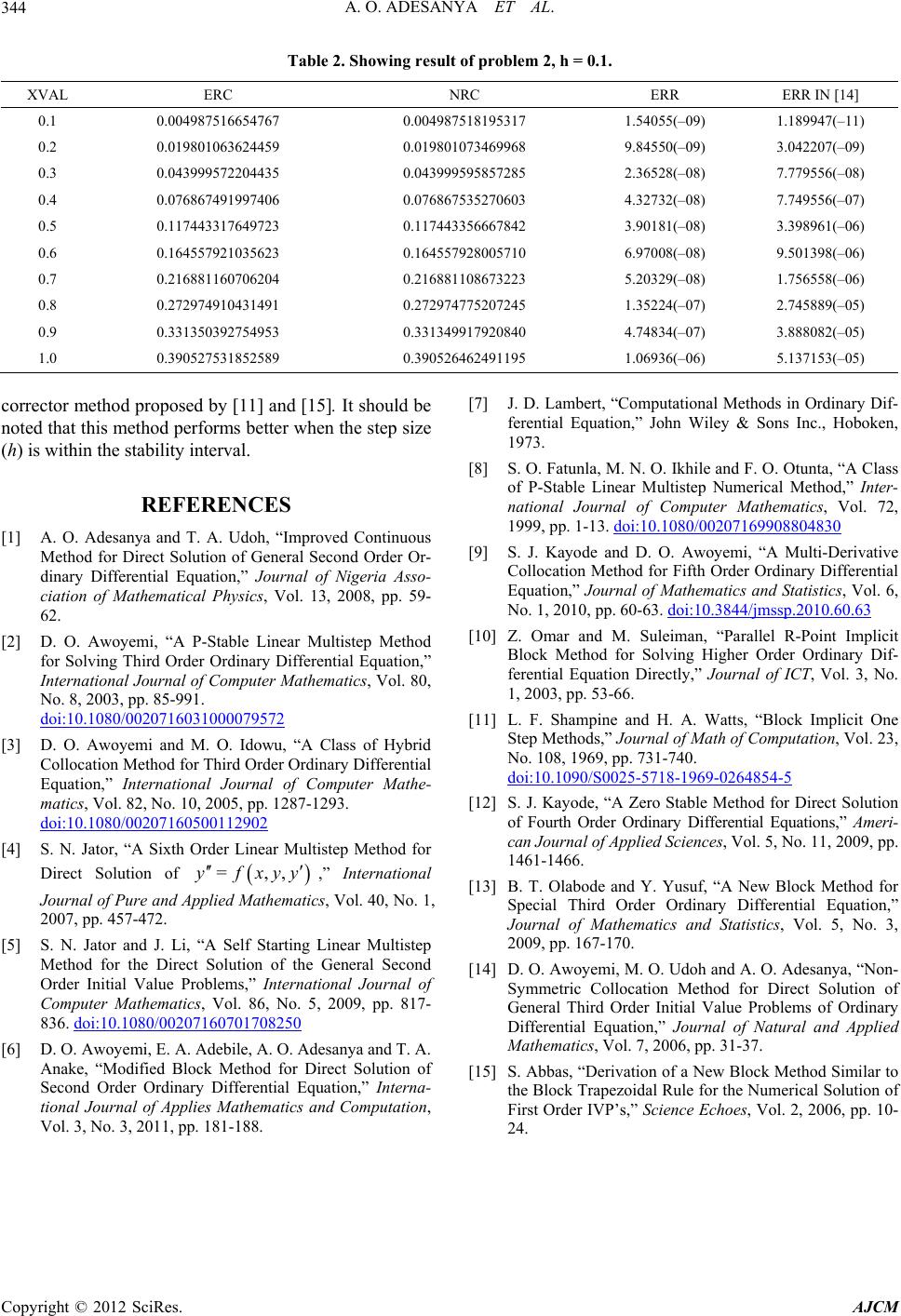
Table 2. Showing result of pr oble m 2, h = 0.1.
XVAL ERC NRC ERR ERR IN [14]
0.1 0.004987516654767 0.004987518195317 1.54055(–09) 1.189947(–11)
0.2 0.019801063624459 0.019801073469968 9.84550(–09) 3.042207(–09)
0.3 0.043999572204435 0.043999595857285 2.36528(–08) 7.779556(–08)
0.4 0.076867491997406 0.076867535270603 4.32732(–08) 7.749556(–07)
0.5 0.117443317649723 0.117443356667842 3.90181(–08) 3.398961(–06)
0.6 0.164557921035623 0.164557928005710 6.97008(–08) 9.501398(–06)
0.7 0.216881160706204 0.216881108673223 5.20329(–08) 1.756558(–06)
0.8 0.272974910431491 0.272974775207245 1.35224(–07) 2.745889(–05)
0.9 0.331350392754953 0.331349917920840 4.74834(–07) 3.888082(–05)
1.0 0.390527531852589 0.390526462491195 1.06936(–06) 5.137153(–05)
corrector method proposed by [11] and [15]. It should be
noted that this method performs better when the step size
(h) is within the stability interval.
REFERENCES
[1] A. O. Adesanya and T. A. Udoh, “Improved Continuous
Method for Direct Solution of General Second Order Or-
dinary Differential Equation,” Journal of Nigeria Asso-
ciation of Mathematical Physics, Vol. 13, 2008, pp. 59-
62.
[2] D. O. Awoyemi, “A P-Stable Linear Multistep Method
for Solving Third Order Ordinary Differential Equation,”
International Journal of Computer Mathematics, Vol. 80,
No. 8, 2003, pp. 85-991.
doi:10.1080/0020716031000079572
[3] D. O. Awoyemi and M. O. Idowu, “A Class of Hybrid
Collocation Method for Third Order Ordinary Differential
Equation,” International Journal of Computer Mathe-
matics, Vol. 82, No. 10, 2005, pp. 1287-1293.
doi:10.1080/00207160500112902
[4] S. N. Jator, “A Sixth Order Linear Multistep Method for
Direct Solution of

=,,
y
fxyy
 ,” International
Journal of Pure and Applied Mathematics, Vol. 40, No. 1,
2007, pp. 457-472.
[5] S. N. Jator and J. Li, “A Self Starting Linear Multistep
Method for the Direct Solution of the General Second
Order Initial Value Problems,” International Journal of
Computer Mathematics, Vol. 86, No. 5, 2009, pp. 817-
836. doi:10.1080/00207160701708250
[6] D. O. Awoyemi, E. A. Adebile, A. O. Adesanya and T. A.
Anake, “Modified Block Method for Direct Solution of
Second Order Ordinary Differential Equation,” Interna-
tional Journal of Applies Mathematics and Computation,
Vol. 3, No. 3, 2011, pp. 181-188.
[7] J. D. Lambert, “Computational Methods in Ordinary Dif-
ferential Equation,” John Wiley & Sons Inc., Hoboken,
1973.
[8] S. O. Fatunla, M. N. O. Ikhile and F. O. Otunta, “A Class
of P-Stable Linear Multistep Numerical Method,” Inter-
national Journal of Computer Mathematics, Vol. 72,
1999, pp. 1-13. doi:10.1080/00207169908804830
[9] S. J. Kayode and D. O. Awoyemi, “A Multi-Derivative
Collocation Method for Fifth Order Ordinary Differential
Equation,” Journal of Mathematics and Statistics, Vol. 6,
No. 1, 2010, pp. 60-63. doi:10.3844/jmssp.2010.60.63
[10] Z. Omar and M. Suleiman, “Parallel R-Point Implicit
Block Method for Solving Higher Order Ordinary Dif-
ferential Equation Directly,” Journal of ICT, Vol. 3, No.
1, 2003, pp. 53-66.
[11] L. F. Shampine and H. A. Watts, “Block Implicit One
Step Methods,” Journal of Math of Computation, Vol. 23,
No. 108, 1969, pp. 731-740.
doi:10.1090/S0025-5718-1969-0264854-5
[12] S. J. Kayode, “A Zero Stable Method for Direct Solution
of Fourth Order Ordinary Differential Equations,” Ameri-
can Journal of Applied Sciences, Vol. 5, No. 11, 2009, pp.
1461-1466.
[13] B. T. Olabode and Y. Yusuf, “A New Block Method for
Special Third Order Ordinary Differential Equation,”
Journal of Mathematics and Statistics, Vol. 5, No. 3,
2009, pp. 167-170.
[14] D. O. Awoyemi, M. O. Udoh and A. O. Adesanya, “Non-
Symmetric Collocation Method for Direct Solution of
General Third Order Initial Value Problems of Ordinary
Differential Equation,” Journal of Natural and Applied
Mathematics, Vol. 7, 2006, pp. 31-37.
[15] S. Abbas, “Derivation of a New Block Method Similar to
the Block Trapezoidal Rule for the Numerical Solution of
First Order IVP’s,” Science Echoes, Vol. 2, 2006, pp. 10-
24.