J. BHATT ET AL.
298
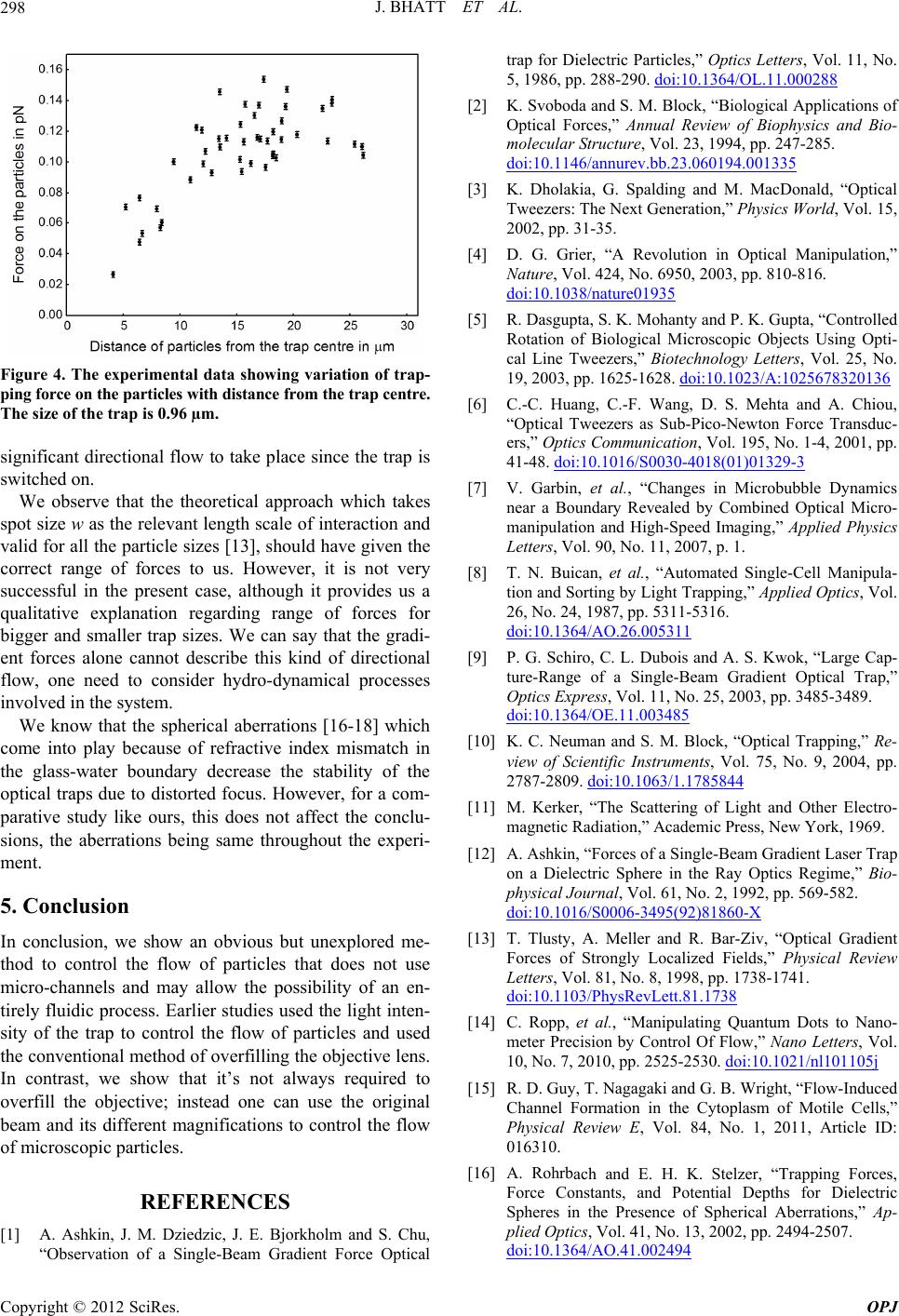
Figure 4. The experimental data showing variation of trap-
ping force on the particles with distance from the trap centre.
The size of the trap is 0.96 µm.
significant directional flow to take place since the trap is
switched on.
We observe that the theoretical approach which takes
spot size w as the relevant length scale of interaction and
valid for all the particle sizes [13], should have given the
correct range of forces to us. However, it is not very
successful in the present case, although it provides us a
qualitative explanation regarding range of forces for
bigger and smaller trap sizes. We can say that the gradi-
ent forces alone cannot describe this kind of directional
flow, one need to consider hydro-dynamical processes
involved in the system.
We know that the spherical aberrations [16-18] which
come into play because of refractive index mismatch in
the glass-water boundary decrease the stability of the
optical traps due to distorted focus. However, for a com-
parative study like ours, this does not affect the conclu-
sions, the aberrations being same throughout the experi-
ment.
5. Conclusion
In conclusion, we show an obvious but unexplored me-
thod to control the flow of particles that does not use
micro-channels and may allow the possibility of an en-
tirely fluidic process. Earlier studies used the light inten-
sity of the trap to control the flow of particles and used
the conventional method of overfilling the objective lens.
In contrast, we show that it’s not always required to
overfill the objective; instead one can use the original
beam and its different magnifications to control the flow
of microscopic particles.
REFERENCES
[1] A. Ashkin, J. M. Dziedzic, J. E. Bjorkholm and S. Chu,
trap for Dielectric Particles,” Optics Letters, Vol. 11, No.
5, 1986, pp. 288-290.
“Observation of a Single-Beam Gradient Force Optical
doi:10.1364/OL.11.000288
[2] K. Svoboda and S. M. Block, “Biological Applications of
Optical Forces,” Annual Review of Biophysics and Bio-
molecular Structure, Vol. 23, 1994, pp. 247-285.
doi:10.1146/annurev.bb.23.060194.001335
[3] K. Dholakia, G. Spalding and M. MacDonald, “Optical
Revolution in Optical Manipulation,”
Tweezers: The Next Generation,” Physics World, Vol. 15,
2002, pp. 31-35.
[4] D. G. Grier, “A
Nature, Vol. 424, No. 6950, 2003, pp. 810-816.
doi:10.1038/nature01935
[5] R. Dasgupta, S. K. Mohanty and P. K. Gupta, “Controlled
Rotation of Biological Microscopic Objects Using Opti-
cal Line Tweezers,” Biotechnology Letters, Vol. 25, No.
19, 2003, pp. 1625-1628. doi:10.1023/A:1025678320136
[6] C.-C. Huang, C.-F. Wang, D. S. Mehta and A. Chiou,
“Optical Tweezers as Sub-Pico-Newton Force Transduc-
ers,” Optics Communication, Vol. 195, No. 1-4, 2001, pp.
41-48. doi:10.1016/S0030-4018(01)01329-3
[7] V. Garbin, et al., “Changes in Microbubble Dynamics
ingle-Cell Manipula-
near a Boundary Revealed by Combined Optical Micro-
manipulation and High-Speed Imaging,” Applied Physics
Letters, Vol. 90, No. 11, 2007, p. 1.
[8] T. N. Buican, et al., “Automated S
tion and Sorting by Light Trapping,” Applied Optics, Vol.
26, No. 24, 1987, pp. 5311-5316.
doi:10.1364/AO.26.005311
[9] P. G. Schiro, C. L. Dubois and A. S. Kwok, “Large Cap-
ture-Range of a Single-Beam Gradient Optical Trap,”
Optics Express, Vol. 11, No. 25, 2003, pp. 3485-3489.
doi:10.1364/OE.11.003485
[10] K. C. Neuman and S. M. Block, “Optical Trapping,” Re-
view of Scientific Instruments, Vol. 75, No. 9, 2004, pp.
2787-2809. doi:10.1063/1.1785844
[11] M. Kerker, “The Scattering of Light and Other Electro-
magnetic Radiation,” Academic Press, New York, 1969.
[12] A. Ashkin, “Forces of a Single-Beam Gradient Laser Trap
on a Dielectric Sphere in the Ray Optics Regime,” Bio-
physical Journal, Vol. 61, No. 2, 1992, pp. 569-582.
doi:10.1016/S0006-3495(92)81860-X
[13] T. Tlusty, A. Meller and R. Bar-Ziv, “Optical Gradient
Forces of Strongly Localized Fields,” Physical Review
Letters, Vol. 81, No. 8, 1998, pp. 1738-1741.
doi:10.1103/PhysRevLett.81.1738
[14] C. Ropp, et al., “Manipulating Quantum Dots to Nano-
meter Precision by Control Of Flow,” Nano Letters, Vol.
10, No. 7, 2010, pp. 2525-2530. doi:10.1021/nl101105j
[15] R. D. Guy, T. Nagagaki and G. B. Wright, “Flow-Induced
ach and E. H. K. Stelzer, “Trapping Forces,
Channel Formation in the Cytoplasm of Motile Cells,”
Physical Review E, Vol. 84, No. 1, 2011, Article ID:
016310.
[16] A. Rohrb
Force Constants, and Potential Depths for Dielectric
Spheres in the Presence of Spherical Aberrations,” Ap-
plied Optics, Vol. 41, No. 13, 2002, pp. 2494-2507.
doi:10.1364/AO.41.002494
Copyright © 2012 SciRes. OPJ