Communications and Network, 2010, 2, 166-182
doi:10.4236/cn.2010.23025 Published Online August 2010 (http://www.SciRP.org/journal/cn)
Comparison of 4 Multi-User Passive Network Topologies
for 3 Different Quantum Key Distribution
Fabio Garzia, Roberto Cusani
INFOCOM Department, SAPIENZA – Universi t y of Rome , Rome, Italy
Received March 30, 2010; revised May 7, 2010; accepted J une 1, 2010
Abstract
The purpose of this paper is to compare the performance of four passive optical network topologies in im-
plementing multi-user quantum key distribution, using 3 protocols proposed by quantum cryptography (B92,
EPR, and SSP). The considered networks are the passive-star network, the optical-ring network based on the
Signac interferometer, the wavelength-routed network, and the wavelength-addressed bus network. The
quantum bit-error rate and sifted key rate for each of these topologies are analysed to determine their suit-
ability for providing quantum key distribution-service to networks of various sizes. The efficiency of the
three considered protocols is also determined.
Keywords: Quantum Cryptography, Quantum Key Distribution
1. Introduction
The quantum cryptography term repres ents the s et of the
techniques which allow two entities, Alice and Bob, to
exchange reserved information by means of a quantum
channel. A qu antum ch anne l is an op tic a l channel gover ned
by the quantum mechanics. The job in cryptographic
field of the quantum mechanics allows results impossible
to be obtained with the only mathematics. More precisely,
talking about quantum key distribution (QKD) is oppor-
tune: the quantum channel is used to transmit a sequence
of bits, well known only to Alice and Bob and then able
to constitute the secret key of a cryptographic system.
Therefore the next communications which are ciphered
with such key can be made on a conventional channel
(not quantum). Quantum key distribution is a method for
securely distributing one-time-use encryption keys that
are used for secure communications. These quantum
systems are based on the theorem of Heisenberg [1],
according to which the measurement of a quantum sys-
tem generally perturbs it and gives an incomplete piece
of information on his state preceding the measurement,
and on the quantum no-cloning theorem [2], which for-
bids the perfect copying of two non-orthogonal quantum
states.
Therefore the quantum nature of a channel makes sure
that any interception is noticed. Hence an eavesdropper,
Eve, cannot get any information about the communica-
tion without i ntroducing pertur bati ons whic h would reveal
her presence.
To share a secret key, Alice and Bob must follow a
protocol (BB84, B92, EPR, SSP). Once developed the
procedure requested by the protocol, if any eavesdropper
were not noticed, Alice and Bob share a secret key,
which exchanged themselves without having to turn to a
third reliable part and initially sharing no information,
except that the one necessary to authenticate their com-
munications part. The frequency used by Alice and Bob
to share the sifted secret key is denominated sifted key
rate (RSIFT). To reveal the presence of an eavesdropper,
Eve, Alice and Bob monitor the quantum bit error rate
(QBER). If the QBER exceeds a certain threshold the
made communication is just considered as not safe and
therefore the secret key is discarded. The security
threshold depends on the used protocol. The QBER and
the RSIFT are considered the fundamental parameters to
evaluate the performances of a quantum channel. This
analysis has already been done for BB84 protocol [3].
The purpose of this paper is to extend the mentioned
analysis to other three common protocols that are B92,
EPR and SSP.
The remainder of this paper is organized as follows.
Section II provides a review of 3 protocols used in addi-
tion to BB84 protocol [3]. Section III outlines the four
network topologies to be compared. The security thresh-
old for every used protocol is determined in the Section
IV. Section V provides a review of the physical princi-
ples used for the simulations for each protocol. The re-

F. GARZIA ET AL.167
sults of the comparison of the networks are presented in
Sections 7 and 8, after having reported in Section 6 the
parameters values employed. This is followed by a re-
sults discussion, Section 4, and conclusions, Section5.
2. Protocols
2.1. BB84: First QKD Protocol
The first protocol has been proposed in 1984 by Charles
H. Bennett and Gilles Brassard [4], hence the name
BB84 unde r w hi c h t hi s pr ot o c ol is recognized nowa day s .
Alice wants to communicate Bob a bit sequence
(qubits). The qubits are encoded with polarized photons.
The protocol uses 4 polarization states: 0°, 90°, +45°,
–45°.
These states are represented in the following way:
horizontal |H> , vertical |V>, |45°> and |-45°>, where
H≡0° and V≡90°. This states are assembled in 2
non-orthogonal basis : rectilinear (|H> ; |V>) and diago-
nal (|+45°> ; |–45°>). The bases are maximally conjugate
in the sense that any pair of vectors, one from each basis,
has the same overlap: 1/2 .
Conventionally, one attributes the binary value 0 to
states |H> and |45°>| and the value 1 to the other two
states. In the first step, Alice sends individual photon to
Bob in states chosen at random between the 4 basic
states. Next, Bob measures the incoming photons in one
of the two bases, chosen at random. If both Alice and
Bob choose the same random basis, then Bob’s meas-
urements have a deterministic outcome. If they do not
choose the same basis, the outcome of his measurement
becomes probabilistic. Once made all the measurements,
Bob obtains a bit sequence said raw key. In the second
step, Alice and Bob communicate over a public channel
to compare the bases in which the qubits were encoded
and measured. The qubits that are sent and measured in
incompatible bases are discarded. The remaining qubits
shared between Alice and Bob form the sifted keys.
2.2. B92
In an article of 1992 Charles Bennett proposed a new
protocol, B92 [5,6].
The B92 quantum coding scheme is similar to the
BB84 coding scheme but used only 2 out of the 4 BB84
states. It encodes classical bits in two non-orthogonal
BB84 states. Since no measurement can distinguish two
non-orthogonal quantum states, it is impossible to iden-
tify the bit with certainty. Moreover, any atte mpt to learn
the bit will modify the state in a noticeable way. This is
the basic idea behind the quantum key distribution pro-
tocol B92. By contrast to the BB84 case, the B92 coding
scheme allows the receiver to learn whenever he gets the
bit sent without further discussion with Alice. Since it
uses only 2 quantum states, the B92 coding scheme is
sometimes easier to implement. However, the security it
provides is more difficult to be established in certain
experimental settings and very often turns out to be
to tally in secu re. Th e polar izatio n en code d version of B92
proceeds as follows for an idealized system.
Both the transmitter “Alice” and the receiver “Bob”
generate an independent random bit sequence. Alice then
transmits her random bit sequence t o Bob using a clocked
sequence of linearly polarized individual photons with
polarization angles chosen according to her bit values as
given by 0° ≡ 0 and 45° ≡ 1. In each time period, Bob
makes a polarization measurement on an incoming pho-
ton by orientating the transmission axis of his polarizer
according to his bit value as given by –45° ≡ 0 and 90° ≡
1. It can be seen that Bob detect only a photon (with
probability one half) in the time slots where h is polarizer
is not crossed with that of Alice. We refer to these in-
stances as “unambiguous” since when they occur, Alice
and Bob can be sure that their polarization settings were
not orthogonal and, consequently, that their bit values
were the same (both 0 or both 1). Conversely, the
instances in which Bob receives no photon are referred
to as “ambiguous” since they can arise either from the
cases where Alice’s and Bob’s polarisers were crossed or
from the cases where the polarisers were not crossed, but
Bob failed (with probability 1 /2) to detect a photon. Bob
then uses an authen ticated public ch ann el to info rm Alice
of the time slots in which he obtained an unambiguous
result (1/4 on average) and they use the shared subset of
their initial random bit sequences represented by these
time slots as a key.
In this protocol, whose used values are shown in Ta-
ble 1, we see that for the first and fourth bits Alice and
Bob had different bit values, so that Bob doesn’t detect
any bit in each case. However, for the second and third
bits, Alice and Bob have the same bit values and the
protocol is such that there is a probability of 50% that
Bob detects a bit in each case. Of course, we cannot pre-
dict in which of the two cases Bob detects the bit, but in
this example he detects only third bit.
The B92 protocol is intrinsically less efficient than the
given BB84 that, also in ideal conditions (when no bit of
the raw key is to be deleted), only 1/4 of the impulses
gives a key bits, whil e wi th B B 84 p rot ocol fraction is 1/ 2.
Table 1. An example of B92 protocol.
Alice’s sequence 1 0 1 0
Alice’s polarization +45° 0° +45°0°
Bob’s polarization -45° -45° 90° 90°
Bob’s sequence 0 0 1 1
Bob’s bit detected No No Yes No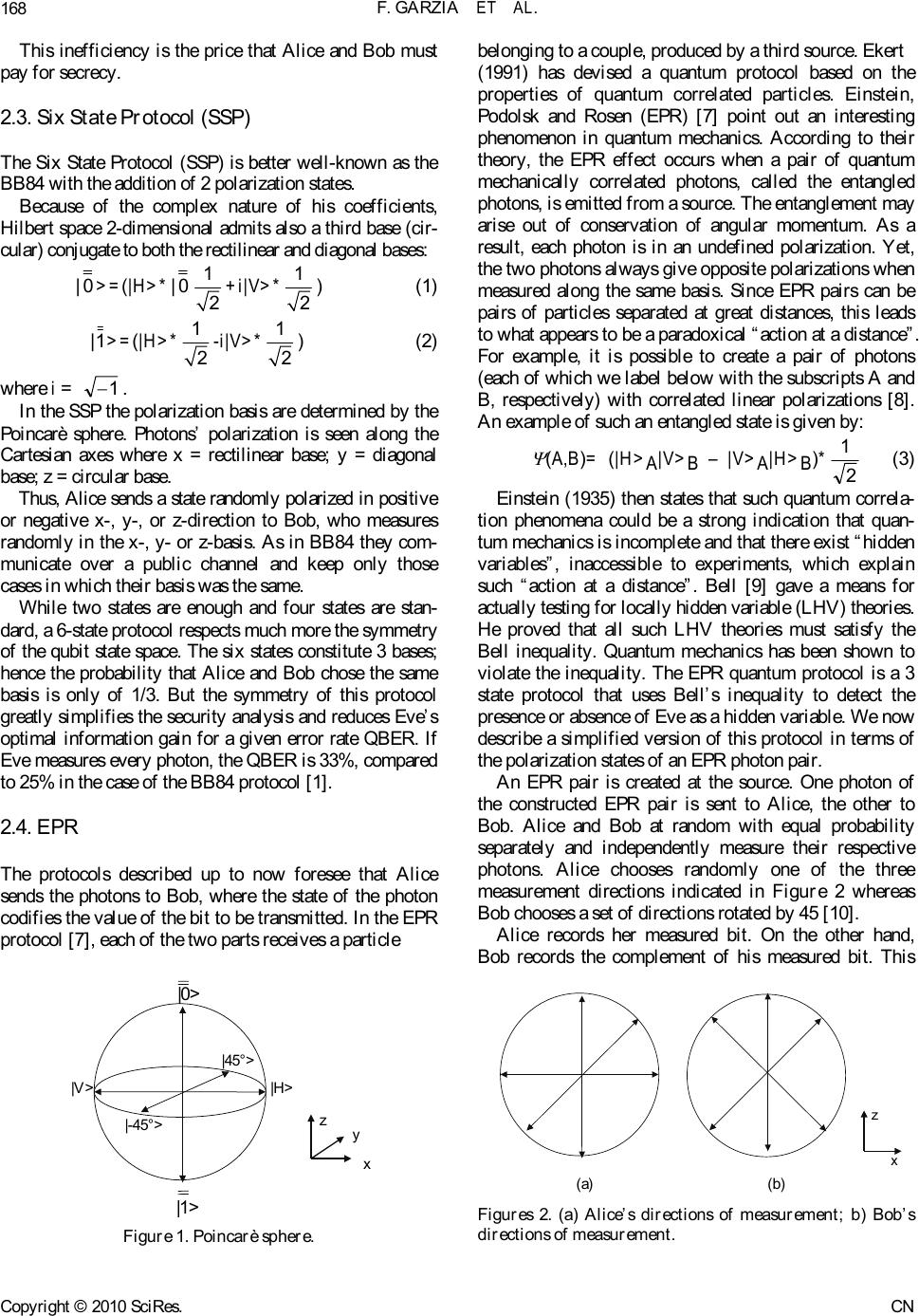

F. GARZIA ET AL. 168
This inefficiency is the price that Alice and Bob must
pay for secrecy.
2.3. Six State Protocol (SSP)
The Six State Protocol (SSP) is better well-known as the
BB84 with the addi t i on of 2 polarization states.
Because of the complex nature of his coefficients,
Hilbert space 2-dimensional admits also a third base (cir-
cular) conjugate to both the rectilinear and diagonal bases:
|0>=(|H>*|0 1
2+i|V>* 1 2) (1)
1| >=(|H>* 1
2-i|V>* 1 2) (2)
where i = 1.
In the SSP the polarization basis are determined by the
Poincarè sphere. Photons’ polarization is seen along the
Cartesian axes where x = rectilinear base; y = diagonal
base; z = circular base.
Thus, Alice sends a state randomly polarized in positive
or negative x-, y-, or z-direction to Bob, who measures
randomly in the x-, y- or z-basis. As in BB84 they com-
municate over a public channel and keep only those
cases in which their basis was the same.
While two states are enough and four states are stan-
dard, a 6-state protocol respects much more the symmetry
of the qubit state space. The six states constitute 3 bases;
hence the probability that Alice and Bob chose the same
basis is only of 1/3. But the symmetry of this protocol
greatly simplifies the security analysis and reduces Eve’s
optimal information gain for a given error rate QBER. If
Eve measures every photon, the QBER is 33%, compared
to 25% in the case of the BB8 4 protocol [ 1].
2.4. EPR
The protocols described up to now foresee that Alice
sends the photons to Bob, where the state of the photon
codifies the value of the bit to be transmitted. In the EPR
protocol [7], each of the two parts receives a particle
|H>|V>
|0>
|1>
|-45°>
|45°>
z
x
y
Figure 1. Poincarè sphere.
belonging to a couple, produc ed by a third sou rce. Ekert
(1991) has devised a quantum protocol based on the
properties of quantum correlated particles. Einstein,
Podolsk and Rosen (EPR) [7] point out an interesting
phenomenon in quantum mechanics. According to their
theory, the EPR effect occurs when a pair of quantum
mechanically correlated photons, called the entangled
photons, is emitted from a source. The entanglement may
arise out of conservation of angular momentum. As a
result, each photon is in an undefined polarization. Yet,
the two photons always give opposite polar izations when
measured along the same basis. Since EPR pairs can be
pairs of particles separated at great distances, this leads
to what appears to be a paradoxical “action at a distance”.
For example, it is possible to create a pair of photons
(each of which we label below with the subscripts A and
B, respectively) with correlated linear polarizations [8].
An example of such an entangled state is given by:
 (A,B)= (|H>A|V>B – |V>A|H>B)*2
1 (3)
Einstein (1935) then states that such quantum correla-
tion phenomena could be a strong indication that quan-
tum mechanics is incomplete and that there exist “hidden
variables”, inaccessible to experiments, which explain
such “action at a distance”. Bell [9] gave a means for
actually tes ting for local ly hidden variab le (LHV) theor i es .
He proved that all such LHV theories must satisfy the
Bell inequality. Quantum mechanics has been shown to
violate the inequality. The EPR quantum protocol is a 3
state protocol that uses Bell’s inequality to detect the
presence or absence of Eve as a hidden variable. We now
describe a simplified version of this protocol in terms of
the polarization states of an EPR photon pair.
An EPR pair is created at the source. One photon of
the constructed EPR pair is sent to Alice, the other to
Bob. Alice and Bob at random with equal probability
separately and independently measure their respective
photons. Alice chooses randomly one of the three
measurement directions indicated in Figure 2 whereas
Bob chooses a set of directions rotated by 45 [10].
Alice records her measured bit. On the other hand,
Bob records the complement of his measured bit. This
z
x
(a) (b)
Figures 2. (a) Alice’s directions of measurement; b) Bob’s
directions of measurement.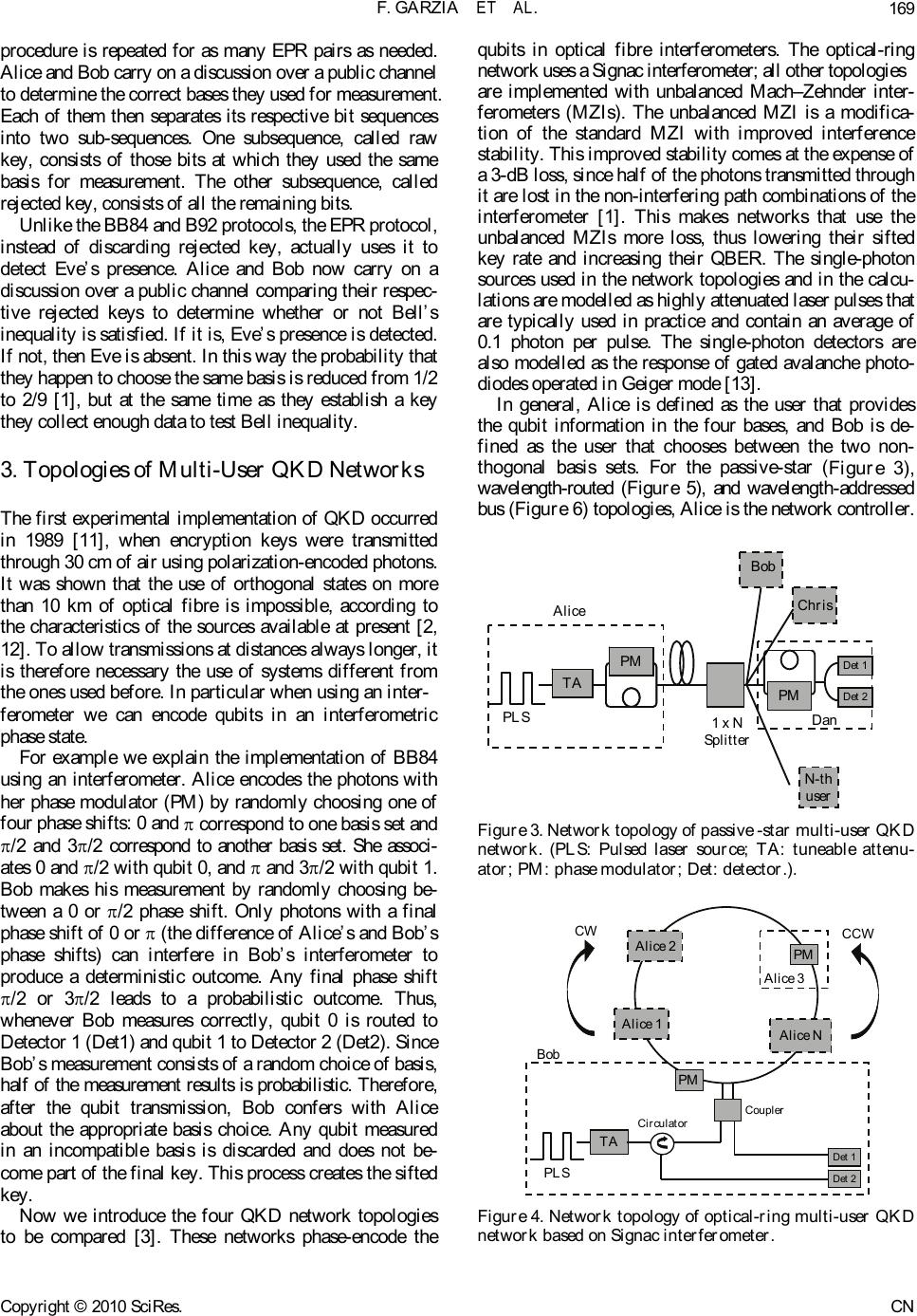

F. GARZIA ET AL.169
procedure is repeated for as many EPR pairs as needed.
Alice and Bob carry on a discussion over a public channel
to determine the correct bases they used for measurement.
Each of them then separates its respective bit sequences
into two sub-sequences. One subsequence, called raw
key, consists of those bits at which they used the same
basis for measurement. The other subsequence, called
rejected key, consists of all the remaining bits.
Unlike the BB84 and B92 protocols, the EPR protocol,
instead of discarding rejected key, actually uses it to
detect Eve’s presence. Alice and Bob now carry on a
discussion over a public channel comparing their respec-
tive rejected keys to determine whether or not Bell’s
inequality is satisfied. If it is, Eve’s presence is detected.
If not, then Eve is absent. In this way the probability that
they happen to choose the same b asis is r educed from 1/2
to 2/9 [1], but at the same time as they establish a key
they collect enough data to test Bell inequality.
3. Topologies of Multi-User QKD Networks
The first experimental implementation of QKD occurred
in 1989 [11], when encryption keys were transmitted
through 30 cm of air using polarization-encoded photons.
It was shown that the use of orthogonal states on more
than 10 km of optical fibre is impossible, according to
the characteristics of the sources available at present [2,
12]. To allow transmissions at distances always longer, it
is therefore necessary the use of systems different from
the ones used before. In particular when using an inter-
ferometer we can encode qubits in an interferometric
phase state.
For example we explain the implementation of BB84
using an interferometer. Alice encodes the photons with
her phase modulator (PM) by randomly choosing one of
four phase shifts: 0 and  correspond to one basis set and
/2 and 3/2 correspond to another basis set. She associ-
ates 0 and /2 with qubit 0, and  and 3/2 with qubit 1.
Bob makes his measurement by randomly choosing be-
tween a 0 or /2 phase shift. Only photons with a final
phase shift of 0 or  (the difference of Alice’s and Bob’s
phase shifts) can interfere in Bob’s interferometer to
produce a deterministic outcome. Any final phase shift
/2 or 3/2 leads to a probabilistic outcome. Thus,
whenever Bob measures correctly, qubit 0 is routed to
Detector 1 (Det1) and qubit 1 to Detector 2 (Det2). Since
Bob’s measurement consists of a random choice of basis,
half of the measurement results is probabilistic. Therefore,
after the qubit transmission, Bob confers with Alice
about the appropriate basis choice. Any qubit measured
in an incompatible basis is discarded and does not be-
come part of the final key. This process creates the sifted
key.
Now we introduce the four QKD network topologies
to be compared [3]. These networks phase-encode the
qubits in optical fibre interferometers. The optical-ring
network uses a Signac interferometer; all other topologies
are implemented with unbalanced Mach–Zehnder inter-
ferometers (MZIs). The unbalanced MZI is a modifica-
tion of the standard MZI with improved interference
sta bility. This improved stability comes at the expense of
a 3-dB loss, since half of the photons transmitted through
it are lost in the non-interfering path combinations of the
interferometer [1]. This makes networks that use the
unbalanced MZIs more loss, thus lowering their sifted
key rate and increasing their QBER. The single-photon
sources used in the network topologies and in the calcu-
lations are modelled as highly attenuated laser pulses that
are typically used in practice and contain an average of
0.1 photon per pulse. The single-photon detectors are
also modelled as the response of gated avalanche photo-
diodes operated in Geiger mode [13].
In general, Alice is defined as the user that provides
the qubit information in the four bases, and Bob is de-
fined as the user that chooses between the two non-
thogonal basis sets. For the passive-star (Figure 3),
wavelength-routed (Figure 5), and wavelength-addressed
bus (Figure 6) topologies, Alice is the network controller.
TA
TA
TA
PM
PLS
Alice
1 x N
Splitter
PM
Det 1
Det 2
Dan
Bob
Chris
N-th
user
Figure 3. Network topology of passive -star multi-user QKD
network. (PLS: Pulsed laser source; TA: tuneable attenu-
ator; PM: phase modulator; Det: detector.).
TA
PLS
Circulator Coupler
Det 1
Det 2
Bob
Alice 1
Alice 2
Alice N
PM
Alice 3
PM
CW CCW
Figure 4. Network topology of optical-ring multi-user QKD
network base d on Signac interferometer.

F. GARZIA ET AL. 170
TA
TA
TA
PM
Tunable
PLS
Alice
AWG
PM
Det 1
Det 2
Dan( 3 )
Bob ( 1)
Chris( 1)
N-th
user( N )
Figure 5. Network topology of wavelength-routed multi-
user QKD network. (AWG: arrayed-waveguide grating).
TA TA TA PM
Tunable
PLS
Alic e
GGG
PM
Det 1Det 2
Bob
PM
Det 1Det 2
Chris
PM
Det 1Det 2
N-th user
12N
Figure 6. Network topology of wavelength-addressed bus
multi-user QKD network. (G: fibre Bragg grating).
She is equipped with an unbalanced MZI, a pulsed laser
source (PLS), a tuneable attenuator (TA), and a four -stat e
PM. The users at the receiving end (Bob, Chris, Dan,
N-th user) choo se between the two non-o r thogon al bases.
Each one of them has another unbalanced MZI, a
two-state PM, and a pair of single-photon detectors (Det1
and Det2). The optical-ring network (Figure 4) is sig-
nificantly different from the others. Here, Bob is the
network controller and services multiple Alice. Bob’s
setup consists of a laser source, two detectors, a two-state
PM, and a circulator. Each Alice only possesses a
four-state PM.
3.1. Passive-Star Network
The topology of the passive-star QKD network is shown
in Figure 3 [3]. A passive-star QKD network was first
demonstrated to connect four users over 5.4 km of opti-
cal fibre [14]. This topology is an extension of the
two-user system, with Alice linked to receivers through a
1xN splitter. Due to the indivisible nature of the photon,
each photon is randomly routed to a single user by the
1xN splitter. This topology can be easily implemented
but suffers from the effective loss induced by the 1 splitter,
which reduces the probability of photons to reach the
detectors of any particular user. This reduction scales
inversely as the number of users on the network. For
example, a three-user network having a 1x2 splitter
reduces the probability that a photon reach the desired
receiver by one half and consequently acts as a 3-dB
attenuator. A 17-user network containing a 1x16 splitter
acts effectively like a 12-dB attenuator, and so on. Al-
though this drawback can be partially mitigated by
higher initial qubit rates, the routing of the photons to
each user is inherently nondeterministic. For example,
the mean detection rate at each user after a 1xN splitter is
1/Nth of the detection rate of a single Bob without the
1xN splitter. However, since the routing of photons to
each user through the 1xN splitter is random, at any
given time, some users receive photons at a rate above
the mean detection rate of 1/Nth, and some users receive
photons at a rate below the mean detection rate. This
nondeterministic detection rate constrains the design of
secure quantum networks by limiting the amount of in-
formation that can be securely encrypted.
3.2. Optical-Ring Network Based on Signac
Interferometer
Figure 4 shows the schematic diagram of the optical- ring
network topology. A two-user QKD system based on the
optical fibre Signac interferometer has been demon-
strated [15]. This topology is significantly different from
the topologies based on the unbalanced MZIs: the single-
photon pulse en ters the Signac interferometer throug h an
optical circulator. This pulse splits into two parts in the
50/50 coupler, and each travels around the Signac loop in
clockwise (CW) and counter clockwise (CCW) direc-
tions, respectively. Any user on the loop that is commu-
nicating with Bob modulates the pulse travelling in the
CW direction. Bob modulates the pulse travelling in the
CCW direction. The position of Bob’s PM is important
since the pulse that it modulates must be returning from
its round trip in the loop in order to prevent any informa-
tion about Bob’s modulation choice from travelling
through the loop. A timing and control mechanism must
also be established so that only one Alice can modulate
the photon at a time. Upon travelling around the loop, the
pulses interfere in the coupler and enter one of two pho-
ton detectors. Photons enter Detector 1 (Det1 in Figure 4)
when they experience a phase shift between the CW and
CCW pulses inside the Signac interferometer. On the
other hand, they enter Detector 2 (Det2 in Figure 4)
when they experience a 2 phase shift between the CW
and CCW pulses inside the Signac interferometer. The
Signac interferometer has the advantage of being free
from thermal fluctuations since the counter propagating
pulses pass through the exact same fibre paths inside the

F. GARZIA ET AL.171
loop. Another potential advantage is that each user on the
network, except Bob, contains only a single-PM and no
photon detectors. This can simplify any deployment of a
secure ring network using the Signac because Bob is the
only user that requires the single-photon detectors.
3.3. Wavelength-Routed Network
The schematic diagram of the wavelength-routed net-
work topology is depicted in Figure 5 [3]. This topo logy
is implemented with unbalanced MZIs and is very similar
in layout to the star network. The greater difference is
that Alice has the ability to control which user receives
the photons by employing a wavelength-routing scheme.
Alice is equipped with a wavelength tuneable pulsed
laser source (PLS) and the receivers are assigned their
own wavelength channel. Alice transmits to a particular
user by tuning her source to that user’s wavelength and
the photons are routed via an arrayed waveguide grating
(AWG). The advantage of this topology is that the inser-
tion loss of the AWG is approximately uniform regard-
less of the number of channels. Theoretically, the num-
ber of users that this type of network supports is limited
only by the channel spacing of the AWG and the band-
width of the fibre. In addition, the single-photon detec-
tors must be sensitive for the entire range of frequencies
used in the network. This is not a concern as
avalanche-photodiode (APD)-based single-photon de-
tectors respond to a much broader spectrum than the
band of wavelengths use d in multi-wavelength networks.
Due to the principles of quantum mechanics described
above, it is impossible for the spy Eve to gain perfect
knowledge of the quantum state sent from Alice to Bob.
Nevertheless, she can acquire some knowledge. Without
interaction of a spy, each two-level quantum system carries
1 bit of information from Alice to Bob. When Eve gets
hold of part of this information, she cannot prevent
causing a disturbance to the state arriving at Bob’s side,
and thus introducing a non-zero error rate. In principle,
Bob can find out about this error rate and thus about the
existence of a spy by communicating with Alice. The
source for Eve’s knowledge is measurements performed
on the signals (quantum states). The simplest eavesdrop-
ping attack (intercept/resend) for Eve would be to meas-
ure each signal just as Bob would do, and then to resend
a signal to Bob which corresponds to the measurement
result. Further we always have some detector noise,
misalignments of detectors and so on. It should be
pointed out that we cannot even in principle distinguish
errors due to noise from errors due to eavesdropping
activity. We therefore assume that all errors are due to
eavesdropping. Another issue, not discussed here, is that
of statistics. Eavesdroppers can be lucky: they create
errors only on average, so in any specific realization the
error rate might be zero (with probability exponentially
small in the key length, of course). We are guided by the
idea that a small error rate, for example 1 %, implies that
an eavesdropper was not very active, while a big error
rate is the signature of a serious eavesdropping attempt.
But what is the meaning of “small” and “big”? From an
information theoretic point of view, the natural measure
of “knowledge” about some signal is represented by the
Shannon information. It is measured in bits and can be
defined for any two parties, the sender of the signal and
the observer (receiver). In general terms, the knowledge
of the observer consists of obtained measurement results
and any additional gathered knowledge, like the an-
nounced basis of signals in the BB84 protocol.
3.4. Wavelength-Addressed Bus Network
The wavelength-addressed bus network is also based on
the unbalanced MZI setup and it is shown in Figure 6
[3].
Like the wavelength-routed network, this network also
allows Alice to route her photons to a desired user by
tuning the photons to be desired wavelength. In such a
system, Alice is equipped with a tuneable PLS, and each
receiver is assigned its own wavelength channel. Alice
selects an intended receiver by tuning her source to that
user’s wavelength and transmits the encoded photons
along the bus. The receivers are connected to the bus line
through a fibre Bragg grating (G), which allows them to
retrieve on ly the pho ton s, in tend ed for them. Th ese grating s
are designed to reflect photons of a specific wavelength
to a given user and transmit all others. The network
accommodates multiple users by placing several fibre
Bragg gratings in series along the bus. One of the merits
of this topology is that it can be easily expanded to
accommodate more users by simply tapping the bus and
inserting a suitable grating.
4. Security Threshold
The QBER, which is indicative of the security and
post-error-correction net key rate, is useful for assessing
the performance of the network. High QBER values in
QKD systems lower the net key rate during the error
correction stage of the protocol [1]. In addition, high
QBER allows an eavesdropper to gain more information
about the transmitted keys at the expense of the legiti-
mate receiver. It has been shown that for QBERs above a
security threshold, an eavesdropper can actually gain
more information than the legitimate receiver. If this
happens, it is not possible to use any privacy-amplification
technique. Therefore, when designing a QKD network, it
is necessary to ensure that the baseline QBER is below
this security threshold so that privacy amplification
strategies may be used to eliminate any knowledge

F. GARZIA ET AL. 172
gained by Eve [1]. For QBERs under this threshold
(QBERT), the Shannon information between Alice and
Bob (IAB) is higher than that in Eva’s possession (IE ),
while for superior values that of Eva is greater:
QBER < QBERT I
AB > IE (4)
QBER > QBERT I
AB < IE (5)
Bounds on the obtainable Shannon information for
eavesdropping on single bits can be found in the litera-
ture for different protocols. Fuchs et al. give bounds for
the BB84 [16] and the B92 protocol [17]. A bound for
the Six State Protocol was also obtained [18]. These
bounds are illustrated in Figures 7–9 for each of used
protocol. Note the trade-off between Eve’s information
gain and the disturbance she causes: more information
for Eve means higher error rate for Bob. For reasonably
low error rates Eve’s maximal information is smallest in
the six-state protocol, as it uses the largest ensemble of
input states.
Figure 7. Shannon Information (in normalized units) with
B92 protocol.
Figure 8. Shannon Information (in normalized units) with
SSP.
Figure 9. Shannon Information (in normalized units) with
EPR protocol.
Furthermore comparing Eva’s Shannon Information with
the Shannon information between Alice and Bob, we are
able to determinate the threshold for the QBER for each
of the used protocols.
4.1. B92
Security Threshold for B92 protocol is:
QBERT  14% (6)
4.2. SSP
Security Threshold for the Six State Protocol is:
QBERT  17% (7)
4.3. EPR
Security Threshold for EPR protocol is:
QBERT  15% (8)
5. Key Parameters in QKD
QBER and RSIFT are two parameters used to gauge the
performance of network topologies which offer QKD
service. The QBER and sifted key rate equations that are
used in the simulations are reviewed in this section.
More detailed discussions on the physical principles
underlying these equations are provided in references [1]
and [19]. The sifted keys are those keys shared by Alice
and Bob when they make compatible basis choices [19]:
RSIFT = q RRAW (9)
RRAW = fREP  tLINK  (raw key rate) (10)
where q depends on protocol (for example, in BB84 pro-
tocol q = 1/2 because half of the time Alice and Bob
bases are not compatible), fREP is the repetition frequency,
µ is the average number of photons per pulse, tLINK is the

F. GARZIA ET AL.173
transmission coefficient of the link and  is Bob’s detec-
tion efficiency. The transmission coefficient is related to
the loss lF (in dB per km) and length L (in km) of the
fibre, the loss due to the number of users lN(N) (in dB),
and the topology selected, by
tLINK =




10/
10 TNF lNlLl  (11)
The topology choice introduces a topology loss con-
stant lT (in dB) that is an overhead of loss involved in
working with a particular topology. This quantity is con-
stant regardless of a network’s fibre length and number
of users. The topology loss has 4 components: end-user
losses arising from losses in the receiver’s interferometer,
routing loss caused by the device that selects the user
that receives the photon, the non-interfering path combi-
nation loss in the unbalanced MZIs (for those topologies
that use them), and miscellaneous losses, such as those
caused by connectors and splices.
The QBER is defined as the number of wrong bits of
the total number of received bits and is normally in the
order of a few percent. In the following we use it ex-
pressed as a function of rates [1]:
SIFT
error
errorSIFT
error R
R
RR
R
QBER 

 (12)
One can distinguish three different contributions to
RERROR. The first one arises because of photons ending
up in the wrong detector, due to imperfect interference or
polarization contrast. The rate ROPT is given by the product
of the sifted key rate and the probability POPT of a photon
going in the wrong detector:
ROPT = RSIFT POPT (13)
This contribution can be considered, for a given set-up,
as an intrinsic error rate indicating the suitability to use it
for QKD. Imperfect phase matching in the interferometer s
results in reduced fringe visibilities that lead to an
increased probability of routing photons to the wrong
detectors. The probability of this type of error POPT is
related to the fringe visibility (V) by:
POPT = 2
1V (14)
The second contr ibution, RDARK, arises from the detec-
tor dark counts (or from remaining environmental stray
light in free space setups). This rate is independent of the
bit rate and depends only on the characteristic of the
photon counter [13]. Of course, only dark counts falling
in a short time window when a photon is expected give
rise to errors: RDARK = k fREP PDARK (15)
where PDARK is the probability of registering a dark count
per time-window and per detector, and the k factor is
related to the fact that a dark count has a k % chance to
happen with Alice and Bob having chosen incompatible
bases (thus eliminated during sifting). Finally error
counts can arise from uncorrelated photons, because of
imperfect phot on sources:
RACC = 2
1fREP
 tLINK  PACC (16)
This factor appears only in systems based on entangled
photons, where the photons belonging to different pairs,
but arriving in the same time window, ar e no t necessar ily
in the same state. The quantity PACC is the probability to
find a second pair within the time window, knowing that
a first one was created. The QBER can now be expressed
as follows:
SIFT
ACCDARKOPT R
RRR
QBER   (17)
6. Parameter Values
The results are based on calculations assuming the
following parameter values, which are held constant for
each topology [1,13,14,20,21] :
Pulse repetition rate (fREP) 1 MHz
Mean number of photon per p ulse ( ) 0.1
Detector efficiency @1310 nm ( ) 20%
Detector efficiency @1550 nm ( ) 10%
Dark count probability (PDARK ) 10-5
Fringe visibility (V) 98%
The transmission coefficient link tLINK varies from one
topology to another. The values used in the simulations
that contribute to tLINK are outlined for each topology in
Table 2. In the table the contributions to the topology
losses are also shown; namely, the end- user loss, routing
loss, non-interfering path combination loss, and miscel-
laneous loss.
The end-user loss arises from the excess loss in the
couplers and PM in the receiver’s interferometer.
Routing loss is th e lo ss in th e dev ic e th a t rou tes the phot ons
to each user. In the star, wavelength-routed, and bus
networks, which are all based on the unbalanced MZI
design, a 3-dB loss arises from non-interfering path
combinations. The miscellaneous loss stems from losses
Table 2. Losses contributing to the transmission coefficient
tLINK for the 4 considered network topologies.
Loss SourceStar Ring W.RoutedBus
Topology Loss
End User Loss (dB)
Routing Loss (dB)
N
on interfer. path Loss (dB)
Miscellaneous Loss (dB)
0.3
0.1
3.0
1.0
0.49
0.0
0.0
1.0
0.3
3.0
3.0
1.0
0.3
0.02
3.0
1.0
Total Topology Loss (dB)4.4 1.49 7.34.32
Fiber Loss (dB/km)0.35 @ 1310 nm
0.25 @ 1550 nm
User number Loss ( dB)10log(N) 0.1N 00.2(N-1)

F. GARZIA ET AL. 174
such as those due to connectors, splices, and imperfections
in the network all of which occur in practical optical
network setups.
Now we are able to analyse the QBER and the RSIFT
for every QKD protocol considered previously. The
results of QBER for each topology are presented in sur-
face plots which relate the QBER to the number of users
and distance. The term “distance” is defined as the total
fibre length used in the transmission of the photons. For
the optical ring, it is the total length of the Signac loop.
For all the other topologies, it is the total fibre length
spanning Alice and Bob (or Chris, Dan, etc.). The system
performances at the 1310-nm and 1550-nm telecommu-
nications wavelength windows are shown in the results.
The shaded regions in the QBER surface plots corre-
spond to the combinations of distance and number of
network users for which the QBER is less than QBERT.
This threshold, previously mentioned in section IV, is the
value below which secure key distribution can be per-
formed on the network. Thus, the shape and area of the
shaded regions allow one to easily determine the suit-
ability of a given topology to support a given number of
users. In addition, these plots also serve to show a net-
work’s sensitivity to expanding the number of users.
7. QBER Performance
7.1. B92
As previously explained in section 2.2, B92 protocol is
intrinsically less efficient than the given BB84 where,
also in ideal conditions (when no bit of the raw key is to
be deleted), only 1/4 of the impulses gives a key bits,
while with BB84 this protocol fraction is 1/2. This in-
efficiency is the price that Alice and Bob must pay for
secrecy.
RSIFT = 4
1RRAW =4 1 f
REP
 tLINK  (18)
ROPT =4
1 f
REP
 tLINK  POPT (19)
where
POPT = 2
1V= 0.01 = 1% (20)
since fringe visibility is 98%.
RDARK = 2
1 f
REP PDARK (21)
The 3-D QBER surface of the four topologies using a
carrier wavelength of 1310 nm is illustrated in Figure 10,
and the 3-D QBER surface at 1550 nm is illustrated in
Figure 11. Table 3 and Ta ble 4 summarize the informa-
tion obtained from Figure 10 and Figure 11.
7.2. SSP
As previously explained in Subsection 2.3, the six states
(a)
(b)
(c)
(d)
Figure 10. B92-protocol topologies at 1310 nm. QBER sur-
face as a function of users and distance. Users range: 2-128
users. Distance range: 0- 80 km. QBER<14% in shaded re-
gion. (a) Passive Star B92 1310 nm; (b) Optical Ring B92
1310 nm; (c) Wavelength-routed B92 1310 nm; (d) Wave-
length-addressed bus B92 1310 nm.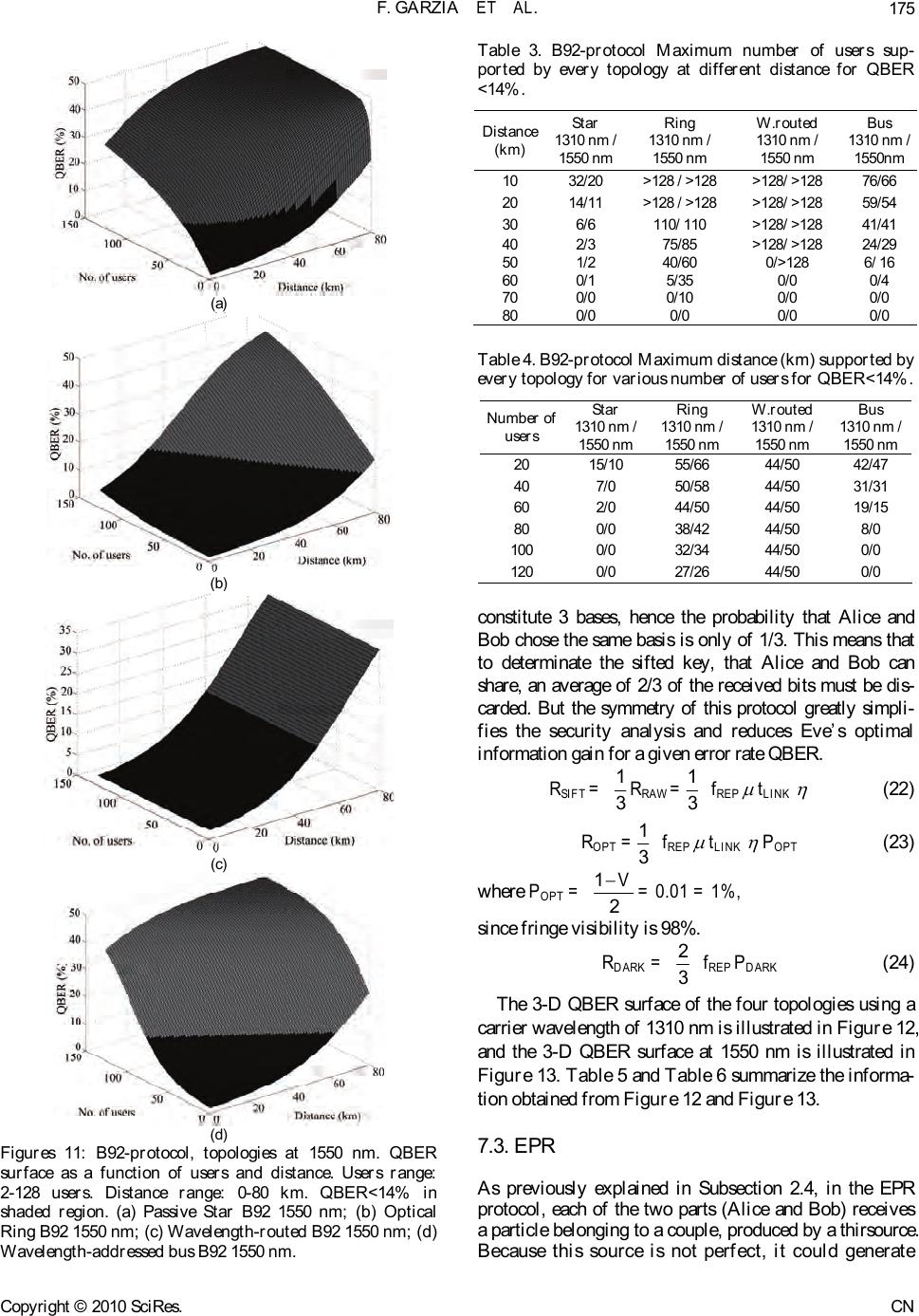

F. GARZIA ET AL.175
(a)
(b)
(c)
(d)
Figures 11: B92-protocol, topologies at 1550 nm. QBER
surface as a function of users and distance. Users range:
2-128 users. Distance range: 0-80 km. QBER<14% in
shaded region. (a) Passive Star B92 1550 nm; (b) Optical
Ring B92 1550 nm; (c) Wavelength-routed B92 1550 nm; (d)
Wavelength-addressed bus B92 1550 nm.
Table 3. B92-protocol Maximum number of users sup-
ported by every topology at different distance for QBER
<14%.
Distance
(km)
Star
1310 nm /
1550 nm
Ring
1310 nm /
1550 nm
W.routed
1310 nm /
1550 nm
Bus
1310 nm /
1550nm
10 32/20 >128 / >128 >128/ >128 76/66
20 14/11 >128 / >128 >128/ >128 59/54
30 6/6 110/ 110 >128/ >128 41/41
40 2/3 75/85 >128/ >128 24/29
50 1/2 40/60 0/>128 6/ 16
60 0/1 5/35 0/0 0/4
70 0/0 0/10 0/0 0/0
80 0/0 0/0 0/0 0/0
Table 4. B92-protocol Maximum distance (km) supported by
every topology for various number of users for QBER<14% .
Number of
users
Star
1310 nm /
1550 nm
Ring
1310 nm /
1550 nm
W.routed
1310 nm /
1550 nm
Bus
1310 nm /
1550 nm
20 15/10 55/66 44/50 42/47
40 7/0 50/58 44/50 31/31
60 2/0 44/50 44/50 19/15
80 0/0 38/42 44/50 8/0
100 0/0 32/34 44/50 0/0
120 0/0 27/26 44/50 0/0
constitute 3 bases, hence the probability that Alice and
Bob chose the same basis is only of 1/3. This means that
to determinate the sifted key, that Alice and Bob can
share, an average of 2/3 of the received bits must be dis-
carded. But the symmetry of this protocol greatly simpli-
fies the security analysis and reduces Eve’s optimal
information gain for a given e rro r rate QBER .
RSIFT = 3
1RRAW =3 1 f
REP
 tLINK  (22)
ROPT =3
1 f
REP
 tLINK  POPT (23)
where POPT = 2
1V

= 0.01 = 1%,
since fringe visibility is 98%.
RDARK = 3
2 f
REP PDARK (24)
The 3-D QBER surface of the four topologies using a
carrier wavelength of 1310 nm is illustrated in Figure 12,
and the 3-D QBER surface at 1550 nm is illustrated in
Figure 13. Table 5 and Table 6 summarize the informa-
tion obtained from Figure 12 and Figure 13.
7.3. EPR
As previously explained in Subsection 2.4, in the EPR
protocol, each of the two parts (Alice and Bob) receives
a particle belonging to a couple, produced by a thirsource.
Because this source is not perfect, it could generate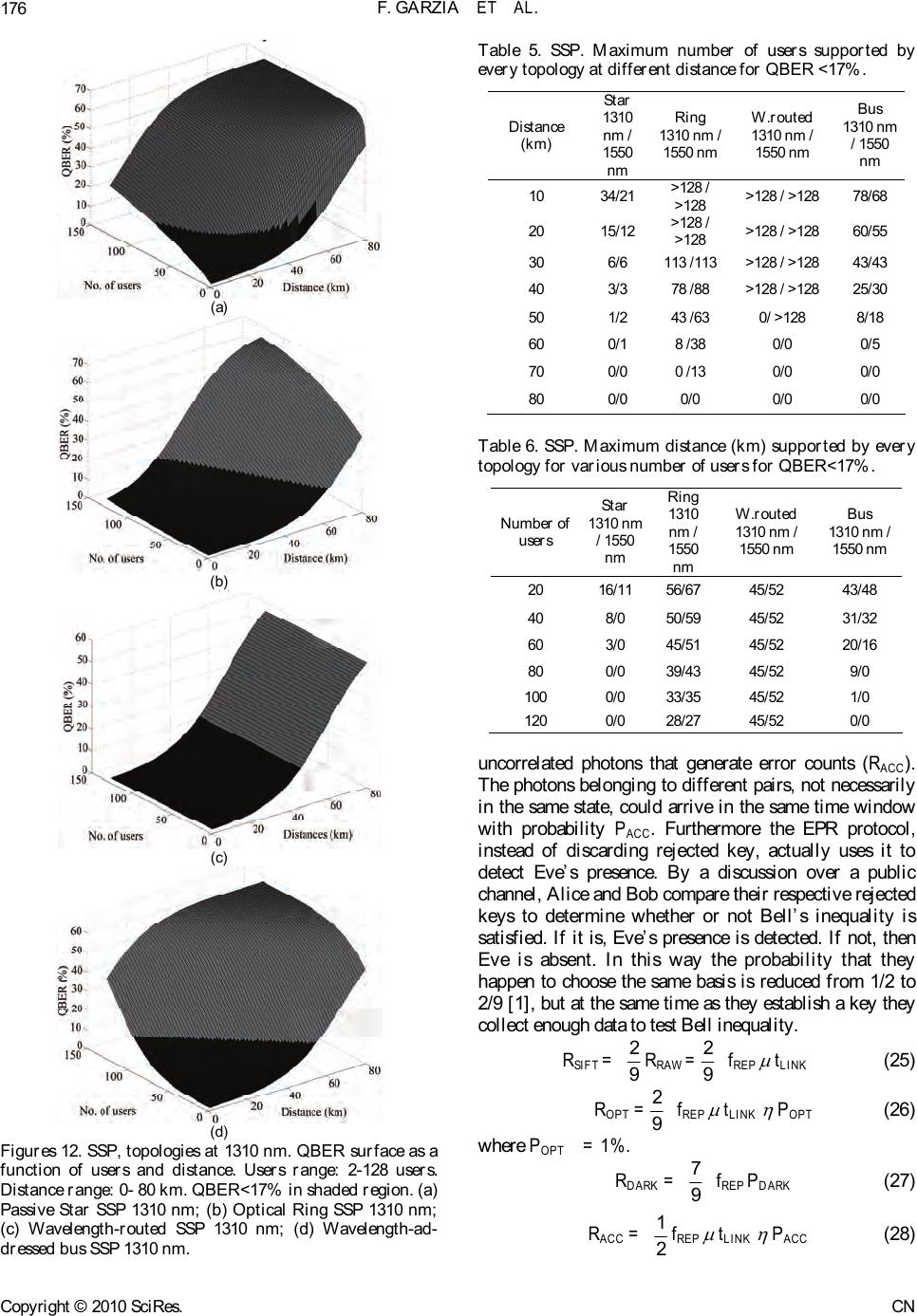

F. GARZIA ET AL. 176
(a)
(b)
(c)
(d)
Figures 12. SSP, topologies at 1310 nm. QBER surface as a
function of users and distance. Users range: 2-128 users.
Distance range: 0- 80 km. QBER<17% in shaded region. (a)
Passive Star SSP 1310 nm; (b) Optical Ring SSP 1310 nm;
(c) Wavelength-routed SSP 1310 nm; (d) Wavelength-ad-
dressed bus SSP 1310 nm.
Table 5. SSP. Maximum number of users supported by
every topology at different distance for QBER <17%.
Distance
(km)
Star
1310
nm /
1550
nm
Ring
1310 nm /
1550 nm
W.routed
1310 nm /
1550 nm
Bus
1310 nm
/ 1550
nm
10 34/21
>128 /
>128 >128 / >128 78/68
20 15/12
>128 /
>128 >128 / >128 60/55
30 6/6 113 /113 >128 / >128 43/43
40 3/3 78 /88 >128 / >128 25/30
50 1/2 43 /63 0/ >128 8/18
60 0/1 8 /38 0/0 0/5
70 0/0 0 /13 0/0 0/0
80 0/0 0/0 0/0 0/0
Table 6. SSP. Maximum distance (km) supported by every
topology for various number of users for QBER<17%.
Number of
users
Star
1310 nm
/ 1550
nm
Ring
1310
nm /
1550
nm
W.routed
1310 nm /
1550 nm
Bus
1310 nm /
1550 nm
20 16/11 56/67 45/52 43/48
40 8/0 50/59 45/52 31/32
60 3/0 45/51 45/52 20/16
80 0/0 39/43 45/52 9/0
100 0/0 33/35 45/52 1/0
120 0/0 28/27 45/52 0/0
uncorrelated photons that generate error counts (RACC).
The photons belonging to different pairs, not necessarily
in the same state, could arrive in the same time window
with probability PACC. Furthermore the EPR protocol,
instead of discarding rejected key, actually uses it to
detect Eve’s presence. By a discussion over a public
channel, Alice and Bob compare their respective rejected
keys to determine whether or not Bell’s inequality is
satisfied. If it is, Eve’s presence is detected. If not, then
Eve is absent. In this way the probability that they
happen to choose the same basis is reduced from 1/2 to
2/9 [1], but at the same time as they establish a key they
collect enough data to test Bell inequality.
RSIFT = 9
2RRAW =9 2 f
REP
 tLINK (25)
ROPT =9
2 f
REP
 tLINK  POPT (26)
where POPT = 1%.
RDARK = 9
7 f
REP PDARK (27)
RACC = 2
1fREP
 tLINK  PACC (28)

F. GARZIA ET AL.177
PACC = 2
1

2 = 000.5 (29)
The 3-D QBER surface of the four topologies using a
carrier wavelength of 1310 nm is illustrated in Figure 14,
and the 3-D QBER surface at 1550 nm is illustrated in
Figure 15. Tabl e 7 and Table 8 summarize the informa-
tion obtained from Figure 14 and Figure 15.
8. RSIFT Performance
To be able to make more visible the difference between
the various topologies, we compare the sifted key rate of
each topology as a function of distance for 4, 32, 64, 128
users. They were obviously assembled for every used
protocol.
The Sifted Key Rate performances of the four network
topologies are the same for each used protocol. We use a
grading system ranging from 1–4, where 1 indicates the
network topology with the best performance, and 4 indi-
cates the network topology with the worst performance,
to summarize the results of the comparison of the sifted
key rate performance of the network topologies. This is
shown in Table 9.
Another observation that is made is the distance (30
km) that the 1550- and 1310-nm sifted key rate lines for
a particular network cross each other. This distance,
which we conveniently call th e crossover distance, is the
same for all four topologies and determines when the
sifted key rate v alues at 1550 nm are greater or less than
the key rates at 1310 nm. For distances less than the
crossover distance, the sifted key rate values at 1310 nm
are always greater than at 1550 nm.
The situation reverses for distances beyond the cross-
over distance so that the sifted key rate values at 1550
nm become greater.
9. Results Discussion
Passive star network, that at first glance appears to be the
easiest to implement, turns out to be the wor st net topology
because:
1) supports the smallest number of users for any
given distance;
2) is very sensitive to change in the distance and/or
in number of the users;
3) has the lowest RSIFT .
Furthermore it requires each user to have their own
interferometer and photo-detectors. From this point of
view, the ring topology is the simpler design, requiring
each user to have only one four-state.
Optical ring network is characterized from:
1) higher stability against polarization and phase
fluctuations than the other three topologies since each
pulse travels through the same fibre length in both the
CW and CCW directions [2 2] ;
(a)
(b)
(c)
(d)
Figure 13. EPR- protocol, topologies at 1310 nm. QBER
surface as a function of users and distance. Users range:
2-128 users. Distance range: 0-80 km. QBER < 15% in
shaded region. (a) Passive Star EPR 1310 nm; (b) Optical
Ring EPR 1310 nm; (c) Wavelength-routed EPR 1310 nm;
(d) Wavelength-addressed bus EPR 1310 nm.

F. GARZIA ET AL. 178
(a)
(b)
(c)
(d)
Figure 14. EPR- protocol, topologies at 1550 nm. QBER
surface as a function of users and distance. Users range:
2-128 users. Distance range: 0-80 km. QBER < 15% in
shaded region. (a) Passive Star EPR 1550 nm; (b) Optical
Ring EPR 1550 nm; (c) Wavelength-routed EPR 1550 nm;
(d) Wavelength-addressed bus EPR 1550 nm.
Table 7. EPR-protocol Maximum number of users sup-
ported by every topology at different distance for QBER <
15%.
Dis-
tance
(km)
Star
1310 nm
/1550 nm
Ring
1310 nm
/1550 nm
W.routed
1310 nm /
1550 nm
Bus
1310 nm
/1550 nm
10 3/1 79 / 58 >128/ >128 26/16
20 1/1 44 / 33 0/ 0 8/3
30 0/0 9/9 0/ 0 0/0
40 0/0 0/0 0/0 0/0
50 0/0 0/0 0/0 0/0
60 0/0 0/0 0/0 0/0
70 0/0 0/0 0/0 0/0
80 0/0 0/0 0/0 0/0
Table 8. EPR-protocol Maximum distance (km) supported
by every topology for various number of users for QBER <
15%.
Number
of users
Star
1310 nm
/ 1550
nm
Ring
1310
nm /
1550
nm
W.routed
1310 nm /
1550 nm
Bus
1310 nm
/ 1550
nm
20 0/0 26/25 15/10 13/7
40 0/0 21/17 15/10 2/0
60 0/0 15/9 15/10 0/0
80 0/0 9/1 15/10 0/0
100 0/0 4/0 15/10 0/0
120 0/0 0/0 15/10 0/0
Table 9. Comparison of the Sifted Key Rate performance for
the 4 network topologies.
Number of
users Passive star Optical ring W.routed Bus
4 4 1 3 2
32 4 1 2 3
64 4 1 1 3
128 3 2 1 4
2) lowest structure loss (1.49 dB, Table2);
3) lowest QBER with less than 64 users;
4) highest Sifted Key Rate with less than 64 us ers
5) being more susceptible to Trojan horse attacks
than systems based on the unbalanced MZI [3].
Wavelength ne twork:
1) is the most suitable for networks with more than
64 users, because its Sifted key Rate is independent of
the number of users on the network;
2) it may not be the best choice for networks that
are not expected to expand beyond 64 users because
since it has the highest structure loss (7.3 dB).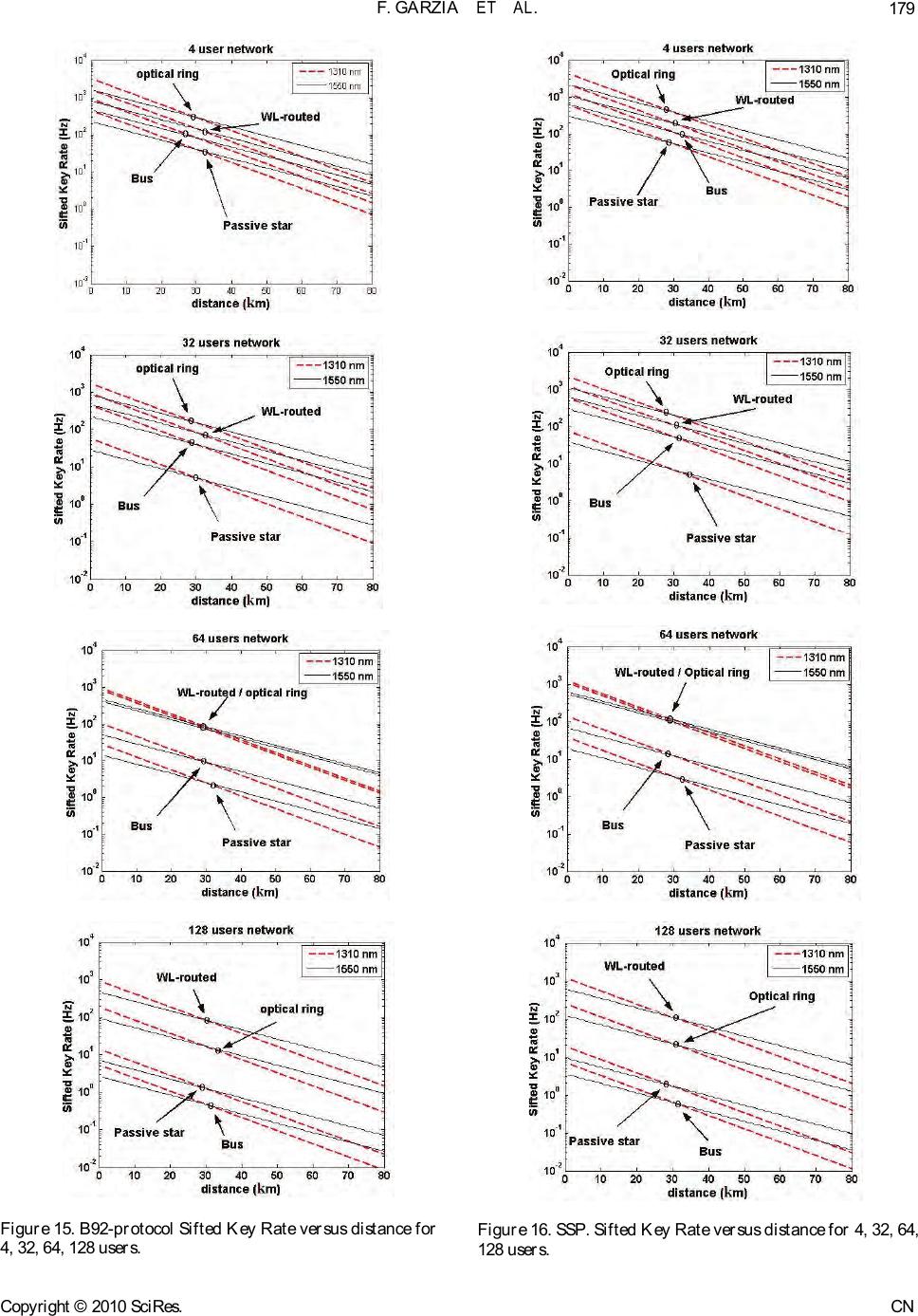

F. GARZIA ET AL.179
Figure 15. B92-protocol Sifted Key Rate versus distance for
4, 32, 64, 128 users.
Figure 16. SSP. Sifted Key Rate versus distance for 4, 32, 64,
128 users.

F. GARZIA ET AL. 180
Figure 17. EPR-protocol. Sifted Key Rate versus distance
for 4, 32, 64, 128 users.
Wavelength-addressed-bus network:
1) is the most favourable for networks with less
than 20 users because it can be easily expanded and has
moderate structure loss (4.32 dB);
2) is unadvisable for networks with large number
of users because it has a higher per-user loss than the
ring network.
It has also been shown that there is a crossover dis-
tance (30 km) that determines the optimum wavelength
(1310 or 1550 nm) to use in the QKD network.
About QKD analyzed protocol only B92 and SSP
turned out the most efficient. The EPR protocol is the less
efficient. The difficulty to handle couples of particles
without changing their correlation does no t allow to obtai n
high performances.
The results obtained at the moment are the least
encouraging for all the four net topologies. The maximum
reachable distance was of 30 km with 9 user maximum
using the Optical-Ring topology. Only for distances lower
than 10 km it is possible to obtain sufficient performances
avoiding however the Passive-Star topology. Six States
Protocol and the B92 present praiseworthy results. The
B92 is the protocol of QKD more used and allows to
make less communications on public channel. Six State
Protocol prevails on everybody because, having a security
threshold of 17%, allows to have a high number of users
also beyond the 60 km, furthermore it has the fastest
Sifted Key Rate.
Commonly used technologies and techniques have
been applied in order to evaluate the performances
(QBER and Sifted Key Rate). To avoid that Eva can take
some photons and measure their polarization without
disturbing the one of the photons which arrives to Bob, it
was considered an attenuation of the transmitted radia-
tion, obtaining an average 0.1 photons per impulse.
Commonly used technologies and techniques have been
applied in order to evaluate the performances (QBER and
Sifted Key Rate). Furthermore we considered pho-
ton-detectors with efficiency of 10% at 1550 nm and
20% at 1310 nm. Obviously, this choice implies a non-
neglectable reduction of the performances, but this com-
promise solution has been chosen to operate a more realistic
analysis. Technological improvements in single-photon
detectors are able to reduce the number of photons lost
and therefore to increase the system performances.
10. Conclusions
In this paper the performances of four passive optical
network topologies in implementing multi-user QKD,
using 3 protocols proposed by quantum cryptography
(B92, EPR, and SSP) have been compared. The QBER
and sifted key rate for each of these topologies have been
analysed to determine their suitability for providing service
to networks of vari ous sizes.

F. GARZIA ET AL.181
11. References
[1] N. Gisin, G. Ribordy, W. Tittel and H. Zbinden, “Quan-
tum Cryptography,” Reveiw of Modern Physics, Vol. 2,
Septemner 2001, pp. 1-57.
[2] W. K. Wootters and W. Zurek, “A Single Quantum Can-
not be Cloned,” Nature, Vol. 299, London, 1982. pp.
802-803.
[3] P. Kumavor, A. Beal, S. Yelin, E. Donkor and B. Wang,
“Comparison of Four Multi-user Quantum Key Distribu-
tion Schemes Over Passive Optical Networks,” Journal
of Lightwave Technology, Vol 23, No.1 January 2005,
p.268.
[4] C. H. Bennett and G. Brassard, “Quantum Cryptography:
Public Key Distribution and Coin Tossing,” IEEE
Conference on Computers, Systems Signal Processing,
Bangalore, 1984.
[5] C. Bennett, “Quantum Cryptography Using Any Two
Non-orthogonal States,” Physical Review Letter, Vol. 68,
1992, p. 3121,
[6] K. J. Gordon, V. Fernandez, P. D. Townsend and G. S.
Buller, “A Short Wavelength Giga Hertz Clocked
Fiber-Optic Quantum Key Distribution System,” IEEE
Journal of Quantum Electronics, Vol 40, No7, July 2004,
pp. 900-908.
[7] A. Einstein, B. Podolsky and N. Rosen, “Can Quantum-
Mechanical Description of Physical Reality be Considered
Complete,” Physical Review, Vol. 41, May 1935, p. 777.
[8] M. M. Ishtiaq Khan and M. Sher, “Protocols for Secure
Quantum Transmission: A Review of Recent Develop-
ments,” Pakistan Journal of Information and Technology，
Vol. 2, No. 3, 2003, pp. 265-276.
[9] J. Bell, “On the Einstein, Podolsky, Rosen Paradox,”
Physics, Vol. 1, 1964, pp. 195-200.
[10] D. Bruß and N. Lutkenhaus, “Quantum Key Distribution:
From Principles to Practicalities,” Vol. 2, September
1999.
[11] C. Bennett, F. Bessette, G. Brassard, L. Salvail and J.
Smolin, “Experimental Quantum Cryptography,” Journal
of Cryptography, Vol. 5, No. 1, 1992, pp. 3-28.
[12] A. Muller, J. Breguet and N. Gisin, “Experimental
Demonstration of Quantum Cryptography Using Pola-
rized Photons in Optical Fiber Over More Than 1 km,”
Europhysics Letter, Vol. 23, 1993, pp. 383-388.
[13] D. Stucki et al., “Photon Counting for Quantum Key
Distribution with Peltier Cooled InGaAs/InP APD’s,”
Journal of Modern Optics, Vol. 48, No. 13, 2001, pp.
1967-1981.
[14] P. D. Townsend, “Quantum Cryptography on Multi-User
Optical Fiber Networks,” Journal of Nature, Vol. 385,
No.2, 1997, pp. 47-49.
[15] T. Nishioka, H. Ishizuka, T. Hasegawa and J. Abe,
“Circular Type Quantum Key Distribution,” IEEE Photon
Technical Letter, Vol. 14, No. 4, April 2002, pp. 576-578.
[16] C. A. Fuchs, N. Gisin, R. B. Griffiths, C. S. Niu and A.
Peres, “Optimal Eavesdropping in Quantum Crypto-
graphy I,” P hysical Review A, Vol. 56, 1997, pp. 1163.
[17] C. A. Fuchs and A. Peres, “Quantum State Disturbance
VS. Information Gain: Uncertainty Relations for
Quantum Information,” Physical Review A, Vol. 53, 1996,
pp. 2038-2045.
[18] D. Bruß, “Optimal Eavesdropping in Quantum Crypto-
graphy With Six States,” Physical Reveiw Letter, Vol. 81,
1998, p. 3018.
[19] D. Stucki, N. Gisin, O. Guinnard, G. Ribordi and H.
Zbinden, “Quantum Key Distribution over 67 km with a
Plug&Play System,” New Journal of Physics, Vol. 4, July
2002, pp. 1-8.
[20] P. A. Hiskett et al., “Eighty Kilometer Transmission
Experiment Using an InGaAs/InP SPAD-based Quantum
Cryptography Receiver Operating at 1.55 m,” Journal
of Modern Optics, Vol. 48, No. 13, July 2001, pp.
1957-1966.
[21] D. S. Bethune and W. P. Risk, “Autocompensating Quan-
tum Cryptography,” New Journal of Physics, Vol. 4, July
2002, pp. 1-15.
[22] X. Fang and R. O. Claus, “Polarization-Dependent All-
fiber Wavelength Division Multiplexer Based on a
Signac Interferometer,” Optical Letter, Vol. 20, No. 20,
October 1995, pp. 2146-2148.
[23] P. D. Townsend, J. G. Rarity and P. R. Tapste r, “Enhanced
Single Photon Fringe Visibility in a 10 km-long
Prototype Quantum Cryptography Channel,” Electronics
Letter, Vol. 29, July 1993, pp. 1291-1293.
[24] C. Marand and P. D. Townsend, “Quantum Key Distri-
bution over Distances as Long as 30 km,” Optical Letter,
Vol. 20, No. 16, August 1995, pp. 1695-1697.
[25] H. Zbinden, “Interferometry With Faraday Mirrors for
Quantum Cryptography,” Electronics Letter, Vol. 33,
1997, pp. 586-588.
[26] H. Kosaka, A. Tomita, Y. Nambu, N. Kimura and K.
Nakamura, “Single Photon Interference Experiment over
100 km for Quantum Cryptography System Using a
Balanced Gated-Mode Photon Detector,” Electronics
Letter, Vol. 39, No. 16, 2003, pp. 1199-1201.
[27] S. J. D. Phoenix et al., “Multi-User Quantum Crypto-
graphy on Optical Networks,” Journal of Modern Optics,
Vol. 42, No. 6, January 1995, pp. 1155–1163.
[28] A. Muller, T. Herzog, B. Huttner, W. Tittel, H. Zbinden,
and N. Gisin, “Plug and Play Systems for Quantum
Cryptography,” Applied Physics Letter, Vol. 70, No. 7,
February 1997, pp. 793–795.
[29] E. Waks et al., “Secure Communication: Quantum
Cryptography with a Photon Turnstile,” Nature, Vol. 420,
London, December 2002, p. 762.
[30] E. Moreau et al., “Single-Mode Solid-State Single Photon
Source Based on Isolated Quantum Dots in Pillar
Microcavities,” Applied Physcis Letter, Vol. 79, No. 18,
October 2001, pp. 2865-2867.
[31] H. Bechmann-Pasquinucci and N. Gisin, “Incoherent and
Coherent Eavesdropping in the 6-state Protocol of

F. GARZIA ET AL. 182
Quantum Cryptography,” Physical Review A, Vol. 59, No.
6, 1998, pp. 1-11.
[32] A. Ekert, “Quantum Cryptography Based on Bell's
Theorem,” Physical Review Letter, Vol. 67, 1991, pp.
661-663.
[33] R. J. Hughes, G. L. Morgan and C. G. Peterson,
“Practical Quantum Key Distribution over a 48-km
Optical Fiber Network,” Physics Division Los Alamos
National Liberatory, NM 87545.