Reconstruction Parameters of Local Scattering Sources of a
Metallic Strip from the Backscattering Pattern
494
ordinates decreases. For
090
ο
ii
y
. (7)
In this case the error of the reconstruction of the longi-
tudinal coordinates in xy system equals to the error of the
reconstruction of the transverse coordinates in x'y' system.
So at the second stage it is better to choose 090
.
3. Numerical Results and Discussion
Later there are the results of the solution of the observing
problem for the metallic strip with the lower edge co-
ordinates (0, −5λ) and upper edge coordinates (0, 5λ)
(Figure 1). The backscattering pattern of the strip was
calculated by the rigorous method of the integral equa-
tions [16] in case of E-polarization (E is directed along z
axis) of the incident plane monochromatic electromag-
netic wave with the amplitude equals 30.
In Figure 3 the dependences of the reconstructed trans-
verse coordinates of two local sources of the strip on the
aspect angle φ are shown. The value of the aspect angles
sector ∆φ = 12˚. In this case two local sources starting
with φ0 = 6˚ are reconstructed when the normal angle to
the strip surface is not thrown into the aspect angles sector
(φ0 = 0˚). Curves 1 and 2, Figure 3 practically equal, so
the first local source is located on the lower edge of the
strip. For the aspect angles φ0 > 48˚ the amplitude of the
first local source is extremely small (Figure 4) and this
local source is not reconstructed. Curves 3 and 4, Figure 3
practically match, so the second local source is located on
the strip upper edge for the aspect angles (6˚, 90˚). The
local scattering sources of the strip are located on its sur-
face, so the reconstruction of its longitudinal coordinates
is obvious.
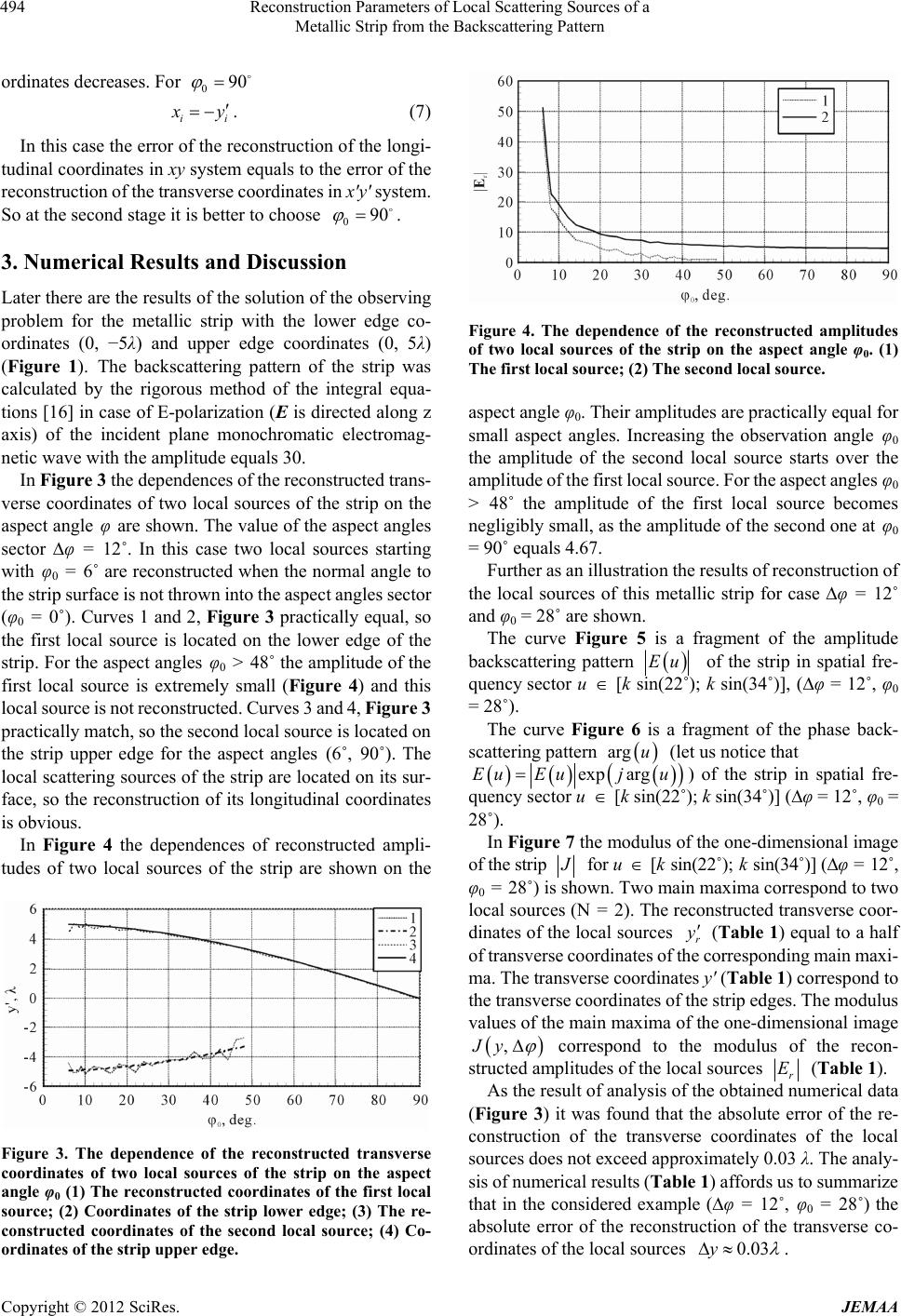
In Figure 4 the dependences of reconstructed ampli-
tudes of two local sources of the strip are shown on the
Figure 3. The dependence of the reconstructed transverse
coordinates of two local sources of the strip on the aspect
angle φ0 (1) The reconstructed coordinates of the first local
source; (2) Coordinates of the strip lower edge; (3) The re-
constructed coordinates of the second local source; (4) Co-
ordinates of the strip upper edge.
Figure 4. The dependence of the reconstructed amplitudes
of two local sources of the strip on the aspect angle φ0. (1)
The first local source; (2) The second local source.
aspect angle φ0. Their amplitudes are practically equal for
small aspect angles. Increasing the observation angle φ0
the amplitude of the second local source starts over the
amplitude of the first local source. For the aspect angles φ0
> 48˚ the amplitude of the first local source becomes
negligibly small, as the amplitude of the second one at φ0
= 90˚ equals 4.67.
Further as an illustration the results of reconstruction of
the local sources of this metallic strip for case ∆φ = 12˚
and φ0 = 28˚ are shown.
The curve Figure 5 is a fragment of the amplitude
backscattering pattern
Eu of the strip in spatial fre-
quency sector u
[k sin(22˚); k sin(34˚)], (∆φ = 12˚, φ0
= 28˚).
The curve Figure 6 is a fragment of the phase back-
scattering pattern
arg u (let us notice that
exEu Euup argj) of the strip in spatial fre-
quency sector u
[k sin(22˚); k sin(34˚)] (∆φ = 12˚, φ0 =
28˚).
In Figure 7 the modulus of the one-dimensional image
of the strip
for u
[k sin(22˚); k sin(34˚)] (∆φ = 12˚,
φ0 = 28˚) is shown. Two main maxima correspond to two
local sources (N = 2). The reconstructed transverse coor-
dinates of the local sources r (Table 1) equal to a half
of transverse coordinates of the corresponding main maxi-
ma. The transverse coordinates y' (Table 1) correspond to
the transverse coordinates of the strip edges. The modulus
values of the main maxima of the one-dimensional image
y
,Jy
correspond to the modulus of the recon-
structed amplitudes of the local sources r
E (Table 1).
As the result of analysis of the obtained numerical data
(Figure 3) it was found that the absolute error of the re-
construction of the transverse coordinates of the local
sources does not exceed approximately 0.03 λ. The analy-
sis of numerical results (Table 1) affords us to summarize
that in the considered example (∆φ = 12˚, φ0 = 28˚) the
absolute error of the reconstruction of the transverse co-
ordinates of the local sources 0.03y
.
Copyright © 2012 SciRes. JEMAA