Engineering, 2010, 2, 573-579
doi:10.4236/eng.2010.28073 Published Online August 2010 (http://www.SciRP.org/journal/eng).
Hydrogen Pick up in Zircaloy-4: Effects in the Dimensional
Stability of Structural Components under Nuclear Reactor
Operating Conditions
Pablo Vizcaíno, Cintia Paola Fagundez, Abraham David Banchik
Centro Atómico Ezeiza, Comisión Nacional de Energía Atómica, Presbítero J. González y Aragón Nº 15,
Buenos Aires, Argentina
Received December 3, 2009; revised February 11, 2010; accepted February 15, 2010
Abstract
In the present work, the expansion coefficient due to hydrogen incorporation was measured for the axial di-
rection of a Zircaloy-4 cooling channel, similar to that installed in the Atucha I PHWR, Argentina, trying to
simulate the nuclear power reactor operating conditions. As a first step, the solubility curve of hydrogen in
Zircloy-4 was determined by two techniques: differential scanning calorimetry and differential dilatometry.
The comparison with classical literature curves showed a good agreement with them, although the calorimet-
ric technique proved to be more accurate for these determinations. Dilatometry was able to detect the end of
hydride dissolution from concentrations around 60 wppm-H up to 650 wppm-H, where the eutectoid reaction:
     takes place (at 550oC). We assume that this ability is a good indicator of the aptitude of the
technique to measure dimensional changes in the given hydrogen concentration range. Then, the expansion
of Zircaloy-4 homogeneously hydrided samples was measured at 300oC, the typical operating temperature of
a nuclear power reactor, obtaining a relative expansion of 2.21 × 10-4% per wppm-H. Considering the rela-
tive expansion observed for Zircaloy-4 at room temperature due to hydriding, starting from a hydrogen free
sample, the total relative expansion rate is calculated to be 5.21 × 10-4% per wppm-H.
Keywords: Thermal Analysis, Dimensional Change, Hydrides, Zircaloy-4
1. Introduction
Most of the core structural components of the nuclear
power reactors are made of Zicaloy-4, a reference zirco-
nium alloy in many structural nuclear applications. Dur-
ing reactor operation, the initial dimensions of the Zr-
base components could increase due to three different
degradation processes: hydrogen pick up, irradiation gro-
wth and creep.
The hydrogen incorporated into the matrix is a fraction
of the total amount of hydrogen produced during the
corrosion reaction between the zirconium and the coolant,
according to the reaction:
Zr + 2H2O  ZrO2 + 4H
The crystalline defects produced by the fast neutron
irradiation induce changes in the initial dimensions of the
components depending on the fabrication texture. On the
other hand, the creep contribution to these processes de-
pends on the magnitude of the external stress applied to
the component.
The pick up of hydrogen atoms by the metal induces
an expansion of its initial length. This expansion contin-
ues after crossing the solubility limit at the reactor oper-
ating temperature, since the hydrogen in excess to that
limit precipitates as ZrH1,5+x after some supersaturation in
solid solution. Due to the higher specific volume of the
zirconium hydride with respect to the zirconium matrix,
the onset of precipitation induces an additional dimen-
sional change. This change in length depends on both,
the orientation at which the hydrides precipitated in the
matrix and the crystalline texture of the component.
The material under study in the present work is Zir-
caloy-4 taken from cooling channels similar to those in-
stalled in the Atucha I PHWR. These tubes have a fully
recrystallized microstructure, which induces hydride
precipitation at the grain boundaries. In addition, these
components show a strong texture in a quasi-radial direc-
tion: the c axis of the -Zr hexagonal cell is oriented in a
cone surrounding the radial direction of the tube [1]. The

P. VIZCAÍNO ET AL. 574
aim of the present work is to determine the expansion
coefficient of Zircaloy-4 for the axial direction of the
channel at the reactor operating temperature (300oC) [2].
2. Experimental Procedure
2.1. Material and Sample Preparation
The Zircaloy-4 samples were taken from an off cut of a
cooling channel similar to those installed in the Atucha I
reactor. Rectangular samples of dimensions 10 × 5 × 1.7
mm were cut from the tube, with the length of the sample
parallel to the axial direction of the tube, as it is shown in
Figure 1.
The tubes are cold-shaped and welded (by the tungsten
inert gas method) from fully recrystallized Zircaloy-4
sheets. The typical Kearns texture factors were measured
in a previous work for the [0002] pole (c axis of the
hexagonal cell). The range of values was: Faxial = 0.05-
0.07, Ftangential = 0.22-0.26, Fradial = 0.67-0.73. Thus, about
6% of the c poles are aligned in the axial direction, 24%
in the tangential and 70% in the radial direction [1]. The
microstructure was fully recrystallized with a grain size
of 15-20 m. It can be observed in Figure 2.
Figure 1. Orientation and dimensions of the dilatometric
samples.
20 m
Figure 2. Fully recrystallized microstructure of a Zircaloy-4
cooling channel. The typical size of the equiaxed grains is 20
± 6 m.
2.2. Hydriding
The hydrogen was incorporated by the cathodic charge
technique. The process was carried out in an electrolytic
cell at 80 ± 2°C. A diluted aqueous solution of sulfuric
acid was used as electrolyte, circulating a current density
of 5 mA/cm2 through the sample from periods of 18 to
96 h. As a result, hydride layers of different thickness
(from a few microns up to 50 microns) formed in the
samples.
The hydrogen was diffused into the bulk during the
dilatometric experiments. After the experiments, the
samples were polished to eliminate the oxide and any
remaining hydride layer on the surfaces. Finally, the hy-
drogen content was measured using a LECO RH-404
hydrogen meter. The error of these determinations is of
2%.
The hydrogen range of the samples hydrided in this
way varied from 50 to 650 wppm-H.
2.3. Differential Dilatometry
A Shimadzu TMA-60H vertical push rod differential
dilatometer, DD, was used to measure the difference in
expansion between a reference sample and a similar hy-
drided sample.
The experiments were carried out under inert gas at-
mosphere (high purity N2, 99.998%). As reference, an
uncharged Zircaloy-4 sample was used, containing about
20 wppm-H, which is incorporated during the fabrication
process of the channels. The minimum detection capacity
of DD is 0.25 m.
During the test, a constant load of 0.1 N was applied to
both samples. All the samples were subject to a nominal
thermal cycle made of a heating step at a rate of 5°C/min.
After keeping the samples 30 minutes at the maximum
temperature they were cooled down at 5°C/min. To avoid
the effect of the      transformation, the maxi-
mum temperature was a few degrees below 550oC.
2.4. Differential Scanning Calorimetry
The calorimetric experiments were made using a thermal
flux differential scanning calorimeter Shimadzu, model
DSC-60. The dimensions of the samples were 4 × 4 × 1.7
mm, which were cut from the dilatometric samples after
the dilatometric experiments finished.
Two runs were performed for each sample at 5oC/min,
in order to compare the results with the dilatometric data,
but the first one was discarded and TTSSd were deter-
mined in the second run. Figure 3 shows the calorimetric
heating curve of a hydrided sample where the points
usually associated with hydride dissolution are indicated:
the peak of the curve (pT, peak temperature), the maxi-
mum at the derivative of the DSC curve (msT, maximum
5 m
m
10 m
m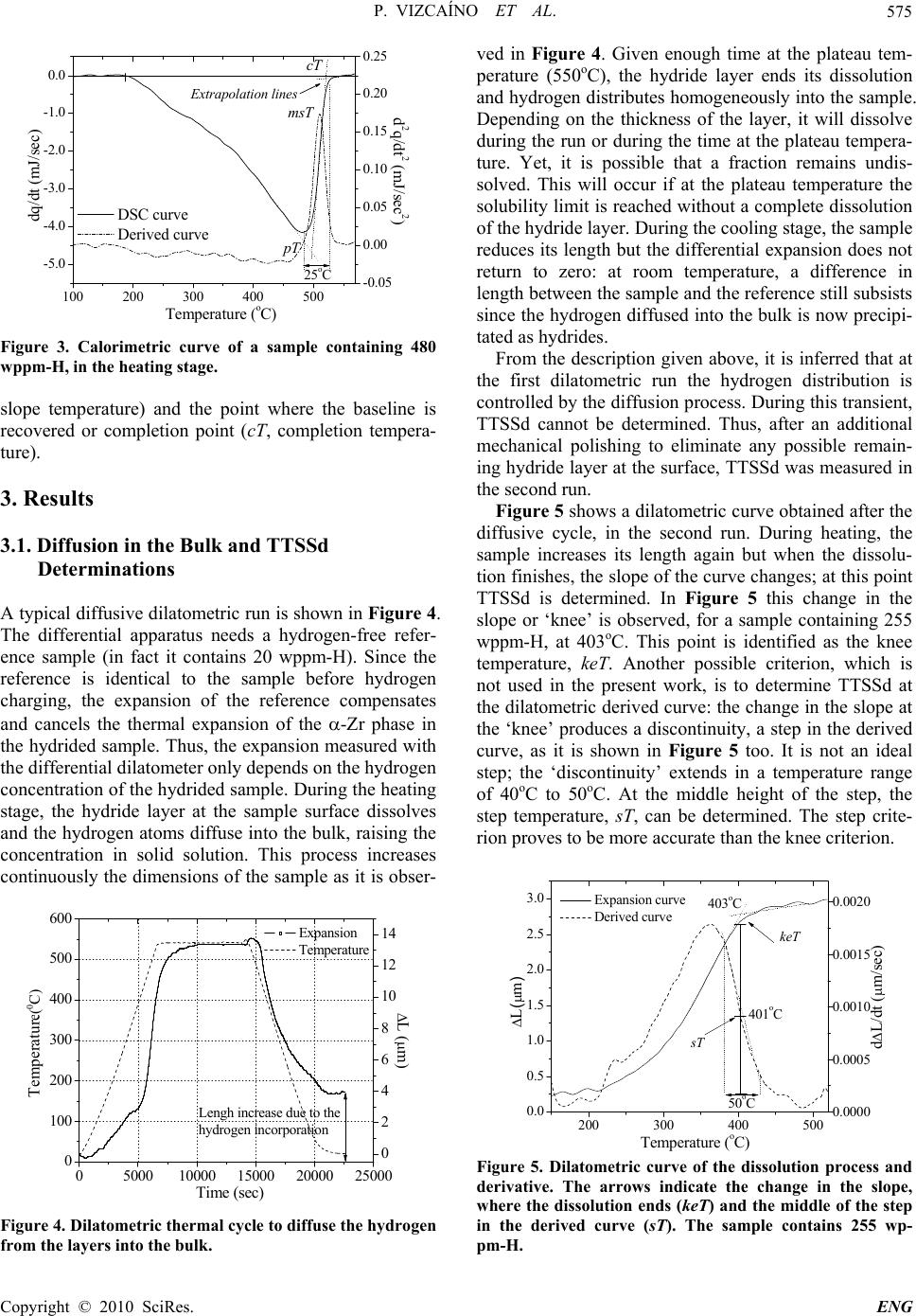

P. VIZCAÍNO ET AL. 575
100 200 300400 500
-5.0
-4.0
-3.0
-2.0
-1.0
0.0
msT
cT
pT
DSC curve
Derived curve
Temperature (oC)
dq/dt (mJ/sec)
25oC-0.05
0.00
0.05
0.10
0.15
0.20
0.25
Extrapolation lines
d2q/dt2 (mJ/sec2)
Figure 3. Calorimetric curve of a sample containing 480
wppm-H, in the heating stage.
slope temperature) and the point where the baseline is
recovered or completion point (cT , completion tempera-
ture).
3. Results
3.1. Diffusion in the Bulk and TTSSd
Determinations
A typical diffusive dilatometric run is shown in Figure 4.
The differential apparatus needs a hydrogen-free refer-
ence sample (in fact it contains 20 wppm-H). Since the
reference is identical to the sample before hydrogen
charging, the expansion of the reference compensates
and cancels the thermal expansion of the -Zr phase in
the hydrided sample. Thus, the expansion measured with
the differential dilatometer only depends on the hydrogen
concentration of the hydrided sample. During the heating
stage, the hydride layer at the sample surface dissolves
and the hydrogen atoms diffuse into the bulk, raising the
concentration in solid solution. This process increases
continuously the dimensions of the sample as it is obser-
0500010000 15000 20000 25000
0
100
200
300
400
500
600 Expansion
Temperature
Time (sec)
Temperature (篊)
0
2
4
6
8
10
12
14
L (m)
Lengh increase due to the
hydrogen incorporation
Figure 4. Dilatometric thermal cycle to diffuse the hydrogen
from the layers into the bulk.
ved in Figure 4. Given enough time at the plateau tem-
perature (550oC), the hydride layer ends its dissolution
and hydrogen distributes homogeneously into the sample.
Depending on the thickness of the layer, it will dissolve
during the run or during the time at the plateau tempera-
ture. Yet, it is possible that a fraction remains undis-
solved. This will occur if at the plateau temperature the
solubility limit is reached without a complete dissolution
of the hydride layer. During the cooling stage, the sample
reduces its length but the differential expansion does not
return to zero: at room temperature, a difference in
length between the sample and the reference still subsists
since the hydrogen diffused into the bulk is now precipi-
tated as hydrides.
From the description given above, it is inferred that at
the first dilatometric run the hydrogen distribution is
controlled by the diffusion process. During this transient,
TTSSd cannot be determined. Thus, after an additional
mechanical polishing to eliminate any possible remain-
ing hydride layer at the surface, TTSSd was measured in
the second run.
Figure 5 shows a dilatometric curve obtained after the
diffusive cycle, in the second run. During heating, the
sample increases its length again but when the dissolu-
tion finishes, the slope of the curve changes; at this point
TTSSd is determined. In Figure 5 this change in the
slope or ‘knee’ is observed, for a sample containing 255
wppm-H, at 403oC. This point is identified as the knee
temperature, keT. Another possible criterion, which is
not used in the present work, is to determine TTSSd at
the dilatometric derived curve: the change in the slope at
the ‘knee’ produces a discontinuity, a step in the derived
curve, as it is shown in Figure 5 too. It is not an ideal
step; the ‘discontinuity’ extends in a temperature range
of 40oC to 50oC. At the middle height of the step, the
step temperature, sT, can be determined. The step crite-
rion proves to be more accurate than the knee criterion.
200 300 400 500
0.0
0.5
1.0
1.5
2.0
2.5
3.0
50oC
Expansion curve
Derived curve
Temperature (oC)
L(m)
403oC
401oC
keT
sT
0.0000
0.0005
0.0010
0.0015
0.0020
d
L/dt
(

m/sec
)
Figure 5. Dilatometric curve of the dissolution process and
derivative. The arrows indicate the change in the slope,
where the dissolution ends (keT) and the middle of the step
in the derived curve (sT). The sample contains 255 wp-
pm-H.
(
0C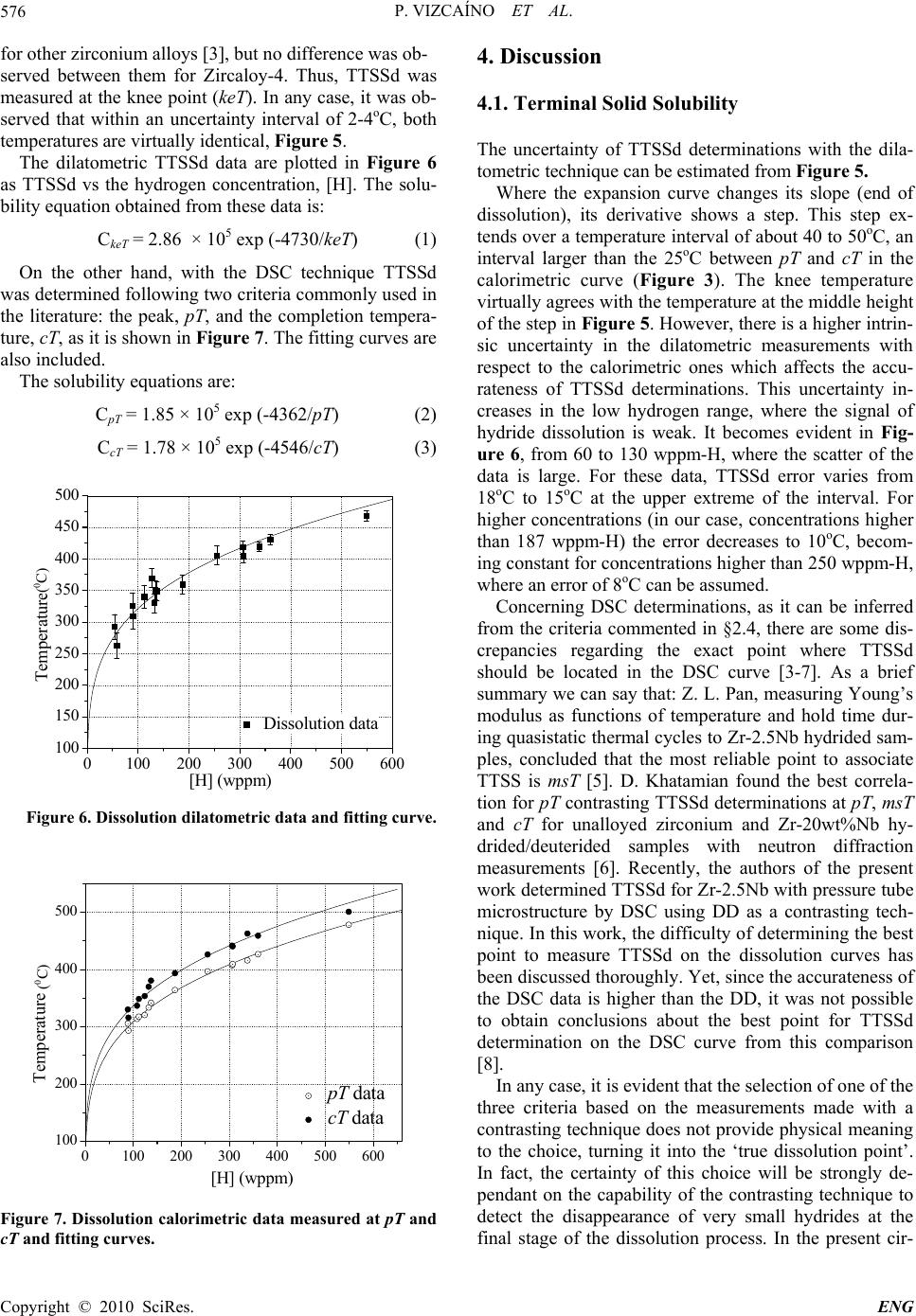
)

P. VIZCAÍNO ET AL. 576
for other zirconium alloys [3], but no difference was ob-
served between them for Zircaloy-4. Thus, TTSSd was
measured at the knee point (keT). In any case, it was ob-
served that within an uncertainty interval of 2-4oC, both
temperatures are virtually identical, Figure 5.
The dilatometric TTSSd data are plotted in Figure 6
as TTSSd vs the hydrogen concentration, [H]. The solu-
bility equation obtained from these data is:
CkeT = 2.86 × 105 exp (-4730/keT) (1)
On the other hand, with the DSC technique TTSSd
was determined following two criteria commonly used in
the literature: the peak, pT, and the completion tempera-
ture, cT, as it is shown in Figure 7. The fitting curves are
also included.
The solubility equations are:
CpT = 1.85 × 105 exp (-4362/pT) (2)
CcT = 1.78 × 105 exp (-4546/cT) (3)
0100 200 300 400 500 600
100
150
200
250
300
350
400
450
500
Dissolution data
Temperature (癈)
[H] (wppm)
Figure 6. Dissolution dilatometric data and fitting curve.
0100 200 300 400 500 600
100
200
300
400
500
pT data
cT data
Temperature (癈)
[H] (wppm)
Figure 7. Dissolution calorimetric data measured at pT and
cT and fitting curves.
4. Discussion
4.1. Terminal Solid Solubility
The uncertainty of TTSSd determinations with the dila-
tometric technique can be estimated from Figure 5.
Where the expansion curve changes its slope (end of
dissolution), its derivative shows a step. This step ex-
tends over a temperature interval of about 40 to 50oC, an
interval larger than the 25oC between pT and cT in the
calorimetric curve (Figure 3). The knee temperature
virtually agrees with the temperature at the middle height
of the step in Figure 5. However, there is a higher intrin-
sic uncertainty in the dilatometric measurements with
respect to the calorimetric ones which affects the accu-
rateness of TTSSd determinations. This uncertainty in-
creases in the low hydrogen range, where the signal of
hydride dissolution is weak. It becomes evident in Fig-
ure 6, from 60 to 130 wppm-H, where the scatter of the
data is large. For these data, TTSSd error varies from
18oC to 15oC at the upper extreme of the interval. For
higher concentrations (in our case, concentrations higher
than 187 wppm-H) the error decreases to 10oC, becom-
ing constant for concentrations higher than 250 wppm-H,
where an error of 8oC can be assumed.
Concerning DSC determinations, as it can be inferred
from the criteria commented in §2.4, there are some dis-
crepancies regarding the exact point where TTSSd
should be located in the DSC curve [3-7]. As a brief
summary we can say that: Z. L. Pan, measuring Young’s
modulus as functions of temperature and hold time dur-
ing quasistatic thermal cycles to Zr-2.5Nb hydrided sam-
ples, concluded that the most reliable point to associate
TTSS is msT [5]. D. Khatamian found the best correla-
tion for pT contrasting TTSSd determinations at pT, msT
and cT for unalloyed zirconium and Zr-20wt%Nb hy-
drided/deuterided samples with neutron diffraction
measurements [6]. Recently, the authors of the present
work determined TTSSd for Zr-2.5Nb with pressure tube
microstructure by DSC using DD as a contrasting tech-
nique. In this work, the difficulty of determining the best
point to measure TTSSd on the dissolution curves has
been discussed thoroughly. Yet, since the accurateness of
the DSC data is higher than the DD, it was not possible
to obtain conclusions about the best point for TTSSd
determination on the DSC curve from this comparison
[8].
In any case, it is evident that the selection of one of the
three criteria based on the measurements made with a
contrasting technique does not provide physical meaning
to the choice, turning it into the ‘true dissolution point’.
In fact, the certainty of this choice will be strongly de-
pendant on the capability of the contrasting technique to
detect the disappearance of very small hydrides at the
final stage of the dissolution process. In the present cir-
(0C) (0C)

P. VIZCAÍNO ET AL. 577
cumstances we judged that it would be most advisable to
choose the criteria that better agree with the highly ref-
erenced curve of Kearns [9] and the equilibrium solvus
line by Zuzek et al. [10], as done by other authors [3,4].
This comparison is shown in Figure 8. Regarding the
DSC data, the best agreement with Kearns and Zuzek
equilibrium curves corresponds to the completion point
(cT). Beyond the criterion chosen for TTSSd determina-
tion, the error at pT, msT or cT is always smaller than
2oC and the reproducibility is excellent.
Although the present comparison has shown that DD
is less accurate than DSC for solubility determinations, it
must be alleged in its favor that the technique was capa-
ble to detect the hydride dissolution for samples with
concentrations from 60 wppm-H. This implies that the
sensitivity is good enough to detect dimensional changes
at very low concentrations. Since the main objective of
the present work is to detect dimensional changes for
hydrogen contents like 200 or 300 wppm-H, typical of
cooling channels that remained for about 10 years in the
reactor at full power operation [11], the performance of
the technique is suitable for these purposes. This matter
will be developed in the following section.
4.2. Axial Elongation of a Cooling Channel
In order to simulate and determine the total elongation of
the cooling channels due to the hydrogen pick up in ser-
vice, a similar but faster process must be developed in
the laboratory. Hydrogen should be incorporated into the
bulk, starting from a hydrogen-free material. Instead of
the slow hydrogen incorporation due to the corrosion in
service, the hydrogen in the hydride layer diffuses into
the bulk during the heating ramp and the subsequent iso-
thermal annealing at 550oC. At this stage, the hydrogen
in the bulk remains in solid solution and the sample
reaches its maximum length. During cooling, the hydro-
gen precipitates as hydrides reducing the sample length,
but as it was shown in Figure 4, the final length is larger
than the initial. After cooling, the final dimension of the
sample is measured in situ in the dilatometer, obtaining a
differential value. The length increase due to the hydro-
gen incorporation into the bulk was measured at room
temperature and reported in a recent paper [1]. A linear
dependence on the hydrogen concentration was found for
fully recrystallized Zircaloy-4. For this type of micro-
structure, hydrides precipitate on the grain boundaries,
but some tendency to precipitate in the rolling direction
050100 150 200 250 300 350 400
100
200
300
400
500
DSC (pT)
Kearns
Zuzek
Temperature (oC)
[H] (wppm)
DSC (cT)
DD (knee)
Figure 8. Comparison between the curves obtained in the
present work and classical literature curves.
recalling the cold rolling process still subsists after the
recrystallization treatment. The competition of these two
ways of precipitation generates some scatter in the data.
However, a linear model seemed to be a good choice to
represent the expansion vs. [H]. The linear assumption
was made by simplicity, based on the values of the sta-
tistical parameters, considering an error of 0.5 m for the
micrometer, Table 1.
The following relation was obtained:
5.60.054 [] μm ΔL μmH
wppm
 (1)
Dividing Equation (1) by the initial length of the sam-
ples from which Equation (1) was obtained (L0 = 18600
m), the relative expansion is:
-4-6 1
)3.2 10 3.0010 []
room
0
ΔLH
Lwppm
  (2)
where (L/Lo)room is the relative increase after the hy-
drogen diffusion into the bulk at room temperature.
As the cooling channels operate in the two-phase field, in
order to determine the total expansion in service, the
contribution of both, the fraction of H atoms in solid so
lution and that of the zirconium hydrides at the reactor
operating temperature (300oC) should be added to the
growth due to the hydrides already present at room tem-
perature. The measurements made at 300oC are listed in
Table 2.
The relation found between the expansions and the
hydrogen concentration is linear too. Both, the data and
Table 1. Interception, slope, standard errors (SE) and lower and upper confidence limit (LCL and UCL). The standard de-
viation (SD) and R-value (R) are also given (97% of confidence).
Intercept Slope Statistics
Value SE LCL UCL Value SE LCL UCL R SD
5.6 2.0 0.6 10.7 0.054 0.004 0.044 0.064 0.9 5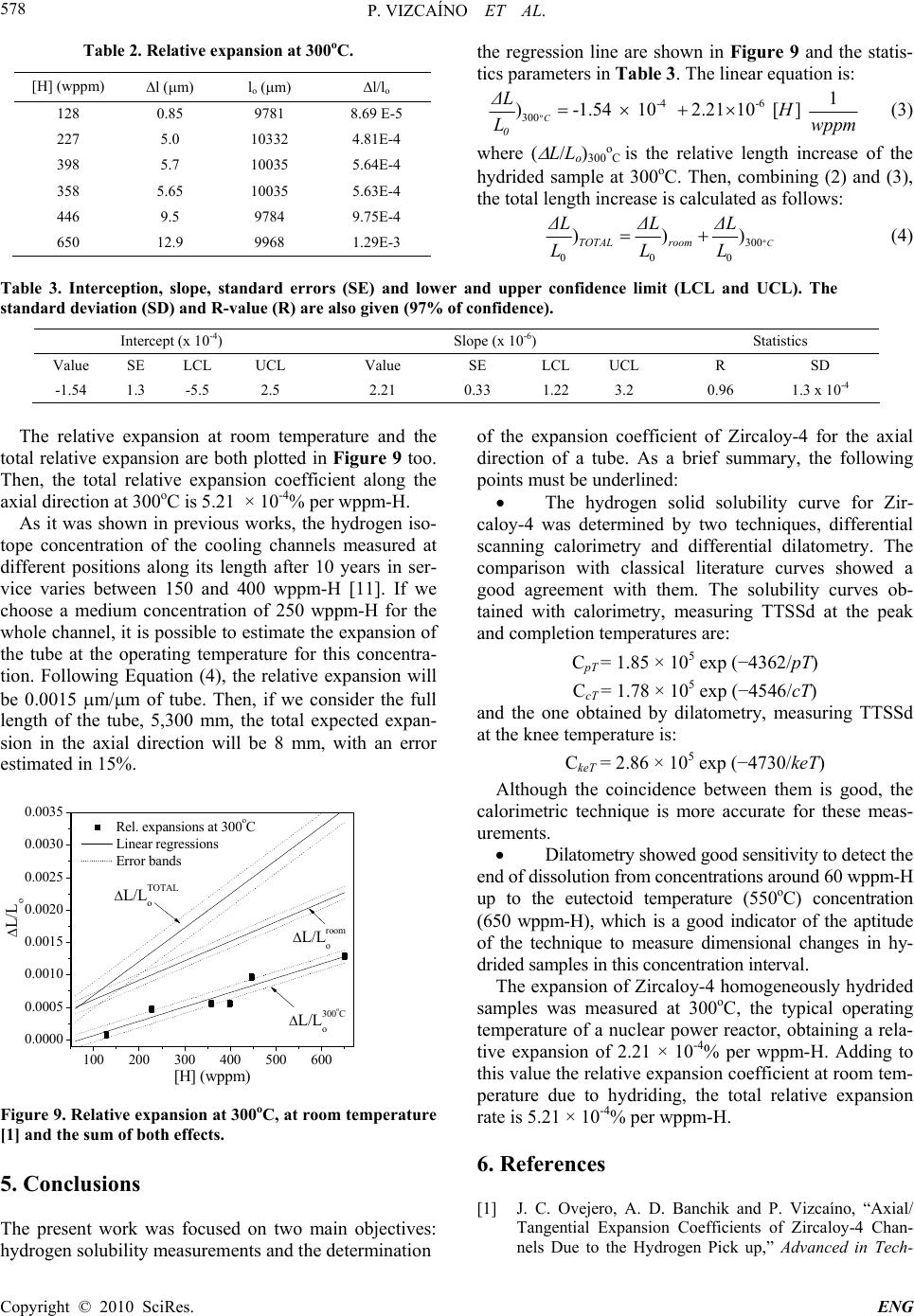

P. VIZCAÍNO ET AL.
578
Table 2. Relative expansion at 300oC.
[H] (wppm) l (m) lo (m) l/lo
128 0.85 9781 8.69 E-5
227 5.0 10332 4.81E-4
398 5.7 10035 5.64E-4
358 5.65 10035 5.63E-4
446 9.5 9784 9.75E-4
650 12.9 9968 1.29E-3
the regression line are shown in Figure 9 and the statis-
tics parameters in Table 3. The linear equation is:
º
-4 -6
300
1
)-1.54 10 2.2110 []
C
0
ΔLH
Lwppm
 (3)
where (  L/Lo)300oC is the relative length increase of the
hydrided sample at 300oC. Then, combining (2) and (3),
the total length increase is calculated as follows:
º300
000
)))
CTOTAL room
ΔLΔLΔL
LLL
 (4)
Table 3. Interception, slope, standard errors (SE) and lower and upper confidence limit (LCL and UCL). The
standard deviation (SD) and R-value (R) are also given (97% of confidence).
Intercept (x 10-4) Slope (x 10-6) Statistics
Value SE LCL UCL Value SE LCL UCL R SD
-1.54 1.3 -5.5 2.5 2.21 0.33 1.22 3.2 0.96 1.3 x 10-4
The relative expansion at room temperature and the
total relative expansion are both plotted in Figure 9 too.
Then, the total relative expansion coefficient along the
axial direction at 300oC is 5.21 × 10-4% per wppm-H.
As it was shown in previous works, the hydrogen iso-
tope concentration of the cooling channels measured at
different positions along its length after 10 years in ser-
vice varies between 150 and 400 wppm-H [11]. If we
choose a medium concentration of 250 wppm-H for the
whole channel, it is possible to estimate the expansion of
the tube at the operating temperature for this concentra-
tion. Following Equation (4), the relative expansion will
be 0.0015 m/m of tube. Then, if we consider the full
length of the tube, 5,300 mm, the total expected expan-
sion in the axial direction will be 8 mm, with an error
estimated in 15%.
100 200 300 400 500 600
0.0000
0.0005
0.0010
0.0015
0.0020
0.0025
0.0030
0.0035
L/LTOTAL
o
L/Lroom
o
L/L300oC
o
[H] (wppm)
Rel. expansions at 300oC
Linear regressions
Error bands
L/Lo
Figure 9. Relative expansion at 300oC, at room temperature
[1] and the sum of both effects.
5. Conclusions
The present work was focused on two main objectives:
hydrogen solubility measurements and the determination
of the expansion coefficient of Zircaloy-4 for the axial
direction of a tube. As a brief summary, the following
points must be underlined:
 The hydrogen solid solubility curve for Zir-
caloy-4 was determined by two techniques, differential
scanning calorimetry and differential dilatometry. The
comparison with classical literature curves showed a
good agreement with them. The solubility curves ob-
tained with calorimetry, measuring TTSSd at the peak
and completion temperatures are:
CpT = 1.85 × 105 exp (−4362/pT)
CcT = 1.78 × 105 exp (−4546/cT)
and the one obtained by dilatometry, measuring TTSSd
at the knee temperature is:
CkeT = 2.86 × 105 exp (−4730/keT)
Although the coincidence between them is good, the
calorimetric technique is more accurate for these meas-
urements.
 Dilatometry showed good sensitivity to detect the
end of dissolution from concentrations around 60 wppm-H
up to the eutectoid temperature (550oC) concentration
(650 wppm-H), which is a good indicator of the aptitude
of the technique to measure dimensional changes in hy-
drided samples in this concentration interval.
The expansion of Zircaloy-4 homogeneously hydrided
samples was measured at 300oC, the typical operating
temperature of a nuclear power reactor, obtaining a rela-
tive expansion of 2.21 × 10-4% per wppm-H. Adding to
this value the relative expansion coefficient at room tem-
perature due to hydriding, the total relative expansion
rate is 5.21 × 10-4% per wppm-H.
6. References
[1] J. C. Ovejero, A. D. Banchik and P. Vizcaíno, “Axial/
Tangential Expansion Coefficients of Zircaloy-4 Chan-
nels Due to the Hydrogen Pick up,” Advanced in Tech-

P. VIZCAÍNO ET AL. 579
nology of Materials and Materials Processing Journal,
Vol. 10, No. 1, 2008, pp. 1-8.
[2] C. P. Fagundez, P. Vizcaíno, D. Bianchi and A. D. Ban-
chik, “Dilatometría del Sistema Zr-H,” Proceedings of
the Congress SAM/CONAMET 2005, Mar del Plata,
18-21 October 2005.
[3] J. P. Giroldi, P. Vizcaíno, A. V. Flores and A. D. Banchik,
“Hydrogen Terminal Solid Solubility Determinations in
Zr-2.5 Nb Pressure Tube Microstructure in an Extended
Concentration Range,” Journal of Alloys and Compounds,
Vol. 474, No. 1-2, 2009, pp. 140-146.
[4] D. Khatamian and V. C. Ling, “Hydrogen Solubility Lim-
its In - and -Zirconium,” Journal of Alloys and Com-
pounds, Vol. 253-254, No. 20, 1997, pp. 162-166.
[5] A. McMinn, E. C. Darby and J. S. Schofield, “The Ter-
minal Solid Solubility of Hydrogen in Zirconium Al-
loys,” Proceedings of the 12th International Symposium
of the Zirconium in the Nuclear Industry, ASTM STP
1354, 2000, pp. 173-195.
[6] Z. L. Pan and M. P. Puls, “Precipitation and Dissolution
Peaks of Hydride in Zr-2.5 Nb during Quasistatic Ther-
mal Cycles,” Journal of Alloys and Compounds, Vol. 310,
No. 1-2, 2000, pp. 214-218.
[7] D. Khatamian and J. H. Root, “Comparison of TSSD
Results Obtained by Differential Scanning Calorimetry
and Neutron Diffraction,” Journal of Nuclear Materials,
Vol. 372, No. 1, 2008, pp. 106-113.
[8] P. Vizcaíno, A. D. Banchik and J. P. Abriata, “Calorimet-
ric Determination of the -Hydride Dissolution Enthalpy
in Zircaloy-4,” Metallurgical and Materials Transactions
A, Vol. 35A, No. 8, 2004, pp. 2343-2349.
[9] J. Kearns, “Terminal Solubility and Partitioning of Hy-
drogen in the Alpha Phase of Zirconium,” Journal of Nu-
clear Materials, Vol. 22, No. 3, 1967, pp. 292-303.
[10] E. Zuzek, J. P. Abriata and A. San Martín, “H-Zr (Hy-
drogen-Zirconium),” Bulletin of Alloy Phase Diagrams,
Vol. 11, No. 4, 1990, pp. 385-395.
[11] P. Vizcaíno, A. D. Banchik and J. P. Abriata, “Solubility
of Hydrogen in Zircaloy-4: Irradiation Induced Increase
and Thermal Recovery,” Journal of Nuclear Materials,
Vol. 304, No. 2-3, 2002, pp. 96-106.