J. Mod. Phys., 2010, 1, 171-174
doi:10.4236/jmp.2010.13025 Published Online August 2010 (http://www.scirp.org/journal/jmp)
Statistical Modeling of the Influence of Electron
Degeneracy on the Interatomic Interactions
Anatoly M. Dolgonosov
Vernadsky Institute of Geochemistry and Analytical Chemistry, Russian Academy of Sciences, Moscow, Russia
Received April 15, 2010; revised July 2, 2010; accepted July 30, 2010
Abstract
It is shown that electrons forming simple and multiple covalent bonds may have different contributions to the
interatomic interactions due to the degeneracy of electron states. A simple relationship between the length of
covalent bond, its order and atomic numbers of the interacting atoms is deduced.
Keywords: Interatomic Interactions, Covalent Bond, Degeneracy of Electron States, Theory of Generalized
Charges, Bond Length Ratio
1. Introduction
In the semiempirical methods describing interatomic
interactions, the contribution to the interaction energy of
-bond is assumed to be larger than that of -bond [1,2 ].
The ratio of - to -electron weight factors equal to 1.41
was empirically determined in [3,4] and checked many
times for the adsorption of unsaturated hydrocarbons.
We will show that this ratio can be evaluated from the
characteristics of covalent bonds (and vice versa).
It follows from the theoretical and empirical equations
found by London, Heitler, Lennard-Jon es, an d others th at
the parameters of atoms symmetrically enter the expres-
sion for interatomic bond energy [5]. A quasi-classical
method for describing the self-consistent field of multi-
component electron gas was developed in [6]. This
method enables us to express the interatomic interaction
energy. In particular it is shown that this energy is asso-
ciated with the volume V of each atom through a sym-
metrical operati on – v ol umes product

 
1212 12
121 212
,;
ij
ij
EfXr
X
VVv v


 (1)
where 12 r is the internuclear distance; v is a “volume”
of one electron, or the elementary volume, which is equal
to unity for a nondegenerate electron; V is the electronic
volume of the atom equal to the sum of elementary vol-
umes of its electrons; the indices enumerate all electrons
participating in bonding; and the figures at the summa-
tion symbol indicate summation over every electron of
the corresponding atom.
According to [6], the shielding radius, which is in-
versely proportional to the square root of the height of
the potential barrier that the electron overcomes, can be
used as a criterion of the participation of the electron in
interatomic interactions. A sphere centered at the atomic
nucleus with radius equal to the shielding radius of elec-
trons (the shielding sphere) bounds the electrons that
participate in bonding. An electron contributes to the
electronic volume of its atom only if the nucleus of the
atom with which the interaction is considered is situated
within the electron shielding sphere.
It is very important to distinguish between the elec-
tronic volume, the key concept of the theory of general-
ized charges developed in [6], and the corresponding
number of electrons, though these characteristics often
quantitatively coincide. It will be shown below that the
elementary volume of a degenerate electron larger than
that of a nondegenerate one, i.e. larger than unity.
2. Theory
Let us consider the sums in (1) in detail
 
 

12 12
12
1
2
ij
ij
ij ijij
ij ijij
VVv v
vv vvvv




  (2)
where 1
ij
vv

. The summation is over all possible
pairs of electrons. The coefficient ½ appears because
there are no limitations on the permutation of indices.
Let us apply (2) to pairs of - and -bond electrons.
For a -bond, the orbital moment projection (m) of its
electrons onto the internuclear axis has a single (zero)
value. A pair of -electrons has therefore one state only
 


212 12
11
2ij ji
vvvvv



.

A. M. DOLGONOSOV 172
For -bond electrons, the orbital moment projections
onto the same axis take equal values, either +1 or –1
(depending on whether the right- or left-handed coordi-
nate system is used; here we use atomic units of mo-
ment). We therefore have four terms for -bond electrons
  

21212 12
111
12 1
1[
2
]2
ijji ij
mmm
jim
vvvvv vv
vv

  

 

This gives 2v

. It follows that the n-fold state of
degeneracy corresponds to the n-fold increase in the sum
of pair electronic products, that enhances the elementary
volume of the pair of bond electrons, e vn. It is ne-
cessary to bear in mind that the electron balance condi-
tion imposes the following restriction: if aa VZ

,
then 1
e
v and, vice versa, if at least one e v value is
larger than 1, then aa VZ

.
The role of the product of volumes in describing the
interaction of atoms is clarified by the following identity:

2
12121 2
1
2
VVVVV V



(3)
The appropriately normalized electronic volume of an
atom corresponds to the probability of that its electrons
belong to the bond under consideration. In terms of
probability, the expression in brack ets is the excess value,
which appears as a result of bond formation.
On the other hand, the covalent bond energy is a func-
tion of the excess electron density in the internuclear
space
 
 


12
121 2
1 21212
**
*,
1
*2
lr l
rZZd d
ZZrr A
A





 




 



(4)
where 12
,
Z
Z are the charges of nuclei in the elemen-
tary charge units; d

is the space volume element; l is
the interatomic distance; and the bars denote the averag-
ing over the scale indicated in parentheses, which coin-
cides with one of the arguments of the functional relation.
The first integral equals the probability for an atomic
electron to occur between the planes passing through the
nuclei normally to the interatomic axis at distance 12 r
from each other. The second integral gives the same
probability at infinite interatomic distance. The integra-
tion in (4) is performed taking into account th at the elec-
tronic wave function in the internuclear space depends
on bond length. When atoms are infinitely separated, the
excess electron density is zero, and exactly one-half of
all the electrons occur in the internuclear space.
We can therefore write


12 12
EFr  


12 12
,
f
Xr
that gives




121 2
rgVV   (5)
Comparing (3) and (4) by their sense and taking into
account (5), we obtain

*
1 212121212
1
2
VVZ Zrrr
 

 


(6)
According to (6), the excess density in the intern uclear
space is proportional to the geometric mean of the prob-
abilities for atomic electrons to take part in the bond un-
der consideration.
To simplify (6), let us make the substitution
  
12
121 12212
12 12
11
22
ZZ
rrr
ZZ ZZ
 

(7)
where




12 exp; 1,2 aa a
ria    . We obtain:


** 12
12121212
1cos
2
VV
ZZ

 

  (8)
Let us clear the combinatorial meaning of

: its
square is the relative part of the cases when a pair of th e
particles belonging to two nonoverlapping sets of 1
Z
and 2
Z
particles occurs among the set of 12 VV

par-
ticles, where 1 V particles belong to the first set and 2 V
particles, to the second one. This interpretation suffers
from the shortcoming that the electronic volume is a
more complex concept that the number of electrons.
The above-mentioned quantity

12aa
r

  is the
characteristic of ato m depending on bond length . In such
a case the a

phase is a function of the scalar product
of the wave vector of atomic electrons (ka) and the in-
ternuclear vector


''
;
,'1,2;';0,1, 2,...
i
aa aaia aa
i
A
aaaa i
 


krkr (9)
The presence of even exponents in expansion (9) is
inessential because of their zero contribution to the dif-
ference of phases in (8) when the atoms are identical. For
the same reason, at least one of the odd constants Ai in (9)
is nonzero. When the internuclear distance in its tending
to zero falls beneath a certain value, the number of elec-
trons forming the bond becomes nonzero. Negative ex-
ponents are therefore absent in (9). The absolute term (i
= 0) in (8) is annulled and therefore does not play any
role. Thus, we can keep in (9) only the linear term, and
set, without loss of generality, the constant А1 equal to

A. M. DOLGONOSOV 173
unity

'aaaa
 kr ;


12 1212
  rk k (10)
Rewrite expression (8) taking into acco unt (10):
  
12 1212
coscos r rk  

rk k, (11)
where r
k
is the sum of projections of the wave vec-
tors of bond electron s on the bond axis. Finding this sum
from (11), w e get
1
12 arccos
r
kr



 (12)
The remarkable feature of r
k

is its independence
of

-electrons of the bond, because the limit value of

-electron moment projection on the bond axis corre-
sponds to the zero projection of its wave vector in this
direction
12
,0
rrr r
kk kk
 
 

(13)
Combining (11) with (12) and (13), we find the gen-
eral expression for a covalent bond

12
12 12
coscos arccos
r
r
rk r


 




 (14)
where primed and unprimed values relate to different
cases of the bond between the same atoms. Note that (14)
is valid for both double and triple bonds. Similar to (8),
we can write
12
12
VV
Z
Z



,
where V differs from the single bond property V by
the replacement of one or two electrons by -electrons of
double or trip le bond , corresp onding ly. Thus, th e number
of electrons forming a covalent bond is independent of
its order and, due to the above-introduced normalization
of electronic volume, is equal to the electronic volume of
atom for the case of single bond (a
V). Taking into ac-
count the value 2v

 obtained above, we find


21 1
aa
VV n
  
(15)
where 1,2, 3n is the bond order. In particular, it fol-
lows from (14) that for homo-nuclear bonds
12
12
cos arccos
rV
VZ rZ




(16)
It is quite natural to assume that a
V can differ from
a
Z
by the number of closed shell electrons (for which
the shielding radius is smaller than that for outer shell
electrons), equal to two for the second-period atoms that
yields 2
aa
VZ. This expression in combination with
(15) and (16) gives after simple transformations (see
Appendix)





12
12
22111
arcsinarcsin (17)
2
21 1
1(18)
2
n
r
ZZ r
n




The last result valid for large atomic numbers is valu-
able due to its independence of the kind of atoms, dis-
playing only the dependence of the bond orde r.
The calculation using (18) gives 12
12
0.890
r
r
 for
double bond and 12
12
0.765
r
r

 for triple bond. Formula
(18) is approximately valid for hetero-nuclear com-
pounds as well.
The comparison of the theoretical result obtained with
experimental data is given in the Tabl e.
For some possible compounds there is no information
on bond length. This lack of knowledge can be elimi-
nated by theoretical forecast. For example, the triple
bond of boron with carbon or nitrogen is possible in
principle. Its length will be about 24% shorter than the
corresponding single bond.
3. Conclusions
Thus, there is good compliance between experimental
and theoretical values that confirms the necessity to dis-
tinguish in interatomic interactions the contributions of
differently degenerated electrons. The contribution of

-electron to such an additive property of the interactive
atom as its electronic volume is in 2 times larger than
that of

-electron. This effect can be explained by dif-
ferent symmetries of the states with different degeneracy
of - and -electrons. In present work, the simple ex-
pression (17, 18) for covalent bond length ratio which
shows strong influence of bond orders and weak de-
pendence on atomic numbers is obtained.
Table. Ratios of the bond lengths for compounds of the
second-period elements: reference data [7,8] versus calcula-
tion results.
Atoms bonded Bond length ratio:
double to single
Bond length ratio:
triple to single
C-C 0.865 0.778
N-N 0.862 0.757
O-O 0.813(O2);
0.861(O3) 0.766(O2+)[8]
C-N 0.910 0.786
C-O 0.852 0.791
N-O 0.897 0.779(NO+)[8]
Theory:
formula (18) 0.890 0.765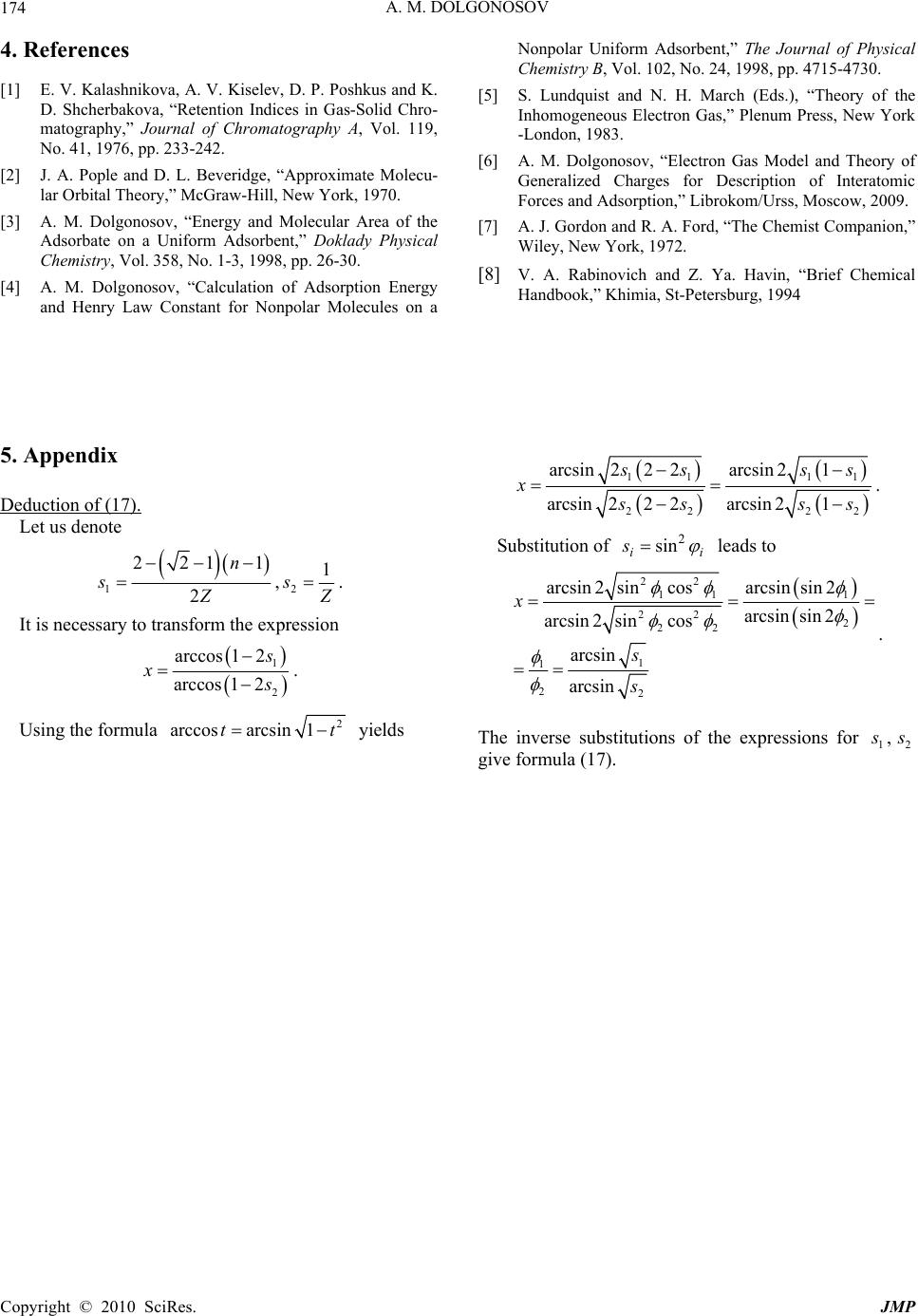

A. M. DOLGONOSOV 174
4. References
[1] E. V. Kalashnikova, A. V. Kiselev, D. P. Poshkus and K.
D. Shcherbakova, “Retention Indices in Gas-Solid Chro-
matography,” Journal of Chromatography A, Vol. 119,
No. 41, 1976, pp. 233-242.
[2] J. A. Pople and D. L. Beveridge, “Approximate Molecu-
lar Orbital Theory,” McGraw-Hill, New York, 1970.
[3] A. M. Dolgonosov, “Energy and Molecular Area of the
Adsorbate on a Uniform Adsorbent,” Doklady Physical
Chemistry, Vol. 358, No. 1-3, 1998, pp. 26-30.
[4] A. M. Dolgonosov, “Calculation of Adsorption Energy
and Henry Law Constant for Nonpolar Molecules on a
Nonpolar Uniform Adsorbent,” The Journal of Physical
Chemistry B, Vol. 102, No. 24, 1998, pp. 4715-4730.
[5] S. Lundquist and N. H. March (Eds.), “Theory of the
Inhomogeneous Electron Gas,” Plenum Press, New York
-London, 1983.
[6] A. M. Dolgonosov, “Electron Gas Model and Theory of
Generalized Charges for Description of Interatomic
Forces and Adsorption,” Librokom/Urss, Moscow, 2009.
[7] A. J. Gordon and R. A. Ford, “The Chemist Companion,”
Wiley, New York, 1972.
[8] V. A. Rabinovich and Z. Ya. Havin, “Brief Chemical
Handbook,” Khimia, St-Petersburg, 1994
5. Appendix
Deduction of (17).
Let us denote


1
2211
2
n
s
Z

,21 s
Z
.
It is necessary to transform the expression



1
2
arccos 12
arccos 12
s
x
s

.
Using the formula 2
arccosarcsin 1tt

 yields




11 11
22 22
arcsin222arcsin 21
arcsin222arcsin 21
s
sss
x
s
sss



.
Substitution of ii
s 
2
sin leads to


22
11 1
22 2
22
1
1
22
arcsin 2sincosarcsinsin 2
arcsinsin 2
arcsin 2sincos
arcsin
arcsin
x
s
s
 







.
The inverse substitutions of the expressions for 12
,
s
s
give formula (17).