Journal of Modern Physics, 2012, 3, 1842-1848
http://dx.doi.org/10.4236/jmp.2012.312231 Published Online December 2012 (http://www.SciRP.org/journal/jmp)
How Travels a Bohmian Particle?
Sofia Wechsler
Israel Institute of Technology, Haifa, Israel
Received August 31, 2012; revised October 13, 2012; accepted October 25, 2012
ABSTRACT
Bohm’s mechanics was built for explaining individual results in measurements, and mainly for getting rid of the enig-
matic reduction postulate. Its main idea is that particles have at any time definite positions and velocities. An additional
axiom is that particles follow continuous trajectories that admit the first derivative in time, the velocity. In the quantum
theory, if the position of a quantum object is well-defined at some time, a Δt time later the object may be found any-
where in space, so, the velocity defined as Δx/Δt is completely undefined. This incompatibility is regarded in standard
quantum theory as nature’s property. The disagreement between quantum and Bohm’s mechanics is particularly strong
in wave-like phenomena, e.g. interference. For a particle traveling through an interference fringe, Bohm’s velocity for-
mula shows a dependence of the time-of-flight on the fringe length. Such a dependence is not supported by the quantum
theory. Thus, for deciding which prediction is correct one has to measure times-of-flight. But this is a problem. If one
detects a particle at two positions and records the detection times, the time difference is meaningless, because the first
position measurement disturbs the particle’s Bohm velocity (if exists). This text suggests a way around: instead of
measuring positions and times, the particles are raised to an excited, unstable level, by passing them through a laser
beam. The unstable level will decay in time, s.t. the density of probability of the excited atoms will indicate the time
elapsed since excitation. For comparing the Bohmian and quantum predictions, this text proposes in continuation to
send the beam of excited particle upon a mirror. Bohm’s velocity leads to anomalies in the reflected wave.
Keywords: Bohmian Particle; Bohmian Velocity; Bohmian Trajectory; Group Velocity; Interference; Time-of-Flight
1. Introduction
Bohm’s mechanics (BM) was built with the purpose of
offering a simple and plausible alternative to the quan-
tum theory (QT). The latter doesn’t predicting measure-
ment results of individual systems, only the statistics
thereof, and regards this limitation as a property of the
nature,
“It requires us to give up the possibility of even
conceiving precisely what might determine the be-
havior of an individual system at the quantum level,
without providing adequate proof that such a renun-
ciation is necessary” [1,2].
BM has an opposite view,
“Permits us to conceive of each individual system as
being in a precisely definable state, whose changes
with time are determined by definite laws, analo-
gous to (but not identical with) the classical equa-
tions of motion” [1,2].
BM is a hidden variable theory. It assumes that at a
given time 0 a particle has a well-defined position, and
this is the hidden variable of the theory. BM assumes that
the density of probability for positions at t0 is given by
t

the absolute square of the wave-function, 0
2
Ψ, tr
0
t t

,
and proves, [3], that at any the density of prob-
2
Ψ, tr
ability of the positions is , the connection be-



Ψ, tr and tween

0
Ψ being given by Schrödin-
ger’s equation. (An extensive analysis of BM may be
found in [4]).
, tr

So far, no contradiction with QT seems to appear.
However, BM assumes an additional assumption, that
the Bohmian particle travels along a continuous trajec-
tory that admits also the first derivative with the time, the
velocity. For this velocity BM postulates the expression

1
, , tSt
M
rrv, (1)
where M is the mass of the particle, and S is the function
that appears in the exponent if the wave-function is put in
the form


ΨexpRS  
0
r1 r
with R and S real.
Then, if the Bohmian trajectory and velocity exist, the
time-of-flight between two points and on a tra-
jectory should be the integral

 
BM
01
flight , d, tLt
rr rv, (2)
C
opyright © 2012 SciRes. JMP

S. WECHSLER 1843
where the position vector sweeps the trajectory, dL is
the element of trajectory length, and is the pro-
jection upon dL of the Bohmian velocity at and t (the
time when the particle passes through the point ).
r

, trv
r
r
r
r
tt




1
1
, = 2πe,
, = 2πe.
xz
xz
κ
The standard QT disagrees with Equation (1). The un-
certainty principle forbids the coexistence of definite
values for position and velocity, s.t. Equation (2) is also
meaningless in QT.
A good tool for examining the Equations (1) and (2)
are experiments on single particle interference. As shown
in the next section, Equation (1) may entail that a Boh-
mian particle that enters an interference fringe is locked
in it and has to travel along it until the end of that fringe.
In consequence, if some fringes are longer and others
shorter as happens when a beam falls obliquely on a
mirror, the particles that enter short fringes have a short
way to go through the interference region, and the parti-
cles that enter long fringes have a long way through this
region. A difference in time-of-flight follows from this.
No such difference is predicted by the QT.
There remains a problem. In order to decide between
the two theories one has to measure experimentally the
times-of-flight. This is not a trivial task. The procedure
of sensing the particle (without absorption) when it
passes through the point 0 and recording the time t0,
then detecting the particle when passing through the
point 1 and recording the time t1, is worthless. The first
position measurement disturbs the Bohmian velocity (if
exists), s.t. the time difference is meaningless.
10
There is a wide literature on the arrival-time topic. Ar-
rival-time distributions and averages for different ex-
perimental configurations are calculated theoretically,
see for instance the review [5], the general treatment in
[6], and references therein. Though, how to measure
times-of-flight without the disturbance at t0, is not clear.
An interesting idea of Muga et al. [7] (see also [8]),
was to raise the particle to an unstable state by passing
the particle through a laser beam. The unstable state de-
cays with photon emission, and the photon detection in-
dicates the presence of the particle.
Although [7,8] don’t address the problem of the dis-
turbance at t0, the present text uses their idea for finding
an alternative to the position measurement at t0. The
movement of a beam of unstable atoms is studied. The
decay renders a set of such atoms more and more de-
pleted with the distance from the laser beam, s.t. the de-
gree of depletion of the set indicates how long time the
set traveled.
The beam is sent onto a mirror for atoms. An interfer-
ence tableau of non-maximal visibility is obtained, through
which the Bohm velocity (if exists) would drag the atoms
in such a way that abnormal effects would appear in the
reflected wave.
The following sections are organized as follows. Sec-
tion 2 illustrates the difference between the BM and QT
predictions on a simple, ideal case, then shows a possible
implementation. Section 3 examines the behavior of a
beam of unstable atoms reflected by a mirror and finds
the time-of-flight and the Bohmian trajectories. Section 4
comprises discussions.
2. Times-of-Flight of Bohmian Particles
through Interference Patterns
2.1. An Ideal Case
Consider a beam of particles falling on a perfectly re-
flecting mirror. For simplicity, let’s assume that the beam
is produced in a tilted form, Figure 1, (eventually by
means of fields). Let’s approximate the direct and the
reflected beam by plane waves,
x
zt
D
κx zωt
R
ψt
ψt




r
r
 
(3)
For the incidence angle of 45˚ one has .
xz
So, in the region of interference the wave function is







1
, , + , 2
2πecos
x
IDR
κxωt
ψtψtψt
κz


 


rrr
-

, trv

(4)
(The subscript “I” stands for “interference”).
Now, let’s find the trajectories of two Bohmian parti-
cles, 1 and 2, that pass simultaneously through the points
Q1, respectively Q2, Figure 1(a). We will work below
with a more practical expression for than (1),
 

, ,
, Im,
tt
t
Mt













rr
rr v, (5)
which is typically used in the literature. Substituting


D in this equation one gets for both particles the
Bohm velocity
ψr


xz M
 vv. Substituting

R
ψr
one gets for them
xz
M


vv .
However, in the fringes the wave-function expression
is (4), and the formula (5) yields the same
x
v0v, but z

.
That implies that once in a fringe, the Bohmian particle
travels along that fringe until the end of that fringe,
without passing from one fringe to another. At the fringe
end, the control of the particle is taken by the returning
wave


R, and the particle begins to move with the
Bohm velocity
ψr
xz
M


vv as calculated above.
Figure 1(a) shows the consequences of these facts,
and Figure 1(b) shows the quantum replica.
In Figure 1(a) one can see that particle 2 has a longer
way to the detector than particle 1. From the points Q1
and Q2 down to the dotted line, both particles travel equal
path-lengths, and so from the dotted line to the detector.
But in the fringes particle 1 passes immediately from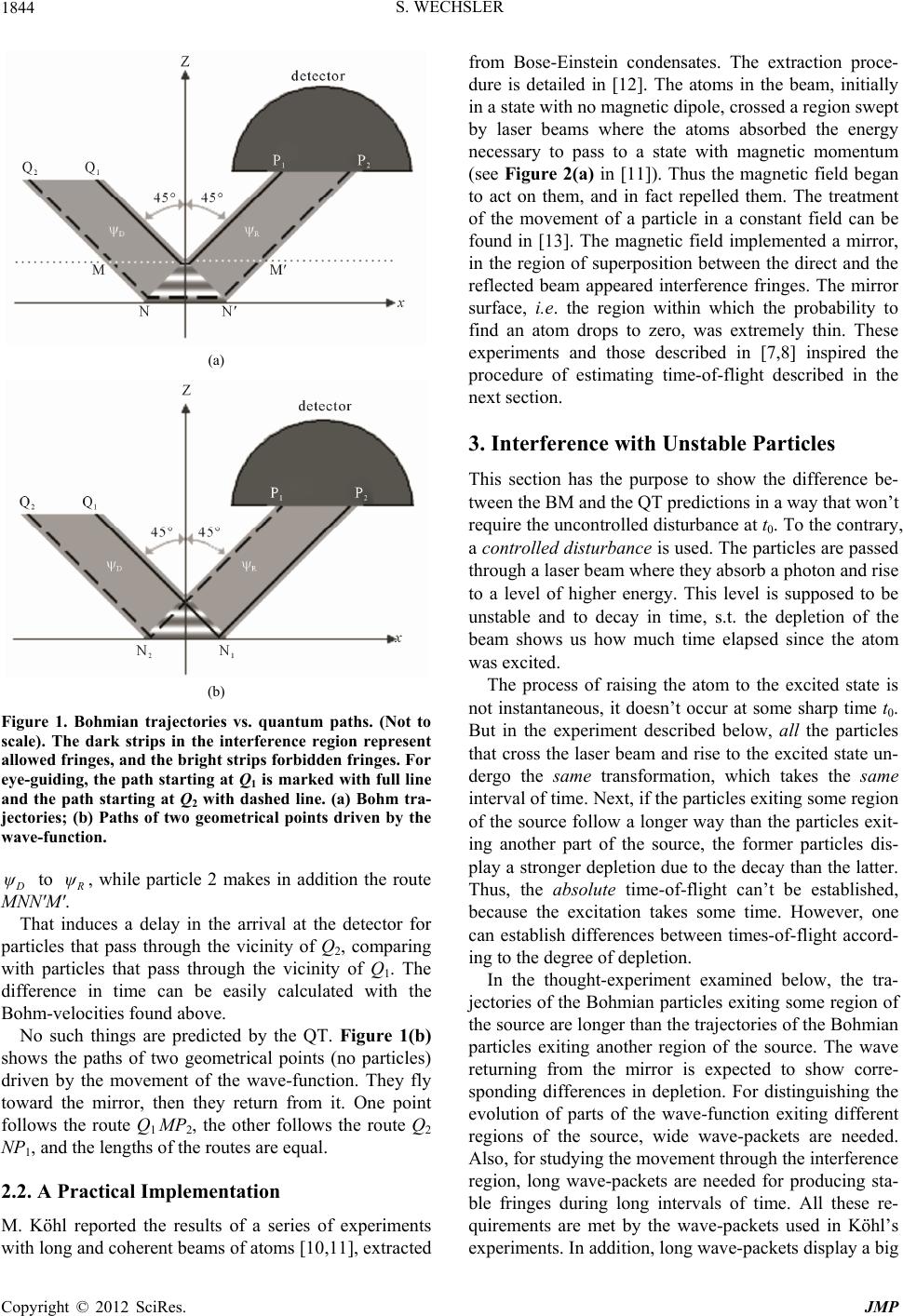

S. WECHSLER 1844
(a)
(b)
Figure 1. Bohmian trajectories vs. quantum paths. (Not to
scale). The dark strips in the interference region represent
allowed fringes, and the bright strips forbidde n fringes. For
eye-guiding, the path starting at Q1 is marked with full line
and the path starting at Q2 with dashed line. (a) Bohm tra-
jectories; (b) Paths of two geometrical points driven by the
wave-function.
D
ψ to
R
ψ, while particle 2 makes in addition the route
MNN'M'.
That induces a delay in the arrival at the detector for
particles that pass through the vicinity of Q2, comparing
with particles that pass through the vicinity of Q1. The
difference in time can be easily calculated with the
Bohm-velocities found above.
No such things are predicted by the QT. Figure 1(b)
shows the paths of two geometrical points (no particles)
driven by the movement of the wave-function. They fly
toward the mirror, then they return from it. One point
follows the route Q1 MP2, the other follows the route Q2
NP1, and the lengths of the routes are equal.
2.2. A Practical Implementation
M. Köhl reported the results of a series of experiments
with long and coherent beams of atoms [10,11], extracted
from Bose-Einstein condensates. The extraction proce-
dure is detailed in [12]. The atoms in the beam, initially
in a state with no magnetic dipole, crossed a region swept
by laser beams where the atoms absorbed the energy
necessary to pass to a state with magnetic momentum
(see Figure 2(a) in [11]). Thus the magnetic field began
to act on them, and in fact repelled them. The treatment
of the movement of a particle in a constant field can be
found in [13]. The magnetic field implemented a mirror,
in the region of superposition between the direct and the
reflected beam appeared interference fringes. The mirror
surface, i.e. the region within which the probability to
find an atom drops to zero, was extremely thin. These
experiments and those described in [7,8] inspired the
procedure of estimating time-of-flight described in the
next section.
3. Interference with Unstable Particles
This section has the purpose to show the difference be-
tween the BM and the QT predictions in a way that won’t
require the uncontrolled disturbance at t0. To the contrary,
a controlled disturbance is used. The particles are passed
through a laser beam where they absorb a photon and rise
to a level of higher energy. This level is supposed to be
unstable and to decay in time, s.t. the depletion of the
beam shows us how much time elapsed since the atom
was excited.
The process of raising the atom to the excited state is
not instantaneous, it doesn’t occur at some sharp time t0.
But in the experiment described below, all the particles
that cross the laser beam and rise to the excited state un-
dergo the same transformation, which takes the same
interval of time. Next, if the particles exiting some region
of the source follow a longer way than the particles exit-
ing another part of the source, the former particles dis-
play a stronger depletion due to the decay than the latter.
Thus, the absolute time-of-flight can’t be established,
because the excitation takes some time. However, one
can establish differences between times-of-flight accord-
ing to the degree of depletion.
In the thought-experiment examined below, the tra-
jectories of the Bohmian particles exiting some region of
the source are longer than the trajectories of the Bohmian
particles exiting another region of the source. The wave
returning from the mirror is expected to show corre-
sponding differences in depletion. For distinguishing the
evolution of parts of the wave-function exiting different
regions of the source, wide wave-packets are needed.
Also, for studying the movement through the interference
region, long wave-packets are needed for producing sta-
ble fringes during long intervals of time. All these re-
quirements are met by the wave-packets used in Köhl’s
experiments. In addition, long wave-packets display a big

S. WECHSLER 1845
indetermination in position, which entails a small inde-
termination in the linear momentum. In absence of fields,
such wave-packets can be well approximated by plane-
waves. To get an image of how long were these wave-
packets, one can look at Figure 1 in [12].
The inconvenient with Köhl’s experiments is that the
atoms were accelerated by fields, and that complicates
the calculi. In this section we will consider again the
ideal case in which the direct and the reflected beam
have, each, a quite well-defined linear momentum. (A
magnetic field will be used too, however for removing
unwanted particles). The question of how to implement
the mirror for atoms will be left aside, there are different
ways to do it and we won’t deal here with that.
3.1. A Thought-Experiment
Consider a long beam of atoms as in [10,11], prepared in
a state with magnetic number m. The atom beam
passes through a laser beam where the atom absorbs a
photon and jumps to a higher energy level with
1
0m

.
In continuation, the atom beam lands on a mirror and is
reflected, Figure 2. Suppose that the state with 0m

is
unstable and decays to a lower energy state with 1m

by emitting a photon. The magnetic field B pushes away
the atoms with from the atom beam. 1m

The decay of the excited state is assumed to obey the
exponential law 0 et PtP



 
, where P0 is the prob-
ability to find the atom in the unstable state at a certain
time taken as , and P(t) is the probability to find it
still in this state after an interval of time t.1 We study
here the beam of excited atoms. We will approximate the
direct and returning beam by plane waves as in the ex-
pressions (3), however we will take in consideration the
losses due to decay. For simplicity let’s assume
,
0t
xz
 

, + ,
2
ee
DR
tψtrr

, =
eψ
ψtr, (6)

2
e
1
, 2π
D
t
κx ztτ
 

e
D
ψt r, (7)

2
e
1
, 2π
R
t
κx κzωtτ   
e
R
ψt r.2 (8)
(The upper-script “e” stands for “excited”).
D
Figure 2. An interference experiment with unstable atoms.
The figure illustrates (not to scale) the depletion increasing
with the distance traveled from the laser beam. The de-
excited atoms are not shown. The level z = 0 is the mirror
surface. zI is the top level of the interference region. The
layer between the two horizontal lines is considered as
moving with the group velocity. C is a fix point in space.
when returning from the mirror. The group velocity of
our wave-packet is given by
t is the
time needed to a thin horizontal layer of the wave-packet
to travel from the top of the interference region, zI , to a
fix point C of height z;
R
tis the time interval since the
layer was at zI until its second visit of the layer at C, i.e.
κ
gr
M
v, [14], and
Since
x
z



, we have ,,
g
rx grz
vv. We can write
,,
,
II
DR
g
rz grz
zz zz
tt
vv


 ,
and we will assume in continuation
,
2
I
gr z
zv  
 

. (9)
Introducing tD, tR and the convention (9) in the Equa-
tions (7) and (8), the direct and the returning beam be-
come
1+
, 2πee
I
κx κzωtzz
e
D
ψt


r


, (10)
1+
, 2πee
I
κx κzωtzz
e
R
ψt


r

. (11)
From the Equations (6), (10) and (11) there results the
wave-function in the interference region,

11
e e
, e
8πe
II
zz
zz
κx - ωt
e
I
+
ψt
 


 


r

, (12)
whose intensity is



2
2
cosh2+ cos2
,
2πe
I e
I
zz κz
ψtr. (13)
1It is known that the decay doesn’t evolve always exponentially in time,
see [9], but here is addressed the typical situation.
2The fraction This intensity entails a z-dependence of the fringe
visibility V. Considering a small vicinity of some level z,
the fringe visibility is the ratio of the difference between

2tτ that appears here instead of tτ as in the decay
law, is due to the fact that the decay law refers to probabilities, while
the expressions (6), (7), (8), give amplitudes of probability.

S. WECHSLER 1846
the maximal and minimal intensity in that vicinity, divided
by the sum of the two. One gets



1cosh2
I
Vz zz
e
.
The next subsection examines the movement of the
Bohm particle through this pattern, and the implications.
3.2. The Bohm Velocity and the Time-of-Flight
For the calculus of the time-of-flight we will use the in-
tegral (2). Therefore, the Bohm velocity will be needed.
Introducing
D
ψ from (10) in the Equation (5), one
gets xz. Introducing vv   e M
R
ψ from (11) in (5)
one gets the same
x
v, but
z
v changes sign. Let’s no-
tice that these values are equal with the group velocity
components, ,,gr xgrz
In the interference region the things are more compli-
cated. One can check that
vv M  .
x
v remains the same, but, to
the difference from the experiment in Section 2, here
z
v
isn’t zero in the fringes. From the Equation (12) results
 

 

2
sin2
e
z
,
,
sinh 2
1
2π
I
I
I
I
t
tz
zz
z



 




 


r
r
.
Using this expression and the intensity (13) in the for-
mula (5) one obtains


sin 2
+
+ cos2
I
z
κz
zκz

sinh 2
cosh 2
I
z
I
zz
κ
Mz
 v. (14)
The quantity
I
κz is very big since the fringe width is
a couple of orders of magnitude smaller than zI. Thus,
 
sinh 2sin 2
I
I. Noticing that the lead-
ing factor in the RHS is the x component of the Bohm
velocity, (as calculated above,
κzκzzz
x
vM  ), we get


sinh
cosh 2
zx
I
vv zz  2
cos 2
I
zz
z
 . (15)
The leading sign “−” indicates that as long as a particle
is in the fringes, it only falls, never goes up, see Figure 3.
We will see in the next subsection the implications of
this fact.
With this velocity we can calculate the time-of-flight
of a Bohmian particle along a Bohmian trajectory.
Given two points A(xA, zA) and B(xB, z
B) on a Bohm
trajectory, we have according to the Equations (2) and
(15),



BM
flight
d
,
cosh 2+
1
sinh 2
A
B
A
B
z
zz
zI
z
z
tAB
zz




v
v
cos 2d
xI
κz
z
zz
Since the fringe width is extremely small comparing
Figure 3. Bohmian trajectories. The interference fringes are
not shown because they are too narrow. The black lines
represent a comb of Bohmian trajectories of the excited
atoms. The numbers on the top of the figure label the tra-
jectories in the comb. The trajectories 0 - 19 begin at equal
distances, while the trajectory 20 is slightly closer to 19.
with zI, the hyperbolic functions are practically constant
over intervals in which changes many times
and its values cancel mutually. There remains

cos 2κz
 

BM
flight
sinh2
, ln
sinh 2
AI
I
xBI
zz
z
tAB zz v2

. (16)
Note: this time-of-flight is between two points on the
same Bohmian trajectory and in the fringe region. Out-
side the fringe region
flight , AB AB
tABzz zz 
gr, zx
vv
BM QT
tt
. (17)
This expression is also valid in QT. Indeed, consider-
ing a thin layer that travels with the wave-packet, as we
considered in Section 3.1, the time of flight from A to B
is given by the Equations (17), with the sign “−” for a
direct flight from A to B, and the sign “+” for an indirect
flight, first from A to mirror, then from mirror to B.
Let’s repeat for the sake of clarity: outside the fringes
flightflight and is given by the expression (17), but in-
side the fringes BM gives for the time-of-flight the ex-
pression (16), while in QT is still valid (17).
3.3. Bohm Trajectories and the Reflected Wave
For the rationale that follows we will need the Bohmian
trajectories. Then, let’s first find their equation.
The x component of the Bohm velocity is constant and
the same inside and outside the interference region. So,
we can write for two points A(xA, zA) and B(x, z) on a
same Bohmian trajectory,

 
BM
flight , A
tABxx x v
BM
t
t
. (18)
Equating with flight from Equation (16) for the region
inside the fringes, and with flight from Equation (17) for
the regions outside the fringes, we get, respectively,


sinh2
lnsinh2
I
A
IAI
zz
xx
zzz
 . (19)


AA
x
xzz , (20)

S. WECHSLER 1847
where “−” is for the direct wave and “+” for the reflected
wave.
Figure 3 illustrates a comb of Bohmian trajectories,
labeled 0 - 20 that begin at equal distances, except for the
trajectory 20 which is slightly closer to 19. One can see
that the interference region behaves as a convergent lens
bringing the trajectories closer to one another. Toward
the bottom of the interference region the trajectories ag-
glomerate and some of them overlap. On the other hand,
the border between the interference region and the re-
flected wave e
R
ψ
e
has a divergent effect. In the reflected
wave the trajectories appear very rarefied.
These facts open a couple of problems.
1) In Figure 3 different trajectories overlap toward the
bottom of the interference region. However, as long as
the gradient of the wave-function is single-valued at each
point (which is the present case), QT doesn’t allow sev-
eral lines of flux to merge into one, or one flux line to
split into several.
Of course, examining the trajectories in Figure 3 at a
higher resolution it will be found that the apparently
overlapping trajectories are though separated by small
distances. But under a higher resolution one can draw a
denser comb of trajectories. Again there will be adjacent
trajectories that merge into one, and the problem will
reappear at the new scale.
1) Assume that trajectories don’t overlap, i.e. there is a
minimal distance between trajectories (assumption even
more plausible if one works with fermions). Still, another
problem appears.
Let’s imagine a transversal section through the direct
beam, and consider the set of excited atoms present on
this transversal section at the same time. Let’s denote by
δ the smallest distance between two atoms in this set.
That means, δ is the distance between two neighbor
Bohmian trajectories in
D
ψ
e
.
The requirement of simultaneity is needed because sets
of particles that begin their journey at different times
may have their nets of trajectories displaced, one net with
respect to the other. The distance between two trajecto-
ries that begin at different times may be arbitrarily small.
From the trajectory formulas (19) and (20) one finds
out that the trajectories that pass through the neighbor-
hood of the point C are about 16 times more rarefied than
they are in
D
ψ.
BM tells us that the trajectories passing through the
vicinity of C are short, see Figure 3, so the loss of parti-
cles by de-excitation is small and good statistics could be
gathered. Then one should get that the distance between
two particles detected at the same time in the vicinity of
the point C never falls below 16δ.
To the contrary, toward the RHS border of the re-
flected wave, the distance measured between two simul-
taneously detected particles should decrease. The calcu-
lus shows that on the RHS border this distance may be as
small as 30δ. Of course, BM tells us that the trajecto-
ries here are much longer, so the statistics is poor. Two
neighbor particles may not reach the detector together
because one of them was lost by de-excitation. Though,
examining many sets of simultaneously detected particles,
one should obtain sometimes distances smaller than the
minimal distance obtained in the neighborhood of the
point C.
QT does not confirm such effects.
4. Discussions
Bohm’s mechanics is a salutary trial to get rid of the
non-understandable reduction postulate of von Neumann.
The explanation that a click in a detector on the branch
a of the wave-function and the silence of the detectors
on the other branches, is caused by something in the
branch a that isn’t in the other branches, is most
plausible and appealing. Indeed, the detector doesn’t
click at its whim, it responds at a stimulus present in the
wave-function.
Vis-à-vis this explanation, the reduction postulate of-
fers no explanation on why this detector responds and the
others don’t.
It is therefore important to see if Bohm’s explanation,
together with the other assumptions of BM, are contra-
diction-free. If a contradiction though appears, it is de-
sirable to find which one of the assumptions causes it.
The present analysis puts under question mark Bohm’s
velocity formula.
In a theory that aims at producing the same predictions
as QT, the idea of simultaneously well defined values for
position and velocity raises suspicions. This text doesn’t
prove that this idea is wrong. It proves less, that Bohm’s
formula for velocity creates problems.
Whether this formula can be replaced by a better one
for building a Bohm-like mechanics, is still ahead to be
investigated. It wouldn’t be a simple task because Bohm’s
velocity formula fits very well in the continuity equation,
and any other formula should preserve this property.
Also, any Bohm-like mechanics should be able to explain
why in single particle interference, the probability to find
the particle in the bright fringes is bigger and in the dark
fringes smaller.
5. Acknowledgements
I wish to express my special thanks to Prof. Willem de
Muynck for important questions and remarks on different
parts of this work. I am also thankful to Prof. Detlef Dürr
for explanations about Bohm’s mechanics and for his
endless kindness. I thank to Dr. Michael Köhl for expla-
nations about the experiments with coherent atom beams,

S. WECHSLER 1848
and to Prof. Basil Hiley for relevant information and ma-
terial.
REFERENCES
[1] D. Bohm, “A Suggested Interpretation of the Quantum
Theory in Terms of ‘Hidden’ Variables. I,” Physical Re-
view, Vol. 85, No. 2, 1952, pp. 166-179.
doi:10.1103/PhysRev.85.166
[2] D. Bohm, “A Suggested Interpretation of the Quantum
Theory in Terms of ‘Hidden’ Variables. II,” Physical Re-
view, Vol. 85, No. 2, 1952, pp. 180-193.
doi:10.1103/PhysRev.85.180
[3] D. Dürr, S. Goldstein, R. Tumulka and N. Zanghì, “Boh-
mian Mechanics,” quant-ph/0903.2601, p. 3.
[4] D. Dürr and S. Teufel, “Bohmian Mechanics the Physics
and Mathematics of Quantum Theory,” Springer, 2008.
[5] J. G. Muga, R. Sala and J. P. Palao, “The Time of Arrival
Concept in Quantum Mechanics,” quant-ph/9801043v1.
[6] C. Anastopoulos and N. Savvidou, “Time-of-Arrival Prob-
abilities for General Particle Detectors,” quant-ph/1205.27
81v3.
[7] J. G. Muga, A. D. Baute, J. A. Damborenea and I. L.
Egusquiza, “Model for the Arrival-Time Distribution in
Fluorescence Time-of-Flight Experiments,” quant-ph/00
09111v1.
[8] J. A. Damborenea, I. L. Egusquiza, G. C. Hegerfeldt and J.
G. Muga, “Atomic Time-of-Arrival Measurements with a
Laser of Finite Beam Width,” quant-ph/0302201v1.
[9] C. Anastopoulos, “Time-of-Arrival Probabilities and Quan-
tum Measurements: III Decay of Unstable States,” quant-
ph/0706.2496v2.
[10] M. Köhl, T. W. Hänsch and T. Esslinger, “Measuring the
Temporal Coherence of an Atom Laser Beam,” cond-
mat/0104384v2.
[11] I. Bloch, M. Köhl, M. Greiner, T. W. Hänsch and T.
Esslinger, “Optics with an Atom Laser Beam,” Physical
Review Letters, Vol. 87, No. 3, 2001, Article ID: 030401.
doi:10.1103/PhysRevLett.87.030401
[12] I. Bloch, T. W. Hänsch and T. Esslinger, “Atom Laser
with a cw Output Coupler,” cond-mat/9812.258v1.
[13] L. D. Landau and E. M. Lifshitz, “Quantum Mechanics,”
Pergamon Press, Oxford, 1958, p. 24.
[14] G. Baym, “Lectures on Quantum Mechanics,” 3rd Edition,
W. A. Benjamin, Inc., Massachusetts, 1974, p. 64.