Open Journal of Applied Sciences, 2012, 2, 224-227
doi:10.4236/ojapps.2012.24033 Published Online December 2012 (http://www.SciRP.org/journal/ojapps)
HG-LG Mode Conversion with Stressed 3-Mode Fibers
under Polarization
Henning Soller
Institut für Theoretische Physik, Rupprecht-Karls-Universität Heidelberg, Heidelberg, Germany
Received September 11, 2012; revised October 14, 2012; accepted October 25, 2012
ABSTRACT
We have coupled an upright HG mode into a fiber-optic waveguide and used the application of stress to generate a
Laguerre-Gaussian laser mode. We have generalized previous results by McGloin et al. by using a polarized input beam,
a true 3-mode fiber and by applying the stress on a stripped piece of the optical waveguide. These generalizations are
necessary in order to perform quantum information experiments and obtain reliable information on the stress imposed
on the optical fiber.
Keywords: Mode Conversion; Stress; Optical Fiber; Laguerre-Gaussian Laser Mode
1. Introduction
Recently the production of Laguerre-Gaussian (LG) laser
modes has again attracted considerable interest [1]. It
arose mainly from the fact that these modes have a well
defined orbital angular momentum equal to
[2] which may be used to transfer information. The an-
gular momentum content of LG beams has been trans-
ferred successfully to microscopic particles [3] and a
comparison of the spin angular momentum has shown it
to be ([4,5]). Orbital angular momentum is
of special interest for quantum information processing
since different Fourier components of LG beams have
been shown to be independently addressable [6]. Also
their importance for producing heralded single photons in
arbitrary spatial modes has been addressed [7]. Four dif-
ferent possibilities for creating LG modes have been
discussed. First, LG beams can be produced from a laser
directly [8]. Furthermore, there are two classes of mode
converters for Hermite-Gaussian (HG) laser modes that
can be obtained more easily in open cavity lasers. The
first type of mode converter includes spiral phase plates
[9,10] and computer-generated holographic converters
[11,12]. In these devices, an azimuthal phase depen-
dence is introduced into an00 beam. The third class of
converters is based on cylindrical lenses [13,14]. Unlike
the methods using spiral phase plates or holographic
converters this method allows to produce pure LG modes.
Finally, the fourth type of converter uses a stressed fi-
ber-optic waveguide and an HG mode input laser [1].
Compression of the fiber optic waveguide causes the
non-cylindrically symmetricand modes to
experience differing phase velocities as they propagate
through the fiber. The nonzero relative phase shift is
controllable by the magnitude and direction of the ap-
plied stress. If these phase shifts are such that the two
HG modes undergo phase shifts that differ by 90˚ the
resultant field distribution is an LG mode with
photon/l
01
photon/l
HG
HG 10 HG
1

l
and 0

p.
In this paper we want to show how the result of [1] can
be generalized in three ways: first, in the previous ex-
periment the stress has been put onto the fiber directly.
We wanted to see whether it is possible to strip the fiber
and consequently allow to apply stress in a more con-
trolled manner. Second we did not want to use an HG
mode that is coupled in at a specific angle but just to use
an upright HG mode and see whether leaking of one HG
mode into the other inside the optical fiber can produce
the required angle by itself. Finally quantum information
experiments (e.g. [7]) require to do the experiments also
for polarized input beams and fibers as close to a 3-mode
fiber as possible. We will illustrate the possibility to pro-
duce Laguerre-Gaussian laser modes with heralding pho-
tons in the second part of this report.
2. Experiment
In this research we used a fiber of 1-m length. Using dif-
ferent types of optical fibers we made sure to have a real
3-mode fiber. Figure 1 shows the experimental apparatus
used to convert the HG input beam. The input laser was
forced to oscillate in an HG10 mode by the inclusion of
an intracavity cross wire. The output beam is polarized
nd expanded to fill the back aperture of a microscope a

H. SOLLER 225
Figure 1. The figure shows the experimental arrangement. We couple in an upright HG mode, that leaks into the rotated
mode and can therefore be transformed into an LG mode. The incoming mode is polarized and coupled into an optical
waveguide via a microscope objective. The stress is applied via lead blocks on the stripped fiber.
objective mounted on an x, y, z stage to couple the beam
into a 3-mode optical fiber (780 nm). The fiber was
stressed over a 100 mm region by lead weights put di-
rectly on top of the stripped fiber. One lead block on a
stage was put below the fiber and two old fiber pieces of
the same kind were used on the sides to get homogene-
ous stress.
The input mode of the optical fiber before the com-
pression stage may be assumed to be a 45 degree rotated
HG mode
4501 10
HGHG HG
, (1)
where we leave out normalization factors. Depending on
the stress induced a relative phase shift between the
modes occurs that may lead to a relative phase factor of
so that
π/2
eii
1
4501100
HGHGHGLG ,i
  (2)
Further stress leads to relative phase shift of
so that [1]
3π/2
eii
1
450110 0
HGHGHGLG ,i
 (3)
Consequently both linear momentum components of
the first LG mode can be addressed using the compres-
sion method.
The overall efficiency of this mode converter has been
measured to be approximately 2% - 3%. We should
stress that the usage of a 3-mode fiber instead of the 1.06
μm fiber used in [1] considerably complicates the cou-
pling but only this way higher order LG modes can be
discarded. We used different methods for stripping the
optical waveguide, e.g. burning the cladding, chemical
etching and mechanical stripping. Both chemical etching
and burning have caused considerable damage to the
optical waveguide and only mechanical stripping has
proven to allow for controllable operation of the fiber
afterwards.
We needed about 1.5 kg of lead to observe the mode
change to , again also under polarization. After the
addition of 1.5 kg of further lead blocks the output went
back to an HG mode rotated approximately 90˚ with re-
spect to the input mode. No further mode changes have
been observed even under extreme stress of about 11 kg
of lead. However, it is remarkable that the fiber works
even under such extreme conditions.
1
0
LG
3. Heralded Single Photons
This setup may now be used in combination with the
Sagnac interferometer described in [7] to produce her-
alded single photons, see Figure 2. In the setup a 45˚
rotated HG laser pump is used.
We had shown above that one could also use a dif-
ferent rotation and an optical fiber to achieve the required
rotated beam. Using the nonlinear crystal one achieves
that the resulting input state is predominantly a superpo-
sition of the vacuum and a two-photon state ph2  that
we can use to achieve the heralding of the photons. Since
the vacuum state is not relevant for us we focus on the

H. SOLLER 226
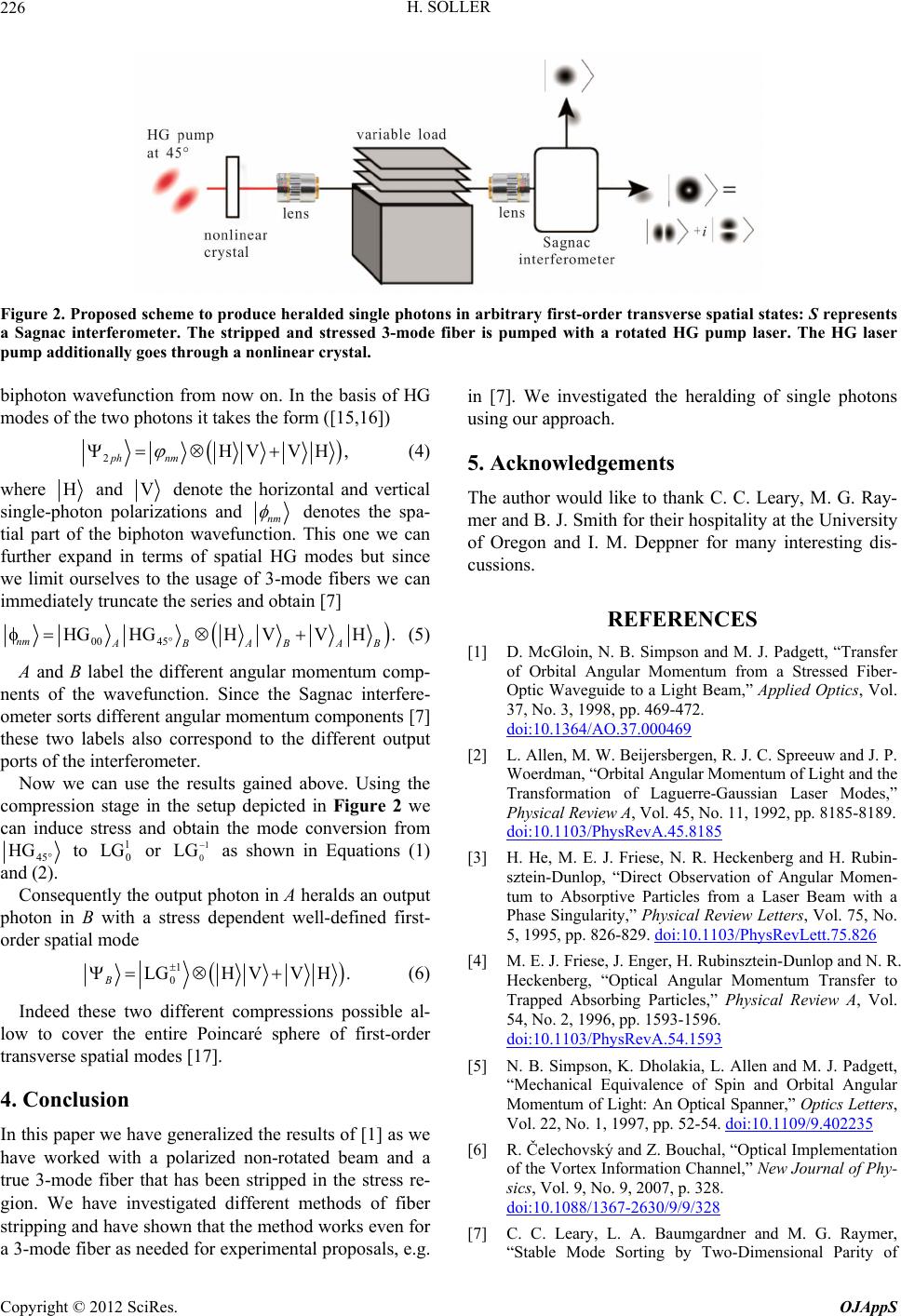
Figure 2. Proposed scheme to produce heralded single photons in arbitrary first-order transverse spatial states: S represents
a Sagnac interferometer. The stripped and stressed 3-mode fiber is pumped with a rotated HG pump laser. The HG laser
pump additionally goes through a nonlinear cry stal.
biphoton wavefunction from now on. In the basis of HG
modes of the two photons it takes the form ([15,16])

2HV VH, ph nm
   (4)
where H and V denote the horizontal and vertical
single-photon polarizations and nm

denotes the spa-
tial part of the biphoton wavefunction. This one we can
further expand in terms of spatial HG modes but since
we limit ourselves to the usage of 3-mode fibers we can
immediately truncate the series and obtain [7]

00 45
HG HGHV VH
nm AB ABAB 
 . (5)
A and B label the different angular momentum comp-
nents of the wavefunction. Since the Sagnac interfere-
ometer sorts different angular momentum components [7]
these two labels also correspond to the different output
ports of the interferometer.
Now we can use the results gained above. Using the
compression stage in the setup depicted in Figure 2 we
can induce stress and obtain the mode conversion from
45
HG  to or as shown in Equations (1)
and (2).
1
0
LG 1
0
LG
Consequently the output photon in A heralds an output
photon in B with a stress dependent well-defined first-
order spatial mode

1
0
LGHVVH.
B
  (6)
Indeed these two different compressions possible al-
low to cover the entire Poincaré sphere of first-order
transverse spatial modes [17].
4. Conclusion
In this paper we have generalized the results of [1] as we
have worked with a polarized non-rotated beam and a
true 3-mode fiber that has been stripped in the stress re-
gion. We have investigated different methods of fiber
stripping and have shown that the method works even for
a 3-mode fiber as needed for experimental proposals, e.g.
in [7]. We investigated the heralding of single photons
using our approach.
5. Acknowledgements
The author would like to thank C. C. Leary, M. G. Ray-
mer and B. J. Smith for their hospitality at the University
of Oregon and I. M. Deppner for many interesting dis-
cussions.
REFERENCES
[1] D. McGloin, N. B. Simpson and M. J. Padgett, “Transfer
of Orbital Angular Momentum from a Stressed Fiber-
Optic Waveguide to a Light Beam,” Applied Optics, Vol.
37, No. 3, 1998, pp. 469-472.
doi:10.1364/AO.37.000469
[2] L. Allen, M. W. Beijersbergen, R. J. C. Spreeuw and J. P.
Woerdman, “Orbital Angular Momentum of Light and the
Transformation of Laguerre-Gaussian Laser Modes,”
Physical Review A, Vol. 45, No. 11, 1992, pp. 8185-8189.
doi:10.1103/PhysRevA.45.8185
[3] H. He, M. E. J. Friese, N. R. Heckenberg and H. Rubin-
sztein-Dunlop, “Direct Observation of Angular Momen-
tum to Absorptive Particles from a Laser Beam with a
Phase Singularity,” Physical Review Letters, Vol. 75, No.
5, 1995, pp. 826-829. doi:10.1103/PhysRevLett.75.826
[4] M. E. J. Friese, J. Enger, H. Rubinsztein-Dunlop and N. R.
Heckenberg, “Optical Angular Momentum Transfer to
Trapped Absorbing Particles,” Physical Review A, Vol.
54, No. 2, 1996, pp. 1593-1596.
doi:10.1103/PhysRevA.54.1593
[5] N. B. Simpson, K. Dholakia, L. Allen and M. J. Padgett,
“Mechanical Equivalence of Spin and Orbital Angular
Momentum of Light: An Optical Spanner,” Optics Letters,
Vol. 22, No. 1, 1997, pp. 52-54. doi:10.1109/9.402235
[6] R. Čelechovský and Z. Bouchal, “Optical Implementation
of the Vortex Information Channel,” New Journal of Phy-
sics, Vol. 9, No. 9, 2007, p. 328.
doi:10.1088/1367-2630/9/9/328
[7] C. C. Leary, L. A. Baumgardner and M. G. Raymer,
“Stable Mode Sorting by Two-Dimensional Parity of

H. SOLLER 227
Photonic Transverse Spatial States,” Optics Express, Vol.
17, No. 4, 2009, pp. 2435-2452.
doi:10.1364/OE.17.002435
[8] M. Harris, C. A. Hill and J. M. Vaughan, “Optical Helices
and Spiral Interference Fringes,” Optics Communications,
Vol. 106, No. 4-6, 1994, pp. 161-166.
doi:10.1016/0030-4018(94)90314-X
[9] M. W. Beijersbergen, R. P. C. Coerwinkel, M. Kristensen
and J. P. Woerdman, “Helical-Wavefront Laser Beams
Produced with a Spiral Phaseplate,” Optics Communica-
tions, Vol. 112, No. 5-6, 1994, pp. 321-327.
doi:10.1016/0030-4018(94)90638-6
[10] G. A. Turnbull, D. A. Robertson, G. M. Smith, L. Allen
and M. J. Padgett, “The Generation of Free-Space La-
guerre-Gaussian Modes at Millimetre-Wave Frequen-
cies by Use of a Spiral Phaseplate,” Optics Communica-
tions, Vol. 127, No. 4-6, 1996, pp. 183-188.
doi:10.1016/0030-4018(96)00070-3
[11] N. R. Heckenberg, R. McDuff, C. P. Smith and A. G.
White, “Generation of Optical Phase Singularities by
Computer-Generated Holograms,” Optics Letters, Vol. 17,
No. 3, 1992, pp. 221-223. doi:10.1364/OL.17.000221
[12] N. R. Heckenberg, R. McDuff, C. P. Smith, H. Rubinsz-
tein-Dunlop and M. J. Wegener, “Laser Beams with
Phase Singularities,” Optical and Quantum Electronics,
Vol. 24, No. 9, 1992, pp. 951-962.
doi:10.1007/BF01588597
[13] E. Abramochkin and V. Volostnikov, “Beam Transfor-
mations and Nontransformed Beams,” Optics Communi-
cations, Vol. 83, No. 1-2, 1991, pp. 123-135.
doi:10.1016/0030-4018(91)90534-K
[14] M. W. Beijersbergen, L. Allen, H. E. L. O. van der Veen
and J. P. Woerdman, “Astigmatic Laser Mode Converters
and Transfer of Orbital Angular Momentum,” Optics Com-
munications, Vol. 96, No. 1-3, 1993, pp. 123-132.
doi:10.1016/0030-4018(93)90535-D
[15] P. G. Kwiat, K. Mattle, H. Weinfurter, A. Zeilinger, A. V.
Sergienko and Y. Shih, “New High Intensity Source of
Polarisation-Entangled Photon Pairs,” Physical Review
Letters, Vol. 75, No. 24, 1995, pp. 4337-4341.
doi:10.1103/PhysRevLett.75.4337
[16] S. P. Walborn, S. Pádua and C. H. Monken, “Conser-
vation and Entanglement of Hermite-Gaussian Modes in
Parametric Down-Conversion,” Physical Review A, Vol.
71, No. 5, 2005, p. 053812.
doi:10.1103/PhysRevA.71.053812
[17] S. J. van Enk, “Geometric Phase, Transformations of
Gaussian Light Beam and Angular Momentum Transfer,”
Optics Communications, Vol. 102, No. 1-2, 1993, pp. 59-
64. doi:10.1016/0030-4018(93)90472-H