Journal of Minerals and Materials Characterization and Engineering, 2012, 11, 1085-1094
Published Online November 2012 (http://www.SciRP.org/journal/jmmce)
Wear Behaviour of Al-SiCp Metal Matrix Composites and
Optimization Using Taguchi Method and
Grey Relational Analysis
Shouvik Ghosh, *Prasanta Sahoo, Goutam Sutradhar
Department of Mechanical Engineering, Jadavpur University, Kolkata, India
Received July 25, 2012; revised August 28, 2012; accepted September 7, 2012
ABSTRACT
Aluminium metal matrix composite is a relatively new material that has proved its position in automobile, aerospace
and other engineering design applications due to its wear resistance and substantial hardness. Need for improved tri-
bological performance has led to the design and selection of newer variants of the composite. The present investigation
deals with the study of wear behaviour of Al-SiCp metal matrix composite for varying reinforcement content, applied
load, sliding speed and time. Aluminium metal matrix composites reinforced with SiC particles are prepared by liquid
metallurgy route using LM6 alumin ium alloy an d silicon carb ide particles (size ~ 37 µm) by varying the weight fraction
of SiC in the range of 5% - 10%. The material is synthesized by stir casting process in an electric melting furnace. The
materials are then subjected to wear testing in a multitribotester using block on roller configuration. A plan of experi-
ments based on L27 Taguchi orthogonal array is used to acq uire the wear data in a controlled way. An analysis of vari-
ance is employed to investigate the influence of four controlling parameters, viz., SiC content, normal load, sliding
speed and sliding time on dry sliding wear of the compos ites. It is observed that SiC conten t, sliding speed and normal
load significantly affect the dry sliding wear. The optimal combination of the four controlling parameters is also ob-
tained for minimum wear. The microstructure study of worn surfaces indicates nature of wear to be mostly abrasive.
Keywords: Metal Matrix Composite; Al-SiCp; Wear; Optimization; Grey-Taguchi
1. Introduction
Metal Matrix Composites (MMC) synthesized by incor-
porating hard ceramic particles like Silicon Carbide (SiC)
into aluminium alloys achieve good mechanical proper-
ties. These composites are both light weight and show
good hardness property which qualifies it as structural
material especially for wear resistant and weight critical
applications. Such applications motivate researchers to
study the wear behaviour of this category of metal matrix
composites.
The composites are synthesized by different techniques
but mostly pressure infiltration technique [1], powder
metallurgy [2-6] and stir casting techniques [7-9] are used.
Researchers in general consider the volume fraction of
reinforcement silicon carbide in the range of 0% - 30%
[2-21]. Some researchers have used higher volume frac-
tion in the range of 60% [1] and 10% - 40% [19,20].
The wear tests are conducted by varying applied load
and sliding speed. Al-Rubaie et al. [2,3] studied the abra-
sive wear behaviour of Al-SiC MMC by varying the
volume fraction of SiC reinforcement in the range of 5%
- 20% and particle size 10, 27 and 43 µm. Thus a varied
range of abrasive study was conducted and the results
showed that wear rates increase with increase in abrasive
particle size but decreased with increase in volume frac-
tion. Another abrasive wear test conducted by Ahlatci et
al. [1] for varying particle size of Al2O3 abrasive particle
infers that with increase in particle size of Al2O3 the wear
rate increased. Thus the infiltration of SiC increases the
abrasive wear resistance of the aluminium alloy.
The effect of applied load on wear behaviour of Al-
5%SiC and Al-10%SiC was studied by Chen et al. [8].
The results suggested that with increase in volume frac-
tion of reinforcement particle th e wear rate increased but
with gradual increase in applied load the wear rates de-
creased. Chen et al. [9] considered the effect of heat treat-
ment on the fretting wear behaviour of Al-SiC MMC syn-
thesized by reinforcing 15 vol% SiC in A356 aluminium
alloy and observed that heat treatment of the composite
increases the hardness of the mater ial thus increasing fret-
ting wear resistance.
Wear behaviour of Al-Mg-Cu alloy reinforced with SiC
*Corresponding author.

S. GHOSH ET AL.
1086
particle were studied by Hassan et al. [11]. The compara-
tive study of alloy and alloy reinforced with SiC sug-
gested that wear resistant property of the alloy increased
considerably with addition of SiC particle. Another simi-
lar study of wear behaviour was performed by Kwok and
Lim [13]. High speed wear tests were performed on alu-
minium alloy reinforced with SiC particle. The compos-
ites were prepared by three different powder metallurgy
techniques using different matrix metal, reinforcement
volume fraction and reinforcement particle size. The
wear studies were conducted at varying load, speed. The
wear rates are found to increase with increase in applied
load and sliding speed.
In most of the studies the researchers have considered
particle reinforcement while some of the researchers
have used whisker reinforcement [4,5,12]. Bai et al. [4]
incorporated aluminium 2024 alloy with 15 vol% SiC
whiskers to study the wear behaviour of the material. The
wear tests were performed in an oscillating wear tester
using a 52100 steel ball as counter material. The test re-
sults indicated that Al-SiC composite showed better wear
resistance that the aluminium alloy. Another wear study
was conducted b y the same research group using Al-Mo-
SiC composite [5]. The composite was fabricated by rein-
forcing 15 vol% SiC and 15 vol% Mo in aluminium 2024
alloy. Wear tests were performed at both dry and lubri-
cated condition. Liquid paraffin and sulphurized olefin
was used as lubricants. The study reveals that inclusion
of molybdenum powder into the composite increases the
wear resistance of the alloy. It is also revealed that wear
rates decrease in presence of lubricants. Iwai et al. [12]
performed dry sliding wear test on Al-SiC composited
reinforced with SiC whisker. The volume fraction of SiC
whisker was in the range of 0% - 16%. The wear rates are
found to decrease gradually with increase in reinforce-
ment volume fraction of SiC whisker into the aluminium
alloy.
Straffelini et al. [14] studied the effect of applied load
and temperature on the wear behaviour of the composite
and found that with increasing load the contact tempera-
ture increases beyond 1500 C which increases the wear
rate of the material. Rao and Das [16] considered the ef-
fect of sliding distance on the wear behaviour of Al-SiC
and reported that wear rates increase with increase in
load and sliding speed while wear resistance improves
with heat treatment. Sharma et al. [17] studied the effect
of volume fraction of reinforcement SiC. A volume frac-
tion range of 1% - 5% was chosen and wear t est s were car-
ried out at varying loads and sliding speeds. It was re-
ported that wear rates decrease with increase in volume
fraction of SiC but increase with increase in applied load
and sliding speed.
It can be seen that researchers mostly have chosen pro-
cess parameters like volume fraction, applied load, slid-
ing speed and reinforcement particle size. Some resear-
chers have varied some different process parameters to
study the wear behaviour of the composite. Rao and Das
[15] studied the effect of matrix alloy on the wear be-
haviour of Al-SiC composite. Three different matrix al-
loys were used for casting of Al-SiC composite using
10%, 15% and 25% SiC reinforcement. The wear tests
showed that the matrix alloy with highest percentage of
copper had higher wear rate than the rest. The allo y with
highest percentage of zinc showed lower wear rate than
the rest. Addition of SiC reinforcement decreased the
wear rate for all the composites. Another study was con-
ducted by Yalcin and Akbulut [21] using two different
melting routes for fabricatio n of Al-SiC. For th e material
fabrication a volume fraction range of 0% - 20% was
chosen. The wear test conducted at varying load indicated
that wear rates decrease with increase in volume fraction
of SiC reinforcement. The wear rates of material fabri-
cated by vortex method decrease less rapidly than the
materials prepared by dilution material with increase in
SiC volume fraction and applied load. Similar studies on
wear behaviour of Al-SiC composite have also been re-
ported by other researchers [22,23].
From the review of existing literature it is apparent
that many studies have been carried out on the wear be-
haviour of Al-SiC particulate composite, but no study is
available on optimization of process parameters for mini-
mum wear response. The present study considers opti-
mization of wear behaviour of Al-SiCp composite using
Taguchi orthogonal design with four process parameters
viz. volume fraction of reinforcement, applied load, slid-
ing speed and time. A multi tribotester with block on roller
configuration is used for the wear test. The test results
are analyzed for optimal combination of process para-
meters for minimum wear. A confirmation test is done to
validate the optimal combinatio n of process parameter as
predicted by Taguchi method. Furthermore, Analysis of
Variance (ANOVA) is carried out to analyze the effect of
process parameters and their in teractions on the wear be-
haviour of the material. The wear mechanism is studied
using Scanning Electron Microscopy (SEM) of worn sur-
faces.
2. Taguchi Method
The Taguchi method [24,25] is a powerful tool for de-
signing high quality systems based on Orthogonal Array
(OA) experiments that provide much reduced variance
for the experiments with an optimum setting of process
control parameters. It introduces an integrated approach
that is simple and efficient to find the best range of de-
signs for quality, performance and computational cost.
This method achieves the integration of Design of Ex-
periments (DOE) [26] with the parametric optimization
of the process yielding the desired results. Th e traditional

S. GHOSH ET AL. 1087
experimental design procedures focus on the average
process performance characteristics. But the Taguchi me-
thod concentrates on the effect of variation on the process
quality characteristics rather than on its averages. That is,
the Taguchi approach makes the process performance
insensitive (robust) to variation in uncontrolled or noise
factors. Taguchi recommends that this can be done by the
proper design of parameters during the “parameter de-
sign” phase of off-line quality control. He designed cer-
tain standard OAs by which simultaneous and indepen-
dent valuation of two or more parameters for their ab ility
to affect the variability of a particular product or process
characteristic can be done in a minimum number of tests.
Using OA, the Taguchi method explores the entire design
space through a small number of experiments in order to
determine all of the parameter effects and several of the
interactions. These data are then used to predict the op-
timum combination of the design parameters that will
minimize the objective function and satisfy all the con-
straints. In addition to locating a near optimum objective
function, the Taguchi method provides information on
parameter trends and noise sensitivities thereby enabling
a robust design. The parameter design phase of the Ta-
guchi method generally includes the following steps: 1)
identify the objective of the experiment; 2) identify the
quality characteristic (performance measure) and its mea-
surement systems; 3) identify the factors that may influ-
ence the quality characteristic, their levels and possible
interactions; 4) select the appropriate OA and assign the
factors at their levels to the OA; 5) conduct the test de-
scribed by the trials in the OA; 6) analysis of the experi-
mental data using the Signal-to-Noise (S/N) ratio, factor
effects and the Analysis of Variance (ANOVA) to see
which factors are statistically significant and to find the
optimum levels of factors; 7) verification of the optimal
design parameters through confirmation experiment. The
OA requires a set of well- balanced (minimum experi-
mental runs) experiments. The Taguchi method uses a
statistical measure of performance called (S/N) ratios,
which are logarithmic functions of desired output to serve
as objective functions for optimization. The S/N ratio
takes both the mean and the variability into account and
is defined as the ratio of the mean (signal) to the standard
deviation (noise). The ratio depends on the quality char-
acteristics of the product/process to be optimized. The
three categories of S/N ratios are used: Lower the Better
(LB), Higher th e Better (HB) and Nominal the Best (NB).
The parameter level combination that maximizes the ap-
propriate S/N ratio is the optimal setting. For the case of
minimization of wear, LB characteristic needs to be used.
Furthermore, a statistical Analysis of Variance (ANOVA)
[27] is performed to find which process parameters are
statistically significant. With the S/N ratio and ANOVA
analyses, the optimal combination of the process para-
meters can be predicted. Finally, a confirmation experi-
ment is conducted to verify the optimal process parame-
ters obtained from the parameter design.
3. Experimental Details
3.1. Material Processing
For the fabrication process aluminium alloy, LM6 is used
as matrix metal that has been reinforced with SiC parti-
cles of 400 mesh size (size ~ 37 µm). The reinforcement
percentage (herein termed as volume fraction of rein-
forcement) is varied in the range 5% - 10% by weight.
The chemical composition of the matrix material (LM6)
is given in Table 1. The composite material is fabricated
by liquid metal stir casting process since it is both simple
and less expensive. The small ingots of LM6 are melted
in clay graphite crucible using an electric resistance fur-
nace and 3 wt% Mg is added with the liquid metal, in
order to achieve a strong bonding by decreasing the sur-
face energy (wetting angle) between the matrix alloy and
the reinforcement particles. The addition of pure magne-
sium also enhances the fluidity of the molten metal. Be-
fore mixing of the silicon carbide particles with the liqu id
LM6, the particles are preheated at 850˚C - 900˚C for 2 -
3 hours to make their surface oxidized. The melt is me-
chanically stirred by using a mild steel impeller and then
the pre-heated silicon carbide particles are added to the
stirred liquid metal. The processing of the composite is
carried out at a temperature of 750˚C with a stirrin g speed
of 400 - 500 rpm. The melt is then poured into a green
silica sand mould. The material is then cooled and sam-
ples for wear testing are prepared by different machining
processes.
3.2. Design of Experiment
DOE technique allows carrying out modelling and ana-
lysis of the influence of process variables on the response
variables. The response variables are the unknown func-
tions of the process variables also known as Design fac-
tors. Design factors or control factors are those which are
varied during the experimental tests. From the literature
it was clear that many tribological process parameters
can affect the wear behaviour of Al-SiC composites. But
it is impossible to consider all process parameters in a
single study. For the current study of wear behaviour of
Al-SiC, the control parameters chosen are volume fraction
Table 1. Chemical composition of LM6.
Si (10 - 13) Cu (0.1) Mg (0.1)
Fe (0.6) Mn (0.5) Ni (0.1)
Zn (0.1) Pb (0.1) Ti (0.2)
Elements (%)
Rest Al

S. GHOSH ET AL.
1088
of reinforcement (V), applied load (L), sliding speed (S)
and sliding time (T). Table 2 shows the design factors
with their levels. Three levels for each parameter are con-
sidered so that the non-linear effects if any can be ob-
served. Since wear behaviour is to be optimized, wear is
taken as response variable.
Based on Taguchi method, an Orthogonal Array (OA)
is considered to reduce the number of experiments re-
quired to determine the optimal wear for Al-SiC metal
matrix composite. The orthogonal array provides the
shortest possible matrix of combination in which all the pro-
cess parameters are varied to consider their direct effect
as well as interactions simultaneously. For this experi-
mental purpose L27 orthogon al array is cho sen . Selection
of proper orthogonal array is done based on the total
number of Degrees of Freedom (DOF). The L27 OA has
27 rows corresponding to the number of tests and the de-
gree of freedom is 26 with 13 columns with three levels.
The degree of freedom of each design factor is 2 and for
two way interaction of the factors the DOF is 4. So, the
total degree of freedom for the conducted experiment is
(2 × 4 + 4 × 3 = 20). As per Taguchi method the total
DOFs of selected OA must be greater than or equal to the
total DOFs required for the experiment. Thus, the L27
OA is chosen for the present case. The 1st column is as-
signed to volume fraction (V), 2nd column is assigned to
applied load (L), 5th column is assigned to sliding speed
(S) and 9th column is assigned to time (T) or duration of
wear test. Six columns are assigned to the two ways in-
teractions of the first three factors while the remaining
three columns are error terms. Table 3 shows the or
Table 2. Design factors with levels.
Levels
Design Factors Unit 1 2 3
Volume fraction
of reinforcement (V) % by weight 5 7.5i 10
Load (L) N 50 75i 100
Speed (S) RPM 180 200i 220
Time (T) MIN 20 30i 40
i = initial condition
Table 3. L27 Orthogonal Array with design factors.
Column
Trial
No. 1 (V) 2 (L) 3 (V × L) 4 (V × L) 5 (S) 6 (V × S) 7 (V × S) 8 (L × S) 9 (T) 10 - 11 (L×S) 12 - 13 -
1 1 1 1 1 1 1 1 1 1 1 1 1 1
2 1 1 1 1 2 2 2 2 2 2 2 2 2
3 1 1 1 1 3 3 3 3 3 3 3 3 3
4 1 2 2 2 1 1 1 2 2 2 3 3 3
5 1 2 2 2 2 2 2 3 3 3 1 1 1
6 1 2 2 2 3 3 3 1 1 1 2 2 2
7 1 3 3 3 1 1 1 3 3 3 2 2 2
8 1 3 3 3 2 2 2 1 1 1 3 3 3
9 1 3 3 3 3 3 3 2 2 2 1 1 1
10 2 1 2 3 1 2 3 1 2 3 1 2 3
11 2 1 2 3 2 3 1 2 3 1 2 3 1
12 2 1 2 3 3 1 2 3 1 2 3 1 2
13 2 2 3 1 1 2 3 2 3 1 3 1 2
14 2 2 3 1 2 3 1 3 1 2 1 2 3
15 2 2 3 1 3 1 2 1 2 3 2 3 1
16 2 3 1 2 1 2 3 3 1 2 2 3 1
17 2 3 1 2 2 3 1 1 2 3 3 1 2
18 2 3 1 2 3 1 2 2 3 1 1 2 3
19 3 1 3 2 1 3 2 1 3 2 1 3 2
20 3 1 3 2 2 1 3 2 1 3 2 1 3
21 3 1 3 2 3 2 1 3 2 1 3 2 1
22 3 2 1 3 1 3 2 2 1 3 3 2 1
23 3 2 1 3 2 1 3 3 2 1 1 3 2
24 3 2 1 3 3 2 1 1 3 2 2 1 3
25 3 3 2 1 1 3 2 3 2 1 2 1 3
26 3 3 2 1 2 1 3 1 3 2 3 2 1
27 3 3 2 1 3 2 1 2 1 3 1 3 2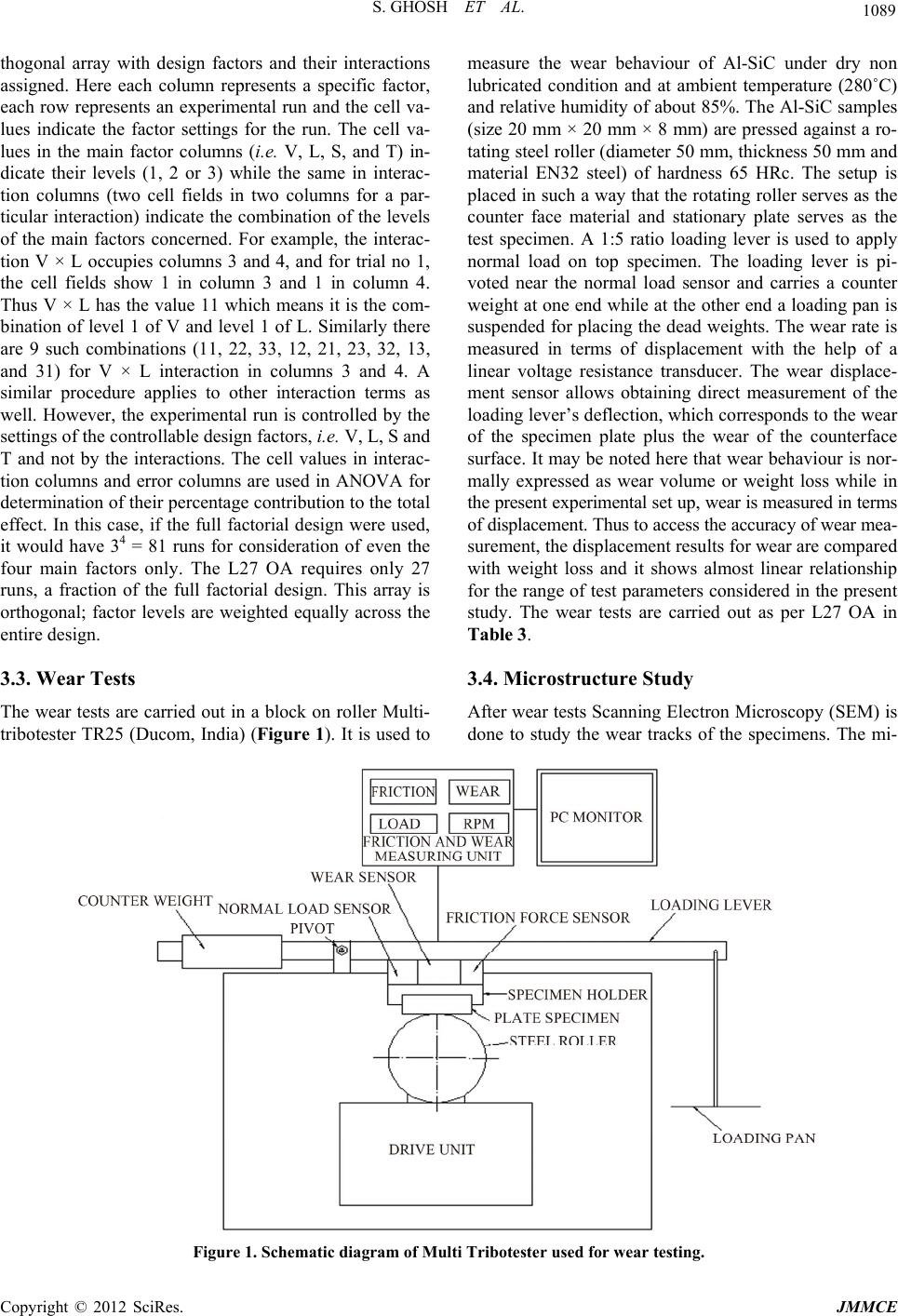

S. GHOSH ET AL. 1089
thogonal array with design factors and their interactions
assigned. Here each column represents a specific factor,
each row represents an experimental run and the cell va-
lues indicate the factor settings for the run. The cell va-
lues in the main factor columns (i.e. V, L, S, and T) in-
dicate their levels (1, 2 or 3) while the same in interac-
tion columns (two cell fields in two columns for a par-
ticular interaction) indicate the combination of the levels
of the main factors concerned. For example, the interac-
tion V × L occupies columns 3 and 4, and for trial no 1,
the cell fields show 1 in column 3 and 1 in column 4.
Thus V × L has the value 11 which means it is the com-
bination of level 1 of V and level 1 of L. Similarly there
are 9 such combinations (11, 22, 33, 12, 21, 23, 32, 13,
and 31) for V × L interaction in columns 3 and 4. A
similar procedure applies to other interaction terms as
well. However, the experimental run is controlled by the
settings of the controllable design factors, i.e. V, L, S and
T and not by the interactions. The cell values in interac-
tion columns and error columns are used in ANOVA for
determination of their percentage contribution to the total
effect. In this case, if the full factorial design were used,
it would have 34 = 81 runs for consideration of even the
four main factors only. The L27 OA requires only 27
runs, a fraction of the full factorial design. This array is
orthogonal; factor levels are weighted equally across the
entire design.
3.3. Wear Tests
The wear tests are carried out in a block on roller Multi-
tribotester TR25 (Ducom, India) (Figure 1). It is used to
measure the wear behaviour of Al-SiC under dry non
lubricated condition and at ambient temperature (280˚C)
and relative humidity of abo ut 85%. The Al-SiC samples
(size 20 mm × 20 mm × 8 mm) are pressed against a ro-
tating steel roller (diameter 50 mm, thickness 50 mm and
material EN32 steel) of hardness 65 HRc. The setup is
placed in such a way that the rotating roller serves as the
counter face material and stationary plate serves as the
test specimen. A 1:5 ratio loading lever is used to apply
normal load on top specimen. The loading lever is pi-
voted near the normal load sensor and carries a counter
weight at one end while at the other end a loading pan is
suspended for placing the dead weights. The wear rate is
measured in terms of displacement with the help of a
linear voltage resistance transducer. The wear displace-
ment sensor allows obtaining direct measurement of the
loading lever’s d eflection, which corresponds to th e wear
of the specimen plate plus the wear of the counterface
surface. It may be noted here that wear behaviour is nor-
mally expressed as wear volume or weight loss while in
the present experimental set u p, wear is measured in terms
of displacement. Thus to acces s the accuracy of wear mea-
surement, the displacement results for wear are compared
with weight loss and it shows almost linear relationship
for the range of test parameters considered in the present
study. The wear tests are carried out as per L27 OA in
Table 3.
3.4. Microstructure Study
After wear tests Scanning Electron Micro scopy (SEM) is
done to study the wear tracks of the specimens. The mi-
Figure 1. Schematic diagram of Multi Tribotester used for wear testing.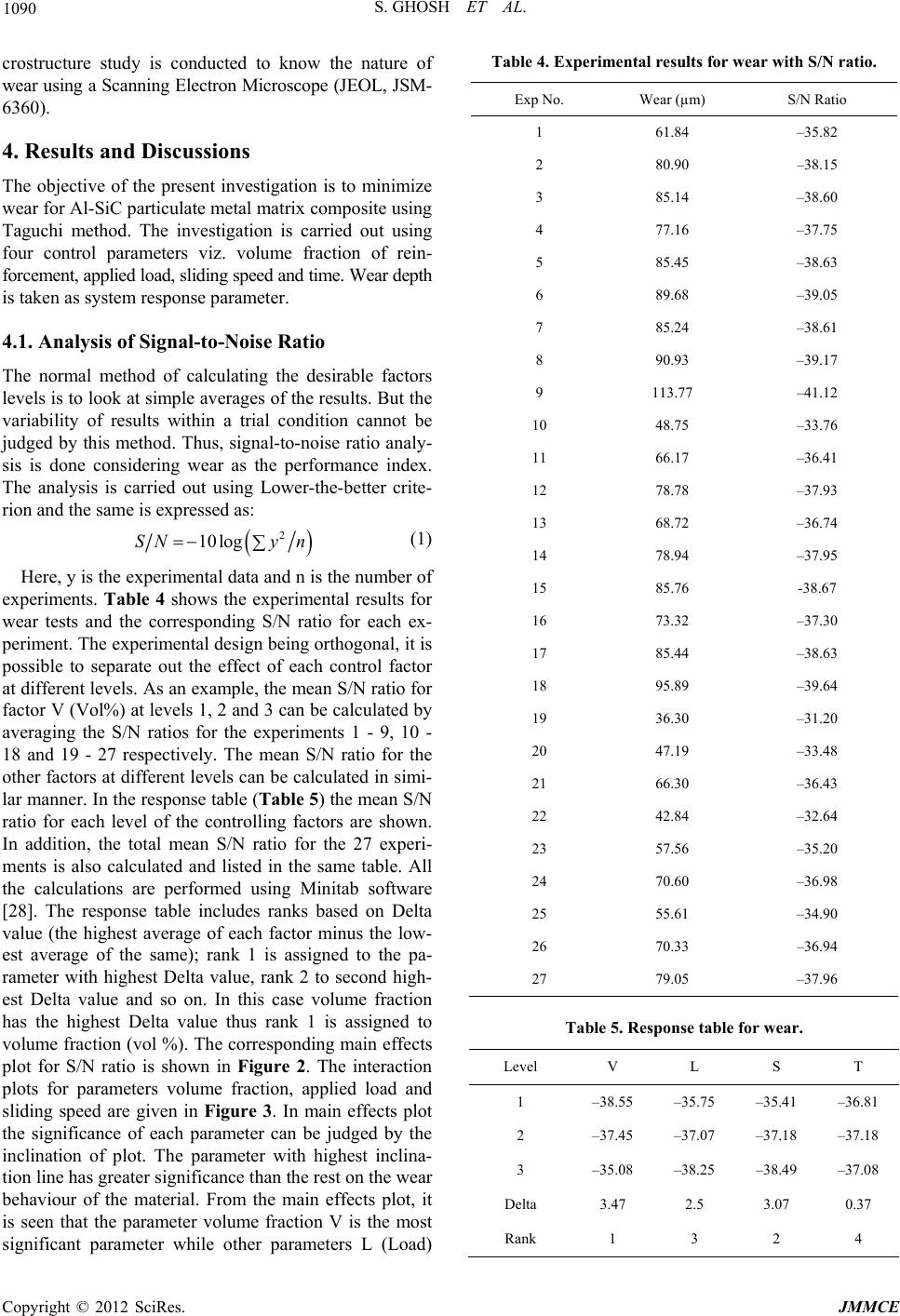

S. GHOSH ET AL.
1090
crostructure study is conducted to know the nature of
wear using a Scanning Electron Microscope (JEOL, JSM-
6360).
4. Results and Discussions
The objective of the present investigation is to minimize
wear for Al-SiC particulate metal matrix composite using
Taguchi method. The investigation is carried out using
four control parameters viz. volume fraction of rein-
forcement, app lied lo ad, sl id ing sp eed and time . Wear depth
is taken as system response parameter.
4.1. Analysis of Signal-to-Noise Ratio
The normal method of calculating the desirable factors
levels is to look at simple averages of the results. But the
variability of results within a trial condition cannot be
judged by this method. Thus, signal-to-noise ratio analy-
sis is done considering wear as the performance index.
The analysis is carried out using Lower-the-better crite-
rion and the same is expressed as:

2
10logSNy n 

(1)
Here, y is the experimental data and n is the number of
experiments. Table 4 shows the experimental results for
wear tests and the corresponding S/N ratio for each ex-
periment. The experimental design being orthogonal, it is
possible to separate out the effect of each control factor
at different levels. As an example, the mean S/N ratio for
factor V (Vol%) at levels 1, 2 and 3 can be calculated by
averaging the S/N ratios for the experiments 1 - 9, 10 -
18 and 19 - 27 respectively. The mean S/N ratio for the
other factors at different levels can be calculated in simi-
lar manner. In the response table (Table 5) the mean S/N
ratio for each level of the controlling factors are shown.
In addition, the total mean S/N ratio for the 27 experi-
ments is also calculated and listed in the same table. All
the calculations are performed using Minitab software
[28]. The response table includes ranks based on Delta
value (the highest average of each factor minus the low-
est average of the same); rank 1 is assigned to the pa-
rameter with highest Delta value, rank 2 to second high-
est Delta value and so on. In this case volume fraction
has the highest Delta value thus rank 1 is assigned to
volume fraction (vol %). The corresponding main effects
plot for S/N ratio is shown in Figure 2. The interaction
plots for parameters volume fraction, applied load and
sliding speed are given in Figure 3. In main effects plot
the significance of each parameter can be judged by the
inclination of plot. The parameter with highest inclina-
tion line has greater significance than the rest on the wear
behaviour of the material. From the main effects plot, it
is seen that the parameter volume fraction V is the most
significant parameter while other parameters L (Load)
Table 4. Experimental results for wear with S/N ratio.
Exp No. Wear (µm) S/N Ratio
1 61.84 –35.82
2 80.90 –38.15
3 85.14 –38.60
4 77.16 –37.75
5 85.45 –38.63
6 89.68 –39.05
7 85.24 –38.61
8 90.93 –39.17
9 113.77 –41.12
10 48.75 –33.76
11 66.17 –36.41
12 78.78 –37.93
13 68.72 –36.74
14 78.94 –37.95
15 85.76 -38.67
16 73.32 –37.30
17 85.44 –38.63
18 95.89 –39.64
19 36.30 –31.20
20 47.19 –33.48
21 66.30 –36.43
22 42.84 –32.64
23 57.56 –35.20
24 70.60 –36.98
25 55.61 –34.90
26 70.33 –36.94
27 79.05 –37.96
Table 5. Response table for wear.
Level V L S T
1 –38.55 –35.75 –35.41 –36.81
2 –37.45 –37.07 –37.18 –37.18
3 –35.08 –38.25 –38.49 –37.08
Delta 3.47 2.5 3.07 0.37
Rank 1 3 2 4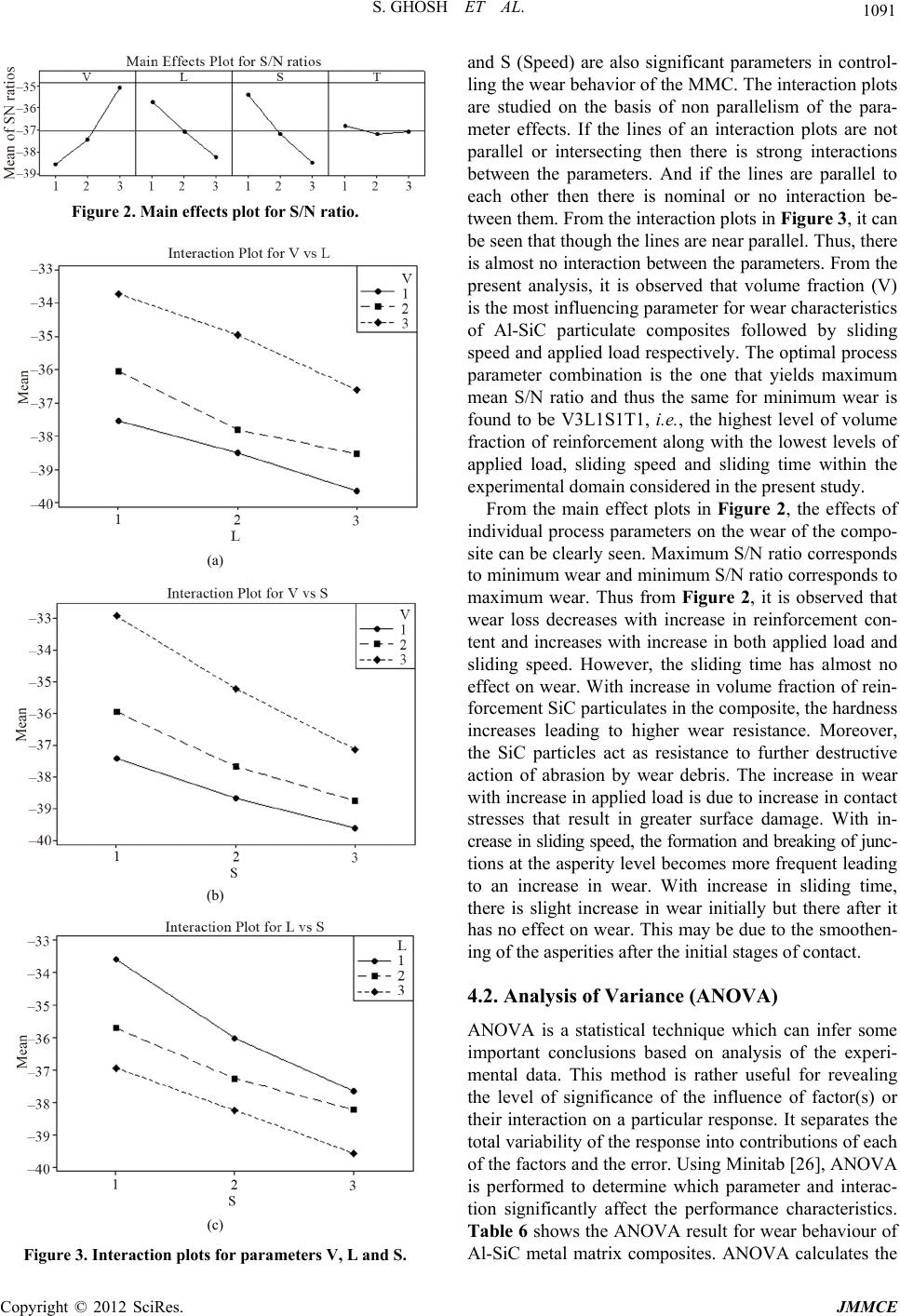

S. GHOSH ET AL. 1091
Figure 2. Main effects plot for S/N ratio.
(a)
(b)
(c)
Figure 3. Interaction plots for parameters V, L and S.
and S (Speed) are also significant parameters in control-
ling the wear behavior of the MMC. The interaction plots
are studied on the basis of non parallelism of the para-
meter effects. If the lines of an interaction plots are not
parallel or intersecting then there is strong interactions
between the parameters. And if the lines are parallel to
each other then there is nominal or no interaction be-
tween them. From the interaction plots in Figure 3, it can
be seen that though the lines are near parallel. Thus, there
is almost no in teraction between the paramet ers. From the
present analysis, it is observed that volume fraction (V)
is the most influencing parameter for wear characteristics
of Al-SiC particulate composites followed by sliding
speed and applied load respectively. The optimal process
parameter combination is the one that yields maximum
mean S/N ratio and thus the same for minimum wear is
found to be V3L1S1T1, i.e., the highest level of volume
fraction of reinforcement along with the lowest levels of
applied load, sliding speed and sliding time within the
experimental domain considered in the present study.
From the main effect plots in Figure 2, the effects of
individual process parameters on the wear of the compo-
site can be clearly seen. Maximum S/N ratio corr esponds
to mi ni mu m w e ar an d mi ni mu m S /N ra ti o co rr es po nd s t o
maximum wear. Thus from Figure 2, it is observed that
wear loss decreases with increase in reinforcement con-
tent and increases with increase in both applied load and
sliding speed. However, the sliding time has almost no
effect on wear. With increase in volume fraction of rein-
forcement SiC particulates in the composite, the hardness
increases leading to higher wear resistance. Moreover,
the SiC particles act as resistance to further destructive
action of abrasion by wear debris. The increase in wear
with increase in applied load is due to in crease in contact
stresses that result in greater surface damage. With in-
crease in sliding speed, the formation and breaking of junc-
tions at the asperity level beco mes more frequent leading
to an increase in wear. With increase in sliding time,
there is slight increase in wear initially but there after it
has no effect on wear. This may be due to the smoothen-
ing of the asperities after the initial stages of contact.
4.2. Analysis of Variance (ANOVA)
ANOVA is a statistical technique which can infer some
important conclusions based on analysis of the experi-
mental data. This method is rather useful for revealing
the level of significance of the influence of factor(s) or
their interaction on a particular response. It separates the
total variability of the response into con tributions of each
of the factors and the error. Using Minitab [26], ANOVA
is performed to determine which parameter and interac-
tion significantly affect the performance characteristics.
Table 6 shows the A NOVA result for wear behaviour of
Al-SiC metal matrix composites. ANOVA calculates the

S. GHOSH ET AL.
1092
F-ratio, which is the ratio between the regression mean
square and the mean square error. The F-ratio, also called
the variance ratio, is the ratio of variance due to the ef-
fect of a factor and variance due to the error term. This
ratio is used to measure the significance of the parame-
ters under investigation with respect to the variance of all
the terms included in the error term at the desired sig-
nificance level, α. If the calculated value of the F-ratio is
higher than the tabulated value of the F-ratio, then the
factor is significant at a desired α level. In general, when
the F value increases the significance of the parameter
also increases. The ANOVA table shows the percentage
contribution of each parameter. From the ANOVA table
it is seen that parameter V, i.e. volume fraction is the
most significant parameter influencing the wear beha-
viour at the confidence level of 99% while parameters L
(applied load) and S (sliding speed) are also significant
within the specific test range. Sliding time and the inter-
action of parameters have almost no influence on wear
property of the composite.
4.3. Confirmation Test
After the optimal level of testing parameters have been
found, it is necessary that verification tests are carried
out in order to evaluate the accuracy of the analysis and
to validate the experimental results. The estimated S/N
ratio η, using the optimal level of the testing parameters
can be calculated as:

1
ˆo
mim
i



 
 (2)
where,

m is the total mean S/N ratio,

i is the mean S/N
ratio at the optimal testing parameter level and o is the
number of main design process parameters that signifi-
cantly affect the wear behaviour of Al-SiC metal matrix
composite. Table 7 shows the comparison of the esti-
mated wear result with the actual wear using the optimal
condition. It may be observed that good agreement takes
place between the estimated and experimental results.
There is improvement of S/N ratio from initial to op timal
condition that yields nearly 17% decrease in wear from
the initial condition. This is a significant improvement.
4.4. Wear Mechanism
Microstructur e study of the wear tracks are carried out to
analyze the wear mechanism that the composites undergo
during tribological testing. Figure 4 shows wear tracks
of samples having three different volume fraction of re-
inforcement, Al-5%SiC, Al-7.5%SiC and Al-10%SiC.
From the SEM micrographs, it can be observed that the
worn surface mainly consists of longitudinal grooves and
partially irregular pits. The presence of grooves indicates
micro-cutting and micro-ploughing effect. Thus wear mech-
anism is found to be dominated by abrasive wear. Also
presence of pits and prows can be observed in the micro-
graphs, thus occurrence of adhesive wear is also visible.
So, from overall microstructure study it can be concluded
that mostly abrasive wear has taken place with some
traces of adhesive wear.
In the present investigation the effect of four process
parameters volume fraction, applied load, sliding speed
and time on the wear behaviour of Al-SiC particulate
composite is studied. Apart from these, other factors like
heat treatment, temperature change and particle size of rein-
forcement are assumed constant during this experimental
study. In future, studies related to effects of these other
factors on the wear behaviour of Al-SiC can be carried
out.
5. Conclusion
Wear behaviour of Al-SiCp metal matrix composite is
studied for varying reinforcement content, applied load,
sliding speed and time using Taguchi orthogonal array
design. It is observed that parameter V, i.e. volume frac-
tion of reinforcement is the most significant parameter
influencing the wear behaviour at the confidence level of
99% while parameters L (applied load) and S (sliding
speed) are also significant within the specific test range.
Sliding time and the interaction of parameters have almost
Table 6. ANOVA table for wear.
SourceDFSS MS F Contribution %
V 2 56.506 28.253 116.9# 41.50
L 2 28.126 14.063 58.19# 20.66
S 2 42.752 21.376 88.45# 31.40
T 2 0.658 0.329 1.36 00.48
V*L 4 1.070 0.267 1.11 00.79
V*S 4 3.221 0.805 3.33 2.37
L*S 4 2.376 0.594 2.46 1.75
Error 6 1.450 0.241 1.05
Total 26 136.1627 100
#Significant parameters (F 0.01, 2, 8 = 8.65)
Table 7. Results of confirmation test.
Optimal parameter
Initial parameter
Prediction Experimental
Level V2L2S2T2 V3L1S1T1 V3L1S1T1
Wear (µm)82.24 68.46
S/N ratio (dB)–38.302 –31.975 –36.709

S. GHOSH ET AL. 1093
(a)
(b)
(c)
Figure 4. SEM micrograph of worn surfaces of (a) Al-5%
SiC, (b) Al-7.5%SiC, and (c) Al-10%SiC.
no influence on wear property of the composite. From
the Taguchi analysis the optimal combination of process
parameter for minimum wear is found to be V3L1S1T1,
i.e., the highest level of vo lume fraction of reinforcement
along with the lowest levels of applied load, sliding
speed and sliding time. Wear depth is reduced by nearly
17% from initial to optimal process parameter condition.
From the present study it is revealed that a proper control
of process parameters can result in improved design of
the Al-SiC composite for tribological applications. From
the microstructure study of worn surfaces, it is observed
that mostly abrasive wear mechanism has occurred on
the wear tracks with some traces of adhesive wear me-
chanism.
REFERENCES
[1] H. Ahlatci, E. Candan and H. Cimenoglu, “Abrasive
Wear Behaviour and Mechanical Properties of Al-Si/SiC
Composites,” Wear, Vol. 257, No. 5-6, 2004, pp. 625-632.
doi:10.1016/j.wear.2004.03.006
[2] K. S. Al-Rubaie, H. Goldenstein and J. D. Biasoli de
Mello, “Three-Body Abrasion of Al-SiC Composites,”
Wear, Vol. 225-229, 1999, pp. 163-173.
doi:10.1016/S0043-1648(99)00009-5
[3] K. S. Al-Rubaie, H. N. Yoshimura and J. D. Biasoli de
Mello, “Two-Body Abrasive Wear of Al-SiC Compos-
ites,” Wear, Vol. 233-235, 1999, pp. 444-454.
doi:10.1016/S0043-1648(99)00185-4
[4] M. Bai, Q. Xue, X. Wang, Y. Wan and W. Liu, “Wear
Mechanism of SiC Whisker-Reinforced 2024 Aluminum
Alloy Matrix Composites in Oscillating Sliding Wear
Tests,” Wear, Vol. 185, No. 1-2, 1995, pp. 197-202.
doi:10.1016/0043-1648(95)06617-9
[5] M. Bai, Q. Xue and Q. Ge, “Wear of 2024 Al-Mo-SiC
Composites under Lubrication,” Wear, Vol. 195, No. 1-2,
1996, pp. 100-105. doi:10.1016/0043-1648(95)06808-2
[6] M. Bai and Q. Xue, “Investigation of Wear Mechanism of
SiC Particulate-Reinforced Al-20Si-3Cu-1Mg Aluminium
Matrix Composites under Dry Sliding and Water Lubri-
cation,” Tribology International, Vol. 30, No. 4, 1997, pp.
261-269. doi:10.1016/S0301-679X(96)00047-3
[7] H. Chen and A. T. Alpas, “Wear of Aluminium Matrix
Composites Reinforced with Nickel-Coated Carbon Fi-
bers,” Wear, Vol. 192, No. 1-2, 1996, pp. 186-198.
doi:10.1016/0043-1648(95)06795-7
[8] R. Chen, A. Iwabuchi, T. Shimizu, H. S. Shin and H.
Mifune, “The Sliding Wear Resistance Behavior of NiAI
and SiC Particles Reinforced Aluminum Alloy Matrix Com-
posites”, Wear, Vol. 213, No. 1-2, 1997, pp. 175-184.
doi:10.1016/S0043-1648(97)00123-3
[9] R. Chen, A. Iwabuchi and T. Shimizu, “The Effect of a
T6 Heat Treatment on the Fretting Wear of a SiC Parti-
cle-Reinforced A356 Aluminium Alloy Matrix Compo-
site”, Wear, Vol. 238, No. 2, 2000, pp. 110-119.
doi:10.1016/S0043-1648(99)00328-2
[10] S. K. Ghosh and P. Saha, “Crack and Wear Behaviour of

S. GHOSH ET AL.
1094
SiC Particulate Reinforced Aluminium Based Metal Ma-
trix Composite Fabricated by Direct Metal Laser Sinter-
ing Process,” Materials and Design, Vol. 32, No. 1, 2011,
pp. 139-145. doi:10.1016/j.matdes.2010.06.020
[11] A. M. Hassan, A. Alrashdan, M. T. Hayajneh and A. T.
Mayyas, “Wear Behaviour of Al-Mg-Cu-Based Compo-
sites Containing SiC Particles,” Tribology International,
Vol. 42, No. 8, 2009, pp. 1230-1238.
doi:10.1016/j.triboint.2009.04.030
[12] Y. Iwai, H. Yoneda and T. Honda, “Sliding Wear Beha-
viour of Sic Whisker-Reinforced Aluminium Composite,”
Wear, Vol. 181-183, No. 2, 1995, pp. 594-602.
[13] J. K. M. Kwok and S. C. Lim, “High-Speed Tribological
Properties of Some Al/SiCp Composites: I. Frictional and
Wear-Rate Characteristics”, Composites Science and Tech-
nology, Vol. 59, No. 1, 1999, pp. 55-63.
doi:10.1016/S0266-3538(98)00055-4
[14] G. Straffelini, M. Pellizzari and A. Molinari, “Influence
of Load and Temperature on the Dry Sliding Behavior of
Al-Based Metal-Matrix-Composites Against Friction Ma-
terial”, Wear, Vol. 256, No. 7-8, 2004, pp. 754-763.
doi:10.1016/S0043-1648(03)00529-5
[15] R. N. Rao and S. Das, “Effect of Matrix Alloy and Influ-
ence of SiC Particle on the Sliding Wear Characteristics
of Aluminium Alloy Composites,” Materials and Design,
Vol. 31, No. 3, 2010, pp. 1200-1207.
doi:10.1016/j.matdes.2009.09.032
[16] R. N. Rao and S. Das, “Effect of Sliding Distance on the
Wear and Friction Behaviour of as Cast and Heat-Treated
Al-SiCp Composites,” Materials and Design, Vol. 32, No.
5, 2011, pp. 3051-3058.
doi:10.1016/j.matdes.2011.01.033
[17] S. C. Sharma, B. M. Girish, R. Kamath and B. M. Satish,
“Effect of SiC Particle Reinforcement on the Unlubri-
cated Sliding Wear Behaviour of ZA-27 Alloy Compo-
sites”, Wear, Vol. 213, No. 1-2, 1997, pp. 33-40.
doi:10.1016/S0043-1648(97)00185-3
[18] B. S. Unlu, “Investigation of Tribological and Mechanical
Properties Al2O3-SiC Reinforced Al Composites Manu-
factured by Casting or P/M Method,” Materials and De-
sign, Vol. 29, No. 10, 2008, pp. 2002-2008.
doi:10.1016/j.matdes.2008.04.014
[19] B. Venkataraman and G. Sundararajan, “The Sliding
Wear Behaviour of Al-SiC Particulate Composite-I
Macro Behaviour,” Acta Materialia, Vol. 44, No. 2, 1996,
pp. 451-460. doi:10.1016/1359-6454(95)00217-0
[20] B. Venkataraman and G. Sundararajan, “The Sliding
Wear Behaviour of Al-SiC Particulate Composite II—The
Characterization of Subsurface Deformation and Correla-
tion with Wear Behavior,” Acta Materialia, Vol. 44, No.
2, 1996, pp. 461-473. doi:10.1016/1359-6454(95)00218-9
[21] Y. Yalcin and H. Akbulut, “Dry Wear Properties of
A356-SiC Particle Reinforced MMCs Produced by Two
Melting Routes,” Materials and Design, Vol. 27, No. 10,
2006, pp. 872-881. doi:10.1016/j.matdes.2005.03.007
[22] Z. Hasan, R. Pandey and D. Sehgal, “Wear Characte-
ristics in Al-SiC Particulate Composites and the Al-Si
Piston Alloy,” Journal of Minerals and Materials Char-
acterization and Engineering, Vol. 10, No. 14, 2011, pp.
1329-1335.
[23] M. Singla, L. Singh and V. Chawla, “Study of Wear
Properties of Al-SiC Composites,” Journal of Minerals
and Materials Characterization and Engineering, Vol. 8,
No. 10, 2009, pp. 813-821.
[24] G. Taguchi, “Introduction to Quality Engineering,” Asian
Productivity Organization, Tokyo, 1990.
[25] P. J. Ross, “Taguchi Technique for Quality Engineering,”
2nd Edition, McGraw Hill, New York, 1996.
[26] D. C. Montgomery, “D esign and Analysis of Experiments,”
John Wiley Sons, New York, 2001.
[27] R. A. Fisher, “Design of Experiments,” Oliver & Boyd,
Edinburgh, 1951.
[28] Minitab User Manual, “Making Data Analysis Easier,”
Minitab Inc., Pennsylvania State University, Pennsylva-
nia, 2001