Modern Economy, 2010, 1, 100-111
doi:10.4236/me.2010.12010 Published Online August 2010 (http://www. SciRP.org/journal/me)
Environmental Standards and Trade Volume
Nicholas Mangee*, Bruce Elmslie
University of New Hampshire, Department of Economics, Whittemore School of Business and Economics, Durham USA
Received June 10, 2010; revised July 20, 2010; accepted July 24, 2010
Abstract
This paper presents a theoretical and empirical analysis of the effects of environmental regulation on bilateral
trade volume. We use a gravity model of trade flows and find weak evidence that differences in regulation
are a source of comparative advantage. We also find evidence against the race-to-the-bottom hypothesis in
that increases in standards in both high and low standard countries increase bilateral trade volume. We use
1999 data on GDP, population, and environmental stringency for 39 countries.
Keywords: Environmental Standards, Trade Volume, Gravity Model, Comparative Advantage
1. Introduction
The link between environmental regulation and interna-
tional trade has warranted considerable attention by
economists, environmentalists and policy makers alike.
A substantial amount of theoretical and empirical re-
search has addressed the question of how environmental
stringency affects international trade. Conventional trade
theory suggests that country characteristics, such as land
and capital define comparative advantage. Environ-
mental quality, endowments, capacities and policies have
also been considered as determinants of each country’s
comparative advantage1.
This paper develops a model of comparative advan-
tage based on differences in environmental regulation
between countries. We then use a gravity model to test
the hypothesis that as the differences in environmental
regulation across countries increase, bilateral trade flows
also increase as predicted by the theory. Differences in
regulation are found to have a positive but weak effect
on bilateral trade volume.
This result could also be consistent with a race-to-the-
bottom in terms of environmental standards for low stan-
dard countries. Therefore, we develop a second test bet-
ween overall standards and trade volume. We find strong
evidence that increased standards by both countries pro-
motes bilateral trade volume. Thus, even if trade volume
is positively associated with differences in regulation, no
incentive appears to exist for low standard countries to
increase trade flows by lowering standards.
The existing literature has yet to demonstrate the ef-
fect of differentiated regulation on gross bilateral trade
flows across a large set of countries. Previous studies
have examined the effect that regulation has on the com-
position of trade regarding one country or a small set of
countries. Other studies have demonstrated regulation in
isolation, failing to examine the collective differences
between stringency as a source of comparative advantage.
Given the ubiquitous nature of comparative advantage in
the theoretical determination of trade patterns between
countries, it is surprising that many of the existing stud-
ies have eschewed this approach. This paper represents
the first study of the effects of environmental regulation
on trade volume that fully incorporates comparative ad-
vantages created by differentiated environmental regula-
tion between trading partners.
Within the last three decades, two popular positions
have emerged regarding the interaction of stringency and
international trade in environmentally intensive goods
rather than in overall trade volume. The first hypothesis
suggests that, as a country imposes higher environmental
regulations, the cost of production increases. This may
result in a decrease in exports of pollution intensive
goods and an increase in imports. Thus, higher strin-
gency may lead to a decrease in comparative advantage
in pollution intensive goods for a country [3,4]. Fur-
thermore, to maintain international competitiveness, a
country may purposefully set lax environmental stan-
dards. This potential global degradation in stringency is
consistent with the race-to-the-bottom hypothesis. These
theories hypothesize that in order to not lose the business
*We want to thank Robert Mohr, Michael Goldberg, Edinaldo Tebaldi,
Torsten Schmidt and Sinthy Kounlasa for useful discussion and assis-
tance.
1For analysis on the environment and comparative advantage see Pethig
[1] and Siebert [2].

N. MANGEE ET AL.
101
of pollution intensive goods whose production may be
shipped abroad, countries deliberately race-to-the-bot-
tom in environmental regulations. Similarly, these costs
incurred by increasing a firm’s total cost of production
coupled with the “pollution haven hypothesis” suggest
that pollution intensive production will migrate to re-
gions of lax regulations [5,6]. With low environmental
demand, developing countries may attempt to increase
their share of the global market by setting lax regulations
creating a comparative advantage in pollution intensive
goods [7]. The empirical performance of these hypothe-
ses has been poor [3,8,9]. Our results represent the first
clear empirical case that comparative advantage is not
associated with the development of pollution havens,
because higher standards of low standard countries in-
creases rather than decreases bilateral trade volume.
The contrasting hypothesis concerning the effect of
environmental stringency on foreign trade is the Porter
hypothesis [10,11]. This hypothesis predicts that an in-
crease in environmental regulation will stimulate ad-
vancements in environmentally friendly technology. This
tightening of standards will encourage firms to seek new
technology for the long run and possibly differentiate
their products by producing environmentally friendly
ones. Porter, however, makes no distinction between
high and low standard countries in terms of the relation-
ship between inventiveness and trade volume. However,
a loose interpretation of Porter is that countries should
not fear that increased regulation will necessarily de-
crease trade volume. It may even increase trade volume
as increased standards increase the incentive to innovate.
The test that we develop supports this loose interpreta-
tion of Porter.
2. Empirical Framework and Literature
Review
There is a vast literature on the relationship between sta-
ndards and international trade.2 Typically, the methodo-
logical approach for determining the effect of regulation
on trade involves a supply side model incorporating the
determinants of international trade. The conventional su-
pply side approach has followed a Heckscher-Ohlin-Sa-
muelson (HOS) theoretical framework and often the He-
ckscher-Ohlin-Vanek (HOV) model in empirical work.
The HOS model predicts that a country will export the
good that intensively uses its relatively abundant factor.
The environment is incorporated in HOS as a factor of
production predicting that a country with a greater envi-
ronmental capacity for pollutants is relatively better en-
dowed and will export pollution intensive goods. The
HOV model allows for a direct empirical test by predict-
ing the factor composition of trade for a country based
on factor abundance. This model has been widely applied
within the environmental trade literature as it allows for
trade to be decomposed by pollution intensity and indus-
try.
Tobey [9] uses a cross section HOV model to investi-
gate whether increased domestic regulation during the
1960’s and 1970’s affected trade patterns in pollution
intensive industries. Using 23 countries and 1975 data,
he regresses trade in pollution intensive commodities
across countries on resource endowment characteristics
such as land area, literate workers, and capital. The re-
sults suggest that there is no significant effect of in-
creased environmental stringency on net exports of pol-
lution intensive goods. Tobey [9] does not investigate the
role of regulatory differences specifically in explaining
trade flows between countries.
Ratnayake [3] takes an inter industry trade approach
consisting of 109 industries over a 13 year period (1980-
1993) in New Zealand. Following the HOS/HOV models,
New Zealand, as a developed capital abundant country is
expected to possess a comparative advantage in the pro-
duction of goods that intensively use capital in produc-
tion. Ratnayake uses a revealed comparative advantage
(RCA) index to determine if increased environmental
standards lead to a decrease in competitiveness in the
manufacturing sector. By comparing trade behavior of
New Zealand’s to four other country groups (world,
OECD, ASEAN, and DC’s) this study finds that, in spite
of high environmental stringency in New Zealand, it’s
exports in pollution intensive, or environmentally sensi-
tive goods was not decreased.
Unlike Ratnayake, a number of other studies have sho-
wn an increase in environmental standards to have a ne-
gative impact on the exports of pollution intensive indus-
tries. Wilson and colleagues [14] takes a developing
country perspective of stringency on trade by examining
the export behavior of 24 countries (6 OECD and 18
non-OECD) between 1994 and 1998 for 5 pollution in-
tensive industries. Utilizing the HOV model, this study
finds that more stringent environmental standards result
in lower exports of pollution intensive goods. Moreover,
this study suggests that increased environmental standa-
rds have a significantly greater impact on exports of de-
veloping countries than developed ones.
It is well known that the HOV equation is inconsistent
with trade data [15]. Given these weak general founda-
tions, it is not surprising that the results of HOV models
applied to the relationship between environmental stan-
dards and trade volume have been inconsistent at best.
Alternative approaches, therefore, need to be considered.
Given the empirical problems with the HOV model,
we utilize a gravity framework that incorporates differ-
entiated environmental regulation as a source of bilateral
trade volume. Such a model of international trade was
first developed by Tinbergen [16] to model trade volume
2For an overview of the literature and methodologies see Alpay [12]
and Van Beers and Van Den Bergh [13].

N. MANGEE ET AL.
102
between two countries in terms of their GDP’s and the
geographical distance between them.3 The gravity model,
after being log linearized, takes the following form:
ijijjiij DISTGDPGDPX






 3210 lnlnln
(1)
Where:
ln denotes the natural logarithm;
ij
X denotes gross bilateral trade flows between coun-
try i and j;
i
GDP , j GDP denote the GDP of country i and j re-
spectively;
ij
DIST denotes the distance between country i and j;
ij
 denotes the error term.
3210 ,,,




are parameters.
The expected relationship between these variables is
as follows: since the dependent variable is gross bilateral
trade volume, the signs of 1

and 2

are expected to
be positive since larger countries trade more with each
other. The parameter 3

is expected to be negative due
to transportation costs. However, the variable ij DIST
may represent any factor that impedes trade. Such factors
that have been considered include language, historical,
and cultural differences. These characteristics are ex-
pected to have a negative relationship with the volume of
trade between two countries. In addition, a dummy vari-
able controlling for a border effect is often included in
empirical work and is normally found to be significant
and positive for countries sharing a border. The gravity
model is used in the present study because it incorporates
gross bilateral trade volume between countries as the
dependant variable and it allows for a large number of
countries to be analyzed. Furthermore, in contrast to the
HOS/HOV performance, the gravity model performs
much better empirically. The gravity model has not yet
been utilized as a theoretical tool revealing comparative
advantage arising from differences in environmental reg-
ulation.
The empirical literature incorporating regulation into
the gravity model include Jug and Mirza [18]; Grether
and Melo [5]; Van Beers and Van Den Bergh [19]; and
Harris and colleagues [20]. Some studies applying the
gravity model decompose total trade into imports and
exports and further into (non) pollution intensive imports
and/or exports, in an attempt to capture the effect of in-
creased stringency on total trade volume. Jug and Mirza
[18]4 show that increases in environmental expenditures
result in a decrease in net exports. These results are con-
sistent with the pollution havens hypothesis and run
counter to our so-called “loose” interpretation of the
Porter hypothesis.
In testing the effects of increased stringency on bilat-
eral exports, Van Beers and Van Den Bergh [19] find a
strong negative relationship between total exports and
total imports of 21 OECD countries. Part of this study
gives evidence to support the pollution havens hypothe-
sis while the negative effect of standards on a developed
country’s imports presents a surprising result. This result
may suggest the presence of import barriers when regu-
lation is increased within a country. Another study that
decomposes bilateral trade volume into imports and ex-
ports is Grether and Melo [5]. They test total imports
against pollution intensive imports as well as non-res-
ource based (footloose) imports against resource based
(non-footloose) imports. This study finds support for the
pollution haven hypothesis for the footloose industries
due to the increase in imports for developed countries
when firms are allowed to migrate across countries.
The gravity studies have shown no robust conclusive
evidence of the effect of regulation on trade flows [18].
The lack of consensus within this literature may stem
from the lack of a comprehensive measure of the enviro-
nment or regulatory stringency. Measures of stringency
should incorporate various indicators of a country’s stan-
dards, enforcement and policy implementation. Most da-
ta sets used in previous studies are either not comprehen-
sive or they cover a small sample of countries. The pre-
sent study utilizes a recent 2001 comprehensive measure
of environmental stringency over a large sample of coun-
tries. In addition to the data problem, the literature to
date has been limited by the Solomon-like choice betw-
een theory and empirics. Researchers choosing the gen-
eral HOS-HOV approach are able to base their study on
a solid theoretical foundation, but must pay in terms of
weak empirical support. The well-known empirical shor-
tcomings of the HOV model with regard to the factor co-
ntent of trade in factors such as capital and various quali-
ties of labor also plague environmental endowment me-
asures. On the other hand, researchers choosing the grav-
ity approach are able to tap into a solid empirical frame-
work but must pay in terms of theoretical underpinnings.
As a result, this literature has developed on an ad hoc
basis.
We argue that such a choice is not necessary. We aug-
ment the work of Helpman and Krugman [21] and Hel-
pman [22] on the theoretical foundation of a gravity equ-
ation to make theoretical predictions regarding the effect
of differential standards on trade volume. In so doing, we
are able to develop an empirical gravity model with the
strong theoretical underpinnings of an HOS approach.
3. Theory
The relationship between environmental standards and
3For an overview of the gravity model see Head [17].
4For previous studies applying the gravity model to trade and the envi-
ronment see Jug and Mirza [18] and references therein.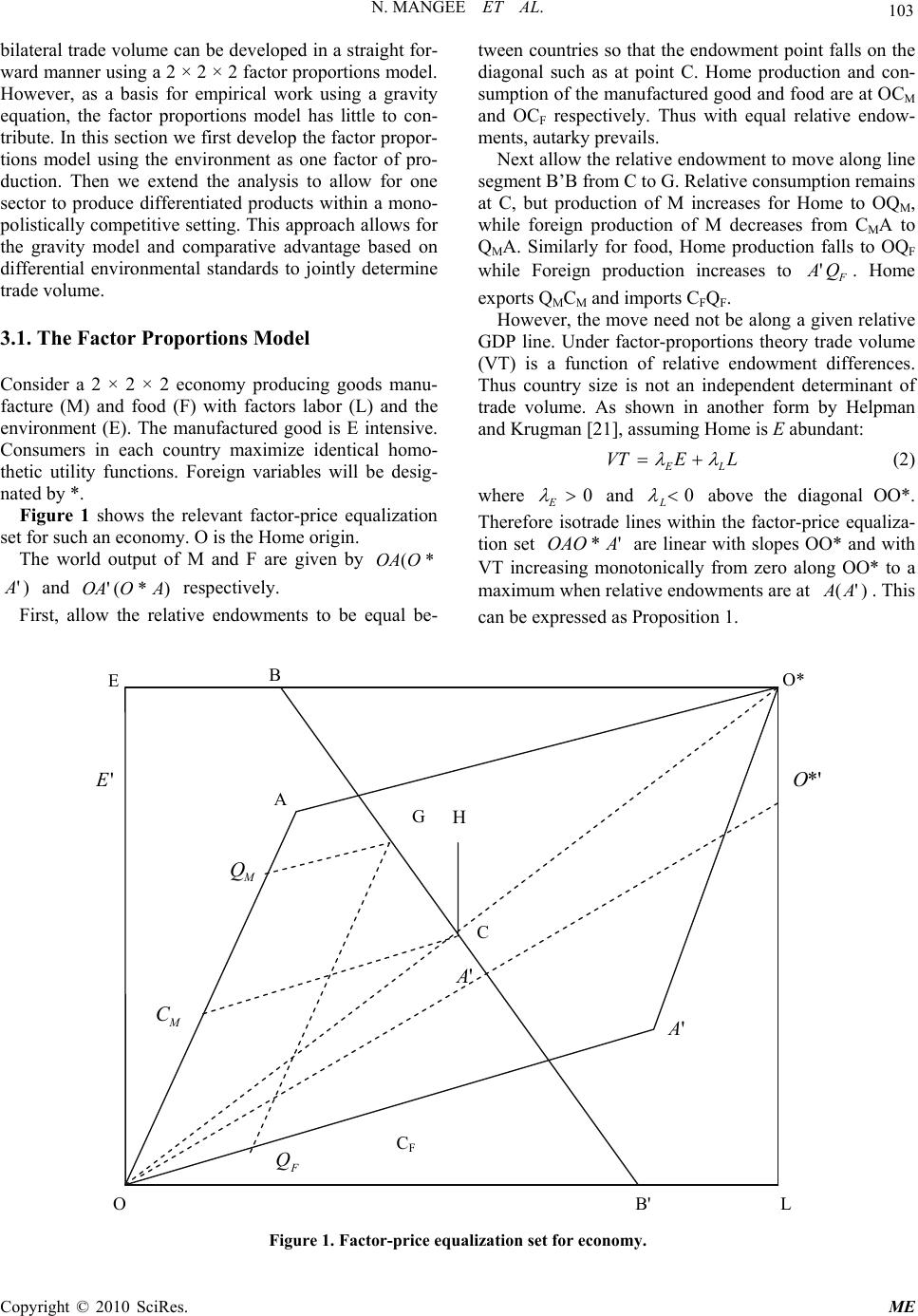

N. MANGEE ET AL.
103
bilateral trade volume can be developed in a straight for-
ward manner using a 2 × 2 × 2 factor proportions model.
However, as a basis for empirical work using a gravity
equation, the factor proportions model has little to con-
tribute. In this section we first develop the factor propor-
tions model using the environment as one factor of pro-
duction. Then we extend the analysis to allow for one
sector to produce differentiated products within a mono-
polistically competitive setting. This approach allows for
the gravity model and comparative advantage based on
differential environmental standards to jointly determine
trade volume.
3.1. The Factor Proportions Model
Consider a 2 × 2 × 2 economy producing goods manu-
facture (M) and food (F) with factors labor (L) and the
environment (E). The manufactured good is E intensive.
Consumers in each country maximize identical homo-
thetic utility functions. Foreign variables will be desig-
nated by *.
Figure 1 shows the relevant factor-price equalization
set for such an economy. O is the Home origin.
The world output of M and F are given by *( OOA
)'A and )*(' AOOA respectively.
First, allow the relative endowments to be equal be-
tween countries so that the endowment point falls on the
diagonal such as at point C. Home production and con-
sumption of the manufactured good and food are at OCM
and OCF respectively. Thus with equal relative endow-
ments, autarky prevails.
Next allow the relative endowment to move along line
segment B’B from C to G. Relative consumption remains
at C, but production of M increases for Home to OQM,
while foreign production of M decreases from CMA to
QMA. Similarly for food, Home production falls to OQF
while Foreign production increases to F QA'. Home
exports QMCM and imports CFQF.
However, the move need not be along a given relative
GDP line. Under factor-proportions theory trade volume
(VT) is a function of relative endowment differences.
Thus country size is not an independent determinant of
trade volume. As shown in another form by Helpman
and Krugman [21], assuming Home is E abundant:
LEVT LE




(2)
where 0
E

and 0

L

above the diagonal OO*.
Therefore isotrade lines within the factor-price equaliza-
tion set '* AOAO are linear with slopes OO* and with
VT increasing monotonically from zero along OO* to a
maximum when relative endowments are at )'(AA . This
can be expressed as Proposition 1.
H
E
'
E
*'O
O*
A
M
Q
M
C
C
F
F
Q
G
C
O L
'A
B
'A
B'
Figure 1. Factor-price equalization set for economy.

N. MANGEE ET AL.
104
Proposition 1
Trade volume within the factor-price equalization
set is maximized when relative endowment differenc-
es are maximized.
Within the factor-price equalization set the world en-
dowment of E and L are fixed at OE and OL respectively.
Utilizing such a framework the effect of differential en-
vironmental standards takes on a very particular inter-
pretation. Beginning with the endowment at C, a move
from C involves a switching of either or both factors bet-
ween countries. For example, a move from C to H imp-
lies that Foreign gives CH of its environmental endow-
ment to Home.
While such an exercise is useful to see how environ-
mental policy can influence comparative advantage, it is
not a realistic interpretation of operating standards. A
more realistic interpretation of an increase in environm-
ental standards is the decreasing of a country’s effective
environmental endowment while holding the other coun-
try’s endowment fixed. Using this interpretation neces-
sarily involves moving from one factor-price equaliza-
tion set to another. However, the analysis is surprisingly
simple.
Consider the original endowment point at C. This po-
int is on the VT = 0 isotrade line. If the foreign country
adopts environmental standards that decrease its endow-
ment, the world endowment of environment is decreased
from OE to 'OE. The new origin for Foreign is *'O.
The new diagonal is shown as OO*′. Now C is above the
new *'OO VT = 0 isotrade line. Thus, the change in
standards for Foreign away from those of Home incre-
ases trade volume. Given linear isotrade lines this is a
general result and can be expressed as Proposition 2.
Proposition 2
Within the factor proportions model, a change in
environmental regulation that increases relative end-
owment differences between countries increases trade
volume.
b) Monopolistic Competition
Proposition 2 generates an empirically testable link be-
tween relative regulatory environmental differences and
trade volume. However, it is inconsistent with an emp-
irical model that includes country size as an independent
determinant of trade volume. Therefore, we move to a
monopolistically competitive setting that links relative
standards and trade volume within a gravity framework.
Allow M to be monopolistically competitive where
each variety m is equally priced, equally produced and
earns zero profit. OA is now interpreted as many varie-
ties of M. If n is the number of firms in industry mM,
nM /.
Returning to Figure 1, allow the initial endowment to
be at G. Given that m = m*, Home is a net exporter of
manufacturing goods. The value of Home exports is For-
eign’s share of world GDP (s*) times the value of Home
production of nmpsMM
*, . Let the Home production
of varieties be given by mnM H. Given that trade
must be balanced in the absence of international borrow-
ing overall trade volume is:
HMM MpsnmpsVT *2*2 

(3)
Assume each country is of equal size in terms of world
GDP. The situation is depicted in Figure 2 where OC =
O*C and the endowment point is G.
Using the standard ^ notation to refer to relative chan-
ge 




s
ds
sge ˆ .,. log differentiation of 3 generates:
H
Ms
VT ˆ
*
ˆ
 (4)
Given that GDP + GDP* = world GDP which is con-
stant:
GDP
s
s
GDP
s   * **
ˆ (5)
Along H M
GDP
OZ ˆ ,
 so ,GDP
GDP
s
s
VT

 


or
^
1*
s
VT GDP
s




(6)
Using Equation (6), at 1
*
,
s
s
G implying that
0

VT . Moving from O to G along ssOZ*, im-
plying that 0

VT . Moving from G to Z, s > s*, thus
0

VT . Trade volume increases from O to G and de-
creases from G to Z leading to Proposition 3.
Proposition 3
Trade volume is maximized when countries are of
equal size (gravity equation).
Now, allow the endowment point to move from C
along 'BB to 'G so that holding relative size constant,
Home is getting more E abundant. Along 0*
ˆ '

s BB,
thus:
H
M
VT ˆ

 (7)
From C to 'G, OMH
ˆ, trade volume is increasing
resulting in Proposition 4.
Proposition 4
Within a factor-price equalization set, given coun-
try size is constant, trade volume increases as endow-
ments become less similar.
Taking Propositions 3 and 4 together trade volume is
maximized at 'G. Holding country size constant, as
environmental regulations effectively makes the relative

N. MANGEE ET AL.
105
E
L
'
B
O
'
A
C
G
Z
'G
A
B O*
Figure 2. Factor-price equalization set when countries are of equal size.
endowments less similar between countries, bilateral tra-
de volume increases. However, interpreting an increase
in regulation as decreasing the effective size of the envi-
ronmental endowment has a negative effect on GDP.
Given the non linearity of isotrade lines within the mo-
nopolistic competitive framework [21], no general
proposition can be stated beyond Proportion 4.
Our empirical framework is developed using Proposi-
tion 4. In that framework we regress bilateral trade vol-
ume against, size, distance and environmental standards.
The coefficient on environmental standards is interpreted
holding relative size constant, thus moving along 'BB
toward 'G is a change in relative environmental stan-
dards. From Propositions 2 and 4, the models based on
the factor proportions model of interindustry trade pre-
dict that increases in the differences in stringency of en-
vironmental standards between countries increases bilat-
eral trade volume.
4. The Environmental Index
The environmental index utilized in this study comes fr-
om the Global Competitiveness Report 2001-2002 [23].
The Environmental Regulatory Regime Index (ERRI) ca-
ptures the effects of several variables in constructing one
absolute ranking for 71 countries to measure environ-
mental stringency5. This number of countries far exceeds
other indices such as Walter and Ugelow [24] and Das-
gupta and colleaugues [25] which include 23 and 31
countries respectively. The absolute ranking in the ERRI
for each country ranges from –1.743 for Paraguay to
2.303 for Finland. The statistical methodology follows
bilateral regressions and collects all significant variables
to construct the ERRI.
For the purposes of the present paper, the variables
that directly influence the ERRI are presented in detail
below. For an explanation of the dependent variables and
other indirect explanatory variables within the index see
the Appendix. The ERRI is divided into two groups of
independent variables. The first group is comprised of
six categories: 1) stringency and environmental pollution
standards, 2) sophistication of regulatory structure, 3)
quality of the environmental information available, 4)
extent of subsidization of natural resources, 5) strictness
of government, and 6) quality of environmental institu-
tions [23].
The six indicators of environmental stringency listed
above give a very inclusive measure for regulation. In-
sight into a country’s regulatory regime can be collected
from these indicators because a variety of data collection
processes are utilized in their construction.6 The data
5For a list of all 71 countries see Etsy and Porter [23].
6Data sources include the Global Competitiveness Report (GCR), En-
vironmental Sensitivity Index (ESI), World Bank, and the World Eco-
nomic Forum [23,26].

N. MANGEE ET AL.
106
sources are mentioned below with a detailed description
of the stringency indicator. The variables mentioned be-
low represent the best available and comprise one of the
most extensive, inclusive and accurate measurements of
a country’s environmental stringency.
The stringency and pollution standards category uses
the Global Competitiveness Report (GCR) survey to me-
asure air, water, toxic waste, and chemical regulation for
a country. It is expected that this regulatory measure has
an inverse relationship with all three dependent variables
defined in the Appendix. Higher regulation results in
lower urban particulates, lower SO2 concentrations and
greater energy efficiency.
The sophistication of regulatory structure category
measures the characteristics of the regime. This concerns
the clarity in which the regulations are defined, the pro-
gressive nature of the regime, the structure of the regime
to promote competitiveness, and the relationship between
business and government. This category is also expected
to have an inverse relationship with the environmental
performance (dependent) variables.
The category regarding the quality of environmental
information available rests on data from the World Eco-
nomic Forum and the Environmental Sustainability In-
dex (ESI). This measures the extent to which environ-
mental and economic data are available for policy mak-
ing and regulatory enforcement. This study relies on four
proxy variables for this measure: 1) the extent to which
data is collected, 2) the extent to which sustainable de-
velopment data is available coupled with plans support-
ing environmental policy, 3) structure assessing envi-
ronmental decisions, and 4) the extent to which a country
has plans for environmental action. Again, there should
be a negative relationship between this category and the
dependent variables.
The extent of the subsidization of natural resources
category recovers data from the GCR survey. This capt-
ures the extent to which a country subsidizes energy and
natural resources. However, there is expected to be a po-
sitive relationship between the natural resource subsidies
and the three environmental performance variables.
The strictness of enforcement category measures two
factors within a country. The enforcement of environme-
ntal regulations are measured coupled with the extent to
which a country follows through with international ag-
reements regarding environmental policy. There should
be an inverse relationship between this measure and the
dependent variables as countries that have high environ-
mental enforcement should expect to have lower level of
pollution concentration and higher energy efficiency.
The final category involves the quality of environ-
mental institutions of a country. This measure captures
the effect that nongovernmental organizations (NGOs)
have on enforcing the environmental decisions and ac-
tions of the government. This may include environmental
organizations that further research to aid government
endeavors or even institutions that become substitutes for
the governmental sector. These NGOs may increase a
country’s ability to control for pollution by increasing
awareness and information on environmental issues.
Data is gathered from the ESI and this measure is ex-
pected to have a negative relationship with the dependant
variables.
5. Empirical Analysis and Data
The data on bilateral trade volume was recovered from
the World Bank for 1999. The aggregate of bilateral
trade volume from country i to country j was collected
by summing the total exports from i to j with the imports
from j to i. Since, duplicate pairings within the data set
would cause statistical problems the country that is alp-
habetically first was considered i and no duplicate pair-
ings were included. Table 1 expresses the pairings of
bilateral trade volume and ERRI Index value for each
country.
The data for the remaining variables was collected as
follows. The real 1999 GDP data is in billions of 2000
U.S. dollars while the per capita 1999 data was in 2000
U.S. dollars and found at the World Bank development
indicators. The distance measure is in kilometers.7 This
model is measured across 39 countries for the year
1999.8
The gravity model augmented to include differences in
environmental regulation is given in Equation (8). Equa-
tions (9) to (11) represent logical extensions of the model
that are explained in more detail below. Each country’s
ERRI number is represented as . The well-known
effect of sharing a border is represented with a dummy
variable ij

with a value of 1 representing countries i
and j sharing a border.
01 2
34
5
lnln ln
ln
ln
iji j
ij ij
ijij
X
GDP GDP
DIST
 


 
 

(8)
01 2
345
2
6
lnln ln
ln
iji j
ijijij
ij ij
XGDP GDP
DIST
 



 
 

(9)
7For distance and border data see www.macalester.edu/research/eco-
nomics/page/Haveman/Trade.Resources/Data/Gravity/dist.txt.
8The countries are Argentina, Australia, Austria, Bulgaria, Bolivia,
Canada, Chile, China, Colombia, Costa Rica, Denmark, Ecuador,
Egypt, Spain, Finland, France, United Kingdom, Greece, Hungary,
Indonesia, India, Ireland, Italy, Jordan, Japan, Korea, Mexico, Malaysia
N
etherlands, Norway, New Zealand, Peru, Philippines, Poland, Por
t
u-
gal, Sweden, Thailand, United States and Venezuela.

N. MANGEE ET AL.
107
Table 1. Pairings of bilateral trade volume and ERRI index
across countries.
Country Bilateral Trade Volume
(1999: Billions of US$)* ERRI (1999)
Argentina 23.98 –0.732
Australia 69.11 1.083
Austria 49.67 1.641
Bulgaria 3.30 –0.584
Bolivia 2.22 –0.743
Canada 370.96 1.297
Chile 17.77 0.177
China 220.04 –0.348
Colombia 11.88 –0.416
Costa Rica 7.63 –0.078
Denmark 52.82 1.384
Ecuador 3.29 –1.616
Egypt 1.51 –0.224
Finland 45.11 2.303
France 312.75 1.464
Greece 21.68 –0.619
Hungary 12.45 0.283
Indonesia 46.19 –0.758
India 34.47 –0.759
Ireland 82.67 0.546
Italy 228.14 0.498
Jordan 2.23 0.002
Japan 473.60 1.057
Korea 168.83 –0.121
Mexico 240.43 –0.602
Malaysia 85.43 –0.127
Netherlands 177.71 1.747
Norway 34.67 1.045
New Zealand 18.47 1.299
Peru 6.74 –0.722
Philippines 31.96 –1.014
Poland 24.08 0.005
Portugal 38.48 –0.028
Spain 158.27 0.437
Sweden 75.25 1.772
Thailand 54.68 –0.389
UK 345.73 1.185
United States 815.80 1.184
Venezuela 17.79 –1.079
*Bilateral trade volume based on sample of 39 countries
 
01 2
34 5
78
lnln ln
ln ln
min ,max ,
iji j
ijiji j
ijij ij
XGDP GDP
DIST
 
 

 
 


(10)


01 2
345
2
67
8
lnln ln
ln
min ,
max ,
iji j
ijijij
ij ij
ij ij
XGDP GDP
DIST
 


 

 
 


(11)
The results from Equations (8) through (11) are pre-
sented in Table 2. Equation (8) represents the log lin-
earized gravity model with a regulatory distance variable,
ij

 , included as the absolute value of environme-
ntal differences. The absolute value is used to express the
effective differences without regard to sign. Gravity the-
ory suggests that the larger the masses the greater the
force. The significance of both GDPi and GDPj is no
surprise as larger countries trade more with each other.
As we further expect, there is a significant positive bor-
der effect captured by ij

and a significant negative
distance effect captured by DISTij. These four measures
appear strongly significant across the four equations
above. The regulatory distance, ij
 , displays a
positive but weak effect on bilateral trade volume.
Equation (9) includes a quadratic regulatory distance
term, 2
ij

 , measuring any diminishing affect of
differences in standards. We would expect that this mea-
surement be negative. The natural logarithm is dropped
in this regression to avoid perfect multicollinearity
among the exogenous variables. The absolute distance is
still weakly positive but the point estimate is indicating a
much stronger effect. The quadratic measure expresses a
very weak negative relationship.
Equations (10) and (11) add the log of the minimum
and maximum regulations per country.9 The results from
Equations (8) and (9) can be interpreted as giving weak
support to the pollution havens hypothesis and the
race-to-the-bottom for low standard countries since a
decrease in standards for the low standard country in-
creases trade volume. To better control for this effect, we
add the high and low value of standards. A negative
value on θ7 , which is the coefficient for the low standard
country, would indicate that a decrease in standards of
the low standard country increases trade volume. Both
regressions produce positive and generally significant
effects of increased standards on trade volume for each
country. When the regulatory distance measure is drop-
ped, Equation (11) shows a positive and significant relat-
ionship between an increase in the standards of the
higher regulated country and trade volume. Both equa-
tions yield results refuting the race-to-the-bottom hy-
pothesis. No country has an incentive to lower environ-
mental standards in order to increase bilateral trade vol-
ume given that the coefficient for the low standards
country is positive and significant at the 10% level in
both equations.10
9The original ERRI values ran from –1.743 to 2.303. In order to take
the natural log of the max and min we added 2 to each value insuring
that all values are positive.
10We also ran regressions without taking the natural log of R max and
R min. The results were consistent though less significant.

N. MANGEE ET AL.
108
Table 2. Results from OLS regression of the natural log of bilateral trade volume between country i and j on explanatory var-
iables in column one.
(8) (9) (10) (11)
ln Xij ln Xij ln Xij ln Xij
ln GDPi 1.061***
(35.75)
1.059***
(35.57)
1.025***
(32.52)
1.024***
(32.46)
ln GDPj 0.941***
(31.41)
0.939***
(31.29)
0.909***
(28.99)
0.911***
(28.90)
δij 1.268***
(4.20)
1.268***
(4.20)
1.344***
(4.47)
1.346***
(4.48)
ln DISTij –0.897***
(–16.35)
–0.897***
(–16.29)
–0.848***
(–14.98)
–0.852***
(–14.98)
ij
 0.217
(1.15) 0.287 (1.11)
ln ij  0.038
(0.96) 0.034 (0.53)
2
ij
 –0.059
(–0.88) –0.041 (–0.062)
ln min


,
ij

0.269*
(1.68)
0.446
(1.57)
ln max


,
ij

0.235
(0.94)
–0.076
(–0.16)
*,**,*** denotes significance levels of 90%, 95%,
and 99% respectively. Test statistics are in parentheses
R2 = 0.785
# of obs. = 741
R2 = 0.785
# of obs. = 741
R2 = 0.788
# of obs. = 741
R2 = 0.788
# of obs. = 741
Where: ln denotes the natural logarithm; Xij denotes gross bilateral trade flows between country i and j; GDPi, GDPj denote the GDP of country i and j
respectively; DISTij denotes the distance between country i and j; ,
ij

 denote the environmental regulation index of country i and j respectively;
δij denotes a dummy variable equal to 1 if country i and j share a border, 0 otherwise; ij

denote the error terms.
To control for country size, per capita GDP is substi-
tuted into Equations (8), (9), (10), and (11) to generate
Equations (12) through (15). A useful economic inter-
pretation of the gravity model is that size is a measure of
the purchasing power of each country. Therefore an al-
ternative measure of size is income per capita. Countries
closer in terms of per capita income will be expected to
trade more.
01 2
34 5
ln lnln
ln ln
j
i
ij
ij
ijijij ij
GDP
GDP
XNN
DIST


 
 
 
 
 

 
(12)
01 2
34 5
2
6
ln lnln
ln
j
i
ij
ij
ijiji j
ij ij
GDP
GDP
XNN
DIST


 
 
 
 

 
 

(13)
 
01 2
34 5
78
ln lnln
ln ln
min ,max,
j
i
ij
ij
ijijij
ijij ij
GDP
GDP
XNN
DIST


 
 
 
 

 
 


(14)


01 2
34 5
2
67
8
ln lnln
ln
min ,
max ,
j
i
ij
ij
ijijij
ij ij
ij ij
GDP
GDP
XNN
DIST
+



 
 


 

 
 


(15)
The results from Equations 12 through 15 are prese-
nted in Table 3. Surprisingly, Equation (12) shows a
negative but insignificant relationship between regulat-
ory differences and trade volume. In controlling for di-
minishing returns by including the quadratic term, Equa-
tion (13) shows a positive marginally significant effect.
As found in the previous results, Equations (14) and (15)
present strong evidence against the race-to-the-bottom
hypothesis. Holding income per capita, distance, borders,
and regulatory differences constant, increased standards
of the low standard country increases trade volume. The
point estimates indicate that a 1% increase in the stan-
dards of the low standard country results in an increase
of trade volume of 0.8% and 0.9%. Using the ERRI these
results indicate that, for example, countries with index
values of 2 and 1.5 trade more with each other than
countries with index values with 1 and 0.5 even after
controlling for the effect of income per capita.

N. MANGEE ET AL.
109
Table 3. Results from OLS regression of the natural log of bilateral trade volume between country i and j on explanatory
variables in column one.
(12) (13) (14) (15)
ln Xij ln Xij ln Xij ln Xij
ln i
i
GDP
N



0.742***
(11.58)
0.737***
(11.49)
0.572***
(6.59)
0.601***
(6.64)
ln
j
j
GDP
N




0.65***
(9.88)
0.641***
(9.74)
0.483***
(5.52)
0.516***
(5.55)
δij 1.139**
(2.14)
1.151**
(2.17)
1.284**
(2.43)
1.29**
(2.45)
ln DISTij –0.677***
(–6.81)
–0.663***
(–6.65)
–0.626***
(–6.28)
–0.629***
(–6.33)
ij
 0.461
(1.38) 0.947** (2.01)
ln ij  –0.071
(–1.00) 0.057 (0.49)
2
ij
 –0.217*
(–1.84) –0.185 (–1.59)
ln min


,
ij

0.931***
(3.25)
1.38***
(2.74)
ln max


,
ij

0.109
(0.21)
–0.874
(–0.88)
*,**,*** denotes significance levels of 90%, 95%,
and 99% respectively. Test statistics are in parentheses
R2 = 0.332
# of obs. = 741
R2 = 0.335
# of obs. = 741
R2 = 0.338
# of obs. = 741
R2 = 0.347
# of obs. = 741
6. Conclusions
This paper makes four contributions. First, we develop a
theoretical model of the relationship between environm-
ental standards and bilateral trade volume within a factor
proportions framework. We further extend this model to
consider monopolistic competition that generates a grav-
ity equation. This allows us to produce an empirical
equation of the relationship between standards and trade
volume with strong theoretical foundations.
Second, we utilize a new more comprehensive index
of environmental stringency in our empirical analysis of
the stringency-trade volume relationship than has been
utilized in previous studies. This index represents a com-
prehensive measure of overall environmental stringency
by country and allows for a relatively large sample of co-
untries. We conduct our empirical analysis over 39 de-
veloped and less developed countries.
Third, we fully utilize the concept of comparative adv-
antage in a gravity equation framework to address the
question of the relationship between environmental stan-
dards and bilateral trade volume. We find that different-
ces in environmental standards have a weak but positive
effect on trade volume. At the very least, our results in-
dicate that differential standards between countries do
not hinder trade volume. A country that is considering a
unilateral move to increase standards will not appear to
pay in terms of decreased trade volume.
Finally, we develop a test of whether low standard co-
untries have an incentive to decrease standards in order
to increase trade volume. Controlling for environmental
differences, and measures of country size (GDP and
GDP per capita) we find that trade volume increases as
the standards of the low standard country increase. Cou-
ntries higher on the ERRI trade more with each other
than similarly differenced countries lower on the index.
This evidence refutes the race-to-the-bottom and pollu-
tion havens hypotheses of trade, because neither high nor
lower standard countries increase trade volume by re-
ducing standards. All of our evidence runs in the oppo-
site direction.
7. References
[1] R. Pethig, “Pollution, Welfare and Environmental Policy
in the Theory of Comparative Advantage,” Journal of
Environmental Economics and Management, Vol. 2, No.
3, 1976, pp. 160-169.
[2] H. Siebert, “Environmental Quality and Gains from
Trade,” Kyklos, Vol. 30, No. 4, 1977, pp. 657-673.
[3] R. Ratnayake, “Do Stringent Environmental Regulations
Reduce International Competitiveness? Evidence from an

N. MANGEE ET AL.
110
Inter-Industry Analysis,” International Journal of the
Economics of Business, Vol. 5, No. 1, 1998, pp. 77-96.
[4] R. Stewart, “Environmental Regulation and International
Competitiveness,” Yale Law Journal, Vol. 102, No. 8,
1993, pp. 2039-2106.
[5] J. M. Grether and J. D. Melo, “Globalization and Dirty
Industries: Do Pollution Havens Matter?” Center for
Economic Policy Research, No. 3932, 2003.
[6] A. Levinson and S. Taylor, (2004). “Unmasking the Pol-
lution Haven Effect,” NBER Working Paper Series, No.
10629, 2004, pp. 1-32.
[7] J. A. Frankel and A. K. Rose, “Is Trade Good or Bad for
the Environment? Sorting Out the Causality,” Review of
Economics and Statistics, Vol. 87, No. 1, February 2005,
pp. 85-91.
[8] A. Levinson, “Environmental Regulations and Manufac-
tures’ Location Choice: Evidence from the Census of
Manufactures,” Journal of Public Economics, Vol. 62,
No. 1, 1996, pp. 5-29.
[9] J. Tobey, “The Effects of Domestic Environmental Poli-
cies and Patterns of World Trade: An Empirical Test,”
Kyklos, Vol. 43, No. 2, 1990, pp. 191-209.
[10] M. E. Porter, “America’s Green Strategy,” Scientific
American, Vol. 264, No. 4, April 1991, p. 168.
[11] M. Porter and C.V.D. Linde, “Towards a New Concep-
tion of the Environment-Competitiveness Relationship,”
Journal of Economic Perspectives, Vol. 9, No. 4, 1995,
pp. 97-118.
[12] S. Alpay, “What Do We Know About the Interactions
between Trade and the Environment? A Survey of the
Literature,” Bilkent University Working Paper, No. 991,
1999.
[13] C. van Beers and J.C.J.M. van den Bergh, “An Overview
of Methodological Approaches in the Analysis of trade
and the Environment”, Journal of World Trade, Vol. 30,
No. 1, 1996, pp. 143-167.
[14] J. Wilson, T. Otsuki and M. Sewadeh, “Dirty Exports and
Environmental Regulation: Do Standards matter to
Trade?” Developmental Research Group, the World Bank,
2002.
[15] D. Trefler, “The Case of the Missing Trade and Other
Mysteries,” The American Economic Review, Vol. 85, No.
5, 1995, pp. 1029-1046.
[16] J. Tinbergen, “Shaping the World Economy: Suggestions
for an International Economic Policy,” Twentieth Cen-
tury Fund, New York, 1962.
[17] K. Head, “Gravity for Beginners,” 2003. http://econo-
mics.ca./keith/gravity.pdf
[18] J. Jug and D. Mirza, “Environmental Regulations in
Gravity Equations: Evidence from Europe,” The World
Economy, Vol. 28, No. 11, 2005, pp. 1591-1615.
[19] C. van Beers and J. C. J. M. van den Bergh, “An Empiri-
cal Multi-Country Analysis of the Impact of Environ-
mental Regulations on Foreign Trade Flows,” Kyklos,
Vol. 50, No. 1, 1997, pp. 29-46.
[20] M. N. Harris, L. Konya and L. Matyas, “Modeling the
Impact of Environmental Regulations on Bilateral Trade
Flows: OECD, 1990-1996,” The World Economy, Vol. 25,
No. 3, 2002, pp. 387-405.
[21] E. Helpman and P. Krugman, “Market Structure and For-
eign Trade: Increasing Returns, Imperfect Competition,
and the International Economy,” MIT Press, Cambridge,
1985.
[22] E. Helpman, “Imperfect Competition and International
Trade: Evidence from Fourteen Industrial Countries,” Jo-
urnal of the Japanese and International Economies, Vol.
1, No. 1, 1987, pp. 62-81.
[23] D. Esty and M. Porter, “Ranking National Environmental
Regulation and Performance: A Leading Indicator or Fu-
ture Competitiveness?” The Global Competitiveness Re-
port 2001-2002, Oxford University Press, New York,
2001.
[24] I. Walter and J. Ugelow, “Environmental policies in de-
veloping Countries,” Ambio, Vol. 8, No. 23, pp. 102-109.
[25] S. Dasgupta, A. Mody, S. Roy and D.Wheeler, “Envi-
ronmental Regulation and Development: A Cross Coun-
try Empirical Analysis,” Oxford Development Studies,
Vol. 29, 2001, pp. 173-187.
[26] World Bank,“Trade Database and Developmental Indi-
cators,”1999. URL:http://web.worldbank.org.

N. MANGEE ET AL.
111
Appendix
There are three dependent variables used in the ERRI.
They are 1) the level of urban particulate matter, 2) av-
erage SO2 concentration which is normalized by urban
population, 3) energy efficiency. Particulate matter is
collected from the World Bank and the World Health
organization (WHO). This measures the concentration of
air-born dust and is therefore a measure of air quality. A
higher particulate concentration corresponds to a higher
pollution level. Similarly, the SO2 concentration also
measures the quality of air and serves as a gauge for lev-
els of pollution. The energy efficiency measure utilizes
U.S. Department of Energy data and captures the aggre-
gate amount of energy consumption per unit of GDP for
each country. The higher the level of energy efficiency
the lower the amount of energy consumed per unit of
GDP and therefore the more efficient a country’s energy
consumption.
The ERRI is divided into two groups of independent
variables. The first group is comprised of six categories:
1) stringency and environmental pollution standards, 2)
sophistication of regulatory structure, 3) quality of the
environmental information available, 4) extent of sub-
sidization of natural resources, 5) strictness of govern-
ment, and 6) quality of environmental institutions [23].
The second group of independent variables is concerned
with a country’s economic and legal context. This group
can be further divided into two categories: 1) administra-
tive infrastructure which includes but is not limited to
measures of civil/political rights, private property protec-
tion, corruption, and judiciary independence and 2) a
country’s technical capacity measuring scientific and
technological advancement. A number of proxies are
used in this category such as the number of scientists and
engineers, intellectual property protection, strength of
scientific community, government commitment to tech-
nological research and advancements, and the adoption
of foreign technologies.