Theoretical Economics Letters, 2012, 2, 391-394
http://dx.doi.org/10.4236/tel.[phone] Published Online October 2012 (http://www.SciRP.org/journal/tel)
International Outsourcing and Long-Run Growth in a
Variety Expansion Model
Ken-ichi Hashimoto
Graduate School of Economics, Kobe University, Kobe, Japan
Received May 31, 2012; revised June 26, 2012; accepted July 23, 2012
ABSTRACT
We develop a North-South trade model including the opportunity for outsourcing in a variety expansion framework and
derive the effect of an increase in outsourcing on long-run growth. We find that the effect of increased outsourcing on
the growth rate of product variety is contingent on the labor size of the Northern and Southern economy. In particular, if
the relative labor size of South to North is smaller, outsourcing the production of intermediate goods to Southern
economy can have negative effects on economic growth.
Keywords: Outsourcing; Long-Run Growth; North-South Trade; Variety Expansion Model
1. Introduction
Many developed countries have opened up to trade and
are increasingly outsourcing production. Firms shift the
production of some components abroad and assemble the
components into final goods at home. According to em-
pirical work by Feenstra and Hanson [1] and Crinò [2],
the share of international outsourcing by US firms was
11.61% in 1990 and 18.1% in 2002. According to sur-
veys, as reported by Ito et al. [3], 21% of manufacturing
industries in Japan use offshoring. Since outsourcing in-
fluences the structure of labor markets, the effects are an
important contemporary economic issue in offshoring
economy.
In their pioneering work, while Glass and Saggi [4]
develop a North-South trade model including the oppor-
tunity for outsourcing, they only employ a quality ladder
approach1. They demonstrate that increased outsourcing
always encourages long-run growth. In this paper, en-
dogenous technical progress drives productivity growth
in the form of variety expansion. A study closest to ours
is Naghavi and Ottaviano [7], who consider the endoge-
nous determinants of offshoring in a variety expansion
model. They compare the growth rate of offshoring eco-
nomy with that of no offshoring economy. Turning to our
model, we focus on the property of offshoring economy
only and examine the effect of increased international
outsourcing on long-run growth in offshoring economy.
Thus, this paper adopts the exogenous opportunity of
international outsourcing, where firms cannot offshore
more than a certain share in the same way as Glass and
Saggi [4] and Rodrigues-Clare [8], and reexamines their
results using a variety expansion framework of Grossman
and Helpman [9] in which Northern country engages in
innovative R & D and Southern country engages in imi-
tative R & D2.
Our contribution to the literature is to present a North-
South trade model incorporating international outsourc-
ing opportunities and show how it can be used to shed
light on the relationship between international outsourc-
ing and long-run growth. We show that the effect of in-
creased outsourcing on long-run growth depends upon
the structure of North and South; i.e., population size, the
productivity of research and development (R & D), etc.
Our main finding is that the increased outsourcing of
production in the North proves to encourage (discourage)
long-run growth when the population size of the South is
larger (smaller).
2. The Model
2.1. Households
Assume two countries, North (N) and South (S), with LN
and LS being the respective number of consumers in each
country. The utility function is:
 

1/
00
eln dd
01
n
t
iiii
UXttXtxj,j








，
where

is the rate of time preference, xi(j) is the de-
1For a static analysis of the effect of increased outsourcing, see Feen-
stra and Hanson [5] and Arndt [6].
2For a different approach to long-run growth, Rodrigues-Clare [8]
analyses the increased ofshoring in a process innovation framework o
f
Eaton and Kortum [10].
C
opyright © 2012 SciRes. TEL

K. HASHIMOTO 392
mand of household i ( N, S) for variety j of the mass
varieties n = nN + nS. Given instantaneous utility, the de-
mand function of xi(j) is given by



iji
x
jEp  j
where




11
0d
n
jpjpj j
 

 
,
Ei is consumption expenditure in country i, and p(j) is the
price of x(j). The aggregate demand function of x(j) is
then:
 
jE
xj pj
  (1)
where
 
N
NS S
x
jxjLxjL and
N
NS
EEL EL
S
.
Using these equations, it is easy to establish that util-
ity-maximizing total expenditure obeys the familiar Euler
equation ii
EE r


, where r is the rate of interest
determined in the global financial market. The Euler equa-
tion for country i can then be easily arranged into:
EE r



 (2)
2.2. Firm Sectors
Local monopolists holding patents for the goods produce
differentiated products. To obtain patents, a firm must
first succeed in R & D. Economies where product innova-
tion takes place are in the North and their imitation takes
place in the South. First, consider the production of
North. The production in the R & D sector takes the fol-
lowing form:
NN
naRn
 (3)
where aN is the productivity of innovative R & D, RN is the
number of R & D workers and the presence of n captures
knowledge spillover in innovative R & D. We assume the
random imitation of Northern products. More specifically,
a given Northern product is assumed to be copied with an
instantaneous probability of SN
hnn during a time
interval dt, given that the range of Southern goods in-
creases by S during dt. Let VN denote the expected
present value of profits earned by Northern monopoly
firms that succeed in innovative R & D.
n

NNN
rVV hV 
 (4)
Now define N nn

 as the share of Northern goods
in all variety goods. Using this definition, we can rewrite
the Poisson rate of imitation h as
1
S
S
n
hn



 .
We assume free entry in the R & D sector. Therefore,
given the R & D technology, the following condition
holds:
NN N
Van w (5)
where
N
w is the wage rate in the North.
Final output x(j) is produced with two intermediate
goods
N
z and S. The intermediate good zi is pro-
duced in country i = N, S. One worker is required to pro-
duce one unit of intermediate goo
z
ds


,
N
S
z. More
specifically, if a firm produces x(j) units of final output
goods, its marginal cost (MC) becomes:
z




1
N
S
M
Cjw w

 
where S denotes the wage rate in the South, and w

is
the share of intermediate goods from the South and the
remaining 1


is the share of intermediate goods from
the North. Then the total cost (TC) of x(j) is given by:




1NS TCjwwx j    


.
Hence, unit cost is the same for all differentiated prod-
ucts in the North. Given the price elasticity of demand
1/(1 )


, the representative Northern producer sets the
price:


11,

0,
j
NNS
ppw wjn 

 

N
(6)
and earns profit:


1
NE
  N
S
(7)
Turn now to the South. The production function of the
imitative R & D sector takes the following form:
SSS
naRn

 (8)
where aS is the productivity of imitative R & D, RS is the
number of R & D workers and the presence of nS captures
knowledge spillover in imitative R & D. Let VS denote
the expected present value of profits earned by Southern
monopoly firms that succeed in imitative R & D, as de-
fined by:
π
SS
rV VS

 (9)
We assume free entry in the R & D sector. Therefore,
given the R & D technology, the following condition
holds.
SSS S
Van w (10)
Final output firms that succeed in imitative R & D can
produce goods with labor inputs with a marginal cost of
S. The Southern firms charge the monopoly price. We
assume wide gap equilibrium where the Southern mo-
nopoly price does not exceed the marginal cost of pro-
duction in the North. We then have:
w

1,0,

j
SS
ppwj n

 S (11)
and the profits of Southern firms are:


1
SE
  S (12)

K. HASHIMOTO 393
2.3. Labor Market Conditions
In the North, there are two sources of labor demand: in-
novative R & D and intermediate goods. In the R & D sec-
tor, /
N
N
Rnna workers are employed. In the manu-
facturing of intermediate goods, labor demand is
N
N
nz.
Using Shepard’s lemma, we obtain
N
NN
wzTC .

11
N
NN
N
n
L
an
 
n
x
(13)
Conversely, in the South there are three sources of la-
bor demand: imitative R & D, the manufacture of South-
ern production goods, and intermediate goods exported to
the North. In the imitative R & D sector, SNNS Rnna
workers are employed. Labor demand is SS
for
Southern manufacturing production and
nx
N
S for the
intermediate production exported to the North. Using
Shepard’s lemma, we obtain
nz
SN
S wzTC .
1S
SSS
SS
n
Lnx
an
 

NN
nx (14)
2.4. Steady State Equilibrium and the Effect of
Increased Outsourcing
We now consider the market equilibrium conditions. In
the steady state, SS
nnn ng
 is satisfied. We then
have following equations (see the Appendix for their de-
rivation):

1
111
NNN
g
g
La

 

 a
(15)
1
111
SSS N
g
gg
Laa a
 



 


(16)
where . From (15) and (16), we can ex-
plicitly solve for the growth rate as follows.

/
SN
ww  
   
11 1
1
11
S
SN
S
S
a
gaa
L
aN
L





 

 






(17)
It can be easily confirmed that the long-run growth
rate depends on the population size of the North-South
countries, the parameter for the share of production out-
sourced, and the remaining parameters. We assume the
following parameter conditions for positive rates of
growth (g > 0).
11
,
111 1
N
SN
SS
a
LL
aa





 
  
Now consider the effect of increased outsourcing on
long-run economic growth. An increase in internationally
outsourcing is interpreted as an increase in the ratio of
foreign production of outsourced intermediate goods to
domestically produced intermediate goods. This shift in
production from home to foreign arises with changes in
the economic environment, e.g. changes in local contents
requirement or quotas on the usage of foreign inputs, and
is described by an increase in

3. The purpose of this
paper is not to discuss the desirable level of outsourcing
share but to show the relationship between the proportion
of outsourcing and the rate of economic growth. From
(17), the impact on long-run growth is:
11
11
N
SN
SS
a dg LL
daa




 





where
  


2
22
11
0
1
11() 1
S
N
SN
a
a
aa





 
Then we have obtain the following results:
d0
d
g

 for 11
11
N
SN
SS
a
LL
aa







d0
d
g


for 11
11
N
SN
SS
a
LL
aa







This is formally stated in the following proposition.
Proposition 1: Increased outsourcing in Northern coun-
try to Southern country (  ) raises (resp. lowers) the growth
rate of product variety if the labor in Southern country is
larger (resp. smaller): LS > (resp. <)


1
S
a

  +


1
NS
aa






Intuitively, the effect is as follows. An increase in
outsourcing suggests more of the labor force in the North
is devoted to the innovative R & D sector, and this in-
creases economic growth. In contrast, outsourcing has a
negative effect on economic growth through a decrease
in the labor employed in imitative R & D sector in the
South. Thus, increased outsourcing can have a negative
effect on long-run growth depending on the labor en-
dowment in the Northern-Southern country. If the rela-
tive labor size of South to North is smaller, the negative
effect overweighs. Fi gure 1 depicts the graphical result.
LN.
3. Conclusion
This paper constructs a North-South trade model with
outsourcing opportunity in a variety expansion frame-
work and examines the effect of increased outsourcing
on the economic growth. In the literature of quality lad-
der based growth model, Glass and Saggi [4] find that the
3Weakening local contents requirements or relaxing import quotas on
intermediate goods are considered for a policy to have positive effect
on outsourcing opportunities. This approach for modeling outsourcing
opportunities is followed by Glass and Saggi [4].

K. HASHIMOTO 394
[2] R. Crinò, “Offshoring, Multinationals and Labour Market:
A Review of the Empirical Literature,” Journal of Eco-
nomic Surveys, Vol. 23, No. 2, 2009, pp. 197-249.
doi:10.1111/j.1467-6419.2008.00561.x
0dgd  

1as

 
0dg d


0dg d


[3] B. Ito, E. Tomiura and R. Wakasugi, “Dissecting Off-
shore Outsourcing and R & D: A Survey of Japanese Ma-
nufacturing Firms,” Discussion Paper 07-E-060, Research
Institute of Economy, Trade, and Industry, 2007.
[4] A. J. Glass and K. Saggi, “Innovation and Wage Effects
of International Outsourcing,” European Economic Re-
view, Vol. 45, No. 1, 2001, pp. 67-86.
doi:10.1016/S0014-2921(99)00011-2
[5] R. C. Feenstra and G. H. Hanson, “Foreign Investment,
Outsourcing and Relative Wages,” In: R. C. Feenstra, G.
M. Grossman and D. A. Irwin, Eds., The Political Econ-
omy of Trade Policy: Papers in Honor of Jagdish Bhag-
wait, MIT Press, Cambridge, 1996, pp. 89-127.
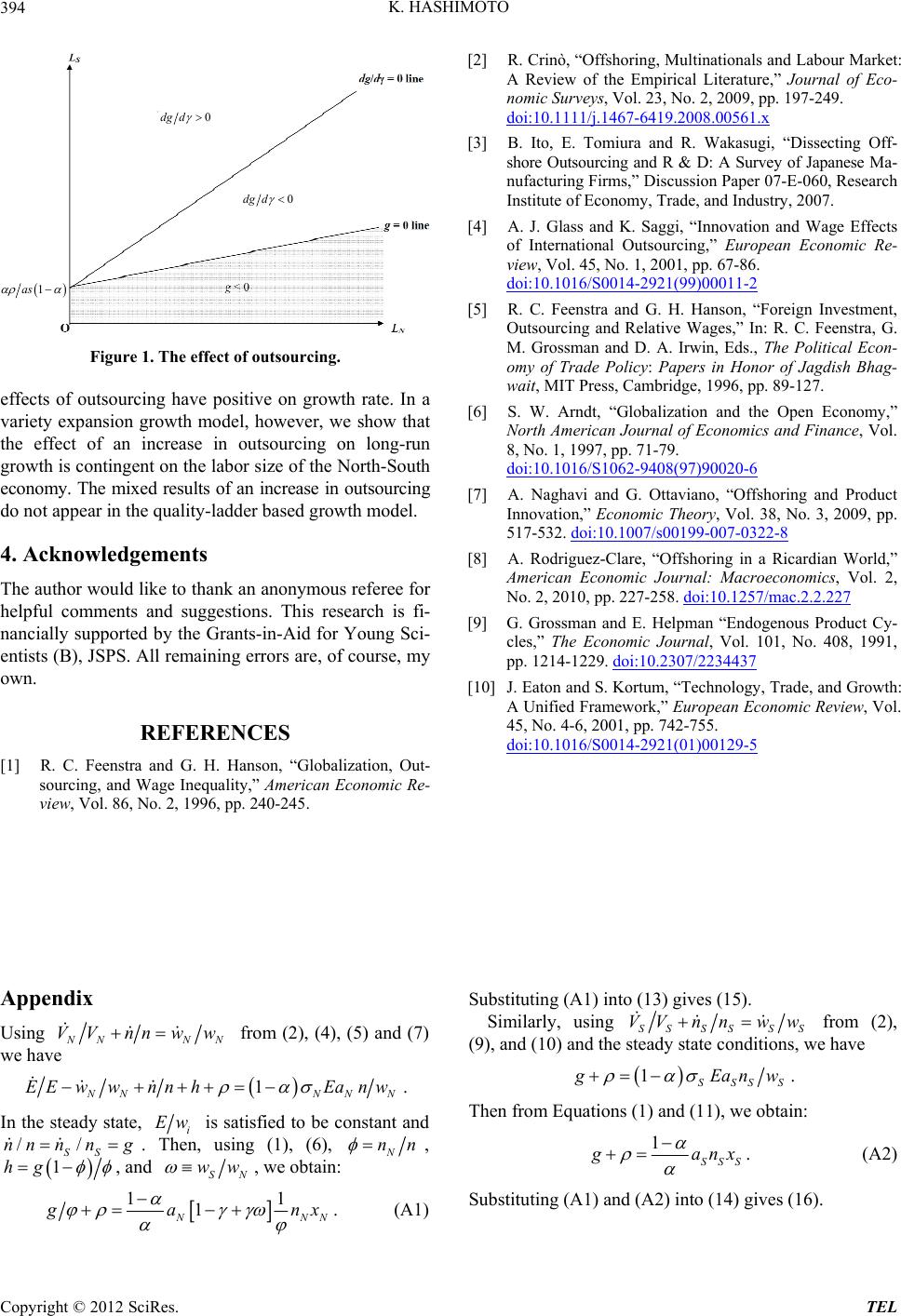
Figure 1. The effect of outsourcing.
ffects of outsourcing have positive on growth rate.
4. Acknowledgements
k an anonymous referee for
REFERENCES
[1] R. C. Feenstrlobalization, Out-
eIn a [6] S. W. Arndt, “Globalization and the Open Economy,”
North American Journal of Economics and Finance, Vol.
8, No. 1, 1997, pp. 71-79.
doi:10.1016/S1062-9408(97)90020-6
variety expansion growth model, however, we show that
the effect of an increase in outsourcing on long-run
growth is contingent on the labor size of the North-South
economy. The mixed results of an increase in outsourcing
do not appear in the quality-ladder based growth model. [7] A. Naghavi and G. Ottaviano, “Offshoring and Product
Innovation,” Economic Theory, Vol. 38, No. 3, 2009, pp.
517-532. doi:10.1007/s00199-007-0322-8
[8] A. Rodriguez-Clare, “Offshoring in a Ricardian World,”
American Economic Journal: Macroeconomics, Vol. 2,
No. 2, 2010, pp. 227-258. doi:10.1257/mac.2.2.227
The author would like to than
helpful comments and suggestions. This research is fi-
nancially supported by the Grants-in-Aid for Young Sci-
entists (B), JSPS. All remaining errors are, of course, my
own.
[9] G. Grossman and E. Helpman “Endogenous Product Cy-
cles,” The Economic Journal, Vol. 101, No. 408, 1991,
pp. 1214-1229. doi:10.2307/2234437
[10] J. Eaton and S. Kortum, “Technology, Trade, and Growth:
A Unified Framework,” European Economic Review, Vol.
45, No. 4-6, 2001, pp. 742-755.
doi:10.1016/S0014-2921(01)00129-5
a and G. H. Hanson, “G
sourcing, and Wage Inequality,” American Economic Re-
view, Vol. 86, No. 2, 1996, pp. 240-245.
Appendix
Using
N
NNN
nnww
 from (2), (4), (5) and (7)
e
VV

we hav

1
N
NNNN
wwnnhEanw  
 .
In the steady state,
EE

i
Ew is satisfied to be constant
en
and
//
SS
nn nng
 . Th, using (1), (6), N
nn


,

1hg

  , and SN ww

, we obtain:

11
1
N
NN
an  

  (A1)
Substituting (A1) into (13) gives (15).
x
 .
SS SS
VV nnw

Similarly, using S S
w from (2),
(9tate conditions, w), and (10) and the steady se have


1SSSS
g
Ea nw   .
Then from Equations (1) and (11), we obtain:
1
SSS
g
anx



 .
g

(A2)
Substituting (A1) and (A2) into (14) gives (16).