 Theoretical Economics Letters, 2012, 2, 369-372 http://dx.doi.org/10.4236/tel.2012.24068 Published Online October 2012 (http://www.SciRP.org/journal/tel) Revenue Sharing in Hierarchica l Organiza tions: A New Interpretation of the Generalized Banzhaf Value Roland Pongou1, Bertrand Tchantcho2,3, Narcisse Tedjeugang2 1Department of Economics, University of Ottawa, Ottawa, Canada 2École Normale Supérieure, Yaoundé, Cameroon 3Université de Cergy Pontoise, Cergy-Pontoise, France Email: rpongou@uottawa.ca, btchantcho@yahoo.fr, tedjeugang@yahoo.fr Received February 24, 2012; revised March 23, 2012; accepted April 19, 2012 ABSTRACT This paper examines the distribution of earnings in a new model of hierarchical multi-task organizations. Each such organization is defined by a finite set of workers and tasks together with a production function that maps each allocation of workers to the tasks into an aggregate output. Tasks are ordered in degree of importance so that aggregate output increases when a worker climbs up the organization ladder. We show that the generalized Banzhaf value proposed by Freixas [1] can be used as a theory of revenue sharing in such organizations, and provide a new interpretation and for- mulation of this sharing ru le, proving th at a worker’s pay is proportional to the difference between his marginal produc- tivity at the top level and at the bottom level of the hierarchy summed over all the possible configurations of the or- ganization. This new formulation also facilitates computation. Keywords: Hierarchical Organizations; Earnings; Marginal Productiv ity; Generalized Banzhaf Value 1. Introduction This paper studies the distribution of earnings in a new model of hierarchical organizations introduced in Pongou et al. [2]. Each such organization is defined by a finite set of workers and tasks, and a production function that maps each allocation of workers to the tasks into an aggregate output. Tasks are ordered in degree of im- portance so that aggregate output increases when a worker climbs up the organization ladder. Assuming that the organization is competitive, output converts directly into revenue. We adapt the generalized Banzhaf value proposed by Freixas [1] as a theory of revenue sharing in such organi- zations. This notio n is an ex tension of the concep t known as the Banzhaf-Coleman value. It was independently pro- posed by Banzhaf [3] and Coleman [4] as a measure of the ability of a voter to affect the outcome of a voting game. An axiomatic study of this value was conducted by Dubey and Shapley [5] and Laruelle and Valenciano [6]. Important applications of the Banzhaf value to corporations and corporate governance can be found in Leech [7,8]. Feltkamp [9] generalizes the axiomatic approach to any transferable utility cooperative game. In the context of the firm, a cooperative game can be viewed as a hierarchical organization that has only two levels. In an attempt to generalize this notion, Freixas and Zwick er [10] introduce the concept of simple games. A ,jk ,jk simple game can be interpreted as a hierarchical organization that has levels of hierarchy, and is equipped with a real-valued production function whose range has cardinality . In our model of a hierarchical organization, is not fixed, but is endogenously determined by the production function. Freixas [1] ge- neralizes the relative Banzhaf value to this class of games. Diffo Lambo and Moulen [11] and Freixas et al. [12] study this value in relation to other theories within the class of (2, 2) simple games, and Tchantcho et al. [13] and Pongou et al. [14] extend this an alysis to the class of (3, 2) simple games. j k k A new formulation and interpretation of the genera- lized Banzhaf value is provided. It proves that in a hie- rarchical organization, the Banzhaf value of a worker is proportional to the difference between his marginal pro- ductivity at the top level and at the bottom level of the hierarchy summed over all the possible configurations of the organization. This new formulation also makes this notion very easy to compute. The remainder of the paper is organized as follows. Section 2 defines hierarchical organizations. In Section 3, a new formulation of the generalized Banzhaf value is provided. Section 4 conc ludes. 2. Hierarchical Organiz ati ons An organization is a list ,,NTV where is a non- N C opyright © 2012 SciRes. TEL  R. PONGOU ET AL. 370 empty finite set of workers, 1 =,, TT T a finite col- lection of finite sets of tasks with cardinility =2Tj, and a production function that maps each allocation of workers to the tasks into a real number which measures the aggregate output or productivity of the organization under that particular configuration (Pongou et al. [2]). For every , i is a set of identical tasks. Denote by i a representative task of i. An allocation of workers to the tasks is a j-partition 1 V =, =1,ijT t T , j AA of , where i N is the set of w ork e rs assigned to type tasks (or simply to task i). Under an allocation 1j i tt =, , AA, i may be empty for any , which would mean that no worker has been assigned to task . The set of all possible allocations is denoted =1,ij i t . In a hierarchical organization, tasks are ordered in de- gree of importance: 1 is the most important task, 2 the second most important task, and so on. A typical ex- ample is a military organization where each higher- ranking member is more competent and has more im- portant tasks than his subordinates. A hierarchical organi- zation is said to be monotonic if aggregate output in- creases as a worker moves from a less important task to a more important one. The concept of monotonicity is for- malized below. t t Let 1 =,, j A 1,2,l A j be an ordered j-partition of . For any , denote by 1l N ,= l A =1, l A j the set of workers executing a task at least as important as task . Let 1 be an ordered j-parti- tion of such that for any. That is, is obtained from by moving some workers to more important tasks. This is denoted by l tN B =,BB l A , jB l B A j B. A multi-task organization is said to be monotonic if for any ordered j-parti tions ,,NTV , AB such that j B, VAVB1. 3. The Generalized Banzhaf Value and a New Formulation The generalized Banzhaf value developed by Freixas [1] is adapted to our framework as follows. Let ,,VNT be a monotonic hierarchical organization, and the range of Without loss of generality, assume 12 vv Let 12 ,..vv ,., k v.V >. k v>> be an al- location of workers and a worker. Denote by the task assigned to in the allocation pp ,pA , and by the allocation obtained from by moving ne level down the organization ladder : p o ,= p pA ,1,pA ,=, p qA qA . ,qNq p Let ,1,2,,lm k be such that . is said to be 1< lmk p ,lm-critical in if: => = lm p vVAVA v . Denote by ,lm pV the number of allocations in which p is , -critical,lm and by ,lm ip V num- ber of allocations the ,...,j1 = AA in which is p ,lm-critical and p i (1 follows that: 1). Itij 1 ,, =1 =. j lm lm pip i VV The Banzhaf value is defined below. Definition 1. Let ,,NTV be a mon dNp otonic hierarchi- cal organizatio n, an a worker. 1) The Banzhaf scor is: e of p V 2) The normalized Banzhaf index of is: p =km V v 1, =2 =1 lm pl m ml v p =, pV V j pi i V The Banzhaf index of a worker measures his earnings in terms of the proportion of the organization’s revenue he is expected to obtain. Our goal is to give a new interpretation of this sharing rule. Let be an al- location of workers and pN a worker. Denote by 1 the allocation obtained by moving p from his position in up to the top level of the organization. Similarly, denote by the allocation obtained by moving p from his position in down to the bottom level of the organization. We show below that the Banzhaf score of p is proportional to the difference between his marginal productivity at the top level of the organization ( p VA VA) and his marginal pro- ductivity at the bottom level ( j p VA VA) summed over all the possible worker allocations. Theorem 1. Let 1 ,,NTV be a mono hierarchi- cal organization, and pN tonic a worker. The Banzhaf score of p is given by: 1 1 =. j p V pp A VAVA j The definition below will be needed in the proof of Theorem 1. Definition 2. Let and B be two j-partitions of N and p a worker. B is said to be p-euivalenqt to if ,qN q,p ,= ,.q qA B obvious that the relation defined ave is er o It isbo an equi- 1A monotonic hierarchical organization as defined here can be viewed as a reinterpretation of the notion of (j, k) simple games (Freixas and Zwicker [10]). Note, however, that not all hierarchical organizations are monotonic (Pongou et al. [2]). valencerelation. The numbf elements in each equi- Copyright © 2012 SciRes. TEL 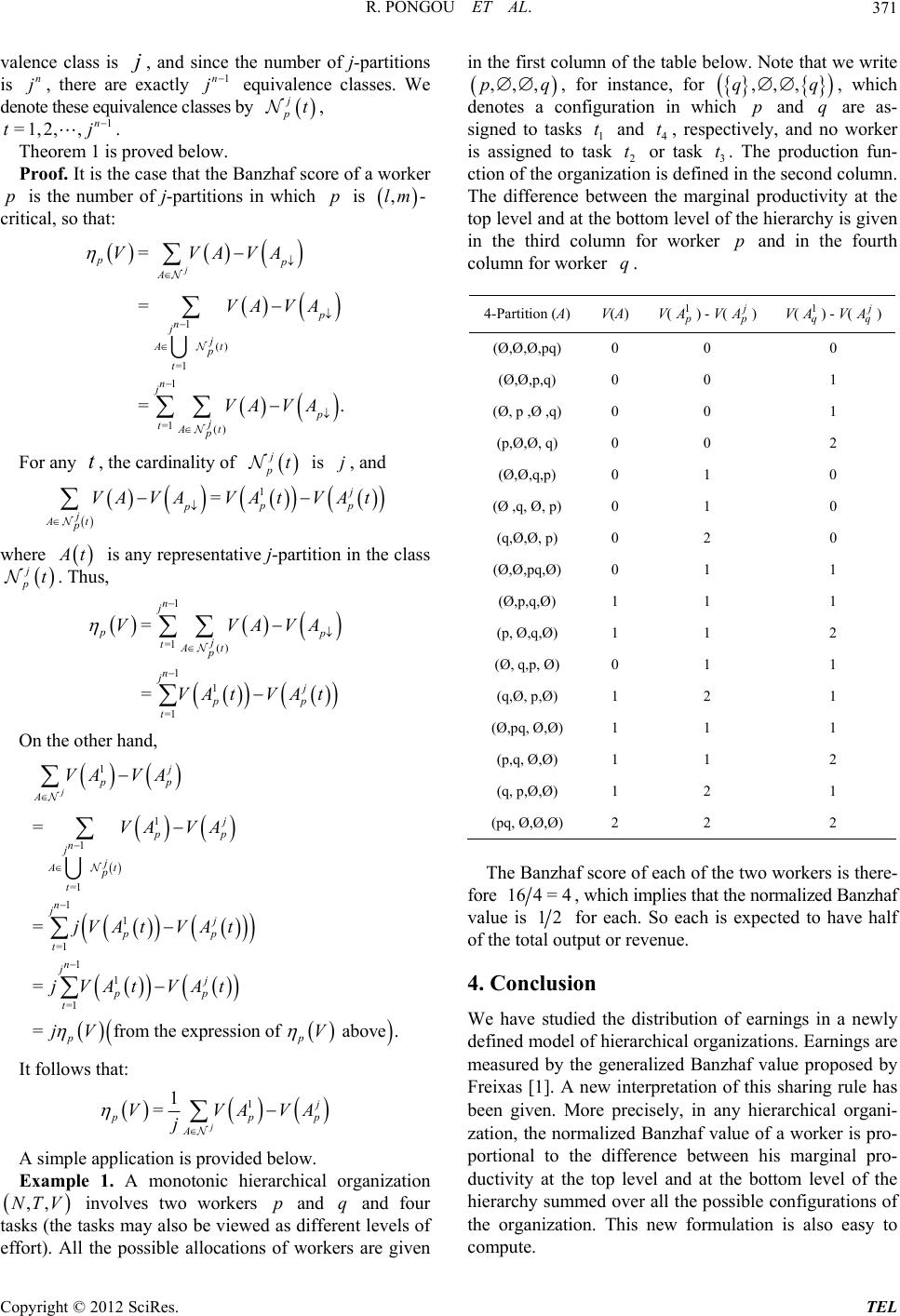 R. PONGOU ET AL. 371 valence class is , and since the number of j-partitions is n j, there are exactly 1n j equivalence classes. We denote th ese equi vale nce classe s by j pt, 1 =1,2,, n tj . Theorem 1 is proved below. Proof. It is the case that the Banzhaf score of a worker s in which is p is the number of j-partition p ,-lm cr For any , the cardinality of is , and where itical, so that: = pVVAVA 1 () =1 1 =1 () = =. jp A p n jj At p t n j p j tAt p VA VA VA VA t j ptj = p j At p A VAVVt 1j pp VAt A t . Thusis any representative j-partition in the class , A On the other hand, p It follows that: j pt 1n j pV =1 () 1 1 =1 = = p j tAt p n jj pp t VAV VAt VAt jpp A VA VA 1 1 1 =1 1 1 =1 1 1 =1 = = = =from the expression of above. j j pp n jj At p t n jj pp t n jj pp t p VA VA jV AtV At jVAt VAt jV V 1 1 =j pp A VAVA j A simple application is provided below. Example 1. A monotonic hierarchical organization and four effort). Ars V N tas ,,TV involves two workers p and q ks (the tasks may also be viewed as different levels of ll the possible allocations of worke are given in the first column of the table below. Note that we write ,,,pq, for instance, for ,,,qq , which denotes a configuration in which p and q are as- sks 1 t and 4 t, respe worker is assigned to task 2 t or task 3 t. T prodution fun- ction of the organization is defined in the second column. The difference betwen the marginal productivity at the top level and at the bottom level of the hierarchy is given in the third column for worker p and in the fourth column for worker q. 4-Partition (A) V(A signed to tactively, and no he c e ) V(1 V( ) V(1 q ) - V( q )) - (Ø,Ø,Ø,pq) 0 0 0 (Ø,Ø,p,q) 0 0 1 ( ( ( ( ( Ø, p ,Ø ,q)0 0 1 (p,Ø,Ø, q) 0 0 2 (Ø,Ø,q,p) 0 1 0 Ø ,q, Ø, p)0 1 0 (q,Ø,Ø, p) 0 2 0 Ø,Ø,pq,Ø) 0 1 1 (Ø,p,q,Ø) 1 1 1 (p, Ø,q,Ø) 1 1 2 (Ø, q,p, Ø) 0 1 1 (q,Ø, p,Ø) 1 2 1 Ø,pq, Ø,Ø)1 1 1 (p,q, Ø,Ø) 1 1 2 (q, p,Ø,Ø) 1 2 1 pq, Ø,Ø,Ø)2 2 2 f of each workere- re The Banzhascore of the two rs is the fo 164=4, which implies that normBanzhaf va the alized lue is 12 for each. So each is expected to have half of theut or revenue. 4. Conclusion total outp distribution rning a newly hical ornizationsngs are We have studied t defined model of hh ie erarc of ga eas in . Earni measured by the generalized Banzhaf value proposed by Freixas [1]. A new interpretation of this sharing rule has been given. More precisely, in any hierarchical organi- zation, the normalized Banzhaf value of a worker is pro- portional to the difference between his marginal pro- ductivity at the top level and at the bottom level of the hierarchy summed over all the possible configurations of the organization. This new formulation is also easy to compute. Copyright © 2012 SciRes. TEL  R. PONGOU ET AL. Copyright © 2012 SciRes. TEL 372 ixas, “The Banzhaf Measures for Games with Sev- eral Levels of Approval in the Input and Output,” Annals of Operations 1, 2005, pp. 45-66. doi:10.1007/s1 REFERENCES [1] J. Fre Research, Vol. 137, No. 0479-005-2244-9 [2] R. Pongou, B. Tchantcho and N. Tedjeugang, “On-the- Job Trial Tournament: Productivity Rank and Earnings,” Mimeo, 2012. J. Banzhaf, “Weighted Voting D[3] oesn’t Work: A Mathe- matical Analysis,” Rutgers Law Review, Vol. 19, No.2, 1965, pp. 317-343. J. Coleman, “C[4] ontrol of Collectivities and the Power of a Collectivity to Act,” In: B. Lieberman, Ed., Social Ch oice , Gordon and Breach, New York, 1971, pp. 269-300. P. Dubey and L. Sha[5] pley, “Mathematical Properties of the Banzhaf Power Index,” Mathematics of Operations Re- search, Vol. 4, No. 2, 1979, pp. 99-131. doi:10.1287/moor.4.2.99 [6] A. Laruelle and F. Valenciano, “Shapley-Shubik and Banz- haf Indices Revisited,” Mathematics of Operations Re- search, Vol. 26, No. 1, 2001, pp. 89-104. .10589doi:10.1287/moor.26.1.89 er Indices for ience, Vol. 34, No. 4, [7] D. Leech, “The Relationship between Shareholding Con- centration and Shareholder Voting Power in British Com- panies: A Study of the Application of Pow Simple Games,” Management Sc 1988, pp. 509-529. doi:10.1287/mnsc.34.4.509 [8] D. Leech, “Computing Power Indices for Large Voting Games,” Management Science, Vol. 49, No. 6, 2003, pp. 831-838. doi:10.1287/mnsc.49.6.831.16024 [9] V. Feltkamp, “Alternative Axiomatic Characterizations of the Shapley and Banzhaf Values,” International Journal of Game Theory, Vol. 24, No. 2, 1995, pp. 179-186. doi:10.1007/BF01240041 [10] J. Freixas and W. S. Zwicker, “Weighted Voting, Absten- tion, and Multiple Levels of Approval,” Social Choice and Welfare, Vol. 21, No. 3, 2003, pp. 399-431. doi:10.1007/s00355-003-0212-3 [11] L. Diffo Lambo and J. Moulen, “Ordinal Equivalence of Power Notions in Voting Games,” Theory and Decision, Vol. 53, No. 4, 2002, pp. 313-325. doi:10.1023/A:1024158301610 [12] J. Freixas, D. Marciniako and M. Pons, “On the Ordinal Equivalence of the Johnston, Banzhaf and Shapley Power Indices,” European Journal of Operation Research, Vol. 216, No. 2, 2011, pp. 367-375. doi:10.1016/j.ejor.2011.07.028 [13] B. Tchantcho, L. Diffo Lambo, R. Pongou and B. Mbama Engoulou, “Voters’ Power in Voting Games with Absten- tion: Influence Relation and Or dinal Equivalence of Power Theories,” Games and Economic Behavior, Vol. 64, No. 1, 2008, pp. 335-350. doi:10.1016/j.geb.2007.10.014 [14] R. Pongou, B. Tchantcho and L. Diffo Lambo, “Political Influence in Multi-Choice Institutions: Cyclicity, Ano- nymity, and Transitivity,” Theory and Decision, Vol. 70, No. 2, 2011, pp. 157-178. doi:10.1007/s11238-010-9211-x
|