Vol.2, No.8, 793-802 (2010) Natural Science
http://dx.doi.org/10.4236/ns.2010.28100
Potentiometric, spectrophotometric, conductimetric and
thermodynamic studies on some transition metal
complexes derived from 3-methyl-1-phenyl- and
1, 3-diphenyl-4-arylazo-5-pyrazolones
Samir A. Abdel-Latif1*, Saber E. Mansour2, Abdulrahman A. Fatouh2
1Chemistry Department, Faculty of Science, Helwan University, Helwan, Egypt; *Corresponding Author: [e-mail]
2Chemistry Department, Faculty of Science, Omar Al-Mukhtar University, Al-Beidaa , Li by a
Received 6 March 2010; revised 23 April 2010; accepted 28 April 2010.
ABSTRACT
A new 3-m et h yl-1-phenyl-4-arylazo-5-pyrazolone
and 1, 3-diphenyl-4-arylazo-5-pyrazolone have
been synthesized and characterized by ele-
mental analysis, IR, mass and 1H NMR spectra.
The acid dissociation constants (pKa values) of
the investigated ligands were determined po-
tentiometrically and spectrophotometrically. The
stability constants of the transition metal ions
(VO2+, Cr3+, Mn2+, Co2+, Ni2+, Cu2+ and Zn2+) with
the investigated ligands were determined po-
tentiometrically at different ionic strengths (0.167,
0.1, 0.05 and 0.025 M) NaCl at 25ºC and different
temperature (25, 30, 35, and 45ºC). The values of
stability constants were found to decrease with
increasing ionic strengths and temperature. The
stoichiometries were studied using spectro-
photometric and conductimetric methods, the
results indicate the existence of 1:1 and 1:2 (M:L)
metal:ligand species. The relationships between
the stability constants of the complexes, ioniza-
tion constants of the ligands have been dis-
cussed and correlated. The thermodynamic pa-
rameters (∆G, ∆H and ∆S) and the thermody-
namic stability constants for all of the investi-
gated complexes were determined potentiome-
trically.
Keywords: Azopyrazolones; Lonization Constants;
Stability Constants; Thermodynamic Parameters;
Transition Metal Complexes; Potentiometry;
Spectrophotometry
1. INTRODUCTION
Pyrazolone and azopyrazolone compounds are widely
used as analytical reagents, they are capable of form-
ing chelates with a number of metal cations [1,2], the
formation of which is accompanied by change in color,
pH, conductivity, and absorption spectra [3]. Azo de-
rivatives have attracted much attention by virtue of
their applicability as potential ligands for a large
number of metal ions [1]. The 4-position of pyrazo-
line-5-one system is highly reactive and undergoes
coupling reaction with diazonium salts to give 4-ary-
lazo derivatives [4]. The azo-derivatives of 5-pyra-
zolones as well as their metal complexes have wide
applications in dye industry as well as analytical rea-
gents for determination of trace metals and it is pre-
dicted to have some medical and biological applica-
tions [5,6]. Different methods were reported for the
syntheses of azopyrazolone derivatives [7-12].
The present paper deals with the determination of
the stability constants of the binary complexes of
VO2+, Cr3+, Mn2+, Co2+, Ni2+, Cu2+ and Zn2+ metal
ions with the investigated ligands (L1-L6) in the
presence of 0.167, 0.1, 0.05 and 0.025 M at 25ºC. The
thermodynamic parameters (∆G, ∆H and ∆S) and the
thermodynamic stability constants of the investigated
complexes were evaluated in the presence of 0.1 M
NaCl in the temperature range 25-45ºC. The Irving
and Rossotti pH-metric titration using Sarin and
Munchi technique [13] was used to determine the acid
dissociation constants as well as the formation con-
stants for the various complexes at 25ºC. The acid
dissociation constants were also done in 40% etha-
nolic buffer solutions of varying pH values spectro-
photometrically [14]. The molar ratio of the metal
ions to ligands [M]/[L] were studied spectrophotome-
trically using molar-ratio and continuous variation
methods and were also determined using conducti-
metric titrations in aqueous ethanolic solutions (40%
v/v).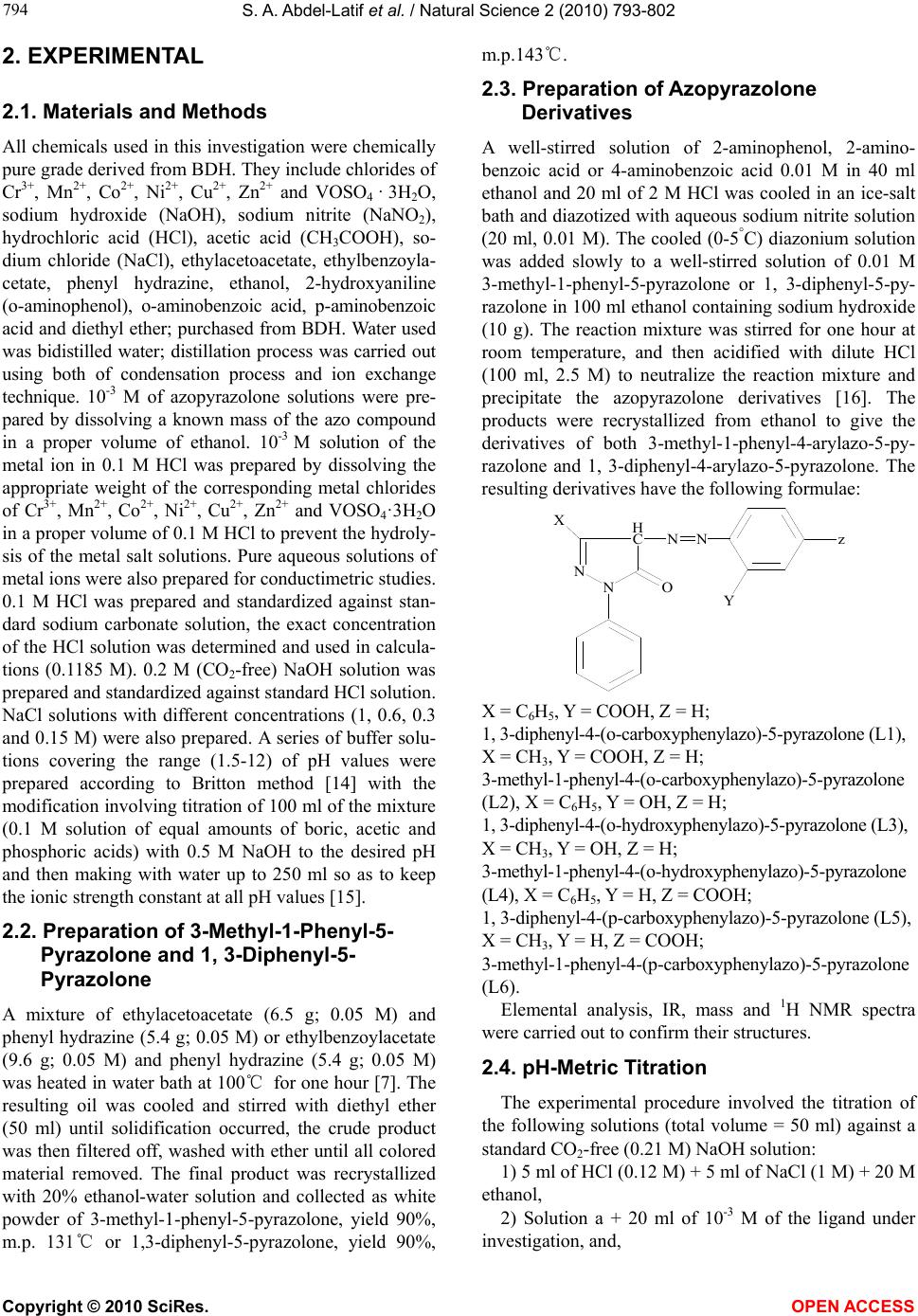

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
794
2. EXPERIMENTAL
2.1. Materials and Methods
All chemicals used in this investigation were chemically
pure grade derived from BDH. They include chlorides of
Cr3+, Mn2+, Co2+, Ni2+, Cu2+, Zn2+ and VOSO4 · 3H2O,
sodium hydroxide (NaOH), sodium nitrite (NaNO2),
hydrochloric acid (HCl), acetic acid (CH3COOH), so-
dium chloride (NaCl), ethylacetoacetate, ethylbenzoyla-
cetate, phenyl hydrazine, ethanol, 2-hydroxyaniline
(o-aminophenol), o-aminobenzoic acid, p-aminobenzoic
acid and diethyl ether; purchased from BDH. Water used
was bidistilled water; distillation process was carried out
using both of condensation process and ion exchange
technique. 10-3 M of azopyrazolone solutions were pre-
pared by dissolving a known mass of the azo compound
in a proper volume of ethanol. 10-3 M solution of the
metal ion in 0.1 M HCl was prepared by dissolving the
appropriate weight of the corresponding metal chlorides
of Cr3+, Mn2+, Co2+, Ni2+, Cu2+, Zn2+ and VOSO4·3H2O
in a proper volume of 0.1 M HCl to prevent the hydroly-
sis of the metal salt solutions. Pure aqueous solutions of
metal ions were also prepared for conductimetric studies.
0.1 M HCl was prepared and standardized against stan-
dard sodium carbonate solution, the exact concentration
of the HCl solution was determined and used in calcula-
tions (0.1185 M). 0.2 M (CO2-free) NaOH solution was
prepared and standardized against standard HCl solution.
NaCl solutions with different concentrations (1, 0.6, 0.3
and 0.15 M) were also prepared. A series of buffer solu-
tions covering the range (1.5-12) of pH values were
prepared according to Britton method [14] with the
modification involving titration of 100 ml of the mixture
(0.1 M solution of equal amounts of boric, acetic and
phosphoric acids) with 0.5 M NaOH to the desired pH
and then making with water up to 250 ml so as to keep
the ionic strength constant at all pH values [15].
2.2. Preparation of 3-Methyl-1-Phenyl-5-
Pyrazolone and 1, 3-Diphenyl-5-
Pyrazolone
A mixture of ethylacetoacetate (6.5 g; 0.05 M) and
phenyl hydrazine (5.4 g; 0.05 M) or ethylbenzoylacetate
(9.6 g; 0.05 M) and phenyl hydrazine (5.4 g; 0.05 M)
was heated in water bath at 100℃ for one hour [7]. The
resulting oil was cooled and stirred with diethyl ether
(50 ml) until solidification occurred, the crude product
was then filtered off, washed with ether until all colored
material removed. The final product was recrystallized
with 20% ethanol-water solution and collected as white
powder of 3-methyl-1-phenyl-5-pyrazolone, yield 90%,
m.p. 131℃ or 1,3-diphenyl-5-pyrazolone, yield 90%,
m.p.143℃.
2.3. Preparation of Azopyrazolone
Derivatives
A well-stirred solution of 2-aminophenol, 2-amino-
benzoic acid or 4-aminobenzoic acid 0.01 M in 40 ml
ethanol and 20 ml of 2 M HCl was cooled in an ice-salt
bath and diazotized with aqueous sodium nitrite solution
(20 ml, 0.01 M). The cooled (0-5°C) diazonium solution
was added slowly to a well-stirred solution of 0.01 M
3-methyl-1-phenyl-5-pyrazolone or 1, 3-diphenyl-5-py-
razolone in 100 ml ethanol containing sodium hydroxide
(10 g). The reaction mixture was stirred for one hour at
room temperature, and then acidified with dilute HCl
(100 ml, 2.5 M) to neutralize the reaction mixture and
precipitate the azopyrazolone derivatives [16]. The
products were recrystallized from ethanol to give the
derivatives of both 3-methyl-1-phenyl-4-arylazo-5-py-
razolone and 1, 3-diphenyl-4-arylazo-5-pyrazolone. The
resulting derivatives have the following formulae:
X = C6H5, Y = COOH, Z = H;
1, 3-diphenyl-4-(o-carboxyphenylazo)-5-pyrazolone (L1),
X = CH3, Y = COOH, Z = H;
3-met h y l-1-phenyl-4-(o-carboxyphenylazo)-5-pyrazolone
(L2), X = C6H5, Y = OH, Z = H;
1, 3-diphenyl-4-(o-hydroxyphenylazo)-5-pyrazolone (L3),
X = CH3, Y = OH, Z = H;
3-met h y l-1-phenyl-4-(o-hydroxyphenylazo)-5-pyrazolone
(L4), X = C6H5, Y = H, Z = COOH;
1, 3-diphenyl-4-(p-carboxyphenylazo)-5-pyrazolone (L5),
X = CH3, Y = H, Z = COOH;
3-methyl-1-phenyl-4-(p-carboxyphenylazo)-5-pyrazolone
(L6).
Elemental analysis, IR, mass and 1H NMR spectra
were carried out to confirm their structures.
2.4. pH-Metric Titration
The experimental procedure involved the titration of
the following solutions (total volume = 50 ml) against a
standard CO2-free (0.21 M) NaOH solution:
1) 5 ml of HCl (0.12 M) + 5 ml of NaCl (1 M) + 20 M
ethanol,
2) Solution a + 20 ml of 10-3 M of the ligand under
investigation, and,
N
N
C
H
O
X
N N
Y
z

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
795
795
3) Solution b + 5 ml of metal salt solution (10-3 M).
These titrations were repeated for 1) ionic strengths I
= 0.167, 0.1, 0.05, and 0.25 M NaCl, at 25ºC, 2) various
temperatures (25, 30, 35, and 45ºC) at I = 0.167 M NaCl.
The pH measurements were carried out using a Jen-
way, pH-meter 3310 with a glass combined electrode.
The water thermostat was a Thermo Haake WKL 26,
Karlsruhe, Germany accurate to ±0.1ºC. The solutions
were equilibrated in the thermostat for about 15 min
before titration. The equations used for the various cal-
culations [17] were programmed into IBM computer.
2.5. Determination of the Thermodynamic
Parameters
The following thermodynamic parameters: ∆G°, ∆H° and
∆S° were determined for each chelate depending on their
stability constants [18]. The free energy of formation
(∆G°) of a complex is related to its stability constant by
the relation [18]: –∆G° = 2.303 RT logβ where, R = uni-
versal gas constant, T = absolute temperature and logβ =
stability constant of the complex. Enthalpy of formation
(∆H°) and entropy (∆S°) were calculated by plotting logβ
versus 1/T. We can specify the quantitative dependence
of the stability constant on temperature from the relation
[18]:
∆G° = ∆H° – T∆S°
∆G° = –2.303 RT logβ = ∆H° – T∆S°
By rearranging, we get
logβ = –∆H°/(2.303 RT) + ∆S°/(2.303 R)
This is a linear equation of the form y = mx + b,
where y = logβ, m = –∆H°/(2.303 R) = slope, x = 1/T,
and b = ∆S°/(2.303 R) = intercept. This means that if the
values of K for a given reaction are determined at vari-
ous temperature, a plot of logβ versus 1/T will be linear,
with slope ∆H°/(2.303 R) and intercept ∆S°/(2.303 R).
This result assumes that both ∆H° and ∆S° are indepen-
dent of temperature over the temperature range consi-
dered. This assumption is a good approximation over a
relatively small temperature range [18].
2.6. Conductimetric Measurements
The conductivities of VO2+, Cr3+, Mn2+, Co2+, Ni2+, Cu2+
and Ni2+ metal ions with the investigated ligands (L1-L6)
were measured using conductivity meter (Philips pw
9526, digital conductivity meter). The experimental
procedure involves a conductimetric titration of 40 ml
alcohol-water mixture (50% v/v) solution containing 4 ×
10-5 M of a given azopyrazolone derivative against a
standard solution of 10-3 M aqueous metal ion solution
using microburette with continuous magnetic stirring.
The instrument reading was recorded after each addition.
All of the conductivity values were corrected for the
effect of dilution during titration. Corrections were made
by multiplying conductivity value (instrument reading)
by the ratio (V + v)/V, where V is the original volume of
the titrand (40 ml and v is the added volume of titrant
[19]). The corrected conductivity values were plotted
versus the molar ratio (L:M). The resulting curves are
composed of straight lines with inflection points indi-
cating the number of ligands around each central metal
ion.
2.7. Spectrophotometric Method Applied for
Determination of PKa Values
The absorption spectra of azopyrazolone compounds
under investigation (L1-L6) were scanned over a range
of wavelengths in universal buffer solutions of different
pH values. For this purpose a known volume of (10-3 M)
solution of the azopyrazolone derivative was added to
the buffer solution in a 10 ml volumetric flask dropwise
with continuous shaking. The mixture was then made up
to the mark with the buffer solution of appropriate pH.
The spectra were obtained at room temperature using
spectrophotometer (Jenway, 6305 UV-VIS spectropho-
tometer).
The method applied for the determination of pKa val-
ues of the different azopyrazolone derivatives is the half
height method [20]. This method depends on the fact that
the limiting absorbance (A1) represents complete con-
version of the compound from one form to other. Since
pKa is equal to the pH value at which the two forms
exist in equivalent amounts (pKa = pH (at A½), the pH
corresponding to half the height of the absorbance-pH
curve (A½) is equal to pKa. The (A½) value is given by
the relation:
1
12
2
−
= +
min
min
AA
AA
/
where A1 = maximum absorbance, Amin = minimum ab-
sorption.
The absorption spectra of 3 × 10-5 M solutions of the
azopyrazolones under investigation (ligands L1-L6)
were dissolved in 40% v/v ethanolic buffer solutions of
varying pH values.
2.8. Determination of the Molar Ratio of the
Metal Lons to the Ligands
Spectrophotometrically
The mole ratio of the metal ions to the ligands was stu-
died spectrophotometrically using molar ratio and con-
tinuous variation methods. The spectrophotometric me-
thod was used to confirm the data obtained by conduc-
timetric and pH-metric methods. UV absorption can be
used to determine stoichiometry of the complexes and
this method appears to be valuable for studying com-
plexes with low stabilities.

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
796
2.8.1. Molar Ratio Method
In this investigation, the concentration of the metal ions
were maintained constant and the ligands concentrations
were varied [21], so a series of metal-ligand aqueous
ethanolic solutions were prepared with different [L]/[M]
ratios. The absorptions of these solutions were measured
using UV spectrophotometer at λmax of the expected
complex ML2. Absorbance versus [L]/[M] curves were
drawn for all complexes.
2.8.2. Continuous Variation Method
This method was used to confirm the data obtained using
molar ratio and conductivity methods. In this method,
the mole fraction was varied by changing the concentra-
tions of the two components, maintaining the total num-
ber of moles constant [21]. A series of metal-ligand
aqueous ethanolic solutions were prepared with different
metal mole fractions. The mole fraction of the metal was
plotted against the absorbance of the expected complex
at λmax of the complex. The measured absorbance in-
crease as the molar ratio [M]/([L] + [M]) increase until
the actual molar ratio of the complex is reached, after
this point the absorbance becomes lower because the
metal has no absorption at λmax of the complex.
3. RESULTS AND DISCUSSION
3.1. Determination of Stability Constants
Titration curves were obtained for the titrations of VO2+,
Cr3+, Mn2+, Co2+, Ni2+, Cu2+ and Zn2+ metal ions in the
presence of different molarities of NaCl and different
temperatures. The stability constants of the formed com-
plexes were investigated in the pH range of 4-6.
The mean values of  (the average number of pro-
tons associated with the ligand) for the investigated li-
gands (L1-L6) at different pH values were calculated
from the titration curves of solutions 1 and 2 by em-
ploying the relationship derived by Irving and Rossotti
[17]. On plotting log/(1 – ) vs. pH, a straight lines
having an intercept equal to pKa on the pH axis are ob-
tained. From the titration curves of solutions 1-3, the
metal + ligand formation number values � (the average
number of ligand molecules coordinated to the metal ion)
of the metal complexes were obtained at various pH
values. The � values were found to be less or equal to 2,
indicating the formation of 1:1 and 1:2 complexes. The
� values were calculated using the Irving and Rossotti
formulation [17]. The free ligand exponent pL was cal-
culated from the so obtained values of � by the equa-
tion:
1
0
1
10
log
=
=




+


=−



∑
yH
yB
y
c
LM
VV
V
C
n
pL C
ο
ο
β
where, CM is the concentration of ions Mn+ used, CL is
the concentration of the ligand, y is the number of disso-
ciable protons (
y = 1 for the investigated ligands), and Vo
is the original volume (50 ml), Vc is the volume of alkali
(NaOH) consumed to reach the same pH values in curve
c corresponding to the titration of solution 3, 
 is the
formation constant values of the investigated ligands,
and B is the pH value. The mean pKa values obtained
from the corresponding different experimental formation
curves using the average value and straight line methods.
The results obtained for proton-ligand systems (pKa
values) were 3.98, 4.04, 7.68, 7.93, 3.45 and 3.66 for the
investigated ligands L1-L6, respectively. Referring to
these data, the pKa values of ligands L5 and L6 have the
lowest values from all of the six ligands used in this
work because the ionizble group (–COOH) locates in the
para position where there is no attraction with the car-
bonyl group of the pyrazolone ring, so it is easy to lose
H+ ion, whereas the ligands L1 and L2 have pKa values
higher than L5 and L6 because the ionizable group
(–COOH) locates in ortho position, so there is a hydro-
gen bond between the carbonyl group of the pyrazolone
ring and –COOH, as a result; it is more difficult to lose
H+, so these two ligands have pKa values higher than L5
and L6 [22]. The pKa values of ligands L3 and L4 have
the highest values from all because the ionizable group
of these two ligands (–OH) locates in ortho position with
lower ability to librate H+ ion because hydroxyl group
has lower acidity than carboxyl group and because of the
presence of hydrogen with the carbonyl group of the
pyrazolone ring [23]. Ligand L1 has pKa value < L2, and
L3 < L4, and L5 < L6, that is because of the presence of
additional phenyl group (electron withdrawing group) in
ligands L1, L3 and L5. The formation curves for the
complexes were obtained by plotting the relation be-
tween average number of ligands attached per metal ion
(�) and free ligand exponent (pL), � and pL were calcu-
lated as previously mentioned. To compute successive
stability constants (logβ1 and logβ2) the method of in-
terpolation is used [17], where logβ1 and logβ2 are equal
to the values of (pL) when (�) = 0.5 and 1.5, respectively.
logβ1 and logβ2 for all complexes are given in Table 1.
3.2. Relations between the Properties of
Central Metal Lons and the Stability
Constants of the Complexes
The transition metal ions form predominantly ionic and
coordinate bonds. If the bonds are ionic, the born rela-

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
797
797
Table 1. Collective data of stability constants (logβ1 and logβ2) for all of the investigated complexes.
Zn
2+
Cu
2+
Ni
2+
Co
2+
Mn
2+
Cr
3+
VO
2+
Ligand
logβ2 logβ1 logβ2 logβ1 logβ2 logβ1 logβ2 logβ1 logβ2 logβ1 logβ2 logβ1 logβ2 logβ1
6.23 4.02 6.60 4.21 6.38 4.08 6.18 3.99 5.29 3.53 6.04 3.92 5.83 3.78 L1
7.25 4.82 7.41 4.87 7.53 4.83 8.04 4.90 7.52 4.71 7.31 4.78 7.70 4.72 L2
9.87 5.73 9.94 5.76 10.07 5.75 10.06 5.67 8.81 5.43 9.93 5.65 9.54 5.63 L3
10.19 5.84 10.47 5.94 10.14 5.90 9.91 5.80 9.50 5.63 10.15 5.79 9.76 5.77 L4
6.66 3.78 5.68 3.83 6.71 3.83 5.85 3.75 5.34 3.25 5.68 3.60 4.29 3.26 L5
8.37 4.46 8.01 4.58 8.55 4.52 8.05 4.37 6.78 4.25 6.78 4.33 6.34 4.26 L6
tion (E = Z2/2r [1 – 1/D]) [24] can be hold for the energy
change on complexation of an ion of charge (Z) and ra-
dius (r) [25-27] in a medium of dielectric constant (D).
Since the stability constant is related directly to this
energy, logβ values should increase linearly with Z2/r.
The stability constants of some transition metal com-
plexes show different behaviors which suggest the
probable existence of linearity and nonlinearity of logβ
with Z2/r [28]. The plots of logβ1 and logβ2 versus Z2/r of
the transition metal ions (VO2+, Cr3+, Mn2+, Co2+, Ni2+,
Cu2+ and Zn2+) complexes with the investigated ligands
(L1-L6), does not exhibit linear increase of logβ with
increase of Z2/r. Interpretation in term of the assumption
about ionic character of metal-ligand on which the li-
nearity based is not valid. The other probable case is
steric effects. It is also interest to study the logβ1 and
logβ2 values of the transition metal complexes with the
investigated ligands (L1-L6) as a function of atomic
number 1/r (the inverse of ionic radius) as well as EN
(electronegativity) [28] of metal ions. In this investiga-
tion, it was found that the stability constants of the first
raw transition metal complexes with the investigated
ligands (L1-L6) (logβ values) are generally increase with
decreasing ionic radius r (or increasing 1/r) and increase
with increasing atomic number [29], also logβ increases
with increasing electonegativity, this is because upon
increasing electronegativity of the metal ions, the elec-
tronegativity difference between the metal atom and the
donor atom of the ligand would expectedly associate
with increase of covalent character of the metal-ligand
bond. These relations are not linear and there are some
exceptions from the mentioned observations because of
the presence of steric effects and ionic bonds. The rela-
tions between the ionization constants (pKa) of the li-
gands and the stability constants (logβ1, logβ2) of their
complexes with the transition metal ions (VO2+, Cr3+,
Mn2+, Co2+, Ni2+, Cu2+ and Zn2+) can be studied. It is
observed that as pKa of the ligand increases, the stability
constant logβ of the complex increase. The semi linear
correlation observed in this study demonstrates that fac-
tors which increase or decrease pKa of the ligand also
affect the logKML values for the metal ions in a parallel
fashion. This means that substituent groups that tend to
increase electron density on donor atom and hence tend
to increase the coordination ability of the ligand increase
logKML, and also increase the value of pKa of the ioniza-
ble hydrogen [29]. The assumption here is that ligand
basicity is directly related to ligand stability i.e., more
stable complexes being formed from basic ligands [29].
Such correlation cannot be generalized because of the
presence of some exceptions from linearity and because
this result was observed form the ligands and the metal
ions used in this study only.
3.3. Effect of Lonic Strength on Stability
Constants
The stability constants of the metal ions (VO2+, Cr3+,
Mn2+, Co2+, Ni2+, Cu2+ and Zn2+) complexes with the
investigated ligands (L1-L6) were found to decrease
with increasing ionic strength of the medium as shown
in Table 2. For each complex the relationship between
the stability constant and the square root of the ionic
strength is plotted in Figures 1, 2 and was in agreement
with the Debye-Hűckel equation [30]. The thermody-
namic stability constants (logβ°) were obtained by
extrapolating the straight line plots of logβ versus √I to
zero ionic strength. From the plots of logβ versus √I
which illustrated in Figures 1 and 2, the logβ° (thermo-
dynamic stability constants) values were obtained and
listed in Table 3. Also values of pKa were found to de-
crease with increasing ionic strength of the medium
which are in agreement with Debye-Hűckel equation
[30,31]. The equation ∆G = –2.303 RT logβ gives the
relationship between the thermodynamic stability con-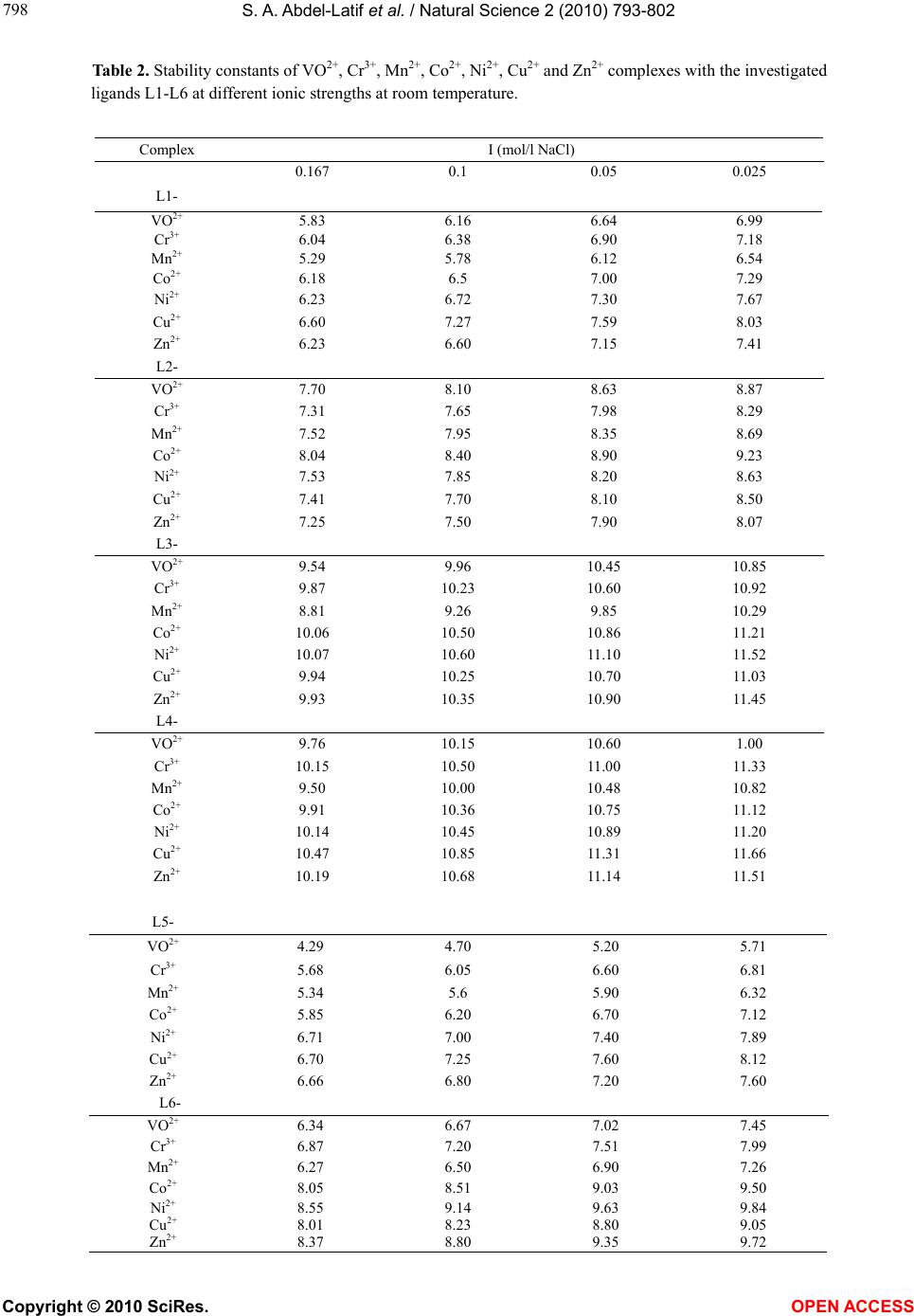

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
798
Table 2. Stability constants of VO2+, Cr3+, Mn2+, Co2+, Ni2+, Cu2+ and Zn2+ complexes with the investigated
ligands L1-L6 at different ionic strengths at room temperature.
I (mol/l NaCl) Complex
0.025
0.05
0.1
0.167
L1-
6.99
6.64
6.16
5.83
VO2+
7.18
6.90
6.38
6.04
Cr3+
6.54
6.12
5.78
5.29
Mn2+
7.29
7.00
6.5
6.18
Co2+
7.67 7.30 6.72 6.23 Ni
2+
8.03 7.59 7.27 6.60 Cu
2+
7.41
7.15
6.60
6.23
Zn2+
L2-
8.87
8.63
8.10
7.70
VO2+
8.29 7.98 7.65 7.31 Cr
3+
8.69 8.35 7.95 7.52 Mn
2+
9.23
8.90
8.40
8.04
Co2+
8.63
8.20
7.85
7.53
Ni2+
8.50
8.10
7.70
7.41
Cu2+
8.07 7.90 7.50 7.25 Zn
2+
L3-
10.85 10.45 9.96 9.54 VO
2+
10.92
10.60
10.23
9.87
Cr3+
10.29
9.85
9.26
8.81
Mn2+
11.21
10.86
10.50
10.06
Co2+
11.52 11.10 10.60 10.07 Ni2+
11.03 10.70 10.25 9.94 Cu
2+
11.45
10.90
10.35
9.93
Zn2+
L4-
1.00
10.60
10.15
9.76
VO2+
11.33 11.00 10.50 10.15 Cr3+
10.82 10.48 10.00 9.50 Mn
2+
11.12
10.75
10.36
9.91
Co2+
11.20 10.89 10.45 10.14 Ni
2+
11.66
11.31
10.85
10.47
Cu2+
11.51
11.14
10.68
10.19
Zn2+
L5-
5.71 5.20 4.70 4.29 VO2+
6.81 6.60 6.05 5.68 Cr
3+
6.32
5.90
5.6
5.34
Mn2+
7.12
6.70
6.20
5.85
Co2+
7.89
7.40
7.00
6.71
Ni2+
8.12 7.60 7.25 6.70 Cu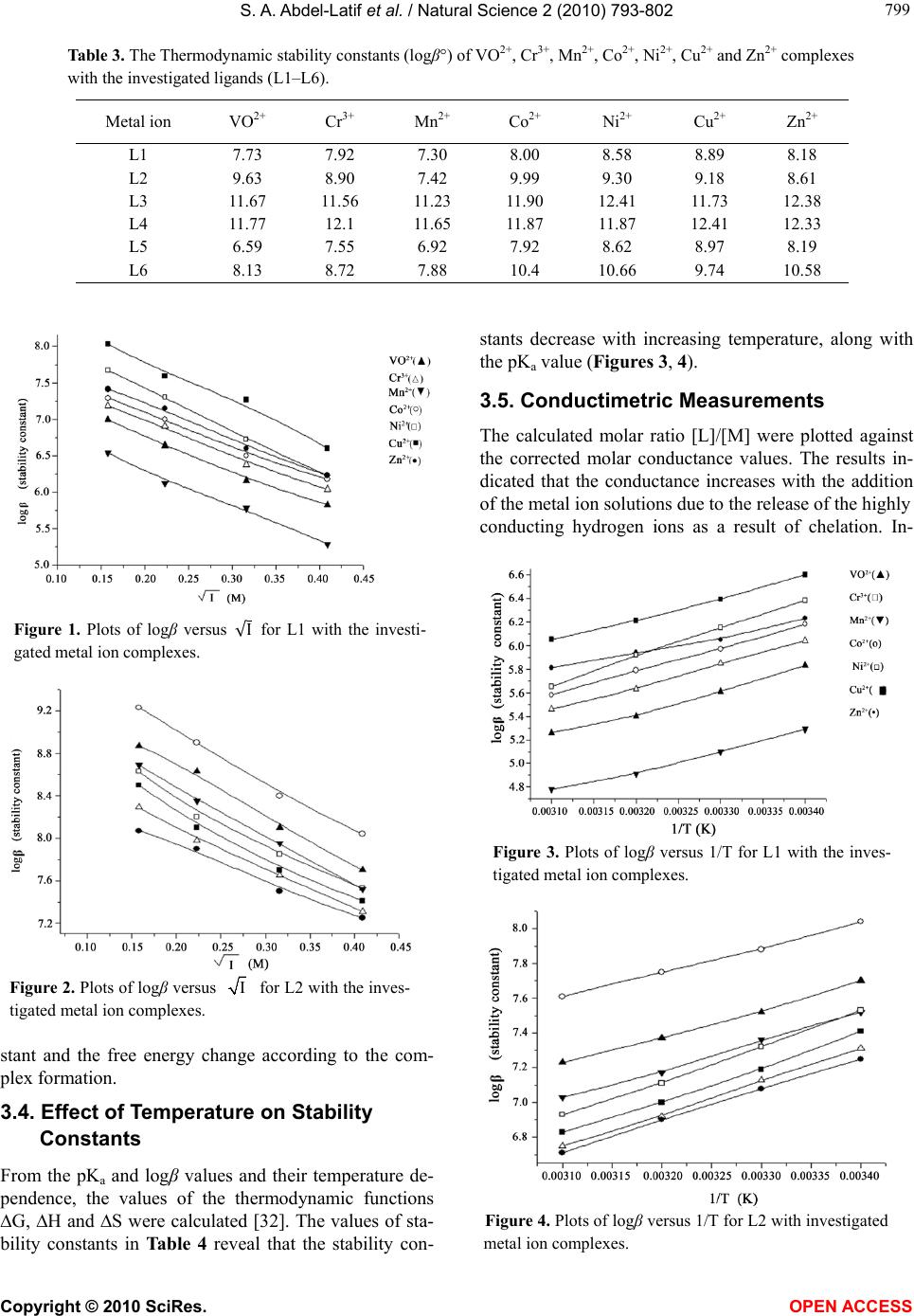
2+
7.60
7.20
6.80
6.66
Zn2+
L6-
7.45
7.02
6.67
6.34
VO2+
7.99
7.51
7.20
6.87
Cr3+
7.26
6.90
6.50
6.27
Mn2+
9.50
9.03
8.51
8.05
Co2+
9.84
9.63
9.14
8.55
Ni2+
9.05
8.80
8.23
8.01
Cu2+
9.72
9.35
8.80
8.37
Zn2+

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
799
799
Table 3. The Thermodynamic stability constants (logβ°) of VO2+, Cr3+, Mn2+, Co2+, Ni2+, Cu2+ and Zn2+ complexes
with the investigated ligands (L1–L6).
Figure 1. Plots of logβ versus √I for L1 with the investi-
gated metal ion complexes.
Figure 2. Plots of logβ versus
I
for L2 with the inves-
tigated metal ion complexes.
stant and the free energy change according to the com-
plex formation.
3.4. Effect of Temperature on Stability
Constants
From the pKa and logβ values and their temperature de-
pendence, the values of the thermodynamic functions
∆G, ∆H and ∆S were calculated [32]. The values of sta-
bility constants in Table 4 reveal that the stability con-
stants decrease with increasing temperature, along with
the pKa value (Figures 3, 4).
3.5. Conductimetric Measurements
The calculated molar ratio [L]/[M] were plotted against
the corrected molar conductance values. The results in-
dicated that the conductance increases with the addition
of the metal ion solutions due to the release of the highly
conducting hydrogen ions as a result of chelation. In-
Figure 3. Plots of logβ versus 1/T for L1 with the inves-
tigated metal ion complexes.
Figure 4. Plots of logβ versus 1/T for L2 with investigated
metal ion complexes.
Zn2+ Cu2+ Ni2+ Co2+ Mn2+ Cr3+ VO2+ Metal ion
8.18
8.89
8.58
8.00
7.30
7.92
7.73
L1
8.61
9.18
9.30
9.99
7.42
8.90
9.63
L2
12.38
11.73
12.41
11.90
11.23
11.56
11.67
L3
12.33
12.41
11.87
11.87
11.65
12.1
11.77
L4
8.19
8.97
8.62
7.92
6.92
7.55
6.59
L5
10.58 9.74 10.66 10.4 7.88 8.72 8.13 L6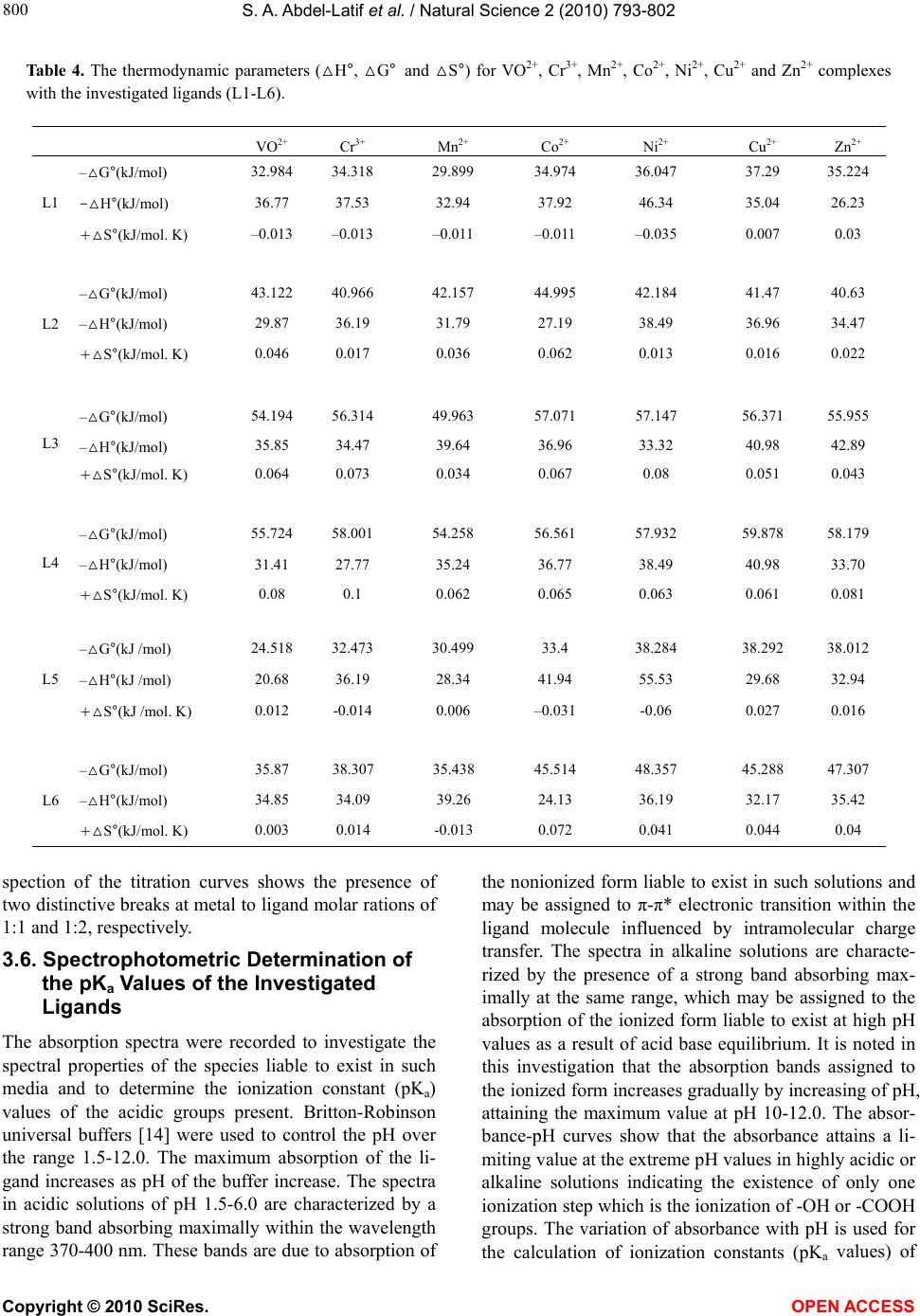

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
800
Table 4. The thermodynamic parameters (△H°, △G° and △S°) for VO2+, Cr3+, Mn2+, Co2+, Ni2+, Cu2+ and Zn2+ complexes
with the investigated ligands (L1-L6).
Zn
2+
Cu
2+
Ni
2+
Co
2+
Mn
2+
Cr
3+
VO
2+
35.224 37.29 36.047 34.974 29.899 34.318 32.984
–△G°(kJ/mol)
L1 26.23 35.04 46.34 37.92 32.94 37.53 36.77
-△H°(kJ/mol)
0.03 0.007 –0.035 –0.011 –0.011 –0.013 –0.013
+△S°(kJ/mol. K)
40.63 41.47 42.184 44.995 42.157 40.966 43.122 –△G°(kJ/mol )
L2 34.47 36.96 38.49 27.19 31.79 36.19 29.87 –△H°(kJ/mol )
0.022 0.016 0.013 0.062 0.036 0.017 0.046
+△S°(kJ/mol. K)
55.955 56.371 57.147 57.071 49.963 56.314 54.194
–△G°(kJ/mol )
L3 42.89 40.98 33.32 36.96 39.64 34.47 35.85
–△H°(kJ/mol )
0.043 0.051 0.08 0.067 0.034 0.073 0.064
+△
S°(kJ/mol. K)
58.179 59.878 57.932 56.561 54.258 58.001 55.724
–△G°(kJ/mol )
L4 33.70 40.98 38.49 36.77 35.24 27.77 31.41
–△H°(kJ/mol )
0.081 0.061 0.063 0.065 0.062 0.1 0.08
+△S°(kJ/mol. K)
38.012 38.292 38.284 33.4 30.499 32.473 24.518 –△G°(kJ /mol)
L5 32.94 29.68 55.53 41.94 28.34 36.19 20.68 –△H°(kJ /mol)
0.016 0.027 -0.06 –0.031 0.006 -0.014 0.012 +△S°(kJ /mol. K)
47.307 45.288 48.357 45.514 35.438 38.307 35.87
–△G°(kJ/mol )
L6 35.42 32.17 36.19 24.13 39.26 34.09 34.85 –△H°(kJ/mol )
0.04 0.044 0.041 0.072 -0.013 0.014 0.003
+△S°(kJ/mol. K)
spection of the titration curves shows the presence of
two distinctive breaks at metal to ligand molar rations of
1:1 and 1:2, respectively.
3.6. Spectrophotometric Determination of
the pKa Values of the Investigated
Ligands
The absorption spectra were recorded to investigate the
spectral properties of the species liable to exist in such
media and to determine the ionization constant (pKa)
values of the acidic groups present. Britton-Robinson
universal buffers [14] were used to control the pH over
the range 1.5-12.0. The maximum absorption of the li-
gand increases as pH of the buffer increase. The spectra
in acidic solutions of pH 1.5-6.0 are characterized by a
strong band absorbing maximally within the wavelength
range 370-400 nm. These bands are due to absorption of
the nonionized form liable to exist in such solutions and
may be assigned to π-π* electronic transition within the
ligand molecule influenced by intramolecular charge
transfer. The spectra in alkaline solutions are characte-
rized by the presence of a strong band absorbing max-
imally at the same range, which may be assigned to the
absorption of the ionized form liable to exist at high pH
values as a result of acid base equilibrium. It is noted in
this investigation that the absorption bands assigned to
the ionized form increases gradually by increasing of pH,
attaining the maximum value at pH 10-12.0. The absor-
bance-pH curves show that the absorbance attains a li-
miting value at the extreme pH values in highly acidic or
alkaline solutions indicating the existence of only one
ionization step which is the ionization of -OH or -COOH
groups. The variation of absorbance with pH is used for
the calculation of ionization constants (pKa values) of

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
801
801
the investigated ligands using the half height method
[20]. The results obtained are of the same order com-
pared to those obtained potentiometrically and are 3.98,
4.08, 7.67, 7.98, 3.40 and 3.64 for the investigated li-
gands (L1-L6), respectively. The ionization of strong
acidic carboxylic group is ionized at lower pH value, due
to the high stability of the corresponding anion by re-
sonance. They do not impart any spectral changes as the
ionizable proton is not conjugated with the π-system of
the molecule.
3.7. Determination of the Stoichiometry of
the Complexes Spectrophotometrically
The mole ratio of the metal ions to the ligands was stu-
died spectrophotometrically using molar ratio and con-
tinuous variation methods. The spectrophotometric me-
thod was used to confirm the data obtained by conduc-
timetric method. UV absorption spectra can be used to
determine stoichiometry of the complexes, and this me-
thod appears to be valuable for studying complexes with
low stabilities.
3.7.1. Molar Ratio Method
It was observed that the absorption increases linearly as
the ligand concentration increase, because of the forma-
tion of the complex until the solution reaches the actual
molar ratio of the investigated complex. At this point all
of the added materials were completely reacted and the
absorption observed is the absorption of the investigated
complex alone. After this point, the excess amount of the
added ligand causes an inflection in the straight line that
is because the ligand has an absorption value differ from
that of the complex at λmax of the complex [21]. [L]/[M]
ratio corresponding to the inflection point in (Abs-
[L]/[M] curve) indicates to the actual [L]/[M] ratio of the
investigated complex. It was found that all of the com-
plexes in this investigation are able to be stable in the
form ML2.
3.7.2. Continuous Variation Method
This method was used to confirm the data obtained using
molar ratio and conductivity methods. In this method,
The measured absorbance increases as the molar ratio
[M]/([L] + [M] ) increase until the actual molar ratio of
the complex is reached, after this point the absorbance
becomes lower because the metal has no absorption at
λmax of the complex. It was found that all of the curves
have inflection points at mole fraction around (0.33);
this means that all of the complexes in this investigation
have the form ML2 and this form is the most stable form.
4. CONCLUSIONS
The results obtained from the potentiometric measure-
ments for proton-ligand systems (pKa values) were 3.98,
4.04, 7.68, 7.93, 3.45 and 3.66 for the investigated li-
gands L1-L6, respectively. It is observed that as pKa of
the ligand increases, the stability constant logβ of the
complex increase. The stability constants of the metal
ions (VO2+, Cr3+, Mn2+, Co2+, Ni2+, Cu2+ and Zn2+) com-
plexes with the investigated ligands (L1-L6) were found
to decrease with increasing ionic strength of the medium
which was in agreement with the Debye-Hűckel equa-
tion. The thermodynamic stability constants (logβ°) were
obtained by extrapolating the straight line plots of logβ
versus √I to zero ionic strength. Also the values of pKa
were found to decrease with increasing ionic strength of
the medium. The values of the thermodynamic functions
∆G, ∆H and ∆S were calculated. The values of stability
constants reveal that the stability constants decrease with
increasing temperature, along with the pKa value. Con-
ductimetric measurements show the presence of two
distinctive breaks at metal to ligand molar rations of 1:1
and 1:2, respectively. The results obtained from the
spectrophotometric measurements are of the same order
compared to those obtained potentiometrically and are
3.98, 4.08, 7.67, 7.98, 3.40 and 3.64 for the investigated
ligands (L1-L6), respectively. The ionization of strong
acidic carboxylic group is ionized at lower pH value due
to the high stability of the corresponding anion by re-
sonance. They do not impart any spectral changes as the
ionizable proton is not conjugated with the π-system.
The mole ratio of the metal ions to the ligands was stu-
died spectrophotometrically using molar ratio and con-
tinuous variation methods. All the investigated com-
plexes are found to be stable in the form ML2, which is
also the most stable one.
REFERENCES
[1] Wisniewski, M.Z. (1997) Pd(II) complexes with some
derivatives of pyrazol-5-one. Polish Journal of Chemi-
stry, 71(2), 259-260.
[2] Lu, J., Zhang, L., Li u, L., Liu, G., Jia, D., Wu, D. and Xu,
G. (2008) Study of fluorescence properties of several
4-acyl pyrazolone derivatives and their Zn(II) complexes.
Spectrochimica Acta Part A, 71(3), 1036-1041.
[3] Kuncheria, B. and Indrasenan, P. (1988) Thorium (IV)
nitrate complexes with some substituted pyrazole-5-ones.
Indian Journal of Chemistry, 27A, 1005-1007.
[4] Vogel, I. (1978) A text book of practical organic chemi-
stry. 4th Edition, Longman, London.
[5] Tantawy, F., Goda, F. and Abdelal, A.M. (1995) Synthesis
and characterization of certain new 3-m et hy l-4 - (subs-
tituted phenylazo) isoxazol-5-ones and 3-methy l-4-
(substituted phenylazo)-pyra zol e-5-ones as potential an-
tibacterial agents. The Chinese Pharmaceutical Journal,
47(1), 37-45.

S. A. Abdel-Latif et al. / Natural Science 2 (2010) 793-802
802
[6] Rao, S. and Mittra, A.S. (1978) Synthesis and fungitoxic-
ity of 1-pheny l -3-methyl-4-mono arylidene-2-pyrazolin-
5-thione. Journal of the Indian Chemical Society, 55(7),
745-746.
[7] Fitton, A.O. and Smalley, R.K. (1968) Practical hetero-
cyclic chemistry. Academic Press, London and New
York.
[8] Karci, F. and Ertan, N. (2002) Hetarylazo disperse dyes
derived from 3-methy l-1-(3′, 5′-dipiperidino-s-triazinyl)-
5-pyrazolone as coupling component. Dyes and Pigments,
55(2-3), 99-108.
[9] Ibrahim, S.A., El-Gahami, M.A., Khafagi, Z. A. and
El-G yar, S.A. (1991) Structure and antimicrobial activity
of some new azopyrazolone chelates of Ni(II) and Cu(II)
acetates, sulfates and nitrates. Journal of Inorganic Bio-
chemistry, 43(1), 1-7.
[10] Abdel-Latif, S.A. (2001) Synthesis and characterization
of some 3-phenyl-4-arylazo-5-pyrazolones with La(III),
Ce(III), Th(IV) and UO2(VI) complexes. Synthesis and
Reactivity in Inorganic, Metal-O rganic and Chemistry,
31(8), 1355-1374.
[11] Abdel-Latif, S.A. and Hassib, H.B. (2002) Studies of
Mn(II), Co(II), Ni(II) and Cu(II) Chelates with 3-
Phenyl-4-(p-methoxyphenylazo)-5-pyrazolone . Journal of
Thermal Analysis and Calorimetry, 68(3), 983-995.
[12] Hassib, H.B. and Abdel-Latif, S.A. (2003) Potentiometric,
spectrometric thermal and conductimetric studies on
some 3-phenyl -4-(arylazo)-5-pyrazolones and their com-
plexes with divalent cobalt metal ion. Spectrochimica
Acta Part A, 59(11), 2425-2434.
[13] Sarin, R. and Munshi, K.N. (1972) Thermodynamics of
complex formation of indium metal ion with mercapto
hydroxyl and amino substituted succinic acid. Journal of
Inorganic and Nuclear Chem istry, 34(2), 581-590.
[14] Britton, H.T.S. (1952) Hydrogen ions. 2nd Edition,
Chapman and Hall, London.
[15] West, T.S. (1969) Complexemetry with EDTA and re-
lated reagents. 3rd Edition, B. D. H. Chemical Ltd Poole,
London.
[16] Vogel, I. (1956) Practical organic chemistry including
quantitative organic analysis. 3rd Edition, Longmans,
London.
[17] Irving, H. and Rossotti, H.S. (1953) Methods for compu-
ting successive stability constants from experimental
formation curves. Journal of Chemical Society, 3397-
3405.
[18] Zumdal, S.S. (1997) Chemistry. 4th Edition, Houghton
Mifflin Company.
[19] Vogel, A.I. (1956) Practical organic chemistry including
quantitative organic analysis. 3rd Edition, Longmans,
London.
[20] Issa, Y. M., Abdel -Ghani, N.T. and Abdel-Lati f, S.A.
(1996) Spectrophotometric studies on some hydroxy ni-
trocoumarins. Egyptian Journal of Chemistry, 39(3),
235-248.
[21] Bauer, H.H., Christian, G.D. and O’Reilly, J.E. (1978)
Instrumental analysi s. Allyn and Bacon Inc.
[22] McMurry, J. (2000) Organic chemistry. 5th Edition,
Brooks/Cole, USA.
[23] Schwarzenbach, G. and Flaschk, H. (1969) Complex-
ometric titrations. Methuen and Co. Ltd., London.
[24] Moeller, T., Martin, D.F., Thompson, L.C., Ferrus, R.,
Feistel, G.R. and Randal, W.J. (1965) The coordination
chemistry of yttrium and the rare earth metal ions.
Chemical Reviews, 65(1), 1-50.
[25] Gutmann, K. (1966) Conductimetric titration of charge
transfer complexes in solution. Electrochimica Acta,
11(6), 555-568.
[26] Singh, P. and Ojha, A.C. (1980) Synthesis of N-isoni-
ctinyl-3-methyl -4-(substituted-hydrazono)-2-py razolin-5-
one. Journal of the Indian Chemical Socie ty, 57(12),
1172-1173.
[27] Snavely, F. A. and Krecker, B. D. (1959) Metal derivatives
of arylazopyrazolone dyes III, molarity quotients of para-
and meta-substituted pyrazolone dyes. Journal of the
American Chemical Soc iety, 81(16), 4199-4200.
[28] Willia m, W.P. (1993) Inorganic chemistry. 2nd Edition,
Academic Press Inc.
[29] Manku, G.S., Gupta, R.D., Bhat, A.N. and Jain, B.D.
(1968) Determination of the stability constants of some
lanthanon complexes with 1-nitroso-2-naphthol, 2-ni-
troso-1-naphthol, and 3-nitroso-4-hydroxycoumarin. Jour-
nal of the Less Common Metals, 16(4), 343-350.
[30] Debye, P. and Hückel, E. (1923) Physik. Z., 24, 185-206.
[31] Saxena, R.S. and Gupta, A. (1984) Potentiometric deter-
mination of dissociation constants of dithiodipropionic
acid in different media. Journal of the Electrochemical
Society India, 33(1), 89-90.
[32] Yadav, S.S. and Saxena, R.S. (2009) Formation constants
and thermodynamic parameters of some tri-ligand com-
plexes of lanthanides. Journal of the Indian Chemical
Society, 86(2), 168-170.