American Journal of Industrial and Business Management, 2012, 2, 166-175
http://dx.doi.org/10.4236/ajibm.2012.24022 Published Online October 2012 (http://www.SciRP.org/journal/ajibm)
Development of Economic Models for EOL Service Parts
Control
Wan Seon Shin1, Han Sang Lee2, Heungsoon Felix Lee3
1System Management Engineering, Sungkyunkwan University, Suwon, South Korea; 2Energy Solution, LG Chem, Ochang, South
Korea; 3Industrial & Manufacturing Engineering, Southern Illinois University Edwardsville, Illinois, USA.
Received May 30th, 2012; revised June 29th, 2012; accepted July 27th, 2012
ABSTRACT
This paper deals with a decision problem for final purchase quantity needed for the appropriate support of service parts
for S Electronics which is one of the largest electronics companies in the world. The cost elements of the final purchase
quantity, the economic models, the optimal solution methods, and the expected effect are presented.
Keywords: Spare Parts; Final Purchase; Economic Model
1. Introduction
S Electronics has business around the world. Procure-
ment of service parts about a variety of products for sale
is an important competitive factor [1]. The company
views the effective order management of electronic
products after services as the key factor to customer sat-
isfaction and cost saving. Annual service parts inventory
procurement costs over $100 million domestically alone.
It is therefore clear that controlling service part invento-
ries is of great importance in reducing costs and enhanc-
ing service competitiveness.
[2] divides the service part life cycle into three differ-
ent phases: Initial, normal, and final phases.
1) The initial phase: New components and sub-as-
semblies are being introduced. Very little is known about
their failure behavior and demand [3].
2) The normal phase: Some experiences have been
gained for parts in use longer than the initial phase. Often
times, a demand forecast model is used for production
planning.
3) The final phase: Production of the product has
stopped, but the service period however continues. Service
managers often place a final production order at the begin-
ning of this phase to deal with the on-going services [4,5].
One important issue in service parts supply for S Elec-
tronics is to determine the order quantity of service parts
in the final phase (which we call the end of life phase,
EOL phase), which is the focus of this paper. [5] term
this as the final order. In this paper, we call it “Lastbuy”
(denote as LTB) according to the actual field terminol-
ogy at S Electronics.
Apparently, the control of service parts is a complex
matter. Common statistical models for inventory control
lose their applicability, because the demand process is
different from those assumed. An essential element in
many models such as forecasting demand requires some
historical demand figures which are unavailable or in-
valid for slow moving parts. Moreover, the shorter life
cycles of products and better product quality further
reduce the possibility of collecting historical demand
figures for service parts. In this paper, we present eco-
nomic models more reflected by characteristics of parts
and management’s polices and knowledge from statis-
tical models.
This study addresses a method that determines the
economic Lastbuy quantity. There are three specific re-
search topics. The first topic is to identify the cost ele-
ments related to the last buy and develop the economic
model. The second topic is to develop a solution method
to determine the optimal Lastbuy quantity. The last topic
is to analyze the effects of the proposed economic model
and the optimal Lastbuy quantity.
2. Literature Review
In the service area, service parts management is one of
the most important topics. Its main element is the control
of the inventories of service parts. All over the world
billions of dollars have been invested in these inventories.
Due to the uncertainty in demand both in time and loca-
tion, however, there can be stock-outs causing many
emergency transports and even production losses. On the
other hand, stocks regularly need to be discarded: Typi-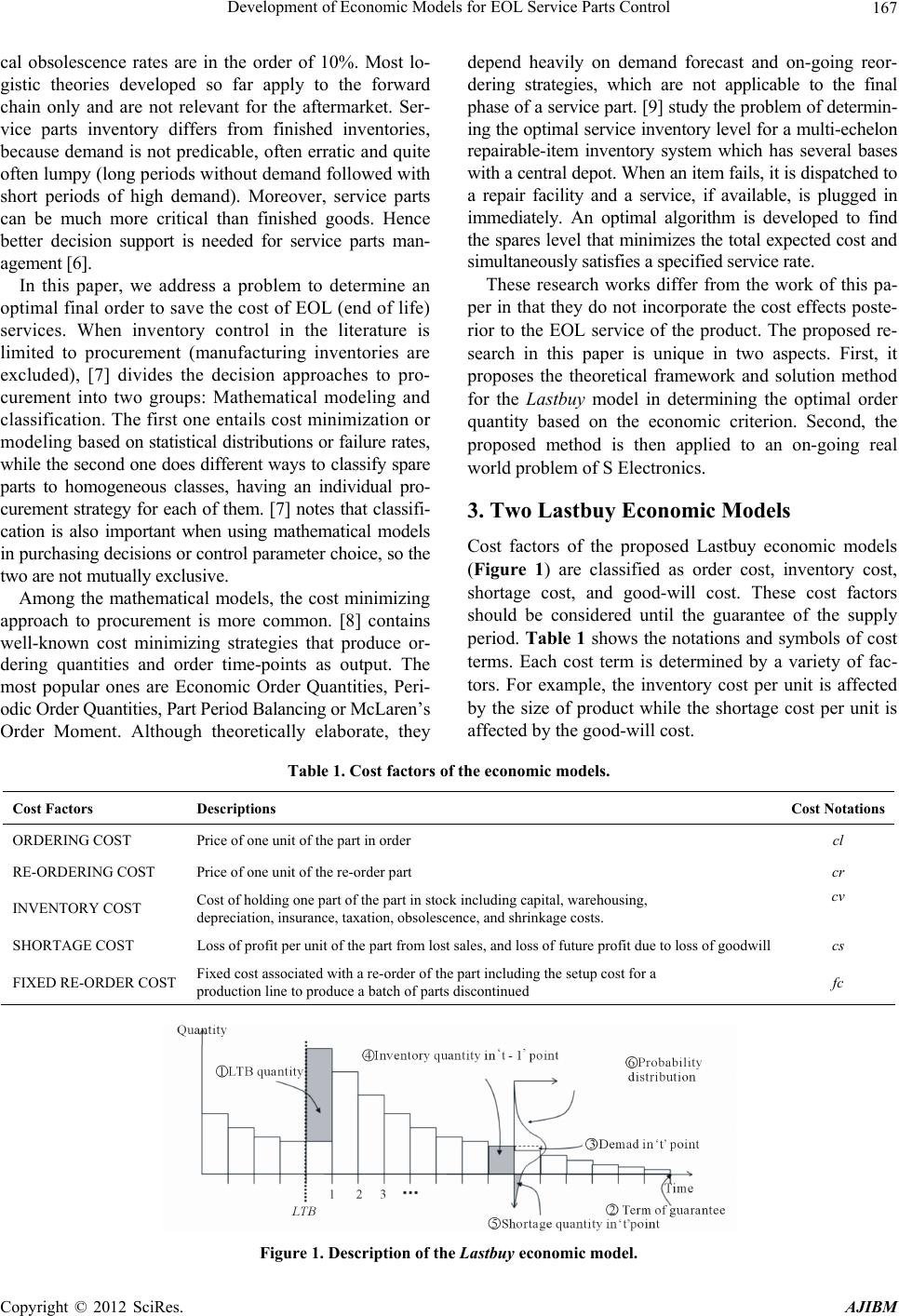

Development of Economic Models for EOL Service Parts Control 167
cal obsolescence rates are in the order of 10%. Most lo-
gistic theories developed so far apply to the forward
chain only and are not relevant for the aftermarket. Ser-
vice parts inventory differs from finished inventories,
because demand is not predicable, often erratic and quite
often lumpy (long periods without demand followed with
short periods of high demand). Moreover, service parts
can be much more critical than finished goods. Hence
better decision support is needed for service parts man-
agement [6].
In this paper, we address a problem to determine an
optimal final order to save the cost of EOL (end of life)
services. When inventory control in the literature is
limited to procurement (manufacturing inventories are
excluded), [7] divides the decision approaches to pro-
curement into two groups: Mathematical modeling and
classification. The first one entails cost minimization or
modeling based on statistical distributions or failure rates,
while the second one does different ways to classify spare
parts to homogeneous classes, having an individual pro-
curement strategy for each of them. [7] notes that classifi-
cation is also important when using mathematical models
in purchasing decisions or control parameter choice, so the
two are not mutually exclusive.
Among the mathematical models, the cost minimizing
approach to procurement is more common. [8] contains
well-known cost minimizing strategies that produce or-
dering quantities and order time-points as output. The
most popular ones are Economic Order Quantities, Peri-
odic Order Quantities, Part Period Balancing or McLaren’s
Order Moment. Although theoretically elaborate, they
depend heavily on demand forecast and on-going reor-
dering strategies, which are not applicable to the final
phase of a service part. [9] study the problem of determin-
ing the optimal service inventory level for a multi-echelon
repairable-item inventory system which has several bases
with a central depot. When an item fails, it is dispatched to
a repair facility and a service, if available, is plugged in
immediately. An optimal algorithm is developed to find
the spares level that minimizes the total expected cost and
simultaneously satisfies a specified service rate.
These research works differ from the work of this pa-
per in that they do not incorporate the cost effects poste-
rior to the EOL service of the product. The proposed re-
search in this paper is unique in two aspects. First, it
proposes the theoretical framework and solution method
for the Lastbuy model in determining the optimal order
quantity based on the economic criterion. Second, the
proposed method is then applied to an on-going real
world problem of S Electronics.
3. Two Lastbuy Economic Models
Cost factors of the proposed Lastbuy economic models
(Figure 1) are classified as order cost, inventory cost,
shortage cost, and good-will cost. These cost factors
should be considered until the guarantee of the supply
period. Tabl e 1 shows the notations and symbols of cost
terms. Each cost term is determined by a variety of fac-
tors. For example, the inventory cost per unit is affected
by the size of product while the shortage cost per unit is
affected by the good-will cost.
Table 1. Cost factors of the economic models.
Cost Factors Descriptions Cost Notations
ORDERING COST Price of one unit of the part in order cl
RE-ORDERING COST Price of one unit of the re-order part cr
INVENTORY COST Cost of holding one part of the part in stock including capital, warehousing,
depreciation, insurance, taxation, obsolescence, and shrinkage costs.
cv
SHORTAGE COST Loss of profit per unit of the part from lost sales, and loss of future profit due to loss of goodwill cs
FIXED RE-ORDER COST Fixed cost associated with a re-order of the part including the setup cost for a
production line to produce a batch of parts discontinued fc
Figure 1. Description of the Lastbuy economic model.

Development of Economic Models for EOL Service Parts Control 168
Definitions of the economic model terms are given in
Table 2 Part demand quantity during period t is based on
part reliability and a number of parts currently being in
use by customers. We assume that this part demand D(t)
at t follows a Poisson distribution with mean μD(t), which
is commonly used in reliability engineering [10]. Since
parts are reliable and the number of parts in use is large,
we approximate the Poisson distribution with a normal
distribution with mean μD(t) and variance

2
D
t
 . Note
that the variance value equals the mean value for a Pois-
son distribution.
However, this normal distribution requires some
modification since part demand can not take on a nega-
tive value. The modified normal distribution is given as
follows:






2
1
2
Pr
1
10
2π
00
Dt
Dt
Dt
y
Dt
Dt F
e























This distribution takes on a positive discrete probabil-
ity that D(t) = 0, i.e., that no part fails in “t” point. Since
the Poisson distribution cannot take on a negative num-
ber, this modified normal distribution better approxi-
mates the Poisson distribution.
There are three assumptions for developing economic
models for the Lastbuy problem. First, a product (assem-
bly) consists of several (components) parts and the
probability that multiple parts in a product fail in the
same t point is negligible. Second, any part shortage is
not recovered in the later period (i.e., no backlogging is
allowed). Third, the Lastbuy decision is made at the be-
ginning of each period and the order is supplied within
the given period. This allows us to eliminate a lead time
factor. It is told by the field managers that these assump-
tions are close to real situations at S Electronics, where
relatively high quality products in the market are sup-
ported through an efficient supply chain management
system.
3.1. The Simple Economic Model
The total cost of the economic model is divided into
three parts: Ordering cost, inventory cost, and shortage
cost. The total cost TC(x) is computed by the following
formula:


  



 




10
1
d
d
ht
UTL
t
UTL
tht
TC xcl x
cvh tDtfD tD
csDthtfD tD






 








 








where
 
  
0
1max, 00
htx fort
ht ht Dtfort






We call this formula as the simple order economic
model. Note that there is no setup cost in this model. This
is because the production line is still up running at this
point of Lastbuy order. A nonnegative h(t) is used in
calculating the expected shortage cost due to the assump-
tion of no backlogging policy.
3.2. The Re-Order Economic Model
There are also occasions when the company is allowed to
place one additional order (i.e., one time re-order). The
company desires to know the amount of savings that
Table 2. Definitions of the economic model terminologies.
Variables Descriptions Notations
Lastbuy quantity LTB order quantity of a part under consideration X
Re-order quantity One time re-order quantity after Lastbuy, when allowed. Y
Re-order point The period when the re-order is issued. z
Term of guarantee Mandatory supply period of the part specified by law (or private law) UTL
Demand in “t” point Demand for the part in time “t” point D(t)
Inventory quantity in “t” point Inventory quantity of the part in “t” point. (h(0) is the inventory
on hand at the beginning before Lastbuy order.) h(t)
Shortage quantity in “t” point Expected number of the part of shortage in time “t” point
    

d
ht
D
thtfDtD



Demand probability distribution
D(t) follows a variant of normal distribution
 


2
,
Dt Dt
N  ,
where
 
2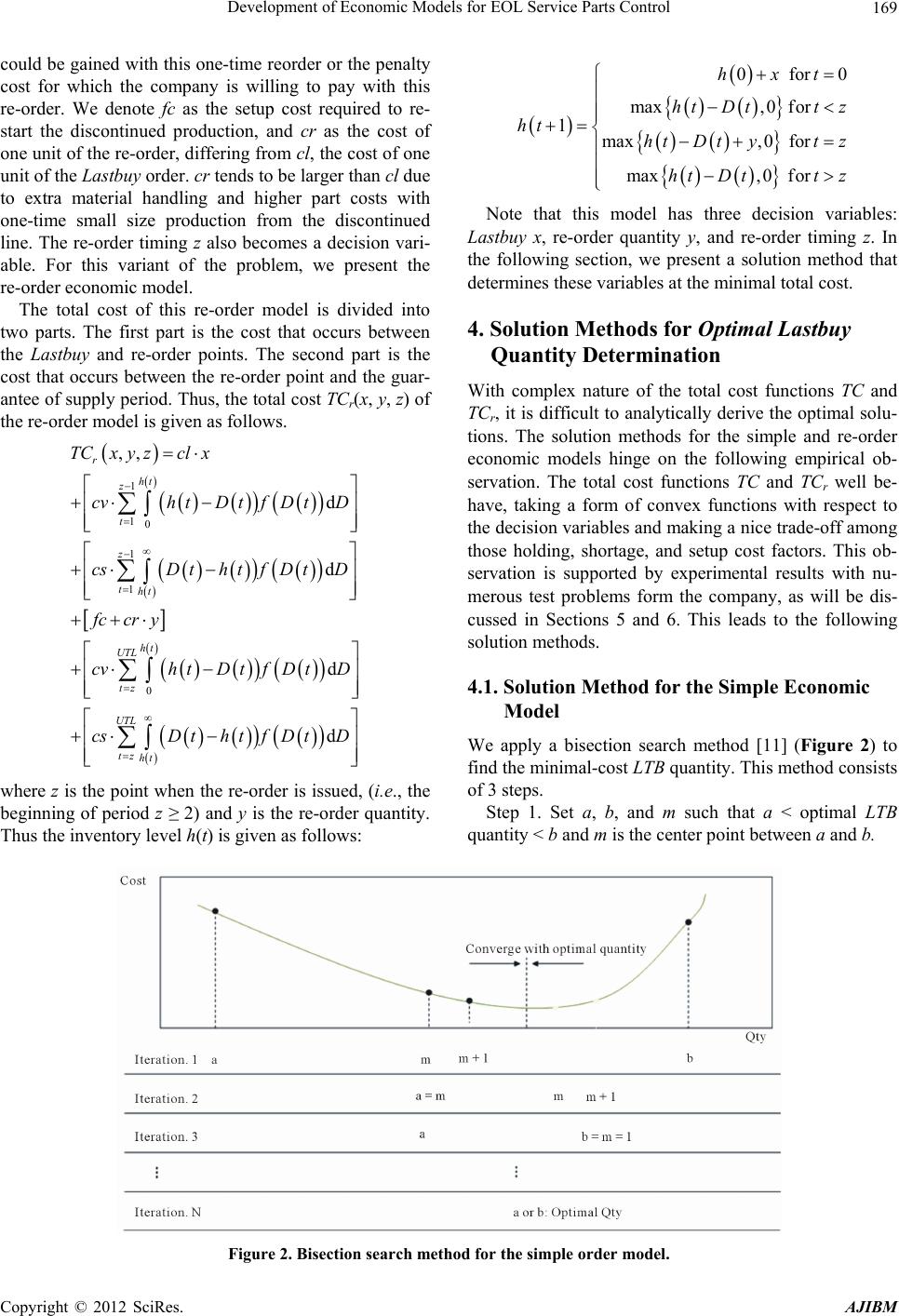
D
tDt

 .
f(D(t))

Development of Economic Models for EOL Service Parts Control 169
could be gained with this one-time reorder or the penalty
cost for which the company is willing to pay with this
re-order. We denote fc as the setup cost required to re-
start the discontinued production, and cr as the cost of
one unit of the re-order, differing from cl, the cost of one
unit of the Lastbuy order. cr tends to be larger than cl due
to extra material handling and higher part costs with
one-time small size production from the discontinued
line. The re-order timing z also becomes a decision vari-
able. For this variant of the problem, we present the
re-order economic model.
The total cost of this re-order model is divided into
two parts. The first part is the cost that occurs between
the Lastbuy and re-order points. The second part is the
cost that occurs between the re-order point and the guar-
antee of supply period. Thus, the total cost TCr(x, y, z) of
the re-order model is given as follows.

  

 
  

 

  

 
  

 
1
10
1
1
0
,,
d
d
d
d
r
ht
z
t
z
tht
ht
UTL
tz
UTL
tz
ht
TCx y zclx
cvhtD tfDtD
csD thtfD tD
fccry
cvh tD tfDtD
csDthtfD tD










 




 





 




 




















where z is the point when the re-order is issued, (i.e., the
beginning of period z ≥ 2) and y is the re-order quantity.
Thus the inventory level h(t) is given as follows:



  
  
 

0for
max,0for
1max,0 for
max,0 for
hxt
htDtt z
ht htDtyt z
htDtt z
 




 




0
Note that this model has three decision variables:
Lastbuy x, re-order quantity y, and re-order timing z. In
the following section, we present a solution method that
determines these variables at the minimal total cost.
4. Solution Methods for Optimal Lastbuy
Quantity Determination
With complex nature of the total cost functions TC and
TCr, it is difficult to analytically derive the optimal solu-
tions. The solution methods for the simple and re-order
economic models hinge on the following empirical ob-
servation. The total cost functions TC and TCr well be-
have, taking a form of convex functions with respect to
the decision variables and making a nice trade-off among
those holding, shortage, and setup cost factors. This ob-
servation is supported by experimental results with nu-
merous test problems form the company, as will be dis-
cussed in Sections 5 and 6. This leads to the following
solution methods.
4.1. Solution Method for the Simple Economic
Model
We apply a bisection search method [11] (Figure 2) to
find the minimal-cost LTB quantity. This method consists
of 3 steps.
Step 1. Set a, b, and m such that a < optimal LTB
quantity < b and m is the center point between a and b.
Figure 2. Bisection search method for the simple order model.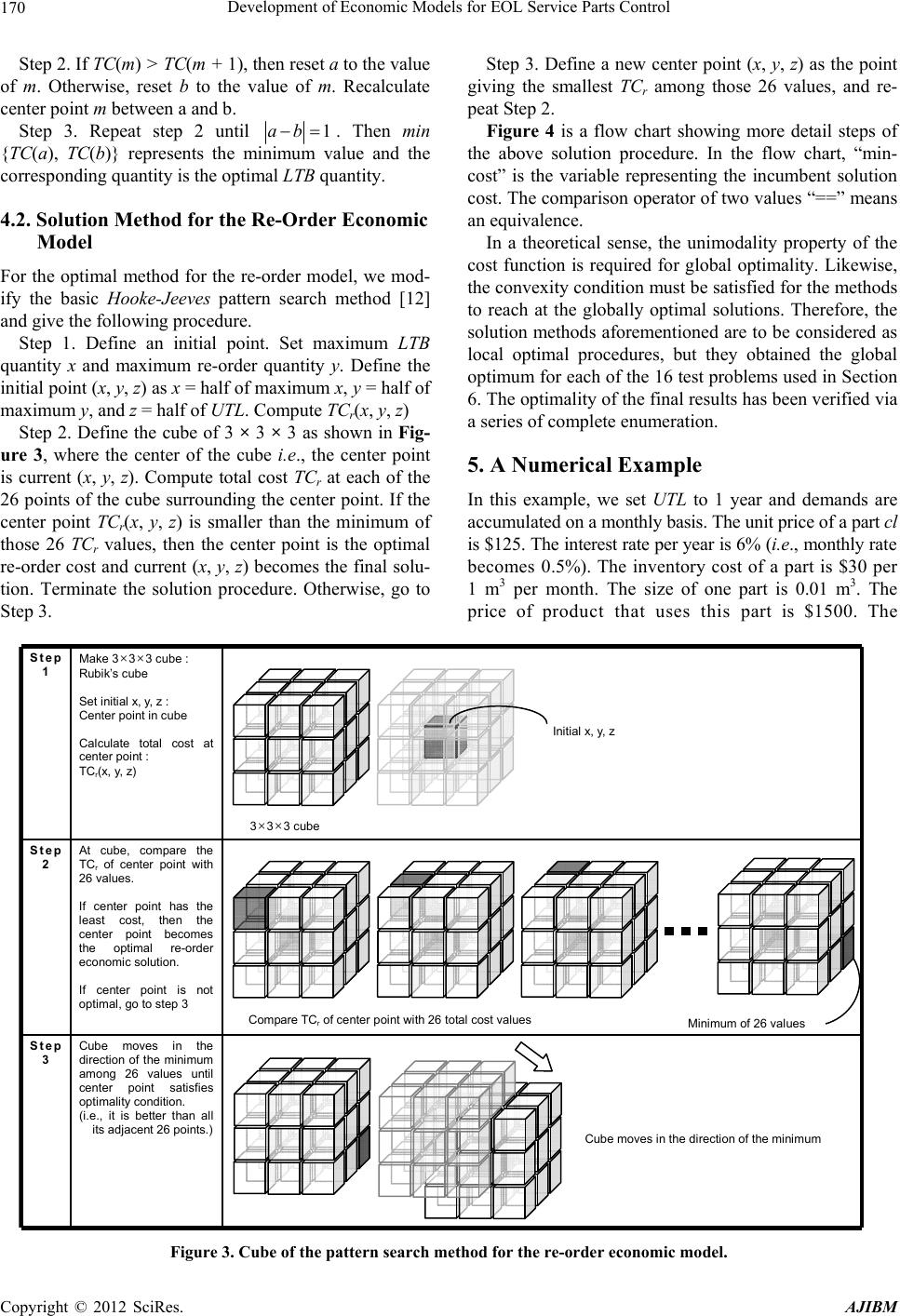

Development of Economic Models for EOL Service Parts Control 170
Step 2. If TC(m) > TC(m + 1), then reset a to the value
of m. Otherwise, reset b to the value of m. Recalculate
center point m between a and b.
Step 3. Repeat step 2 until 1ab. Then min
{TC(a), TC(b)} represents the minimum value and the
corresponding quantity is the optimal LTB quantity.
4.2. Solution Method for the Re-Order Economic
Model
For the optimal method for the re-order model, we mod-
ify the basic Hooke-Jeeves pattern search method [12]
and give the following procedure.
Step 1. Define an initial point. Set maximum LTB
quantity x and maximum re-order quantity y. Define the
initial point (x, y, z) as x = half of maximum x, y = half of
maximum y, and z = half of UTL. Compute TCr(x, y, z)
Step 2. Define the cube of 3 × 3 × 3 as shown in Fig-
ure 3, where the center of the cube i.e., the center point
is current (x, y, z). Compute total cost TCr at each of the
26 points of the cube surrounding the center point. If the
center point TCr(x, y, z) is smaller than the minimum of
those 26 TCr values, then the center point is the optimal
re-order cost and current (x, y, z) becomes the final solu-
tion. Terminate the solution procedure. Otherwise, go to
Step 3.
Step 3. Define a new center point (x, y, z) as the point
giving the smallest TCr among those 26 values, and re-
peat Step 2.
Figure 4 is a flow chart showing more detail steps of
the above solution procedure. In the flow chart, “min-
cost” is the variable representing the incumbent solution
cost. The comparison operator of two values “==” means
an equivalence.
In a theoretical sense, the unimodality property of the
cost function is required for global optimality. Likewise,
the convexity condition must be satisfied for the methods
to reach at the globally optimal solutions. Therefore, the
solution methods aforementioned are to be considered as
local optimal procedures, but they obtained the global
optimum for each of the 16 test problems used in Section
6. The optimality of the final results has been verified via
a series of complete enumeration.
5. A Numerical Example
In this example, we set UTL to 1 year and demands are
accumulated on a monthly basis. The unit price of a part cl
is $125. The interest rate per year is 6% (i.e., monthly rate
becomes 0.5%). The inventory cost of a part is $30 per
1 m3 per month. The size of one part is 0.01 m3. The
price of product that uses this part is $1500. The
Step
1 Make 3×3×3 cube :
Rubik’s cube
Set initial x, y, z :
Center point in cube
Calculate total cost at
center point :
TC
r
(x, y, z)
Step
2 At cube, compare the
TC
r
of center point with
26 values.
If center point has the
least cost, then the
center point becomes
the optimal re-order
economic solution.
If center point is not
optimal, go to step 3
Step
3 Cube moves in the
direction of the minimum
among 26 values until
center point satisfies
optimality condition.
(i.e., it is better than all
its adjacent 26 points.)
3×3×3 cube
Initial x, y, z
Compare TC
r
of center point with 26 total cost values
Minimum of 26 values
Cube moves in the direction of the minimum
Figure 3. Cube of the pattern search method for the re-order economic model.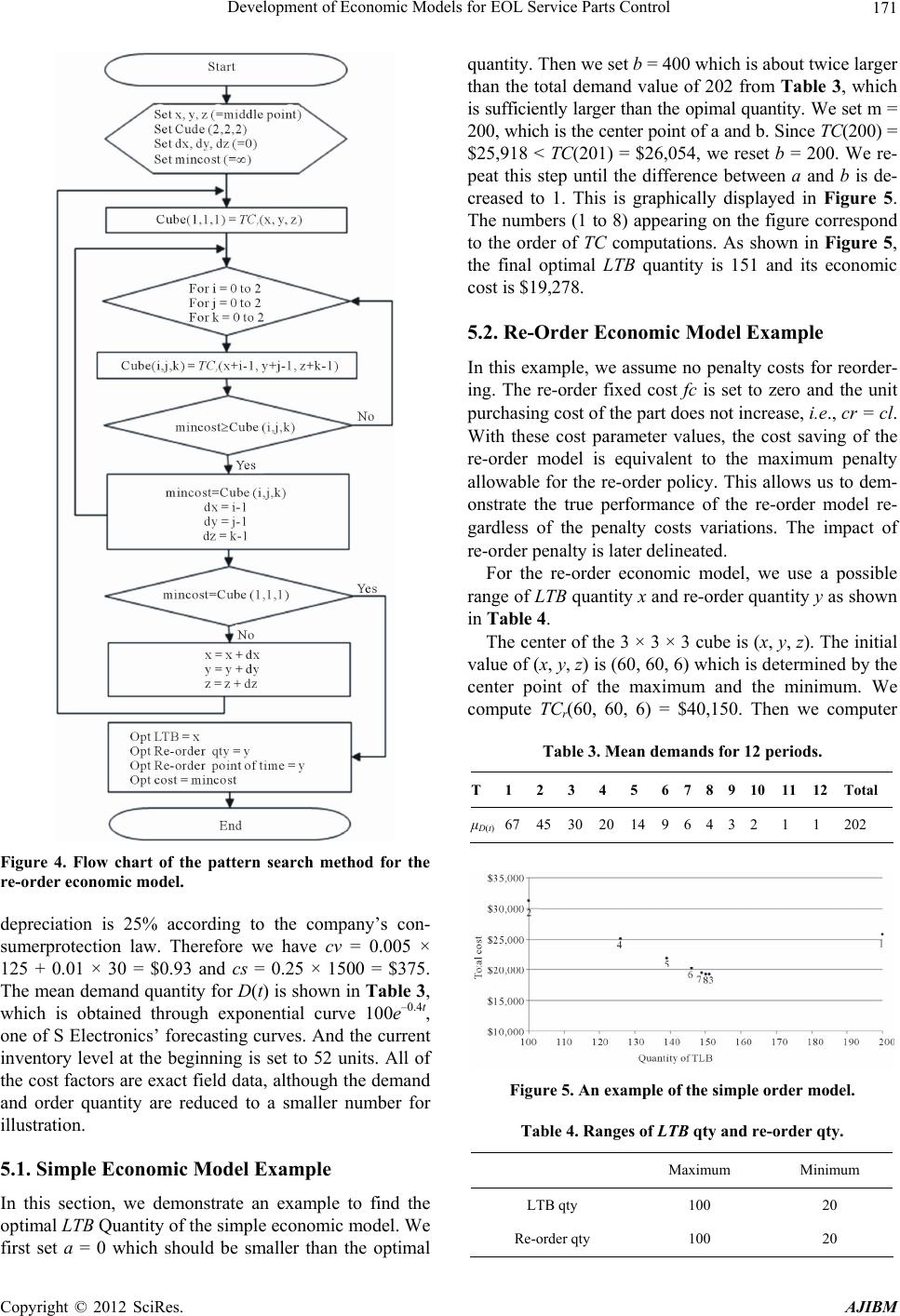

Development of Economic Models for EOL Service Parts Control 171
Figure 4. Flow chart of the pattern search method for the
re-order eco nomic model.
depreciation is 25% according to the company’s con-
sumerprotection law. Therefore we have cv = 0.005 ×
125 + 0.01 × 30 = $0.93 and cs = 0.25 × 1500 = $375.
The mean demand quantity for D(t) is shown in Ta ble 3 ,
which is obtained through exponential curve 100e–0.4t,
one of S Electronics’ forecasting curves. And the current
inventory level at the beginning is set to 52 units. All of
the cost factors are exact field data, although the demand
and order quantity are reduced to a smaller number for
illustration.
5.1. Simple Economic Model Example
In this section, we demonstrate an example to find the
optimal LTB Quantity of the simple economic model. We
first set a = 0 which should be smaller than the optimal
quantity. Then we set b = 400 which is about twice larger
than the total demand value of 202 from Table 3, which
is sufficiently larger than the opimal quantity. We set m =
200, which is the center point of a and b. Since TC(200) =
$25,918 < TC(201) = $26,054, we reset b = 200. We re-
peat this step until the difference between a and b is de-
creased to 1. This is graphically displayed in Figure 5.
The numbers (1 to 8) appearing on the figure correspond
to the order of TC computations. As shown in Figure 5,
the final optimal LTB quantity is 151 and its economic
cost is $19,278.
5.2. Re-Order Economic Model Example
In this example, we assume no penalty costs for reorder-
ing. The re-order fixed cost fc is set to zero and the unit
purchasing cost of the part does not increase, i.e., cr = cl.
With these cost parameter values, the cost saving of the
re-order model is equivalent to the maximum penalty
allowable for the re-order policy. This allows us to dem-
onstrate the true performance of the re-order model re-
gardless of the penalty costs variations. The impact of
re-order penalty is later delineated.
For the re-order economic model, we use a possible
range of LTB quantity x and re-order quantity y as shown
in Table 4.
The center of the 3 × 3 × 3 cube is (x, y, z). The initial
value of (x, y, z) is (60, 60, 6) which is determined by the
center point of the maximum and the minimum. We
compute TCr(60, 60, 6) = $40,150. Then we computer
Table 3. Mean demands for 12 periods.
T 1 2 3 4 5 67 8 9 10 11 12Total
μD(t)674530201496 4 3 2 1 1 202
Figure 5. An example of the simple order model.
Table 4. Ranges of LTB qty and re-order qty.
Maximum Minimum
LTB qty 100 20
Re-order qty 100 20

Development of Economic Models for EOL Service Parts Control 172
the other 26 total costs in the cube: TCr(59, 59, 5),
TCr(59, 59, 6), TCr(59, 59, 7), TCr(59, 60, 5), TCr(59, 60,
6), TCr(59, 60, 7), TCr(59, 61, 5), TCr(59, 61, 6), TCr(59,
61, 7), TCr(60, 59, 5), TCr(60, 59, 6), TCr(60, 59, 7),
TCr(60, 60, 5), TCr(60, 60, 7), TCr(60, 61, 5), TCr(60, 61,
6), TCr(60, 61, 7), TCr(61, 59, 5), TCr(61, 59, 6), TCr(61,
59, 7), TCr(61, 60, 5), TCr(61, 60, 6), TCr(61, 60, 7),
TCr(61, 61, 5), TCr(61, 61, 6), TCr(61, 61, 7).
TCr(61, 59, 5) = $34,312 is the smallest cost among
these 26 costs. Since TCr(60, 60, 6) > TCr(61, 59, 5), the
cube moves in the direction of (+1, –1, –1). We have a
new (x, y, z), which is (61, 59, 5). We repeat this process
until TCr(x, y, z) is smaller than all the 26 costs in the
cube. In this example, the cube moves 17 times. This is
graphically displayed in Figure 6. The optimal solution
found is (x, y, z) = (77, 74, 3) with total cost $19,145.
According to this solution, an order of 77 units is made at
the beginning of period 1 and a re-order of 74 units is
made at the beginning of period 3.
The cost saving of this re-order model over the simple
model is $133 (i.e., 19,278 – 19,145 = 133), which can
be interpreted as the maximum penalty cost allowable for
the re-order policy. With the assumption of no part cost
increase, the fixed cost is allowed as large as $133. Or
the penalty may be imbedded in the increased part pur-
chase price for the re-order parts. Figure 7 shows the
cost impact of re-oder decisions when the part price in-
creases. As the re-order part price increases, the optimal
re-order quantity decreases toward zero.
Figure 6. A numerical example of the re-order economic model.
Figure 7. The cost impact of price increments of the re-order part.

Development of Economic Models for EOL Service Parts Control 173
6. The Effects of the Economic Models
We tested the proposed economic models by a set of
computational studies using the real company data sets.
The outcomes of the proposed methods were compared
with those of the current practice by the company, which
is to determine the Lastbuy quantity by summing the
forecasted demands minus the initial inventory level on
hand with no re-order.
For the validation purpose, we tested 16 parts of 8 dif-
ferent products manufactured by the company using its
past records. We applied our proposed methods and the
company practice to these 16 parts and Table 5 presents
a summary of the comparison between the two outcomes.
Columns A and B of Tab le 5 represent the relative Last-
buy quantity difference and the relative total cost differ-
ence for the simple model, respectively. Co- lumns C and
D are the corresponding values for the re-order model.
Column E shows the maximum ratio of part price incre-
ment after re-order. This can be served as an indication
of the economic (or penalty) impact of the re-order
model.
The total cost saving of the proposed method with the
simple economic model is 0.57% on the average with
Table 5. A performance study of the proposed economic models.
A: 
company L as tbuy qt y - si mple econo mic model qty100
company Lastbuy qty B: 
company Lastbuy c ost - simple econ omic mode l cost100
company Lastbuy cost
C: 
company L astb uy qty -re ord er eco nomic mode l qty100
company Lastbuy qty D: 
company Last buy cost - r eorder economic model cost100
company Lastbuy cost
E: 

company L ast buy cos t - r eo rde r econo mic model c ost100
re-order qty price of part
Simple economic model Re-order economic model
No. Part description A B C D E
1 HHP WINDO-009 –0.08% 2.34% –0.09% 6.90% 30.79%
2 HHP CAMERA-001 –0.02% 0.21% –0.02% 0.70% 12.07%
3 HHP CAMERA-031 –0.27% 0.27% –0.27% 1.21% 12.02%
4 HHP UPPER-017 –0.12% 0.49% –0.11% 2.40% 9.26%
5 AUDIO DECK-001 –0.24% 0.06% –0.24% 2.63% 10.27%
6 AUDIO DECK-018 –0.72% 0.40% –0.72% 9.12% 33.34%
7 WASHINGMACHINE PBA-004 –0.58% 2.55% –0.67% 9.54% 35.25%
8 WASHINGMACHINE PBA-014 –0.32% 0.94% –0.39% 5.88% 106.97%
9 DVD PBA-003 –0.36% 0.56% –0.36% 1.59% 4.74%
10 DVD DECK-007 –0.93% 0.61% –0.93% 2.19% 3.23%
11 MP3PLAYER PBA-014 –0.01% 0.00% –0.01% 1.39% 5.62%
12 MP3PLAYER PBA-030 –0.09% 0.00% 0.00% 0.33% 1.49%
13 NOTE PC BOARD-007 –0.26% 0.09% –0.26% 2.40% 9.72%
14 NOTE PC BOARD-010 –0.07% 0.01% –0.13% 3.42% 13.36%
15 LCD MONITER MAIN-058 –0.66% 0.50% –0.81% 4.80% 15.79%
16 PDP TV MODULE-001 –1.27% 0.07% –1.27% 2.84% 7.02%
Average –0.38% 0.57% –0.39% 3.58% 19.43%

Development of Economic Models for EOL Service Parts Control 174
$0
$100
$200
$300
$400
$500
$600
0%10% 20% 50%100%150%200%
Figure 8. Cost saving as the rate of standard deviation in-
creases.
$0
$50
$100
$150
$200
$250
$300
0%10%20%50%100% 150% 200%
Figure 9. Cost saving cost as the rate of shortage cost in-
creases.
max 2.55% (see column B), whereas that of the re-order
model reaches average 3.58% with max 9.54% (see
column D). It is also observed from column E that the
re-order model solution may allow a penalty of average
19.43% with max 106.97% in the part price increase
when compared to the simple model solution. This shows
a great potential saving that the re-order policy could
bring for the service part management.
In order to further investigate the effectiveness of the
proposed models and solution methods, we conducted a
sensitivity study on two key input parameters: standard
deviation of the demand and shortage cost factor. Fig-
ures 8 and 9 depict the results from the numerical exam-
ple of the simple economic model presented in Section 5.
They show that the proposed method brings more sig-
nificant cost savings as the standard deviation and short-
age cost increase. The similar results are observed for
other parts tested. This means that the effectiveness in-
creases as demand uncertainty and customer expectation
toward products increase. As demand stand deviation
increases, the expected number of shortage increases,
causing the current company practice to experience
higher shortage costs.
7. Conclusion
In this paper, we presented the economic order quantity
models and solution methods to determine the final pur-
chase quantity necessary to support service parts for S
Electronics, one of the largest electronics company in the
world. Our experimental results with the 16 company
data sets show that the proposed methods save the cost
over the company practice by average 0.57% and 3.58%
(max 2.55% and 9.54%) for the simple and re-order
models, respectively. This translates into annual cost
saving of about $0.6 million dollars even in Korea alone.
Considering S Electronics’ world wide business scope
and over 20,000 different parts types per year, the value
of the proposed methods could lead to substantial saving.
The sensitivity study also shows that the saving will in-
crease furthermore as the demand uncertainty and cus-
tomer expectation toward products increase. With these
findings, the company recently adopted the proposed
methods for real usage in its operation.
REFERENCES
[1] L. Fortuin and H. Martin, “Control of Service Parts,”
International Journal of Operations & Production Man-
agement, Vol. 19, No. 9, 1999, pp. 950-971.
doi:10.1108/01443579910280287
[2] L. Fortuin, “The All-Time Requirement of Spare Parts for
Service after Sales: Theoretical Analysis and Practical
Results,” International Journal of Operations & Produc-
tion Management, Vol. 1, No. 1, 1980, pp. 59-70.
doi:10.1108/eb054660
[3] L. Fortuin, “Reduction of the All-Time Requirement for
Spare Parts,” International Journal of Operations &
Production Management, Vol. 2, No. 1, 1981, pp. 29-37.
doi:10.1108/eb054673
[4] L. Fortuin, “Initial Supply and Re-Order Level of New
Service Parts,” European Journal of Operational Re-
search, Vol. 15, No. 3, 1984, pp. 310-319.
doi:10.1016/0377-2217(84)90098-5
[5] R. H. Teunter and L. Fortuin, “End-Of-Life Service,” In-
ternational Journal of Production Economics, Vol. 59,
No. 1-3, 1999, pp. 487-497.
doi:10.1016/S0925-5273(98)00112-1
[6] W. J. Kennedy, J. Patterson, J. Wayne and D. Lawrence,
“An Overview of Recent Literature on Spare Parts
Inventories,” International Journal of Production Eco-
nomics, Vol. 76, No. 2, 2002, pp. 201-215.
doi:10.1016/S0925-5273(01)00174-8
[7] J. Huiskonen, “Maintenance Spare Parts Logistics: Spe-
cial Characteristics and Strategic Choices,” Interna-
tional Journal of Production Economics, Vol. 71, No. 1-3,
2001, pp. 25-133. doi:10.1016/S0925-5273(00)00112-2
[8] T. E. Vollmann, W. L. Berry and D. C. Whybark, “Manu-
facturing, Planning and Control Systems,” 4th Edition,
McGraw-Hill Companies Inc., New York, 1997.
[9] B. W. Kim and S. S. Park, “Optimal Pricing, EOL (End
of Life) Warranty, and Spare Parts Manufacturing Stra-
tegy Amid Product Transition,” European Journal of

Development of Economic Models for EOL Service Parts Control 175
Operational Research, Vol. 188, No. 3, 2008, pp. 723-
745. doi:10.1016/j.ejor.2007.04.036
[10] P. D. T. O’Connor, “Practical Reliability Engineering,”
John Wiley & Sons, New York, 1995.
[11] G. V. Reklaitis, A. Ravindran and K. M. Ragsdell, “En-
gineerng Optimization Methods and Applications,” John
Wiley & Sons, New York, 1983.
[12] R. Hooke and T. A. Jeeves, “Direct Search of Numerical
and Statistical Problems,” The Journal of the ACM, Vol.
8, No. 2, 1966, pp. 212-229. doi:10.1145/321062.321069