Journal of Modern Physics, 2012, 3, 1572-1585
http://dx.doi.org/10.4236/jmp.2012.310194 Published Online October 2012 (http://www.SciRP.org/journal/jmp)
Radiological Concentration Distribution of 131I, 132I, 133I,
134I, and 135I Due to a Hypothetical Accident of
TRIGA Mark-II Research Reactor
M. A. Malek1, K. J. A. Chisty2, M. M. Rahman3
1Department of Electrical and Electronic Engineering, Green University of Bangladesh, Dhaka, Bangladesh
2Department of Electrical and Electronic Engineering, European University of Bangladesh, Dhaka, Bangladesh
3Energy Institute, Atomic Energy Research Establishment, Dhaka, Bangladesh
Received August 12, 2012; revised September 12, 2012; accepted September 20, 2012
ABSTRACT
The present work gives a methodology for assessing radiological concentration of 131I, 132I, 133I, 134I, and 135I due to a
hypothetical accident of TRIGA Mark-II research Reactor at AERE, Savar, Bangladesh. The concentrations were esti-
mated through different pathways like ingestion of vegetation, milk, and meat from air and ground deposition. The
maximum air concentrations for all 16 directions were found at 110 m distance from the core of the reactor and it was
found to be highest in the southern (S) direction. The maximum ground concentration occurred immediately just after
the accident in different directions. In all pathways, the most concentration was found to be in S-direction. The concen-
trations in vegetation of 131I, 133I, 135I were significant, while no concentrations of 132I and 134I were observed. The con-
centration in vegetation for 131I was found to be highest than all other isotopes of iodine. The concentrations of 133I were
found to be higher and concentrations of 134I were observed to be lower in both milk and meat compared to other radio
isotopes of iodine. In the case of a radiological accident, the results of the present study will be a valuable guide for
adopting radiological safety measures for radiation protection against the ingestion of vegetables, milk and meat from
around the research reactor at AERE, Savar, Bangladesh.
Keywords: Concentration; Effective Stack Height; Pathway; S-Direction; TRIGA Mark-II
1. Introduction
Atmospheric diffusion and radiation concentration cal-
culations for accidental releases of radioactive gases and
volatiles are an important contribution to licensing re-
quirements for the selection of site for a nuclear reactor.
The reactor-operating license is obtained from local regu-
latory authorities in accordance with internationally
adopted criteria [1].
Nuclear reactors, specially research reactors, do not
release any significant quantity of radioactive material to
the atmosphere under normal operating conditions. How-
ever, a significant fraction of the radionuclide inventory
in the core may be released to the atmosphere under ac-
cident conditions with severe core damage. In the case of
a hypothetical accident of research reactor, radionuclides
that are predictable to be released through the stack, can
cause direct radiation exposure of the public in the
downwind direction and can also be deposited on the
ground and vegetation resulting in exposure through dif-
ferent pathways such as external irradiation, inhalation,
ingestion etc. [2,3], when cows eat vegetation, milk and
meat become contaminated. Immediately after an acci-
dent, isotopes of iodine such as 131I, 132I, 133I, 134I, and 135I
presents the most serious radiological hazards [4]. The
main doses concerns are those to thyroid due to external
irradiation, inhalation and ingestion of radioiodines.
To evaluate doses, measurement of activity concentra-
tion of radionuclides in different pathways is necessary.
An attempt has therefore been made in this work to find
out the concentration in vegetation, milk and meat due to
deposition of 131I, 132I, 133I, 134I, and 135I considering hy-
pothetical accident of TRIGA MARK-II research reactor
at AERE, Savar. Radionuclides can be deposited on soil
either by direct deposition from the atmosphere or from
the use of surface water for irrigation. When there is a
canopy of vegetation, radionuclides can reach the soil
through leaf fall, leaching, wash-off, herbivore excretion
and trampling [5].
Different assumptions and methodologies have been
taken into account for assessing concentration suggesting
the necessity of site-specific data. Recently IAEA pub-
lished generic methodologies for use in assessing the ra-
diological consequence due to the releases of radioactive
C
opyright © 2012 SciRes. JMP

M. A. MALEK ET AL. 1573
materials in the environment, [5,6]. A computational
code has been developed based on these methodologies
and a hypothetical accident scenario to predict the con-
centration of 13 1 I, 132I, 133I, 13 4 I, and 135I in vegetation,
milk and meat. An IAEA document on research reactors
used 100%, 40% and 1% release fractions for noble gas-
es, halogens and particulates, respectively [6]. In this
work, we have calculated only the release of radioactive
iodine which is volatile, and 40% release fraction was
considered. The input parameters like wind speed and
frequency at different direction, average temperature
needed in the calculation have been collected from Bang-
ladesh Meteorological Department for the AERE site.
Other essential parameters needed in the calculation have
been taken from elsewhere [5].
2. Source Term and Accident Scenario
2.1. Source Term Calculation
The radiological source term describes the amount of the
nuclides which are released to the containment. A num-
ber of mathematical expressions can be applied to calcu-
late the amount of fission products in the research reactor
core as a function of irradiation time. An approximate
formula giving activity i

A
t of an isotope i at time t
after the start of irradiation (t = 0) whose fission yield is γ
and its decay constant is λi irradiated for a time period T
in P (megawatts of thermal power) can be written [6] as:




ei
itT
T



 
(1) 0.821 e
i
At P  
Radiological doses outside the reactor facility can be
caused only by radionuclides with a high degree of mo-
bility. The isotopic data considered here is limited due to
volatile group which is likely to be released in significant
quantities in the event of fuel melting with halogens,
volatiles (e.g. iodine); the most important contributors. A
considerable amount of radionuclides can be released
from the stack of the reactor building.
2.2. Release Rate Calculation
Source analysis addresses the problem of deriving the
source terms that determine the rate at which residual
radioactivity is released into the environment. This is
termed as release rate. The release rate of the radionu-
clides from stack is determined by the concentrations of
the radionuclides present, the ingrowth and decay rates of
the radionuclides, fraction released from fuel to building,
the geometry of the containment, the leak rate parameter
and overall the source term.
The total activity of isotope i released over time τ, Q
(τ), is obtained from the following Equation [6] as:
 
where FP is the fraction released from fuel to building, FB
is the fraction remaining airborne and available to be
released from the building to the atmosphere, λi is the
source term, λl is the leak rate parameter, sec−1, and λr is
the radioactive decay constant, sec−1.
2.3. Assumptions Made in the Calculation
In case of radiological assessment, source term is a very
vital parameter. The radiation received by the population
around the TRIGA reactor facility is directly dependent
on this parameter. Some realistic assumptions have been
made for doing the calculation of the present work.
These are given below:
 The reactor was operated at full power, i.e. 3 MW (t);
 Time after the start of irradiation: 10 days;
 Continuous operation at full power: 10 days;
 Radionuclide release time into the atmosphere from
stack: 2 hours after the accident;
 Radionuclides considered for radiological concentra-
tion assessment into the environment around the re-
actor building: 131I (T1/2 = 8.04 days), 132I (T1/2 = 2.30
hrs), 133I (T1/2 = 20.8 hrs), 134I (T1/2 = 0.876 hrs) and
135I (T1/2 = 6.61 hrs);
 Fraction release [7]: Iodine: 40% of the equilibrium
radioactive iodine (131I, 132I, 133I, 134I, 135I) inventory
developed from maximum fuel power operation of
the core are immediately available for leakage to the
reactor building in the direct proportion to percent of
fuel failure [7];
 Leak rate parameter,

1 = 1.157  10−7 sec−1. i.e., 1%/
day [5].
Considering the above assumptions, the activity of io-
dine (131I, 132I, 133I, 134I and 135I) was calculated consider-
ing the above assumptions and using Equation (1). The
fission yields of the corresponding radionuclides were
obtained as the fission fragment of the total product by
neglecting the filter and shielding efficiency. A constant
reactor power of 3 MW(t) is an acceptable approximation
for the irradiation. The fission product inventory would
be maximum corresponding to the infinite irradiation
time. The calculated activity released rate of 131I, 132I, 133I,
134I and 135I for 10 days operation at 3 MW(t) power level
are given in Table 1. The Table illustrates that the total
activity in the reactor core and released rate from con-
tainment to environment through the stack for 131I, 132I,
133I, 134I, and 135I are comparable with one another. This
is due to the similarity of fission yields and fraction of
release for these radionuclides.
3. Atmospheric Dispersion and Radiological
Concentration Calculation Models
3.1. The Gaussian Plume Model (GPM)

1e lr
l
ipbi
lr
QFFAt

 




 
 (2)
In the case of an accident, the escaped fission products

M. A. MALEK ET AL.
1574
Table 1. Calculated fission product inventory for 10 days
operation at 3 MW(t) power level.
Radionuclides Fission yield
Total activity in
core (Ci)
Released rate
(Bq/sec)
131I 0.0289 4.074 × 104 6.965 × 107
132I 0.054 6.06 × 104 8.960 × 107
133I 0.026 5.982 × 104 1.008 × 108
134I 0.047 2.376 × 104 2.812 × 107
135I 0.050 9.973 × 104 1.622 × 108
from reactor hall generally disperse in the surrounding
atmospheric environment through a stack of the reactor
as a radioactive smoke plume. The dispersion of radioac-
tive plume to the atmosphere depends strongly on the
atmospheric conditions such as temperature, wind fre-
quency, direction, speed and humidity of the atmosphere.
Gaussian plume model is widely applied to calculate
atmospheric dispersion in the atmospheric environment.
This model assuming that a Gaussian distribution in both
lateral and vertical directions can be described as [6]

 
22
22
22
e
zz
HZ






 
2
2
2
,,e e
2π
y
HZ y
i
yza
Q
xyz u





















,,
(3)
where
x
yz 

,,
is the radionuclide concentrations at
point
x
yz (Bq/m3); Qi is source strength or release
rate (Bq/s), ua is the wind speed at the height of the stack
(m/s), H is the stack height (m), σy and σz are the lateral
and vertical dispersion parameters (m), depending on
stability class. For radiological concentration assessment
ground level concentration is required and therefore, z
can be assumed to be zero and Equation (3) can be writ-
ten as

2
eff
22
exp
22
yz
QH










2
,, exp
π
i
yza
y
xyzu






(4)
where eff
H
is the stack height (m). The average con-
centration for release that occurs over a period of time
can be calculated by applying the above equation.
3.2. Effective Stack Height (ESH)
If the effluent has a significant exit velocity (or if it is at
a high temperature), it will rise to a level higher than the
actual stack height. The effective stack height, therefore,
is the sum of the actual stack height (H) plus a factor that
accounts for the exit velocity and/or the temperature of
the effluent gas as [8]
14 Δ
1
vT
uT







eff
a
HH D (5)
where D is the outlet stack diameter (m), v is the exit
effluent velocity (m/s), T is the difference between am-
bient and effluent gas temperatures, T is the absolute
temperature of the effluent.
For a research reactor like a TRIGA Mark-II at AERE,
Savar, the temperature difference, T can be considered
to zero because of active operation of the ventilation
system.
3.3. Average Wind Speed at ESH
Usually, meteorological data for wind speed and direc-
tion are measured at a 10 m height. This speed needs to
be converted into an effective stack height applying the
following relationship [3,9] as
eff
m
z
H
uu z



(6)
where uz is the speed at ground level at a height z = 10 m
and m is the wind coefficient depending on underlying
surface and diffusion category.
3.4. Air Concentration of Radionuclides and
Gaussian Diffusion Factor
In this case the sector averaged form of the GPM may be
used with the following simplifying assumptions:
1) A single wind direction and frequency for each air
concentration calculation;
2) A single long term average wind speed for each di-
rection;
3) A neutral atmospheric stability class (Pasquill-Gif-
ford stability class B) [5].
Air concentration of a radionuclide can be calculated
based on the above mentioned assumptions by using the
Equation [5]
exp
pi
Ai
aa
PFQ
x
Cuu 




(7)
where CA is the ground level air concentration at down-
wind distance x in sector p (Bq/m3); Pp is the fraction of
the time that the wind blows towards the receptor of in-
terest in sector p; ua is the geometric mean of the wind
speed at the height of release (m/s); F is the Gaussian
diffusion factor, appropriate for the height of release Heff
and the downwind distance x being considered (m−2); Qi
is the average annual discharge rate for radionuclide i
(Bq/s); i

is the rate constant for radioactive decay of
radionuclide i.
The Gaussian diffusion factor F as a function of
downwind distance x for a fixed value of eff
H
can be
estimated using the 22.5˚ sector averaged form of the
Gaussian plume model is given by [6]

M. A. MALEK ET AL. 1575
2
eff
2
p2z
z
H
x


3
ex
16
2π
F








 



 (8)
where
z

is the vertical diffusion parameter (m).
These expressions are appropriate for dispersion over
comparatively flat territory without pronounced dills or
valleys which is practically appropriate for our research
rector site. The territory is assumed to be covered with
pastures, forests and small villages. The value of
z

can be calculated on the basis of the following relation-
ship [5]
G
zEx
 

(9)
where E and G are the two parameters depending on the
stability class and on the effective stack height, and x is
the downwind distance.
Here for stability class “B” E = 0.127 and G = 1.108
for release height of 32.36 m at various downwind dis-
tance x m [10-12] was considered.
3.5. Group Deposition
The radioactive material may be removed from the
plume by the action of rain or snow interacting with it. In
general, the process can be assumed to remove radionu-
clides uniformly through the entire vertical extent of the
plume. The removal rate at any distance from the source
corresponds to the total amount of radio nuclides reach-
ing that distance.
It can also be removed from the plume by dry deposi-
tion. The rate at which material is deposited from the
plume will depend on the nature of the airborne material
and the underlying surface and can be estimated using
the concept of a deposition velocity or specifically, depo-
sition coefficient. The deposition coefficient is defined as
the ratio of the amount of activity deposited on the
ground per unit time and the ground level air concentra-
tion. For simplified assessment purposes, the following
relationship is used as

idwA
dVVC

(10)
where di is the total daily average deposition rate on the
ground of a given radionuclide i from both dry and wet
processes, including deposition either on to impervious
surfaces or on to both vegetation and soil (Bq·m−2·d−1);
Vd is the dry deposition coefficient for a given radionu-
clide (m/d); Vw is the wet deposition coefficient for a
given radionuclide (m/d).
The values of Vd and Vw for radionuclides are quite va-
riable. They depend on such factors as the physical and
chemical form of the radionuclide, the nature of the de-
position surface, meteorological conditions and, in the
case of Vw, the precipitation rate [13]. It has been rec-
ommended that a total deposition coefficient, VT (=Vd +
Vw). For deposition of aerosols and radioactive gasses
and radioactive gasses, VT = 1000 m/d may be used for
screening purposes [14]. This value of VT was found to
be consistent with values for radioiodine and radiocesium
from the accident at the Chernobyl nuclear power station
in 1986. Deposition rate can be used to calculate the ra-
dionuclide concentration on vegetation due to direct con-
tamination and the concentration of radionuclide in dry
soil.
3.6. Ground Concentration
The ground concentration mainly depends on the ground
deposition in the earlier section. It also depends on effec-
tive rate constant and duration of the discharge of the
radioactive material. The ground concentration can be
calculated by using the equation [5],
1exp s
i
s
i
ib
E
gr
E
dt
C









 (11)
where di is the total ground deposition rate (Bq·m−2·d−1);
s
i
E

is the effective rate constant for reduction of the
activity in top 10 to 20 cm of soil (d−1); there
s
i
E

=
is



,
s

is the rate constant for reduction of soil ac-
tivity owing to processes other than radioactive decay;
and b is the duration of the discharger of radioactive
material (d).
t

3.7. Concentrations in Vegetation
Radionuclides intercepted by and retained on vegetation
may result from fallout, washout, and irrigation with con-
taminated water or deposition of resuspended matter.
External deposits can be taken up by foliar absorption
into plants. Radionuclides may also be incorporated by
uptake from the soil through roots, followed by internal
redistribution of radionuclides within the plant. Processes
that may lead to the reduction of radionuclide concentra-
tions in vegetation include radioactive decay, growth di-
lution, wash-off of externally deposited radionuclides,
leaching and soil fixation. Further removal of radioactive
material from vegetation may occur due to grazing, har-
vesting, etc.
For conditions of prolonged deposition, such as from
discharges, the following equation may be used to esti-
mate the concentration Cv,i,l due to direct contamination
of nuclide I in and on vegetation,
,,1
1exp v
i
v
i
ie
E
vi
E
dt
C










,,1vi
C
(12)
where is measured in Bq/kg dry matter for vegeta-

M. A. MALEK ET AL.
1576
tion consumed by grazing animals and in Bq/kg fresh
matter for vegetation consumed by humans; i is the
deposition rate (from wet and dry processes ) of radionu-
clide i on to the ground (Bq·m−2·d−1);
d

is the fraction
of deposited activity intercepted by the edible portion of
vegetation per unit mass (or mass interception factor,
m2/kg) as the results of both wet and dry deposition
processes; for pasture forage the unit of mass is conven-
tionally given in terms of dry weight, and for fresh vege-
tables the unit is in wet weight; v
i
E

is the effective rate
constant for reduction of the activity concentration of
radionuclide i from crops (d−1); where v
i
Eiw

  e
t
;
is the time period during which the crops are exposed to
contamination during the growing season (d) (assumed to
be 1 day); w

is the rate constant for reduction of the
concentration of material deposited on the plant surfaces
owing to processes other than radioactive decay (d−1);
and i

is the rate constant for radioactive decay of ra-
dionuclide i (d−1).
3.8. Concentration for Uptake from Soil and Soil
Adhering
The radionuclide concentration in vegetable arising from
indirect processes i.e. uptake from the soil and from soil
adhering to the vegetation is given by following equation
as,
,,2vi 
C
,si
C
v
CF (13)
where ,,2vi is measured in Bq/kg dry matter for vege-
tation consumed by grazing animals and in q/kg fresh
matter for vegetation consumed by humans; v
F
is the
concentration factor for uptake if the radionuclide from
soil by edible parts of crops (Bq/kg plant tissue per Bq/
kg dry soil), it is conservatively assumed that all activity
removed from the atmosphere becomes available for up-
take from the soil; in addition, the selected values also
implicitly take account of the adhesion of soil to the ve-
getation; and ,is is the concentration of radionuclide i
in dry soil (Bq/kg.), which can be defined as ,
C


,
1exp
s
i
ib
si
E
C





s
i
E
dt 
(14)
where
s
i
E

is the effective rate constant for reduction of
the activity concentration in the root zone of soil (d−1),
where s
i
Eis

 ;
s

is the rate constant for reduc-
tion of the concentration of material deposited in the root
zone of soil owing to processes other than radioactive
decay (d−1); b is the duration of the discharge of ra-
dioactive material (d) (assumed to be 1 day); and
t

is a
standardized surface density for the effective root zone in
soil (kg/m2, dry soil) which is 260 [3].
The above equation refers to the total deposition and
neglects the amount, which is adsorbed to the vegetation.
3.9. Total Concentration in Vegetation
The total concentration of the radionuclide on the vegeta-
tion at the time of consumption is



,,,1,,2
exp
vivivii h
CCC t

 
C
(15)
where ,vi is measured in Bq/kg dry matter for vegeta-
tion consumed by grazing animals and in Bq/kg fresh
matter for vegetation consumed by human; i

is the
rate constant for radioactive decay of radionuclide I (d−1);
and h is a decay (hold-up) time that represents the time
interval between harvest and consumption of the food (d)
which is equal to 14 d.
t
3.10. Concentrations in Animal Feed
The transfer of radionuclide to animals will depend on
the intake of the animal and the metabolism of the vari-
ous radionuclides by the animal. The intake of radionu-
clides by animals depends on animal species, mass, age
and growth rate of the animal, the digestibility of feed
and, in the case of lactating animals, the milk is consid-
ered. For generic calculations, grazing animals’ are as-
sumed to be cattle, which during the grazing season, are
on a diet of fresh pasture only. The grazing season de-
pends on latitude and ranges from a few months to the
whole year. The concentration of radionuclide i in animal
feed is calculated by [5],


,, ,aip vip
CfClfC
C
C
0t
i (16)
where ,ai is the concentration of radionuclide i in the
animal feed (Bq/kg dry matter); ,vi is the concentra-
tion of radionuclide i for pasture, calculated using Equa-
tion (15) with h

(Bq/kg, dry matter); ,
p
i
C is the
concentration of radionuclide in stored feeds (Bq/kg, dry
weight), calculated using Equations (11)-(14) and sub-
stituting ,
p
i for ,vi with h days; and
C C90t
p
f
is
the fraction of the year that animals consume fresh pas-
ture vegetation (dimensionless) which is 0.7 [3].
3.11. Concentration in Milk
The concentration of a radionuclide in milk is assumed to
depend directly upon the amount and concentration level
in the feed consumed by the lactating animal. The con-
centration of radionuclide i in milk can be estimated by
using the equation [5],

 
,, exp mimaimwwi m
CFCQCQt   
,mi
C
m
(17)
where is the concentration in milk of radionuclide i
(Bq/L);
F
is the fraction of the animal’s daily intake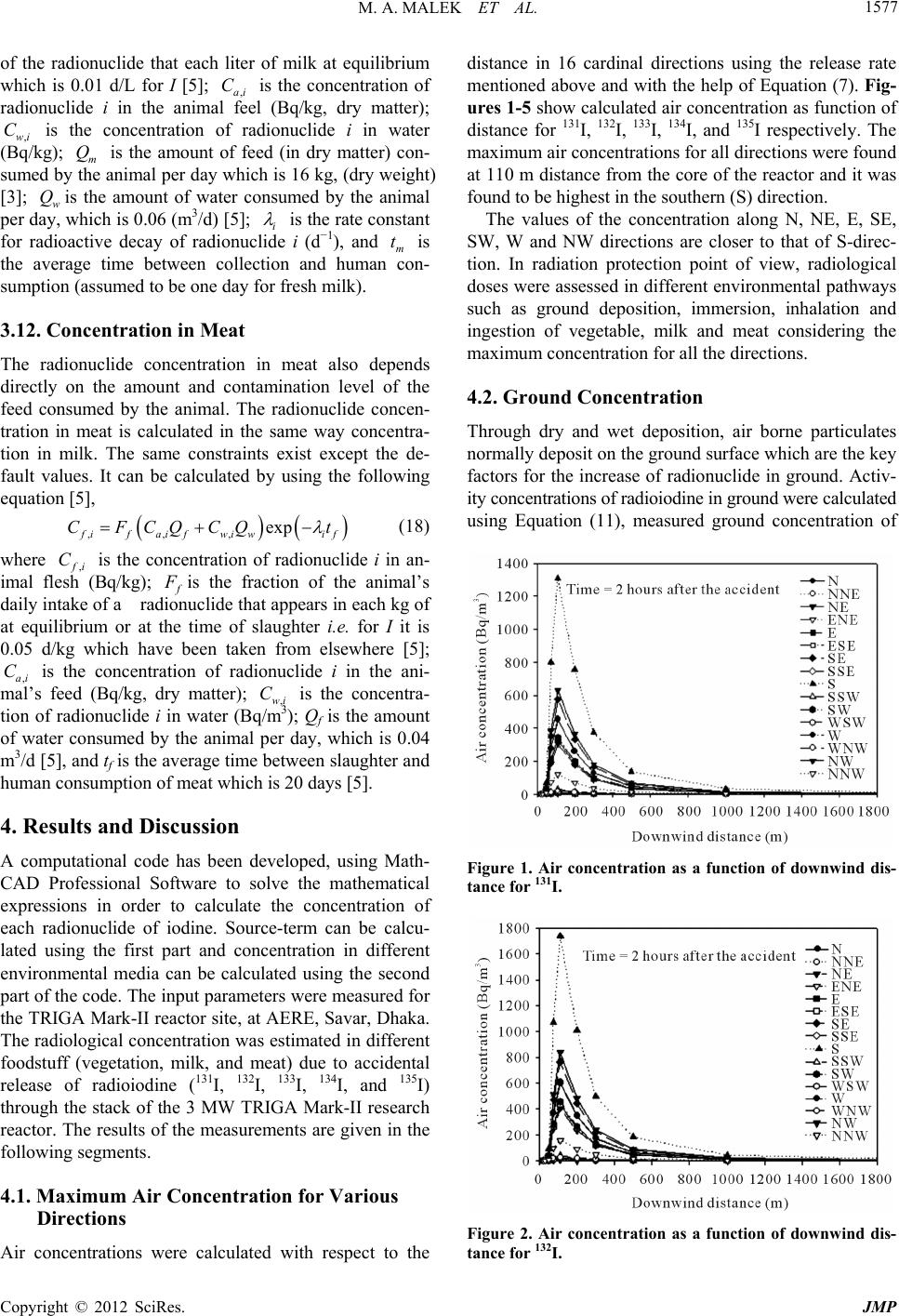

M. A. MALEK ET AL. 1577
of the radionuclide that each liter of milk at equilibrium
which is 0.01 d/L for I [5]; ,ai is the concentration of
radionuclide i in the animal feel (Bq/kg, dry matter);
,wi is the concentration of radionuclide i in water
(Bq/kg); m is the amount of feed (in dry matter) con-
sumed by the animal per day which is 16 kg, (dry weight)
[3]; wis the amount of water consumed by the animal
per day, which is 0.06 (m3/d) [5]; i
C
C
Q
Q

is the rate constant
for radioactive decay of radionuclide i (d−1), and m is
the average time between collection and human con-
sumption (assumed to be one day for fresh milk).
t

,
ex
3.12. Concentration in Meat
The radionuclide concentration in meat also depends
directly on the amount and contamination level of the
feed consumed by the animal. The radionuclide concen-
tration in meat is calculated in the same way concentra-
tion in milk. The same constraints exist except the de-
fault values. It can be calculated by using the following
equation [5],

,, p
f
if
CF
a
C
ifwi
QC
w if
Q t  
(18)
where ,
f
i is the concentration of radionuclide i in an-
imal flesh (Bq/kg);
C
f
F
is the fraction of the animal’s
daily intake of a radionuclide that appears in each kg of
at equilibrium or at the time of slaughter i.e . for I it is
0.05 d/kg which have been taken from elsewhere [5];
,ai is the concentration of radionuclide i in the ani-
mal’s feed (Bq/kg, dry matter); ,wi C is the concentra-
tion of radionuclide i in water (Bq/m3); Qf is the amount
of water consumed by the animal per day, which is 0.04
m3/d [5], and tf is the average time between slaughter and
human consumption of meat which is 20 days [5].
C
4. Results and Discussion
A computational code has been developed, using Math-
CAD Professional Software to solve the mathematical
expressions in order to calculate the concentration of
each radionuclide of iodine. Source-term can be calcu-
lated using the first part and concentration in different
environmental media can be calculated using the second
part of the code. The input parameters were measured for
the TRIGA Mark-II reactor site, at AERE, Savar, Dhaka.
The radiological concentration was estimated in different
foodstuff (vegetation, milk, and meat) due to accidental
release of radioiodine (131I, 132I, 133I, 134I, and 135I)
through the stack of the 3 MW TRIGA Mark-II research
reactor. The results of the measurements are given in the
following segments.
4.1. Maximum Air Concentration for Various
Directions
Air concentrations were calculated with respect to the
distance in 16 cardinal directions using the release rate
mentioned above and with the help of Equation (7). Fig-
ures 1-5 show calculated air concentration as function of
distance for 131I, 132I, 133I, 134I, and 135I respectively. The
maximum air concentrations for all directions were found
at 110 m distance from the core of the reactor and it was
found to be highest in the southern (S) direction.
The values of the concentration along N, NE, E, SE,
SW, W and NW directions are closer to that of S-direc-
tion. In radiation protection point of view, radiological
doses were assessed in different environmental pathways
such as ground deposition, immersion, inhalation and
ingestion of vegetable, milk and meat considering the
maximum concentration for all the directions.
4.2. Ground Concentration
Through dry and wet deposition, air borne particulates
normally deposit on the ground surface which are the key
factors for the increase of radionuclide in ground. Activ-
ity concentrations of radioiodine in ground were calculated
using Equation (11), measured ground concentration of
Figure 1. Air concentration as a function of downwind dis-
tance for 131I.
Figure 2. Air concentration as a function of downwind dis-
tance for 132I.

M. A. MALEK ET AL.
1578
Figure 3. Air concentration as a function of downwind dis-
tance for 133I.
Figure 4. Air concentration as a function of downwind dis-
tance for 134I.
Figure 5. Air concentration as a function of downwind dis-
tance for 135I.
131I, 132I, 133I, 134I and 135I and total concentration (131I +
132I + 133I + 134I + 135I) are graphically illustrated in Fig-
ure 6 for eight (8) cardinal directions. The maximum
ground concentration occurred immediately just after the
accident in different directions which has been shown in
Table 2. Maximum ground concentration was found in
S-direction. With increasing time, the concentrations are
found to decrease exponentially after the accident due to
radioactive decay. This radionuclide is transferred to
plants and then to meat and milk via ingestion and will
be exposed to human directly and indirectly.
5. Concentration in Different Pathways
5.1. Ground Concentration
The activity concentration in vegetation occurs due to
direct deposition of air borne radionuclides on the edible
portion of the vegetables and uptake from soil. The con-
centration in vegetation for 131I, 132I, 133I, 134I, 135I and
total are shown in Table 3 and plotted as a function of
time for 8 directions, shown in Figure 7. This Table pre-
dicts that the concentration of vegetation due to 132I, and
134I is found to be zero which is due to very short half-life
and 131I is the dominant contributor of concentration in
vegetation for long half life. Figure 7 predicts that the
concentration in vegetation decreased exponentially with
time after the accident and within 60 days most of the
activity disappeared and the Sequence of concentration
of iodine is Cveg
131I > Cveg 133I > Cveg 135I.
5.2. Concentration in Milk
The radionuclide in milk arises due to consumption of
contaminated feed by the lactating animal and its con-
centration in milk depends directly on radioactivity con-
centration of the feed consumed by the lactating animal.
Concentrations of 131I, 132I, 133I, 134I, 135I and total (131I +
132I + 133I + 134I + 135I) in milk as a function of time for
different directions are shown in Figure 8 and the con-
centration in milk at time t = 0 is given in Table 4.
From the graph it is clear that the maximum concen-
tration occurs at time t = 0 for each direction and is de-
creasing exponentially with time and become nearly to
zero within 100 days. The Table 4 shows that the maxi-
mum concentration was found in S-direction among all
other directions. It was found the sequence of concentra-
tion as Cmilk
133I > Cmilk 131I > Cmilk 132I > Cmilk 135I > Cmilk
134I, where “CmilkI” refers to the concentration of different
isotopes of iodine in milk. The concentration of 133I in
milk is higher than all other isotopes of iodine because of
its higher concentration in animal’s feed.
5.3. Concentration in Meat
The radionuclide concentration in meat occurs via con-
sumption of contaminated food—in the same way as con-
centration in milk. Table 5 shows the concentration in
meat immediately just after the accident for eight domi-
nant directions. Maximum concentration was found again
in S-direction and the sequence of the concentration of

M. A. MALEK ET AL.
1579
Table 2. Maximum ground concentr ation for differe nt dire c t ions at time t = 0 (i.e. just after the accident).
Ground concentration (Bq/m2)
Direction 131I 132I 133I 134I 135I Total
N 4.301×E+5 8.284E+4 4.652E+5 9.856E+3 6.831E+4 1.056E+6
NE 2.919E+5 5.622E+4 3.157E+5 6.69E+3 4.637E+4 7.168E+5
E 3.106E+5 5.986E+4 3.359E+5 7.129E+3 4.941E+4 7.629E+5
SE 5.493E+5 1.059E+5 5.942E+5 1.262E+4 8.745E+4 1.349E+6
S 1.251E+6 2.411E+5 1.353E+6 2.872E+4 1.99E+5 3.072E+6
SW 3.285E+5 6.331E+4 3.553E+5 7.539E+3 5.226E+4 8.07E+5
W 4.359E+5 8.398E+4 4.715E+5 9.995E+3 6.928E+4 1.071E+6
NW 6.013E+5 1.158E+5 6.503E+5 1.379E+4 9.555E+4 1.477E+6
Table 3. Maximum concentration in vegetation for different directions at time t = 0 (i.e. just after the accident).
Concentration in vegetation (Bq/kg)
Direction 131I 132I 133I 134I 135I Total
N 3.764E+4 0 1.869 0 9.622E−12 3.764E+4
NE 2.554E+4 0 1.268 0 6.531E−12 2.554E+4
E 2.718E+4 0 1.35 0 6.96E−12 2.718E+4
SE 4.807E+4 0 2.387 0 1.232E−11 4.807E+4
S 1.094E+5 0 5.435 0 2.804E−11 1.095E+5
SW 2.875E+4 0 1.428 0 7.361E−12 2.875E+4
W 3.815E+4 0 1.894 0 9.758E−12 3.815E+4
NW 5.262E+4 0 2.612 0 1.346E−11 5.262E+4
Table 4. Concentration in milk for different directions at time t = 0 (i.e. just after the accident).
Concentration in milk (Bq/L)
Direction 131I 132I 133I 134I 135I Total
N 2.814E+4 5.511E+3 3.056E+4 656.123 4.515E+3 6.938E+4
NE 2.04E+4 3.996E+3 2.216E+4 475.711 3.274E+3 5.03E+4
E 1.454E+4 2.848E+3 1.579E+4 339.297 2.335E+3 3.585E+4
SE 2.571E+4 5.038E+3 2.792E+4 600.501 4.132E+3 6.34E+4
S 5.854E+4 1.147E+4 6.357E+4 1.367E+3 9.406E+3 1.444E+5
SW 1.538E+4 3.012E+3 1.67E+4 358.837 2.469E+3 3.792E+4
W 2.04E+4 3.996E+3 2.216E+4 475.711 3.274E+3 5.03E+4
NW 2.814E+4 5.511E+3 3.056E+4 656.123 4.515E+3 6.938E+4
Table 5. Concentration in meat for different directions at time t = 0 (i.e. just after the accident).
Concentration in meat (Bq/kg)
Direction 131I 132I 133I 134I 135I Total
N 7.549E+4 1.476E+4 8.196E+4 1.752E+3 1.21E+4 1.861E+5
NE 5.123E+4 1.002E+4 5.562E+4 1.189E+3 8.212E+3 1.263E+5
E 5.451E+4 1.066E+4 5.919E+4 1.267E+3 8.751E+3 1.344E+5
SE 9.641E+4 1.886E+4 1.047E+5 2.242E+3 1.549E+4 2.377E+5
S 2.195E+5 4.294E+4 2.384E+5 5.104E+3 3.525E+4 5.412E+5
SW 5.766E+4 1.128E+4 6.261E+4 1.34E+3 9.255E+3 1.421E+5
W 7.651E+4 1.496E+4 8.307E+4 1.776E+3 1.227E+4 1.886E+5
NW 1.055E+5 2.063E+4 1.146E+5 2.45E+3 1.692E+4 2.601E+5

M. A. MALEK ET AL.
1580
Figure 6. Ground concentration as a function of time for the directions of E, NE, NW, S, SE, SW, W and N.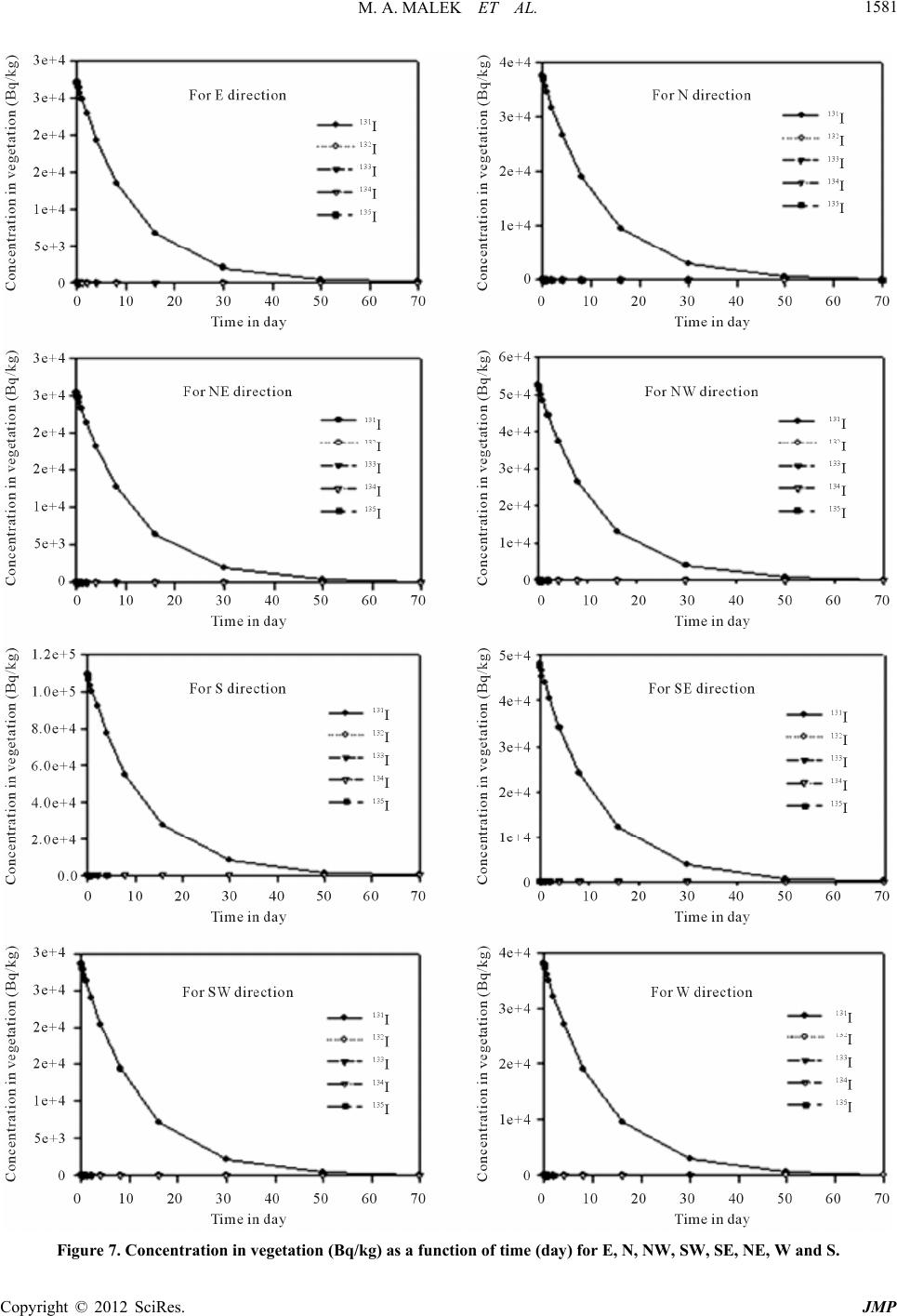

M. A. MALEK ET AL. 1581
Figure 7. Concentration in vegetation (Bq/kg) as a function of time (day) for E, N, NW, SW, SE, NE, W and S.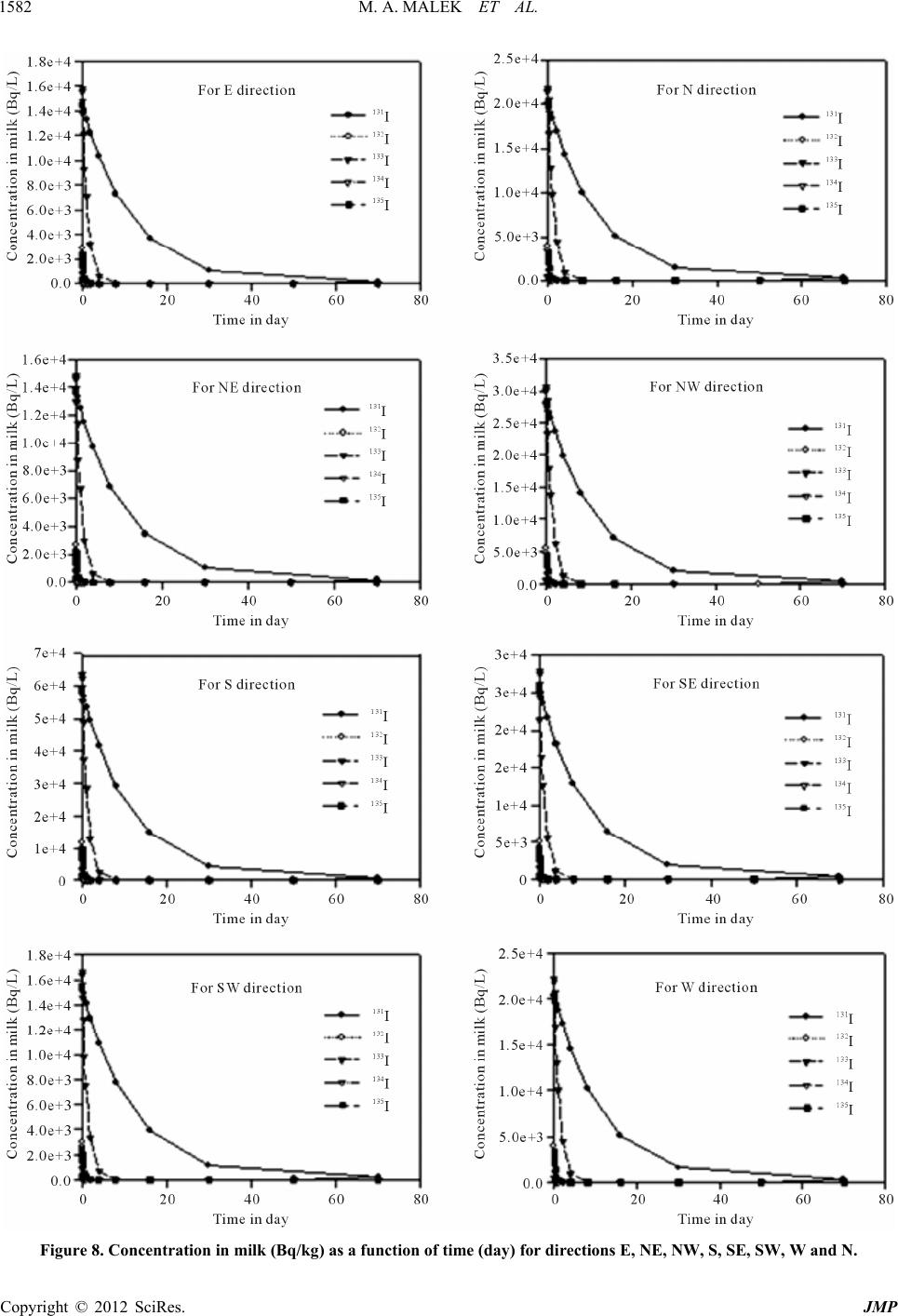

M. A. MALEK ET AL.
1582
Figure 8. Concentration in milk (Bq/kg) as a function of time (day) for directions E, NE, NW, S, SE, SW, W and N.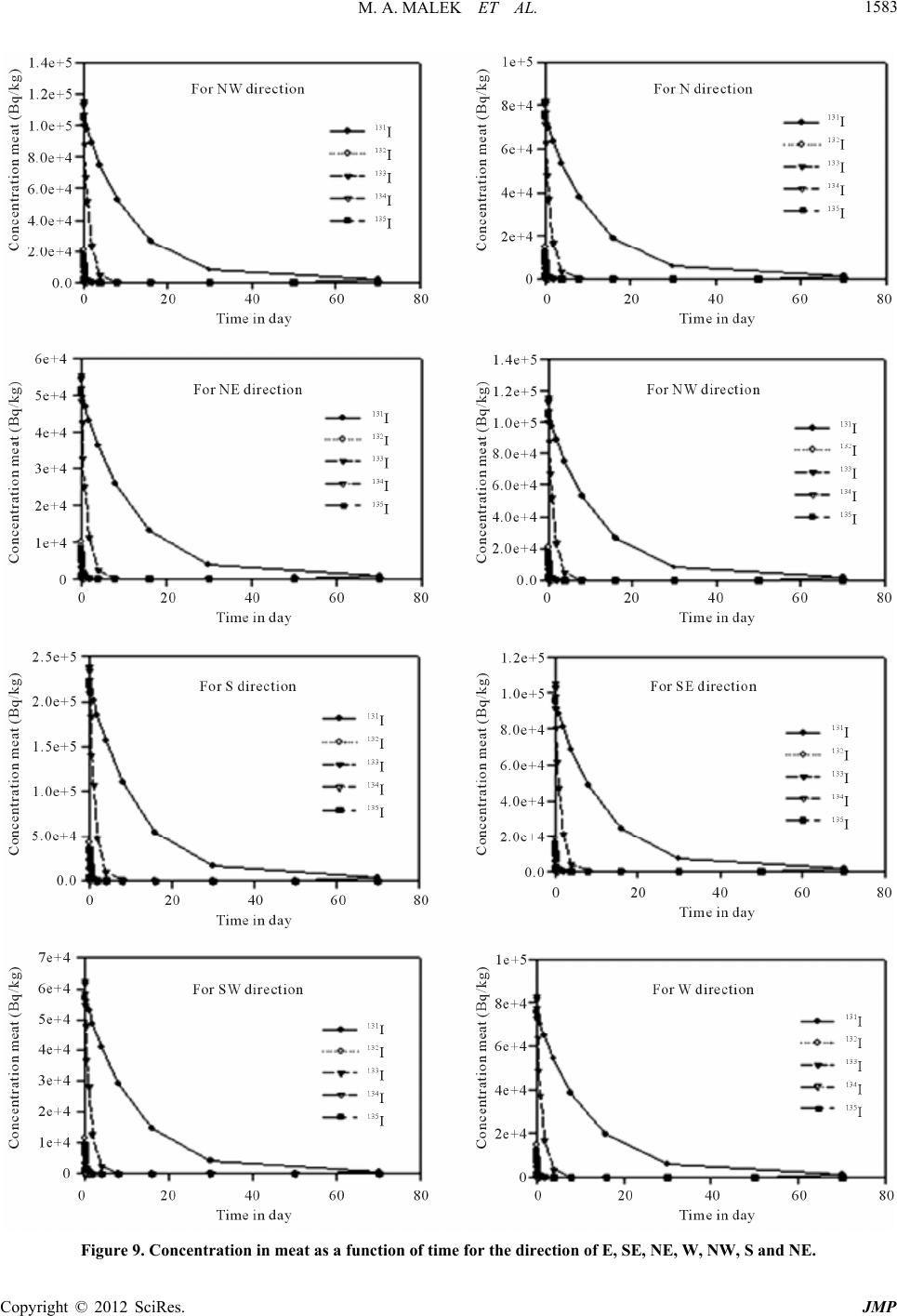

M. A. MALEK ET AL. 1583
Figure 9. Concentration in meat as a function of time for the direction of E, SE, NE, W, NW, S and NE.

M. A. MALEK ET AL.
1584
radioiodine is Cmeat
133I > Cmeat 131I > Cmeat 132I > Cmeat 135I >
Cmeat
134I where “CmeatI” refers to the concentration of
different isotopes of iodine in meat. The time dependent
concentration of meat due to 131I, 132I, 133I, 134I, 135I and
total (131I + 132I + 133I + 134I + 135I) in different directions
were plotted and are shown in Figure 9. The concentra-
tion of 133I is significantly higher than that of all other
isotopes of iodine (i.e. 131I, 132I, 134I, 135I).
6. Concentration in Different Pathways
In this study, a computational code has been developed
Based on the atmospheric dispersion phenomena for as-
sessment of radiological concentration due to release of
radioiodine for a hypothetical accident of the TRIGA
Mark-II research reactor. Based on the assumed hypo-
thetical accidental condition, activity of the radioiodine
131I, 132I, 133I, 134I and 135I in the reactor core and their
release rate were calculated. The air concentrations for
these five isotopes of iodine in 16 directions were pres-
ently calculated with respect to downwind distance from
the core of the reactor. The maximum air concentrations
for all the 16 directions were found at 110 m distance
from the reactor core. Maximum value of air concentra-
tion for all of the radioisotopes of iodine was found along
S-direction and the lowest was found in NE direction.
The ground concentration, concentration in vegetation,
milk, and meat for the above-mentioned radioisotopes of
iodine were measured for 8 directions (i.e. N, NE, E, SE,
S, SW, W, and NW) as a function of time. The concen-
tration in the different pathways of the radioisotopes of
radioiodine are very low in other 8 directions (i.e. NNE,
ENE, ESE, SSE, SSW, WSW, WNW, and NNW), be-
cause of lower frequency of the wind in these directions
and hence the calculation of the concentrations along
these directions were avoided in this work.
Concentration in vegetation for 131I was found to be
highest than all other isotopes and for 132I and 134I the
values were predicted to be zero. Concentration in milk
of 133I was higher and that of 134I was lower within all
other isotopes of iodine. The concentration in meat of 133I
was found to be higher than other isotopes of iodine and
that was lower for 134I. There should be an awareness and
caution about taking plants or vegetable grown at the site
of accident. This study might provide a guideline on the
radiological safety measures that has to be taken for ra-
diation protection due to ingestion of vegetation, milk
and meat from the reactor site at AERE in case of a ra-
diological accident.
7. Acknowledgements
The authors are thankful to the staffs of EI for their sin-
cere efforts to complete the secretarial job.
REFERENCES
[1] International Atomic Energy Agency, Information to be
Submitted in Support of Licensing Application for Nu-
clear Power Plants, “A Safety Guide,” Technical Report
Series No. 50-SG-G2, Vienna, 1979.
[2] A. Ararkrog, “Global Radiological Impact of Nuclear
Activities in the Former Soviet Union,” Proceedings of
International Symposium on Environmental Impact of
Radioactive Releases, Vienna, 8-12 May 1995.
[3] International Atomic Energy Agency, “Generic Models
and Parameters for Assessing the Environmental Transfer
of Radionuclides from Routine Releases,” Safety Series
No. 57, IAEA, Vienna, 1982.
[4] I. I. Kryshev, K. P. Makhonko, T. G. Sazykina, “Dose
Assessment and Reconstruction in the Areas of Russia
Contaminated after the Chernobyl Accident,” IAEA-
TECDOC-755, pp. 105-114.
[5] International Atomic Energy Agency, “Generic Models
for Use in Assessing the Impact of Discharge of Radioac-
tive Substances to the Environment,” Safety Series No.
19, IAEA, Vienna, 2001.
[6] International Atomic Energy Agency, “Research Reactor
Core Conversion Guidebook,” IAEA-TECDOC-643, 2,
1992.
[7] W. L. Woodruff, D. K. Warinner and J. E. Motas, “Re-
search Reactor Core Conversion Guide Book,” IAEA-
TECDOC-643, 2, 1992, pp. 155-178.
[8] H. Chember, “Introduction to Health Physics,” 3rd Edi-
tion, The MeGraw-Hill Companies Inc., New York, 1996.
[9] INTERATOM, Bergisch Glad Bach, Federal Republic of
Germany, “Fundamental Calculation Model for the De-
termination of the Radiological Effects inside and outside
the Research Reactor after Hypothetical Accidents with
Release of High Amount of Fission Products from the
Core, Research Reactor Core Conversion Guidebook,”
IAEA-TECDOC-643, IAEA, Vienna 2, 1992, pp. 211-
232.
[10] H. Geiss, K. Nester, P. Thomas and K. J. Vogt, “In der
Bundesrepublik Deutschland Experimental Ermittelte Aus-
breitungsparameter Fuer 100 m Emissionshoehe,” Reps
Juel-1707, KIK-3095, Kernforschungsanlage Kuelich/Kern-
forschungsze-ntrum, Karlsruhe, 1981.
[11] K. J. Vogt and H. Geiss, “Neue Ausbreitungskoeffi-
zienten Fuer 50 and 100 m Emissionshaoehe,” Internal
Rep., Kernforschungsanlage, Juelich, 1980.
[12] W. Huebschmann, K. Nester, and P. Thomas, “Ausbrei-
tungsparameter Fuer Emissionshoehe, von 160 m und 195
m,” Rep. KfK-2939, Kernforschungszentrum, Karlsruhe,
1980.
[13] National Council on Radiation Protection and Measure-
ments, “Uncertainty in NCRP Screening Models Relating
to Atmospheric Transport, Deposition, and Uptake by
Humans,” NCRP Commentary No. 8, NCRP, Bethesda,
1993.
[14] National Council on Radiation Protection and Measure-
ments, “Screening Techniques for Determining Compli-
ance with Environmental Standards,” Releases of Ra-
dionucliders to the Atmosphere, NCRP Commentary No.

M. A. MALEK ET AL. 1585
3, Revision Plus Addendum, NCRP, Bethesda, 1996.
[15] S. S. Raza, M. Iqbal, A. Salauddin, R. Avila and S. Per-
vez, Time-Integrated Thyroid Dose for Accidental Re-
leases from Pakistan Research Reactor,” Journal of Ra-
diological Protection, Vol. 24, No. 3, 2004, pp. 307- 314.
doi:10.1088/0952-4746/24/3/009
[16] M. Mizanur Rahaman, “Dose Assessment of a Contami-
nated Land Containing Radioactive Materials,” M.Phil
Thesis, Bangladesh University of Engineering and Tech-
nology, Dhaka, 2003.