Modern Economy, 2012, 3, 653-657
http://dx.doi.org/10.4236/me.2012.35084 Published Online September 2012 (http://www.SciRP.org/journal/me)
Game-Theoretic Analysis of Renouncing Membership of a
Party to Announce Candidacy
Jue-Shyan Wang1, Mei-Yin Lin2
1Department of Public Finance, National Chengchi University, Taipei, Chinese Taipei
2Department of Economics, Shih Hsin University, Taipei, Chinese Taipei
Received June 20, 2012; revised July 20, 2012; accepted July 28, 2012
ABSTRACT
A game model is established to analyze the interaction in strategy when there is a candidate who renounces membership
of a party to run for a position. We use the Bayesian Nash equilibrium and sequential equilibrium to discuss two models
in which the candidate without nominations moves first or the decision maker of the other party moves first respectively.
The main finding is that the equilibrium strategy of the decision maker of the other party is identical when the opponent
runs for a position, regardless of who moving first. However, the probability of the candidate without nomination to run
for a position is larger when he moves first.
Keywords: Bayesian Nash Equilibrium; Sequential Equilibrium
1. Introduction
In Taiwan, there are two ways for anyone to be a candi-
date: to be nominated by a party or to run for a position
independently. The nominee will have full support from
the party in the election campaign. However, a member
in the party without nomination should renounce mem-
bership to run for a position. There were some famous
examples of violating the party rule to elect independently
in the electoral history of Taiwan. The examples were Lin
Yang-kang in the presidential election in 1996, James
Soong in the presidential election in 2000. There was an
example in United States too. In spite of being a Democ-
rat originally, George Wallace ran for the US president in
1968 on the American Independent Party.
In general, a candidate that doesn’t acquire a party nomi-
nation has paid the penalty of breaking off relation with
the original party. There may be some benefit for a mem-
ber to renounce membership to run for a position. This
paper intends to study the behavior of the candidate who
withdraws from a party.
Only few economic studies have so far been made at
this issue. Epstein (1967) demonstrates that the candidates
nominated by the party can form the image in the voters’
minds and this is important information for voting deci-
sion. Gallaher (1988) suggests that the party will control
the members of congress by the power of nominations.
Ware (1988) points out the leader of the party will be short
of ability to carry out the nominations if there is a dispute
inside the party.
The previous literature mostly focuses on the nominat-
ing process or the possible influence of nomination on the
election or voter’s decision. This paper sets up a game
model to analyze the interaction in strategy when there is
a candidate who renounces membership to run for a posi-
tion. We concern with the behaviors of the candidate with-
out nomination and the decision maker of the other party.
We first consider the case of the candidate without nomi-
nation moving first. Bayesian Nash equilibrium is used to
analyze this case where there is incomplete information.
Secondly, we discuss the case of the decision maker of
the other party moving first. We use sequential equilib-
rium to describe the equilibrium in the case where the
information is imperfect.
2. Model 1: The Candidate without
Nomination Moving First
Suppose the candidate who is not nominated by the party
(denoted by player 1) makes his decision about whether
to run for a position in the first. Then the player 2, denot-
ing the decision maker of the other party, decides whether
to campaign actively or inactively. Assume there are two
types of player 2. The first type of player 2 is denoted by
type-s which occurs with probability of




0, 1qq.
Type-s player 2 thinks he will get benefit from the dam-
aging image of the split in the opposite party even though
he campaigns inactively and player 1 wins the election.
This benefit of type-s player 2 in this state is set to be




0,1ff. Type-w is defined as the second type of
C
opyright © 2012 SciRes. ME

J.-S. WANG, M.-Y. LIN
654
player 2 with probability of 1 – q. Type-w player 2 thinks
he will get the benefit of value d if he participates in the
election actively, no matter who the winner is in the elec-
tion. The player 1 knows that the value of the probability
is q, but doesn’t observe in advance which type of player
2 he is dealing with.
Suppose the total vote these two players could get is
fixed. And we assume the sum of the winning probability
for the two players is fixed to be r. Then when both of
the two players participate in the election actively, the win-
ning probability of player 1 and player 2 are r – e and e
respectively. It should be noted that we suppose the can-
didate renouncing the membership doesn’t split any vote
from his original party. Finally, the cost of electoral ac-
tions for player 1 is assumed to be the value of a. The
cost of electoral actions for player 2 is set to be the value
of b when he campaigns actively. However, when player
2 campaigns inactively, there is no cost of electoral ac-
tions. We assume r > a which implies that there really
exists the incentive for player 1 to renounce the mem-
bership to run for a position. The whole structure of the
game we described above is shown in Figure 1. And the
normal form of the game is presented in Figure 2.
The symbol “A” indicates the player campaigns actively
and the symbol “I” means the player campaigns inactively.
We describe the strategy for player 2 with type-s in the
former and then the strategy for player 2 with type-w in
the latter. So the four pure strategies for player 2 are (AA,
AI, IA, II). Let Ai and Bi denote the payoffs of player 1
and player 2 in state i respectively, where i belongs to the
set of four strategies of player 2. The definitions for Ai
and Bi are given in the following:


 
qd
qe
qe
qedb


 

1
1
2
2
3
3
4
4
1
1
1
Arae
Beb
Ara
Bqeb
Ara
Bqfr
Ara
Bqfr








Figure 1. The extension form game of model 1.
player 2
AA AI IA II
run (1) A1, B1(2) A2, B2 (3) A3, B3 (4) A4, B4
Player 1
not run(5) 0, r – b(6) 0, r – b (7) 0, r – b(8) 0, r – b
Figure 2. The normal form game of model 1.
For the rationality of analysis, we assume e – b > 0.
This allows for the possibility of player 2 to campaign
actively. Moreover, when the expected payoffs of running
for a position and not running for a position are equal,
player 1 will choose the strategy of not running.
Now we use the Bayesian Nash equilibrium to analyze
this model. We first discuss the strategy of player 2 in
equilibrium. By the assumption of e – b > 0, the follow-
ing two inequalities will be satisfied.


 
1
1
ebqd qeb
qfrq e dbqfr
 
 

0A
Therefore, if player 1 decides to run for a position, player
2 will not choose the strategies (AI) and (II). Comparing
the expected payoffs of the strategies (AA) and (IA), we
get the following result. When e – b > fr, player 2 will
adopt the strategy (AA), otherwise he will select the strat-
egy (IA). If the expected payoffs of these two strategies
are equal, then he can choose either.
Since player 2 will not select the strategies (AI) and
(II), we would compare the expected payoffs of player 1
among A1, A3 and 0. There exist the following three
situations:
1) A1 ≥ 0;
2) A3 ≥ 0 > A1;
3) A3 < 0;
Combining the player’s principle for decision with the
three situations stated above, the results of Bayesian Nash
equilibriums could be summarized in Table 1.
From Table 1, we first conclude that when the exp-
ected payoff of player 1 is smaller 3, player 1 is
unwilling to run for a position because of the negative
expected payoff. Secondly, type-w player 2 is determined
to campaign actively. However, type-s player 2 will cam-
paign actively when player 1 runs for a position only if
the payoff of player 2 is larger than that of campaigning
inactively


eb fr . The reverse is true. Finally, since
A3 is positively related to q, player 1 is very likely to run
for a position when the proportion of type-s in player 2 is
large. The Bayesian Nash equilibriums in this model may
be summarized in the following proposition.
Proposition 1. The decision maker of the other party
with type-w will certainly campaign actively. And type-s
player will campaign actively (inactively) if


eb fr .
Moreover, the higher the proportion q is, the greater the
probability of the candidate without nomination to run
for a position is.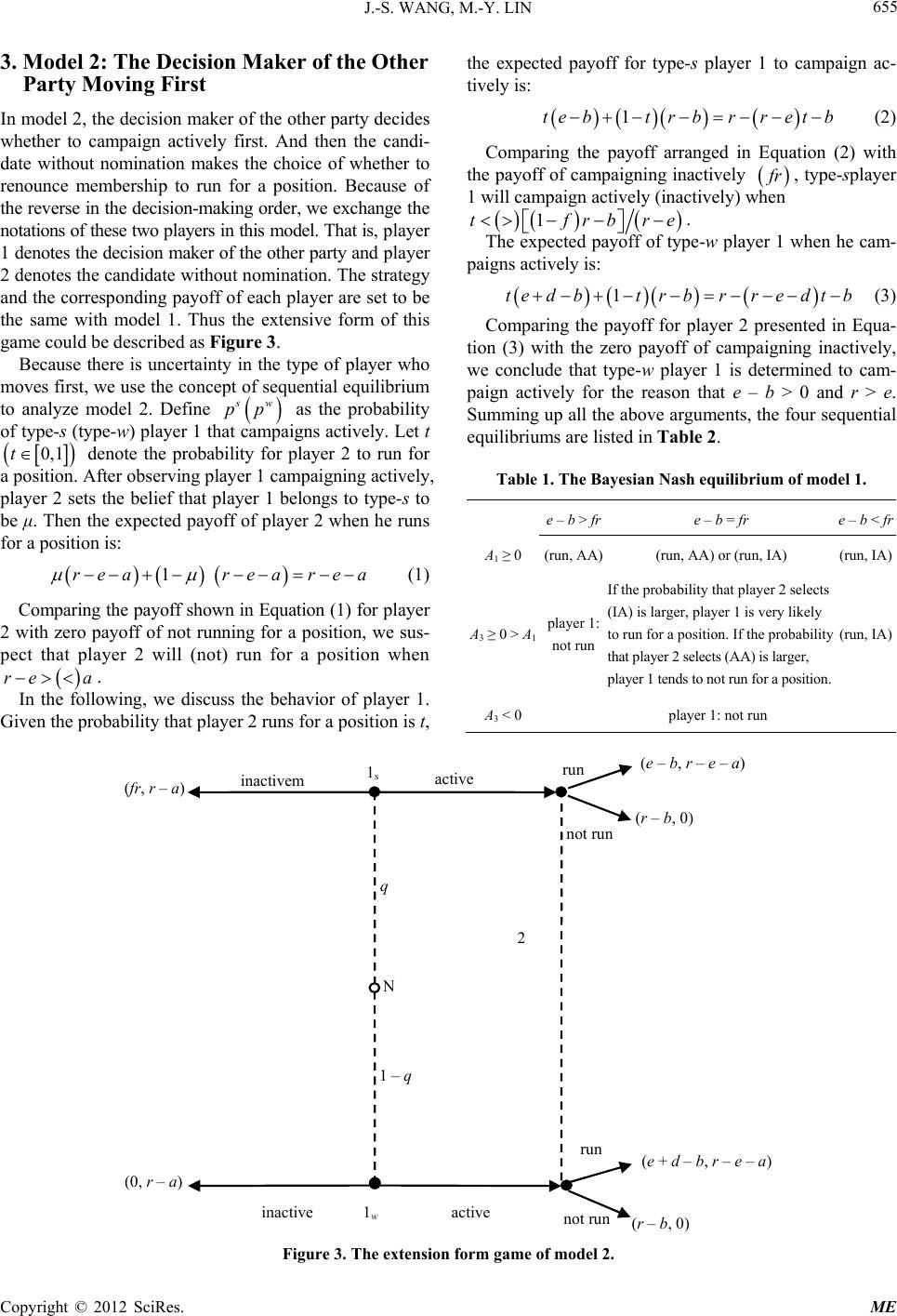

J.-S. WANG, M.-Y. LIN
655

3. Model 2: The Decision Maker of the Other
Party Moving First the expected payoff for type-s player 1 to campaign ac- tively is:






1te btr brret b
In model 2, the decision maker of the other party decides
whether to campaign actively first. And then the candi-
date without nomination makes the choice of whether to
renounce membership to run for a position. Because of
the reverse in the decision-making order, we exchange the
notations of these two players in this model. That is, player
1 denotes the decision maker of the other party and player
2 denotes the candidate without nomination. The strategy
and the corresponding payoff of each player are set to be
the same with model 1. Thus the extensive form of this
game could be described as Figure 3.
Because there is uncertainty in the type of player who
moves first, we use the concept of sequential equilibrium
to analyze model 2. Define
s
w
pp

as the probability
of type-s (type-w) player 1 that campaigns actively. Let t


0, 1t

a rea

re a
denote the probability for player 2 to run for
a position. After observing player 1 campaigning actively,
player 2 sets the belief that player 1 belongs to type-s to
be μ. Then the expected payoff of player 2 when he runs
for a position is:

1rea re    (1)
Comparing the payoff shown in Equation (1) for player
2 with zero payoff of not running for a position, we sus-
pect that player 2 will (not) run for a position when
.
In the following, we discuss the behavior of player 1.
Given the probability that player 2 runs for a position is t,



(2)
Comparing the payoff arranged in Equation (2) with
the payoff of campaigning inactively
f
r, type-splayer
1 will campaign actively (inactively) when






1tfrbre

 

The expected payoff of type-w player 1 when he cam-
paigns actively is:
.





 
1te dbtr brre dtb

  (3)
Comparing the payoff for player 2 presented in Equa-
tion (3) with the zero payoff of campaigning inactively,
we conclude that type-w player 1 is determined to cam-
paign actively for the reason that e – b > 0 and r > e.
Summing up all the above arguments, the four sequential
equilibriums are listed in Table 2.
Table 1. The Bayesian Nash equilibrium of model 1.
e – b > fr e – b = fr e – b < fr
A1 ≥ 0 (run, AA)(run, AA) or (run, IA) (run, IA)
A3 ≥ 0 > A1
player 1:
not run
If the probability that player 2 selects
(IA) is larger, player 1 is very likely
to run for a position. If the probability
that player 2 selects (AA) is larger,
player 1 tends to not run for a position.
(run, IA)
A3 < 0 player 1: not run
b,
r
e
inactivem
inactive
active
active
2
run
not run
q
1 – q
run
not run
(e
–
–
–
a)
(e + d – b, r – e – a)
(r – b, 0)
1
s
1
N
w
(
r
b
–
,
0
)
(fr, r – a)
(0, r – a)
Figure 3. The extension form game of model 2.

J.-S. WANG, M.-Y. LIN
656


10ra qe
Table 2. The sequential equilibrium of model 2.
Strategy of player 1
Strategy of player 2
(when player 1
campaigns actively)
Belief Required
condition
(1) type-s: active
type-w: active run μ = q r – e > a e – b ≥ fr
(2) type-s: inactive
type-w: active run μ = 0 r – e > a e – b < fr
(3) type-s: active
type-w: active not run μ = q r – e ≤ a r – b ≥ fr
(4) type-s: inactive
type-w: active not run μ = 0 r – e ≤ a r – b < fr
As Tab le 2 indicates, the required conditions for these
four equilibriums are unrelated to the proportion of each
type in player 1, but have relationships with the payoff of
each player. We take the case of player 2 for example. If
the left vote, which has subtracted the amount player 1
receiving from the total vote the two players getting, is
larger (smaller) than cost of electoral actions, player 2
will (not) run for a position (i.e.


re a ). In addi-
tion, the decision of player 1 is more complicated. Type-w
player 1 will campaign actively without doubt. For type-s
player 1, considering the state that the opponent runs for
a position, if the expected payoff of competing


eb

is larger (smaller) than that of not competing

f
r, type-s
player 1 will campaign actively (inactively). Moreover,
knowing that the opponent will not run for a position if
player 1 campaigns actively, type-s player 1 will really
campaign actively when expected payoff of competing
alone


rb is larger than that of leaving the opponent
competing alone

f
r. This leads to the following propo-
sition.
Proposition 2. The sequential equilibrium in this model
have no connection with the proportion of each type in
the other party. The candidate without nomination will
(not) run for a position if


re a


r
. The decision
maker of the other party with type-w will campaign ac-
tively certainly. Type- player will campaign actively
(inactively) if when the opponent runs for
a position. And Type- player will campaign actively
(inactively) if when the opponent doesn’t
run for a position.
s

eb fr
s

rb f
Now we compare the equilibriums in these two models.
For the decision maker of the other party, the equilibrium
strategies are identical in these two models when the op-
ponent runs for a position. Namely, type-w player will
campaign actively certainly. The decision of type-s player
is based on the comparison between the values of e – b
and fr when the opponent runs for a position, and the
values of r – b and fr when the opponent doesn’t run for
a position. Consider the equilibrium strategy of the can-
didate without nomination. He decides to run for a posi-
tion if

 
0rae
when he moves first, and if

 10q

eb fr
when he moves late. Since , can-
didate without nomination is more likely to run for a po-
sition when he moves first. This draws the following pro-
position.
Proposition 3. The probability of the candidate without
nomination to run for a position is larger when he moves
first. The decision strategy of the decision maker of the
other party is identical when the opponent is running for
a position, regardless of who moving first.
Proposition 3 may result from the fact that the first mov-
ing candidate without nomination has incomplete infor-
mation of the other party. Thus his decision is based on
the prior belief in the distribution of the opponent’s type
and it leads to him facing more risk.
Because of the property of proposition 3, the decision
maker of the other party with type-s hopes to stand in the
state of model 1 when he tends to campaign inactively. In
other words, the decision maker of the other party with
type-s wants the opponent to move first to raise the prob-
ability of the opponent to run for a position. In reality, the
decision maker of the other party can delay the nominat-
ing date (campaigning inactively) to stimulate the oppo-
nent to run for a position. On the contrary, the type-s player
hopes to be in the state of model 2 when he prefers to
campaign actively. It will decrease the probability of the
opponent to run for a position. That is, the decision maker
of other party will finish the nominating process as soon
as possible (campaigning actively) which reduces the op-
ponent’s intention of running for a position. Thus we may
go on from this to the proposition 4.
Proposition 4. If , the decision maker
of the other party with type-s prefers to move late (first).
4. Conclusions
This paper applies a game model to analyze the interact-
tion in strategy when there is a candidate who renounces
membership of a party to announce running for a position.
We discuss two models in which the candidate without
nominations moves first and the decision maker of the
other party moves first respectively. The main finding is
that the equilibrium strategy of the decision maker of the
other party is identical when the opponent is running for
a position, regardless of who moving first. However, the
probability of the candidate without nomination to run
for a position is larger when he moves first. Therefore,
the decision maker of the other party may prefer to move
first if he wants to campaign actively.
In this paper, we set some simplified assumption which
can be relaxed. First, the candidate without nomination may
split the vote which belongs to the original party. More-
over, if the two players can negotiate in advance, the
negotiation result may change the strategy of the player.

J.-S. WANG, M.-Y. LIN 657
However, this paper would be a good stepping-stone for
the future study about this topic. And it provides the sug-
gestion in strategy for the candidate or the party who
would deal with such issue.
REFERENCES
[1] L. D. Epstein, “Political Parties in Western Democra-
cies,” Praeger, New York, 1967.
[2] M. Gallagher, “Conclusion,” In: M. Gallagher and M.
March, Eds., Candidate Selection in Comparative Per-
spective: The Secret Garden of Politics, SAGE Publica-
tions, London, 1988.
[3] A. Ware, “Citizens, Parties and the State,” Princeton Uni-
versity Press, Princeton, 1988.