Paper Menu >>
Journal Menu >>
 International Journal of Astronomy and Astrophysics, 2012, 2, 174-179 http://dx.doi.org/10.4236/ijaa.2012.23021 Published Online September 2012 (http://www.SciRP.org/journal/ijaa) Kruskal Dynamics for Radial Geodesics Abhas Mitra1,2 1Theoretical Astrophysics Section, Bhabha Atomic Research Centre, Mumbai, India 2Homi Bhabha National Institute, Mumbai, India Email: amitra@barc.gov.in Received February 25, 2012; revised March 28, 2012; accepted April 24, 2012 ABSTRACT The total spacetime manifold for a Schwarzschild black hole (BH) is believed to be described by the Kruskal coordi- nates and , where r and t are the conventional Schwarzschild radial and time coordinates re- spectively. The relationship between r and t for a test particle moving along a radial or non-radial geodesic is well known. Similarly, the expression for the vacuum Schwarzschild derivative for a geodesic, in terms of the constants of motion, is well known. However, the same is not true for the Kruskal coordinates; and, we derive here the expression for the Kruskal derivative for a radial geodesic in terms of the constants of motion. In particular, it is seen that the value of =,uurt =,vvrt dd =1uv ) is regular on the Event Horizon of the Black Hole. The regular nature of the Kruskal derivative is in sharp contrast with the Schwarzschild derivative, dd =tr , at the Event Horizon. We also explicitly obtain the value of the Kruskal coordinates on the Event Horizon as a function of the constant of motion for a test particle on a radial geodesic. The physical implications of this result will be discussed elsewhere. Keywords: Black Hole; Kruskal Coordinates; Spacetime Singularity 1. Introduction It is known for more than 90 years that the region exterior to a point mass or the event horizon () of a Schwarzschild Black Hole (BH) can be described by the vacuum Schwarzschild metric[1,2]: >=2 g rr m 22 22 d= dddd tt rr sgtgrg g 2 (1) where =1 2 tt g mr, 1 =12 rr g mr ,2 = g r , and 22 =sin gr (). Here, we are working with a spacetime signature of ==1Gc 1, 1, 1, 1 and r has a distinct physical significance as the invariant area radius; i.e. 2 =4π A r =0A 2m =2rm indicates invariant/scalar area of sym- metric 2-surfaces. Clearly, then, by definition, the point mass having is at , and hence, the scalar r should continue to be a space-like coordinate even for . The coordinate time t too has a physical sig- nificance as the proper time of a distant inertial observer . At , rr =0r r S g blows up and as , the tt <2rm g and rr g suddenly exchange their signatures and the metric acquires a signature 1, 1, 1, 1 . Such an in- version of signature appears to be unphysical because (1) by, definition, r is spacelike and (2) by principle of equivalance, there must be a local inertial frame where the metric is Lorenzian with the original signature . Further though, the signature of 1, 1, 1, 1 rr g Horizon, =2rm, the signatures of would change if there would be region beneath the Event g and g remain un. Despite such fundaml phys inconsistencies, in the black hole (BH) paradigm, it is believed that the EH is merely a coordinate singularity because the Kretschmann scalar is finite there. But it can easily be seen that, 4 EH changedenta ical K m [3], and the idea that E H K is finite presume integration constant ring in the vacuum Schwarzschild solution =2m es that th appea , is finite. However, it has been shown that, =2 >0m though is indeed finite for an object with radius ch as the Sun or a neutron star, =2 =0m>2 b rm, su utral point particle with =0 b r [4 order to progress, we ignore this scientific fact and continue to work in the artificial and incorrect mathe- matical paradigm of BHs. The detail dynamics of a for a ne-7]. Yet, in “test particle” in the vacuum external spacetime is well known for a very long time and discussion on it is contained in practically every text book or monograpgh on classical General Theory of Re- lativity (GTR) [1,2]. One of the key aspects for studying the kinematics of a test particle is the knowledge about the relevant derivative of the spatial coordinate with the temporal one. For instance for any geodesic having angular momentum or not, one knows the details about the behaviour of the Schwarzschild derivative ddrt or ddtr. And the fact that dd=tr blows the up at C opyright © 2012 SciRes. IJAA  A. MITRA 175 Event utility of othe beenlaimed since 1960 that bo Horizon restricts thethe Schwarzschild dynamics below the EH. This tantamounts to the well known fact that the vacuum Schwarzschild metric fails to describe the spacetime inside 2rm. Some authors, accordingly, swap the nomenclar and t inside the EH. But this is inconsistent because the location of the (1) EH is still denoted by =2rm and not by =2tm and (2) by initial definitionoint mass re- =0r and not at =0t. On ther hand, it has c tures , the p sides at th the exterior and the interior regions of a BH may be described by a one-piece coordinate system suggested by Kruskal and Szekeres [8,9]. In this case too, the nomen- clatures of r and t remain unchanged for <2rm unlike what is done b some authors in a desperat to uphold the BH paradigm. Though, in the intervening 42 years hundreds of articles have been written on Kruskal coordinates, and most of the treatises on GTR too regularly deliberate upon the original work of Kru- skal and Szekers, the fact remains that sufficient effort has not been made to study the kinematics of a test particle in terms of the Kruskal coordinates so that one could have a better insight and appreciation of the kinematics inside the EH. Accordingly, here, we would derive the expressions for the Kruskal derivative ye move dduv for a radial geodesic. For the sake of completene shall start from the usual description about the Kruskal coordinates and first derive the exact expression for the value of the Kruskal coordinates on the EH ( ss, we H u and H v) in terms of r and t. We shall show here t hat H u and H v are alwas non-zero and finite in general. M importantly, we shall explicitly show that unlike the Schwarzschild derivative, the Kruskal derivates are re- gular on the EH. Apparently this might be in accor- dance with the singularity free nature of the Kruskal coordinates. However, in a later paper, we shall show that this is, ironically, not the case. y ore 2. Kruskal Coordinates are defined by pre- crepen First note that, Kruskal coordinates suming that the integration constant appearing in the vacuum Schwarzschild solution is non-zero in variance with the actual result that, even though for an object with >2rm (such as the Sun) >0m, for a point mass [3-7]. Yet, in this papeignore this basic dis- cy. For the region exterior to the EH (Sectors 1 & 3), the Kruskal coordinates are defined as follows [1,2]: =0m r, we 1 1 =cosh; 4 =sinh; 2 4 t 12 4 1=1 2 rm r fr e m (3) Here the plus sign corresponds to “our universe” while th r e negative sign corresponds to the “other universe” [1, 2]. The “other universe” is a legitimate mathematical solution of the Schwarzschild problem irrespective of its observational reality (under the assumption >0m), and is a time reversed mirror image of “our universe”. And for the region interior to the horizon (Sectos 2 & 4), we have 2 2 =sinh; 4 =cosh; 2 4 t ufrm t vfrr m m (4) where 12 4 2=1 2 rm r f re m (5) In terms of u and v, the metric for the entire spacetime is 3 222 22 2 2 32 d=d d dd sin rm m2 s evu r r (6) The metric coefficients are regular everywhere (under th ng zschild For n a radial geodesic, the angular mo- e assumption >0m) except at the intrinsic singularity =0r. Since aftee Kruskal coordinates are defined usi r and t, for a proper understanding of the Kruskal dynamics, it is necessary to recall the inter-relationship between the Kruskal and Schwarzschild coordinates for a radial geodesic. 2.1. Inter Relation between Schwar rall th Coordinates a test particle o mentum is zero, and there is only one conserved quantity, the energy of the particle (per unit rest mass), E, as measured by a distant inertial observer: d12 t Emr ds (7) where s is the proper time. For a massless particle like a photon, we have =E , otherwise E is finite. For a radial geodesic, the mn of the particle is determined by (see Chandrasekhar, p. 98) [10] otio 2 d=1 rEm ufr m t vfrrm m (2) where 2 dr s (8) and d= d12 tE s mr (9) Copyright © 2012 SciRes. IJAA  A. MITRA 176 so that 1 2 12 d= d12 Emr t rEmr (10) Clearly as 2rm, ddtr. The latter relation dd=0rt mcating that the r has a minimum at 2mmum of r i ind ay be indi e mi. But since thnis =0r, it may be icating that, for a point mass 2=0m [3-6]. However, we shall ignore this indication in this paper. Here note that if the particle is released from rest (dd=0rs ) at =i rr at =0t, from Equation (8), it is seen that [10] 2=1 2i Emr (11) or, 1 2 2=1 i rm E (12) It is convenient to introduce a (cyclic) parameter through 22 2 2 2 =2 =2 cos cos 1i mr E (13) Obviously, =1cos i r r =0 when and at the EH, we have =i rr ==2arcsin;=2 HEr m (14) ome ion at the following Equation involving and Now after smanipulat, Chandrasekhar arrived t [10]: 12 4 22 cos 2 d= d22 2 cos cos i r tEm (15) H This Equation can be integrated to find the relation between t and r for a radial geodesic (actually, even for exact non-radial geodesic this Equation would hold good): 32 2 1 =sin1 222 i r tEE mm tan2 tan2 ln tan2 tan2 H H (16) The above Equation may also be written without intro- ducing H and explicitly: (see p. 824 of ref.[1] or p. 34 E 3 of ref. [2]): 12 12 12 21 1 1sin 24 ii ii rm rr rr mm 12 12 211 =ln 2 ii rm rr t m (17) We find from Equations (16) and (17) that, as from Sector 1, the logarithmic term blows up and , which is a well known result. Further Kruskal envisage that approach to the EH s III & IV corresponds to zon lation 2rm t coordinates Sector 2.2. Kruskal In from the =t . Coordinates on the Event Hori Sectors 1 & 3, Kruskal coordinates obey the re =coth4 ut vm (18) And since 2rm correspondts to , at the have EH, we =1; = 2 a 2 H urm (19 H v) other hand, in Sectors On the nd 4, we see =tanh4 vm (20) and as r ut , we are led to 2r m, the same limit, m that 2 (19). t In the sameEquation we find and t, 22 2 1 =exp HH t uv f 2m (21) ght appear that since we wo have to ain the actual It mi 2f he EH values of = 2=0m fm on 12 . Ae has the EH, uld==0 HH uv. But this is incorrect because the temporal part of u and v tends to blow up much more rapidly on tnd on carefully obt H u and H v by g out appropriate lim so we introduce a new workin To do its variable 2 2 =2 i zr E (22) and, let, in the vicinity of the EH, 1= 1 mE 2=1 ;0rm (23) so that 22 i fm H, by retai (24) Then, in the vicinity of the Ening terms first order in , we can rewrite Equation (17) as 12 12 12 12 14 =ln 14 i i r zz tmz r mzz 12 sin 21 4 ii rr mm 2 mz (25) As 0 , the logarithmic term in the above ex- on becomes pressi Copyright © 2012 SciRes. IJAA 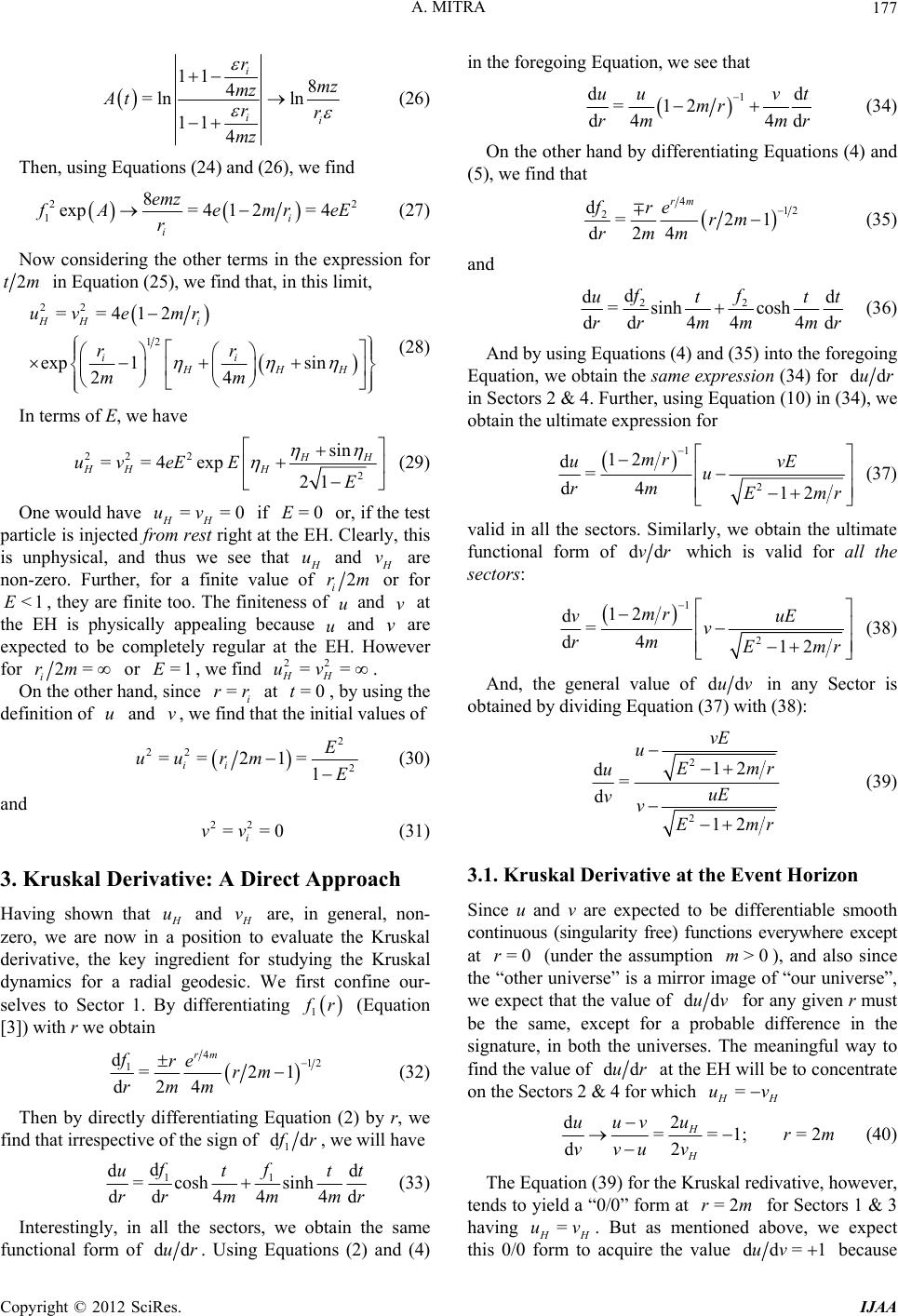 A. MITRA 177 11 8 4 =ln ln 11 4 i ii rmz mz At rr mz (26) Then, using Equations (24) and (26), we find 22 1 8 exp= 412= 4 i i emz f AemreE r (27) Now considering the other terms in the expression for 2tm in Equation (25), we find that, in this limit, 22 12 ==412 exp 1sin 24 HHi ii HHH uv e mr rr mm (28) In terms of E, we have 22 2 2 sin ==4exp 21 E e would have ==0 HH uv if =0E or, if t HH HH H uv eE E (29) On he test particle is injected is unphysical, and t see that from rest hus we right at the EH. Clearly, this H u and H v are non-zero. Further,te value for a fini of 2 i rm and v v Howe or for <1E th ex for , they are finite at e EH is physically apb and are r. ver e too. The finit pealing regula ness of ecause u at the E u Hpected to be completely 2= i rm or =1E, we find22 HH uv== . usinOn the other ha definition of and , we find that the iitial values of nd, since =rr i at =0t, byg the u vn 2 22 2 == 21= 1 ii E uu rmE (30) and 22 ==0 i vv 31) 3. Kruska l Derivative: A Direct Approach Ha ( ving shown that H u and H v sic. rentiating aregeneral, non- zero, we are now in a position to evaluate the Kruskal derivative, thkey inredient for studying the Kruskal , in e dynamics for a raodeWe first con selves to Sector 1.diffe g dial ge By fine our- 1 f r (Equation [3]) with r we obtain 4 1 d= 2 d24 rm fre rm rmm we 12 1 (32) Then by directly differentiating Equation (2) by r, find that irrespective of the sign of 1 dd f r, we will have 11 d dd =coshsinh dd 44 4d ff ut tt rrmm mr (33) Interestingly, in all the sectors, we obn the same functional form of tai ddur. Using Equations (2) and (4) in the foregoing Equation, we see that 1 dd =12 d t r (34) d4 4 uu v mr rm m On the other hand by differentiating Equations (4) and (5), we find that 412 2 d= 21 d24 rm fre rm rmm an (35) d 22 d dd =sinhc dd 44 ff ut tt rr mm osh 4dmr (36) And by using Equations (4) and (35) into the Equation, we obtain the same expression (34) for foregoing ddur in e expression for Sectors 2 & 4. Further, using Equation (10) in (34), we obtain the ultimat 1 2 12 d= d4 12 mr uv u rm Em E r valid in all the sectors. Similarly, we obtain the ultimate functional form of (37) ddvr which is valid for sectors: all the 1 2 12 d= d4 12 mr vuE v rm Emr (38) dduv 37) with And, the general value ofin any Sector is obtained by dividing Equation ((38): 212 d= d Emr uuE v (39) 212 vE u vEm r 3.1. Kruskal Derivative at the Event Horizon Since u and v are expected to be differentiable smooth co also since universe” is a mirror im “our universe”, hat the value of ntinuous (singularity free) functions everywhere except at =0r (under the assumption >0m), and the “other we expect t age of dduv for a bable di he m ny given r must e, except for a pro in both the universes. Teaningful way to fin value of be the sam signature, d the fference in the ddur at the EH o concentrate onSectors 2 & 4 for which will be t = the H H uv 2 d==1; =2 H u uuv rm ( d2 H vv uv The Equation (39) for the Kruskal redivative, however, tends to yield a “0/0” form at =rmfor Sectors 1 & 3 having = 40) 2 H H uv. But as mentioned above, we expect this 0/0 form to acquire the valuedd=1uv because Copyright © 2012 SciRes. IJAA  A. MITRA 178 these Scetors are the mirror images of Sectors 2 & 4. Otherwise the whole idea of having an extended time symmetric Schwild manifold would be incon- sistent. Thus, in general, we must arzsch have dd=1; =2uvr m despite the 0/0 forms in some sectors. The fact that we m (41) ust have dd=1uv for the Sectors 1 & 3 can be reconfirmed in the limiting case of 22 == HH uv for =1E o=0 H for the (unphysical case) =0E directly by using L’ Hospital’s theore Note that, by this rule, we can write, r m = H uv . 0,0 0,0 dd = limlim dd uv uv uur vvr (42) In any case, from Equation (19), we already know that =1uv at . Then we can rearr=2rm ange the fore- going Equation as 0,0 dd =1 lim dd uv ur vr (43) or, 2 du=1 lim d rm v (44) 4. It may bome in A Different Route e of sterest to rederive the limiting value of dduv by using other generic relationships between u and v. As before, to avoid 0/0 forms, we work with Sectors 3 & 4. In particular, in Sector 3, we have =coth4 ut vm (45) By differentiating this equation w.r.t. v, we obtain 2= dvvv 2 1d11 d 44d sinh uu t mtm v (46) By recalling that 1 sinh 4=tm vf, we rewrite the aboation as ve equ 2 1 d1d = d4d f uu t vv mvv (47) Now, from Equations (10) and (38), note that 2 ddd 4 == ttr mE dd d12 vrv vEmr uE(48) And the limiting value of d4 42 == d 2 H H H tmm vuvuuu rm (49) And since , we find from Equation (47) that m 12=0fm d=0; =2 dvv uu rm (50) Or, d =2 S vv rm d== =1; ectors 34 H H uu u u (51) Similarly, for the sake of overall consiste Sectors, 1 & 3, we must have ncy, in dd=1uv at 5.nt Consi ally we could have obtained the above derived unique result in a relatively simpler ma rentiating the Global Equation =2rm. A Differederation Actu nner by diffe- 22 2 =21 rm uvrme (52) w.r.t. v: 2 2 dd 2 2= dd 4 rm urr uv e vv m (53) First let us note from Equation (38) that in Sectors 2 & 4, the limiting value of 412 dmmr r d 21 2=0; H vv u mmr v (54) 2rm Then, by using this above Equation in (53), we find d=; 2 d HH u uvr v m (55) so that d= d v u vv =1;2 H H rm (56) 6. Conclusions The Kruskal coordinates were found w and in the present paper, we have worked out some aspects of the kinematics of a test particle following a rahe precise ay back in 1960, dial Kruskal geodesic. To attain this we used t value of H u and H v i ras a function of the initial conditions of or found that the problem 22 , m E. It is clearly = H H uv eeded is no ge hen toe esion for the Kruskal derivative in terms of and r. We found that the Kruskal derivative is ree EH Scharzschild deivative(s) wher n-zer obtai o in n th neral. xpresWe t procm, gu e E lar at th unlike the ddtr= at the EH. In particular dd=1uv at the EH if we consider the Copyright © 2012 SciRes. IJAA  A. MITRA Copyright © 2012 SciRes. IJAA 179 ose existence is suggested by the full Kreitler, Ed., Focus on Black Hole Research, Nova, New ,” Advances in Space Re- pp. 2917-2919. “other universe” wh York, 2006. [4] A. Mitra, “On the Non-Occurrence of Type I X-Ray Bursts from the Black Hole Candidates search, Vol. 38, No. 12, 2006, Kruskal manifold, and which is a time reversed version of “our universe”. But, if we move to the “our universe”, the expected value of dd=1uv at the EH. The apparent regular nature of the Kruskal derivative may be seen to be in keeping with the notion that Kruskal coodinates are free of singularities at the EH. However, in a subsequen we will delve into this question, and fin doi:10.1016/j.asr.2006.02.074 [5] A. Mitra, “Quantum Information Paradox: Real or Ficti- tious,” Pramana, Vol. 73, No. 3, 2009, pp. 615-620. doi:10.1007/s t paper,12043-009-0113-9 d out other important features of the Kruskal dynamics vis-a-vis the well known Schwarzschild dynamics. Finally, this manuscript is a revision of the Cornell Univ. Electronic Preprint (arXiv: gr-qc/9909062) which has been vie readers in t s, Vol. 50, . ole, ysics and Space Science, [6] A. Mitra, “Comments on ‘The Euclidean Gravitational Action as Black Hole Entropy, Singularities, and Space Time Voids’,” Journal of Mathematical Physic No. 4, 2009, Article ID: 042501 wed by manyhe past 13 years. REFERENCES [1] C. W. Misner, K. S. Thorne and J. Wheeler, “Gravita- tion,” Freeman, San973. [7] A. Mitra, “The Fallacy of Oppenheimer Snyder Collapse: No General Relativistic Collapse at all, No Black H No Physical Singularity,” Astroph Vol. 332, No. 1, 2011, pp. 43-48. doi:10.1007/s10509-010-0578-5 [8] M. D. Kruskal, “Maximal Extension of Schwarzschild Metric,” Physical Review Letters, Vol. 119, No. 5, 1960, Fransisco, 1 [2] S. L. Shapiro and S. A. Teukolsky, “Black Holes, White Dwarfs and Neutron Stars: The Physics of Compact Ob- jects,” Wiley, New York, 1983. doi:10.1002/9783527617661 [3] A. Mitra, “Black Holes or Eternally Collapsing Objects: A Review of 90 Years of Misconceptions,” In: pp. 1743-1745. [9] P. Szekeres, “On the Singularities of a Riemannian Ma- nifold,” Math. Debreca., Vol. 7, 1960, p. 285. [10] S. Chandrasekhar, “The Mathem Holes,” Clarendron, Oxford, 1983. P. V. atical Theory of Black |

