International Journal of Astronomy and Astrophysics, 2012, 2, 156-166
http://dx.doi.org/10.4236/ijaa.2012.23019 Published Online September 2012 (http://www.SciRP.org/journal/ijaa)
A Magnetic Model for Low/Hard States Associated with
Jets in Black Hole X-Ray Binaries
Jiuzhou Wang1, Dingxiong Wang1, Zhaoming Gan2, Changyin Huang1*
1School of Physics, Huazhong University of Science and Technology, Wuhan, China
2Shanghai Astronomical Observatory, Chinese Academy of Sciences, Shanghai, China
Received April 19, 2012; revised May 22, 2012; accepted May 30, 2012
ABSTRACT
A model for the low/hard (LH) state associated with a steady jet in black hole X-ray binaries (BHXBs) is proposed
based on disc-corona model with open magnetic fields trapped in magnetic patches, which arises from “flux expulsion”
effect of convective turbulence. We fit the spectral profiles of the LH state for the BHXBs, 4U 1543-475, GX 339-4,
XTE J1550-564 and GRO J1655-40, and fit the relation between jet power and X-ray luminosity dynamically in the LH
state by adjusting accretion rate and the outer boundary of the corona over the disc.
Keywords: Accretion; Accretion Disks; Black Hole Physics; X-Rays; Binaries; Magnetic Fields
1. Introduction
Spectral states observed in black hole X-ray binaries
(BHXBs) involve a number of unresolved issues in as-
trophysics, displaying complex variations not only in the
luminosities and energy spectra, but also in presence/
absence of jets and quasi-periodic oscillations (QPOs).
Belloni [1] classified the states of BHXBs as low/hard
(LH) state, hard intermediate state (HIM), soft interme-
diate (SIM) state and high/soft (HS) state, which display
different luminosity and hardness associated with diffe-
rent behavior of QPOs and radio loudness. McClintock &
Remillard [2] used four parameters to define X-ray states
based on the very extensive RXTE data archive for
BHXBs, in which three states, i.e., thermal-dominant
(TD) state, low/hard (LH) state and steep power law
(SPL) state are included. In TD state, the flux is domi-
nated by the thermal radiation from the inner accretion
disk, and QPOs are absent or very weak. The spectral
profile of LH state is characterized by a hard power law
component at 2 - 20 keV with a photon index Γ in the
range and an exponential cut-off at about 100
keV, which is associated with a quasi-steady radio jet.
SPL state has a strong power-law component with
, which is associated with high-frequency QPOs.
Although a consensus on classification of spectral states
of BHXBs has not been reached, it is widely accepted
that these states can be reduced to only two basic states,
i.e., a hard state and a soft one, and jets can be observed
in hard states, but cannot be in soft states.
1.4 - 2
.5~2
Just like classification of spectral states agreement has
not been reached on modeling the spectral states of
BHXBs. The accretion flow in LH state is usually sup-
posed to be a truncated thin disk with an inner advec-
tion-dominated accretion flow (ADAF) in the prevailing
scenario [2-5], and henceforth this model is referred to as
truncated ADAF model. Generally speaking, the thermal
component of the spectra of BHXBs can be well fitted by
a standard thin accretion disk, while the non-thermal
component can be interpreted by invoking thermal Com-
ptonization, which originates from either a hot disk
(self-Compton process in ADAF) or disk-corona (exter-
nal Compton process). Although similar spectral profiles
of BHXBs can be fitted either by disk-corona model or
by truncated ADAF model, there exists an essential diffe-
rence between the two types of models, i.e., the cold disk
remains at or near to the innermost stable circular orbit
(ISCO) in disk-corona model, not appearing to truncate
beyond ISCO as in ADAF model.
Disk-corona model seems to be supported by a number
of recent observations. For example, XMM-Newton ob-
servations of GX 339-4 show that a broad iron line
together with a dim, hot thermal component was present
in its spectra during the hard state. This effect seems to
be observed in a few other sources such as Cygnus X-1,
and SWIFT J1753.5-0127 [6,7]. Recently, Reis, Miller &
Fabian [8] studied the Chandra observation of XTE
J1118+480 in the canonical LH state, and a thermal disk
emission with a temperature of approximately 0.21 keV
is found at greater than the 14 level, and they concluded
that this thermal emission most likely originates from an
*Corresponding author.
C
opyright © 2012 SciRes. IJAA

J. Z. WANG ET AL. 157
accretion disk extending close to ISCO. The results of
fits made to both components strongly suggest that a
standard thin disk remains at or near to ISCO, at least in
bright phases of the LH state. Very recently, Reis, Fabian &
Miller [9] presented an X-ray study of eight black holes
in the LH state, and they found that a thermal disk
continuum with a color temperature consistent with
is clearly detected in all eight sources and the
detailed fits to the line profiles exclude a truncated disk
in each case.
4
LT
One of the main characteristics of the LH state of
BHXBs is its association with quasi-steady jets. Although
different mechanisms have been proposed for inter-
preting jet production in black hole systems of different
scales, such as the plasma gun [10], the cosmic battery
[11] and the magnetic tower [12], the most promising
mechanism for powering jets is magneto-centrifugal
mechanism, which relies on an accretion disk threaded
by a poloidal, large-scale magnetic field [13-16].
Recently, Gan et al. [17] proposed a disk-corona mo-
del for BHXBs, in which the closed magnetic field lines
pipe the hot matter evaporated from the disk, and shape it
in the corona above the disk to form a magnetically
induced disk-corona system. Later, we combined epicy-
clic resonances with the disk-corona model given by [17]
to interpret the high frequency QPOs with 3:2 pairs
observed in SPL states of the three BHXBs, i.e., GRO
J1655-40 (450, 300Hz), XTE J1550-564 (276, 184Hz)
and GRS 1915+105 (168, 113 Hz) [18]. Although some
spectral profiles have been fitted in the LH state, a clear
association with a quasi-steady jet has not been revealed,
and this becomes a great challenge to the present theo-
retical models.
We notice that corona is a perfect launching site for
outflow from accretion disk [19], and disk-corona model
provides a possible scenario for interpreting how matter
loading into a jet occurs. In this paper, we intend to
model the LH state of BHXBs based on a disk-corona
model, in which the inner edge of the accretion disk is
assumed to extend to ISCO, and the jets are driven by the
patched open magnetic field suggested by Spruit &
Uzdensky [20]. The reason of taking this kind of mag-
netic field structure lies in the following aspects. 1) The
magnetic patches reduce reconnection across the disk and
thus reduce the outward diffusion of magnetic field; 2)
The magnetic patches lose angular momentum ef-
fectively and thus make the magnetic field drift inward; 3)
The trapped magnetic flux plausibly varies on time-
scales of days or longer, corresponding to the time for
accretion from the outer edge of the disk, and thus is
consistent with the timescales of the state transitions in
BHXBs; and 4) The jet can be driven effectively by the
patched open magnetic field, and the disk luminosity can
be lowered remarkably due to the transfer of energy of
accreting matter into the jet.
This paper is organized as follows. In Section 2, we
present a detailed description of our model, in which the
jet is driven by the patched open magnetic field, and the
coupling of the jet with accretion process is taken into
account based on the conservation of energy and angular
momentum. In Section 3, the spectral profiles of the LH
state of five BHXBs are fitted based on the disk-corona
model, and the relation between jet power and X-ray
luminosity is fitted by adjusting accretion rate and the
outer boundary of the corona over the disk. In Section 4
we propose a scenario of state transitions in BHXBs, in
which a correlation of magnetic field configuration with
the transition from hard to soft states is discussed. In
addition, we suggest that the evolution of the magnetic
field configuration in black hole accretion disk can be
regarded as the second parameter in the state transitions
of BHXBs. Finally, in Section 5, we discuss some issues
related to our model.
Throughout this paper the geometric units G = c = 1
are used.
2. Description of Our Model
In order to interpret the association of the LH state of
BHXBs with a quasi-steady jet, we take the scenario of
magnetic patches suggested by [20], i.e., a large portion
of the net vertical magnetic flux threading a disk gets
concentrated into patches of strong field due to “flux ex-
pulsion” effect of convective turbulence [21,22]. A sche-
matic drawing of disk-corona model with magnetic pat-
ches is shown in Figure 1.
The configuration given in Figure 1 has some advan-
tages on interpreting the LH state of BHXBs. 1) The
outward diffusion of trapped fields by turbulent recon-
nection can be reduced significantly once the magnetic
field locally becomes strong enough to avoid being tan-
gled, and the jet can be driven effectively by the open
large-scale magnetic field concentrated in the patches; 2)
The disk luminosity can be depressed effectively due to
part of energy is transferred into the jet; 3) The corona
geometry is scarcely affected by the magnetic patches,
which distribute dispersedly on the disk and occupy a
small fraction of the disk surface; and 4) The large scale
patched open magnetic field is apt to collimate the jet
from black hole accretion disk. Thus the LH state of
BHXBs associated with a quasi-steady jet can be fitted
based on disk-corona model with the magnetic patches.
As argued in [20], the magnetic field strength
p
B in-
side the patches is estimated by
p
gas MRI
8π,BP   (1)
where


MRI O1
 is a parameter related to magneto-
rotational instability (MRI), and is the gas pressure
gas
P

J. Z. WANG ET AL. 158
Figure 1. A schematic drawing of disc-corona model with
magnetic patches.
dominating over the radiation pressure inside the disk.
According to Balbus & Hawley [23], the interior viscous
process is dominated by tangled small-scale magnetic
field, D, and the viscous pressure is comparable to
magnetic pressure, and thus we assume
B
2
gas magD
~π.
r
tPPB

  8 (2)
In this paper, we introduce two parameters, r

and


, to describe the distribution of magnetic patches in
the disk as follows,
p
p
dd
dd
r
rr

 ,
.








 (3)
In Equation (3) the radial coordinate of the patch
p
r
is related to the disk radius r by the radial fraction factor
r

, and the toroidal coordinate of the patch
p

to the
toroidal coordinate φ of the disk by the toroidal fraction
factor


. Henceforth the subscript “p” represents the
quantities related to the magnetic patches. Thus the
magnetic torque exerted on the magnetic patches is given
by
2
p
pp
d
2πrB A

 d, (4)
where
p
pp
dddArr

 is the area element of the mag-
netic patch, and

is the ratio of the toroidal component
,p
B

to
p
B. The jet power driven by each magnetic
patch is
p
pp
dPd ,


 (5)
where
p
 is the average angular velocity of magnetic
patches. Since the magnetized patch drifts inward with a
speed greater than that of accreting matter,
p
K
should be less than the Keplerian angular velocity, and
is a factor less than unity.
k
k
Incorporating Equations (2)-(5), we have the jet power
within the radius r driven by the open magnetic field of
all patches as follows,

in
22
pppKp
PdP
r
r
rfBr
 d,r (6)
where four parameters ,
k

r

, and


are incur-
porated into pr fk




with 1

.
The flux of angular momentum
p
H
transferred from
the disk into the jet is related to the flux of energy
p
S
by
p
pK
HS , and
p
S can be calculated by


 
1
pp
πdP d,Sr r

4 (7)
Incorporating Equations (1), (6) and (7), we have
p
pgas
2.
H
frP

(8)
Since
p
Pd is the jet power driven by the magnetic
patches in the ring of width dr, the quantities
p
S and
p
H
are respectively the average fluxes of energy and
angular momentum transferred from the ring by the
trapped magnetic fields as shown in Equations (7) and
(8). The coupling of jet and accretion is taken into ac-
count by using the conservation equations of energy and
angular momentum as follows,

†
DD p
4π
†
d
d
M
Eg
r
rQES  (9)

†
d
dML g r


†
Dp
4π, rQL H (10)
where
g
is the viscous torque in the disk, and
DK

 , and the quantities and are the spe-
cific energy and angular momentum given by Bardeen,
Press & Teukolsky [24].
†
E† L
Incorporating Equations (8)-(10), we have the radia-
tion flux from disk as follows,




in
††2
Dg
as
2
2d,
r
r
Fr
fELPrr
r


DA
pD
††
D
dd
Q
r
EL



(11)
 
††
D
D
4π,
dd
EL
g
r
r

 rFr (12)
where DA is the viscously dissipated energy due to
disk accretion, and it reads
Q


in
†††
D
DAD D
2
††
D
dd 1d.
4π
r
r
r
QE
rEL


 
LML
(13)
Inspecting Equation (11), we find that the total radia-
tion flux


F
r is less than DA due to the negative
contribution of the second term at RHS of this equation,
and this term represents the jet effect of reducing the
total radiation. So we expect that disk-corona model with
trapped magnetic fields anchored at the patches can be
applicable to the LH state with a quasi-steady jet ob-
served in several BHXBs, and the detailed fits will be
given in the next section.
Q
According to typical disk-corona scenario, part of the
viscously dissipated energy Q is released as d
Q

in the
disk, emitting eventually as black-body radiation and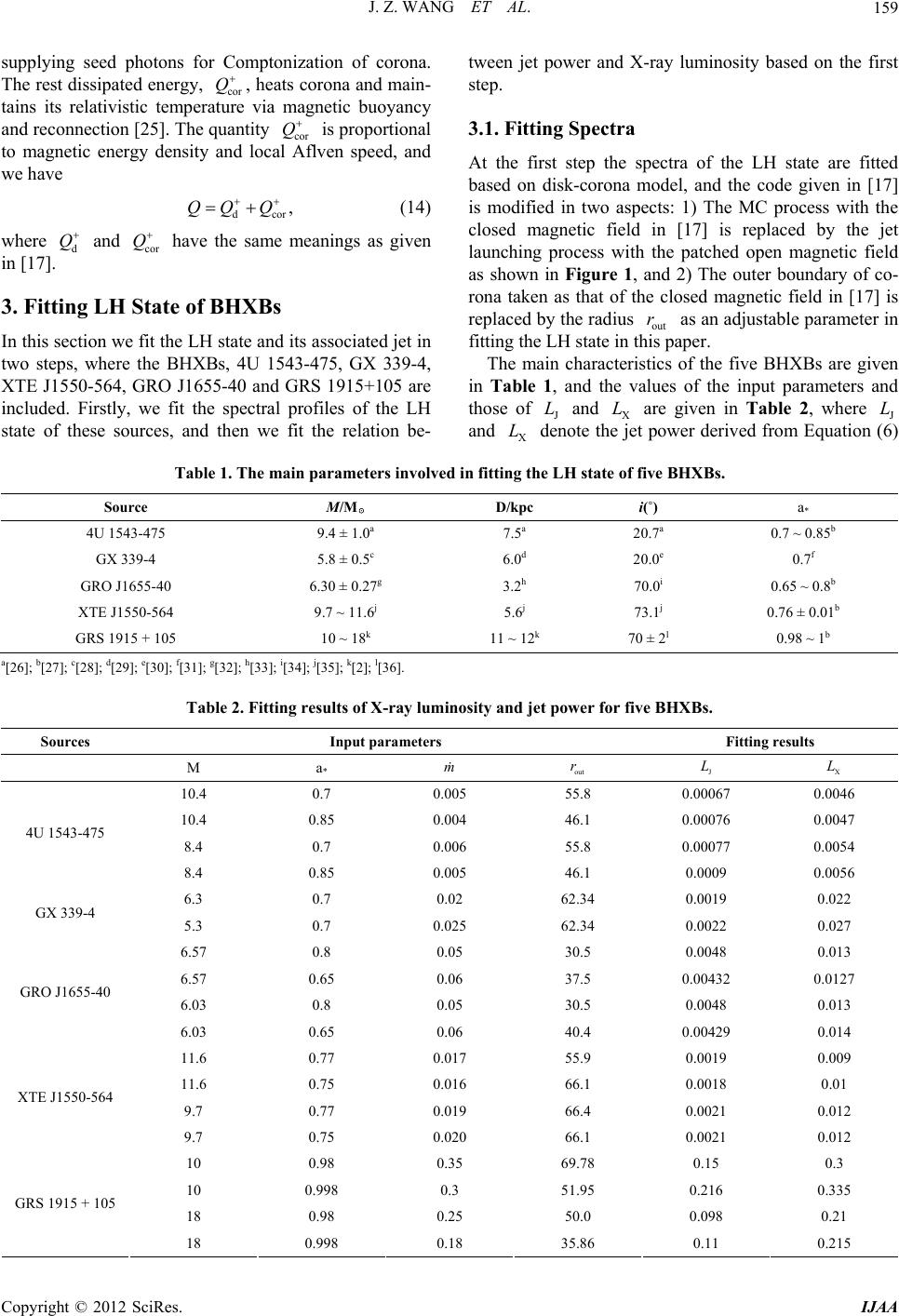

J. Z. WANG ET AL. 159
tween jet power and X-ray luminosity based on the first
step.
supplying seed photons for Comptonization of corona.
The rest dissipated energy, cor Q

, heats corona and main-
tains its relativistic temperature via magnetic buoyancy
and reconnection [25]. The quantity cor is proportional
to magnetic energy density and local Aflven speed, and
we have
Q
cor
,Q

3.1. Fitting Spectra
At the first step the spectra of the LH state are fitted
based on disk-corona model, and the code given in [17]
is modified in two aspects: 1) The MC process with the
closed magnetic field in [17] is replaced by the jet
launching process with the patched open magnetic field
as shown in Figure 1, and 2) The outer boundary of co-
rona taken as that of the closed magnetic field in [17] is
replaced by the radius out as an adjustable parameter in
fitting the LH state in this paper.
r
d

QQ (14)
where and have the same meanings as given
in [17].
d
Q
cor
Q
3. Fitting LH State of BHXBs
In this section we fit the LH state and its associated jet in
two steps, where the BHXBs, 4U 1543-475, GX 339-4,
XTE J1550-564, GRO J1655-40 and GRS 1915+105 are
included. Firstly, we fit the spectral profiles of the LH
state of these sources, and then we fit the relation be-
The main characteristics of the five BHXBs are given
in Table 1, and the values of the input parameters and
those of J and X are given in Table 2, where J
and denote the jet power derived from Equation (6)
L LL
X
L
Table 1. The main parameters involved in fitting the LH state of five BHXBs.
Source M/M☉ D/kpc i(˚) *
a
4U 1543-475 9.4 ± 1.0a 7.5a 20.7a 0.7 ~ 0.85b
GX 339-4 5.8 ± 0.5c 6.0d 20.0e 0.7f
GRO J1655-40 6.30 ± 0.27g 3.2h 70.0i 0.65 ~ 0.8b
XTE J1550-564 9.7 ~ 11.6j 5.6j 73.1j 0.76 ± 0.01b
GRS 1915 + 105 10 ~ 18k 11 ~ 12k 70 ± 2l 0.98 ~ 1b
a[26]; b[27]; c[28]; d[29]; e[30]; f[31]; g[32]; h[33]; i[34]; j[35]; k[2]; l[36].
Table 2. Fitting results of X-ray luminosity and jet power for five BHXBs.
Sources Input parameters Fitting results
M a* m
 out r J
L
X
L
10.4 0.7 0.005 55.8 0.00067 0.0046
10.4 0.85 0.004 46.1 0.00076 0.0047
8.4 0.7 0.006 55.8 0.00077 0.0054
4U 1543-475
8.4 0.85 0.005 46.1 0.0009 0.0056
6.3 0.7 0.02 62.34 0.0019 0.022
GX 339-4 5.3 0.7 0.025 62.34 0.0022 0.027
6.57 0.8 0.05 30.5 0.0048 0.013
6.57 0.65 0.06 37.5 0.00432 0.0127
6.03 0.8 0.05 30.5 0.0048 0.013
GRO J1655-40
6.03 0.65 0.06 40.4 0.00429 0.014
11.6 0.77 0.017 55.9 0.0019 0.009
11.6 0.75 0.016 66.1 0.0018 0.01
9.7 0.77 0.019 66.4 0.0021 0.012
XTE J1550-564
9.7 0.75 0.020 66.1 0.0021 0.012
10 0.98 0.35 69.78 0.15 0.3
10 0.998 0.3 51.95 0.216 0.335
18 0.98 0.25 50.0 0.098 0.21
GRS 1915 + 105
18 0.998 0.18 35.86 0.11 0.215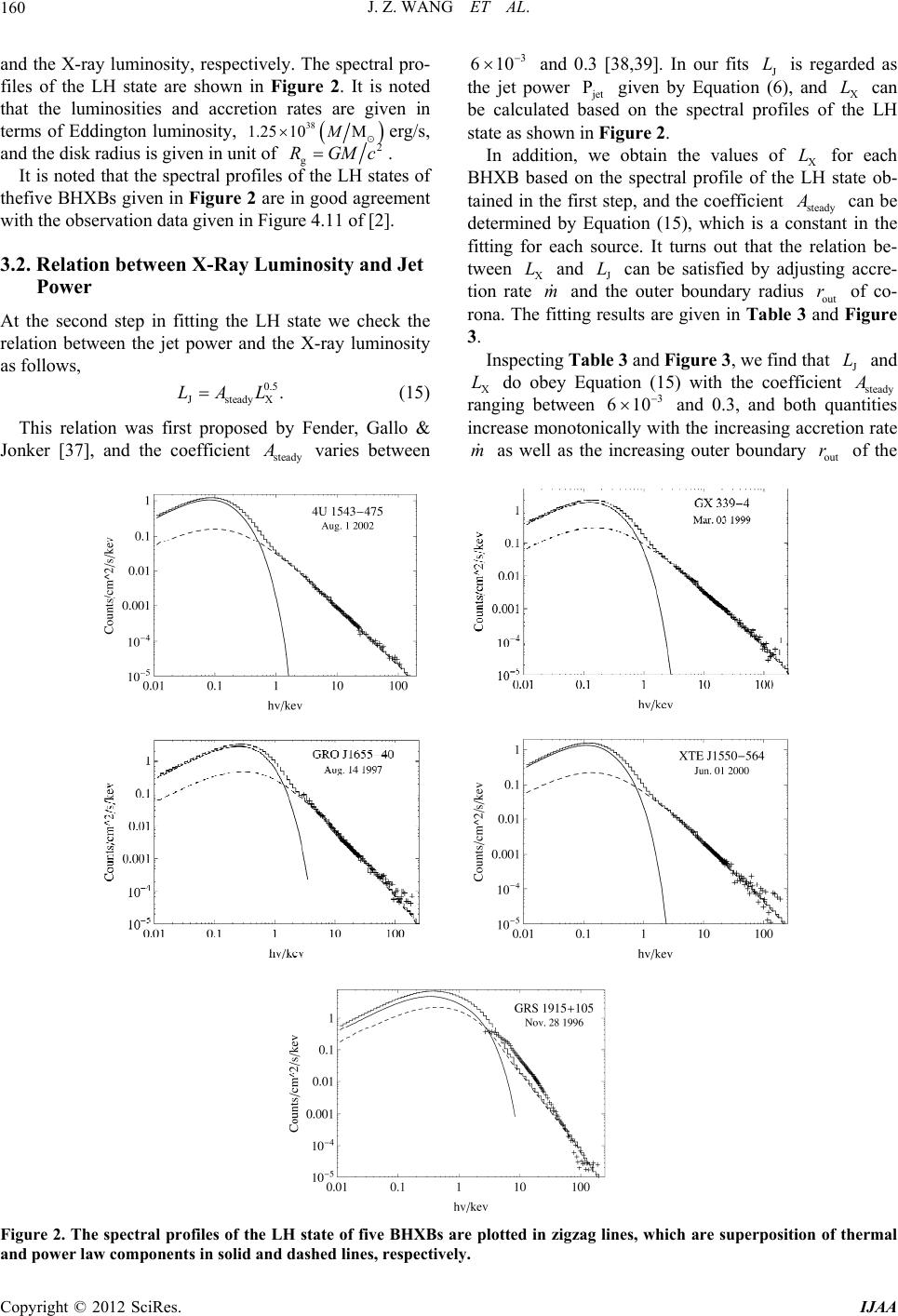

J. Z. WANG ET AL. 160
and the X-ray luminosity, respectively. The spectral pro-
files of the LH state are shown in Figure 2. It is noted
that the luminosities and accretion rates are given in
terms of Eddington luminosity,


38
1.25 10MMerg/s,
and the disk radius is given in unit of 2
g
RGMc.
It is noted that the spectral profiles of the LH states of
thefive BHXBs given in Figure 2 are in good agreement
with the observation data given in Figure 4.11 of [2].
3.2. Relation between X-Ray Luminosity and Jet
Power
At the second step in fitting the LH state we check the
relation between the jet power and the X-ray luminosity
as follows,
0.5
JsteadyX
.LAL (15)
This relation was first proposed by Fender, Gallo &
Jonker [37], and the coefficient varies between
steady
A
3
610

 and 0.3 [38,39]. In our fits J is regarded as
the jet power jet given by Equation (6), and X can
be calculated based on the spectral profiles of the LH
state as shown in Figure 2.
L
PL
In addition, we obtain the values of X for each
BHXB based on the spectral profile of the LH state ob-
tained in the first step, and the coefficient steady can be
determined by Equation (15), which is a constant in the
fitting for each source. It turns out that the relation be-
tween X and J can be satisfied by adjusting accre-
tion rate and the outer boundary radius out of co-
rona. The fitting results are given in Table 3 and Figure
3.
L
A
r
L
m

L
Inspecting Tabl e 3 and Figure 3 , we find that and
X do obey Equation (15) with the coefficient steady
ranging between
J
L
AL
3
610

 and 0.3, and both quantities
increase monotonically with the increasing accretion rate
as well as the increasing outer boundary of the m
out
r
Figure 2. The spectral profiles of the LH state of five BHXBs are plotted in zigzag lines, which are superposition of thermal
and power law components in solid and dashed lines, respectively.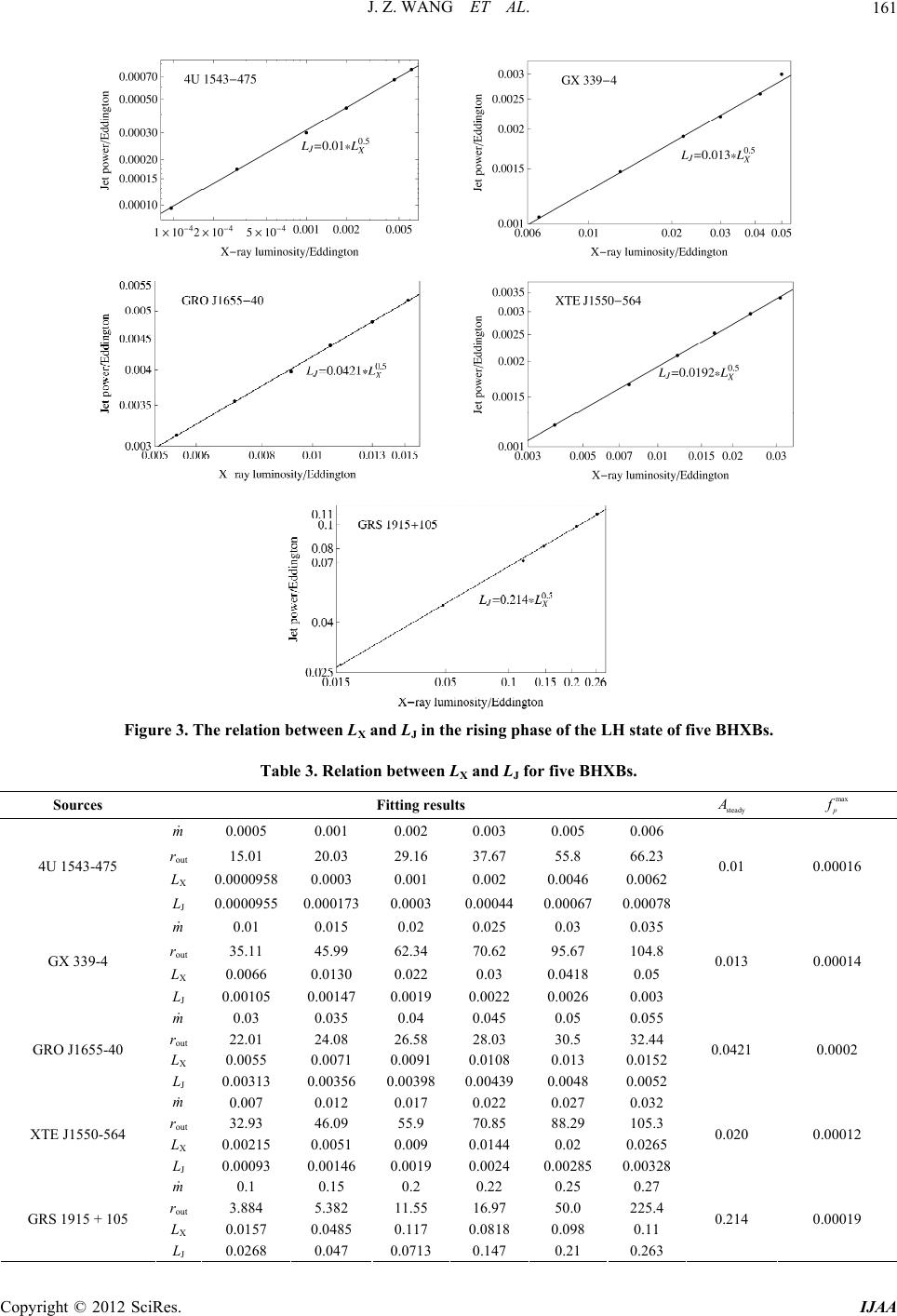

J. Z. WANG ET AL. 161
Figure 3. The relation between LX and LJ in the rising phase of the LH state of five BHXBs.
Table 3. Relation betw ee n LX and LJ for five BHXBs.
Sources Fitting results
steady
A max
p
f
m
 0.0005 0.001 0.002 0.003 0.005 0.006
rout 15.01 20.03 29.16 37.67 55.8 66.23
LX 0.0000958 0.0003 0.001 0.002 0.0046 0.0062
4U 1543-475
LJ 0.0000955 0.000173 0.0003 0.000440.00067 0.00078
0.01 0.00016
m
 0.01 0.015 0.02 0.025 0.03 0.035
rout 35.11 45.99 62.34 70.62 95.67 104.8
LX 0.0066 0.0130 0.022 0.03 0.0418 0.05
GX 339-4
LJ 0.00105 0.00147 0.0019 0.0022 0.0026 0.003
0.013 0.00014
m
 0.03 0.035 0.04 0.045 0.05 0.055
rout 22.01 24.08 26.58 28.03 30.5 32.44
LX 0.0055 0.0071 0.0091 0.0108 0.013 0.0152
GRO J1655-40
LJ 0.00313 0.00356 0.003980.004390.0048 0.0052
0.0421 0.0002
m
 0.007 0.012 0.017 0.022 0.027 0.032
rout 32.93 46.09 55.9 70.85 88.29 105.3
LX 0.00215 0.0051 0.009 0.0144 0.02 0.0265
XTE J1550-564
LJ 0.00093 0.00146 0.0019 0.0024 0.002850.00328
0.020 0.00012
m
 0.1 0.15 0.2 0.22 0.25 0.27
rout 3.884 5.382 11.55 16.97 50.0 225.4
LX 0.0157 0.0485 0.117 0.0818 0.098 0.11
GRS 1915 + 105
LJ 0.0268 0.047 0.0713 0.147 0.21 0.263
0.214 0.00019

J. Z. WANG ET AL. 162
corona. These results are consistent with the rising phase
of transient outburst of the BHXBs.
This relation can be understood roughly in the context
of our model. The X-ray luminosity X arises from the
gravitational energy of the accreting matter released in
the disk accretion, and the jet power J comes from the
kinetic energy of the outflowing particles trapped in the
open magnetic field lines. In fact, the two kinds of lumi-
nosities have the same origin: The gravitational energy of
the matter in accretion disk. The positive exponent in the
correlation implies that the both luminosities increase
with the accretion rate during the LH state, because the
both come from accretion process. However, the reason
for the index ~0.5 remains unclear, since it involves
complicated energy conversion among accretion disk,
corona and outflow.
L
L
4. A Scenario of State Transition in BHXBs
As is well known, state transitions in BHXBs display a
variety of variations not only in luminosities but also in
some spectral characteristics such as hardness and spec-
tral index. The complexity is particularly attractive in the
transition from hard to soft states, with which different
remarkable phenomena are associated. According to [38]
and [1] the transition from hard to soft states can be de-
picted in a more detailed way, e.g. the transition from LH
to HIM and to SIM states occurs successively, being as-
sociated with quasi-steady jet, episodic jet and QPOs,
respectively. A jet line in HID indicates the dividing line
between HIM and SIM states, and the most confusing
behavior in this transition is the occurrence of episodic,
relativistic jets across the jet line.
The fundamental feature in one outburst of BHXBs is
the variation of the luminosity. As shown in HID these
sources always start from “quiescence” of very low lu-
minosity, and then they transit from LH to HIM states
with the increasing luminosity. Afterwards the BHXBs
evolve from HIM to SIM, and from SIM to HS states
with luminosity remaining roughly unchanged. Then
these sources transit from HS to LH states and finally
return to “quiescence” with the decreasing luminosity.
The variation of the X-ray luminosity is interpreted na-
turally by the corresponding variation of accretion rate,
which is widely regarded as the first parameter for go-
verning the state transition of BHXBs. However, accre-
tion rate is not only parameter, since some evolution fea-
tures, such as a hysteretic behavior in the state transition
of BHXBs, namely from hard to soft, takes place at
higher luminosities than the reverse transition later in the
outburst, cannot be interpreted by the variation of accre-
tion rate [38,40,41].
It was suggested by [20] that the size of the central
magnetic flux bundle can be identified with the second
parameter for determining X-ray spectral states of
BHXBs and the presence of relativistic outflows. Very
recently, King et al. [42] pointed out that the magnetic
field might be primarily toroidal in the soft state, but
primarily poloidal in the hard state. In fact, both the ac-
cumulation of the patched magnetic flux in the inner disk
and the change between toroidal and poloidal magnetic
fields can be regarded as evolution of magnetic field con-
figuration. Thus we suggest that magnetic field configu-
ration on the accretion disk is the second parameter for
governing the state transition of BHXBs. This viewpoint
can be interpreted roughly as follows.
By analogy with the coronal mass ejection Yuan et al.
[43] proposed a MHD model for interpreting the episodic
ejections from BHXBs, and GRO J1655-40, XTE J1550-
564 and GRS 1915 + 105 are involved. It turns out that
the magnetic field configuration for launching the epi-
sodic jet contains both open and closed field lines in the
inner accretion flow as shown in Figure 1 of [43]. In
addition, we notice that the three BHXBs with episodic
ejections are exactly those with 3:2 high-frequency QPO
pairs observed in SIM state (or SPL state), which are
fitted by invoking closed magnetic field configuration
with disk corona model in [18]. We also notice that the
transitions from LH to HIM states and then to SIM state
are associated with the transitions from open magnetic
field configuration for LH state (this paper) to open with
closed one for HIM state [43] and to closed one for SIM
state [18]. Coincidentally, the three BHXBs, GRO
J1655-40, XTE J1550-564 and GRS 1915 + 105 are all
involved in the three states, for which the fits are all given
in the frame of convective turbulence of MHD. The corre-
lation of magnetic field configurations with the transition
from hard to soft states is illustrated in Figure 4.
Although the origin of the magnetic field in BHXBs
remains unclear, it is most probably related to accretion
process in the following aspects.
1) Seed magnetic field is regarded as one of the possi-
bilities of the origin of the magnetic field, and seed
magnetic field is brought from a companion in accretion
process;
2) Seed magnetic field can be amplified via dynamo
mechanism, and this mechanism arises from differential
rotation of accretion disk;
3) Large-scale magnetic field might be generated by
toroidal electric current, and this kind of current exist
probably in the accretion due to total deviation from
electric neutrality in accreting plasma coming from its
companion.
Combining the above scenario of transition from hard
to soft states with the prevailing scenario of transition
from “high soft” state return to LH state, we obtain a
complete q-shaped pattern in counter-clockwise direction
consisting of upper and lower branches in HID as follows.
1) The upper branch of the q-shaped pattern (from

J. Z. WANG ET AL. 163
Figure 4. Illustration of correlation of magnetic field configurations with the transition from hard to soft states in BHXBs,
where the magnetic field configurations corresponding to HIM and SIM states are adopted from [43] and [18], respectively.
right to left): “quiescence” → LH state → HIM state →
SIM state;
2) The lower branch of the q-shaped pattern (from left
to right): Soft state → LH state → “quiescence”.
The scenario corresponding to the lower branch is de-
scribed in [2] as follows.
Soft state (thin disk extends close to ISCO)
→ LH state (a truncated thin disk with an inner
ADAF)
→ “quiescence” (accretion rate decreases to very low
level).
However, the difference of X-ray luminosities between
the upper and lower branches remains an open question.
5. Discussion
In this paper, we fit the spectral profiles of five BHXBs
in the LH state associated with the quasi-steady jets by
introducing the large-scale patched magnetic fields into
disk-corona model. It turns out that a quasi-steady jet
does associate with the LH state, and the relation be-
tween the jet power and the X-ray luminosity does hold
based on our model.
It is noted that the coupling of patched magnetic field
with accretion disk plays an essential role in the fits. On
the one hand, the patched magnetic field reduces the lu-
minosity effectively by extracting energy from the accre-
tion disk to drive jets, and thus it affects disk dynamics.
On the other hand, this effect gives rise to a feedback to
the patched magnetic field itself, reducing its outward
diffusion and increasing its drift inward. The main char-
acteristics of the spectral profiles of the LH state can be
retained with a quasi-steady jet driven by the patched
magnetic field distributed dispersedly on the disk-corona
system. Some issues related to our model are addressed
as follows.
(1) The mechanisms of driving jet
In this paper, the magneto-centrifugal mechanism (e.g.,
[13]) is adopted to drive jet via the patched field instead
of the BZ process [44]. Because of the following reasons,
1) The coupling of the patched magnetic fields with the
disk-corona system is realized via the magneto-centri-
fugal mechanism, and it is helpful to interpret the LH
state with a quasi-steady jet; 2) As shown in Figure 1,
the patched fields assumed in different direction dissipate
probably in magnetic reconnection as they drift close to
the innermost region of the disk, and thus the BZ process
cannot work due to very few magnetic fields brought to
the black hole. In addition, this configuration could pro-
vide a possible interpretation for producing episodic jets
in the transition from hard state to soft state [38,43].
(2) Relation between jet power and X-ray lumino-
sity
From the fitting results given in Table 3 and Figure 3
we find that the relation between jet power and X-ray
luminosity is fitted numerically in our model. Both jet
power and X-ray luminosity increases monotonically
with the increasing accretion rate and the outer boundary
of the corona, and these fits are consistent with the rising
phase of transient outburst of the BHXBs as shown in the
X-ray hardness-intensity diagram (HID) given by [38].
These results can be roughly understood as follows.

J. Z. WANG ET AL. 164
On the one hand, both jet power and X-ray luminosity
are powered essentially by gravitational energy released
in accretion process, so these two quantities increase with
the increasing accretion rate. On the other hand, required
by the unchanged hardness of the LH state, the outer
boundary of the corona should increase to contribute
more power law component via Comptonization in the
corona with the increasing accretion rate.
(3) The uncertainty of the fitting parameters
The most difficult problem related to our model is how
to describe the distribution and the drifting motion of the
magnetic patches. The purpose of this paper is to fit the
correlation of J with X rather than to fit the jet
power, as we have no direct observation data of the latter.
Thus we can lower the requirement for describing the
3-D jet, and incorporate the four parameters into one
parameter, pr
L
fk
L


  being taken around 0.0001 in
the calculations. Fortunately, the fitting results are insen-
sitive to the value, i.e., we can obtain the same spectral
profiles with a quasi-steady jet power for
p
f
varying
around 0.0001. The maximum values of
p
f
are con-
strained by the requirement that the radiation flux given
by Equation (11) should be non-negative, and those
maximum values are shown in the rightmost column of
Table 3.
The fitting results presented in this paper show that the
spectral profiles in the LH state could be well fitted by
disk-corona model with the inner edge of disk remains
close to ISCO, thus a cool accretion disk component and
a relativistically-broadened Fe K emission line can be
naturally explained. Nevertheless, we have to make some
assumptions with several parameters for describing the
patched magnetic fields due to lack of knowledge on
“flux expulsion” effect of convective turbulence, and we
hope to improve our model based on further study on
convective turbulence in accretion disk.
6. Acknowledgements
This work is supported by the NSFC (grants 11173011,
11143001, 11103003 and 11045004), the National Basic
Research Program of China (2009CB824800) and the
Fundamental Research Funds for the Central Universities
(HUST: 2011TS159).
REFERENCES
[1] T. M. Belloni, “Black-Hole Transients: From QPOs to
Relativistic Jets,” Advances in Space Research, Vol. 38,
No. 12, 2006, pp. 2801-2804.
doi:10.1016/j.asr.2005.10.048
[2] J. E. McClintock and R. A. Remillard, “Black Hole Bina-
ries,” In: W. Lewin and M. van der Klis., Eds., Compact
Stellar X-Ray Sources, Cambridge University Press, Cam-
bridge, 2006, pp. 157-213.
[3] A. A. Esin, J. E. McClintock and R. Narayan, “Advec-
tion-Dominated Accretion and the Spectral States of Black
Hole X-Ray Binaries: Application to Nova MUSCAE
1991,” The Astrophysical Journal, Vol. 489, No. 2, 1997,
pp. 865-889. doi:10.1086/304829
[4] A. Esin, R. Narayan, W. Cui, J. E. Grove and S. N. Zhang,
“Spectral Transitions in Cygnus X-1 and Other Black
Hole X-Ray Binaries,” The Astrophysical Journal, Vol.
505, No. 2, 1998, pp. 854-868. doi:10.1086/306186
[5] C. Done, M. Gierlinski and A. Kubota, “Modelling the
Behaviour of Accretion Flows in X-Ray Binaries. Every-
thing You Always Wanted to Know about Accretion but
Were Afraid to Ask,” The Astronomy and Astrophysics Re-
view, Vol. 15, No. 1, 2007, pp. 1-66.
doi:10.1007/s00159-007-0006-1
[6] J. M. Miller, J. Homan and G. Miniutti, “A Prominent
Accretion Disk in the Low-Hard State of the Black Hole
Candidate SWIFT J1753.5-0127,” The Astrophysical
Journal Letters, Vol. 652, 2006, pp. L113-L116.
doi:10.1086/510015
[7] J. M. Miller, et al., “A Long, Hard Look at the Low/Hard
State in Accreting Black Holes,” The Astrophysical Jour-
nal, Vol. 653, No. 1, 2006, pp. 525-535.
doi:10.1086/508644
[8] R. C. Reis, J. M. Miller and A. C. Fabian, “Thermal E-
mission from the Stellar-Mass Black Hole Binary XTE
J1118+480 in the Low/Hard State,” Monthly Notices of
the Royal Astronomical Society: Letters, Vol. 395, No. 1,
2009, pp. L52-L56.
doi:10.1111/j.1745-3933.2009.00640.x
[9] R. C. Reis, A. C. Fabian and J. M. Miller, “Black Hole
Accretion Discs in the Canonical Low-Hard State,”
Monthly Notices of the Royal Astronomical Society, Vol.
402, No. 2, 2010, pp. 836-854.
doi:10.1111/j.1365-2966.2009.15976.x
[10] J. Contopoulos, “A Simple Type of Magnetically Driven
Jets: An Astrophysical Plasma Gun,” The Astrophysical
Journal, Vol. 450, 1995, pp. 616-627.
doi:10.1086/176170
[11] I. Contopoulos and D. Kazanas, “A Cosmic Battery,” The
Astrophysical Journal, Vol. 508, No. 2, 1998, pp. 859-
863. doi:10.1086/306426
[12] D. Lynden-Bell, “Magnetic Collimation by Accretion
discs of Quasars and Stars,” Monthly Notices of the Royal
Astronomical Society, Vol. 279, No. 2, 1996, pp. 389-
401.
[13] G. S. Bisnovatyi-Kogan and A. A. Ruzmaikin, “The Ac-
cretion of Matter by a Collapsing Star in the Presence of a
Magnetic Field. II—Selfconsistent Stationary Picture,”
Astrophysics and Space Science, Vol. 42, No. 2, 1976, pp.
401-424. doi:10.1007/BF01225967
[14] R. D. Blandford and D. G. Payne, “Hydromagnetic Flows
from Accretion Discs and the Production of Radio Jets,”
Monthly Notices of the Royal Astronomical Society, Vol.
199, No. 3, 1982, pp. 883-903.
[15] M. Livio, “Astrophysical Spouts: The Jet Set,” Nature,
Vol. 417, No. 6885, 2002, p. 125.
[16] H. C. Spruit, “Theory of Magnetically Powered Jets,” In:

J. Z. WANG ET AL. 165
H. C. Spruit, Ed., The Jet Paradigm, Lecture Notes in
Physics, Springer-Verlag, Berlin, 2010, p. 233.
doi:10.1007/978-3-540-76937-8_9
[17] Z. M. Gan, D. X. Wang and W. H. Lei, “A Model of
Magnetically Induced Disc-Corona for Black Hole Bina-
ries,” Monthly Notices of the Royal Astronomical Society,
Vol. 394, No. 4, 2009, pp. 2310-2320.
doi:10.1111/j.1365-2966.2009.14518.x
[18] C. Y. Huang, Z. M. Gan, J. Z. Wang and D. X. Wang, “A
Resonance Model with Magnetic Connection for 3:2
HFQPO Pairs in Black Hole Binaries,” Monthly Notices
of the Royal Astronomical Society, Vol. 403, No. 4, 2010,
pp. 1978-1982. doi:10.1111/j.1365-2966.2009.16237.x
[19] A. Merloni and A. C. Fabian, “Coronal Outflow Dominat-
ed Accretion Discs: A New Possibility for Low-Lumino-
sity Black Holes?” Monthly Notices of the Royal Astro-
nomical Society, Vol. 332, No. 1, 2002, pp. 165-175.
doi:10.1046/j.1365-8711.2002.05288.x
[20] H. C. Spruit and D. A. Uzdensky, “Magnetic Flux Cap-
tured by an Accretion Disk,” The Astrophysical Journal,
Vol. 629, No. 2, 2005, pp. 960-968.
doi:10.1086/431454
[21] Y. B. Zeldovich, “The Magnetic Field in the Two-Dimen-
sional Motion of a Conducting Turbulent Liquid,” JETP,
Vol. 31, 1956, pp. 154-156.
[22] E. N. Parker, “A Kinematical Theory of Turbulent Hydro-
magnetic Fields,” The Astrophysical Journal, Vol. 138,
1963, p. 226. doi:10.1086/147629
[23] S. A. Balbus and J. F. Hawley, “A Powerful Local Shear
Instability in Weakly Magnetized Disks. I—Linear Ana-
Lysis. II—Nonlinear Evolution,” The Astrophysical Jour-
nal, Vol. 376, 1991, pp. 214-233.
doi:10.1086/170270
[24] J. M. Bardeen, W. H. Press and S. A. Teukolsky, “Rotat-
ing Black Holes: Locally Nonrotating Frames, Energy
Extraction, and Scalar Synchrotron Radiation,” The As-
trophysical Journal, Vol. 178, 1972, pp. 347-370.
doi:10.1086/151796
[25] B. F. Liu, S. Mineshige and K. Shibata, “A Simple Model
for a Magnetic Reconnection-Heated Corona,” The Astro-
physical Journal Letters, Vol. 572, 2002, pp. L173-L176.
doi:10.1086/341877
[26] N. La Palombara and S. Mereghetti, “XMM-Newton Ob-
servation of 4U 1543-475: The X-Ray Spectrum of a
Stellar-Mass Black-Hole at Low Luminosity,” Astronomy
and Astrophysics, Vol. 433, No. 3, 2005, pp. L53-L56.
doi:10.1051/0004-6361:200400123
[27] R. P. Fender, E. Gallo and D. Rusell, “No Evidence for
Black Hole Spin Powering of Jets in X-ray Binaries,”
Monthly Notices of the Royal Astronomical Society, Vol.
406, No. 3, 2010, pp. 1425-1434.
doi:10.1111/j.1365-2966.2010.16754.x
[28] R. I. Hynes, D. Steeghs, J. Casares, P. A. Charles and K.
O’Brien, “Dynamical Evidence for a Black Hole in GX
339-4,” The Astrophysical Journal Letters, Vol. 583,
2003, pp. L95-L98. doi:10.1086/368108
[29] A. A. Zdziarski, J. Poutanen, J. Mikołajewska, M. Gier-
linski, K. Ebisawa and W. N. Johnson, “A Spectral De-
composition of the Variable Optical, Ultraviolet and X-
Ray Continuum of NGC 5548,” Monthly Notices of the
Royal Astronomical Society, Vol. 301, No. 1, 1998, pp.
179-192. doi:10.1046/j.1365-8711.1998.02015.x
[30] J. M. Miller, et al., “Initial Measurements of Black Hole
Spin in GX 339-4 from Suzaku Spectroscopy,” The As-
trophysical Journal Letters, Vol. 679, No. 2, 2008, pp.
L113-L116. doi:10.1086/589446
[31] M. Kolehmainen and C. Done, “Limits on Spin Deter-
mination from Disc Spectral Fitting in GX 339-4,”
Monthly Notices of the Royal Astronomical Society, Vol.
406, No. 4, 2010, pp. 2206-2212.
doi:10.1111/j.1365-2966.2010.16835.x
[32] R. Shafee, et al., “Estimating the Spin of Stellar-Mass
Black Holes by Spectral Fitting of the X-Ray Contin-
uum,” The Astrophysical Journal Letters, Vol. 636, 2006,
pp. L113-L116. doi:10.1086/498938
[33] S. J. Tingay, et al., “Relativistic Motion in a Nearby
Bright X-Ray Source,” Nature, Vol. 374, No. 6518, 1995,
pp. 141-143. doi:10.1038/374141a0
[34] C. D. Bailyn, J. A. Orosz, J. E. McClintock and R. A.
Remillard, “Dynamical Evidence for a Black Hole in the
Eclipsing X-Ray Nova GRO J1655-40,” Nature, Vol. 378,
No. 6553, 1995, pp. 157-159.
doi: 10.1038/378157a0
[35] J. A. Orosz, et al., “Dynamical Evidence for a Black Hole
in the Microquasar XTE J1550-564,” The Astrophysical
Journal, Vol. 568, No. 2, 2002, pp. 845-861.
doi:10.1086/338984
[36] J. Greiner, J. G. Cuby and M. J. McCaughrean, “An Un-
usually Massive Stellar Black Hole in the Galaxy,” Na-
ture, Vol. 414, No. 6863, 2001, pp. 522-525.
[37] R. P. Fender, E. Gallo and P. Jonker, “Jet-Dominated
States: An Alternative to Advection across Black Hole
Event Horizons in ‘Quiescent’ X-Ray Binaries,” Monthly
Notice of the Royal Astronomical Society, Vol. 343, No. 4,
2003, pp. L99-L103.
doi:10.1046/j.1365-8711.2003.06950.x
[38] R. P. Fender, T. M. Belloni and E. Gallo, “Towards a
Unified Model for Black Hole X-Ray Binary Jets,”
Monthly Notices of the Royal Astronomical Society, Vol.
355, No. 4, 2004, pp. 1105-1118.
doi:10.1111/j.1365-2966.2004.08384.x
[39] J. Malzac, A. Merloni and A. C. Fabian, “Jet-Disc Coupl-
ing through a Common Energy Reservoir in the Black
Hole XTE J1118+480,” Monthly Notices of the Royal As-
tronomical Society, Vol. 351, No. 1, 2004, pp. 253-264.
doi:10.1111/j.1365-2966.2004.07772.x
[40] S. Miyamoto, S. Kitamoto, K. Hayahida and W. Egoshi,
“Large Hysteretic Behavior of Stellar Black Hole Candi-
date X-Ray Binaries,” The Astrophysical Journal Letters,
Vol. 442, No. 1, 1995, pp. L13-L16.
doi:10.1086/187804
[41] T. M. Belloni, “The Jet Paradigm,” In: The Jet Paradigm:
from Microquasars to Quasars, Lecture Notes in Physics,
Springer-Verlag, Berlin, 2010, p. 53.
doi:10.1007/978-3-540-76937-8
[42] A. L. King, et al., “An Extreme X-Ray Disk Wind in the

J. Z. WANG ET AL. 166
Black Hole Candidate IGR J17091-3624,” The Astro-
physical Journal Letters, Vol. 746, 2012, p. L20.
doi:10.1088/2041-8205/746/2/L20
[43] F. Yuan, J. Lin, K. W. Wu and C. H. Luis, “A Magneto-
hydrodynamical Model for the Formation of Episodic
Jets,” Monthly Notices of the Royal Astronomical Society,
Vol. 395, No. 4, 2009, pp. 2183-2188.
doi:10.1111/j.1365-2966.2009.14673.x
[44] R. D. Blandford and R. L. Znajek, “Electromagnetic Ex-
traction of Energy from Kerr Black Holes,” Monthly No-
tices of the Royal Astronomical Society, Vol. 179, 1977,
pp. 433-456.