Journal of Modern Physics, 2012, 3, 1261-1271
http://dx.doi.org/10.4236/jmp.2012.329163 Published Online September 2012 (http://www.SciRP.org/journal/jmp)
YinYang Bipolar Atom—An Eastern Road toward
Quantum Gravity*
Wen-Ran Zhang
Department of Computer Science, Georgia Southern University, Statesboro, USA
Received June 19, 2012; revised July 22, 2012; accepted July 31, 2012
ABSTRACT
Based on bipolar dynamic logic and bipolar quantum linear algebra, a causal theory of YinYang bipolar atom is intro-
duced in a completely background independent geometry that transcends spacetime. The causal theory leads to an equi-
librium-based super symmetrical quantum cosmology of negative-positive energies. It is contended that the new theory
has opened an Eastern road toward quantum gravity with bipolar logical unifications of particle and wave, matter and
antimatter, relativity and quantum entanglement. Information recovery after a black hole is discussed. It is shown that
not only can the new theory be applied in physical worlds but also in logical, mental, social and biological worlds. Fal-
sifiability of the theory is discussed.
Keywords: YinYang Bipolar Atom; Bipolar Geometry; Quantum Cellular Automata; Matter and Antimatter;
Information Recovery after a Black Hole; Real World Quantum Gravity
1. Introduction
Stephen Hawking’s black hole theory originally suggested
that the universe would ultimately disappear in a black
hole without information preservation. This suggestion
was criticized for violating the 2nd law of thermodyna-
mics. To remedy the inconsistency, Hawking proposed
black body evaporation [2] and then particle emission [3].
After then he held his position for three decades. In 2004,
he finally conceded a bet and agreed that black hole emis-
sion does in fact preserve information. But so far it is
unclear how to recover the information from the evapo-
ration or particle emission and how the universe will
evolve after a black hole. This uncertainty makes quan-
tum theory incomplete and nihilism unavoidable. For ins-
tance, M-theory predicts that a great many universes
were created out of nothing [4, p. 5].
Equilibrium is a well-known scientific concept that
subsumes symmetry or broken symmetry. Since equilib-
rium is central in the 2nd law of thermodynamics—the
paramount law of existence, energy, life, and information
where bipolar equilibrium is a generic form, YinYang
bipolar equilibrium-based approach to physics and sci-
ence provides a fundamental super symmetrical alterna-
tive for scientific unification. (Note: Equilibrium subsumes
equilibrium, non-equilibrium and quasi-equilibrium be-
cause local non-equilibriums can form global equilibrium
or quasi-equilibrium.)
Atom as a basic unit of matter should follow equilib-
rium or non-equilibrium conditions. It consists of a dense,
central nucleus surrounded by a cloud of negatively char-
ged electrons. The nucleus contains a mix of positively
charged protons and electrically neutral neutrons (except
in the case of hydrogen-1). The electrons of an atom are
bound to the nucleus by the electromagnetic force. Like-
wise, a group of atoms can remain bound to each other,
forming a molecule. In the case of antimatter atom, the
cloud is formed with positively charged positrons and the
atomic nucleus is negatively charged.
Molecule is an electrically neutral group of at least two
atoms held together by covalent chemical bonds. A cova-
lent bond is a form of chemical bonding that is charac-
terized by the sharing of pairs of electrons between atoms.
The stable balance of attractive and repulsive forces be-
tween atoms when they share electrons is known as co-
valent bonding.
An atom containing an equal number of protons and
electrons is electrically neutral. Otherwise, it has a posi-
tive or negative charge. A positively or negatively charged
atom is known as an ion. An atom is classified according
to the number of protons and neutrons in its nucleus: the
number of protons determines the chemical element and
the number of neutrons determines the isotope of the
element. Figure 1 shows some examples.
Legendary Danish physicist Niels Bohr, a father figure
of quantum mechanics, brought YinYang into quantum
theory for his particle-wave complementarity principle.
*The idea has been partially presented in Ref. [1].
C
opyright © 2012 SciRes. JMP

W.-R. ZHANG 1262
(a) (b) (c) (d)
Figure 1. (a) Matter hydrogen atom; (b) Proton of a hydro-
gen surrounded by an electron cloud; (c) Matter helium
atom with a nucleus (two protons and two neutrons) and
two electrons; (d) Tiny nucleus of a helium atom is sur-
rounded by electron cloud (Creative Commons: by User:
Yzmo).
When he was awarded the Order of the Elephant by the
Danish government in 1947, he designed his own coat of
arms which featured in the center a YinYang logo (or
Taiji symbol) with the Latin motto “contraria sunt com-
plementa” or “opposites are complementary”.
While quantum mechanics recognized particle-wave
complementarity it stopped short of identifying the es-
sence of YinYang bipolar coexistence. Without bipola-
rity any complementarity is less fundamental due to the
missing “opposites”. On the other hand, if bipolar equi-
librium is the most fundamental form of equilibrium, any
multidimensional model such as string, superstring or
M-theory cannot be most fundamental. In brief, action-
reaction forces, particle-antiparticle pairs, negative-posi-
tive energies, input and output, or the Yin and Yang in
general are the most fundamental opposites of nature, but
man and woman, space and time, particle and wave, truth
and falsity are not exactly bipolar opposites. This could
be the reason why Bohr believed that a causal description
of a quantum process cannot be attained and we have to
content ourselves with particle-wave complementary des-
criptions [5]. It may also be the reason why modern phy-
sics so far failed to find a definitive battleground for
quantum gravity.
Einstein pointed out: “For the time being we have to
admit that we do not possess any general theoretical ba-
sis for physics which can be regarded as its logical
foundation.” “Physics constitutes a logical system of
thought which is in a state of evolution, whose basis
(principles) cannot be distilled, as it were, from experi-
ence by an inductive method, but can only be arrived at
by free invention.”
In the above light, a causal theory of YinYang bipolar
atom is introduced in this paper based on bipolar dy-
namic logic and bipolar quantum linear algebra [6-8].
The theory provides a springboard to an equilibrium-ba-
sed logical unification of particle and wave, matter and
antimatter, relativity and quantum theory, strings and rea-
lity as well as big bang and black hole. Information reco-
very after a black hole is discussed. The logical, phy-
sical, mental, biological and social implications of this
work are formalized into a Q5 paradigm of quantum gra-
vities [8].
This paper is organized into six sections. Following
this introduction, a background review of the mathema-
tical basis of this work is presented in Section 2. Yin-
Yang bipolar atom is presented in Section 3. Bipolar
quantum cellular automata are introduced in Section 4.
Section 5 presents the theory of YinYang bipolar quan-
tum gravity. Section 6 draws a few conclusions as well as
philosophical distinctions.
2. YinYang Bipolar Dynamic Logic and
Quantum Linear Algebra
2.1. YinYang Bipolar Quantum Lattice and
Bipolar Dynamic Logic (BDL)
Aristotle’s causality principle became controversial in the
18th century after David Hume challenged it from an em-
pirical perspective. Hume argued that causation is irredu-
cible to pure regularity. YinYang bipolar dynamic logic
(BDL) [6,8-10] has changed this situation in a funda-
mental way. BDL is defined on a bipolar quantum lattice
B1 = {–1, 0}  {0, +1} = {(0,0), (0,1), (–1,0), (–1,1)} in
YinYang bipolar geometry as shown in Figure 2. The
four values of B1 form a bipolar set [8] which stand res-
pectively for eternal equilibrium (0,0), non-equilibrium
(–1,0), non-equilibrium (0,+1); equilibrium or harmony
(–1,+1). Equation (1)-(12) in Table 1 provide the basic
operations of BDL. The laws in Table 2 hold on BDL.
Most interestingly, BUMP makes equilibrium-based bipo-
lar quantum causality logically definable.
An axiomatization of BDL (Table 3) has been proven
sound and complete [8]. A key element in the axioma-
tization is bipolar universal modus ponens (BUMP) (Ta-
ble 4) which is a bipolar tautology, a non-linear bipolar
dynamic generalization of classical modus ponens and a
logical representation of bipolar quantum entanglement.
Thus, BDL generalizes Boolean logic to a quantum logic
where  and – are “balancers”;  and  are intuitive
“oscillators”; – and – are counter-intuitive “oscilla-
tors”; & and &– are “minimizers.” The linear, cross-pole,
bipolar fusion, oscillation, interaction and entanglement
properties are depicted in Figure 3. Bipolar relations and
equilibrium relations are defined in [6,8,11,12].
2.2. Bipolar Quantum Linear Algebra (BQLA)
The bipolar lattice B1 = {–1,0}  {0,1} and bipolar fuzzy
lattice BF = [–1,0]  [0,1] can be naturally extended to the
infinite bipolar lattice B = [–,0]  [0,+]. While B1 and
BF are bounded complemented unit square crisp/fuzzy
lattices, respectively, B is unbounded.
 (x,y),(u,v) B

,
Equations (13) and (14) define two major operations.
Tensor Bipolar Multiplication:
(x,y)  (u,v)  (xv+yu, xu+yv); (13)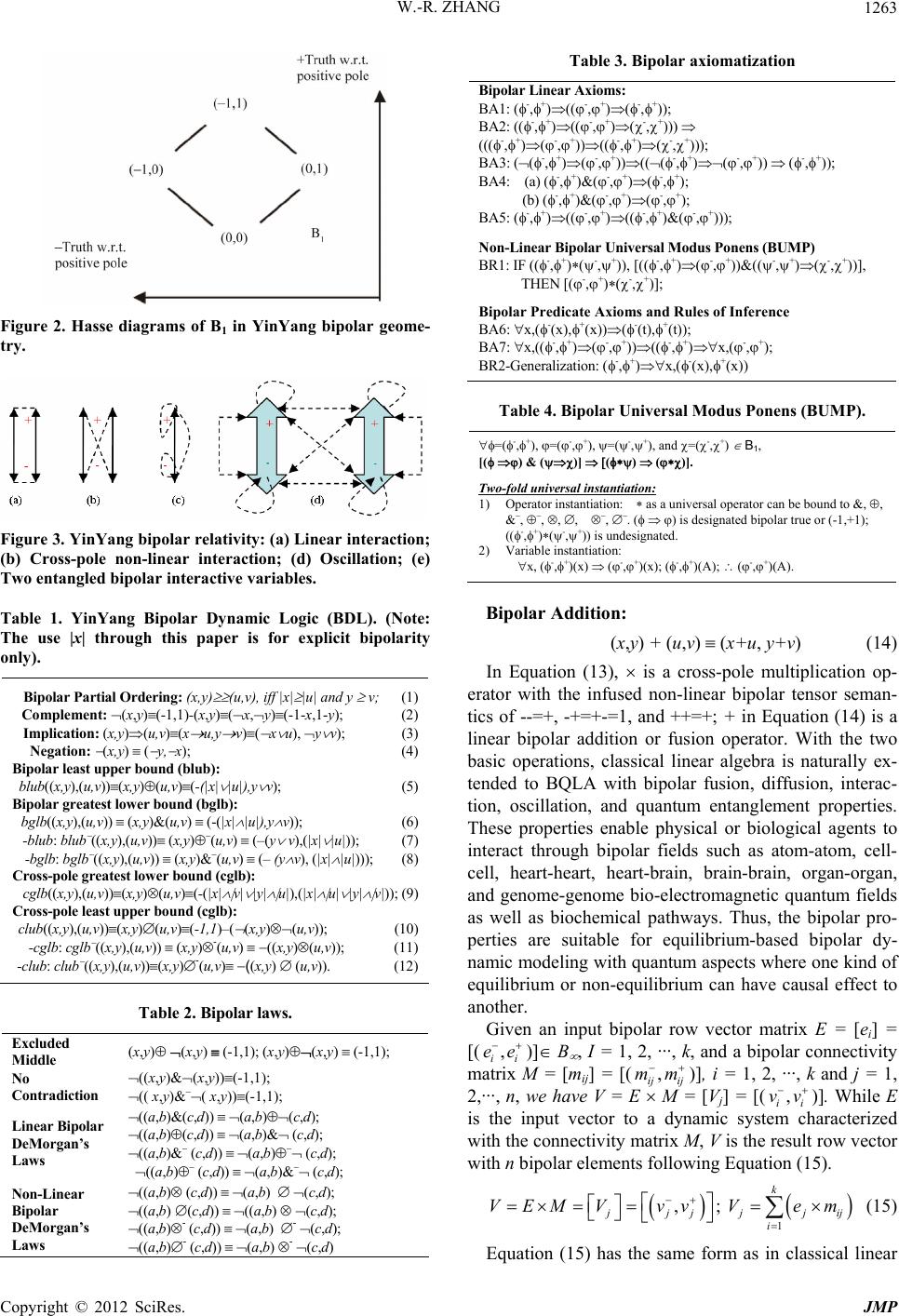

W.-R. ZHANG 1263
Figure 2. Hasse diagrams of B1 in YinYang bipolar geome-
try.
Figure 3. YinYang bipolar relativity: (a) Linear interaction;
(b) Cross-pole non-linear interaction; (d) Oscillation; (e)
Two entangled bipolar inte ractive variables.
Table 1. YinYang Bipolar Dynamic Logic (BDL). (Note:
The use |x| through this paper is for explicit bipolarity
only).
Bipolar Partial Ordering: (x,y)  (u,v), iff |x|  |u| and y  v; (1)
Compl e men t: (x,y)(-1,1)-(x,y)(x,y)(-1-x,1-y); (2)
Implication : (x,y)(u,v)(x  u,y  v)(  x  u), y  v); (3)
Negation:  (x,y)  (  y,  x); (4)
Bipolar least upper bound (blub):
blub((x,y),(u,v))(x,y)(u,v)(-(|x|
 |u|),y  v); (5)
Bipolar greate st lower b ound (b glb):
bglb((x,y),(u,v))  (x,y)(u,v)  (-(|x|  |u|),y  v)); (6)
-blub: blub

((x,y),(u,v)) (x,y)(u,v)  (–(y  v),(|x|  |u|)); (7)
-bglb: bglb

((x,y),(u,v))  (x,y)(u,v)  (– (y  v), (|x|  |u|))); (8)
Cross-pole greatest lower bound (cglb):
cglb((x,y),(u,v))(x,y)(u,v)(-(|x|
 v|  |y|  u|),(|x|  u|  |y|  v|)); (9)
Cross-pole least upper bound (cglb):
club((x,y),(u,v))(x,y)(u,v)(-1,1)–(
 (x,y)(u,v)); (10)
-cglb: cglb

((x,y),(u,v))  (x,y)-(u,v)  ((x,y)(u,v)); (11)
-club: club

((x,y),(u,v))(x,y)-(u,v) ((x,y) (u,v)). (12)
Tab le 2. Bipolar laws.
Excluded
Middle (x,y) (x,y)  (-1,1); (x,y)(x,y)  (-1,1);
No
Contradiction ((x,y)&(x,y))(-1,1);
(( x,y)&( x,y))(-1,1);
Linear Bipolar
DeMorgan’s
Laws
((a,b)(c,d))  (a,b)(c,d);
((a,b)(c,d))  (a,b)& (c,d);
((a,b) (c,d))  (a,b) (c,d);
((a,b) (c,d))  (a,b)& (c,d);
Non-Linear
Bipolar
DeMorgan’s
Laws
((a,b) (c,d))  (a,b) (c,d);
((a,b) (c,d))  ((a,b) (c,d);
((a,b)- (c,d))  (a,b)- (c,d);
((a,b)- (c,d))  (a,b)- (c,d)
Table 3. Bipolar axiomatization
Bipolar Linear Axioms:
BA1: (-,+)((-,+)(-,+));
BA2: ((-,+)((-,+)(-,+))) 
((( -,+)(-,+))((-,+)(-,+)));
BA3: ((-,+)(-,+))(((-,+)(-,+))  (-,+));
BA4: (a) (-,+)&(-,+)(-,+);
(b) (-,+)&(-,+)(-,+);
BA5: (-,+)((-,+)((-,+)&(-,+)));
Non-Linear Bipolar Universal Modus Ponens (BUMP)
BR1: IF ((-,+)(-,+)), [((-,+)(-,+))&((-,+)(-,+))],
THEN [(-,+)(-,+)];
Bipolar Predicate Axioms and Rules of Inference
BA6: x,(-(x),+(x))(-(t),+(t));
BA7: x,((-,+)(-,+))((-,+)x,(-,+);
BR2-Generalization: (-,+)x,(-(x),+(x))
Table 4. Bipolar Universal Modus Ponens (BU M P ) .
=(-,+), =(-,+), =(-,+), and =(-,+)  B1,
[( ) & ()] () ()].
Two-fold universal instantiation:
1) Operator instantiation:  as a universal operator can be bound to &, ,
&, , , , , . ( ) is designated bipolar true or (-1,+1);
((-,+)(-,+)) is undesignated.
2) Variable instantiation:
x, (-,+)(x) (-,+)(x); (-,+)(A);  (-,+)(A).
Bipolar Addition:
(x,y) + (u,v)  (x+u, y+v) (14)
In Equation (13),  is a cross-pole multiplication op-
erator with the infused non-linear bipolar tensor seman-
tics of --=+, -+=+-=1, and ++=+; + in Equation (14) is a
linear bipolar addition or fusion operator. With the two
basic operations, classical linear algebra is naturally ex-
tended to BQLA with bipolar fusion, diffusion, interac-
tion, oscillation, and quantum entanglement properties.
These properties enable physical or biological agents to
interact through bipolar fields such as atom-atom, cell-
cell, heart-heart, heart-brain, brain-brain, organ-organ,
and genome-genome bio-electromagnetic quantum fields
as well as biochemical pathways. Thus, the bipolar pro-
perties are suitable for equilibrium-based bipolar dy-
namic modeling with quantum aspects where one kind of
equilibrium or non-equilibrium can have causal effect to
another.
Given an input bipolar row vector matrix E = [ei] =
[( ii
,ee


,mm
)] B

, I = 1, 2, ..., k, and a bipolar connectivity
matrix M = [mij] = [( ij ij


,vv



1
,;
k
)], i = 1, 2, ..., k and j = 1,
2,..., n, we have V = E  M = [Vj] = [(ii )]. While E
is the input vector to a dynamic system characterized
with the connectivity matrix M, V is the result row vector
with n bipolar elements following Equation (15).
j
jjjj ij
i
VEM VvvVem



  

 (15)
Equation (15) has the same form as in classical linear

W.-R. ZHANG 1264
algebra except for: 1) ej and mij are bipolar elements; 2)
the multiplication operator is defined in Equation (13) on
bipolar variables with bipolar (quantum) entanglement;
and 3) the Σ operator is based on bipolar addition de-
fined on bipolar variables in Equation (14).
BQLA provides a new mathematical tool for modeling
YinYang-n-elements with explicit bipolar equilibrium,
quasi- or non-equilibrium representation for energy and
stability analysis. Energies in a row matrix can be con-
sidered as physical or biological energies of any agents
such as quantum or cosmological negative and positive
energies, repression and activation energies of regulator
proteins. Energies embedded in a connectivity matrix can
be deemed organizational energies that bind the agents
together. The following laws hold for any physical or
biological systems [7,8,13].
YinYang Bipolar Elementary Energy. Given a bipo-
lar element ,

,eee


1) ε−(e) = e– is the Yin or negative energy of e;
2) ε+(e) = e+ is the Yang or positive energy of e;
3) ε(e) = (ε−(e), ε+(e)) = (e–, e+) is the YinYang bipolar
energy measure of e;
4) The absolute total |ε|(e) = |ε−|(e) + |ε+|(e) is the to-
tal energy of e;
5) εimb(e)=|ε+|(e)  |ε−|(e) is the imbalance of e;
6) EnergyBalance(e) = (|ε|(e)−|εimb(e)|)/2.0
= min(|e−|, e+);
7) Harmony(e) = Balance(e) = (|ε|(e) − |εimb(e)|)/|ε|(e).
YinYang Bipolar System Energy. Given an k  n
bipolar matrix M = [mij] = (M−,M+) = (
j
j

,m

j
j
m



),
where M− is the Yin half with all the negative elements
and M+ is the Yang half with all the positive elements,
1) is the negative or
Yin energy of M;

11
M
kn
ij




11
kn
ij ij
ij
m




11
kn
ij ij
ij
m




2) is the positive or
Yang energy of M;

11
M
kn
ij




3) the polarized total, denoted ε(M) = (ε−(M), ε+(M)) is
the YinYang bipolar energy of M of M;
4) the absolute total, denoted |ε|(M) = |ε−|(M) + |ε+|(M),
is the total energy of M;
5) the energy subtotal for row i of M is denoted

*
0
M
n
iij
j





;
6) the energy subtotal for column j of M is denoted

*
0
M
k
j
ij
i





;
7)

 
imb
11 11
M
kn kn
imp ijijij
ij ij
mmm 

 

 
,ee

,mm
is
the YinYang imbalance of M;
8) balance or harmony or stability of M is defined as
Harmony(M) = Balance(M) = Stability(M) = (|ε|(M) −
|εimb(M)|)/|ε|(M);
9) the average energy of M is measured as h =
(ε−(M)/(kn), ε+(M)/(kn)) where kn = k  n is the total
number of elements in M.
Law 1. Elementary Energy Equilibrium Law. (x,y)
 B
 = [–, 0]  [0, +] and (u,v)  B F = [–1,0] 
[0,1], we have
a) [|
 |(u,v)  1.0]  [|  |((x,y) (u,v))  |  |(x,y) ];
b) [|
 |(u,v)<1.0]  [|  |((x,y) (u,v)) < |  |(x,y) ];
c) [|
 |(u,v)>1.0]  [|  |((x,y) (u,v)) > |  |(x,y) ].
Equilibrium/Non-Equilibrium System. A bipolar
dynamic system S is said an equilibrium system if the
system’s total energy |
 |S remains in an equilibrium state
or d(|
 |S)/dt = 0 without external disturbance. Otherwise
it is said a non-equilibrium system. A non-equilibrium
system is said a strengthening system if d(|  |S)/dt > 0; it
is said a weakening system if d(|  |S)/dt < 0.
Law 2. Energy Transfer Equilibrium Law. Given an
n  n input bipolar matrix E = [eik] = [(ikik )], 0 < i, k 
n, an n  n bipolar connectivity matrix M = [mkj] =
[( kj kj

v)], 0 < k, j  n, and V = E  M = [Vij] = [(ij

,
ij
v

)], k, j, let |ε|(Mk*) be the k-th row energy subtotal
and let |ε|(M*j) be the j-th column energy subtotal, we
have, k, j,
a) [|ε|(Mk*)  |ε|(M*j)  1.0 ]  [|  |(V)  |  |(E)];
b) [|ε|(Mk*)  |ε|(M*j)  1.0 ]  [|
 |(V) < |  |(E)];
c) [|ε|(Mk*)  |ε|(M*j) > 1.0 ]  [|
 |(V) > |  |(E)].
From the above, it is clear that without YinYang bipo-
larity, classical linear algebra cannot deal with the coex-
istence of the Yin and the Yang of nature and their causal
interactions in bipolar quantum entanglement.
Law 3. Law of Energy Symmetry. Let t = 0, 1, 2,…,
Y(t+1) = Y(t)  M(t), |ε|Y(t) be the total energy of an
YinYang-N-Element vector Y(t), |ε|M(t) be the total en-
ergy of the connectivity matrix M(t), |ε|Mi*(t) be the en-
ergy subtotal of row i of M(t), |ε|M*j(t) be the energy
subtotal of column j of M(t).
1) Regardless of the local YinYang balance or imbal-
ance of the elements at any time point t, the system will
remain a global energy equilibrium if, t, d(|ε|Y(t))/dt 
0, or (a)i,j, [|ε|(Mi*)  |ε|(M*j)  1.0] and (b) no external
disturbance to the system occurs after the initial vector
Y(0) is given.
2) Under the same conditions of (1), if, t, |ε−(M*j)| >
0 and |ε+(M*j))| > 0, all bipolar elements connected by M
will eventually reach a local YinYang balance
(–|ε|Y(t)/(2N), |ε|Y(t)/(2N)) at time t.
Law 4. Law of Broken Symmetry (Growing). For
the same system with Law 3, if, i, j, |ε|(Mi*)  |ε|(M*j) >
1.0, regardless of the local YinYang balance or imba-
lance of the elements at any time point t, the system en-
ergy will increase and eventually reach a bipolar infinite

W.-R. ZHANG 1265
(–,) or fission state without external disturbance or we
have,t, d(|ε|Y(t))/dt  0.
Law 5. Law of Broken Symmetry (Weakening). For
the same system as for Law 3, if, i, j, |ε|(Mi*)  |ε|(M*j)
< 1.0, regardless of the local YinYang balance or imba-
lance of the elements at any time point t, the system en-
ergy will decrease and eventually reach a (0,0) or decay-
ed state without external disturbance or we have, t,
d(|ε|Y(t))/dt < 0, until |ε|Y(t) = 0.
3. Bipolar Strings and Bipolar Atom
3.1. YinYang Bi pol ar Stri n g s
Fundamentally different from the mainstream string the-
ory or “theory of everything”, BDL and BQLA provide
the logical and physical bipolar bindings for the “strings”
of reality but retain the open-world non-linear dynamic
property of nature tailored for open-ended exploratory
scientific discovery. While strings are far from observable
reality, the non-linear dynamic property of BDL and
BQLA do not compromise the law of excluded middle—a
unique basis for a scalable and observable alternative bi-
polar string theory.
Since (–1,0)  (–1,0) = (–1,0)2 = (0,1) and (–1,1) 
(–1,1) = (–1,1)2 = (–1,1), (–1,0)n defines an oscillatory
non-equilibrium and (–1,1)n defines a non-linear dynamic
equilibrium. Such properties provide a unifying logical
representation for particle-wave duality. For instances,
(P)(f) = (–1,0)n (3  1012) can denote that “particle P
changes polarity three trillion times per second”; (P)(f) =
(–1,1)n (3  1012) can denote that “The two poles of P in-
teract three trillion times per second.”
As strings can be one-dimensional oscillating lines or
points, a bipolar string can be defined as an elementary
bipolar variable or quantum agent e = (–e,+e) and char-
acterized as (e)(f)(m) where (e)B1 or B, f is the fre-
quency of bipolar interaction or oscillation, and m is
mass. If e is massless we have m = 0. The two poles of e
as negative and positive strings are non-exclusive, recip-
rocal, entangled, and inseparable. Thus, bipolar strings
cannot be dichotomous and bipolar string theory is a
non-linear dynamic unification of singularity, bipolarity,
and particle-wave duality.
3.2. YinYang Bipolar Atom
Figure 4 shows a YinYang-n-element bipolar quantum
cellular automaton (BQCA), where each link and each
element is characterized with a bipolar value (n,p). A
negative side n can indicate output of an element or re-
pression of a link weight; a positive side p can indicate
input of an element or activation of a link weight. A set
of dynamic equations have been derived based BQLA for
characterizing the cellular structure in Figure 4. The set
Figure 4. A YinYang-n-element cellular structure.
of equations can be simplified as Y(t+1) = Y(t)  M(t),
where Y(t) is a bipolar vector at time t and M(t) a con-
nection matrix at time t. Now, our questions are:
1) How to use a YinYang-n-element cellular structure
to describe and unify matter and antimatter atoms?
2) How to use a YinYang-n-element cellular structure
to unify particle and wave?
3) How to use a YinYang-n-element cellular structure
to describe and unify quantum theory and relativity?
4) How to integrate multiple YinYang-n-element cel-
lular structures together?
5) How to use BDL, BQLA and BQCA to unify big
bang and black hole as well as space and time?
Dramatically, BQLA and BQCA can be used for rep-
resenting both matter and antimatter atoms as well as
particles and waves. Figure 5(a) shows the bipolar rep-
resentation of a hydrogen atom. Figure 5(b) is a redrawn
of Figure 4 by omitting connectivity. The positrons can
be regrouped to the nucleus of a matter atom as shown in
Figure 5(c), where the negative signs can character elec-
trons or electron cloud. Similarly, an antimatter atom is
shown in Figure 5(d). Thus, both matter and antimatter
atoms can be characterized using Equation (15) in BQLA.
It is evident from Figure 5 that YinYang bipolar atom
has the potential to bridge a gap between black hole and
big bang in a cyclic process model because it allows par-
ticles and antiparticles emitted from a black hole [2,3] to
form matter and antimatter again. While Laws 1 - 5 pro-
vide the axiomatic conditions for energy equilibrium,
growing, and degenerating, we introduce a new law of
oscillation [1] in the following:
Law 6. Law of Oscillation. Let t = 0, 1, 2, …, Y(t+1)
= Y(t)  M(t), |ε|Y(t) be the total energy of an YinYang-
n-element vector Y(t), |ε|M(t) be the total energy of the
connectivity matrix M(t), if, i, j, |ε|(Mi*)(tk)  |ε|(M*j) (tk)
> 1.0 and |ε|(Mi*)(tk+1)  |ε|(M*j) (tk+1) < 1.0, the system’s
total energy will be alternatively increasing at time k and
decreasing at time k + 1.
Evidently, any particle or wave form can be repre-
sented with Yin energy, Yang energy, or unified Yin-
Yang form. But without YinYang, the bipolar coexis-
tence and interaction of the two poles can’t be visualized.
The four cases of equilibrium, growing, degeneration and
oscillation are simulated in Figures 6-9.

W.-R. ZHANG 1266
‐
+
‐ +
‐
‐
‐
‐
+‐ ‐+
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
‐
‐
(a) (b)
‐ ‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
‐
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
‐
‐
‐
‐
‐
‐
‐
‐
‐‐
‐
‐
‐
‐
‐
‐
‐
‐
(c) (d)
Figure 5. (a) Bipolar representation of a hydrogen; (b) Bi-
polar representation of YinYang-n-elements; (c) Matter
atom; (d) Antimatter atom.
Figure 6. Bipolar energy rebalancing wave forms after a
disturbance to one element [8].
Figure 7. YinYang bipolar energy growing [8].
Figure 8. YinYang bipolar energy decreasing [8].
4. Bipolar Quantum Cellular Automata
YinYang bipolar atom leads to bipolar quantum cellular
automata (BQCA) for advancing research in cosmolo-
gical and molecular interactions. YinYang as the basis of
Figure 9. YinYang bipolar energy oscillation [8].
traditional Chinese medicine (TCM) has been in the di-
lemma of lacking a formal logical, mathematical, physi-
cal, and biological foundation. On the other hand, despite
one insightful surprise after another the genome has
yielded to biologists, the primary goal of the Human Ge-
nome Project—to ferret out the genetic roots of common
diseases like cancer and Alzheimer’s and then generate
treatments—has been largely elusive. Although quantum
mechanics provides a basis for chemistry and molecular
biology, it so far has not found unification with Ein-
stein’s relativity theory. This situation provides an oppor-
tunity for YinYang to enter modern science and play a
unifying role. For instance, given the cellular structures
in Figure 10, we have the question: “How to model the
integration, interaction, and equilibrium conditions?”
Law 7 (Following Law 3). Law of Integrated En-
ergy Symmetry. Given Figure 10, let t = 0, 1, 2, …,
Y(t+1) = Y(t)  M(t), |ε|Y( t) be the total energy of the
integrated BQCA vector Y(t), |ε|M(t) be the total energy
of the integrated connectivity matrix M(t), |ε|Mi*(t) be the
energy subtotal of row i of M(t), |ε|M*j(t) be the energy
subtotal of column j of M(t), the integrated BQCA can
satisfy the following two global conditions:
1) Regardless of the local YinYang balance/imbalance
of the subsystems at any time point t, the integrated sys-
tem will remain a global energy equilibrium if, t,
d(|ε|Y(t))/dt  0, or
(a) i,j, [|ε|(Mi*)  |ε|(M*j)  1.0];
(b) no external disturbance or input/output to/from the
system after the initial vector Y(0) is given;
(c) no internal disturbance or energy creation and con-
sumption in the system after the initial vector Y(0) is
given. That is, all the k component BQCA satisfy the
condition, t, d(|ε|Yk(t))/dt  0, or, equivalently, i, j,
[|εk|(Mi*)  |εk|(M*j)  1.0]. Otherwise, there will be in-
ternal disturbance.
2) Under the conditions of (1), if, t, |ε−(M*j)| > 0 and
|ε+(M*j))| > 0, all components connected by M will even-
tually reach a local YinYang balance (–|ε|Y(t)/(2K),
|ε|Y(t)/(2K)) at certain time point t.
Law 8 (Following Law 4). Law of Integrated En-
ergy Broken Symmetry (Growing). For the same inte-
grated BQCA as for Law 7, if, (a) i, j, |ε|(Mi*)  |ε|(M*j)
> 1.0; (b) no external disturbance after the initial vector

W.-R. ZHANG 1267
Figure 10. Integration of bipolar cellular subsystems.
Y(0) is given; (c) no internal disturbance or energy crea-
tion and consummation after the initial vector Y(0), re-
gardless of the local YinYang balance or imbalance of its
local component BQCAs at any time t, the system energy
will increase and eventually reach a bipolar infinite
(–,) or t, d(|ε|Y(t))/d t  0.
Law 9 (Following Law 5). Law of Integrated En-
ergy Broken Symmetry (Weakening). For the same
system as for Law 7, if, (a) i,j, |ε|(Mi*)  |ε|(M*j) < 1.0;
(b) no external disturbance to the system after the initial
vector Y(0) is given; (c) no internal disturbance or en-
ergy creation and energy consumption after the initial
vector Y(0) is given, regardless of the local YinYang
balance/imbalance of its local component BQCAs at any
time t, the system energy will decrease and eventually
reach an eternal equilibrium (–0,+0) state or, equivalently,
t, d(|ε|Y(t))/dt < 0, until |ε|Y(t) = 0.
Law 10 (Following Laws 3-9). Necessary and Suffi-
cient Conditions for Collect ive Bipolar Adaptivity. The
two conditions of Law 3 are necessary for collective bi-
polar adaptivity of any simple or integrated BQCA into
equilibrium and symmetry; the two conditions are suffi-
cient for collective bipolar adaptivity of any simple
BQCA but not for integrated BQCAs; the two conditions
in Law 7 are both necessary and sufficient for collective
bipolar adaptivity of any simple BQCA or integrated
BQCA into equilibrium and symmetry.
5. An Eastern Road to Quantum Gravity
5.1. Q5 Paradigm
Since acceleration is equivalent to gravitation under gene-
ral relativity, any physical, socioeconomic, mental, and
biological acceleration, growth, degeneration or aging
are qualified to be a kind of quantum gravity. It can be
further argued that as a most fundamental scientific uni-
fication not only can quantum gravity be applied in phy-
sical science, but also in computing science, social sci-
ence, brain science, and life sciences as well. This argu-
ment leads to five sub-theories of a Q5 paradigm of
quantum gravities: physical quantum gravity, logical
quantum gravity, mental quantum gravity, biological
quantum gravity, and social quantum gravity [8]. In the
Q5 paradigm, the theory of physical quantum gravity is
concerned with quantum physics; logical quantum gra-
vity is focused on quantum computing; mental quantum
gravity is focused on the interplay of quantum mechanics
and brain dynamics; biological quantum gravity is focus-
ed on life sciences; social quantum gravity spans social
sciences.
The Q5 paradigm may sound like a mission impossible.
It actually follows a single undisputable observation and
a single condition: 1) bipolar equilibrium or non-equili-
brium is a generic form of any multidimensional equili-
brium from which nothing can escape; 2) bipolar quan-
tum entanglement is logically definable with BUMP that
unifies truth, being and dynamic equilibrium with logi-
cally definable causality.
Roger Penrose described two mysteries of quantum
entanglement [14, p. 591]. The first mystery is the phe-
nomenon itself; the second one is: “Why do these ubi-
quitous effects of entanglement not confront us at every
turn?” Penrose remarked: “I do not believe that this sec-
ond mystery has received nearly the attention that it de-
serves.” It is contended that YinYang bipolar quantum
entanglement provides a resolution to the first mystery
and the Q5 paradigm provides a resolution to the second.
Since the Yin and the Yang are two reciprocal oppo-
site poles or energies that are completely background in-
dependent, YinYang bipolar geometry is fundamentally
different from Euclidian, Hilbert, and spacetime geome-
tries. With the background independent property, the new
geometry makes quadrants irrelevant because bipolar iden-
tity, interaction, fusion, separation, and equilibrium can
be accounted for in it even without quadrants (Figure
11).
Defined in YinYang bipolar geometry, BDL and
BUMP make quantum causality logically definable as
equilibrium-based quantum entanglement. It simply states:
For all bipolar equilibrium functions  ,  , ψ, and  , IF
(
   ) & (ψ  ), THEN the bipolar interaction (  ψ)
implies that of (    ). With the emergence of space and
time, BUMP leads to a completely background inde-
pendent theory of YinYang bipolar relativity defined by
Equation (16) [8]. a,b,c,d,




 


 

   
   
x1 y3
x2 y4
12
34
,,&
,,
,,
,,
xx
yy
at pct p
bt pdt p
at pbtp
ct pdt p

















(16)
In Equation (16), a(t1,p1), b(t1,p2), c(t2,p3), d(t2,p4) are
any bipolar agents where a(t,p) stands for “agent a at
time t and space p” (tx, ty, px and py can be the same or
different points in time and space). An agent without
time and space is assumed at any time t and space p. An
agent at time t and space p is therefore more specific.
The symmetrical property of YinYang bipolar geome-

W.-R. ZHANG
JMP
1268
(a) (b) (c)
Figure 11. Background-Independent YinYang bipolar geometry: (a) Magnitudes of Yin and Yang; (b) Growing curve; (c)
Quadrant irrelevant property.



try enables information to be passed through large or
small scale quantum entanglement with or without pass-
ing observable energy or mass. When photon or electron
is passed the speed is limited by the speed of light that
has been proven in physics. Physicists have so far failed
to experimentally verify the existence of graviton and the
speed of gravity. If all action-reaction forces are funda-
mentally equilibrium-based and bipolar quantum entan-
gled in nature, gravity would be logically unified with
quantum mechanics in the form of Equation (16) [8].







 


12
12
,0.0499,
, 0,00.0499,0,0
fStp fEtp
fStpfEt p



For instance, based on general relativity, gravity “tra-
vels” at the speed of light and the effect of a disturbance
to the Sun (S) could take 499 seconds to reach the Earth
(E). Let f(S) = f(E) = (–f,f)(S) = (–f,f)(E) be the gravita-
tional (reaction, action) forces between S and E; let time
t be in second; let p1 and p2 be points for S and E, respec-
tively; let (0,0) (S) be the hypothetical Sun’s vanishment
or eternal equilibrium; we have
   
  
12
2
, 499,
99,0,0
fStp fEtp
p






◆
  
1
,0 ,04fStp fEt



◆
(17a)
If f() is normalized to a bipolar predicate,  can be re-
placed with , and the binding of &, &, , , , or 
to  in Equation (17a) would lead to the vanishment of
the Sun and then the disappearing of the Earth from its
orbit after 499 seconds. Thus, bipolar quantum entan-
glement and general relativity are logically unified under
equilibrium-based YinYang bipolar relativity [8]. Here
bipolar relativity can host space and time emergence fol-
lowing agents’ arrivals.
Equation (17a) assumes that the speed of gravity
equals the speed of light based on general relativity. This
assumption is actually questionable. If we assume gravi-
tation is a kind of large scale quantum entanglement of
action and reaction forces, gravity could have a minimum
lower bound of 10,000 times the speed of light [15] and
would travel from the sun to the Earth in less than 0.0499
second and we would have Equation (17b).




◆◆

(17b)
A comparison of Equations (17b) with (17a) reveals an
equilibrium-based logical “bridge” from relativity to
quantum mechanics—a bridge toward quantum gravity.
Why cannot other logical and statistical systems be used
for the above unification? The answer is that without bi-
polarity a truth value in {0,1} or a probability p  [0,1] is
incapable of carrying any shred of direct physical syntax
or semantics such as equilibrium (–1,+1), non-equili-
brium (–1,0) or (0,+1), quasi-equilibrium (–0.9, +0.9),
eternal equilibrium (0,0) and, therefore, unable to repre-
sent non-linear bipolar dynamic interactions such as bi-
polar fusion, fission, oscillation, quantum entanglement,
and annihilation.
Bipolar relativity can also support causal reasoning
with time reversal because the premise of Equation (16)
could be a future event and the consequent a past one.
Although time travel in physics and cosmology is highly
speculative in nature, time reversal analysis has been pro-
ven very useful in many other scientific, technological,
and engineering research and development.
The equilibrium-based interpretation leads to a number
unifying features for particle-wave, matter-antimatter,
strings and atom as well as black hole and big bang. Evi-
dently, Law 6 provides the basic condition for both
waves and particles; YinYang bipolar atom provides the
unification for matter and antimatter. Since Figures 5(a)-
(d) are redrawing of a bipolar representation like Figure
8 (different only in the number of elements), BQLA,
BQCA, and Laws 1 - 6 all apply to the unipolar repre-
sentations of Figures 5(c) and (d). Thus, BQCA presents
a unifying mathematical model for matter and antimatter
atoms as well as particles and waves. In turn, it makes
the unification of black hole and big bang possible be-
cause the theory allows particles and antiparticles emitted
from a black hole [2,3] to form matter and antimatter

W.-R. ZHANG 1269
again. Thus, it bridges a major gap in quantum cosmo-
logy and set the stage for another cycle of a cyclic pro-
cess model of the universe. The unifying features are
made possible by the complete background independent
property of YinYang bipolar geometry (Figure 11).
YinYang bipolar elements and sets [8] provide an al-
ternative interpretation for strings as well. Different from
mainstream string theory, bipolar strings are scalable and
can be the makings of bipolar atoms (Figure 5). Thus,
the alternative interpretation brings strings into the real
world of matter and antimatter for the first time.
Since action and reaction or negative and positive en-
ergies can be electromagnetic or gravitational in nature,
YinYang bipolar atom can serve as a basis for real world
quantum gravity. If we treat the centrifugal and centripe-
tal forces of a planet similarly as that of an electron (or
positron) rotating around its nucleus, gravity can be a
superposition on quantum interaction. In either case, sin-
ce nothing can escape bipolar equilibrium or non-equi-
librium, renormalization is made possible in equilibrium-
based terms using BQLA and BQCA.
The YinYang negative-positive energies also provide a
possible unification for the many universes in M-theory.
It can be argued that the multiverses have to follow the
same equilibrium or non-equilibrium conditions of the 2nd
law of thermodynamics and become one universe. Other-
wise, the two energies can’t form the regulating force of
the multiverses. Thus, the different laws followed by dif-
ferent universes as described in The Grand Design have
to be unified under the same 2nd law of thermodynamics.
Different from other approaches to quantum gravity,
the equilibrium-based approach is rooted in the real world.
Due to YinYang bipolarity in mental health, bioinformat-
ics, life and social sciences [6-13,17-23], physical and
logical quantum gravity can be naturally extended to
mental, biological and social quantum gravities [8]. Thus,
it is contended that the new approach has opened an East-
ern road toward quantum gravity.
5.2. Falsifiability
Falsifiability is a must for any viable physical theory. It
is of course correct that bipolar quantum entanglement
needs experimental verification. However, 1) bipolar
atom finds its equivalent representation in classical atom
theory (Figure 5); 2) bipolar quantum entanglement or
BUMP is physical and logical; 3) unlike the predicted but
unverified existence of monopoles in string theory, di-
poles are everywhere. Thus, we have:
Postulate 1: Bipolar quantum entanglement is the
most fundamental entanglement in quantum gravity.
Postulate 2: YinYang bipolarity is the most funda-
mental property of the universe.
The two postulates are actually logically provable axi-
oms. For Postulate 1, if a bipolar element (Figures 4 and
5) characterizes the energy superposition of gravitational
and quantum action-reaction, an atom would be a set of
bipolar elements. As the total must be equal to the sum,
without bipolar entanglement there would be no atom
level entanglement. Postulate 2 follows Postulate 1.
Postulate 3: YinYang bipolar atom is a bipolar set of
quantum entangled particle and antiparticle pairs.
Postulate 4: Gravity is fundamentally large or small
scale bipolar quantum entanglement.
Postulate 5: The speed of gravity is limited by the
speed of quantum entanglement and not by that of light.
According to Einstein, “Evolution is proceeding in the
direction of increasing simplicity of the logical basis
(principles).” “We must always be ready to change these
notions—that is to say, the axiomatic basis of phys-
ics—in order to do justice to perceived facts in the most
perfect way logically.” While string and superstring
theories up to 11 or more dimensions failed the simplic-
ity measure, YinYang bipolar atom and bipolar quantum
entanglement are simple and logically comprehendible
with definable causality in BUMP. The bipolar quantum
interpretation coincides with MIT Professor Seth Lloyd’s
startling thesis that the universe is itself a quantum com-
puter [24]. According to Lloyd, the universe is all about
quantum information processing. Once we understand
the laws of physics completely, we will be able to use
small-scale quantum computing to understand the uni-
verse completely as well. Could YinYang bipolar quan-
tum entanglement or BUMP be such a basic law?
6. Conclusions
Based on YinYang bipolar dynamic logic and bipolar
quantum linear algebra, a logically definable causal the-
ory of YinYang bipolar atom has been introduced. The
causal theory has led to an equilibrium-based super sym-
metrical quantum cosmology of negative-positive ener-
gies. It is contended that the new theory has opened an
Eastern road toward quantum gravity with bipolar logical
unifications of matter-antimatter, particle-wave, strings
and reality, big bang and black hole, quantum entangle-
ment and relativity. It has been shown that not only can
the theory be applied in physical worlds but also provides
a Q5 paradigm of physical, logical, mental, biological
and social quantum gravities. Furthermore, it provides a
logically consistent cyclic process model of the universe
with information recovery after a black hole.
The strength of the equilibrium-based approach is its
interpretation and unification aspects. The strength comes
from the background-independent property of YinYang
bipolar geometry that transcends spacetime. The strength
would also be a weakness should YinYang be exclusive
of spacetime geometry. Fortunately, the new geometry is
not exclusive but inclusive. It promotes equilibrium, har-
mony and complementarity by hosting, regulating or in-

W.-R. ZHANG 1270
tegrating background-dependent models as emerging pa-
rameters for more challenging scientific explorations and
unifications.
This work is limited to qualitative simulation, interpre-
tation and unification. A major research topic is bipolar
quantization and space emergence. The negativepositive
energies of an electron-positron pair under certain condi-
tion provides a candidate bipolar unit for quantization
with space emergence as a result of particle-antiparticle
interaction.
Finally, the equilibrium-based approach to quantum
gravity is fundamentally different from other approaches
in philosophical basis. Since all beings must exist in cer-
tain equilibrium or non-equilibrium, a scientific reincar-
nation of philosophy is predicted [25].
REFERENCES
[1] W. R. Zhang, “YinYang Bipolar Atom and Quantum Cel-
lular Automation,” Proceedings of 2011 IEEE Interna-
tional Conference on Bioinformatics and Bioengineering,
Atlanta, 12-15 November 2011, pp. 791-797.
[2] S. Hawking, “Black Hole Evaporation,” Nature, Vol. 248,
No. 5443, 1974, pp. 30-31. doi:10.1038/248030a0
[3] S. Hawking, “Particle Creation by Black Holes,” Com-
munications in Mathematical Physics, Vol. 43, No. 3,
1975, pp. 199-220. doi:10.1007/BF02345020
[4] S. Hawking and L. Mlodinow, “The Grand Design,” Ran-
dom House Digital, Inc., New York, 2010.
[5] N. Bohr, “On the Notions of Causality and Complemen-
tarity,” Dialectica, Vol. 2, No. 3-4, 1948, pp. 312-319.
doi:10.1111/j.1746-8361.1948.tb00703.x
[6] J. Gore and A. van Oudenaarden, “Synthetic Biology: The
Yin and Yang of Nature,” Nature, Vol. 457, No. 7227,
2009, pp. 271-272. doi:10.1038/457271a
[7] W. R. Zhang, H. J. Zhang, Y. Shi and S. S. Chen, “Bipo-
lar Linear Algebra and YinYang-N-Element Cellular Net-
works for Equilibrium-Based Biosystem Simulation and
Regulation,” Journal of Biological Systems, Vol. 17, No.
4, 2009, pp. 547-576.
[8] W. R. Zhang, “YinYang Bipolar Relativity: A Unifying
Theory of Nature, Agents and Causality with Applica-
tions in Quantum Computing, Cognitive Informatics and
Life Sciences,” IGI Global, Hershey, 2011.
doi:10.4018/978-1-60960-525-4
[9] W. R. Zhang and L. Zhang, “YinYang Bipolar Logic and
Bipolar Fuzzy Logic,” Information Sciences, Vol. 165,
No. 3-4, 2004, pp. 265-287.
doi:10.1016/j.ins.2003.05.010
[10] W. R. Zhang, “YinYang Bipolar Lattices and L-Sets for
Bipolar Knowledge Fusion, Visualization, and Decision,”
International Journal of Information Technology and De-
cision Making, Vol. 4, No. 4, 2005, pp. 621-645.
[11] W. R. Zhang, “Equilibrium Energy and Stability Meas-
ures for Bipolar Decision and Global Regulation,” Inter-
national Journal of Fuzzy Systems, Vol. 5, No. 2, 2003,
pp. 114-122.
[12] W. R. Zhang, “YinYang Bipolar Fuzzy Sets and Fuzzy
Equilibrium Relations for Bipolar Clustering, Optimiza-
tion, and Global Regulation,” International Journal of In-
formation Technology and Decision Making, Vol. 5, No.
1, 2006, pp. 19-46.
[13] W. R. Zhang, A. Pandurangi and K. Peace, “YinYang
Dynamic Neurobiological Modeling and Diagnostic Ana-
lysis of Major Depressive and Bipolar Disorders,” IEEE
Transactions on Biomedical Engineering, Vol. 54, No. 10,
2007, pp. 1729-1739. doi:10.1109/TBME.2007.894832
[14] R. Penrose, “The Road to Reality: A Complete Guide to
the Laws of the Universe,” Alfred A. Knopf, New York,
2005.
[15] D. Salart, A. Baas, C. Branciard, N. Gisin and H. Zbinden,
“Testing the Speed of ‘Spooky Action at a Distance’,”
Nature, Vol. 454, No. 7206, 2008, pp. 861-864.
doi:10.1038/nature07121
[16] J. Gore and A. van Oudenaarden, “Synthetic Biology: The
Yin and Yang of Nature,” Nature, Vol. 457, No. 7227,
2009, pp. 271-272. doi:10.1038/457271a
[17] Y. Shi, E. Seto, L. S. Chang and T. Shenk, “Transcriptio-
nal Repression by YY1, a Human GLI-Kruppel Related
Protein, and Relief of Repression by Adenovirus E1A
Protein,” Cell, Vol. 67, No. 2, 1981, pp. 377-388.
doi:10.1016/0092-8674(91)90189-6
[18] S. Vasudevan, Y. Tong and J. A. Steitz, “Switching from
Repression to Activation: Micro RNAs Can Up-Regulate
Translation,” Science, Vol. 318, No. 5858, 2007, pp.
1931-1934. doi:10.1126/science.1149460
[19] W. R. Zhang, S. Chen and J. C. Bezdek, “POOL2: A Ge-
neric System for Cognitive Map Development and Deci-
sion Analysis,” IEEE Transactions on Systems, Man, and
Cybernetics, Vol. 19, No. 1, 1989, pp. 31-39.
[20] W. R. Zhang, S. Chen, W. Wang and R. King, “A Cogni-
tive Map Based Approach to the Coordination of Distrib-
uted Cooperative Agents,” IEEE Transactions on Systems,
Man, and Cybernetics, Vol. 22, No. 1, 1992, pp. 103-
114.
[21] W. R. Zhang and S. S. Chen, “Equilibrium and Non-
Equilibrium Modeling of YinYang WuXing for Diagnos-
tic Decision Analysis in Traditional Chinese Medicine,”
International Journal of Information Technology and De-
cision Making, Vol. 8, No. 3, 2009, pp. 529-548.
[22] W. R. Zhang, “NPN Fuzzy Sets and NPN Qualitative Al-
gebra: A Computational Framework for Bipolar Cogni-
tive Modeling and Multiagent Decision Analysis,” IEEE
Transactions on Systems, Man, and Cybernetics, Vol. 26,
No. 4, 1996, pp. 561-574.
[23] W. R. Zhang, K. A. Pandurangi, K. E. Peace, Y. Zhang
and Z. Zhao, “Mental Squares—A Generic Bipolar Sup-
port Vector Machine for Psychiatric Disorder Classifica-
tion, Diagnostic Analysis and Neurobiological Data Min-
ing,” International Journal on Data Mining and Bioin-
formatics, Vol. 17, No. 4, 2011, pp. 547-576.
[24] S. Lloyd, “Programming the Universe,” Alfred A. Knopf,
Inc., New York, 2006.

W.-R. ZHANG 1271
[25] W. R. Zhang, “Beyond Spacetime Geometry—The Death
of Philosophy and Its Quantum Reincarnation,” Journal
of Modern Physics, Vol. 3, No. 29A, 2012, pp. 1272-1284.
doi:10.4236/jmp.2012.329164