Vol.2, No.7, 760-763 (2010) Natural Science
http://dx.doi.org/10.4236/ns.2010.27095
Multidimensional electrostatic energy and classical
renormalization
Sami M. AL-Jaber
College of Applied Sciences, Palestine Technical University-Kadoorie, Tulkarm, Palestine; Permanent Address: Department of Physics,
An-Najah National University, Nablus, Palestine;
Received 8 April 2010; revised 20 May 2010; accepted 26 May 2010.
ABSTRACT
Recent interest in problems in higher space di-
mensions is becoming increasingly important
and attracted the attention of many investiga-
tors in variety of fields in physics. In this paper,
the electrostatic energy of two geometries (a
charged spherical shell and a non-conducting
sphere) is calculated in higher space dimension,
N. It is shown that as the space dimension in-
creases, up to N = 9, the electrostatic energy of
the two geometries decreases and beyond N = 9
it increases. Furthermore, we discuss a simple
example which illustrates classical renormali-
zation in electrostatics in higher dimensions.
Keywords: Electrostatic Energy; Higher
Dimensions; Renormalization
1. INTRODUCTION
The space dimension N plays an important role in
studying many physical problems. It has been used for
the radial wave functions of the hydrogen like atoms in
N dimensions [1,2]. Exactly solvable models have also
been investigated [3,4]. In addition, a great deal of recent
work in field theory [5], high energy physics [6], and in
cosmology [7] has been conducted. Furthermore, prob-
lems of mathematical interest have been investigated in
higher dimensions [8,9]. One of the fundamental quanti-
ties in physics is the electrostatic energy which is cur-
rently investigated by many workers in various areas
[10-12]. Therefore, the present author is motivated to
consider the effect of space dimension on the electro-
static energy of two simple, but illustrative, systems. A
connected technique to electrostatic energy is the renor-
malization in classical field theory. Renormalization is
needed to eliminate divergences which appear in the
computation of Feyman graphs so that sensible physical
results can be achieved [13-15]. Just recently, Corbò [16]
considered renormalization technique in classical fields
and Tort [17] discussed renormalization of electrostatic
energy. So in the present paper, we will consider an ex-
ample of classical renormalization of electrostatic energy
in higher space dimensions. The organization of the pre-
sent paper is as follows: In Section 2, we consider elec-
trostatic energy in a hyper spherical shell. In Section 3,
we calculate the electrostatic energy of a non conducting
hyper sphere. In Section 4, we present an example of
renormalization of electrostatic energy in higher space
dimensions. Section 5 is devoted for conclusions.
2. ELECTROSTATIC ENERGY OF A
HYPER SPHERICAL SHELL
We consider a charged hyper spherical shell of radius R
and charge Q in N-dimensional space. Our purpose is to
calculate the electrostatic energy of the shell by two
methods. In the first method, we calculate the work done
to bring the charge Q infinitesimally from infinity to the
surface of the shell, while in the second method, we
evaluate the volume integral over the squared of the
electric filed, E

. The two methods require the electric
field and the electric potential in space. Gauss’s Law in
N-dimensions is
1
0
Nenc
q
EdAEr d


 



 (1)
The angular surface integral gives [18],
/2
2
(/2)
N
dN


, (2)
where ()
x

is the Gamma function. Since the charge is
distributed on the surface, the above two equations yield
/2 1
0
(/2)
2NN
QN
Er



, rR (3)
and 0E

for rR

. The electric potential is given by

S. M. AL-Jaber / Natural Science 2 (2010) 760-763
761
761
/2 2
0
(/2) ,
2( 2)
r
NN
QN
VEdr rR
Nr 



 

 (4)
The first method yields the electrostatic energy, W as
2
/2 2
0
1(/2)1
24(2)
NN
QN
WVdA NR 



, (5)
Which can be written as
2
2
0
,
2( 2)
N
Q
WSN R


 (6)
where

is the surface charge density and S is the sur-
face area of a unit shell as given in Eq.2. The second
method enables us to write











R
N
NN drdr
r
QN
dEW 1
22
2
2
2/
0
2
02
)2/(
2
1
2
1



22/
0
21
)2(4
)2/(



NN RN
NQ

, (7)
Which is the same result given in Eq.5. It is interest-
ing to note that our result yields the well-known result
[19] for the three-dimensional case (N = 3), namely
R
Q
W
0
2
8 
. It is noticed that the electrostatic energy of
the hyper shell depends on the space dimension N. It is
illustrative to calculate the electrostatic energy (shell
W),
with R = 1, for different values of N. This is calculated in
units of 0
28/
 Q and is shown in the second column of
Table 1. Our calculated results clearly show that the
electrostatic energy has a minimum at the space dimen-
sion N = 9. This can be explained as follows: In higher
space dimensions, there are more orientations in space
and thus more angles (N-1). This implies that it is rela-
tively easy to assemble electric charges on the hyper
surface of the shell which explains the decrease in the
electrostatic energy as the space dimension increases up
to N = 9. However, beyond this value of N, the surface
area of the shell becomes smaller and smaller so that the
decrease in the surface area, as N increases, dominates
over the increase in the angular orientation. In mathe-
matical terms, the surface area times (N-2) has a maxi-
mum at N = 9 and thus the electrostatic energy has a
minimum at that value of N. It is tempting to investigate
the behavior of the electrostatic energy for very large N.
This can be checked by using Stirling’s formula [20]
nn ennnn 
 2 !)1( 
, (8)
and letting 2/)2(  Nn, one finds for very large N
2/)3(
2/)2(
0
2
)2(
2
1
4









N
N
shell N
e
Q
W

. (9)
In the infinite dimensional space, the above equation
gives an infinite electrostatic energy in the limit as

N. In this limiting case the surface area of the
shell vanishes as can be seen from Eq.2 and the use of
Stirling’s formula. Therefore, the shell behaves like a
point charge in the infinite dimensional space and thus
one expects the divergence of the electrostatic energy as
an infinite self energy of a point particle.
3. ELECTROSTATIC ENERGY OF A
CHARGED NON-CONDUCTING HPER
SPHERE
Our main purpose here is to calculate the electrostatic
energy of a uniformly charged non-conduction sphere in
N-dimensional space. Following the second method of
Section 2, we calculate the electric field inside and out-
side the sphere. The application of Gauss’s Law given in
Eq.1 gives











,
ˆ
ˆ
1
2
)2/( 1
2/
0Rrr
R
r
Rrr
r
NQ
E
N
N
N


(10)
where Q is the charge in the sphere. The electrostatic
energy of the hyper sphere is thus
.
2
)2/(
2
1
2
1
0
2
1
22
1
2
2/
0
0
2
0






















ddr
R
r
dr
r
rNQ
dEW
R
R
N
N
N
N
N
sphere



The integrals in the curly bracket yield 22
1
)4(
2

N RN
N
and the integral over Ω is given by Eq.2, Therefore, the
electrostatic energy is simplified to
,
)4(2
)2/(
222/
0
2


NR
NQN
WNN
sphere

(11)
which can be written as
,
)4( 22
0
2


N
sphere RNV
Q
W

(12)
where ))2/(/2(2/ NNV N
 is the volume of the unit
sphere in the N-dimensional space [18]. Clearly, the above
electrostatic energy depends on the space dimension N,
and it yields the well-known result [19] for N = 3, namely
.
5
3
4
1
)3(
2
0R
Q
NWsphere


It is again constructive to calculate the electrostatic
energy, in units of 0
24/
 Q, with R = 1 for different
values of N. This is shown in the last column of Table 1.
As before, the electrostatic energy has a minimum at the

S. M. AL-Jaber / Natural Science 2 (2010) 760-763
762
space dimension N = 9. But here, the volume of the hy-
per sphere time (N2−4) has a maximum at N = 9 and
hence the electrostatic energy has a minimum at that
value. As it was checked in the previous section, the
electrostatic energy becomes infinite in the infinite di-
mensional space (N). In this limiting case the
volume of the hyper sphere vanishes and thus the sphere
behaves as a point charge with an infinite self energy.
4. RENORMALIZATION OF ELECTRO-
STATIC ENERGY
Renormalization, as is widely believed, is required in
quantum field theory [21-23]. The main task of renor-
malization is to handle and eliminate the divergences so
that one can obtain sensible physical results. Recently, it
has been reported that renormalization can be applied to
classical fields: For example, Corbò [16] gave two ex-
amples for renormalization of electrostatic potential and
Tort [17] presented an example for renormalization of
electrostatic energy. Our purpose here is to generalize
Tort's example to higher space dimension N. Beside its
mathematical interest, we will show that the divergence
(or so-called singularity) of the electrostatic energy per-
sists in the infinite dimensional space. Following Tort’s
model for the classical atom, we consider a point electric
charge of magnitude Ze, where Z is the atomic number
and e is the electron charge, surrounded by a concentric
thin hyper-spherical shell of radius R and electric charge
equal to −Ze. Ionization (partial or total) of this atom
amounts to the removal of part of or the entire negative
charge from the shell. This can be achieved by letting
)1(

 ZeZe , where ]1,0[


. We will show be-
low that the renormalization of the electrostatic energy
(U) in N dimensions is given by
22/
0
2
)2(4
)2/()(



 NN
initialfinal RN
NZe
UUU


(13)
The electric field inside the shell is only due to the
point charge, since there is no contribution comes from
the shell. Thus, the application of Gauss’s Law, given in
Eq.1, yields
Rrr
r
NZe
ENN

0 ,
ˆ
2
)2/(
12/
0


(14)
and 0E

for R
r
. The initial electrostatic energy
before ionization can be calculated as
1
)2(4
)2/(
1
2
)2/(
2
1
2
1
0
22/
0
22
1
0
22
2
2/
0
2
0
R
NN
N
R
NN
N
initial
rN
NeZ
drdr
r
NZe
rdEU



























(15)
Obviously, the function )/(1 1N
r diverges at the origin
and thus we have a singular point at 0r. As Tort
suggested, we can avoid this problem by introducing a
finite non-null radius

for the point charge and thus
.
11
)2(4
)2/(
222/
0
22








 NNN
initial RN
NeZ
U

(16)
Now, when the atom is ionized part of the charge
)( Ze


of the shell will move to infinity and thus the
enclosed charge within a hyper-spherical Gaussian sur-
face of radius R
r
. Will be .)1( ZeZeZeq




It is clear that the electric field, for R
r
, remains the
same as before ionization (see Eq.14) and for R
r

Gauss’s Law immediately gives
Rrr
r
NZe
ENN 

 .
ˆ
2
)2/(
12/
0



(17)
Therefore the final electrostatic energy becomes
























drdr
r
NZe
RN
NeZ
U
R
N
NN
NNN
final
1
2
12/
0
0
222/
0
22
2
)2/(
2
11
)2(4
)2/(




(18)
The first term is just initial
U and the integral in the sec-
ond term has the same form as that of Eq.7 and thus, one
gets
)2(2/
0
21
)2(4
)2/()(



 NN
initialfinal RN
NZe
UU


(19)
Therefore, the change in the electrostatic energy is
,
1
)2(4
)2/()(
22/
0
2



NN
initialfinalRN
NZe
UUU


(20)
which is exactly the same as the electrostatic energy of a
hyper-spherical shell that we found in Section 2. It is no-
ticed that the variation of electrostatic energy is finite for
all values of space dimension N, except for


N
where U

becomes infinite. Therefore, the renormali-
zation of the electrostatic energy works out for all space
dimensions but failed in the infinite dimensional space.
The persistent of the singularity in the infinite dimensional
space is a result of the infinite electrostatic energy of the
hyper-shell in that space, as we outlined in Section 2.
5. CONCLUSIONS
We have obtained the electrostatic energy of two sys-
tems (a charged spherical shell and a charged non-
conducting sphere) in the N-dimensional space. Our
calculated results show that the electrostatic energy
decreases as the space dimension increases up to
9

N and it increases without limit beyond that

S. M. AL-Jaber / Natural Science 2 (2010) 760-763
763
763
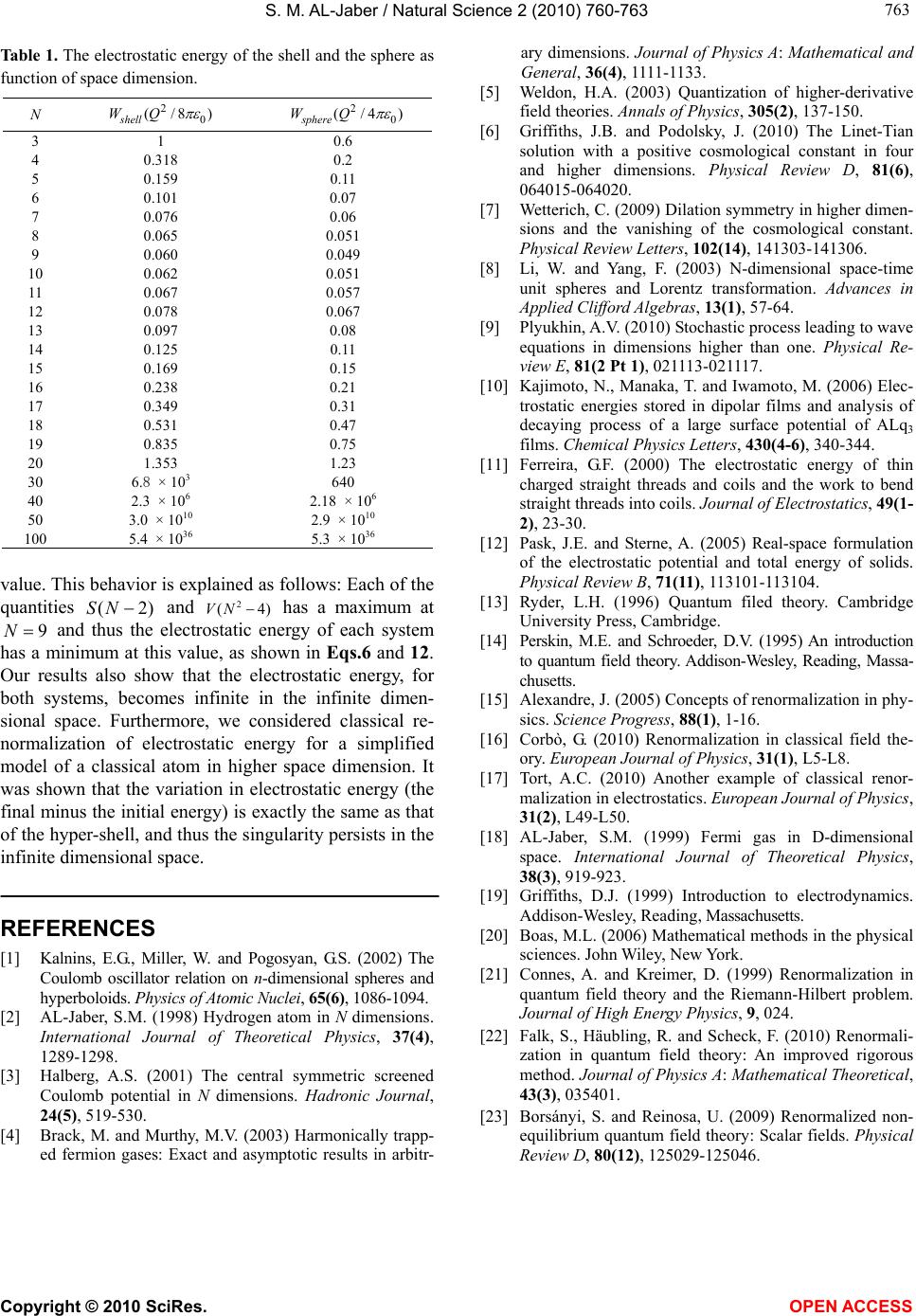
Table 1. The electrostatic energy of the shell and the sphere as
function of space dimension.
N 2
0
(/8)
shell
WQ

 2
0
(/4)
sphere
WQ


3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
30
40
50
100
1
0.318
0.159
0.101
0.076
0.065
0.060
0.062
0.067
0.078
0.097
0.125
0.169
0.238
0.349
0.531
0.835
1.353
6.8 × 103
2.3 × 106
3.0 × 1010
5.4 × 1036
0.6
0.2
0.11
0.07
0.06
0.051
0.049
0.051
0.057
0.067
0.08
0.11
0.15
0.21
0.31
0.47
0.75
1.23
640
2.18 × 106
2.9 × 1010
5.3 × 1036
value. This behavior is explained as follows: Each of the
quantities )2( NS and 2
(4)VN  has a maximum at
9N and thus the electrostatic energy of each system
has a minimum at this value, as shown in Eqs.6 and 12.
Our results also show that the electrostatic energy, for
both systems, becomes infinite in the infinite dimen-
sional space. Furthermore, we considered classical re-
normalization of electrostatic energy for a simplified
model of a classical atom in higher space dimension. It
was shown that the variation in electrostatic energy (the
final minus the initial energy) is exactly the same as that
of the hyper-shell, and thus the singularity persists in the
infinite dimensional space.
REFERENCES
[1] Kalnins, E.G., Miller, W. and Pogosyan, G.S. (2002) The
Coulomb oscillator relation on n-dimensional spheres and
hyperboloids. Physics of Atomic Nuclei, 65(6), 1086-1094.
[2] AL-Jaber, S.M. (1998) Hydrogen atom in N dimensions.
International Journal of Theoretical Physics, 37(4),
1289-1298.
[3] Halberg, A.S. (2001) The central symmetric screened
Coulomb potential in N dimensions. Hadronic Journal,
24(5), 519-530.
[4] Brack, M. and Murthy, M.V. (2003) Harmonically trapp-
ed fermion gases: Exact and asymptotic results in arbitr-
ary dimensions. Journal of Physics A: Mathematical and
General, 36(4), 1111-1133.
[5] Weldon, H.A. (2003) Quantization of higher-derivative
field theories. Annals of Physics, 305(2), 137-150.
[6] Griffiths, J.B. and Podolsky, J. (2010) The Linet-Tian
solution with a positive cosmological constant in four
and higher dimensions. Physical Review D, 81(6),
064015-064020.
[7] Wetterich, C. (2009) Dilation symmetry in higher dimen-
sions and the vanishing of the cosmological constant.
Physical Review Letters, 102(14), 141303-141306.
[8] Li, W. and Yang, F. (2003) N-dimensional space-time
unit spheres and Lorentz transformation. Advances in
Applied Clifford Algebras, 13(1), 57-64.
[9] Plyukhin, A.V. (2010) Stochastic process leading to wave
equations in dimensions higher than one. Physical Re-
view E, 81(2 Pt 1), 021113-021117.
[10] Kajimoto, N., Manaka, T. and Iwamoto, M. (2006) Elec-
trostatic energies stored in dipolar films and analysis of
decaying process of a large surface potential of ALq3
films. Chemical Physics Letters, 430(4-6), 340-344.
[11] Ferreira, G.F. (2000) The electrostatic energy of thin
charged straight threads and coils and the work to bend
straight threads into coils. Journal of Electrostatics, 49(1-
2), 23-30.
[12] Pask, J.E. and Sterne, A. (2005) Real-space formulation
of the electrostatic potential and total energy of solids.
Physical Review B, 71(11), 113101-113104.
[13] Ryder, L.H. (1996) Quantum filed theory. Cambridge
University Press, Cambridge.
[14] Perskin, M.E. and Schroeder, D.V. (1995) An introduction
to quantum field theory. Addison-Wesley, Reading, Massa-
chusetts.
[15] Alexandre, J. (2005) Concepts of renormalization in phy-
sics. Science Progress, 88(1), 1-16.
[16] Corbò, G. (2010) Renormalization in classical field the-
ory. European Journal of Physics, 31(1), L5-L8.
[17] Tort, A.C. (2010) Another example of classical renor-
malization in electrostatics. European Journal of Physics,
31(2), L49-L50.
[18] AL-Jaber, S.M. (1999) Fermi gas in D-dimensional
space. International Journal of Theoretical Physics,
38(3), 919-923.
[19] Griffiths, D.J. (1999) Introduction to electrodynamics.
Addison-Wesley, Reading, Massachusetts.
[20] Boas, M.L. (2006) Mathematical methods in the physical
sciences. John Wiley, New York.
[21] Connes, A. and Kreimer, D. (1999) Renormalization in
quantum field theory and the Riemann-Hilbert problem.
Journal of High Energy Physics, 9, 024.
[22] Falk, S., Häubling, R. and Scheck, F. (2010) Renormali-
zation in quantum field theory: An improved rigorous
method. Journal of Physics A: Mathematical Theoretical,
43(3), 035401.
[23] Borsányi, S. and Reinosa, U. (2009) Renormalized non-
equilibrium quantum field theory: Scalar fields. Physical
Review D, 80(12), 125029-125046.