Paper Menu >>
Journal Menu >>
 Applied Mathematics, 2010, 1, 94-100 doi:10.4236/am.2010.12012 Published Online July 2010 (http://www. SciRP.org/journal/am) Copyright © 2010 SciRes. AM Liouville-Type Theorems for Some Integral Systems Zhengce Zhang College of Science, Xi’an Jiaotong University, Xi’an, China E-mail: zhangzc@mail.xjtu.edu.cn Received March 20, 2010; revised May 21, 2010; accepted May 27, 2010 Abstract In this paper, Liouville-type theorems of nonnegative solutions for some elliptic integral systems are consid- ered. We use a new type of moving plane method introduced by Chen-Li-Ou. Our new ingredient is the use of Stein-Weiss inequality instead of Maximum Principle. Keywords: Liouville Theorem, Integral System, Moving Plane Method 1. Introduction In this paper we consider the nonnegative solutions of the systems of integral equations in 2 NN, 0 N, 12 12 () () N N qq N pp N uy vy ux dy xy vy uy vx dy xy (1) and 12 12 () () N N qq N pp N uy vy ux dy xy vy uy vx dy xy (2) The integral systems are closely related to the follow- ing systems of differential equations in N 12 12 /2 /2 () () qq pp uu v vv u (3) and 12 12 /2 /2 () () qq pp uuv vvu (4) In fact, every positive smooth solution of PDE (3)(or (4)) multiplied by a constant satisfies (1) (or (2)) respec- tively. This equivalence between integral and PDE sys- tems can be verified as in the proof of Theorem 1 in [1]. For single equations, we refer to [2]. Here, in (3) and (4), we have used the following definition: /2 ()( )uu where is the Fourier transformation and its in- verse. The question is to determine for which values of the exponents pi and qi the only nonnegative solution (u, v) of (1) and (2) is trivial, i.e., (u; v) = (0, 0). When 2 , is the case of the Emden-Fowler equation 0,0 u uuk in N (5) When )3)(2/()2(1 NNNk , it has been pro- ved in [3,4] that the only solutions of (5) is u = 0. In di- mension N = 2, a similar conclusion holds for k0. It is also well known that in the critical case, /)2( Nk )2( N, problem (5) has a two-parameter family of so- lutions given by 2 2 2)()( N xxd c xu , (6) where 2 1 ])2([ dNNc with d > 0 and x N . If p1,q1 > 1, p2,q2 ≥ 0 and /)2(2,2min 2121 Nqqpp )2( N, using Pokhozhaev’s second identity, Chen and Lu [5] have proved that the problem (4) has no positive radial solutions with )()( xuxu . Suppose that p1, p 2, q1 and q2 satisfy 1,,1,0 2211 qp qp and other re- lated conditions, using the method of integral relations, Mitidieri [6] has proved that the problem (4) has no posi- tive solutions of C2(N ). In the present paper, we study Problem (1) and Problem (2) by virtue of the moving plane method and obtain the following theorems of non-  Z. C. ZHANG Copyright © 2010 SciRes. AM 95 existence of positive solutions in the weaker regularity. Theorem 1.1: Let (u, v) be a nonnegative solution of Problem (1) and N N qqpp N N 2121,,, but not equal to )/()( NN at the same time. Suppose that 1 loc Lu (N )2 loc L(N ) and 2 loc Lv (N ) 1 loc L (N ) with 1 1 1 1 1 q N N q , 1 1 2 2 2 q N N q , 1 1 1 1 1 p N N p and 1 1 2 2 2 p N N p . Then both u and v are trivial. Theorem 1.2: Let (u, v) be a nonnegative solution of Problem (2) and 1212 , NN ppqq NN but not both equal to )/()( NN . Suppose that u, v () N L () N L with 12 12 1 ()1 qq Nqq N and 12 12 1 ()1 pp Npp N . Then both u and v are trivial. Remark 1.1: In the proof of Theorem 1.1 or Theorem 1.2, we only treat the case 2121 ,,,qqpp N N N N or N N qqpp N N 2121 , respec- tively. The remaining cases can be handled in the same way. We leave this to the interested reader. We shall need the following doubly weighted Hardy- Littlewood-Sololev inequality of Stein and Weiss (see, for example, [7]) () p N NqN ,, ,p,NL L Vx,yfydyf (7) where yyxxyxV ),( , pN /0 , 0/Nq , 1/1/1 pp and qNp /11/)(/1 . There are some related works about this paper. For 2 , 0 11 qp and 2 1p, )2/()2( 2 NNq (but p2 and q2 are not both equal to )2/()2( NN ), Figueiredo and Felmer (see [8]) proved the similar Liou- ville-type theorems to Theorem 1.1 and Theorem 1.2 on nonnegative positive of C2(N ) using the moving plane method and Maximum Principle. Busca and Manásevich obtained a new result (see [9]) using the same method as in [8]. It allows p2 and q2 to reach regions where one of the two exponents is supercritical. In [10], Zhang, Wang and Li first introduced the Kelvin transforms and gave a different proof using the method of moving spheres. This approach was suggested in [11], while Li and Zhang had made significant simplifications in the proof of some Liouville theorems for a single equation in [12]. In this paper, by virtue of Hardy-Littlewood-Sololev inequality instead of Maximum Principle, we consider the integral systems (1) and (2) with N 0 and the general nonlinearities. Therefore, it is a generalization of Liou- ville-type theorems in [5,6,8,9,13-15]. Let us emphasize that considerable attention has been drawn to Liouville-type results and existence of positive solutions for general nonlinear elliptic equations and systems, and that numerous related works are devoted to some of its variants, such as more general quasilinear operators, and domains. We refer the interested reader to [16-24], and some of the references therein. In the following, we shall use C to denote different constants which depend only on N, , i q, i p(i = 1,2) and the solutions u and v in varying places. 2. Kelvin-Type Transform and Proof of Theorem 1.1 To prove Theorem 1.1, we shall use the method of mov- ing planes. We first introduce the Kelvin-type transforms u and v as follows, )()( 2 x x uxxuN and )()( 2 x x vxxv N which are defined for x N 0\ . Then by elementary calculations, one verifies that (1) and (2) are transformed into the following form: 12 12 1212 N N Ns s qq Nr rpp u( x)xyyuyyvydy v( x)xyyvyyuydy (8) and 12 12 12 12 /2 /2 () () ss qq rr pp uxu xv vxv xu (9) with 0 )()( ii qNNs , )()( NNr i )2,1(0 i pi, and 12 12 N N Ns qq Nr pp u( x)xyyuyvydy v( x)xyyvyuydy (10) 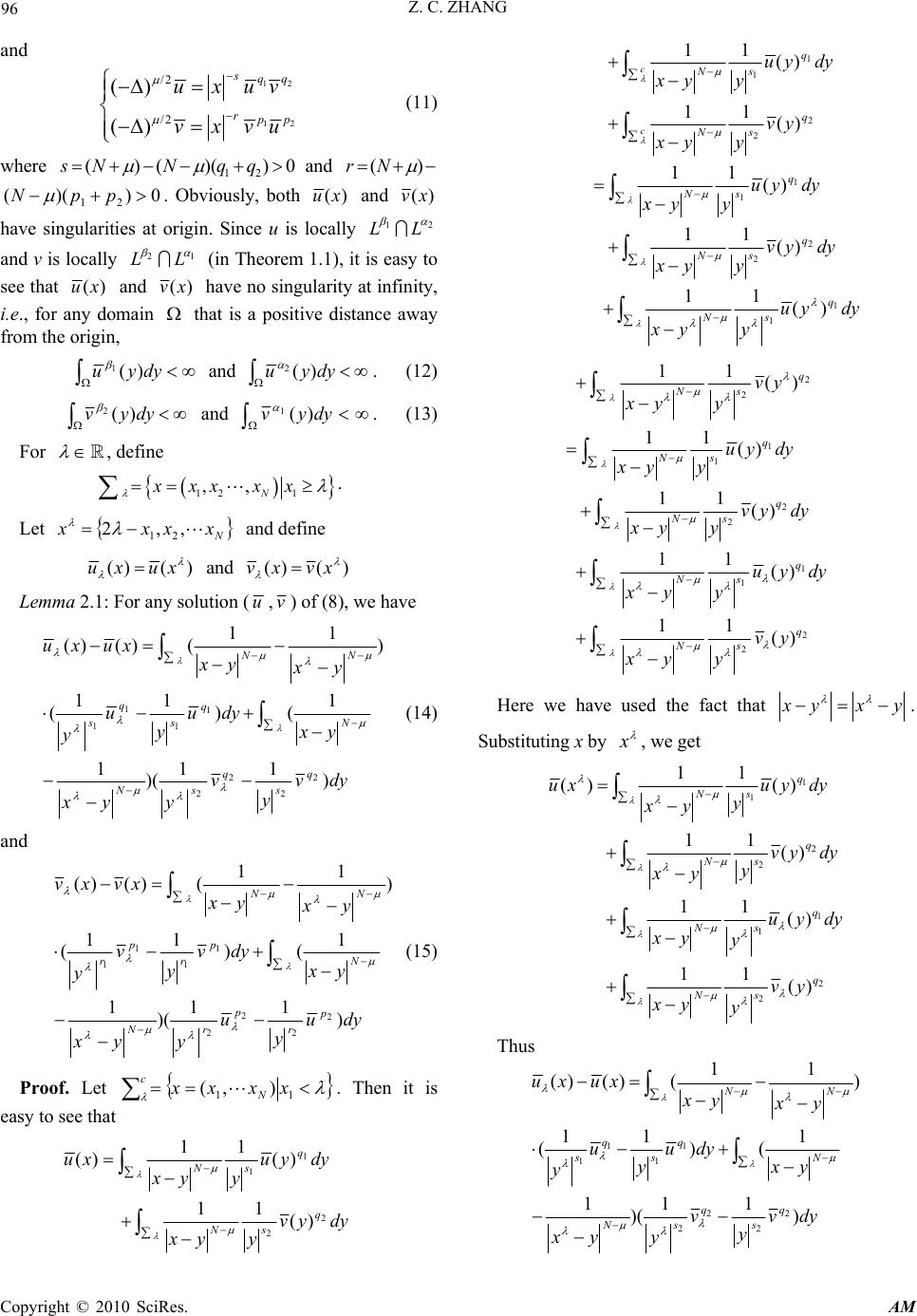 Z. C. ZHANG Copyright © 2010 SciRes. AM 96 and 12 12 /2 /2 () () sqq rpp uxuv vxvu (11) where 0))(()( 21 qqNNs and )( Nr 0))(( 21 ppN . Obviously, both )(xu and )(xv have singularities at origin. Since u is locally 21 LL and v is locally 12 LL (in Theorem 1.1), it is easy to see that )(xu and )(xv have no singularity at infinity, i.e., for any domain that is a positive distance away from the origin, dyyu )( 1 and dyyu )( 2 . (12) dyyv)( 2 and dyyv )( 1 . (13) For , define 12 1 ,, N xxxxx . Let N xxxx ,,221 and define )()( xuxu and )()( xvxv Lemma 2.1: For any solution (u,v) of (8), we have dyv y v yyx yx dyu y u y yx yx xuxu q s q sN N q s q s NN ) 11 )( 1 1 () 11 ( ) 11 ()()( 2 2 2 2 1 1 1 1 (14) and dyu y u yyx yx dyv y v y yx yx xvxv p r p rN N p r p r NN ) 11 )( 1 1 () 11 ( ) 11 ()()( 2 2 2 2 1 1 1 1 (15) Proof. Let c Nxxxx 11 ),(. Then it is easy to see that dyyv yyx dyyu yyx xu q sN q sN 2 2 1 1 )( 11 )( 11 )( dyyu yyx dyyv yyx dyyu yyx yv yyx dyyu yyx q sN q sN q sN q sN q sN c c 1 1 2 2 1 1 2 2 1 1 )( 11 )( 11 )( 11 )( 11 )( 11 2 2 1 1 2 2 1 1 2 2 )( 11 )( 11 )( 11 )( 11 )( 11 q sN q sN q sN q sN q sN yv yyx dyyu yyx dyyv yyx dyyu yyx yv yyx Here we have used the fact that yxyx . Substituting x by x, we get 2 2 1 1 2 2 1 1 )( 11 )( 11 )( 11 )( 11 )( q sN q sN q sN q sN yv y yx dyyu y yx dyyv y yx dyyu y yx xu Thus dyv y v yyx yx dyu y u y yx yx xuxu q s q sN N q s q s NN ) 11 )( 1 1 () 11 ( ) 11 ()()( 2 2 2 2 1 1 1 1  Z. C. ZHANG Copyright © 2010 SciRes. AM 97 This implies (14). Similarly, we can get (15). So Lemma 2.1 is proved. Proof of Theorem 1.1. Outline: Let x1 and x2 be any two points in N . We shall show that )()( 21 xuxu and )()(21 xvxv and therefore u and v must be constants. This is impossi- ble unless u = v = 0. To obtain this, we show that u and v are symmetric about the midpoint (x1 + x2) / 2. Since the integral equations are invariant under translation, we may assume that the midpoint is at the origin. Let uand v be the Kelvin-type transformations of u and v respec- tively. Then what left to prove is that u and v are sym- metric about the origin. We shall carry this out in the following three steps. Step 1. Define )}()( ,{xuxuxx u and )}()( ,{xvxvxx v We want to show that for sufficiently negative values of ¸ both u and v ¸ must be empty. We note that yxyx ¸ x, y . Moreover, since < 0, yy for any y. Then by (14), for any u x , 111 222 111 222 111 1 ()() () (()()) () (()()) (() ()) (()()) [( )] u v N N sqq N N sqq Ns qq Ns qq Ns q ux ux xyx y yuyuydy xyxy yvyvydy xyyuyuydy x yyvyvydy qxyyuuu 221 2 () [()]() u v Ns q ydy qxyyvvvydy It follows from (7) and then the Hölder inequality that, for any )}/(),/(max{ 21 sNNsNN , )( 1 )( 1 1 ))](([ u u u L q sN L dyyuuu yyxC uu 2 2 1 1 2 2 1 () 1 () () 1 () () [()]() v u uu vv Ns q L q LL q LL xy y vvvydy Cuu u vvv (16) where 1 1 1 1 1 q N N q and 1 1 2 2 2 q N N q . Similarly, one can show that 1 1 2 2 () 1 () () 1 () () v vv uu L p LL p LL vv Cvv v uuu (17) where 1 1 1 1 1 p N N p and 1 1 2 2 2 p N N p . Combining (16) and (17), we have 12 12 12 12 () 1 11 ()() 1 11 () () () 1 1 u uu vv u L qp LL pq LL L uu CCu u Cv v uu (18) By conditions (12) and (13), we can choose N suffi- ciently large, such that for N , we have 12 12 12 12 1 11 () () 1 11 () () 1 1 1 . 2 uu vv qp LL pq LL CCu u Cv v Then (18) implies that 0 )( u L uu , and therefore u must be measure zero, and hence empty. Similarly, one can show that v is empty. Step 2. Now we have that for N , )()( xuxu and )()( xvxv x. (19) So the plane can start moving continuously from N to the right as long as (19) holds. Suppose that there exists 0 0 such that, on 0 , )()( 0xuxu and )()( 0xvxv ,  Z. C. ZHANG Copyright © 2010 SciRes. AM 98 but meas 00 ()() 0xuxux or meas 0 x 0 ()() 0vxv x . We shall show that the plane can move further to the right, i.e., there exists an depending on N, pi, qi (i = 1, 2), and the solutions (vu,) such that (19) holds for all ),[00 . Once either meas 00 ()()0xuxux or meas 00 ()()0xvxvx holds, we see by (14) that in )()( 0xuxu the interior of 0 . Let )()(, 00 0 ~ xuxu xx u and 000 , ()() ~ v x xvxvx . It is easy to see that ~ 0 u has measure zero, and ~ 00 0 lim uu . The similar results also hold for v. Let be the reflection of the set about the plane 1 x. From (16) and (17), we deduce 12 12 12 12 () 1 11 (( )*)(( )*) 1 11 (( )*)(( )*) () (1 ) (1) u uu vv u L qp LL pq LL L uu CCuu Cv v uu (20) Again conditions (12) and (13) ensure that one can choose small enough such that, for all 00 [, ) , 12 12 12 12 1 11 (( )*)(( )*) 1 11 (( )*)(( )*) (1 ) 1 (1) . 2 uu vv qp LL pq LL CCuu Cv v Now by (20), we have () 0 u L uu and therefore u must be empty. Similarly, v must be empty, too. Step 3. If the plane stops at 0 0 , then )(xu and )(xv must be symmetric and monotone about the plane x1 = 0 . This implies that )(xu and )(xv have no singularity at the origin. But Equation (8) tells us that this is impossible if )(xu and )(xv are nontrivial. Hence we can move the plane to x1 = 0. Then )(xu and )(xv must be symmetric about the origin. Thus u = v = 0. This completes the proof. 3. Proof of Theorem 1.2 In this section we establish Theorem 1.2. The proof is along the same line of the proof of Theorem 1.1. First establishing Lemma 3.1 which is similar to Lemma 2.1, we omit its proof. Lemma 3.1: For any solution (vu,) of (10), we have dyvu y vu y yxyx xuxu qq s qq s NN ) 11 ( ) 11 ()()( 21 21 (21) and dyuv y uv y yxyx xvxv pp r pp r NN ) 11 ( ) 11 ()()( 21 21 (22) Proof of Theorem 1.2. Here we only provide necessary changes in Step 1, the rest is same as the proof of Theorem 1.1. u and v are defined as above. We want to show that for suffi- ciently negative values of , both u and v must be empty. We note that yxyx x, y. Moreover, since < 0, yy for any y. Then by (21), for any u x , 12 12 21 1 122 12 1 1 2 ()() () ()()()() ()[()()] ()[()()] [()]() u v u N Ns qq qq Ns qq q Ns qq q Ns qq ux ux xyx yy uyvyuyvydy x yyvyuyuydy x yyuyvyvydy qxyyuvuuydy qx 21 1 [()](). v Ns qq yyvuvvydy It follows from (7) and then the Hölder inequality that, for any )/( sNN ,  Z. C. ZHANG Copyright © 2010 SciRes. AM 99 )( 1 )( 1 )( ))](([ ))](([ 12 2 1 u v u u u L qq sN L q q sN L dyyvvuv yyx dyyuuvu yyxC uu 12 12 1 ()() () 1 () ()() u uu vu v qq LLL qq LL L Cuvuu uv vv (23) Similarly, one can show that 12 12 () 11 () ()() 1 () () () v uu u v vv L pp LL L pp L LL vv Cvuu u vuvv (24) where 1)( 1 21 21 pp N N pp and 1)( 1 21 21 qq N N qq . Combining (23) and (24), we have 12 12 12 12 () 1 1 ()() 1 ()() 1 1 () () 1 () ()() (1 (1 u u u vv v v uu u L qq LL qq LL pp L L pp LLL uu CCuv uv Cv u vu uu (25) By the hypotheses in Theorem 1.2, we can choose N sufficiently large, such that for N , 12 12 12 12 1 1 () () 1 ()() 1 1 () () 1 () () (1 (1 1 . 2 u u vv v v uu qq L L qq LL pp L L pp LL CCuv uv Cv u vu Then (25) implies that () 0 u L uu , and therefore u must be measure zero, and hence empty. Similarly v is also empty. 4. Acknowledgements This work is supported by Youth Foundation of NSFC (No. 10701061), and it was completed while the author was visiting University of Notre Dame. 5. References [1] W. X. Chen and C. M. Li, “Classification of Positive Solutions for Nonlinear Differential and Integral Systems with Critical Exponents,” Acta Mathematica Scientia, in press. [2] W. X. Chen, C. M. Li and B. Ou, “Classification of Solu- tions for an Integral Equation,” Communications on Pure and Applied Mathematics, Vol. 59, No. 3, 2006, pp. 330- 343. [3] L. Caffarelli, B. Gidas and J. Spruck, “Asymptotic Sym- metry and Local Behavior of Semilinear Elliptic Equa- tions with Critical Sobolev Growth,” Communications on Pure and Applied Mathematics, Vol. 42, No. 3, 1989, pp. 271-297. [4] B. Gidas and J. Spruck, “Global and Local Behaviour of Positive Solutions of Nonlinear Elliptic Equations,” Communications on Pure and Applied Mathematics, Vol. 34, No. 6, 1981, pp. 525-598. [5] S. H. Chen and G. Z. Lu, “Existence and Nonexistence of Positive Radial Solutions for a Class of Semilinear Ellip- tic System,” Nonlinear Analysis, Vol. 38, No. 7, 1999, pp. 919-932. [6] E. Mitidieri, “Nonexistence of Positive Solutions of Se- milinear Elliptic Systems in N ,” Differential and Inte- gral Equations, Vol. 9, No. 3, 1996, pp. 465-479. [7] E. Lieb, “Sharp Constants in the Hardy-Littlewood- Sobolev and Related Inequalities,” Annals of Mathemat- ics, Vol. 118, 1983, pp. 349-374. [8] D. G. de Figueiredo and P. L. Felmer, “A Liouville-Type Theorem for Elliptic Systems,” Annali della Scuola Nor- male Superiore di Pisa XXI, Vol. 21, No. 3, 1994, pp. 387-397. [9] J. Busca and R. Manásevich, “A Liouville-Type Theorem for Lane-Emden Systems,” Indiana University Mathe- matics Journal, Vol. 51, No. 1, 2002, pp. 37-51. [10] Z. C. Zhang, W. M. Wang and K. T. Li, “Liouville-Type Theorems for Semilinear Elliptic Systems,” Journal of Partial Differential Equations, Vol. 18, No. 4, 2005, pp. 304-310. [11] Y. Y. Li and M. Zhu, “Uniqueness Theorems through the Method of Moving Spheres,” Duke Mathematical Journal, Vol. 80, No. 2, 1995, pp. 383-417. [12] Y. Y. Li and L. Zhang, “Liouville-Type Theorems and Harnack-Type Inequalities for Semilinear Elliptic Equa- tions,” Journal d’Analyse Mathematique, Vol. 90, 2003, pp. 27-87.  Z. C. ZHANG Copyright © 2010 SciRes. AM 100 [13] W. X. Chen, C. M. Li and B. Ou, “Classification of Solu- tions for a System of Integral Equations,” Communica- tions in Partial Differential Equations, Vol. 30, No. 1-2, 2005, pp. 59-65. [14] W. X. Chen, C. M. Li and B. Ou, “Qualitative Properties of Solutions for an Integral Equation,” Discrete and Con- tinuous Dynamical Systems, Vol. 12, No. 2, 2005, pp. 347-354. [15] L. Ma and D. Z. Chen, “A Liouville Type Theorem for an Integral System,” Communications on Pure and Applied Analysis, Vol. 5, No. 4, 2006, pp. 855-859. [16] C. S. Lin, “A Classification of Solutions of a Confor- mally Invariant Fourth Order Equation in N ,” Com- mentarii Mathematici Helvetici, Vol. 73, No. 2, 1998, pp. 206-231. [17] L. A. Peletier and R. C. A. M. van der Vorst, “Existence and Non-Existence of Positive Solutions of Non-Linear Elliptic Systems and the Biharmonic Equation,” Differen- tial and Integral Equations, Vol. 5, No. 4, 1992, pp. 747- 767. [18] J. Serrin and H. Zou, “The Existence of Positive Entire Solutions of Elliptic Hamitonian Systems,” Communica- tions in Partial Differential Equations, Vol. 23, No. 3-4, 1998, pp. 577-599. [19] M. A. Souto, “A Priori Estimates and Existence of Posi- tive Solutions of Semilinear Elliptic Systems,” Differen- tial and Integral Equations, Vol. 8, No. 5, 1995, pp. 1245- 1258. [20] E. M. Stein, “Singular Integral and Differentiability Properties of Functions,” Princeton University Press, Princeton, 1970. [21] Z. C. Zhang and Z. M. Guo, “Structure of Nontrivial Nonnegative Solutions of Singularly Perturbed Quasilin- ear Dirichlet Problems,” Mathematische Nachrichten, Vol. 280, No. 13-14, 2007, pp. 1620-1639. [22] Z. C. Zhang and K. T. Li, “Spike-Layered Solutions of Singularly Perturbed Quasilinear Dirichlet Problems,” Journal of Mathematical Analysis and Applications, Vol. 283, No. 2, 2003, pp. 667-680. [23] Z. C. Zhang and K. T. Li, “Spike-Layered Solutions with Compact Support to Some Singularly Perturbed Qua- silinear Elliptic Problems in General Smooth Domains,” Journal of Computational and Applied Mathematics, Vol. 162, No. 2, 2004, pp. 327-340. [24] L. P. Zhu, Z. C. Zhang and W. M. Wang, “On the Posi- tive Solutions for a Class of Semilinear Elliptic Systems,” Mathematical Applications, Vol. 19, No. 2, 2006, pp. 440- 445. |

