Applied Mathematics, 2010, 1, 87-93
doi:10.4236/am.2010.12011 Published Online July 2010 (http://www. SciRP.org/journal/am)
A Subclass of Harmonic Functions Associated with
Wright’s Hypergeometric Functions
Gangadharan Murugusundaramoorthy, Kalliyapan Vijaya
School of Advanced Sciences, Vellore Institute of Technology University, Vellore, India
Received May 7, 2010; revised May 21, 2010; accepted June 25, 2010
Abstract
We introduce a new class of complex valued harmonic functions associated with Wright hypergeometric
functions which are orientation preserving and univalent in the open unit disc. Further we define, Wright
generalized operator on harmonic function and investigate the coefficient bounds, distortion inequalities and
extreme points for this generalized class of functions.
Keywords: Harmonic Univalent Starlike Functions, Harmonic Convex Functions, Wright Hypergeometric
Functions, Raina-Dziok Operator, Distortion Bounds, Extreme Points
1. Introduction
A continuous function f = u + iv is a complex-valued
harmonic function in a complex domain G if both u and v
are real and harmonic in G. In any simply-connected
domain D ⊂ G, we can write ghf  , where h and
g are analytic in D. We call h the analytic part and g the
co-analytic part of f. A necessary and sufficient condition
for f to be locally univalent and orientation preserving in
D is that |)('||)('| zgzh  in D (see [1]).
Denote by H the family of functions
ghf  (1)
which are harmonic, univalent and orientation preserving
in the open unit disc }1|:|{  zzU so that f is normal-
ized by 01)0()0()0(

 z fhf. Thus, for ghf


∈ H, we may express
,1||,)( 1
12
  



bzbzazzf
m
m
m
m
m
m (2)
where the analytic functions h and g are in the forms
1
21
(),()(|| 1).
mm
mm
mm
hzzazgzb zb


 

We note that the family H of orientation preserving,
normalized harmonic univalent functions reduces to the
well known class S of normalized univalent functions if
the co-analytic part of f is identically zero, that is g ≡ 0.
Let the Hadamard product (or convolution) of two po-
wer series




2
)(
m
m
mzzz
 (3)
and




2
)(
m
m
mzz
 (4)
in S be defined by




2
))(*(
m
m
mm zz
 .
For positive real parameters 1

, A1,..., p

, p A and
1

, B1,..., q

q
B( p, q

N = 1, 2, 3, ...) such that
.,0,1
11
UzAB
p
m
m
q
m
m  
(5)
The Wright’s generalized hypergeometric function [2]









11 11
1, 1,
,,, ,;,, ;
,,;
qppqq
qmm mm
pq
pA ABBz
pABz











is defined by





1, 1,
1
000
,,;
,.
!
pq tttt
pq
pq
tt tt
mtt
m
ABz
mA mB
zzU
m








 

 

 


 
If At = 1 (t = 1, 2, p) and Bt = 1 (t = 1, 2, q) we have

G. MURUGUSUNDARAMOORTHY ET AL.
88
the relationship:



,
!)(,)(
)(,)(
;,;,
;,,
01
1
11
,1,1
m
z
zF
zBA
m
mmqm
mpm
qpqp
q
tt
p
ttqp












 (6)
)};0{.;1( 0UzNNqpqp  is the generalized
hypergeometric function (see for details [3]) where N
denotes the set of all positive integers and n )(

is the
Pochhammer symbol and
 











 


q
t
t
p
t
t
0
1
0
 (7)
By using the generalized hypergeometric function Dz-
iok and Srivastava [3] introduced the linear operator. In
[4] Dziok and Raina extended the linear operator by us-
ing Wright generalized hypergeometric function. First
we define a function




zBA
zBA
q
tt
p
ttqp
q
tt
p
ttqp
;,,
;,,
,1,1
,1,1





Let
  SSBAWq
tt p tt:,,,1,1

be a linear operator
defined by





1, 1,
1, 1,
,,()
:,,;*()
tt tt
pq
pq tttt
pq
WAB z
zABzz

 






We observe that, for f(z) of the form (1), we have

1, 1,
1
2
,,()
:(),
tt tt
pq
n
mm
m
WAB z
zz








(8)
where )( 1


m is defined by
))1(())1(()!1(
))1(())1((
)(
11
11
1


mBmBm
mAmA
qq
pp
m




 (9)
If, for convenience, we write

 )(),(),();(),(
)(
11,1,1
1
zBBAAW
zW
qqpp


 (10)
introduced by Dziok and Raina [4].
It is of interest to note that, if At = 1 (t = 1, 2, ..., p), Bt
= 1 (t = 1, 2, ...,q) in view of the relationship (6) the linear
operator (8) includes the Dziok-Srivastava operator (see
[3]), for more details on these operators see [3,4,6,7] and
[8]. It is interesting to note that Wright generalized hy-
pergeometric function contains, Dziok-Srivastava opera-
tor as its special cases, further other linear operators the
Hohlov operator, the Carlson-Shaffer operator [6], the
Ruscheweyh derivative operator [7], the generalized
Bernardi-Libera-Livingston operator, the fractional de-
rivative operator [8], and so on. For example if p = 2 and
q = 1 with 1
1




)1( 

, 1
2

, 1
1


, then
)(*
)1(
)(
)()1;1,1(
1
2
1
z
z
z
zfD
zW









is called Ruscheweyh derivative of order δ (δ > −1).
From (8) now we define, Wright generalized hyperge-
ometric harmonic function ghf  of the form (1),
as
)(][)(][)(][ 111 zgWzhWzfW p
q
p
q
p
q
  (11)
and we call this as Wright generalized operator on har-
monic function.
Motivated by the earlier works of [1,5,9-13] on the
subject of harmonic functions, we introduce here a new
subclass )],([ 1


H
WS of H.
For ,10



let )],([1


H
WS denote the subfam-
ily of starlike harmonic functions Hf  of the form (1)
such that




))(][(arg 1zfWp
q (12)
equivalently
















)(][)(][
))('][())('][(
Re
11
11
zgWzhW
zgWzzhWz
p
q
p
q
p
q
p
q (13)
where )(][ 1zfW p
q
 is given by (11) and .Uz 
We also let HHHVWSWV  )],([)],([ 11




where
H
V the class of harmonic functions with varying argu-
ments introduced by Jahangiri and Silverman [10], con-
sisting of functions f of the form (1) in H for which there
exists a real number φ such that
,2,0
)1(),2(mod
)1(




m
m
m
m
m



(14)
where )arg( mma


and )arg(mmb

.
In this paper we obtain a sufficient coefficient condi-
tion for functions f given by (2) to be in the class
)],([ 1


H
WS . It is shown that this coefficient condition
is necessary also for functions belonging to the class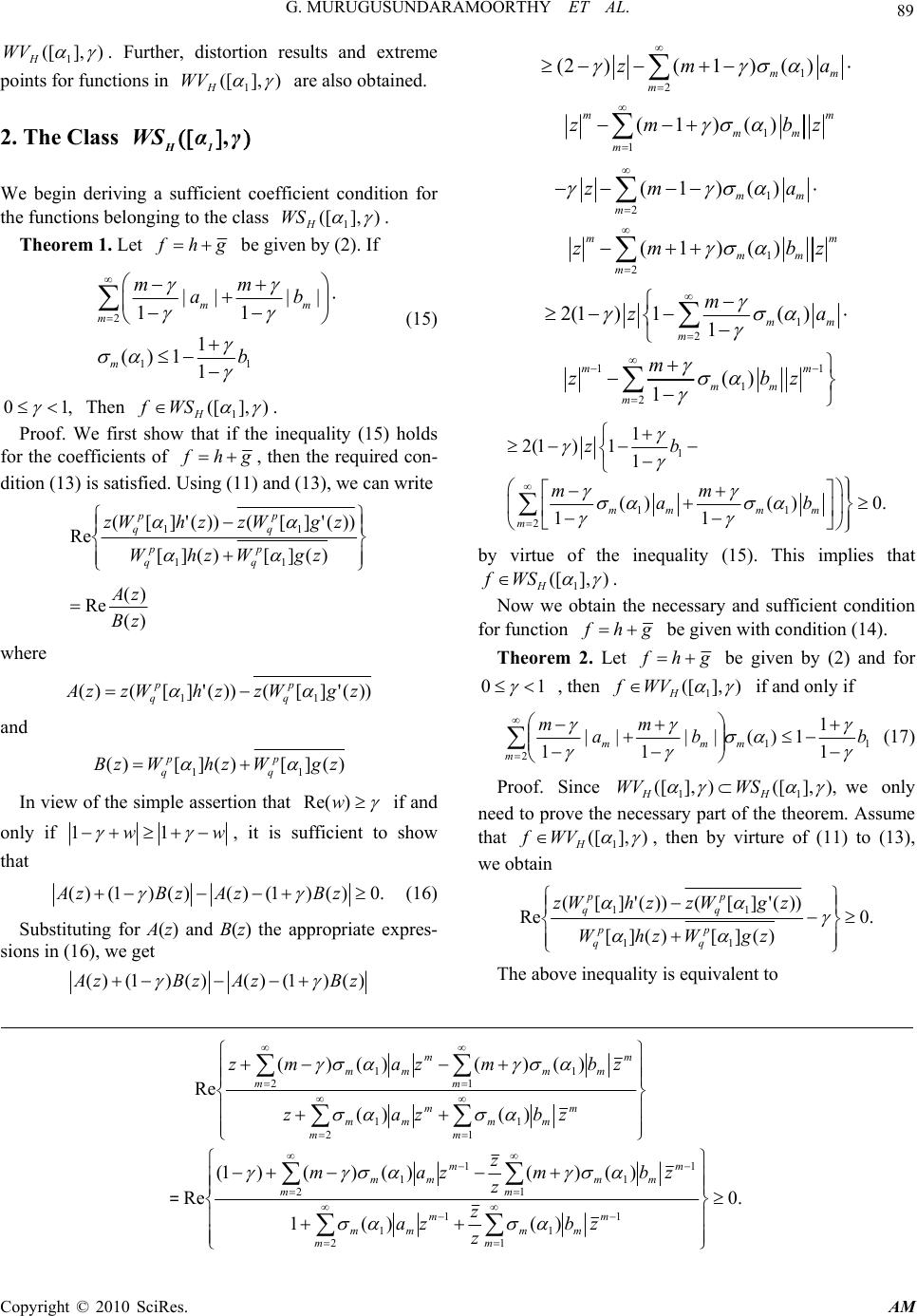

G. MURUGUSUNDARAMOORTHY ET AL.
89
)],([ 1


H
WV . Further, distortion results and extreme
points for functions in )],([ 1


H
WV are also obtained.
2. The Class ,
H1
WS α
γ

We begin deriving a sufficient coefficient condition for
the functions belonging to the class )],([ 1


H
WS .
Theorem 1. Let ghf  be given by (2). If
2
11
|| ||
11
1
()1
1
mm
m
m
mm
ab
b



 


 






 (15)
,10

Then )],([ 1


H
WSf .
Proof. We first show that if the inequality (15) holds
for the coefficients of ghf

, then the required con-
dition (13) is satisfied. Using (11) and (13), we can write
)(
)(
Re
)(][)(][
))('][())('][(
Re
11
11
zB
zA
zgWzhW
zgWzzhWz
p
q
p
q
p
q
p
q
















where
))('][())('][()( 11zgWzzhWzzA p
q
p
q
  
and
)(][)(][)( 11 zgWzhWzB p
q
p
q
  
In view of the simple assertion that

)Re( w if and
only if 11ww   , it is sufficient to show
that
()(1) ()()(1) ()0.AzBz AzBz    (16)
Substituting for A(z) and B(z) the appropriate expres-
sions in (16), we get
()(1) ()()(1)()
A
zBzAzBz   
1
2
1
1
(2)(1)( )
(1)()
mm
m
mm
mm
m
zm a
zm bz

 




 




1
2
1
2
(1)()
(1)()
mm
m
mm
mm
m
zm a
zm bz

 





 




1
2
11
1
2
2(1)1( )
1
()
1
mm
m
mm
mm
m
m
za
m
zbz











 









1
11
2
1
2(1)1 1
()() 0.
11
mm mm
m
zb
mm
ab



 























by virtue of the inequality (15). This implies that
)],([ 1


H
WSf

.
Now we obtain the necessary and sufficient condition
for function ghf


be given with condition (14).
Theorem 2. Let ghf


be given by (2) and for
10



, then )],([ 1


H
WVf

if and only if
11
21
1
1)(||
1
||
1bb
m
a
m
m
m
mm


























(17)
Proof. Since ),],([)],([ 11




HHWSWV we only
need to prove the necessary part of the theorem. Assume
that )],([ 1


H
WVf

, then by virture of (11) to (13),
we obtain
.0
)(][)(][
))('][())('][(
Re
11
11 















zgWzhW
zgWzzhWz
p
q
p
q
p
q
p
q
The above inequality is equivalent to


























m
m
mm
m
m
mm
m
m
mm
m
m
mm
zbzaz
zbmzamz
1
1
2
1
1
1
2
1
)()(
)()()()(
Re



=.0
)()(1
)()()()()1(
Re
1
1
1
1
2
1
1
1
1
1
2
1































m
m
mm
m
m
mm
m
m
mm
m
m
mm
zb
z
z
za
zbm
z
z
zam




G. MURUGUSUNDARAMOORTHY ET AL.
90
This condition must hold for all values of z, such that
|z| = r < 1. Upon choosing φ according to (14) we must
have (18).
If (17) does not hold, then the numerator in (18) is
negative for r sufficiently close to 1. Therefore, there
exists a point oo rz  in (0, 1) for which the quotient in
(18) is negative. This contradicts our assumption that
)],([ 1


H
WVf. We thus conclude that it is both nec-
essary and sufficient that the coefficient bound inequality
(17) holds true when )],([ 1


H
WVf. This completes
the proof of Theorem 2.
If we put k


2
 in (14), then Theorem 2 gives the
following corollary.
Corollary 1. A necessary and sufficient condition for
ghf  satisfying (17) to be starlike is that

)arg( m a
,/)1(2 km   and  2)arg( 
m
b,/)1(2 km

 ,1(

k
).,3,2 
3. Distortion Bounds and Extreme Points
In this section we obtain the distortion bounds for the
functions )],([ 1


H
WVf  that lead to a covering re-
sult for the family )],([ 1


H
WV .
Theorem 3. If )],([ 1


H
WVf  then
2
11
21
11 1
() (1)()22
f
zbr br


 
 




and
2
11
21
11 1
() (1).
()2 2
f
zbrbr

 


 




Proof. We will only prove the right-hand inequality of
the above theorem. The arguments for the left-hand ineq-
uality are similar and so we omit it. Let
H
f
WV


)],([ 1


taking the absolute value of f, we obtain

1
2
()
(1 )m
mm
m
fz
brab r


 



2
1
2
(1 ).
mm
m
br rab


 

This implies that
1
21
2
21 21
2
11
() (1)()2
22
() ()
11
mm
m
fzb r
abr

 

 





 




 



 

 



2
11
21
11 1
(1 )1
()21
brb r

 
 
 





2
11
21
11 1
(1 ),
()22
brb r

 
 
 




which establish the desired inequality.
As consequences of the above theorem and corollary 1,
we state the following corollary.
Corollary 2. Let ghf


and of the form (2) be so
that )],([ 1


H
WVf

. Then
21 21
21
21 21
1
21
2( )1[( )1]
:(2)( )
2()1[()1]().
(2)( )
ww
bfU
 
 

 
 




 




For a compact family, the maximum or minimum of
the real part of any continuous linear functional occurs at
one of the extreme points of the closed convex hull. Un-
like many other classes, characterized by necessary and
sufficient coefficient conditions, the family ],([ 1

H
WV
)

is not a convex family. Nevertheless, we may still
apply the coefficient characterization of the ],([ 1

H
WV
)

to determine the extreme points.
Theorem 4. The closed convex hull of )],([ 1


H
WV
(denoted by clco )],([ 1


H
WV ) is
 .1:
,)(
1
2
12

















bbam
zbzazzf
m
mm
m
m
m
m
m
m
11
11 1
2
1
11 1
21
(1)(1)() ()() ()
0.
1()()
mm
mm mm
m
m
mm mm
mm
bm arm br
babr
 
 


 


 




 




(18)

G. MURUGUSUNDARAMOORTHY ET AL.
91
By setting )()(
1
1



m
mm

 and )()(
1
1



m
mm

,
then for 1
b fixed, the extreme points for clco 1
([ ],
H
WV

)

are

 11
mm
mm
zxzbzzbzxz   (19)
where 2m and ||1|| 1
bx  .
Proof. Any function f in clco )],([1


H
WV be ex-
pressed as
,)(
2
1
2
 




m
m
i
m
m
m
i
mzebzbzeazzf mm


where the coefficient satisfy the inequality (15). Set
,)(
1zzh  ,)( 11 zbzg  ,)( m
i
mm zezzh m

  1
()
m
g
zbz

,
m
im
mez

  for m = 2, 3,… Writing
m
m
m
a
X

,m
m
m
b
Y

 m = 2, 3... and ;1
2
1



m
m
XX
;1
2
1



m
m
YY we get
.)]()([)(
1




m
mmmm zgYzhXzf 
In particular, putting
zbzzf 11)( 
and
m
m
m
mmyzzbxzzzf
  1 )( ,
1
(2, 1)mxy b 
We see that extreme points of functions in clco
H
WV

)}({)],([ 1zfm 


. To see that m f is not an extreme
point if both 0|| x and 0||

y, we will show that it
can then also be expressed as a convex linear combina-
tions of functions in clco )],([1


H
WV . Without loss of
generality, assume |x| ≥ |y|. Choose 0 small enough
so that y
x
 . Set

1
A
and y
x
B  1. We
then see that both
m
m
m
mByzzbAxzzzt
  11 )( 
and
m
m
m
myzBzbxzAzzt )2()2()( 12 
 
are in clco )],([ 1


H
WV , and that )({
2
1
)( 1ztzfm
)}(
2zt. The extremal coefficient bounds show that
functions of the form (19) are the extreme points for clco
)],([ 1


H
WV , and so the proof is complete.
4. Inclusion Relation
Following Avici and Zlotkiewicz [9] (see also Rusch-
eweyh [14]), we refer to the δ-neighborhood of the func-
tion f(z) defined by (2) to be the set of functions F for
which

22
11
2
():(),
.
mm
mm
mm
mm mm
m
NfFz zAzBz
maAbBb B







 



 




(20)
In our case, let us define the generalized δ−neighbor-
hood of f to be the set

1
2
11
() ::()[()
() ](1)(1).
mmm
m
mm
NfFmaA
mbB bB

 








 

(21)
Theorem 5. Let f be given by (2). If f satisfies the
conditions
,1)()(
)()(
1
1
1
2










m
mm
m
m
m
bmm
amm
(21)
10



and 10



, then )],([)( 1


 H
WSfN .
Proof. Let f satisfy (22) and F(z) be given by
,)(
22
 




m
m
m
m
m
mzBzAzzF 
which belongs to N(f). We obtain
)()()(||)1( 1
2
1

mm
m
mBmAmB  



111
1
2
1
2
(1 )(1 )
()()
()
()( )()
mmm
m
mm
mmm
m
Bb b
mAa
mBb
mamb

 

 




 

 








1
1
2
(1 )(1 )
1
()()()
2mmm
m
b
mmamb

 



 



G. MURUGUSUNDARAMOORTHY ET AL.
92
 .1)1()1(
2
1
||)1()1( 11 

 

 bb 
Hence for 












||
1
1
1
2
1
1
b





, we infer that
)],([ 1


H
WS which concludes the proof of Theorem 5.
Now, we will examine the closure properties of the
class )],([ 1


H
WV under the generalized Bernardi-Lib-
era-Livingston integral operator )( fLc which is de-
fined by



z
c
c
ccdttft
z
c
fL
0
1.1,)(
1
)( 
Theorem 6. Let )],([ 1


H
WVf. Then

)( fLc
)],([ 1


H
WV .
Proof. From the representation of )( fLc, it follows
that





z
c
c
cdttgtht
z
c
fL
0
1)()(
1
)( 































z
m
m
m
c
z
m
m
m
c
c
dttbt
dttatt
z
c
01
1
02
1
1

  




12 m
m
m
m
m
mzBzAz , 
where
mma
mc
c
A

1
;mm b
mc
c
B

1
.
Therefore
)(||
1
1
||
1
11
1





m
m
mm b
mc
cm
a
mc
cm



















)(||
1
||
11
1





m
m
mm b
m
a
m















).1(2



Since )],([ 1


H
WVf , therefore by Theorem 2,
)],([)(1


Hc WVfL.
Theorem 7. For ,10




let )],([ 1


H
WVf 
and )],([ 1


H
WVF. Then  )],([)*( 1


H
WVFf
)],([ 1


H
WV .
Proof. Let
)],([)( 1
12

H
m
m
m
m
m
mWVzbzazzf   




and
)],([)(1
12

H
m
m
m
m
m
mWVzBzAzzF  




Then
 




12
)(*)(
m
m
mm
m
m
mm zbBzaAzzFzf 
For )],([)*( 1


H
WVFf

we note that 1
m
A
and 1
m
B. Now by Theorem 2, we have











1
1
2
1
||||
1
)()(
||||
1
)()(
m
mm
m
m
mm
m
Bb
m
Aa
m





 








1
1
2
1||
1
)()(
||
1
)()(
m
m
m
m
m
mb
m
a
m





.1||
1
)()(
||
1
)()(
1
1
2
1





 


m
m
m
m
m
mb
m
a
m





Therefore )],([)],([)*( 11




HH WVWVFf 

and
since the above inequality bounded by )1(2


while
)1(2)1(2





.
5. Concluding Remarks
The various results presented in this paper would provide
interesting extensions and generalizations of those con-
sidered earlier for simpler harmonic function classes (see
[10,12,13]). The details involved in the derivations of
such specializations of the results presented in this paper
are fairly straight-forward.
6. References
[1] J. Clunie and T. Sheil-Small, “Harmonic Univalent Func-
tions,” Annales Academiae Scientiarum Fennicae, Series
A I, Mathematica, Vol. 9, 1984, pp. 3-25.
[2] E. M. Wright, “The Asymptotic Expansion of the Gener-
alized Hypergeometric Function,” Proceedings of the
London Mathematical Society, Vol. 46, 1946, pp. 389-408.
[3] J. Dziok and H. M. Srivastava, “Certain Subclasses of
Analytic Functions Associated with the Generalized Hy-
pergeometric Function,” Integral Transforms and Special
Functions, Vol. 14, No. 1, 2003, pp. 7-18.
[4] J. Dziok and R. K. Raina, “Families of Analytic Func-
tions Associated with the Wright Generalized Hyper-
geometric Function,” Demonstratio Mathematica, Vol. 37,
No. 3, 2004, pp. 533-542.
[5] J. M. Jahangiri, “Harmonic Functions Starlike in the Unit
Disc,” Journal of Mathematical Analysis and Applica-
tions, Vol. 235, No. 2, 1999, pp. 470-477.

G. MURUGUSUNDARAMOORTHY ET AL.
93
[6] B. C. Carlson and D. B. Shaffer, “Starlike and Prestarlike
Hypergeometric Functions,” SIAM Journal on Mathe-
matical Analysis, Vol. 15, No. 4, 1984, pp. 737-745.
[7] S. Ruscheweyh, “New Criteria for Univalent Functions,”
Proceedings of the American Mathematical Society, Vol.
49, No. 1, 1975, pp. 109-115.
[8] H. M. Srivastava and S. Owa, “Some Characterization
and Distortion Theorems Involving Fractional Calculus,
Generalized Hypergeometric Functions, Hadamard Prod-
ucts, Linear Operators and Certain Subclasses of Analytic
Functions,” Nagoya Mathematics Journal, Vol. 106, 1987,
pp. 1-28.
[9] Y. Avici and E. Zlotkiewicz, “On Harmonic Univalent
Mappings,” Annales Universitatis Mariae Curie-Skłodowska,
Sectio A, Vol. 44, 1990, pp. 1-7.
[10] J. M. Jahangiri and H. Silverman, “Harmonic Univalent
Functions with Varying Arguments,” International Jour-
nal of Applied Mathematics, Vol. 8, No. 3, 2002, pp. 267-
275.
[11] J. M. Jahangiri, G. Murugusundaramoorthy and K. Vijaya,
“Starlikeness of Rucheweyh Type Harmonic Univalent
Functions,” Journal of the Indian Academy of Mathemat-
ics, Vol. 26, No. 1, 2004, pp. 191-200.
[12] G. Murugusundaramoorthy, “A Class of Ruscheweyh-Type
Harmonic Univalent Functions with Varying Arguments,”
Southwest Journal of Pure and Applied Mathematics, No.
2, 2003, pp. 90-95.
[13] K. Vijaya, “Studies on Certain Subclasses of Harmonic
Functions,” Ph.D. Thesis, Vellore Institute of Technology
University, Vellore, September 2006.
[14] S. Ruscheweyh, “Neighborhoods of Univalent Func-
tions,” Proceedings of the American Mathematical Soci-
ety, Vol. 81, No. 4, 1981, pp. 521-528.