Applied Mathematics
Vol.4 No.1(2013), Article ID:27224,11 pages DOI:10.4236/am.2013.41022
Optimal System of Subalgebras for the Reduction of the Navier-Stokes Equations
1Department of Mathematics, Faculty of Science, Naresuan University, Phitsanulok, Thailand
2Centre of Excellence in Mathematics, CHE, Bangkok, Thailand
Email: kuntimak@nu.ac.th
Received October 31, 2012; revised November 30, 2012; accepted December 7, 2012
Keywords: Optimal System; Invariant Solutions; Partially Invariant Solutions; Navier-Stokes Equations
ABSTRACT
The purpose of this paper is to find the admitted Lie group of the reduction of the Navier-Stokes equations 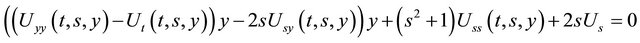 where
where  using the basic Lie symmetry method. This equation is constructed from the Navier-Stokes equations rising to a partially invariant solutions of the Navier-Stokes equations. Two-dimensional optimal system is determined for symmetry algebras obtained through classification of their subalgebras. Some invariant solutions are also found.
using the basic Lie symmetry method. This equation is constructed from the Navier-Stokes equations rising to a partially invariant solutions of the Navier-Stokes equations. Two-dimensional optimal system is determined for symmetry algebras obtained through classification of their subalgebras. Some invariant solutions are also found.
1. Introduction
Mathematical modeling is a basis for analyzing physical phenomena. Almost all fundamental equations of mathematical physics are nonlinear, and in general, are very difficult to solve explicitly. Group analysis is a method for constructing exact solutions of differential equations. This method uses the symmetry properties for constructing exact solutions. There are two types of solutions, the class of invariant solutions and partially invariant solutions which can be obtained by group analysis. Constructing of invariant and partially invariant solutions consists of some steps: choosing a subgroup of the admitted group, finding a representation of solution, substituting the representation into the studied system of equations and the study of compatibility of the obtained (reduced) system of equations.
This paper is devoted to use the basic Lie symmetry method for finding the admitted Lie group of the reduction of the Navier-Stokes equations,
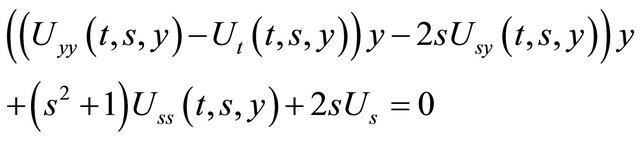 (1)
(1)
where 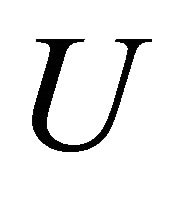 is a dependent variable and
is a dependent variable and 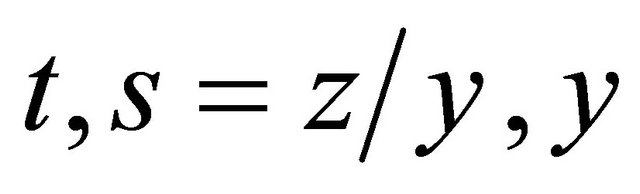 are independent variables. This equation is constructed from the Navier-Stokes equations. Subgroups for studying are taken from the part of optimal system of subalgebras considered for the gas dynamics equations [1]. One subgroup is not admitted the Navier-Stokes equations, partially invariant solutions can be found for the NavierStokes equations. These facts allow us to assume that one can construct partially invariant solution with respect to a Lie group, which is not necessary admitted. The proposed research will deal with two-dimensional optimal system of subalgebras for the reduction of the NavierStokes equations [1]. It is determined for symmetry algebras obtained through classification of their subalgebras. Example of some invariant solutions are also found. They can return to new solutions of the NavierStokes equations.
are independent variables. This equation is constructed from the Navier-Stokes equations. Subgroups for studying are taken from the part of optimal system of subalgebras considered for the gas dynamics equations [1]. One subgroup is not admitted the Navier-Stokes equations, partially invariant solutions can be found for the NavierStokes equations. These facts allow us to assume that one can construct partially invariant solution with respect to a Lie group, which is not necessary admitted. The proposed research will deal with two-dimensional optimal system of subalgebras for the reduction of the NavierStokes equations [1]. It is determined for symmetry algebras obtained through classification of their subalgebras. Example of some invariant solutions are also found. They can return to new solutions of the NavierStokes equations.
2. Invariant and Partially Invariant Solutions
The notion of invariant solution was introduced by Sophus Lie [2]. The notion of a partially invariant solution was introduced by Ovsiannikov [3]. This notion of partially invariant solutions generalizes the notion of an invariant solution, and extends the scope of applications of group analysis for constructing exact solutions of partial differential equations. The algorithm of finding invariant and partially invariant solutions consists of the following steps.
Let 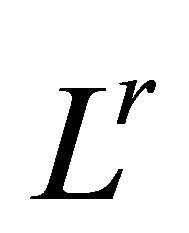 be a Lie algebra with the basis
be a Lie algebra with the basis . The universal invariant J consists of
. The universal invariant J consists of 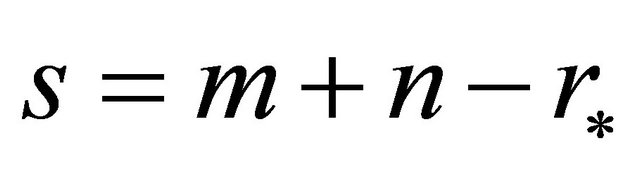 functionally independent invariants
functionally independent invariants
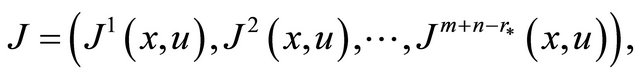
where 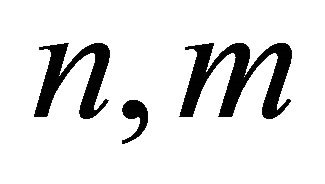 are the numbers of independent and dependent variables, respectively and
are the numbers of independent and dependent variables, respectively and ![]() is the total rank of the matrix composed by the coefficients of the generators
is the total rank of the matrix composed by the coefficients of the generators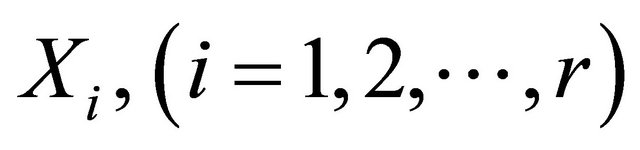 . If the rank of the Jacobi matrix
. If the rank of the Jacobi matrix
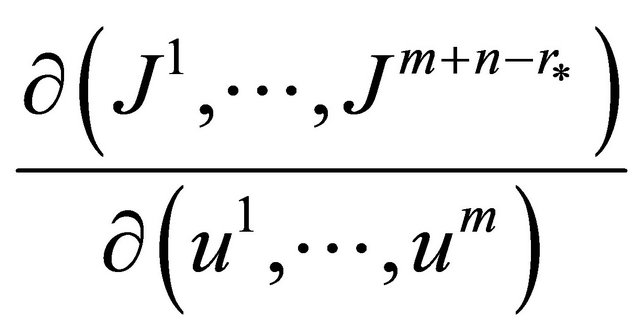 is equal to
is equal to , then one can choose the first
, then one can choose the first 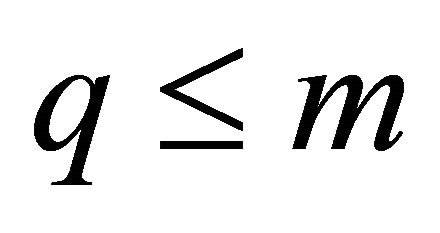 invariants
invariants  such that the rank of the Jacobi matrix
such that the rank of the Jacobi matrix 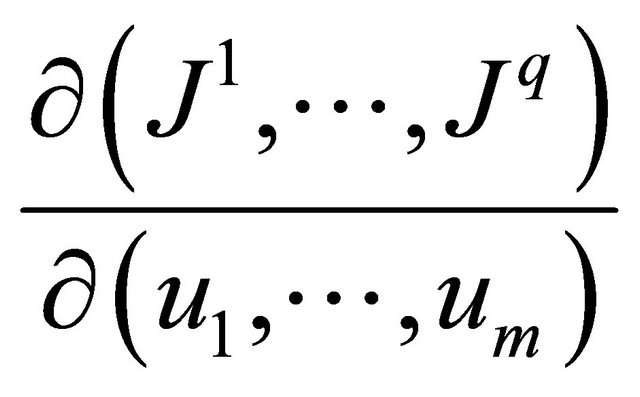 is equal to
is equal to . A partially invariant solution is characterized by two integers:
. A partially invariant solution is characterized by two integers: 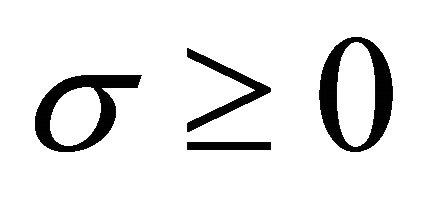 and
and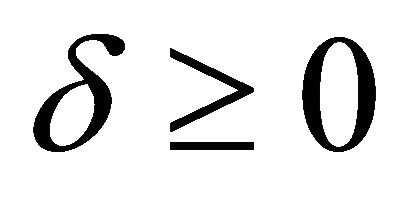 . These solutions are also called
. These solutions are also called  -solutions. The number
-solutions. The number ![]() is called the rank of a partially invariant solution. This number gives the number of the independent variables in the representation of the partially invariant solution. The number
is called the rank of a partially invariant solution. This number gives the number of the independent variables in the representation of the partially invariant solution. The number ![]() is called the defect of a partially invariant solution. The defect is the number of the dependent functions which can not be found from the representation of partially invariant solution. The rank
is called the defect of a partially invariant solution. The defect is the number of the dependent functions which can not be found from the representation of partially invariant solution. The rank ![]() and the defect
and the defect ![]() must satisfy the conditions
must satisfy the conditions
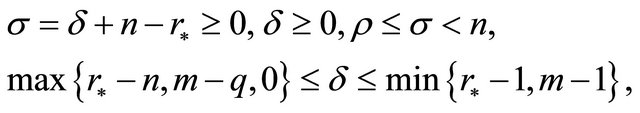
where 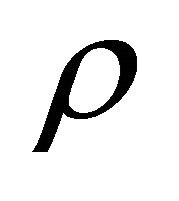 is the maximum number of invariants which depends on the independent variables only. Note that for invariant solutions,
is the maximum number of invariants which depends on the independent variables only. Note that for invariant solutions, 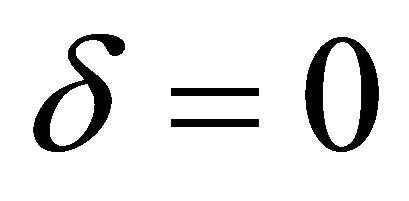 and
and .
.
For constructing a representation of a 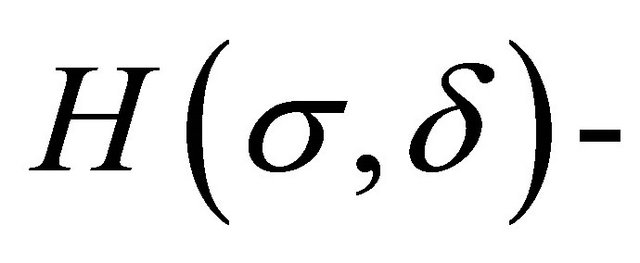 solution one needs to choose
solution one needs to choose  invariants and separate the universal invariant in two parts:
invariants and separate the universal invariant in two parts:
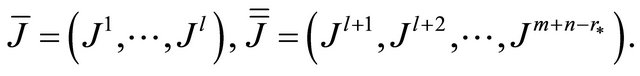
The number  satisfies the inequality
satisfies the inequality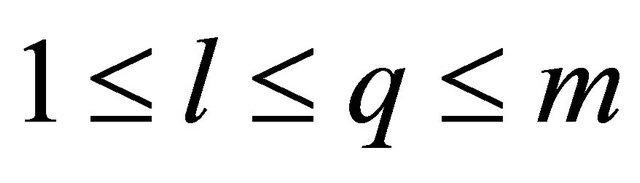 . The representation of the
. The representation of the 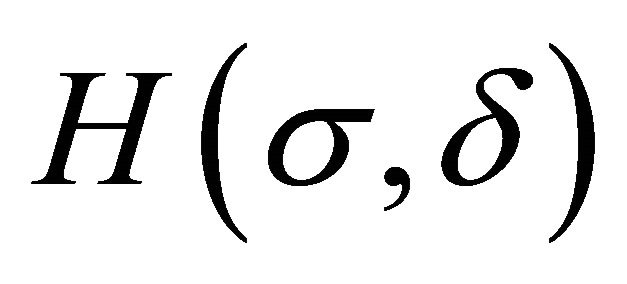 -solution is obtained by assuming that the first
-solution is obtained by assuming that the first  coordinates
coordinates  of the universal invariant are functions of the invariants
of the universal invariant are functions of the invariants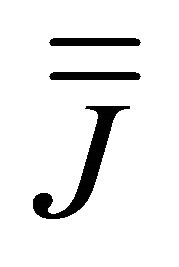 :
:
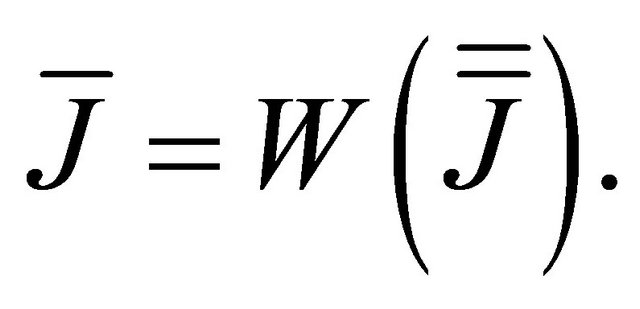 (2)
(2)
Equation (2) form the invariant part of the representation of a solution. The next assumption about a partially invariant solution is that Equation (2) can be solved for the first  dependent functions, for example,
dependent functions, for example,
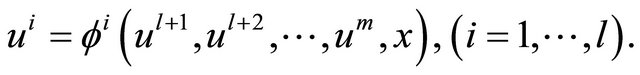 (3)
(3)
It is important to note that the functions 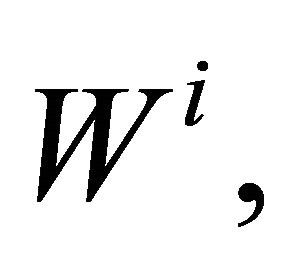
 are involved in the expressions for the functions
are involved in the expressions for the functions . The functions
. The functions 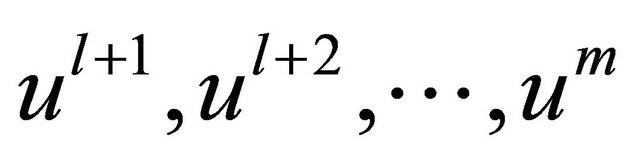 are called superfluous. The rank and the defect of the
are called superfluous. The rank and the defect of the 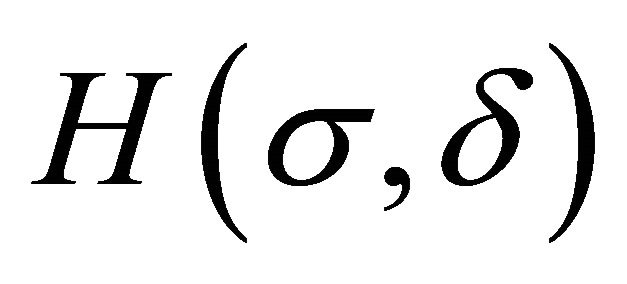 -solution are
-solution are  and
and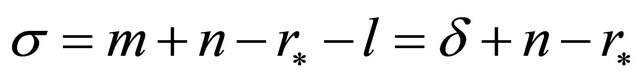 , respectively.
, respectively.
Note that if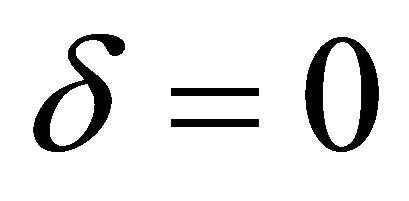 , the above algorithm is the algorithm for finding a representation of an invariant solution. If
, the above algorithm is the algorithm for finding a representation of an invariant solution. If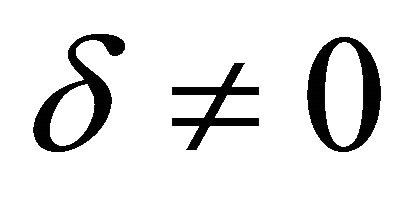 , then Equation (3) do not define all dependent functions. Since a partially invariant solution satisfies the restrictions (2), this algorithm cuts out some particular solutions from the set of all solutions.
, then Equation (3) do not define all dependent functions. Since a partially invariant solution satisfies the restrictions (2), this algorithm cuts out some particular solutions from the set of all solutions.
After constructing the representation of an invariant or partially invariant solution (3), it has to be substituted into the original system of equations. The system of equations obtained for the functions  and superfluous functions
and superfluous functions 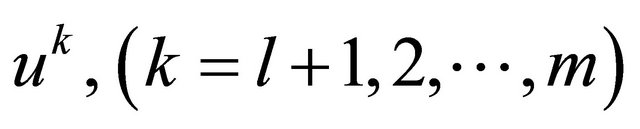 is called the reduced system. This system is overdetermined and requires an analysis of compatibility. Compatibility analysis for invariant solutions is easier than for partially invariant solutions. Another case of partially invariant solutions which is easier than the general case occurs when
is called the reduced system. This system is overdetermined and requires an analysis of compatibility. Compatibility analysis for invariant solutions is easier than for partially invariant solutions. Another case of partially invariant solutions which is easier than the general case occurs when 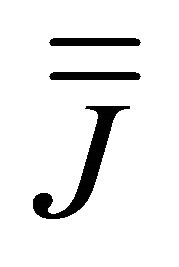 only depends on the independent variables
only depends on the independent variables
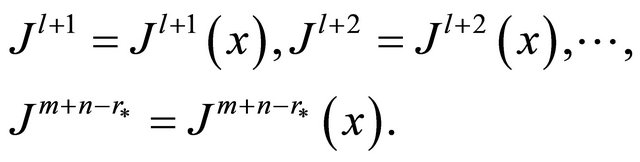
In this case, a partially invariant solution is called regular, otherwise it is irregular. The number 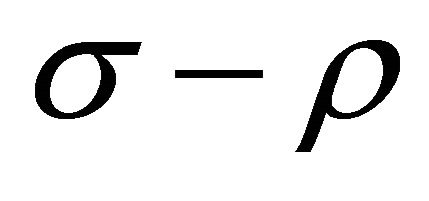 is called the measure of irregularity.
is called the measure of irregularity.
The process of studying compatibility consists of reducing the overdetermined system of partial differential equations to an involutive system. During this process different subclasses of 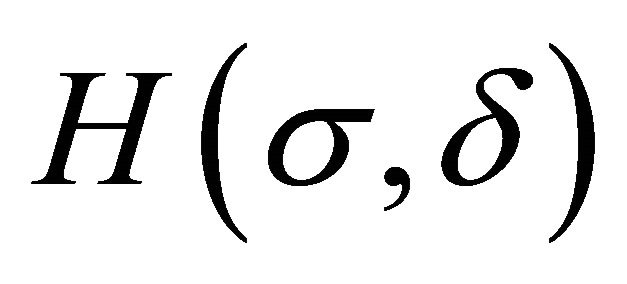 partially invariant solutions can be obtained. Some of these subclasses can be
partially invariant solutions can be obtained. Some of these subclasses can be 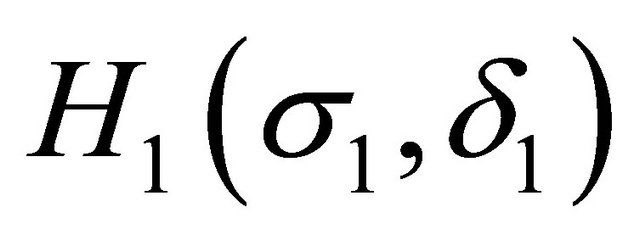 -solutions with subalgebra
-solutions with subalgebra . In this case
. In this case . The study of compatibility of partially invariant solutions with the same rank
. The study of compatibility of partially invariant solutions with the same rank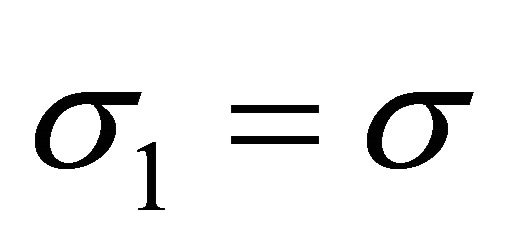 , but with smaller defect
, but with smaller defect  is simpler than the study of compatibility for
is simpler than the study of compatibility for 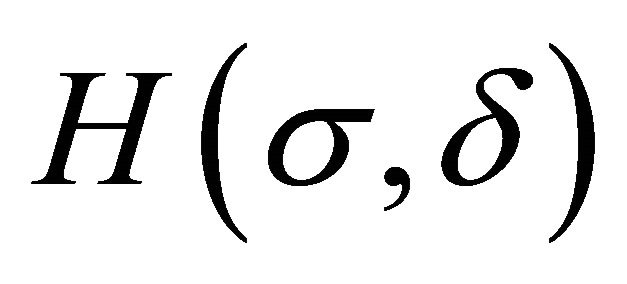 -solutions. In many applications, there is a reduction of a
-solutions. In many applications, there is a reduction of a 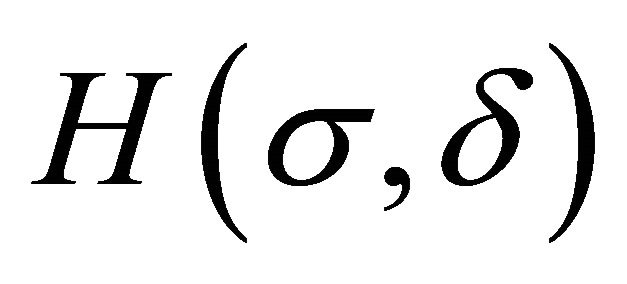 -solution to a
-solution to a  solution. In this case the
solution. In this case the  -solution is called reducible to an invariant solution. The problem of reduction to an invariant solution is important since invariant solutions are usually studied first.
-solution is called reducible to an invariant solution. The problem of reduction to an invariant solution is important since invariant solutions are usually studied first.
3. The Unsteady Navier-Stokes Equations
Unsteady motion of incompressible viscous fluid is governed by the Navier-Stokes equations
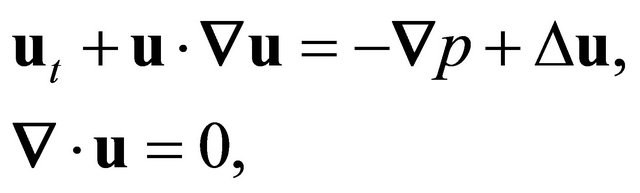 (4)
(4)
where 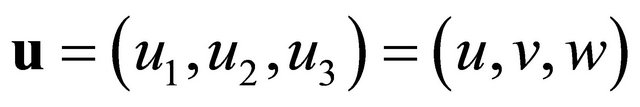 is the velocity field,
is the velocity field, ![]() is the fluid pressure,
is the fluid pressure,  is the gradient operator in the three-dimensional space
is the gradient operator in the three-dimensional space 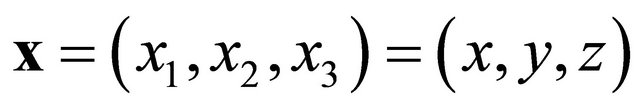 and
and ![]() is the Laplacian. A group classification of the Navier-Stokes equations in the three-dimensional case1 was done in [5]. The Lie group admitted by the NavierStokes equations is infinite. Its Lie algebra can be presented in the form of the direct sum
is the Laplacian. A group classification of the Navier-Stokes equations in the three-dimensional case1 was done in [5]. The Lie group admitted by the NavierStokes equations is infinite. Its Lie algebra can be presented in the form of the direct sum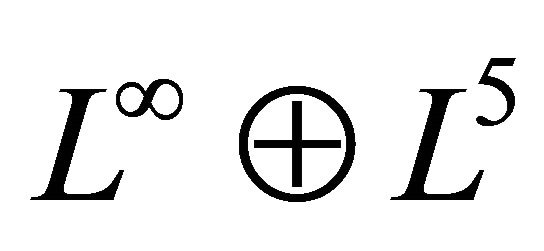 , where the infinite-dimensional ideal
, where the infinite-dimensional ideal 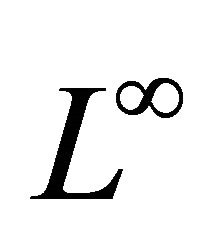 is generated by the operators2
is generated by the operators2
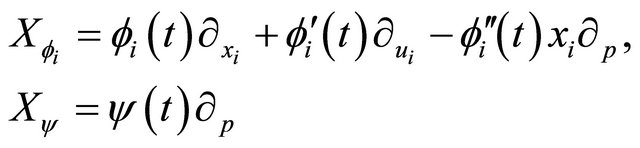
with arbitrary functions 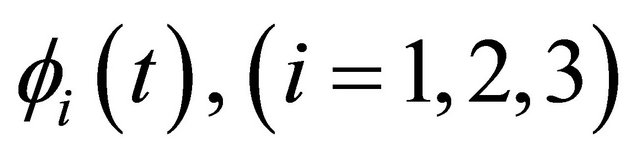 and
and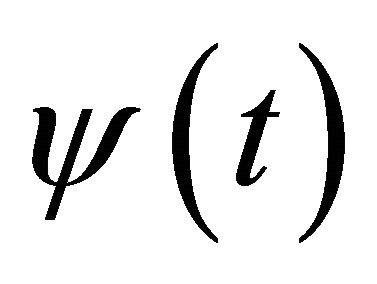 . The subalgebra
. The subalgebra 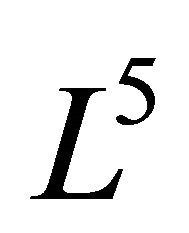 has the following basis:
has the following basis:

The Galilean algebra  is contained in
is contained in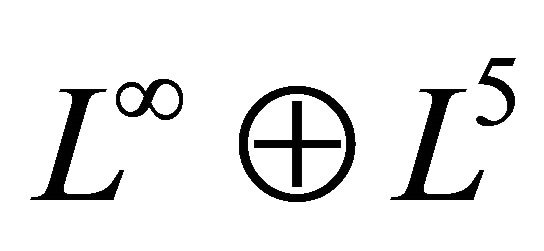 . Several articles [7-13] are devoted to invariant solutions of the Navier-Stokes equations3. While partially invariant solutions of the Navier-Stokes equations have been less studied4, there has been substantial progress in studying such classes of solutions of inviscid gas dynamics equations [18-25].
. Several articles [7-13] are devoted to invariant solutions of the Navier-Stokes equations3. While partially invariant solutions of the Navier-Stokes equations have been less studied4, there has been substantial progress in studying such classes of solutions of inviscid gas dynamics equations [18-25].
4. The Reduction of the Navier-Stokes Equations
The reduction of the Navier-Stokes equations to partial differential equation in three independent variables is described. In this section analysis of compatibility of regular partially invariant solutions with defect 1 and rank 1 of the subalgebras 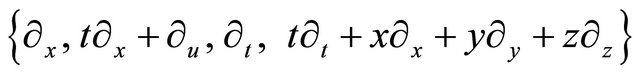 is given. Note that the generator
is given. Note that the generator 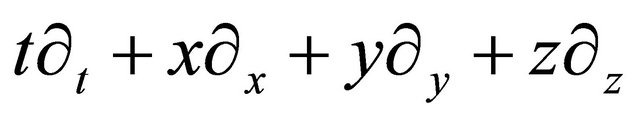 is not admitted by the Navier-Stokes equations. The groups are taken from the optimal system constructed for the gas dynamics equations [26].
is not admitted by the Navier-Stokes equations. The groups are taken from the optimal system constructed for the gas dynamics equations [26].
The Navier-Stokes equations are used in the component form:
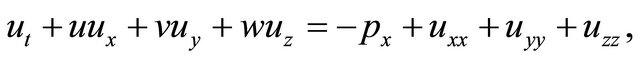 (5)
(5)
 (6)
(6)
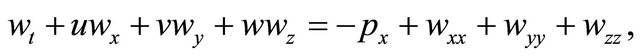 (7)
(7)
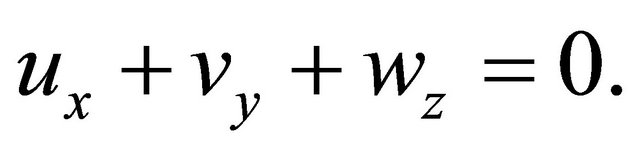 (8)
(8)
The dependent variables 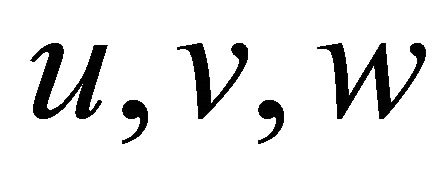 and
and ![]() are functions of the space variables
are functions of the space variables 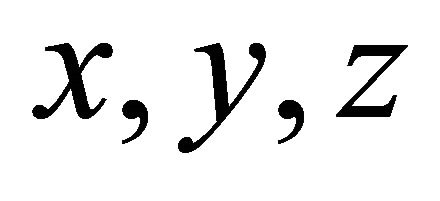 and time
and time 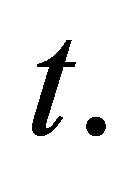
Invariants of the Lie group corresponding to subalgebra generated by  are
are
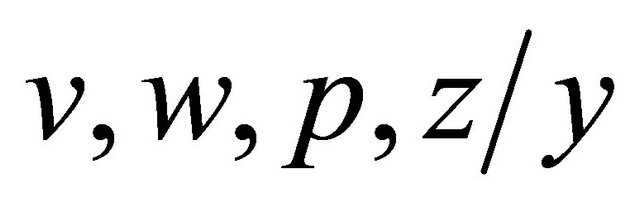
The representation of the regular partially invariant solution is
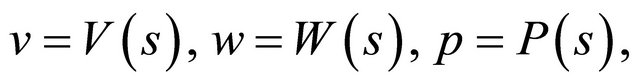 (9)
(9)
where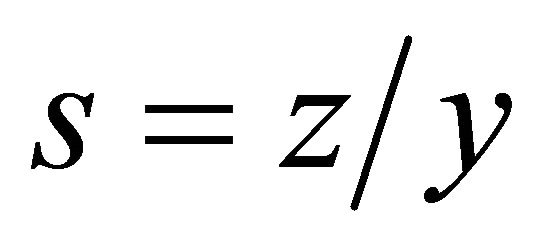 . For the function
. For the function 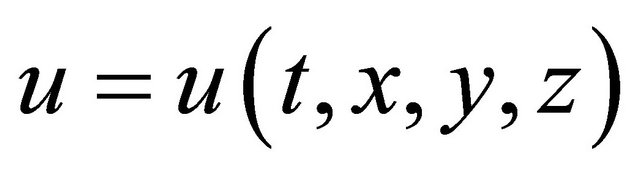 there is no restrictions. Substituting the representation of partially invariant solution (9) into the Navier-Stokes Equations (5)-(8), we obtain
there is no restrictions. Substituting the representation of partially invariant solution (9) into the Navier-Stokes Equations (5)-(8), we obtain
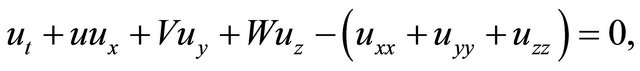 (10)
(10)
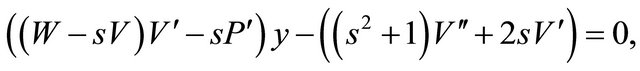 (11)
(11)
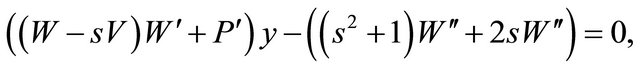 (12)
(12)
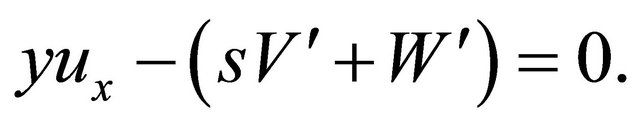 (13)
(13)
Since 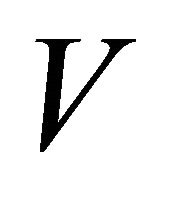 and
and  only depend on
only depend on![]() , Equations (11) and (12) can be split with respect to
, Equations (11) and (12) can be split with respect to![]() :
:
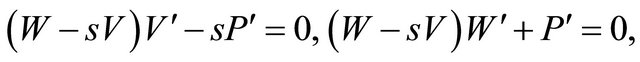 (14)
(14)
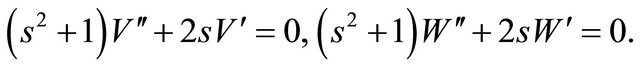 (15)
(15)
Solving Equation (15), we have
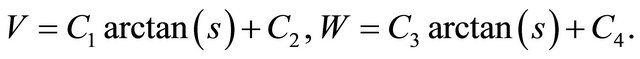
Multiplying the first equation by ![]() and combining it with the second equation of (14), we obtain
and combining it with the second equation of (14), we obtain
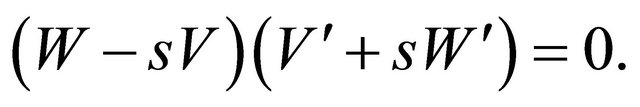
Let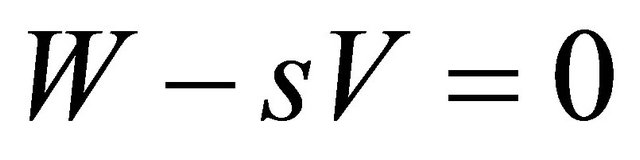 , then
, then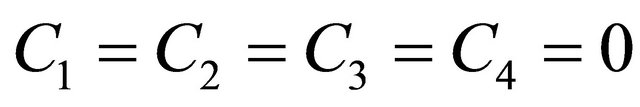 . This means that
. This means that 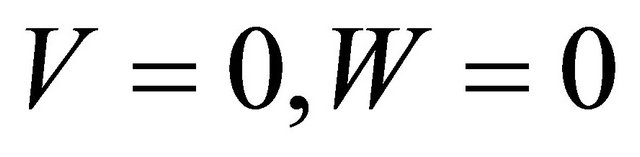 and hence
and hence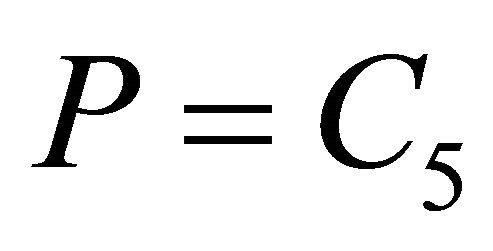 . Substituting
. Substituting 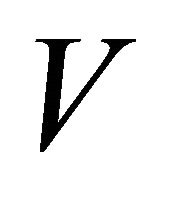 and
and 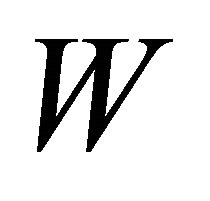 in Equation (13), we have
in Equation (13), we have . It means that
. It means that ![]() depend on
depend on 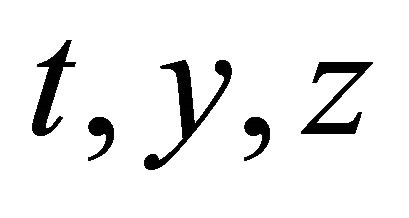 or
or . Equation (10) becomes
. Equation (10) becomes
 (16)
(16)
Thus, there is a solution of the Navier-Stokes equations of the type
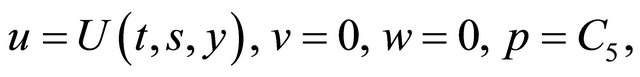
where the function 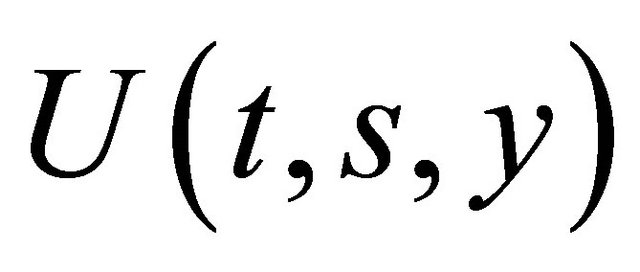 satisfies Equation (16).
satisfies Equation (16).
If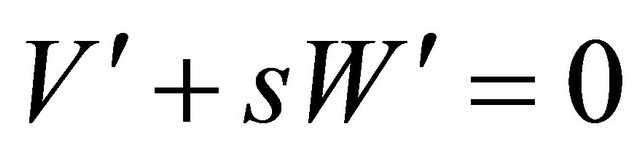 , then
, then 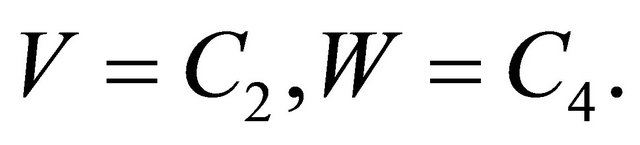 In this case
In this case . Note that the Galilei transformation applied to
. Note that the Galilei transformation applied to 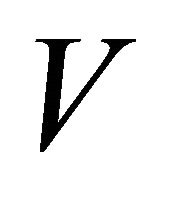 and
and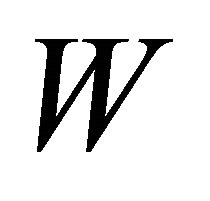 , also change
, also change![]() . Substituting
. Substituting  and
and 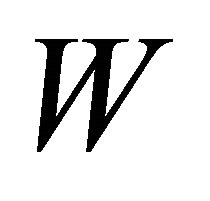 in Equation (13), we have
in Equation (13), we have 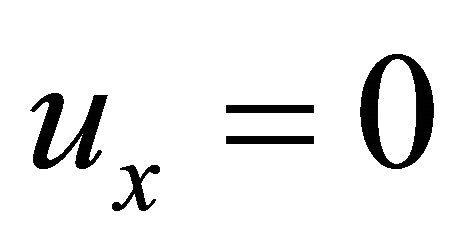 or
or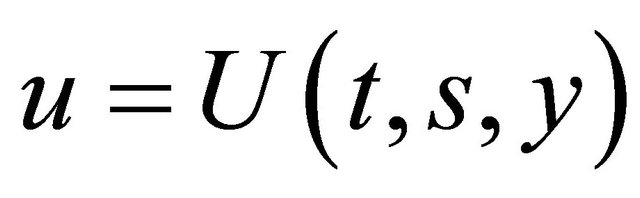 . Equation (10) becomes
. Equation (10) becomes
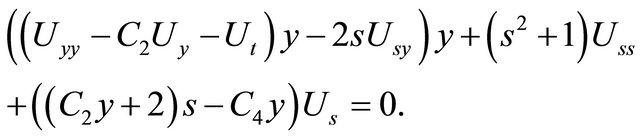 (17)
(17)
Thus, there is a solution of the Navier-Stokes equations of the type
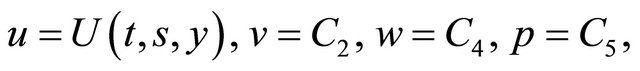
where the function  satisfies Equation (17).
satisfies Equation (17).
These solutions are partially invariant solution with respect to the group which are not admitted Lie algebra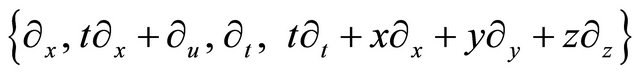 .
.
5. Admitted Group of Equation (16)
In this section, the Lie group admitted by Equation (16) is studied. It was obtained from the Navier-Stokes equations and gives rise to a partially invariant solutions of the Navier-Stokes equations
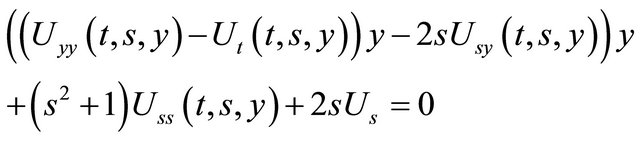
where the function U depends on 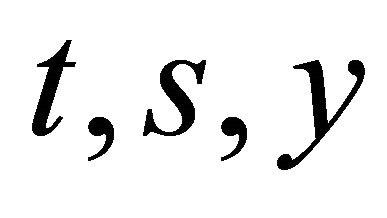 and
and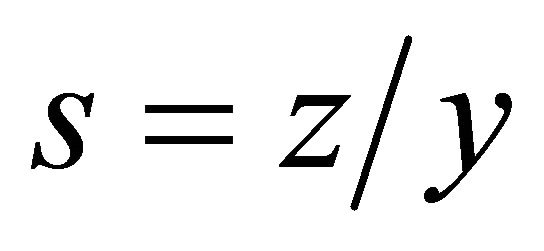 .
.
Assume that the generator has a representation of the form
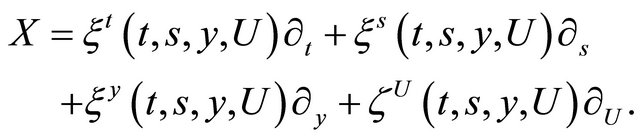
The second prolongation of the operator  is
is
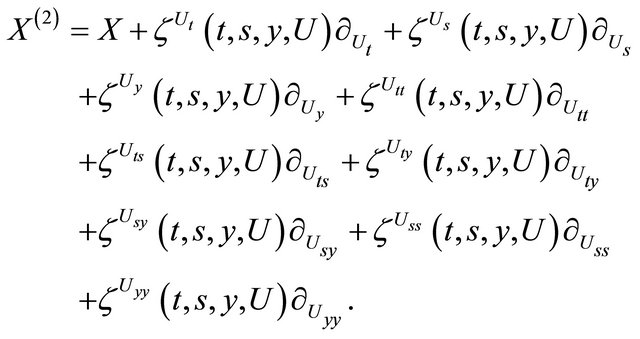
The coefficients of the prolonged operator are defined by formulae

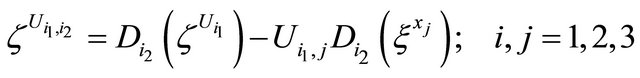
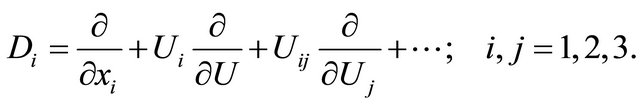
Here we used the notations 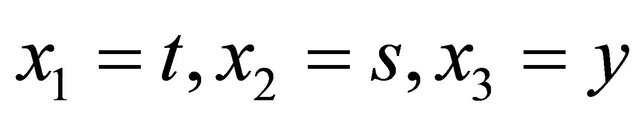 and for the derivatives
and for the derivatives

The determining equations are
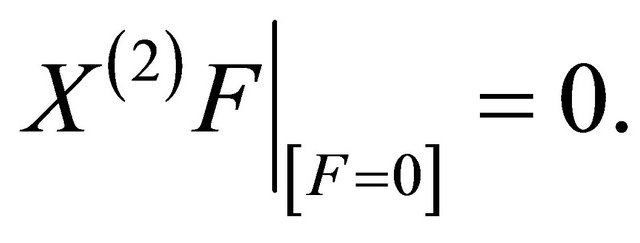 (18)
(18)
All necessary calculations here were carried out on a computer using the symbolic manipulation program REDUCE.
The result of the calculations is the admitted Lie group with the basis of the generators:
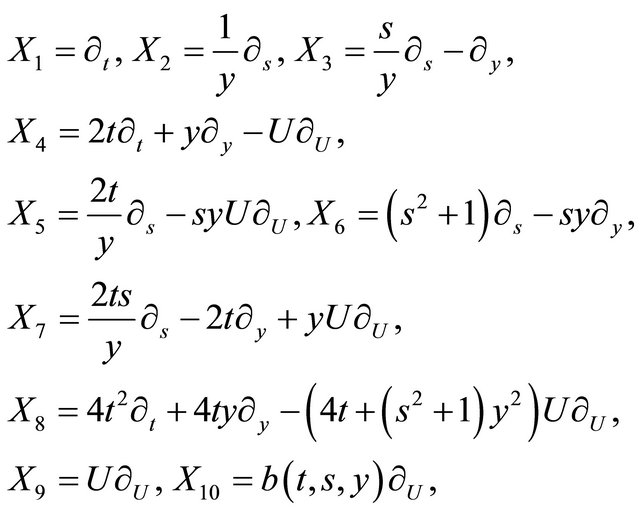 (19)
(19)
where 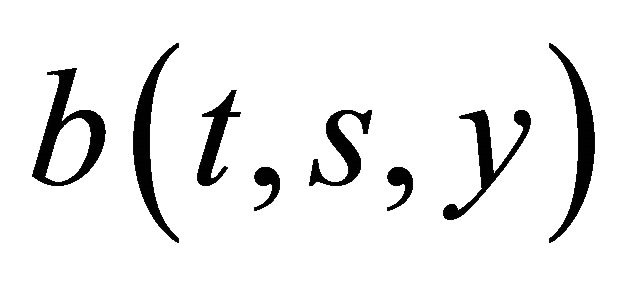 is an arbitrary solution of
is an arbitrary solution of
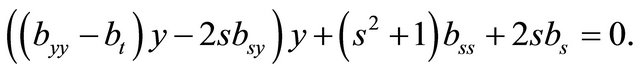
6. Optimal System of Subalgebras
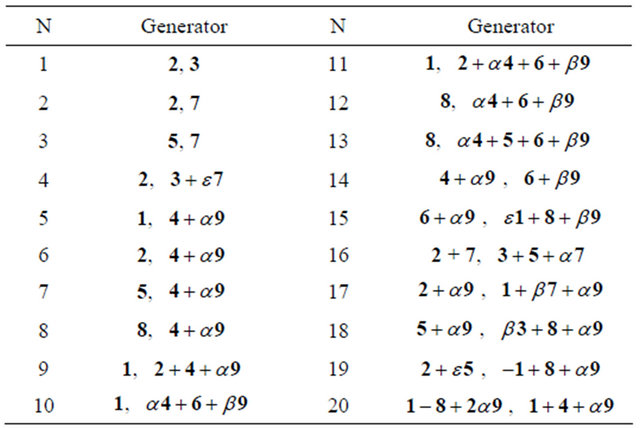
The problem is to construct subalgebras of the algebra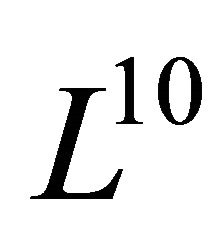 , which can be a source of invariant solutions of Equation (1). The classification of subalgebras can be done relatively easy for small dimensions. The optimal system of subalgebras of the Lie algebra spanned by the generators
, which can be a source of invariant solutions of Equation (1). The classification of subalgebras can be done relatively easy for small dimensions. The optimal system of subalgebras of the Lie algebra spanned by the generators  are constructed here.
are constructed here.
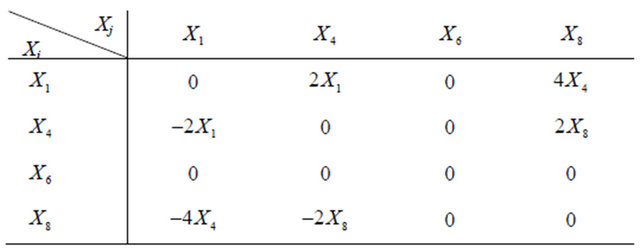
The table of commutators 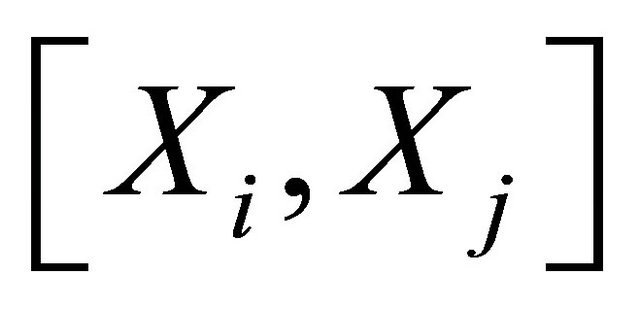 is
is
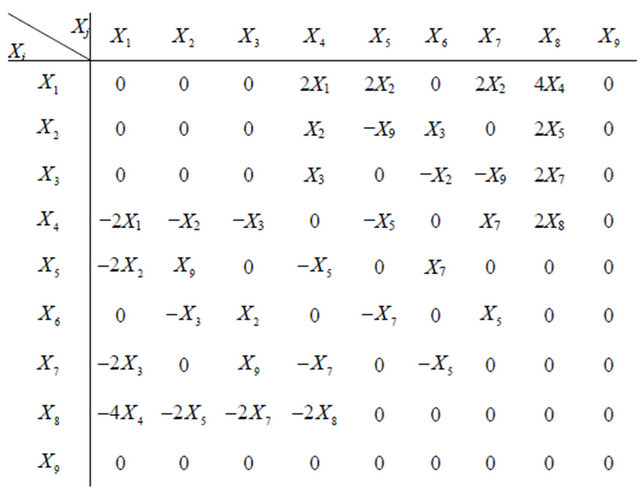
Inner automorphisms [24] are constructed with the help of the table of commutators.
To construct inner automorphisms, one has to solve the Lie equations. For example, for the automorphism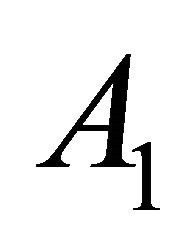 , one has the system of ordinary differential equations
, one has the system of ordinary differential equations
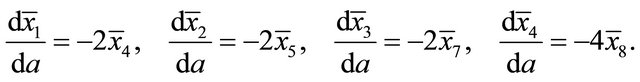
and the initial values at 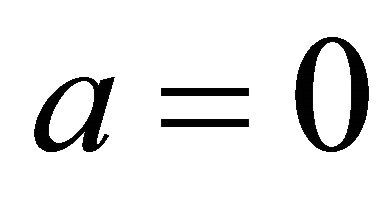
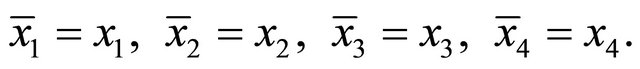
Therefore, the automorphism 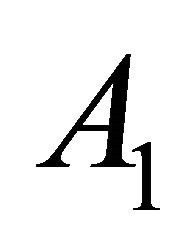 only changes the coordinates
only changes the coordinates 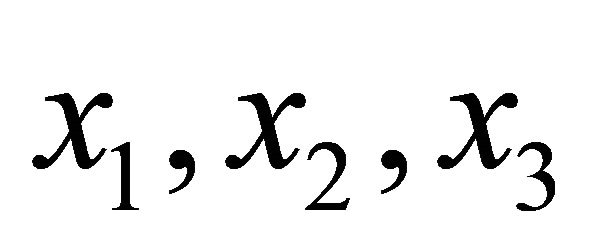 and
and 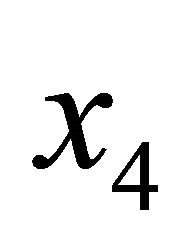 by the formulae
by the formulae
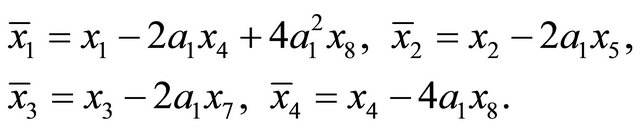
The remaining coordinates are unchanged.
In the same way, one obtains the automorphisms 
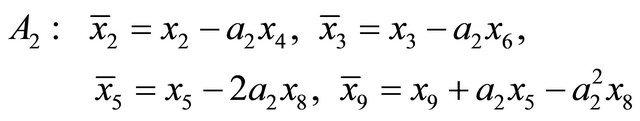
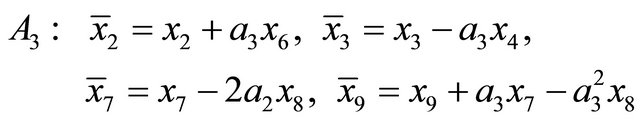

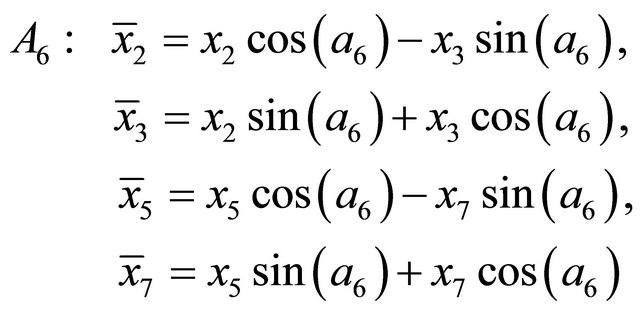
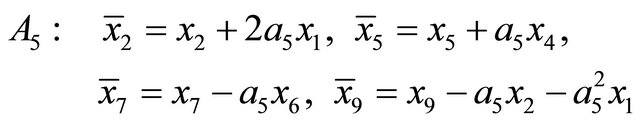
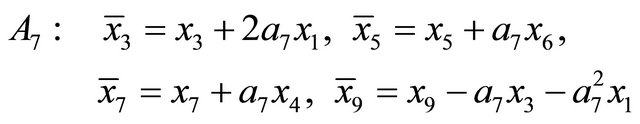
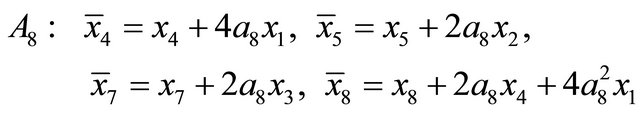

Also there is the involution
6.1. Decomposition of the Algebra 
Before constructing an optimal system, let us study the algebraic structure of the algebra . The algebra
. The algebra 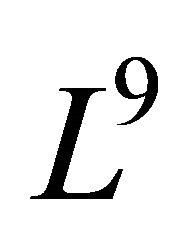 is decomposed as
is decomposed as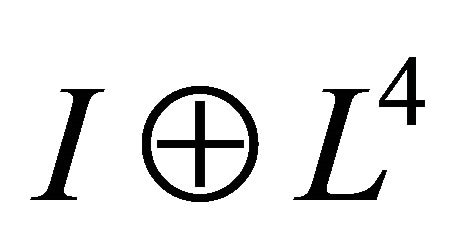 , where
, where  is an ideal and
is an ideal and 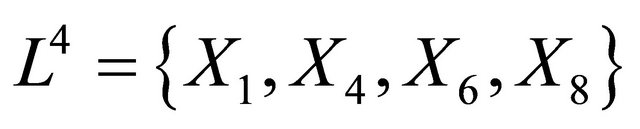 is a subalgebra. According to the algorithm for constructing an optimal system of the algebra
is a subalgebra. According to the algorithm for constructing an optimal system of the algebra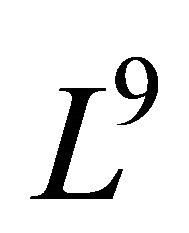 , we use the two-step algorithm developed in [21]. First, an optimal system of subalgebras of the algebra
, we use the two-step algorithm developed in [21]. First, an optimal system of subalgebras of the algebra  is obtained. The next step is to glue the subalgebras from the optimal system of subalgebras of the algebra
is obtained. The next step is to glue the subalgebras from the optimal system of subalgebras of the algebra 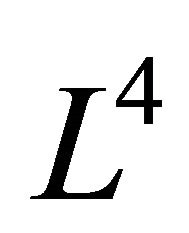 and the ideal
and the ideal  together.
together.
Any subalgebra of a Lie algebra is completely defined by its basis generators. Any vector of the basis is a linear combination of the basis of generator of this Lie algebra. Hence, the subalgebra is completely defined by coefficients of these linear combinations. For example, let 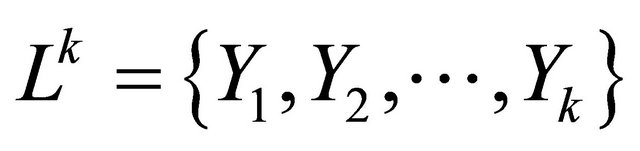 be a
be a 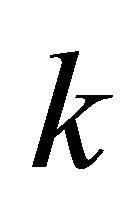 -dimensional subalgebra of the algebra
-dimensional subalgebra of the algebra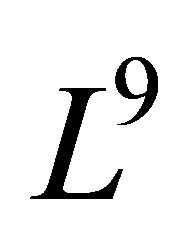 . Operators
. Operators 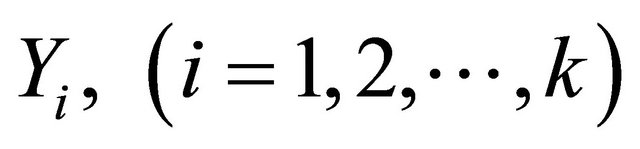 are
are
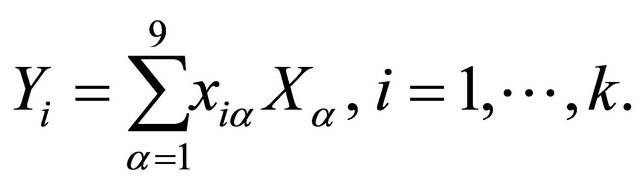
Conditions for 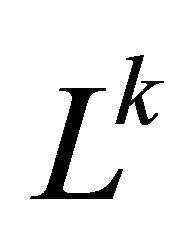 to be a subalgebra are
to be a subalgebra are
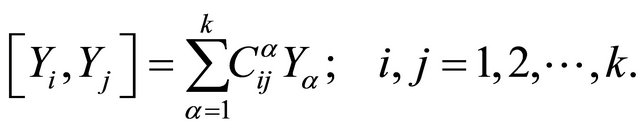
For a classification of subalgebra, the coefficients 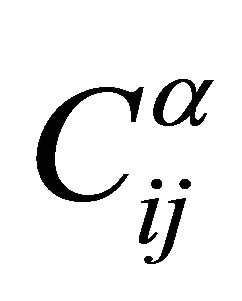 have to be simplified by using the automorphism and subalgebra conditions.
have to be simplified by using the automorphism and subalgebra conditions.
6.2. Classification of the Algebra 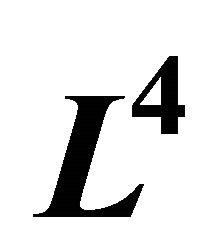
Let us classify the algebra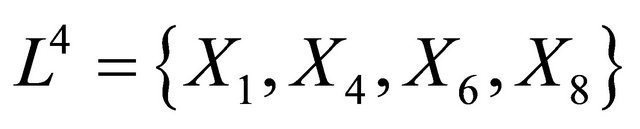 . The table of commutators of the algebra
. The table of commutators of the algebra 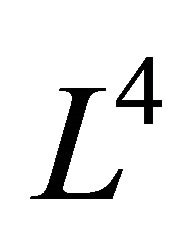 is
is
Since the generator 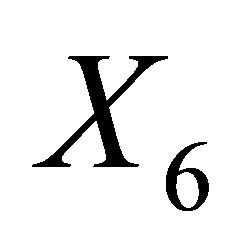 composes the center, the optimal system of subalgebras of
composes the center, the optimal system of subalgebras of  can be easily constructed by classifying the subalgebra
can be easily constructed by classifying the subalgebra 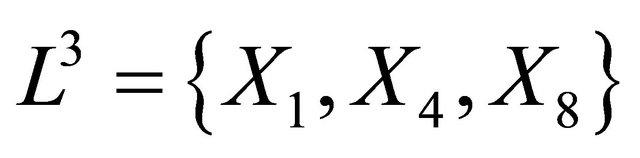 and gluing it with the center
and gluing it with the center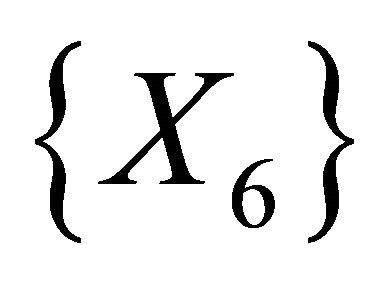 . The idea of construction is as follows.
. The idea of construction is as follows.
Let a subalgebra 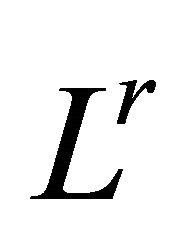 of dimension
of dimension 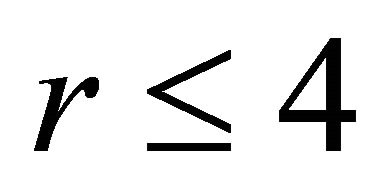 be formed by the operators
be formed by the operators
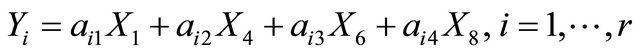
where  are arbitrary constants.
are arbitrary constants.
For the classification of 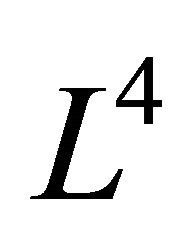 we need to study two steps.
we need to study two steps.
1) All coefficients  are zero,
are zero, 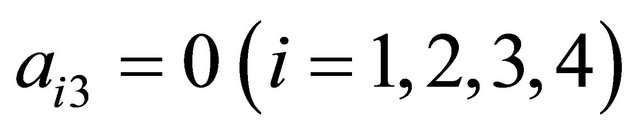 , it means that we will construct an optimal system of the subalgebra
, it means that we will construct an optimal system of the subalgebra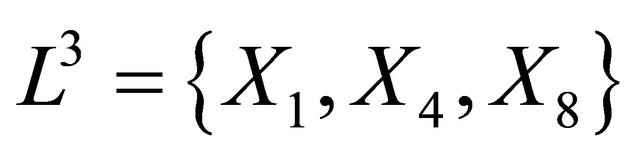 .
.
2) At least one of the coefficients of  is not equal to zero.
is not equal to zero.
Let us study the first step, and construct an optimal system of the subalgebra . For convenience, we will denote the generators
. For convenience, we will denote the generators 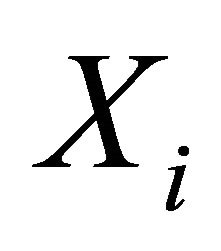 by i.
by i.
6.2.1. One-Dimensional Subalgebras of the Algebra 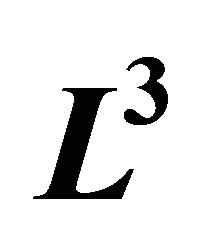
Let  which forms a one-dimensional subalgebra of the algebra
which forms a one-dimensional subalgebra of the algebra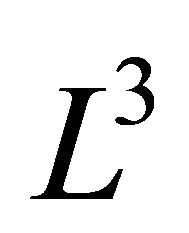 . The process of simplification of the coefficients of the operator
. The process of simplification of the coefficients of the operator ![]() is separated into the following cases.
is separated into the following cases.
Case 1. Assume that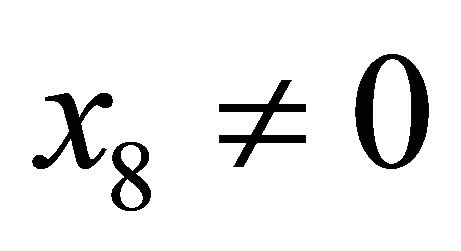 . Then one can divide
. Then one can divide ![]() by
by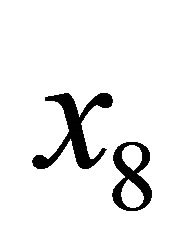 . Hence, without loss of generality one can consider
. Hence, without loss of generality one can consider
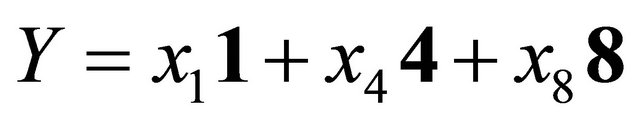
By means of transformation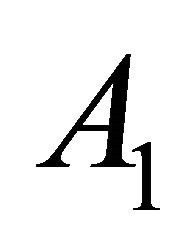 , it can transformed to an operator with
, it can transformed to an operator with .
.
Case 1.1. Let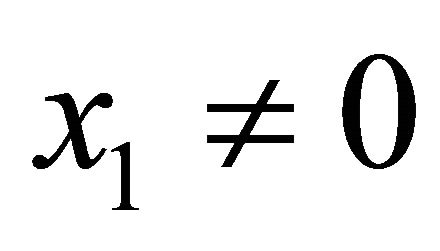 . By means of transformation
. By means of transformation , one can transform it to
, one can transform it to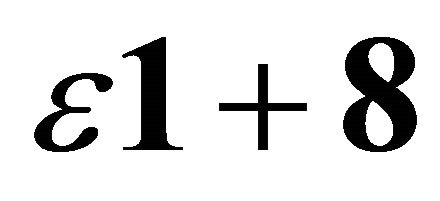 , where
, where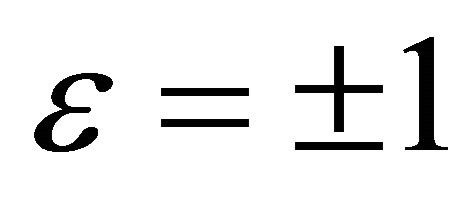 .
.
Case 1.2. Let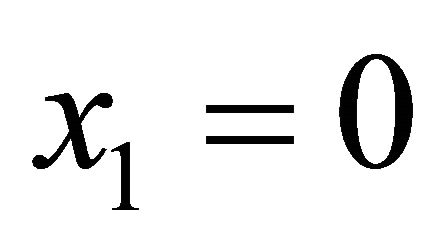 , then the representative of the class is the operator
, then the representative of the class is the operator .
.
Case 2. Assume that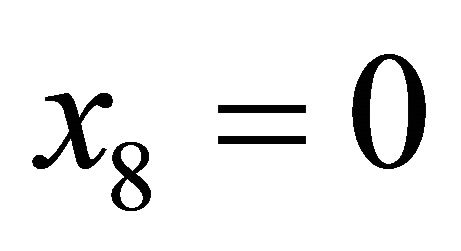 . Then one has
. Then one has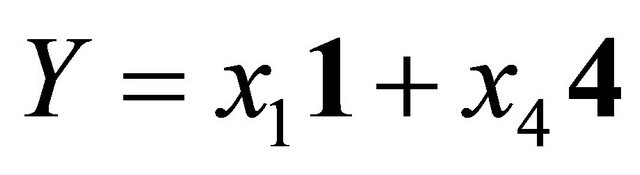 .
.
Case 2.1. Let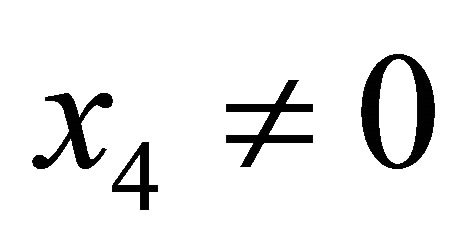 . Dividing the operator
. Dividing the operator ![]() by
by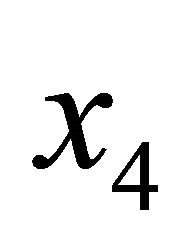 , one obtains
, one obtains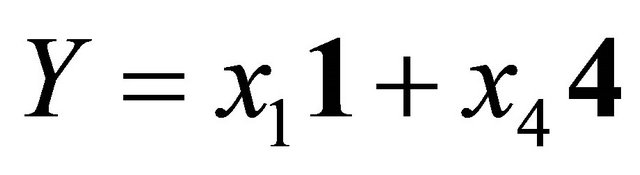 . By using the automorphism
. By using the automorphism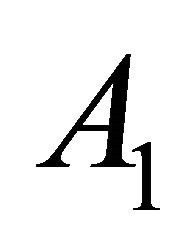 , the operator
, the operator ![]() is transformed to
is transformed to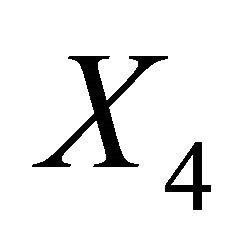 .
.
Case 2.2. Let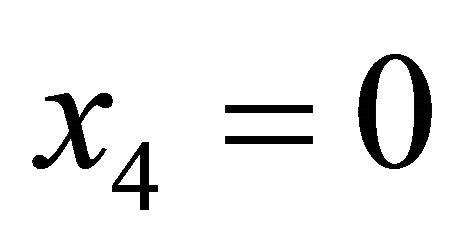 , then
, then .
.
6.2.2. Two-Dimensional Subalgebras of the Algebra 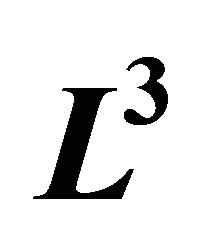
Let a subalgebra be formed by the operators
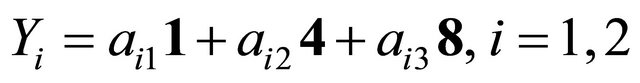
where 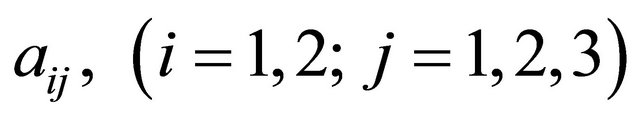 are arbitrary constants.
are arbitrary constants.
Note that the rank of the matrix  is equal to two.
is equal to two.
Case 1. Assume that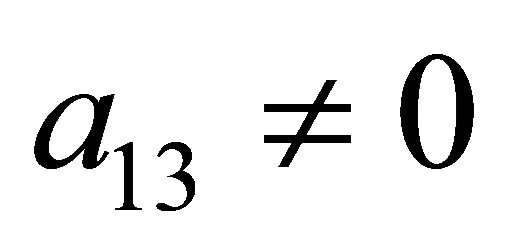 . We can divide
. We can divide ![]() by
by . Hence, by subtracting the operator
. Hence, by subtracting the operator 
from , one can assume
, one can assume 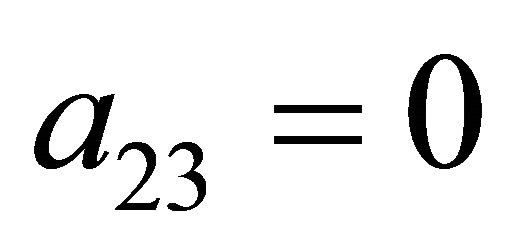 and
and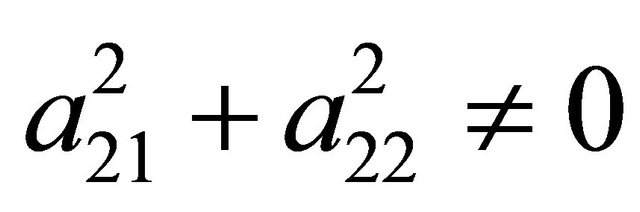 .
.
Using the automorphisms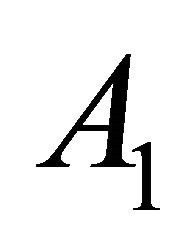 , the operator
, the operator ![]() is transformed to
is transformed to . The subalgebra condition gives
. The subalgebra condition gives
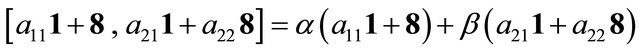
where ![]() and
and 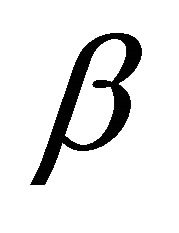 are arbitrary constants. Calculating the left hand side and comparing the coefficients on the left hand side with coefficients on the right hand side, one has
are arbitrary constants. Calculating the left hand side and comparing the coefficients on the left hand side with coefficients on the right hand side, one has
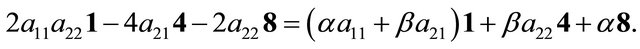
Therefore
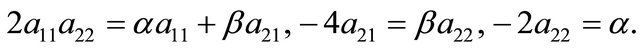
Further consideration depends on values of the coefficients . If
. If , then
, then 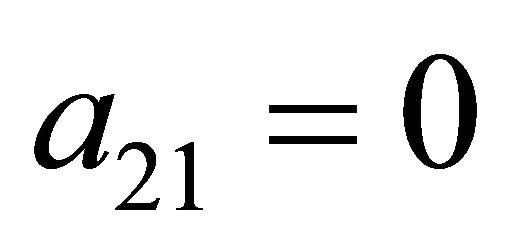 which is a contradiction to the condition
which is a contradiction to the condition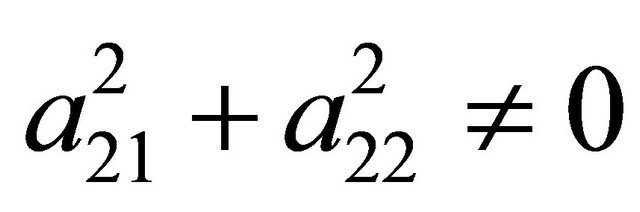 . Hence,
. Hence,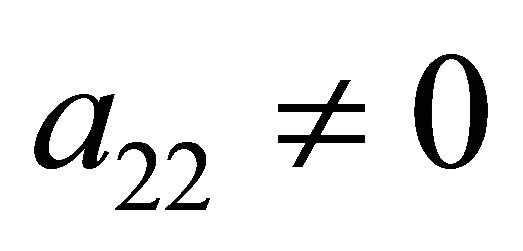 . One can assume that
. One can assume that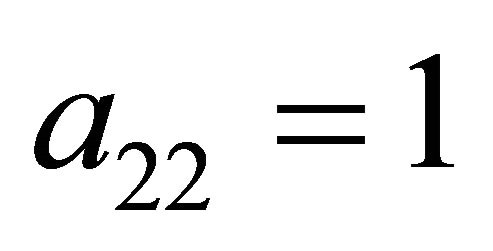 . Therefore
. Therefore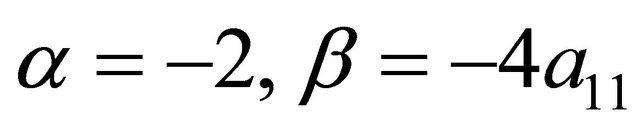 , and
, and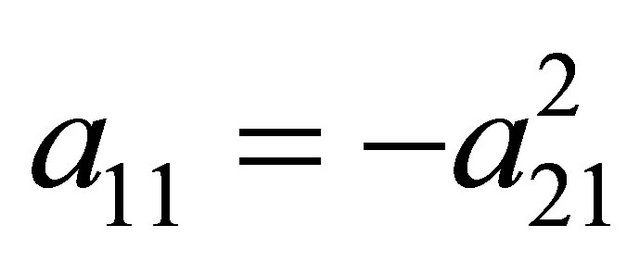 .
.
Case 1.1. If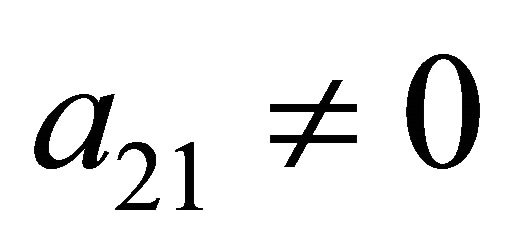 , then using the automorphism
, then using the automorphism , the operators
, the operators ![]() and
and 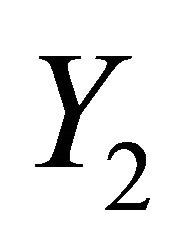 are transformed to
are transformed to ,
, .
.
Case 1.2. If , then the operators
, then the operators ![]() and
and 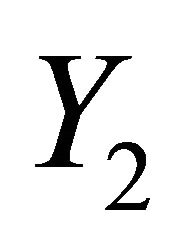 are
are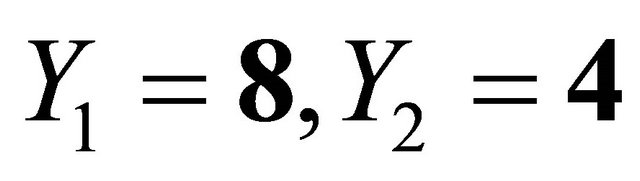 .
.
Case 2. Assume that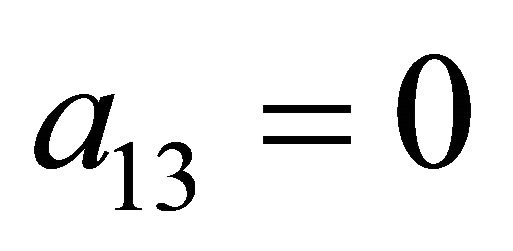 . If
. If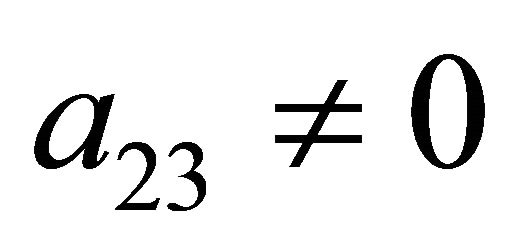 , then by exchanging
, then by exchanging ![]() and
and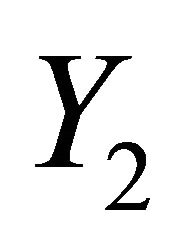 , this becomes the previous case. Hence, one can take
, this becomes the previous case. Hence, one can take . Therefore, the operators are
. Therefore, the operators are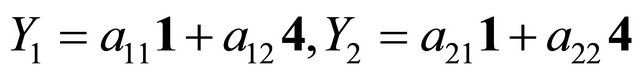 . Because the rank of the matrix
. Because the rank of the matrix

is equal to 2, then by taking linear combinations of the operators ![]() and
and 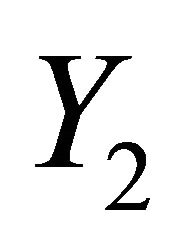 they can be transformed to
they can be transformed to 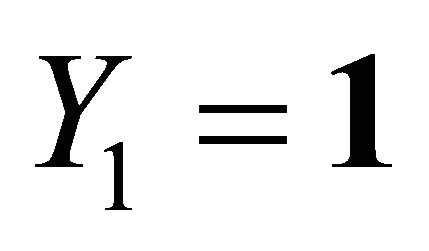 and
and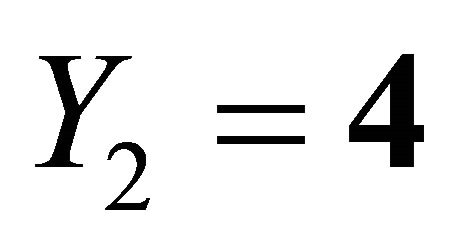 .
.
6.2.3. Three-Dimensional Subalgebras of the Algebra 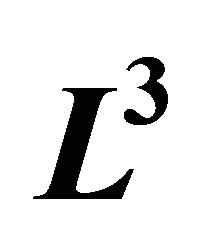
Let a subalgebra be formed by these operators

where 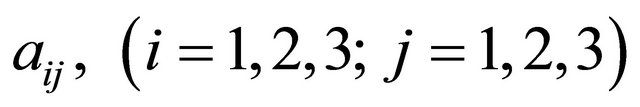 are arbitrary constants. Since the rank of the matrix
are arbitrary constants. Since the rank of the matrix
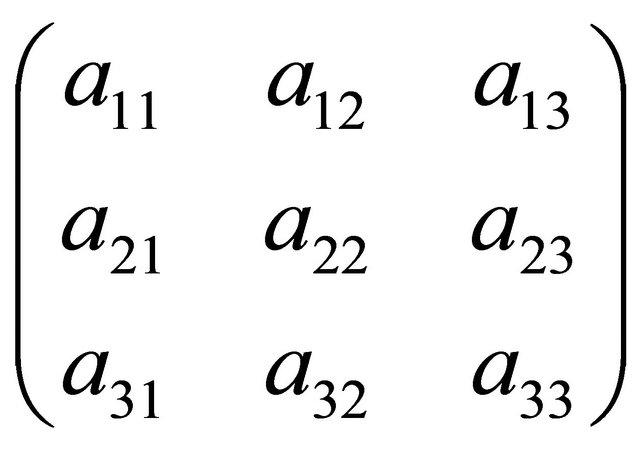
is equal to three, the basis if this subalgebra can be taken as
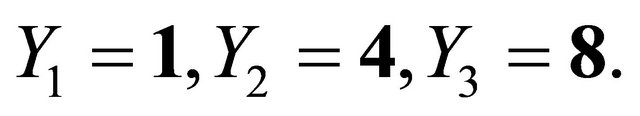
6.2.4. Optimal System of Subalgebras of the Algebra 
The result of classifying the algebra  is the following:
is the following:
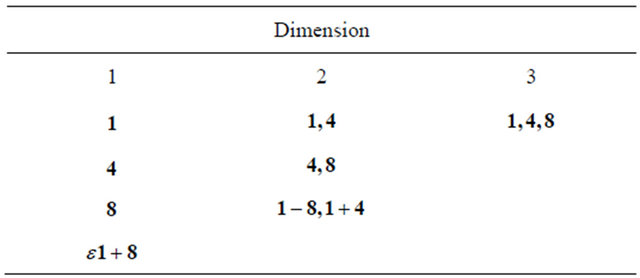
where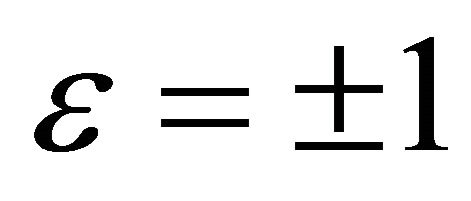 .
.
6.3. Optimal System of Subalgebras of the Algebra 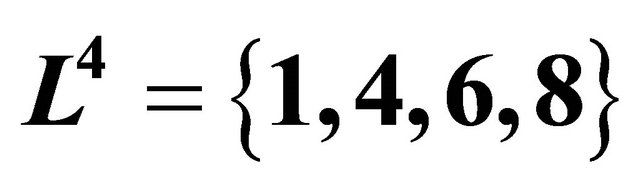
Let us consider the second step where at least one of the coefficients  is not equal to zero. Without loss of generality one can assume that
is not equal to zero. Without loss of generality one can assume that
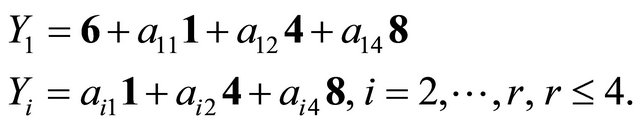
Using the conditions for 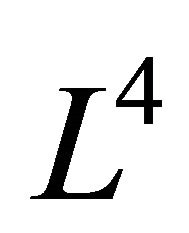 to be a subalgebra, one obtains
to be a subalgebra, one obtains
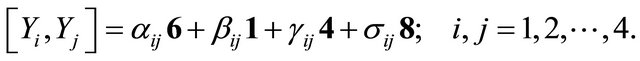
Because 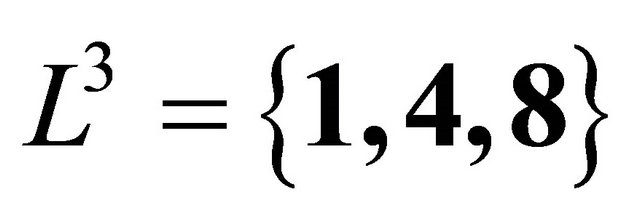 is a subalgebra and the generator 6 forms the center, then
is a subalgebra and the generator 6 forms the center, then
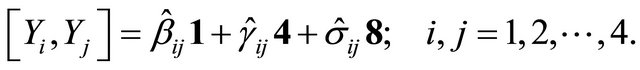
Comparing the coefficients, one obtains 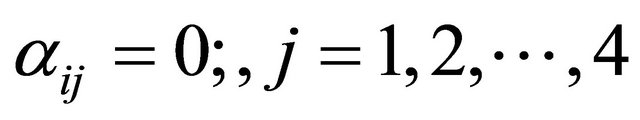 . Because of these results and since the algebra
. Because of these results and since the algebra  has already been classified, therefore this allows simplifying the process of constructing the optimal system of the algebra
has already been classified, therefore this allows simplifying the process of constructing the optimal system of the algebra . This process construct by using the result of the optimal system of algebra
. This process construct by using the result of the optimal system of algebra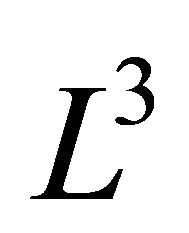 : we have to classify each optimal system of subalgebras of
: we have to classify each optimal system of subalgebras of 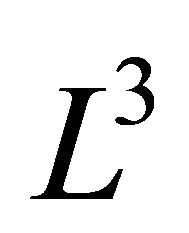 together with the generator
together with the generator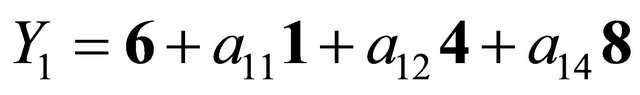 . Here we give one example of this process. Other elements of the optimal system of the algebra
. Here we give one example of this process. Other elements of the optimal system of the algebra 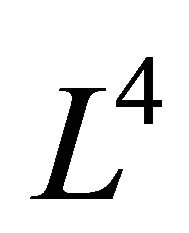 are constructed in the similar way.
are constructed in the similar way.
Let us consider the subalgebra . For constructing three-dimensional subalgebras of the algebra
. For constructing three-dimensional subalgebras of the algebra 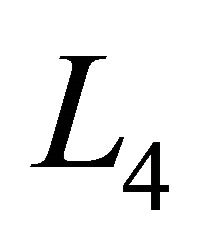 one considers
one considers
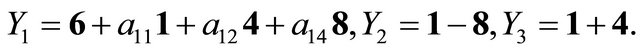
Since ![]() can be written as:
can be written as:

by forming a linear combination with 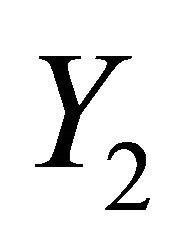 and
and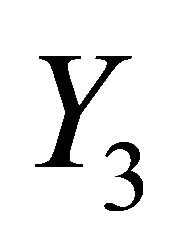 , the operator
, the operator ![]() can be taken in the form
can be taken in the form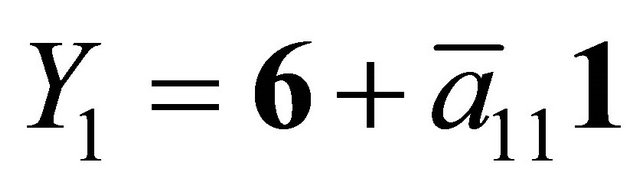 . The subalgebra conditions gives
. The subalgebra conditions gives
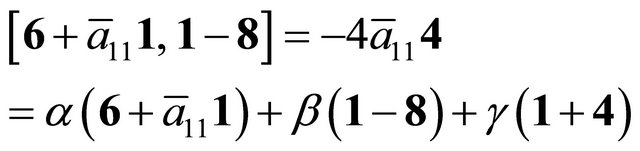
where  and
and 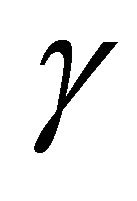 are arbitrary constants. Comparing the coefficients on the left side with the coefficients on the right side, one obtains
are arbitrary constants. Comparing the coefficients on the left side with the coefficients on the right side, one obtains
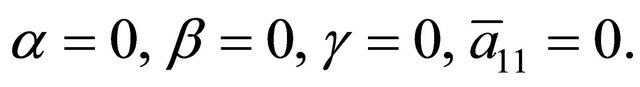
Thus, one obtains that , and the subalgebra is
, and the subalgebra is .
.
The result of calculation is an optimal system of subalgebras of the algebra 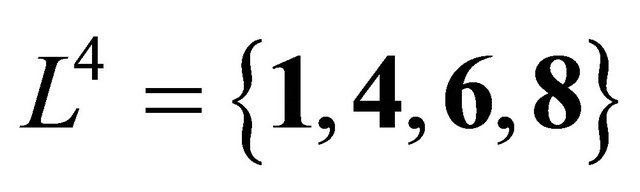 which is
which is

where 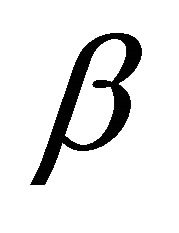 is an arbitrary real parameter and
is an arbitrary real parameter and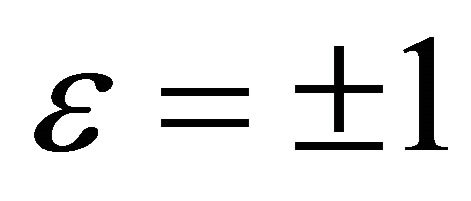 .
.
6.4. Optimal System of Subalgebras of the Algebra 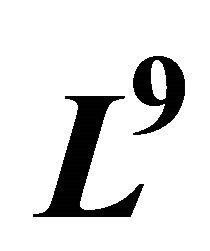
After constructing an optimal system of subalgebras of the algebra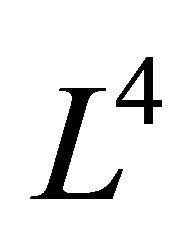 , the next step is the construction of an optimal system of subalgebras of the algebra
, the next step is the construction of an optimal system of subalgebras of the algebra  , by gluing subalgebras from the optimal system of subalgebras of the algebra
, by gluing subalgebras from the optimal system of subalgebras of the algebra 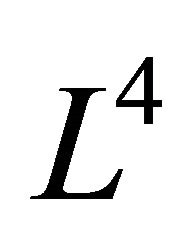 and the ideal
and the ideal  together.
together.
As it was seen for the algebra , the process of constructing an optimal system of subalgebras of the algebra
, the process of constructing an optimal system of subalgebras of the algebra 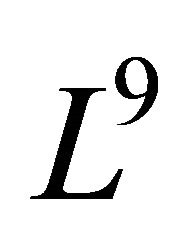 by gluing the algebra
by gluing the algebra 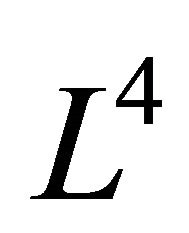 and the ideal
and the ideal  consists of the following steps. In the first step, the vectors
consists of the following steps. In the first step, the vectors
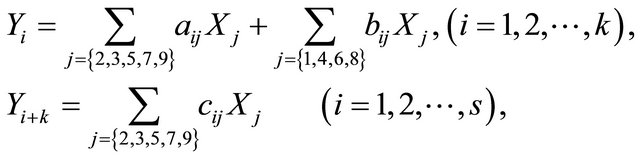
are composed. Here the vectors
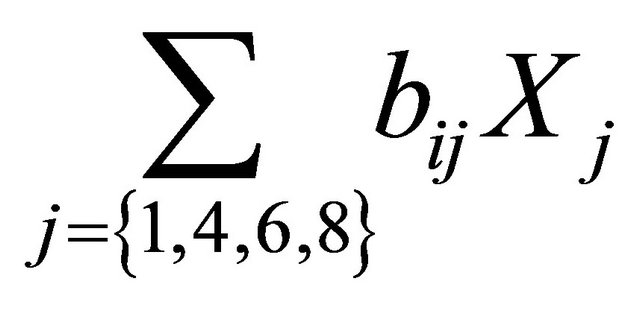
are basis elements from one of the k-dimensional subalgebras 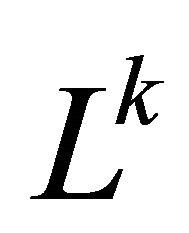 of the optimal system of the algebra
of the optimal system of the algebra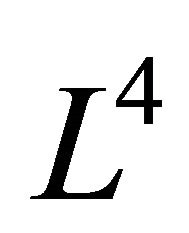 . In matrix form, this step can be explained by the construction of the matrix
. In matrix form, this step can be explained by the construction of the matrix

where the matrices A, B and C consist of the coefficients
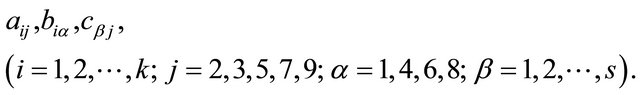
In this step, the matrix A is arbitrary. The rank of the matrix
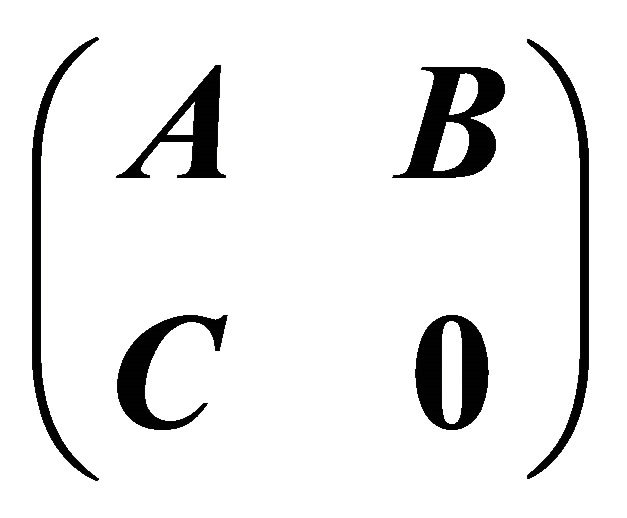
is equal to 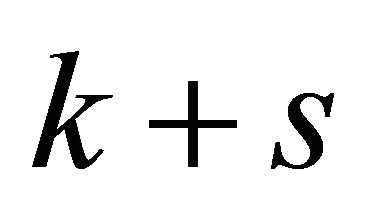 and this is the dimension of the subalgebra of the algebra
and this is the dimension of the subalgebra of the algebra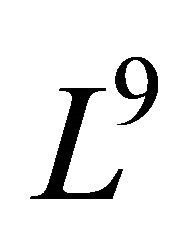 . The matrix C is chosen to be the simplest by taking linear combinations of it columns and has to take all possible values of the given rank s. Note also that the matrix A can be simplified with the help of the matrix C.
. The matrix C is chosen to be the simplest by taking linear combinations of it columns and has to take all possible values of the given rank s. Note also that the matrix A can be simplified with the help of the matrix C.
The next step is the process of checking the subalgebra conditions and checking linear dependence of commutators on the basis generators of the subalgebra.
In this manuscript, we study only two-dimensional subalgebras of the algebra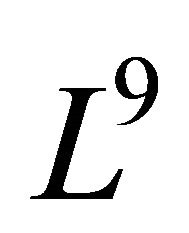 , because the two-dimensional subalgebras allow obtaining invariant solutions which reduce the initial system of partial differential equations to a system of ordinary differential equations.
, because the two-dimensional subalgebras allow obtaining invariant solutions which reduce the initial system of partial differential equations to a system of ordinary differential equations.
Let us give an example for constructing two-dimensional subalgebras, using the subalgebra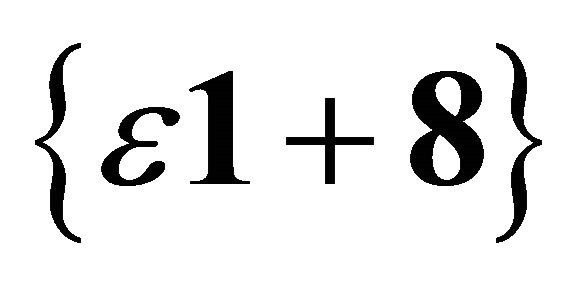 . The maximum possible dimension of a subalgebra of the algebra
. The maximum possible dimension of a subalgebra of the algebra 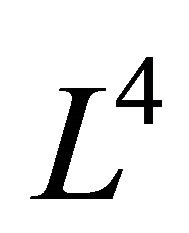 after gluing a subalgebra to the ideal
after gluing a subalgebra to the ideal  is two. In this case, the matrix C is a
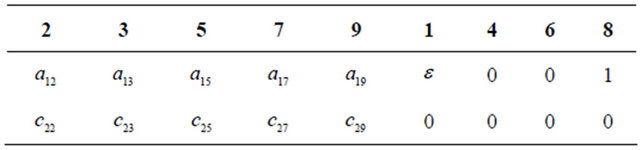
is two. In this case, the matrix C is a 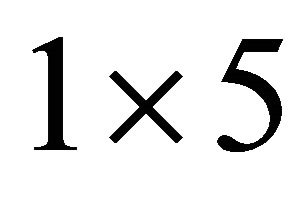 matrix, the rank of which is equal to one:
matrix, the rank of which is equal to one:
By virtue of the automorphism :
:
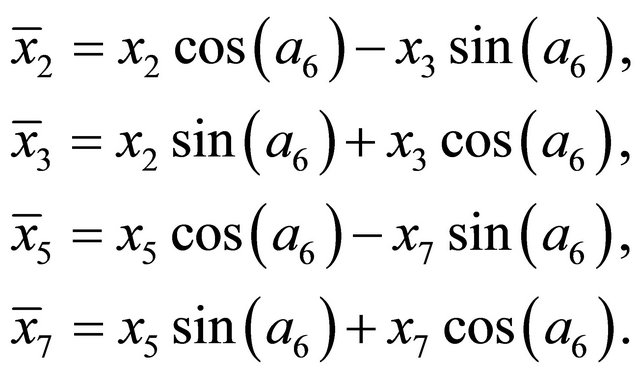
We can consider three cases:
1)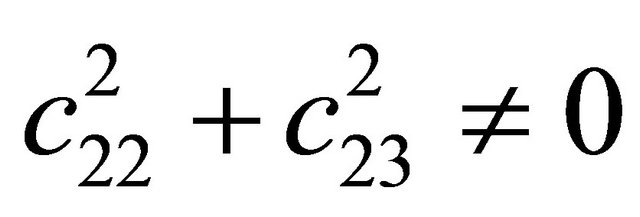 2)
2)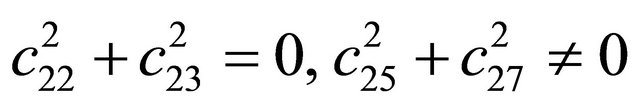 3)
3)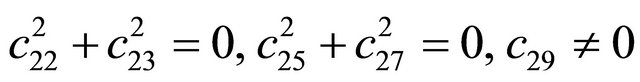 .
.
Case 1. By using the automorphism 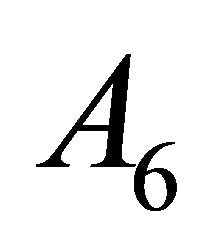 one can assume
one can assume 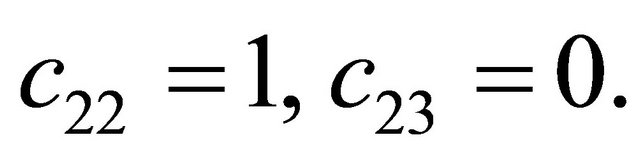 In this case, by means of linear combinations and by the automorphisms
In this case, by means of linear combinations and by the automorphisms 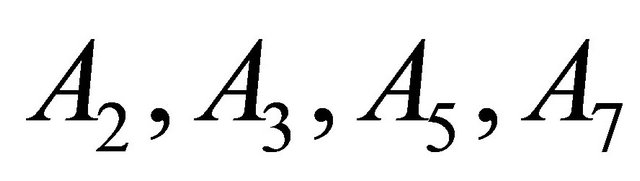 the table of coefficients is transformed to
the table of coefficients is transformed to
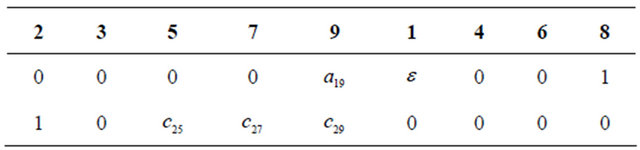
The subalgebra conditions give

where the coefficients ![]() and
and 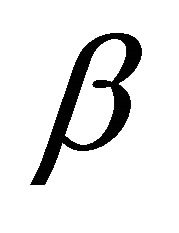 are arbitrary constants. Comparing the coefficients, one obtains
are arbitrary constants. Comparing the coefficients, one obtains
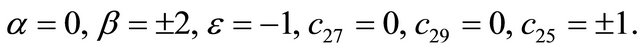
Therefore, in this case the subalgebra is 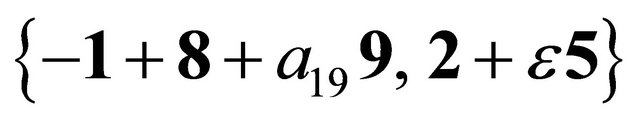 .
.
Case 2. Since , or
, or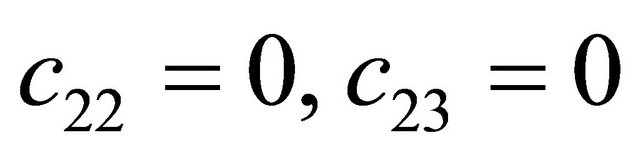 . Because of
. Because of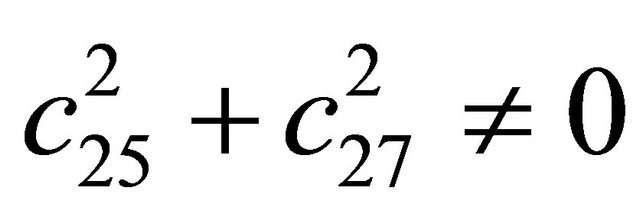 , by virtue of the automorphism
, by virtue of the automorphism  one can take
one can take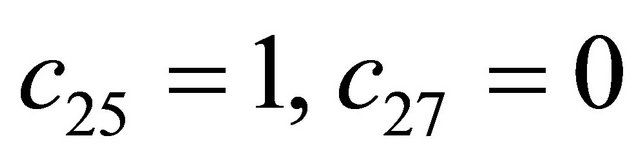 . By means of linear combinations and by the automorphisms
. By means of linear combinations and by the automorphisms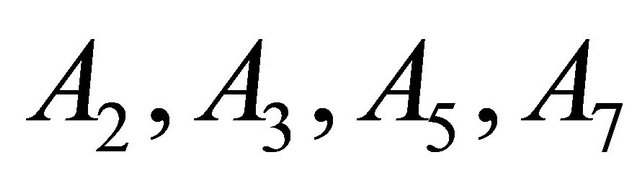 , the coefficients are transformed to
, the coefficients are transformed to

The subalgebra condition gives
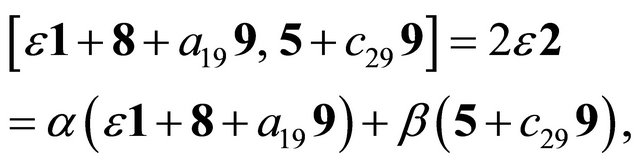
where the coefficients ![]() and
and 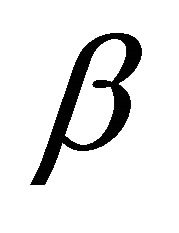 are arbitrary constants. Comparing the coefficients, one obtains
are arbitrary constants. Comparing the coefficients, one obtains
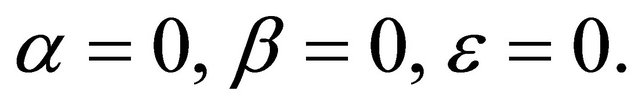
This is a contradiction to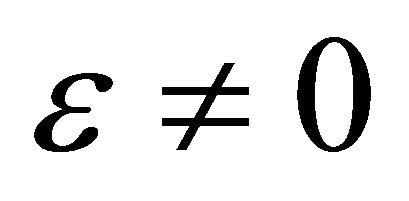 . Therefore, there exists no subalgebra in this case.
. Therefore, there exists no subalgebra in this case.
Case 3. Assume that 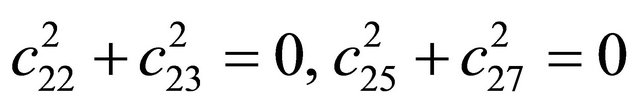 and
and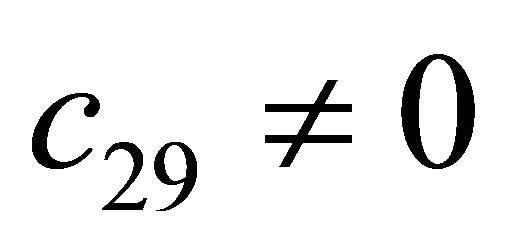 , or
, or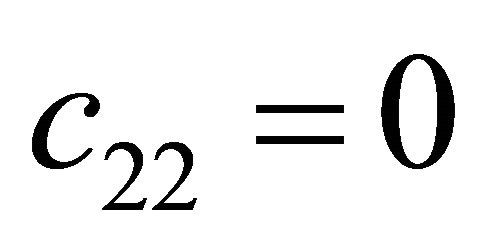 ,
, 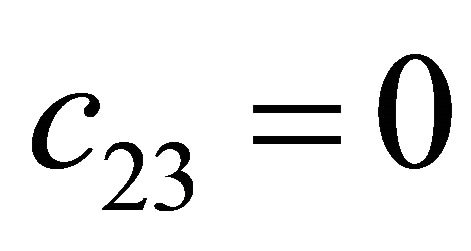 ,
,  ,
,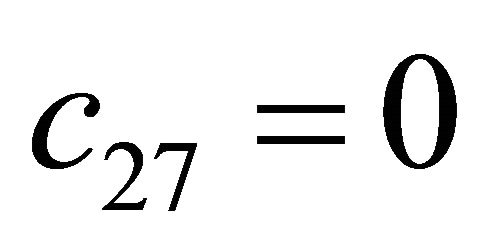 . Since
. Since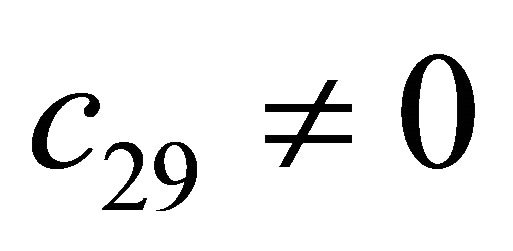 , without loss of generality one can choose
, without loss of generality one can choose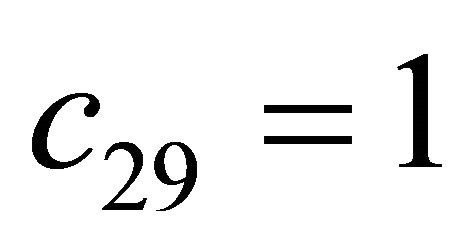 . By taking linear combinations and by virtue of the automorphism
. By taking linear combinations and by virtue of the automorphism 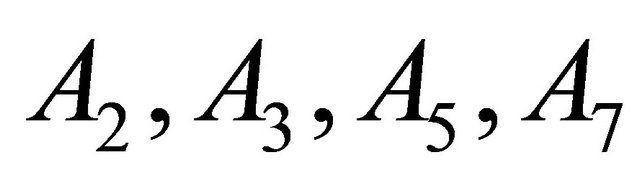 the table of coefficients can be transformed to
the table of coefficients can be transformed to
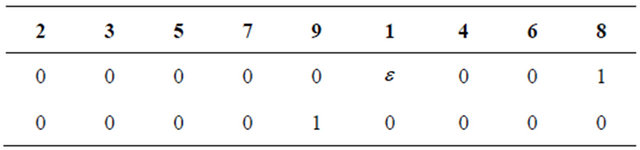
The subalgebra conditions give
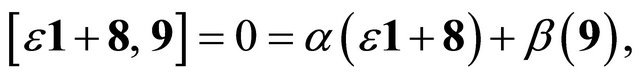
which is satisfied with
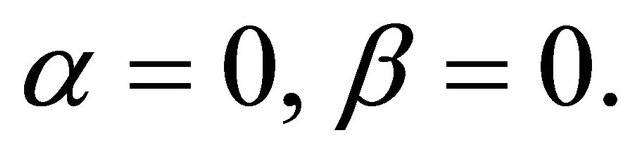
Therefore, the subalgebra is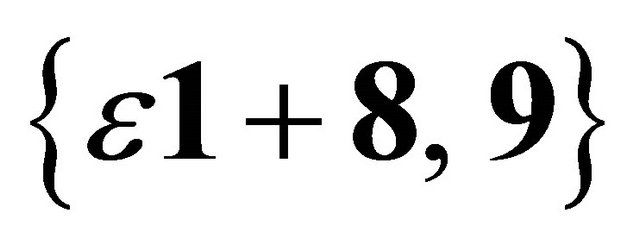 . Other elements of the optimal system of the algebra
. Other elements of the optimal system of the algebra 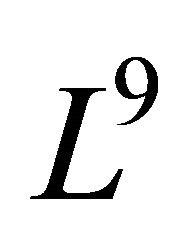 are constructed in the similar way.
are constructed in the similar way.
The list of two-dimensional subalgebras of the optimal system of the algebra  is presented in Table 1.
is presented in Table 1.
7. Invariant Solutions of Equation (1)
Invariant solutions of Equation (1) are presented in this section. Analysis of invariant solutions is presented in details for two examples.
7.1. Subalgebra 7: 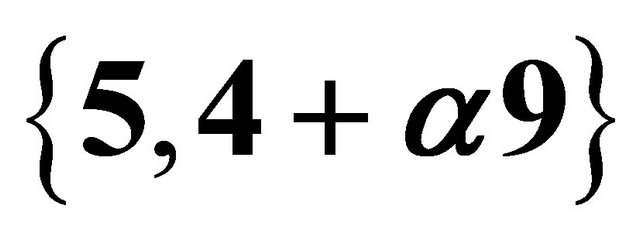
The basis of this subalgebra is
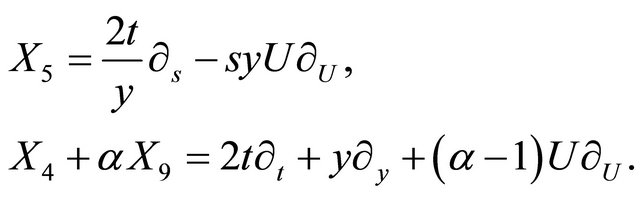
Let a function
Table 1. Two-dimensional subalgebras of the optimal system of the algebra .
.

be an invariant of the generator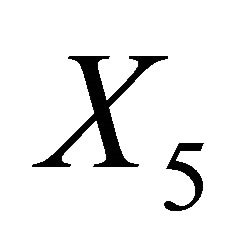 . This means that
. This means that
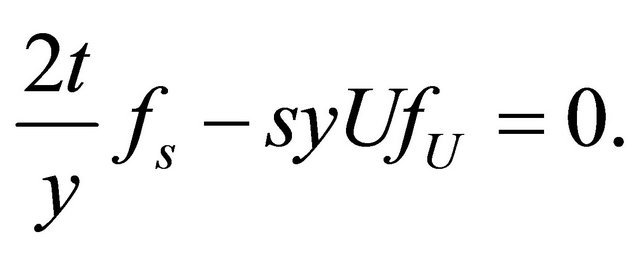
The general solution of this equation is

After substituting it into the equation 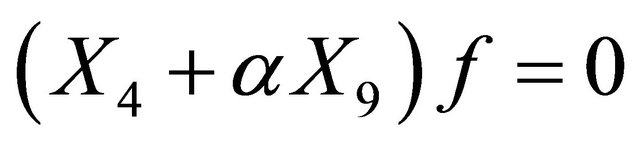 , one obtains the equation
, one obtains the equation
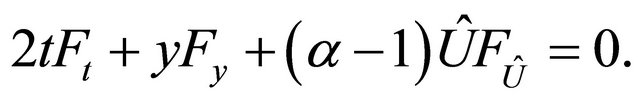
The characteristic system of the last equation is
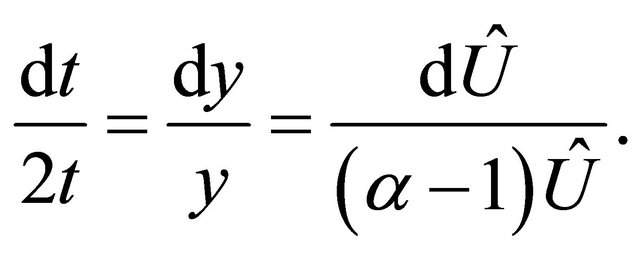
Thus the universal invariant of this subalgebras consists of invariants

Hence, a representation of the invariant solution is
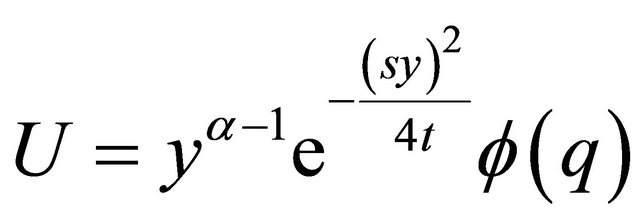
with arbitrary functions 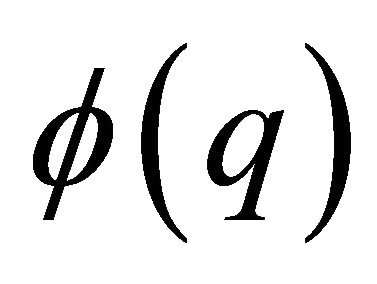 and
and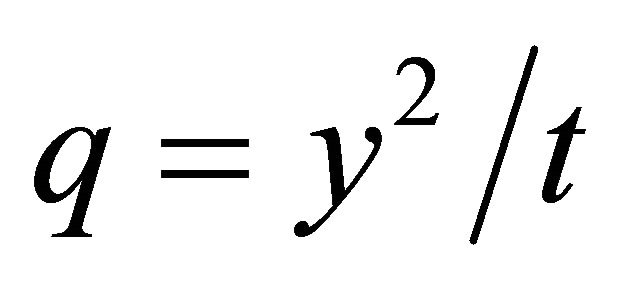 . After substituting this representation into Equation (1), one obtains the ordinary differential equation
. After substituting this representation into Equation (1), one obtains the ordinary differential equation
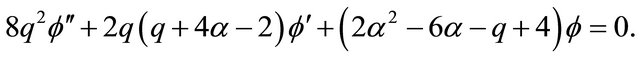
The general solution of the last equation is
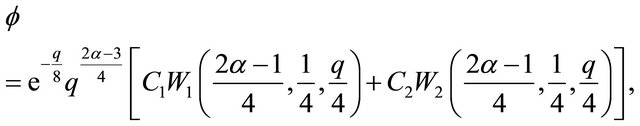
where  are Whittaker functions and
are Whittaker functions and 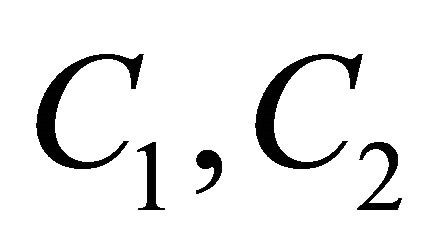 are arbitrary constants.
are arbitrary constants.
7.2. Subalgebra 16: 
The basis of this subalgebra consists of the generators
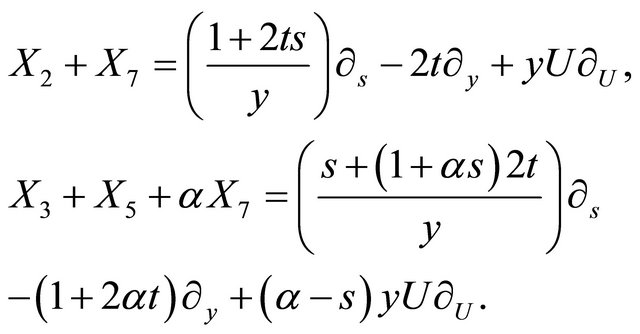
In order to find an invariant solution, one needs to find a universal invariant of this subalgebra. Let a function
be an invariant of the generator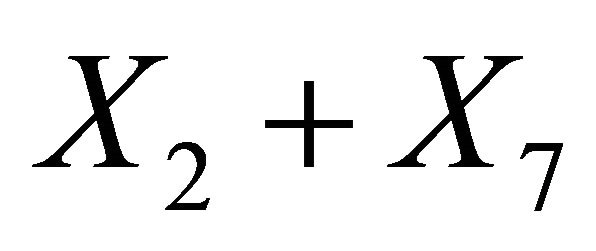 . This means that
. This means that

The characteristic system of the last equation is
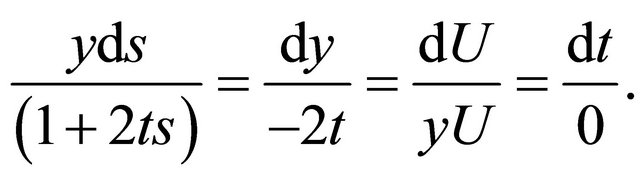
The general solution of this equation is
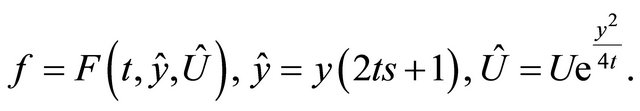
After substituting it into the equation

one obtains the equation
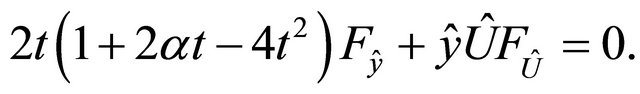
The characteristic system of this equation is
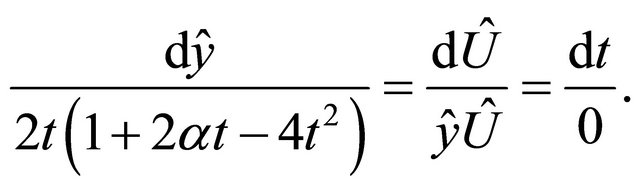
Hence, the universal invariant of this subalgebras consists of invariants
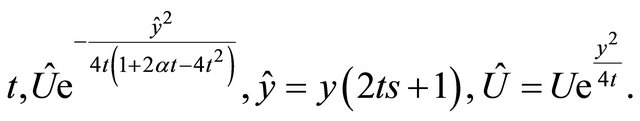
A representation of the invariant solution of this subalgebra has the following form
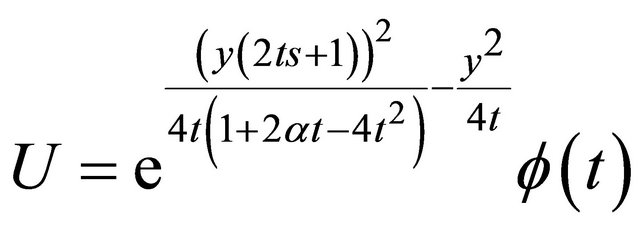
with an arbitrary function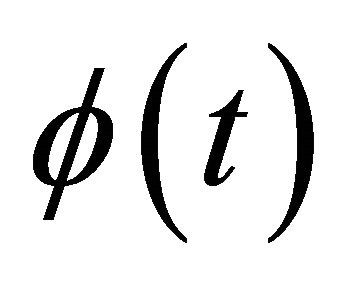 . After substituting the representation of the invariant solution into Equation (1), the functions
. After substituting the representation of the invariant solution into Equation (1), the functions 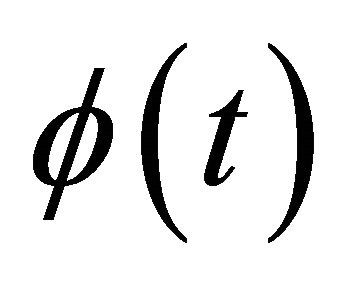 has to satisfy the equation
has to satisfy the equation
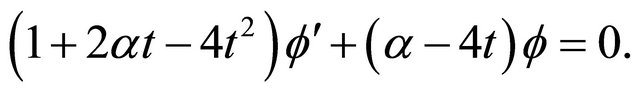
The general solution of the last equation is
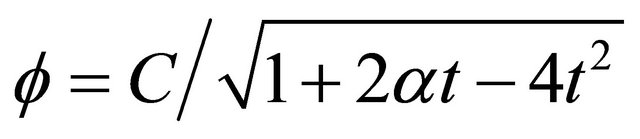
where 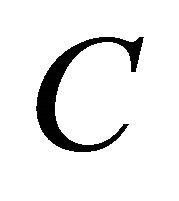 is constant.
is constant.
The two examples showed that there are solutions of the Navier-Stokes equations, which are partially invariant with respect to not admitted Lie algebra  .
.
8. Conclusion
The algorithm of obtaining an optimal system of subalgebras was applied to the reduction of the NavierStokes equations. Some exact invariant solutions corresponding to the optimal system are presented. Examples given in the manuscript showed that this algorithm can be applied to groups, which are not admitted. These possibilities extend an area of using group analysis for constructing exact solutions.
9. Acknowledgements
This research is supported by the Centre of Excellence in Mathematics, the Commission on Higher Education, Thailand.
REFERENCES
- L. V. Ovsiannikov and A. P. Chupakhin, “Regular Partially Invariant Submodels of the Equations of Gas Dynamics,” Journal of Applied Mechanics and Technics, Vol. 6, No. 60, 1996, pp. 990-999.
- S. Lie, “On General Theory of Partial Differential Equations of an Arbitrary Order,” German, No. 4, 1895, pp. 320-384.
- L. V. Ovsiannikov, “Partly Invariant Solutions of the Equations Admitting a Group,” Proceedings of the 11th International Congress of Applied Mechanics, SpringerVerlag, Berlin, 1964, pp. 868-870.
- V. V. Pukhnachov, “Group Properties of the NavierStokes Equations in Two-Dimensional Case,” Journal of Applied Mechanics and Technical Physics, Vol. 44, No. 3, 1960, pp. 317-323. doi:10.1023/A:1023472921305
- V. O. Bytev, “Group Properties of Navier-Stokes Equations,” Chislennye Metody Mehaniki Sploshnoi Sredy, Vol. 3, No. 3, 1972, pp. 13-17.
- S. V. Khabirov, “Partially Invariant Solutions of Equations of Hydrodynamics,” Exact Solutions of Differential Equations and Their Assymptotics, Ufa, 1992.
- B. J. Cantwell, “Introduction to Symmetry Analysis,” Camridge University Press, Camridge, 2002.
- B. J. Cantwell, “Similarity Transformations for the TwoDimensional, Unsteady, Stream-Function Equation,” Journal of Fluid Mechanics, Vol. 85, No. 2, 1978, pp. 257- 271. doi:10.1017/S0022112078000634
- S. P. Lloyd, “The Infinitesimal Group of the NavierStokes Equations,” Acta Mathematica, Vol. 38, 1981, pp. 85-98.
- R. E. Boisvert, W. F. Ames and U. N. Srivastava, “Group Properties and New Solutions of Navier-Stokes Equations,” Journal of Engineering Mathematics, Vol. 17, 1983, pp. 203-221. doi:10.1007/BF00036717
- A. Grauel and W. H. Steeb, “Similarity Solutions of the Euler Equation and the Navier-Stokes Equations in Two Space Dimensions,” International Journal of Theoretical Physics, No. 24, No. 3, 1985, pp. 255-265. doi:10.1007/BF00669790
- N. H. Ibragimov and G. Unal, “Equivalence Transformations of Navier-Stokes Equation,” Bulletin of the Technical University of Istanbul, Vol. 1-2, No. 47, 1994, pp. 203-207.
- R. O. Popovych, “On Lie Reduction of the Navier-Stokes Equations,” Nonlinear Mathematical Physics, Vol. 3-4, No. 2, 1995, pp. 301-311. doi:10.2991/jnmp.1995.2.3-4.10
- W. I. Fushchich and R. O. Popovych, “Symmetry Reduction and Exact Solution of the Navier-Stokes Equations,” Nonlinear Mathematical Physics, Vol. 1, No. 1, 1994, pp. 75-113. doi:10.2991/jnmp.1994.1.1.6
- W. I. Fushchich and R. O. Popovych, “Symmetry Reduction and Exact Solution of the Navier-Stokes Equations,” Nonlinear Mathematical Physics, Vol. 2, No. 1, 1994, pp. 158-188. doi:10.2991/jnmp.1994.1.2.3
- D. K. Ludlow, P. A. Clarkson and A. P. Bassom, “Similarity Reduction and Exact Solutions for the Two-Dimensional Incompressible Navier-Stokes Equations,” Studies in Applied Mathematics, Vol. 103, 1999, pp. 183- 240. doi:10.1111/1467-9590.00125
- V. V. Pukhnachov, “Free Boundary Problems of the Navier—Stokes Equations,” Doctoral Thesis, Novosibirsk, 1974.
- A. F. Sidorov, V. P. Shapeev and N. N. Yanenko, “The Method of Differential Constraints and Its Applications in Gas Dynamics,” Nauka, Novosibirsk, 1984.
- S. V. Meleshko, “Classification of the Solutions with Degenerate Hodograph of the Gas Dynamics and Plasticity Equations,” Doctoral Thesis, Ekaterinburg, 1991.
- S. V. Meleshko, “One Class of Partial Invariant Solutions of Plane Gas Flows,” Differential Equations, Vol. 10, No. 30, 1994, pp. 1690-1693.
- L. V. Ovsiannikov, “Isobaric Motions of a Gas,” Differential Equations, Vol. 10, No. 30, 1994, pp. 1792-1799.
- A. P. Chupakhin, “On Barochronic Motions of a Gas,” Doklady Rossijskoj Akademii Nauk, Vol. 5, No. 352, 1997, pp. 624-626.
- A. M. Grundland and L. Lalague, “Invariant and partially Invariant Solutions of the Equations Describing A NonStationary and Isotropic Flow for an Ideal and Compressible Fluid in (3+1) Dimensions,” Journal of Physics A: Mathematical and General, Vol. 29, No. 8, 1996, pp. 1723-1739. doi:10.1088/0305-4470/29/8/019
- L. V. Ovsiannikov, “Group Analysis of Differential Equations,” Nauka, Moscow, 1978.
- L. V. Ovsiannikov, “Regular and Irregular Partially Invariant Solutions,” Doklady Academy of Sciences of USSR, Vol. 2, No. 343, 1995, pp. 156-159.
- L. V. Ovsiannikov, “Program SUBMODELS. Gas Dynamics,” Journal of Applied Mathematics and Mechanics, Vol. 58, 1994, pp. 30-55.
NOTES
1A classification of the two-dimensional Navier-Stokes equations was studied in [4].
2There is still no complete classification of the subalgebras of the Lie algebra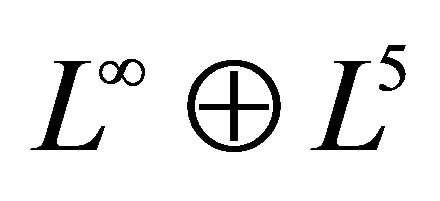 . Classification of infinite—dimensional subalgebras of this algebra was studied in [6].
. Classification of infinite—dimensional subalgebras of this algebra was studied in [6].
3Short reviews devoted to invariant solutions of the Navier-Stokes equations can be found in [14-16].
4Firstly the approach of partially invariant solutions to the NavierStokes equations was applied in [17].

