Applied Mathematics
Vol. 3 No. 11 (2012) , Article ID: 24539 , 6 pages DOI:10.4236/am.2012.311238
A Variational Inequality Approach to a Class of Environmental Equilibrium Problems
1School of Mathematical Sciences, Rochester Institute of Technology, Rochester, USA
2Department of Mathematics and Computer Science, University of Catania, Catania, Italy
Email: bxjsma@rit.edu, fraciti@dmi.unict.it
Received September 13, 2012; revised October 13, 2012; accepted October 20, 2012
Keywords: Nash Equilibrium; Variational Inequalities; Environmental Games
ABSTRACT
In this note we consider a class of environmental games recently proposed in the literature and investigate them by using the powerful tools of variational inequalities. We also consider the case where some data of the problem can depend on a parameter and analyze the regularity of the solution with respect to the parameter. In view of applications to time-dependent or random models, we also introduce the variational inequality formulation in Lebesgue spaces.
1. Introduction
The last decade has witnessed a growing interest in the application of game theory to environmental problems. At an international level, the Durban conference (2011) has revived the importance of global agreements (e.g. the Kyoto Protocol) which require that each signatory country bounds its polluting emission to a prefixed level. Among the vaste literature on environmental games, here we recall that the game theory formulation of Kyoto Protocol has been introduce by Breton et al. [1] in the case of two players, while an n-players environmental game has been formulated by Tidball and Zaccour [2] for a broad class of revenue and damage cost functions. In this paper we further investigate two scenarios proposed in [2]:
1) The noncooperative scenario where each player optimizes their welfare under their own environmental constraints. The players interact through the damage cost, which is a function of the total emission. In this case a solution is a Nash equilibrium.
2) The umbrella (or bubble) scenario, where the players aim to optimize their individual welfare, but under a shared environmental constraint. In this case a solution is a generalized Nash equilibrium, à la Rosen.
We study these two scenarios via the variational inequalities theory which has proved to be a very powerful tool in the theoretical and numerical analysis of many equilibrium problems (see e.g. [3-5]). We first compare the total emissions resulting from the two scenarios, taking explicitely into account the nonnegativity constraints which, for the sake of simplicity, were relaxed in [2].
Then, we consider the case where the operator or the constraints set depend on a parameter, and study the continuity and Lipschitz continuity of the solution with respect to the parameter (see e.g. [6,7]). This parameter can have the meaning of time, or of a random variable reflecting the uncertainty in the decision variables. Finally, we introduce the Lebesgue space formulation which was successfully applied to tackle time-dependent as well as random equilibrium problems, [8-11].
2. The Model and the Variational Inequality Approach
Each player is a subject who produces, pollutes and aims to maximize his/her welfare function, which is defined as the difference between the revenue resulting from the production and the damage cost due to pollution. As usual we assume that pollution is proportional to the industrial output so that the revenue of player i, 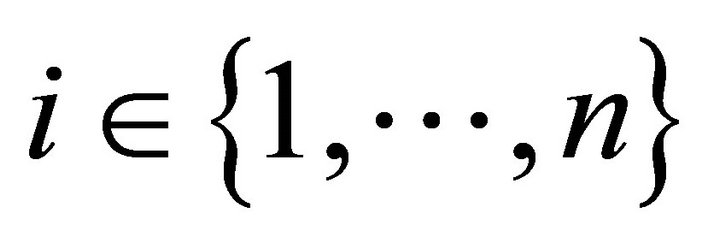 , can be expressed as a function of its polluting emission
, can be expressed as a function of its polluting emission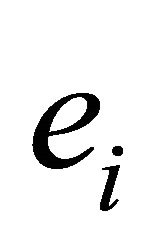 . Let us denote by
. Let us denote by 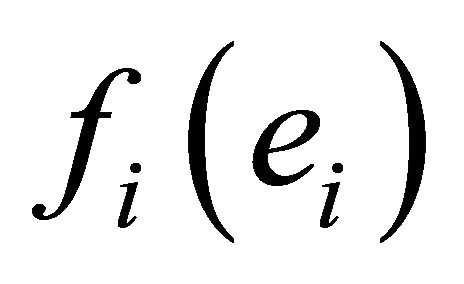 the revenue function of player
the revenue function of player , which is assumed to be nonnegative, increasing, concave and
, which is assumed to be nonnegative, increasing, concave and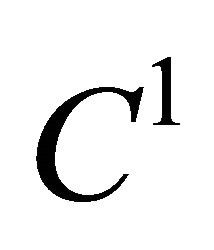 . Assume that the cost of the environmental damage depends on the emissions of all players and denote these functions by
. Assume that the cost of the environmental damage depends on the emissions of all players and denote these functions by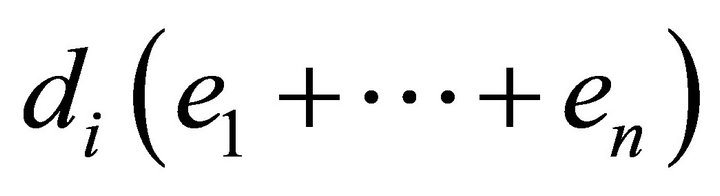 . Each
. Each 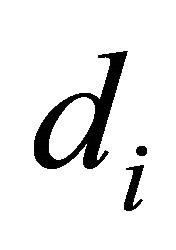 is assumed nonnegative, increasing, convex and
is assumed nonnegative, increasing, convex and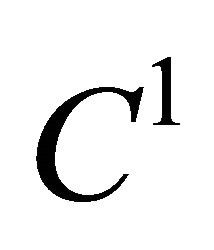 . Thus, the welfare function of player
. Thus, the welfare function of player  is given by:
is given by:
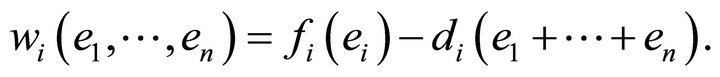 (1)
(1)
In the noncooperative scenario, each player has to satisfy the environmental constraint: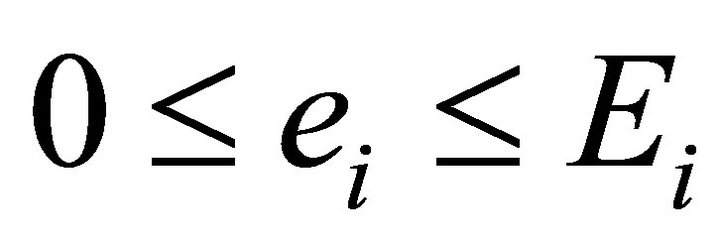 . For each vector
. For each vector 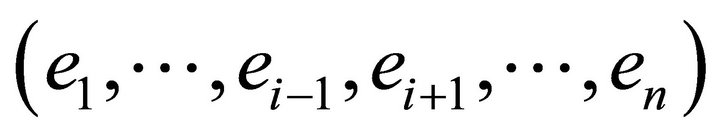 he/she has to solve the optimization problem:
he/she has to solve the optimization problem:
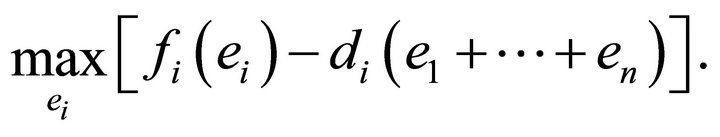
More precisely, the problem under consideration is a Nash equilibrium problem, i.e., to find a vector
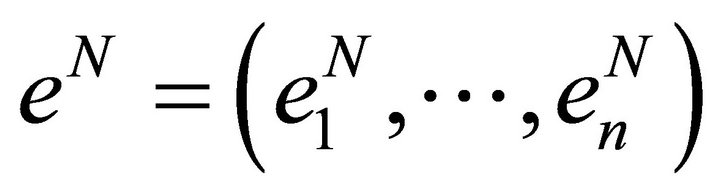 such that for all
such that for all  one has
one has
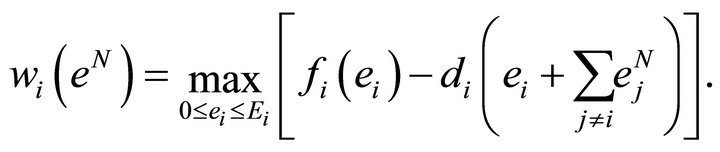
Under the differentiability hypotheses, it is well known (see e.g. [12]) that Nash equilibrium problems are equivalent to variational inequalities. Thus, consider the closed convex set
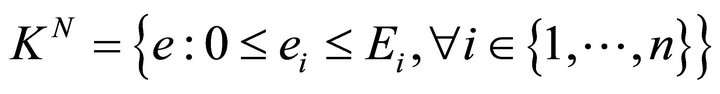
where 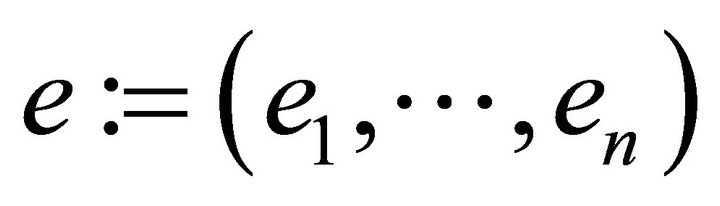 and let
and let  be defined by:
be defined by:
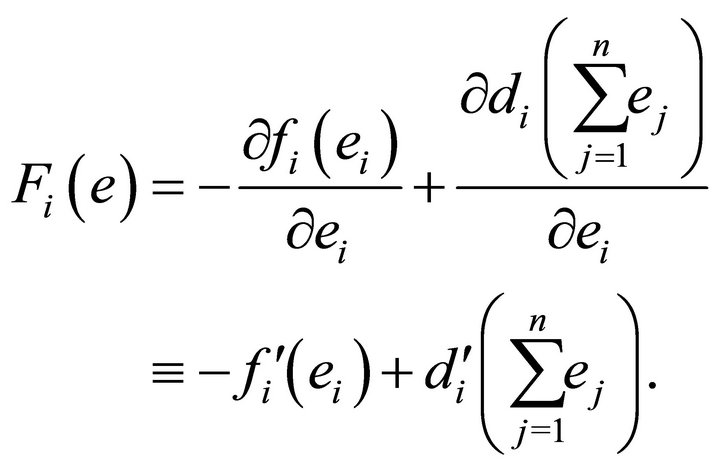
Thus, we can consider the following variational inequality problem: Find 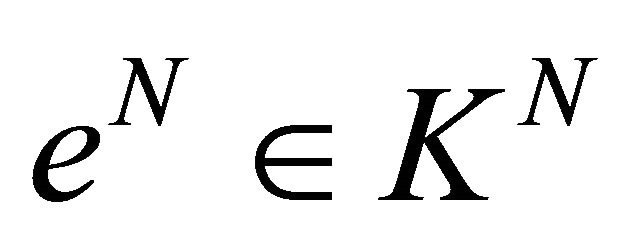
such that
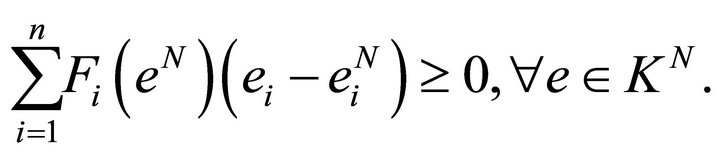 (2)
(2)
In the sequel we shall refer to the above variational inequality as (NVI). Assume that  is a monotone operator i.e.:
is a monotone operator i.e.:
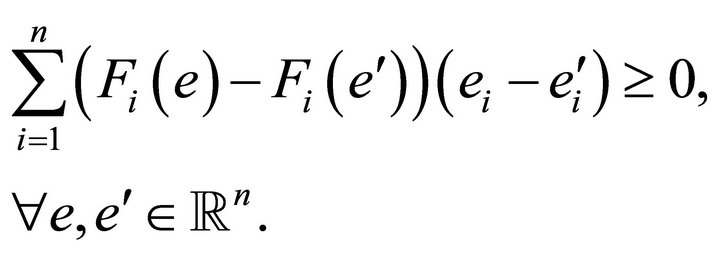 (3)
(3)
In particular, assume that  is strictly monotone in the sense that equality in (3) holds only if
is strictly monotone in the sense that equality in (3) holds only if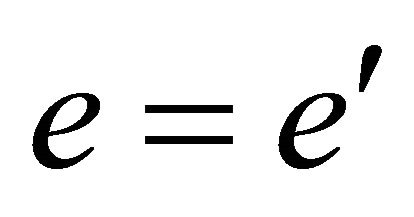 . Since
. Since  is continuous and
is continuous and 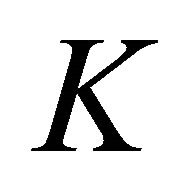 is compact, the Stampacchia theorem applies and (NVI) is solvable (for a survey on existence theorems for variational inequalities see [13]). Moreover the solution is unique under the strict monotonicity hypothesis.
is compact, the Stampacchia theorem applies and (NVI) is solvable (for a survey on existence theorems for variational inequalities see [13]). Moreover the solution is unique under the strict monotonicity hypothesis.
In the umbrella scenario each player  still aims to optimize his/her welfare function, for each vector
still aims to optimize his/her welfare function, for each vector
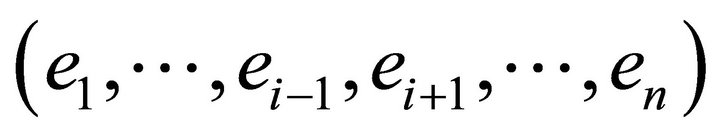 , but the constraints are satisfied jointly by all players. We are then faced with a generalized Nash equilibrium problem (GNEP), that is the problem of finding
, but the constraints are satisfied jointly by all players. We are then faced with a generalized Nash equilibrium problem (GNEP), that is the problem of finding 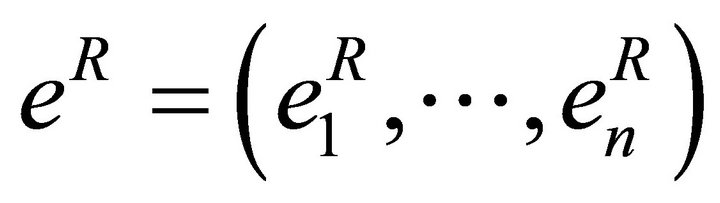 such that for all
such that for all 
one has
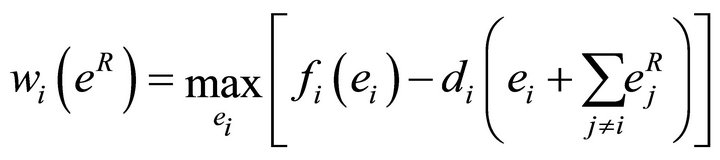
where 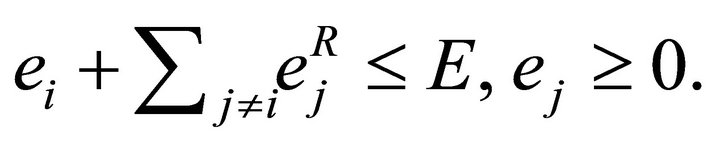
This class of problems was introduced by Rosen in his seminal paper [14] and has been studied quite recently from the point of view of variational inequalities [15]. It is well known that GNEPs have infinite solutions and the problem of selecting certain interesting classes of solutions was already considered by Rosen who introduced the concept of normalized equilibrium. Here we follow and generalize the approach of [15]. Let us fix a vector of positive weights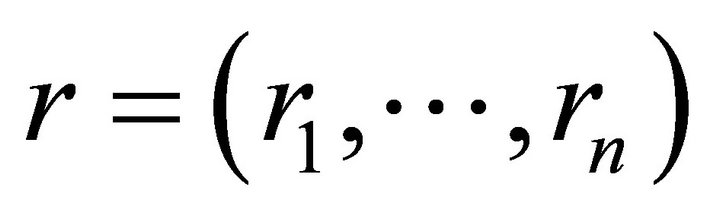 , introduce the operator defined by:
, introduce the operator defined by:
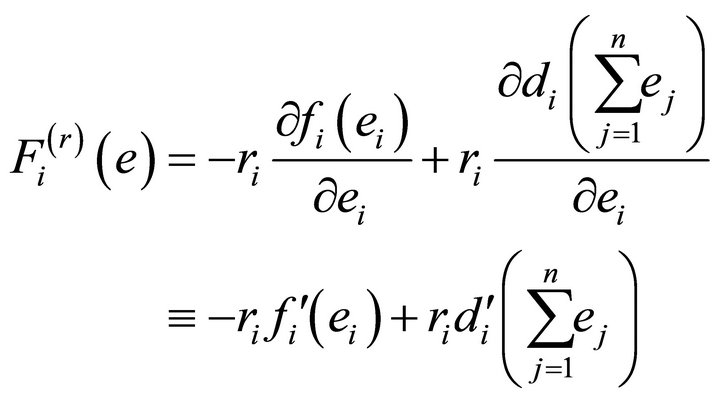
and the closed and convex set:
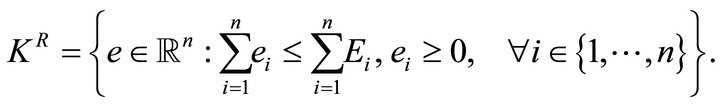
Consider the following variational inequality problem (RVI): Find 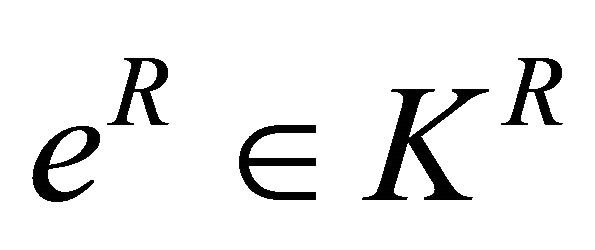 such that
such that
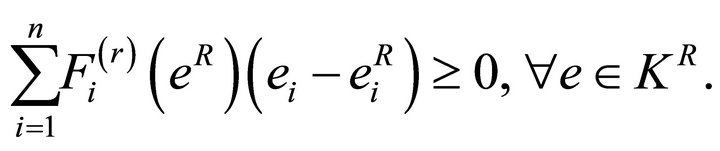 (4)
(4)
For each fixed vector of weights r this variational inequality admits a unique solution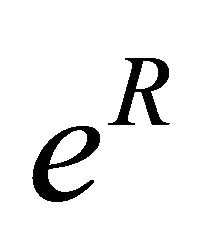 , (since
, (since 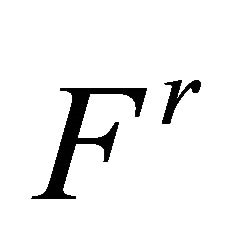 is strictly monotone), which is the normalized Rosen equilibrium corresponding to the given weight. For the economic interpretation of normalized equilibria we refer the interested reader to [2] and to the quoted paper by Rosen. Here we would like to remark that the variational inequality formulation provides a wealth of effective algorithms for the computation of Rosen equilibria.
is strictly monotone), which is the normalized Rosen equilibrium corresponding to the given weight. For the economic interpretation of normalized equilibria we refer the interested reader to [2] and to the quoted paper by Rosen. Here we would like to remark that the variational inequality formulation provides a wealth of effective algorithms for the computation of Rosen equilibria.
3. Comparison between Nash and Rosen Equilibrium
In this section we exploit the variational formulation to compare the total emissions in the two scenarios; we remark that our method does not require the use of Lagrange multipliers. To begin with, let us notice that, if a Rosen equilibrium 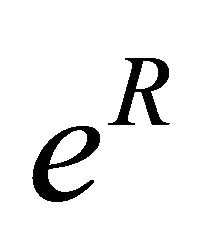 belongs to the interior of
belongs to the interior of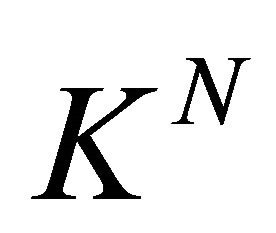 it follows that
it follows that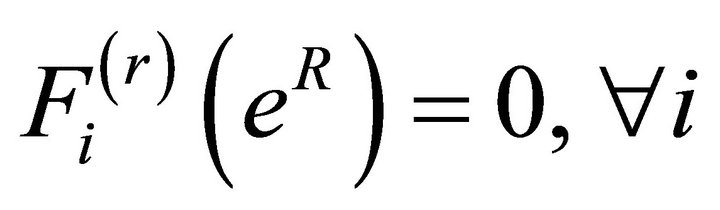 , which also implies
, which also implies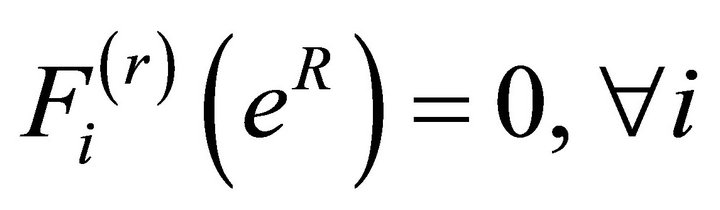 , i.e. Rosen and Nash equilibrium coincide. Hence, we compare Nash and Rosen total emissions in the following family of subsets of
, i.e. Rosen and Nash equilibrium coincide. Hence, we compare Nash and Rosen total emissions in the following family of subsets of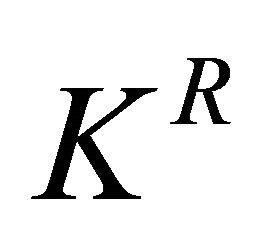 :
:
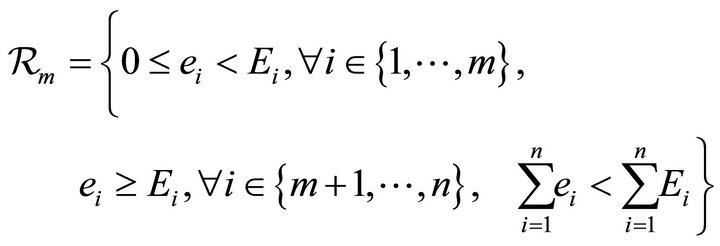
Theorem 1. If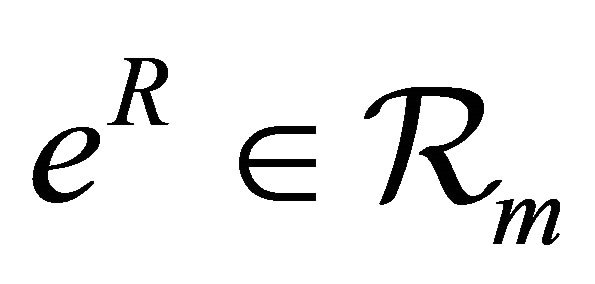 , then
, then
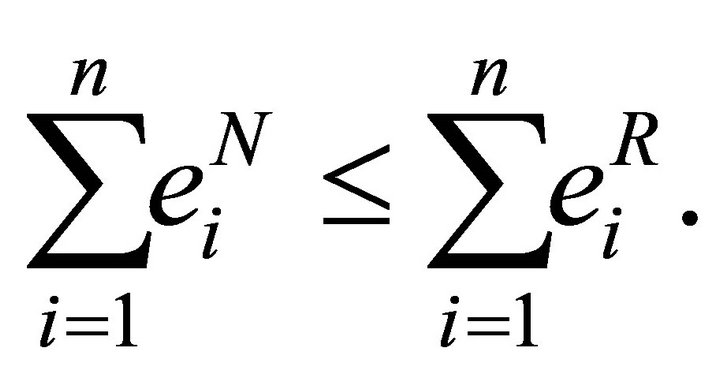
Proof. If the indices  for which
for which 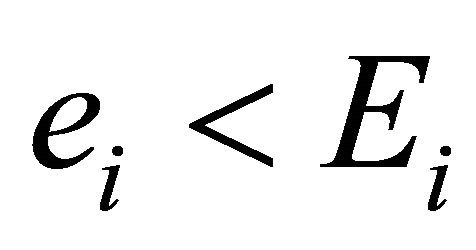 are not the first
are not the first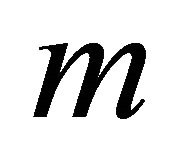 , we can always reorder them and consider this case. If
, we can always reorder them and consider this case. If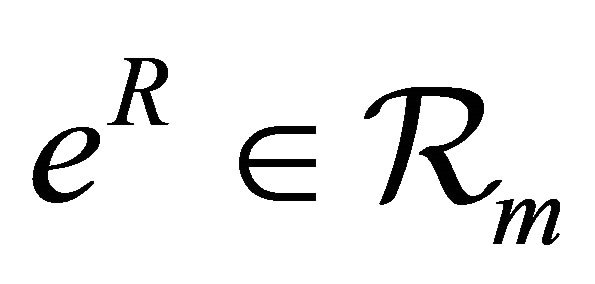 , then
, then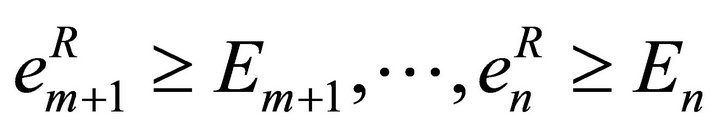 . If
. If  as well, it follows that:
as well, it follows that:
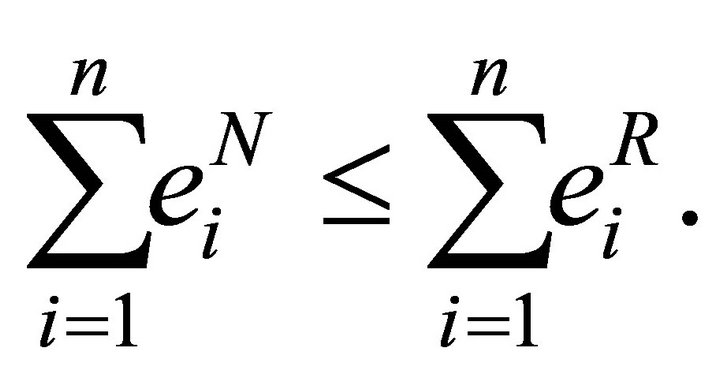 (5)
(5)
We can prove that (5) continue to hold true if 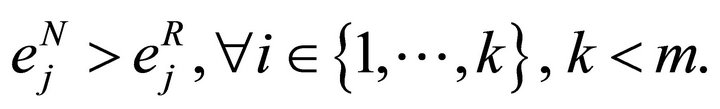
Now, in (4) we can consider as test vector
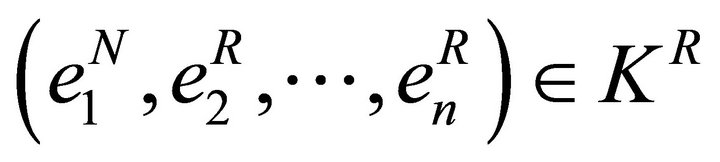 , and obtain,
, and obtain, 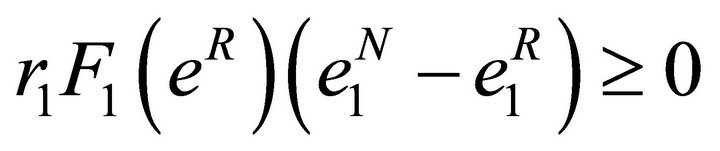 , while in (2) we can choose as test vector
, while in (2) we can choose as test vector
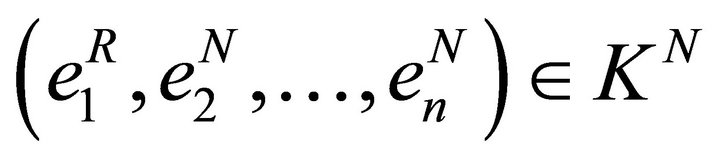 , and obtain
, and obtain 
which still holds true after multiplication by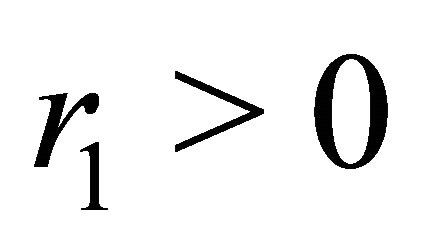 . Summing up these two inequalities we get:
. Summing up these two inequalities we get:
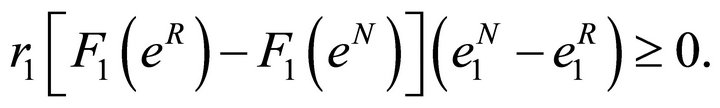 (6)
(6)
Now we write in detail the first factor:
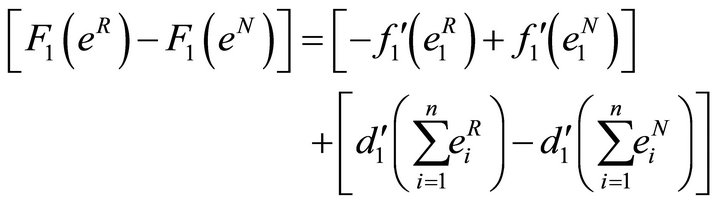
The first expression is negative because 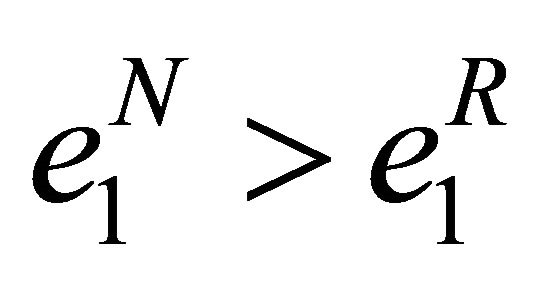 and
and 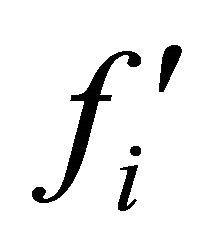 is (strictly) decreasing for all i. Now, let us assumeby contradiction, that
is (strictly) decreasing for all i. Now, let us assumeby contradiction, that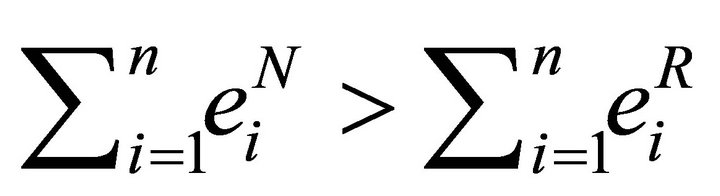 . Then, since
. Then, since 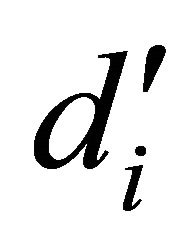
is (strictly) decreasing, the second expression is negative as well. As a consequence we would get
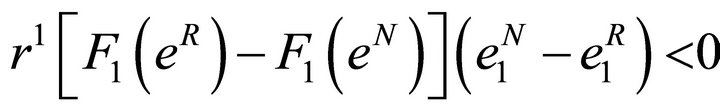 , which contradicts (6).
, which contradicts (6).
4. Extensions of the Model
Let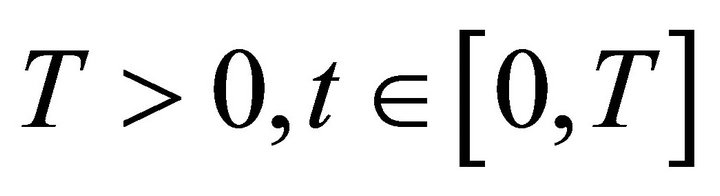 , and assume that both the welfare functions and the constraints can depend on
, and assume that both the welfare functions and the constraints can depend on . More precisely, for each
. More precisely, for each 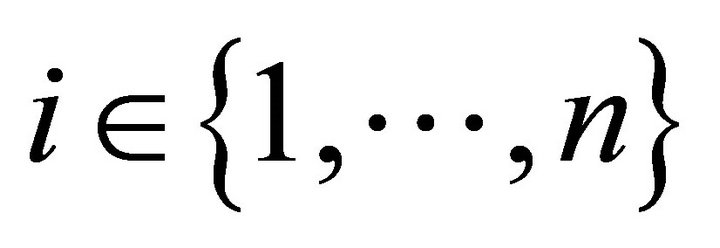 we assume that
we assume that 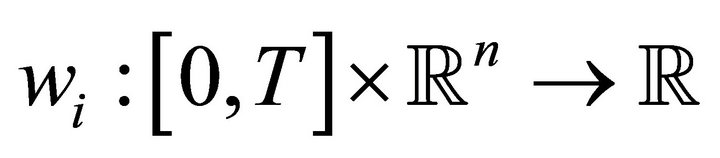 is such that
is such that 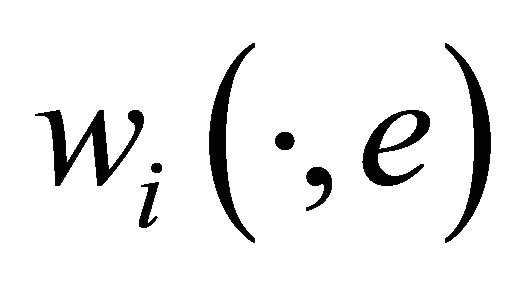 is measurable
is measurable 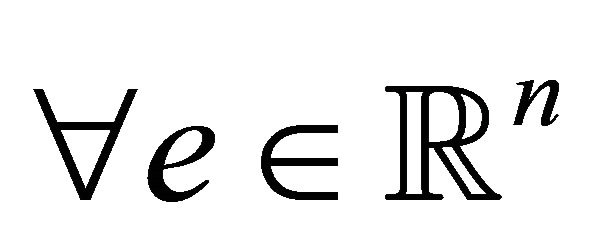 , while
, while  for almost every
for almost every 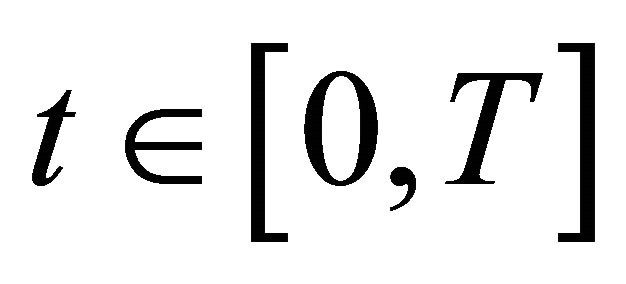 (with respect to the Lebesgue measure). Moreover, we assume that the convexity and the monotonicity assumptions that we did in the nonparametric case hold true a.e. in
(with respect to the Lebesgue measure). Moreover, we assume that the convexity and the monotonicity assumptions that we did in the nonparametric case hold true a.e. in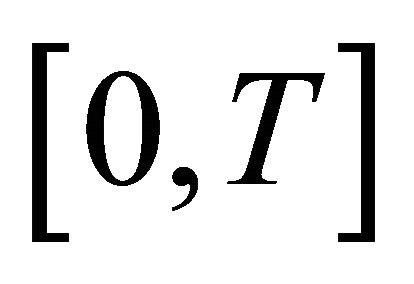 . We are then left with the parametric Nash and Rosen equilibrium problems. The parametric Nash equilibrium problem reads as follows.
. We are then left with the parametric Nash and Rosen equilibrium problems. The parametric Nash equilibrium problem reads as follows.
For a.e.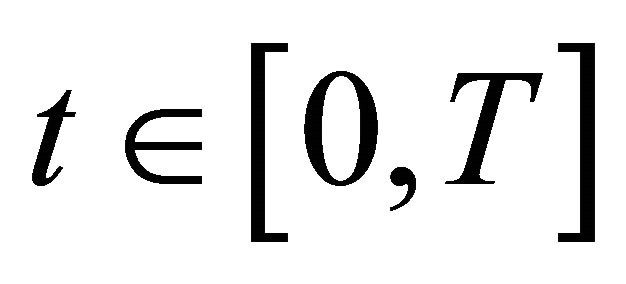 , find
, find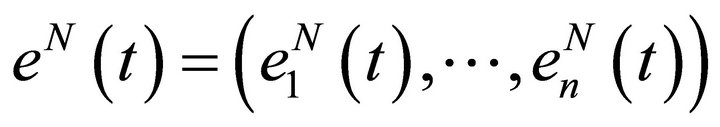 :
:
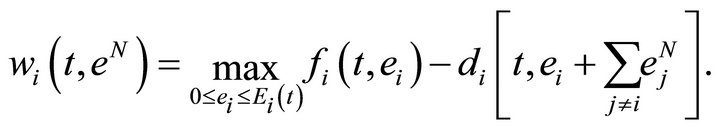
The parametric Rosen equilibrium problem is: for a.e. , find
, find :
:
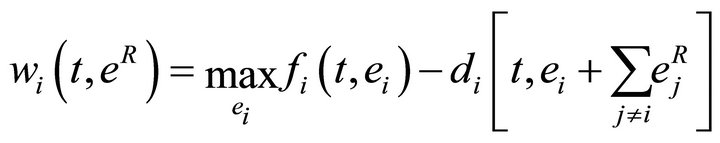
where 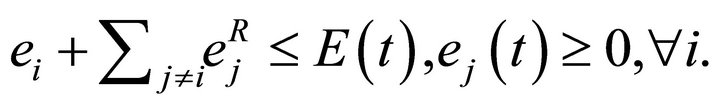
We can then consider the parametric versions of Nash and Rosen variational inequalities. For each  consider the two closed and convex subsets of
consider the two closed and convex subsets of .
.
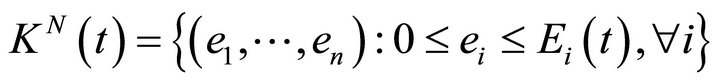
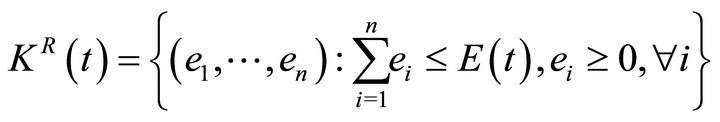
where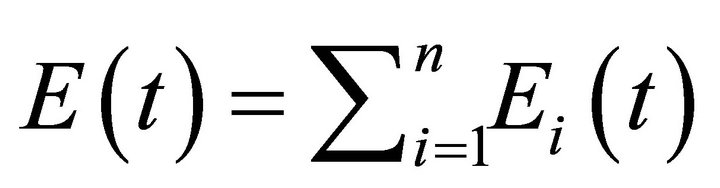 . Moreover, let
. Moreover, let
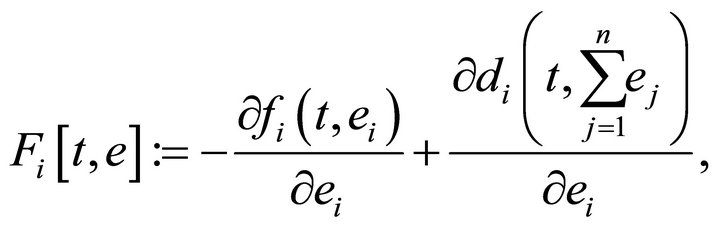
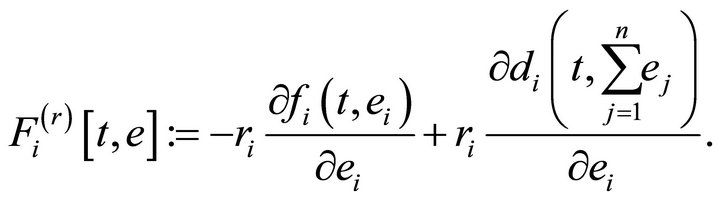
Thus, Nash parametric variational inequality is the following problem:
for a.e.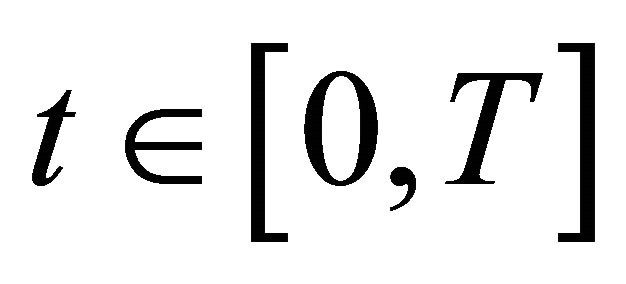 , find
, find 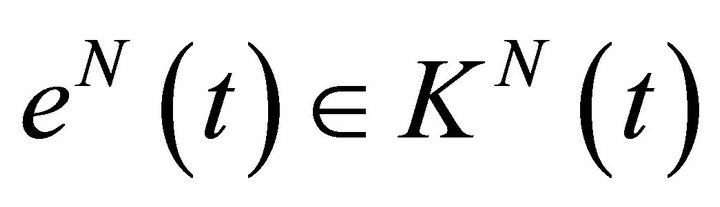 such that
such that
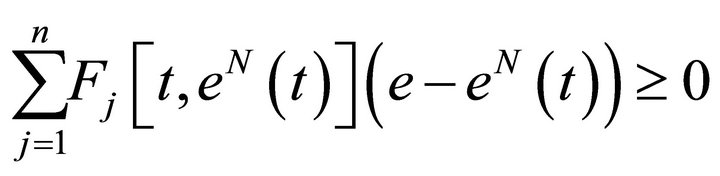 (7)
(7)
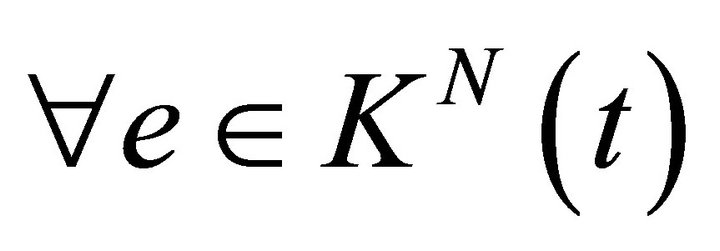 , while Rosen parametric variational inequality reads as follows. For a.e.
, while Rosen parametric variational inequality reads as follows. For a.e.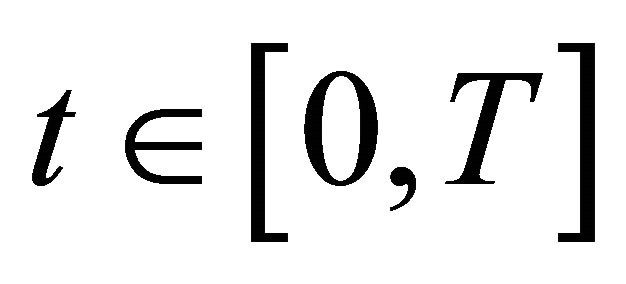 , find
, find 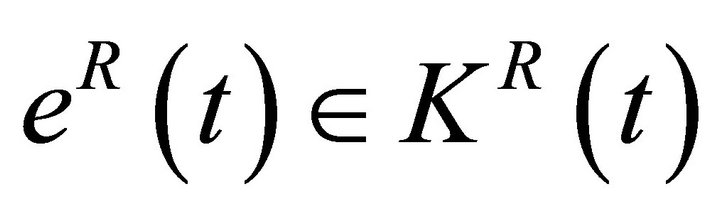 with
with
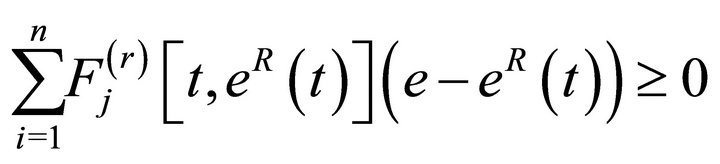 (8)
(8)
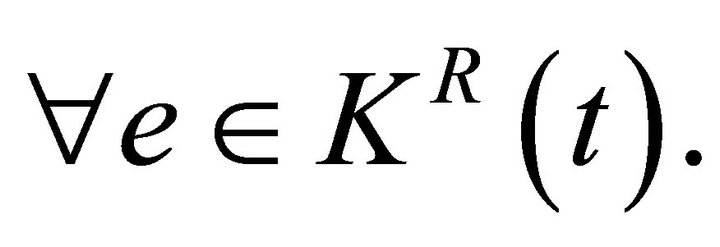 Since
Since  and
and 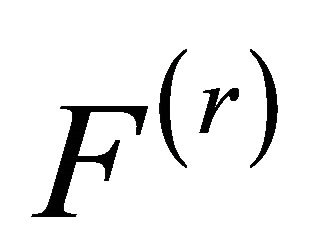 are strictly monotone for a.e.
are strictly monotone for a.e.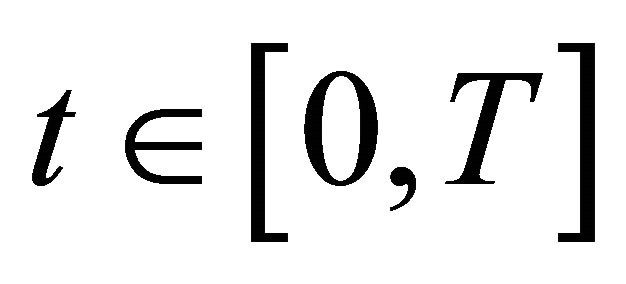 , it follows that the solution maps
, it follows that the solution maps 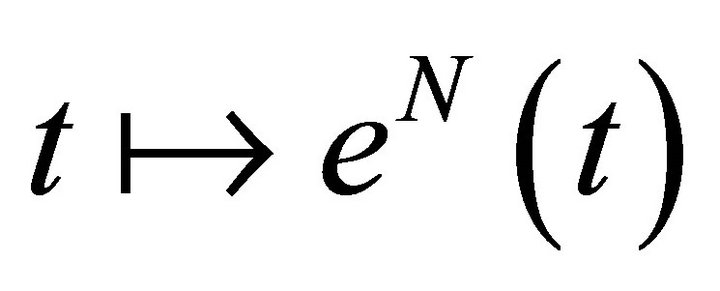 and
and 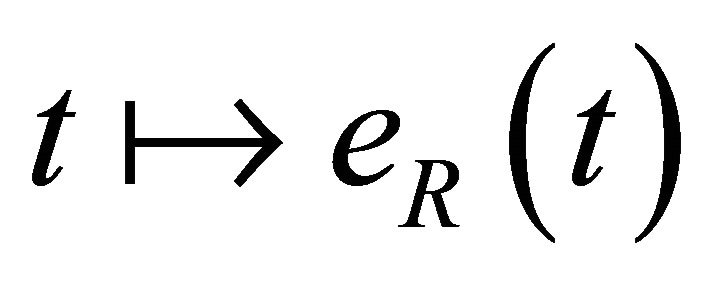 are single valued. To prove the continuity of these maps we state a theorem whose proof can be easly derived along the same line as theorem 2.1 in [6].
are single valued. To prove the continuity of these maps we state a theorem whose proof can be easly derived along the same line as theorem 2.1 in [6].
Theorem 2. Let 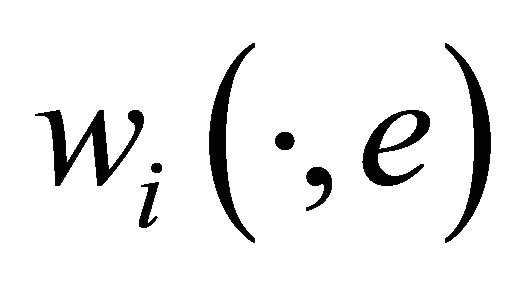 be continuous on
be continuous on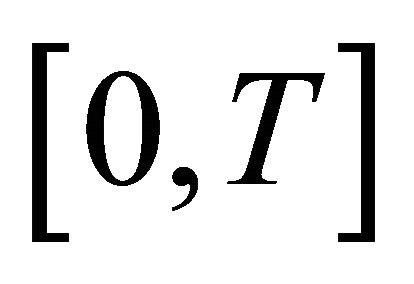 ,
,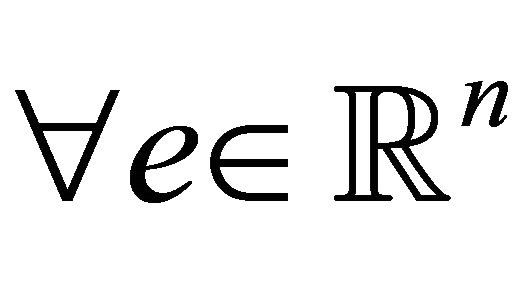 ;
;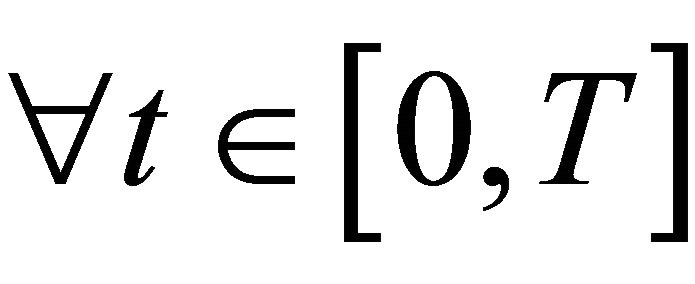
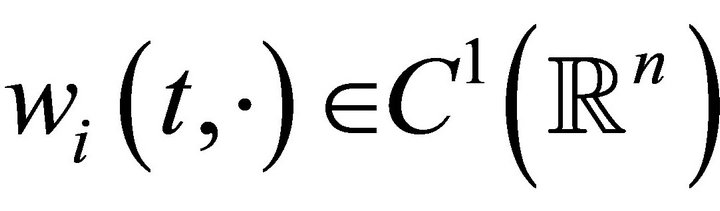 . Moreover, assume that,
. Moreover, assume that, 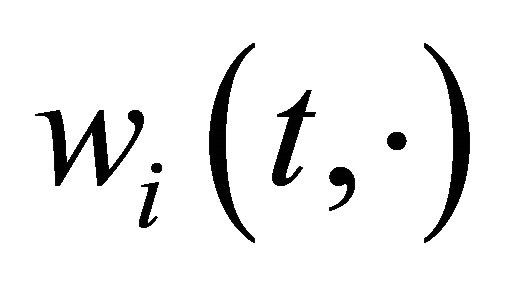 is strictly concave for all i, and
is strictly concave for all i, and 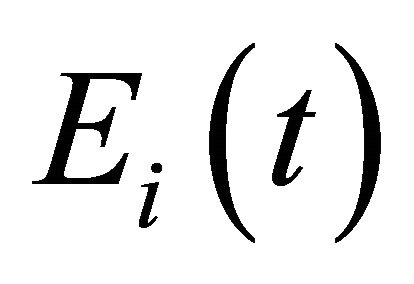 is continuous. Then, (7), (resp. (8)), has a unique solution
is continuous. Then, (7), (resp. (8)), has a unique solution , (resp.
, (resp. 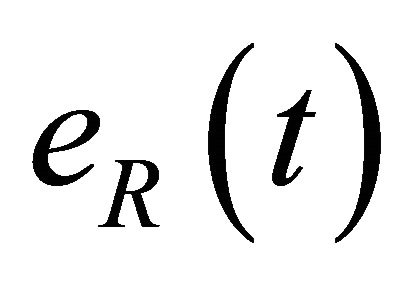 which is continuous on
which is continuous on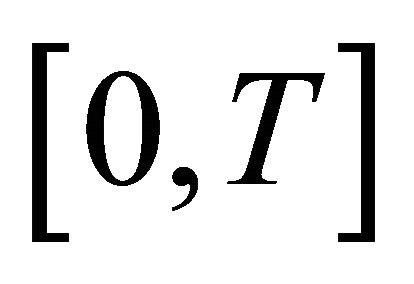 .
.
In the next theorem we need the following property:
Definition 1. Let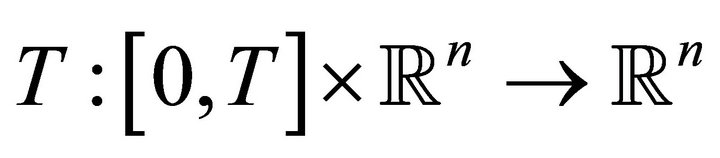 . We call T uniformly strongly monotone on
. We call T uniformly strongly monotone on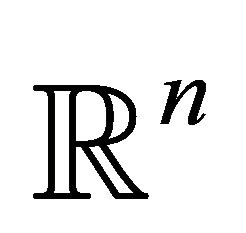 , iff
, iff 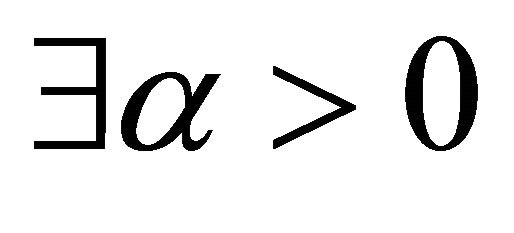 such that:
such that:
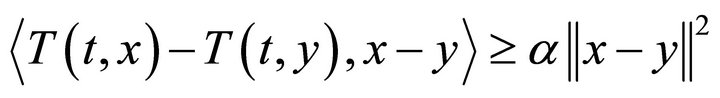 (9)
(9)
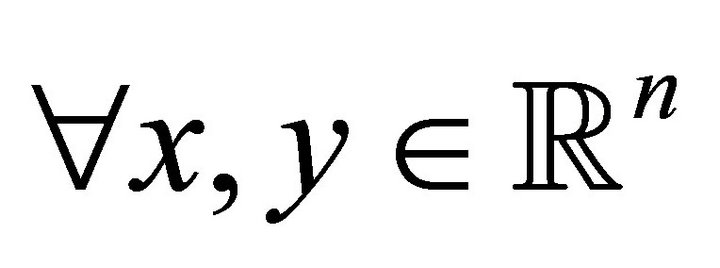 and
and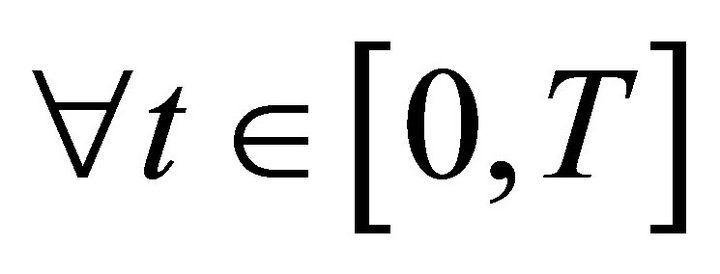 .
.
Theorem 3. Let F, (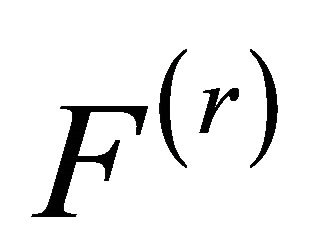 ), be uniformly strongly monotone on
), be uniformly strongly monotone on 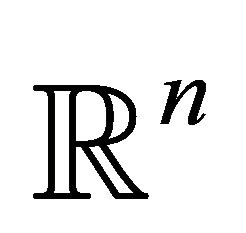 and Lipschitz continuous on
and Lipschitz continuous on 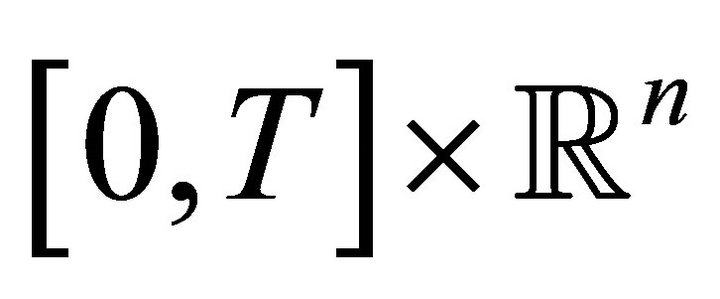 .
.
Moreover, assume that 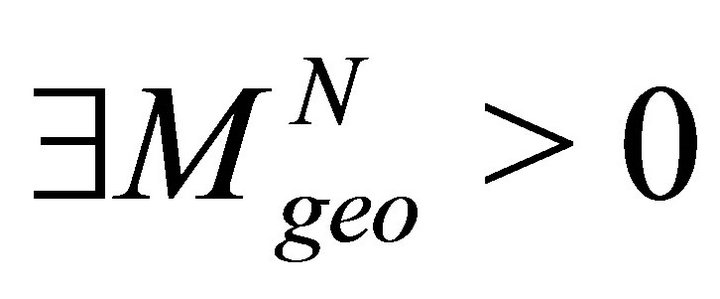 such that,
such that, 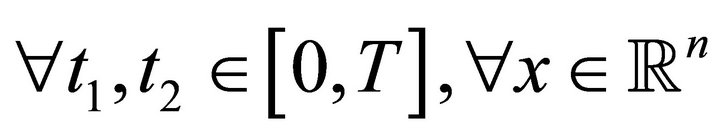
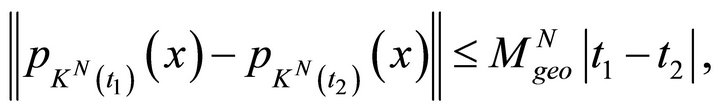
(respectively, 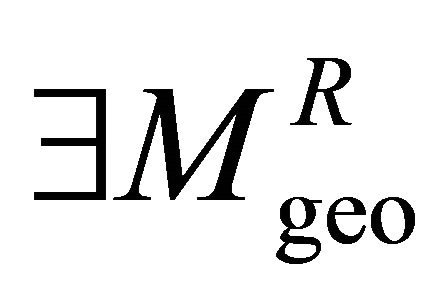 such that
such that
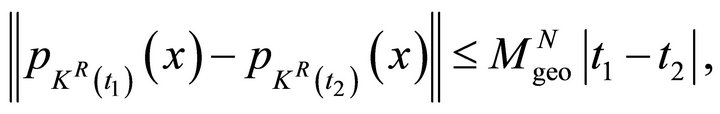 )
)
where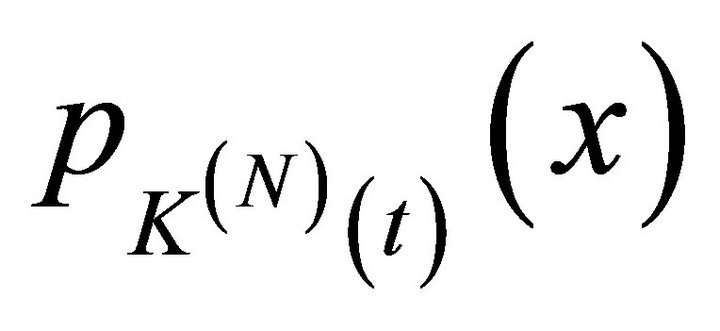 , (
, (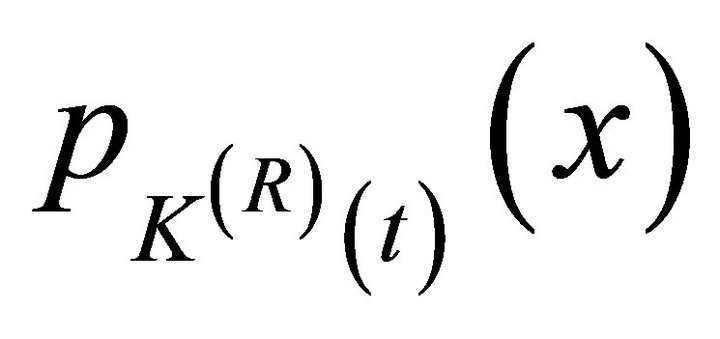 ), denotes the projection of a point
), denotes the projection of a point 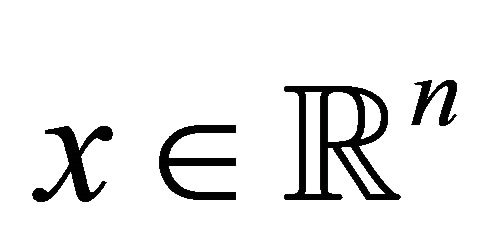 onto the set
onto the set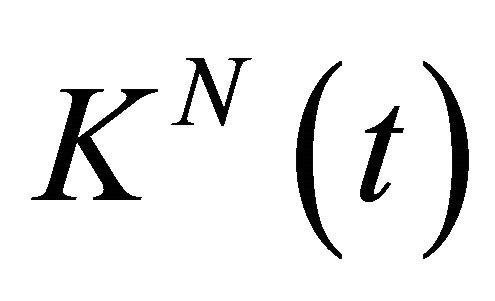 , (
, (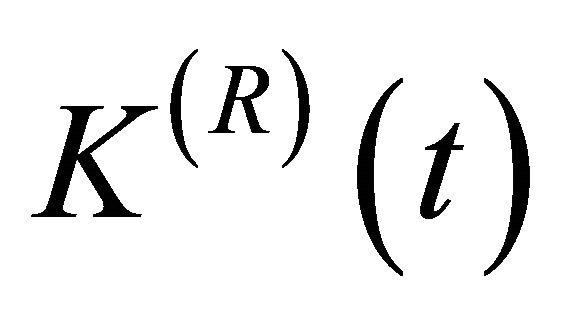 ).
).
Then, the solution 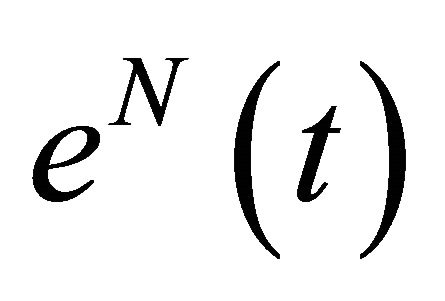 of (7), (
of (7), (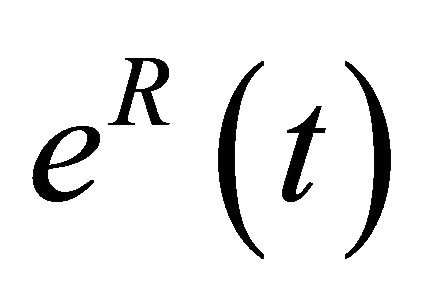 of (8) respectively), is Lipschitz continuous on
of (8) respectively), is Lipschitz continuous on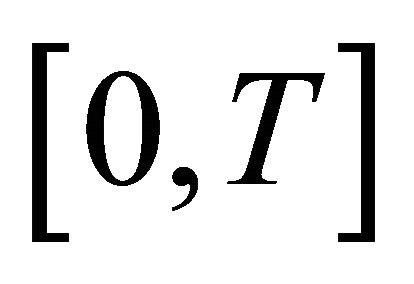 .
.
For the existence and computation of the geometrical constant 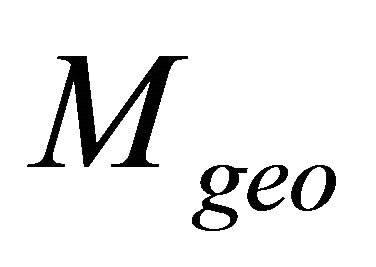 see [7].
see [7].
Now we want to formulate our problems in Lebesgue spaces. For the sake of simplicity we confine ourselves to the Hilbert space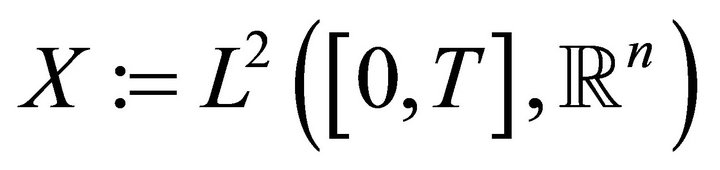 .
.
We shall work under the following set of assumptions:
a) 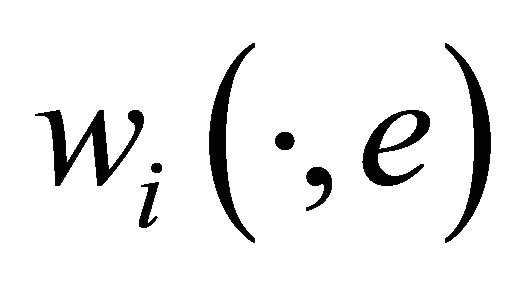 is measurable,
is measurable,  , while
, while 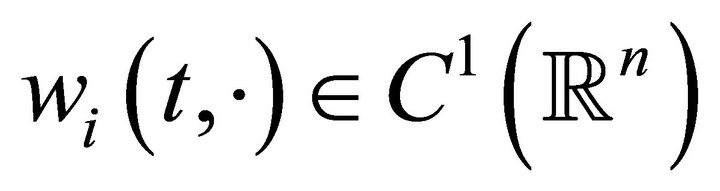 for almost every
for almost every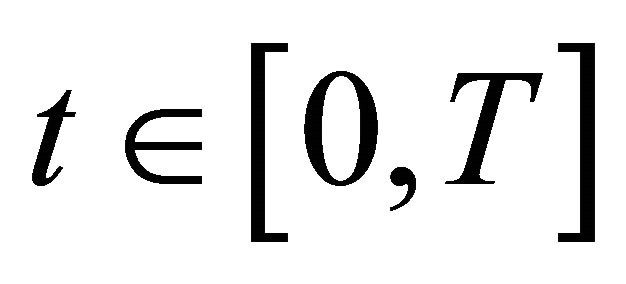 .
.
b)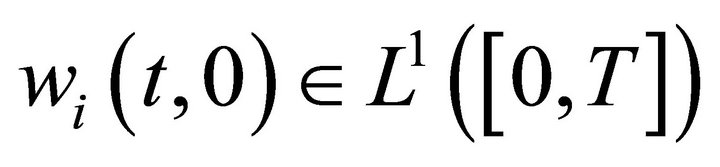 .
.
c) For almost every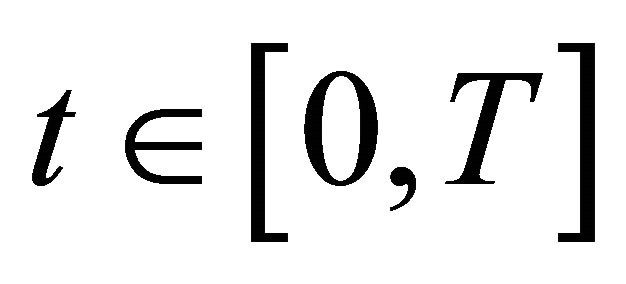 ,
, 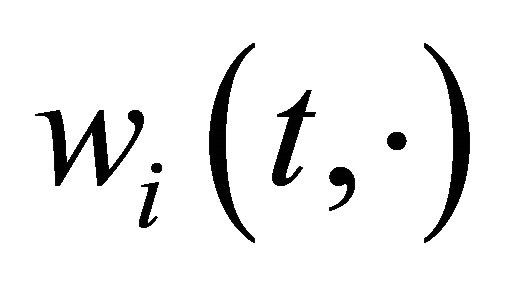 is convex with respect to
is convex with respect to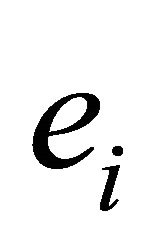 .
.
d)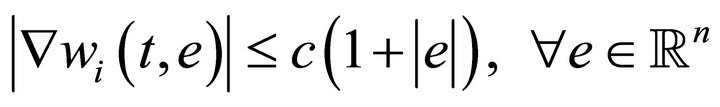 .
.
Consider now, for all i, the functionals defined on X:
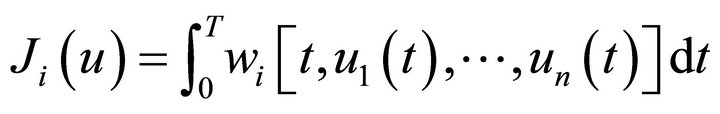 (10)
(10)
We can prove the following theorem.
Theorem 4. Assume that the parametric welfare functions 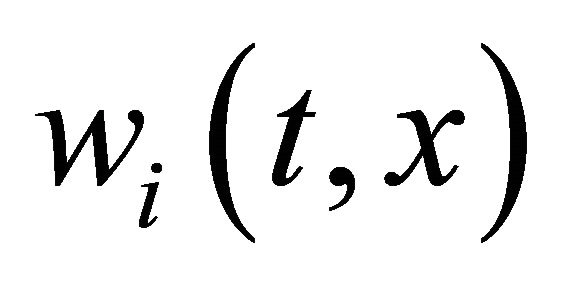 satisfiy the assumptions
satisfiy the assumptions 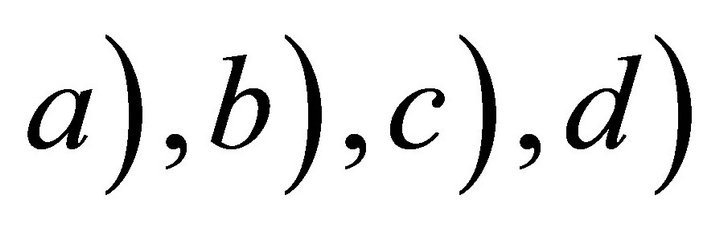 for all i. Then, the functional
for all i. Then, the functional 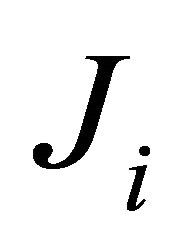 is well defined in X for each i, and
is well defined in X for each i, and 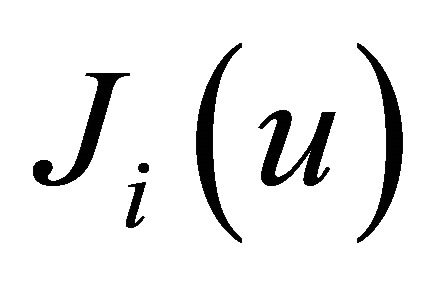 is concave with respect to the variable
is concave with respect to the variable 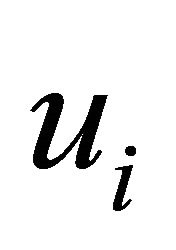 and Gateaux differentiable in
and Gateaux differentiable in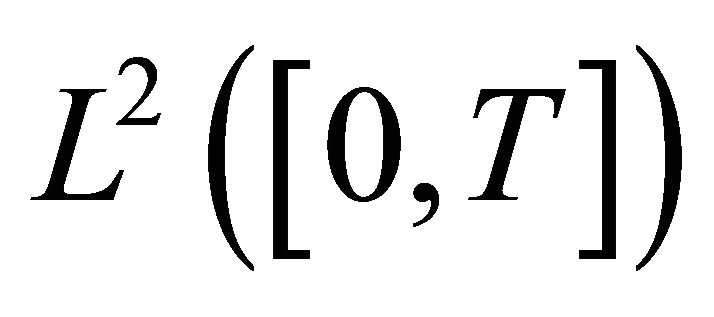 , with respect to
, with respect to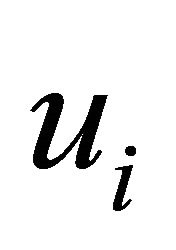 . Moreover, its Gateaux derivative is given by:
. Moreover, its Gateaux derivative is given by:
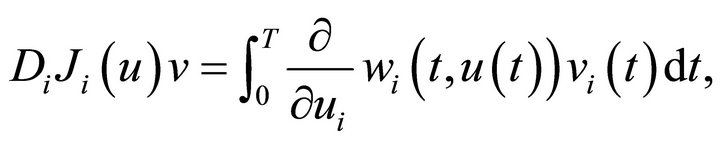
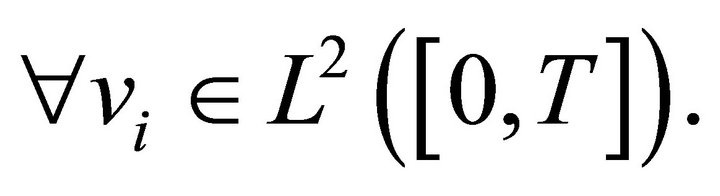
Proof. Notice first that 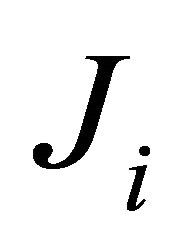 is well defined due to assumption d). Indeed, for each
is well defined due to assumption d). Indeed, for each , there exists
, there exists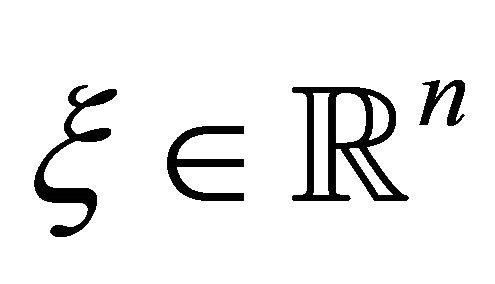 ,
, 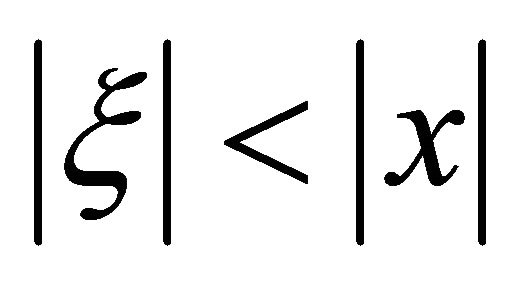 , such that:
, such that:
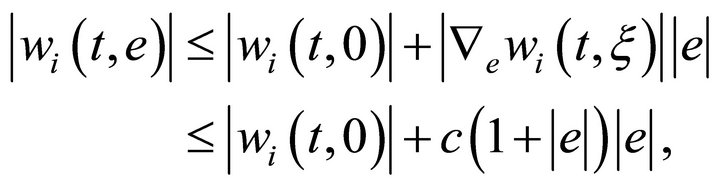
hence, 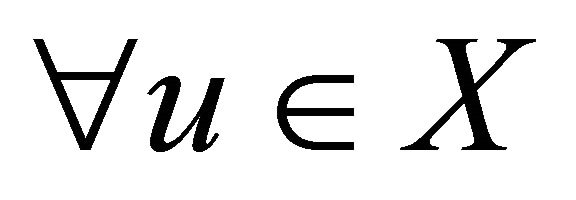 we get
we get
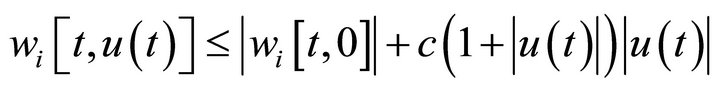
a. e. 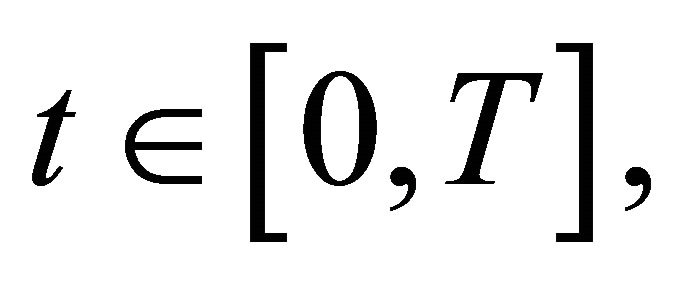 which implies that
which implies that
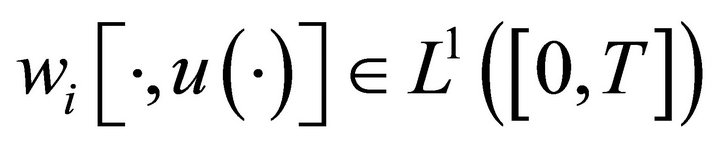 .
.
The concavity of 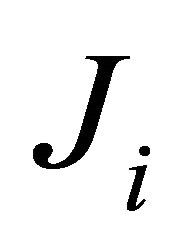 with respect to the variable
with respect to the variable 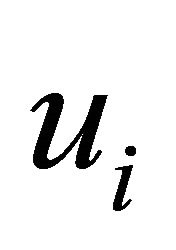 is an immediate consequence of assumption c). Now we prove that
is an immediate consequence of assumption c). Now we prove that 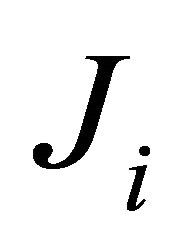 is Gateaux differentiable in X, with respect to
is Gateaux differentiable in X, with respect to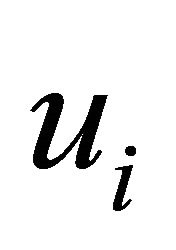 , for every
, for every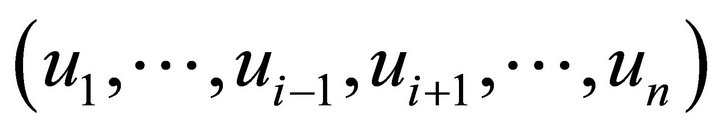 . To simplify the notation we write
. To simplify the notation we write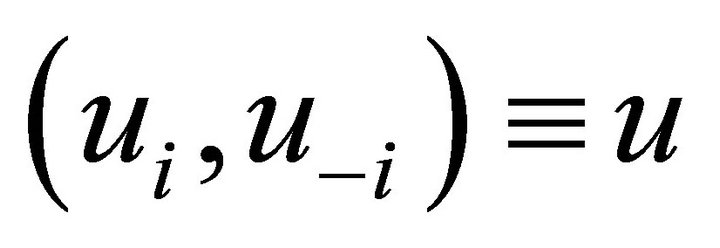 , where
, where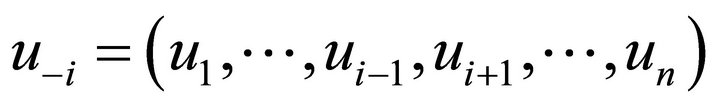 . Hence, fix
. Hence, fix 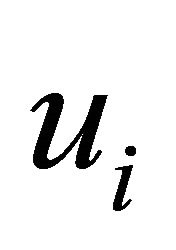 and some direction
and some direction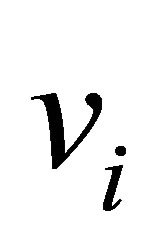 , and for
, and for 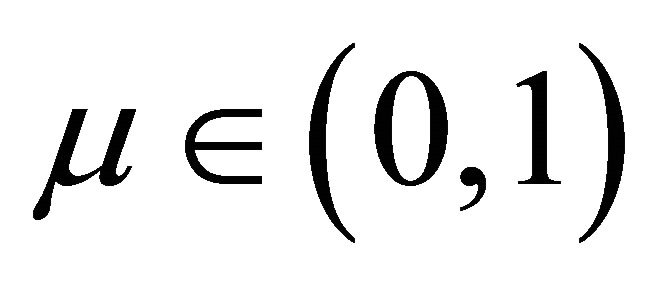 consider the incremental quotient:
consider the incremental quotient:
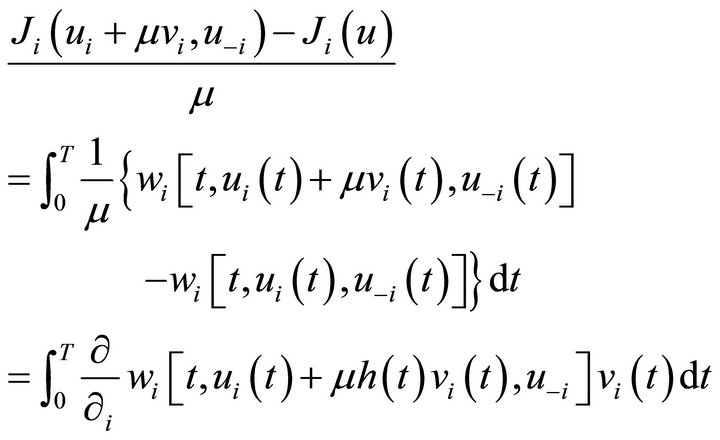
where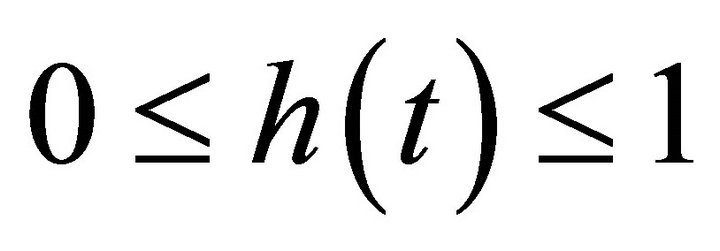 , a.e.
, a.e. . Now, we have that for a.e.
. Now, we have that for a.e. :
:
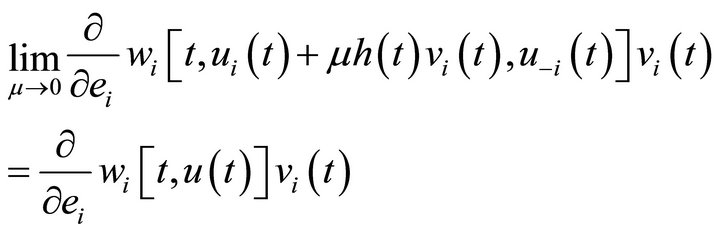
Moreover due to the inequality:
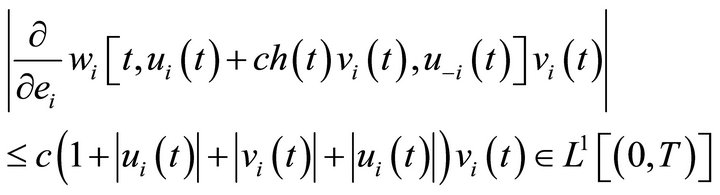
we obtain
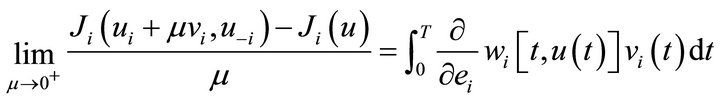
by applying Lebesgue convergence theorem.
In this framework, the Nash equilibrium problem is to find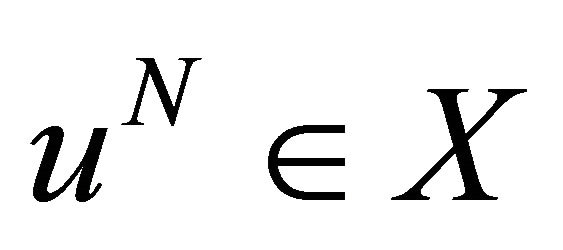 :
:
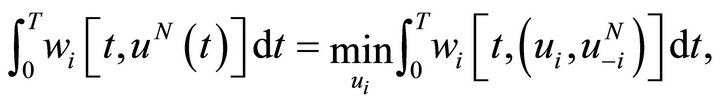
where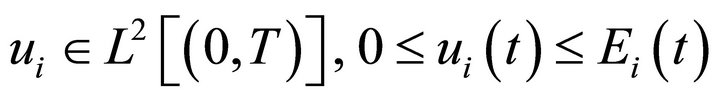 , with
, with
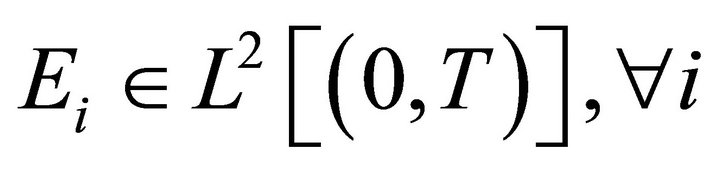 .
.
Rosen Equilibrium problem is to find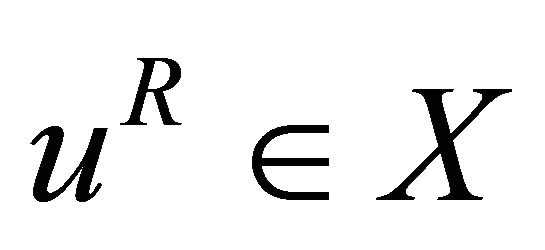 :
:
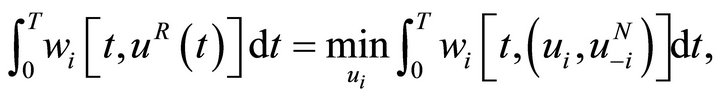
where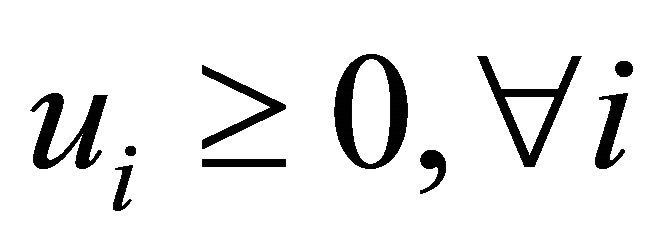 , and
, and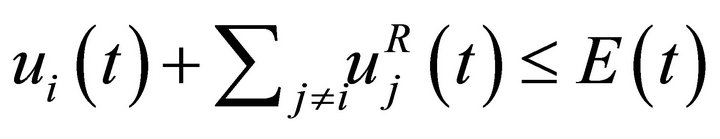 .
.
Once we have formulated Nash and Rosen equilibrium problems in the Lebesgue space we are in position to write the corresponding two variational inequalities:
Find 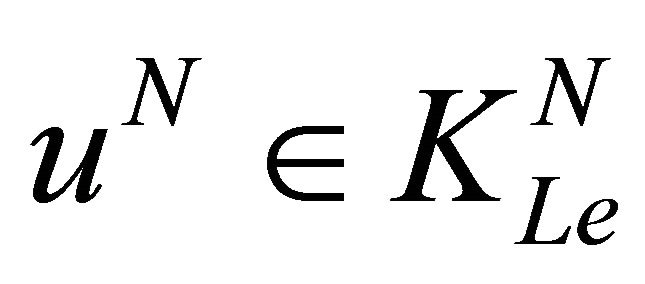 such that
such that
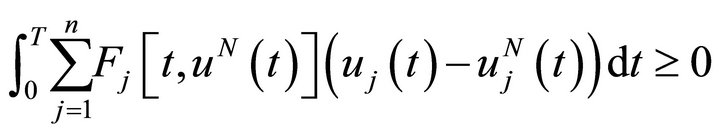 (11)
(11)
for all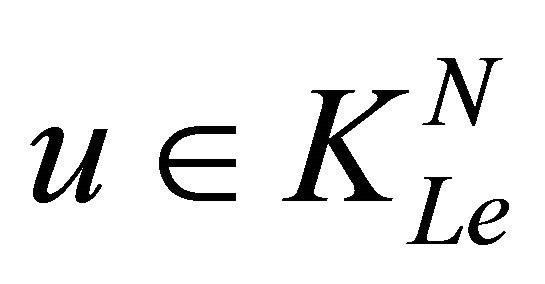 , where
, where
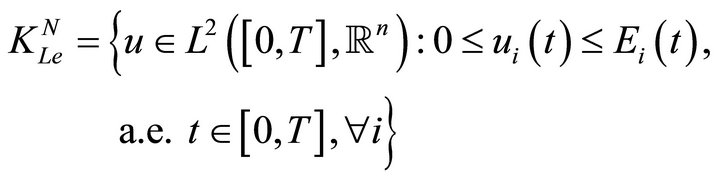
Find 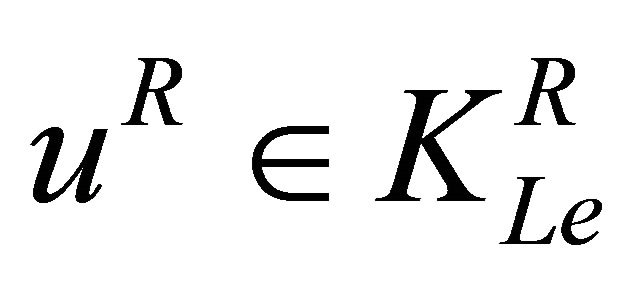 such that
such that
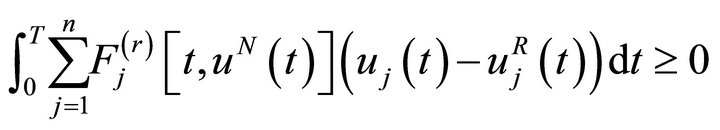 (12)
(12)
for all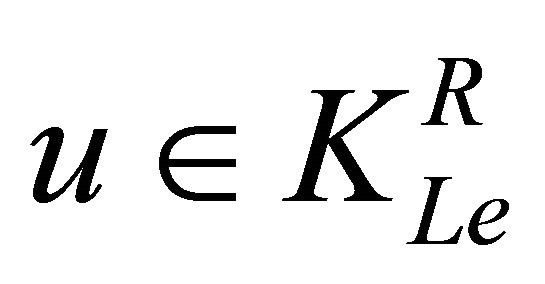 , where
, where
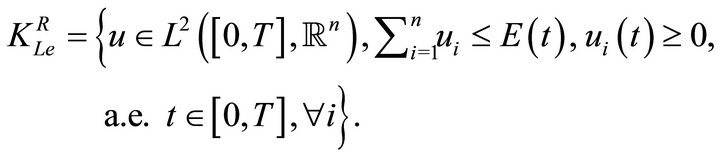
5. Conclusion
In this short note we showed how some environmental models recently proposed can be formulated via the variational inequality theory. Moreover, we extended the previous models admitting the possibility that both the operator and the constraints sets depend on a parameter. The variational inequality approach permits the application of some recent geometric-analytic methods ([6,7]) to study the sensitivity of the solution with respect to perturbations of the parameter. At last, we introduced the Lebesgue-space formulation of the problems under study, which, in the last decade, has been very fruitful to study time-dependent and random equilibrium problems (see e.g. [9,11,16] for the approximate computation of statistical quantities related to the solution). In this respect, Inequalities (11) and (12) (and their generalization to probability spaces) are the starting point for a systematic study of environmental problems which we are planning to carry out in the future.
REFERENCES
- M. Breton, G. Zaccour and M. Zahaf, “A Game-Theoretic Formulation of Joint Implementation of Environmental Projects,” European Journal of Operational Research, Vol. 168, No. 1, 2005, pp. 221-239. doi:10.1016/j.ejor.2004.04.026
- M. Tidball and G. Zaccour, “An Environmental Game with Coupling Constraints,” Environmental Modeling and Assessment, Vol. 10, No. 2, 2005, pp. 153-158. doi:10.1007/s10666-005-5254-8
- F. Giannessi and A. Maugeri, Eds., “Variational Inequalities and Network Equilibrium Problems,” Plenum Press, New York, 1995.
- F. Raciti, “Equilibrium Conditions and Vector Variational Inequalities: A Complex Relation,” Journal of Global Optimization, Vol. 40, No. 1-3, 2008, pp. 353-360. doi:10.1007/s10898-007-9202-9
- J. Gwinner and F. Raciti, “Random Equilibrium Problems on Networks,” Mathematical Computer Modelling, Vol. 43, No. 7-8, 2006, pp. 880-891. doi:10.1016/j.mcm.2005.12.007
- A. O. Caruso, A. A. Khan and F. Raciti, “Continuity Results for Some Classes of Variational Inequalities and Applications to Time-Dependent Equilibrium Problems,” Numerical Functional Analysis and Optimization, Vol. 30 No. 11-12, 2009, pp. 1272-1288. doi:10.1080/01630560903381696
- A. Causa and F. Raciti, “Lipschitz Continuity Results for a Class of Variational Inequalities: A Geometric Approach,” Journal of Optimization Theory and Applications, Vol. 145, No. 2, 2010, pp. 235-248. doi:10.1007/s10957-009-9622-4
- A. Causa and F. Raciti, “Some Remarks on the Walras Equilibrium Problem in Lebesgue Spaces,” Optimization Letters, Vol. 5, No. 1, 2011, pp. 99-112. doi:10.1007/s11590-010-0193-y
- J. Gwinner and F. Raciti, “On a Class of Random Variational Inequalities on Random Sets,” Numerical Functional Analysis and Optimization, Vol. 27, No. 5-6, 2006, pp. 619-636.
- J. Gwinner and F. Raciti, “On Monotone Variational Inequalities with Random Data,” Journal of Mathematical Inequalities, Vol. 3, No. 3, 2009, pp. 443-453.
- J. Gwinner and F. Raciti, “Some Equilibrium Problems under Uncertainty and Random Variational Inequalities, Annals of Operations Research, Vol. 200, No. 1, 2012, pp. 299-319. doi:10.1007/s10479-012-1109-2
- P. T. Harker, “Generalized Nash Games and Quasi-Variational Inequalities,” European Journal of Operational Research, Vol. 54, No. 1, 1991, pp. 81-94. doi:10.1016/0377-2217(91)90325-P
- A. Maugeri and F. Raciti, “On Existence Theorems for Monotone and Nonmonotone Variational Inequalities,” Journal of Convex Analysis, Vol. 16, No. 3-4, 2009, pp. 899-911.
- J. B. Rosen, “Existence and Uniqueness of Equilibrium Points for Concave N-Person Games,” Econometrica, Vol. 33, No. 3, 1965, pp. 520-534. doi:10.2307/1911749
- F. Facchinei, A. Fischer and V. Piccialli, “On Generalized Nash Games and Variational Inequalities,” Operations Research Letters, Vol. 35, No. 2, 2007, pp. 159-164. doi:10.1016/j.orl.2006.03.004
- P. Falsaperla, F. Raciti and L. Scrimali, “A Variational Inequality Model of the Spatial Price Network Problem with Uncertain Data,” Optimization and Engineering, Vol. 13, No. 3, 2012, pp. 417-434. doi:10.1007/s11081-011-9158-y

