Journal of Minerals & Materials Characterization & Engineering, Vol. 10, No.14, pp.1307-1327, 2011
jmmce.org Printed in the USA. All rights reserved
1307
Study of Potentiodynamic Polarization Behaviour of Electroless Ni-B
Coatings and Optimization using Taguchi Method
and Grey Relational Analysis
Suman Kalyan Das and Prasanta Sahoo*
Department of Mechanical Engineering, Jadavpur University, Kolkata 700032, India
*Corresponding author: [e-mail], [e-mail] )
ABSTRACT
Electroless nickel coatings are very popular for their corrosion resistant actions. The present
article attempts to study the corrosion behaviour of electroless Ni-B coatings by varying the
coating parameters viz. bath temperature, reducing agent concentration and nickel source
concentration together with the annealing temperature. The electrochemical parameters viz.,
corrosion potential and corrosion current density are evaluated with the help of
potentiodynamic polarization experimentation. Taguchi based Grey analysis is employed in
order to optimize this multiple response problem and the optimal combination of parameters
for maximum corrosion resistance for Ni-B coatings is presented. Moreover, analysis of
variance reveals that bath temperature and concentration of nickel source have significant
influence on the corrosion performance of the coating. The microstructure characterization
of the coating is also conducted with the help of scanning electron microscopy, energy
dispersive X-ray analysis and X-ray diffraction analysis. The Ni-B coating in general exhibits
a nodular structure and turns crystalline with heat treatment. The corroded surface exhibits
cracks and black spots which imply the occurrence of localized corrosion.
Keywords: Electroless coatings, Ni-B, Corrosion, Grey Taguchi.
1. INTRODUCTION
Electroless nickel coatings have received wide acceptance by the industrialists as well as the
research community due to their ability to provide hardness, wear resistance, corrosion
resistance and low friction coefficient [1, 2]. Moreover, the coating could be engineered to
suit the need for a particular application. The properties of electroless nickel coatings are
greatly affected by the type of reducing agent present in the bath. Hypophosphite reduced
(Ni-P) electroless nickel coatings have already proved their mettle as a coating for
tribological based applications [3-5] and attention has shifted towards borohydride reduced
(Ni-B) coatings [5-12] as the latter can provide improved properties. Electroless Ni-B
coatings are widely used in aerospace and automotive industries particularly due to their high
hardness and hence splendid wear resistance [1]. Ni-B coatings are found to be harder than
Ni-P coatings in as deposited phase [6]. With heat treatment, the hardness of Ni-B coating is
found to increase even more [6, 7]. The increase of hardness of Ni-B coating with heat
treatment is generally attributed to the modification of deposit structure allowing the

1308 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
precipitation of Ni-B phases according to the Ni-B phase diagram [8]. With hardness, comes
the ability to withstand wear and tear and Ni-B acquires high wear resistance particularly
after heat treatment [6, 9].
Corrosion is a deteriorating phenomenon of materials, particularly metals, which often
dictates the life of a product. By careful monitoring and devising newer methods to inhibit
corrosion, device life could be improved preventing loss to the society. Applying coatings has
been a popular way to make metals resistant to corrosion and electroless nickel coatings have
proved to be suitable coatings in this regard. Several electrochemical studies have been
conducted to evaluate the corrosion behaviour of electroless nickel coatings. Previous
electrochemical studies used to quantify corrosion by measuring the loss of weight suffered
by a material exposed to the corrosive environment. This is one of the easiest methods of
evaluating the corrosion performance without the use of any sophisticated instrumentation
and using the least of the resources. But with the development of technology, and
sophisticated instruments being available, more precise investigations of the corrosion
behaviour of a material is now possible. Present generation studies of the corrosion behaviour
of electroless nickel coating are mainly conducted through electrochemical tests viz.
potentiodynamic polarization studies and electrochemical impedance spectroscopy. The
resistance of the coatings towards corrosion is evaluated on the basis of the corrosion
parameters obtained from these studies viz. corrosion potential, corrosion current density,
charge transfer resistance, double layer capacitance, corrosion rate, etc [9-11]. Although Ni-P
coatings are reported to have a better corrosion resistance than Ni-B [6, 12], the properties of
Ni-B are not bad, which is reported to prevent the contact of Ni-P under layer with the
electrolytic solution in Ni-P/Ni-B coating [7]. The difference in corrosion resistance between
electroless Ni-P and Ni-B coatings is mainly due to the difference in their structure. It is
believed that the passivation films that form on Ni-B coated surfaces are not as glassy or
protective enough as those that form on high phosphorous electroless nickel coatings. The
phase boundaries present in Ni-B deposits might also be responsible for causing discontinuity
of the passivation film, which are the preferred sites for the initiation of corrosion process
[10]. Electroless Ni-B coating is applied to increase the corrosion resistance of steel.
Contreras et al [13] have studied the corrosion behaviour of Ni-B coatings applied on
commercial steel in both acidic and neutral environment and found that the coating protects
the steel against corroding in both the environments although being more vulnerable in acidic
environment. Increase in boron content also increases the corrosion resistance of Ni-B
coatings [12]. In general, it is observed that corrosion resistance of as plated electroless Ni-B
deposit is higher than the heat treated deposits [6, 9, 12]. This is assigned to the fact that heat
treatment promotes crystallinity, which again provides grain boundaries that become
favourable sites for attack by the electrolyte. The corrosion resistance of electroless Ni–P and
Ni–B deposits is found to increase with the incorporation of an additional alloying element
such as Cu, Zn, W, Mo, etc. or with the incorporation of second phase particles, such as
silicon nitride, ceria and titania in the metal matrix [6]. Also presence of sodium
hypophosphite in Ni-B bath enhances the corrosion resistance of Ni-B by forming Ni-B-P [9].
Ni-B coating being lesser corrosion resistant than Ni-P coating, an extensive study regarding
the corrosion behavior of the former has remained neglected. But Ni-B coatings are often
preferred in various tribological applications due to their superior hardness and wear
resistance compared to Ni-P coatings. Thus, a systematic study of the electrochemical
behavior of Ni-B coatings is necessary as the coatings in various applications would
definitely encounter corrosion. The present study tries to study the effect of coating
parameters (bath temperature, reducing agent concentration and nickel source concentration)

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1309
and annealing temperature on the corrosion behavior of electroless Ni-B coatings. The
corrosion behavior of the coating is evaluated with the help of potentiodynamic polarization
tests. Taguchi method together with Grey relational analysis is employed to optimize the
process parameters in order to identify the combination of parameters that induce the
maximum corrosion resistant properties in the coating. Analysis of variance is employed to
observe the level of significance of the factors and their interactions. Finally, validation of the
result obtained through the analysis is done with the help of confirmation test. The surface
morphology and composition of Ni-B coatings are studied with the help of scanning electron
microscopy, energy dispersed X-ray analysis and X-ray diffraction analysis.
2. TAGUCHI METHOD
G. Taguchi introduced the Taguchi technique [14-16] and since then it has been widely used
in the engineering domain to get the desired performance characteristics by optimizing the
design parameters. In Taguchi technique, three-stages such as system design, parameter
design, and tolerance design are employed. System design consists of the usage of scientific
and engineering information required for producing a part. Tolerance design is employed to
determine and to analyze tolerances about the optimum combinations suggested by parameter
design. Parameter design is used to obtain the optimum levels of process parameters for
developing the quality characteristics and to determine the product parameter values
depending on the optimum process parameter values. Based on orthogonal arrays, the number
of experiments which may increase the time and cost can be reduced by using Taguchi
technique. Taguchi uses S/N ratio in order to identify the quality characteristics applied for
engineering design problems. The S/N ratio characteristics can be divided on the basis of
three criteria: lower-the-better (LB), higher-the better (HB) and nominal-the best (NB). The
parameter level combination that maximizes the appropriate S/N ratio is the optimal level
setting.
3. GREY RELATIONAL THEORY
Taguchi method is well suited for optimization of single response problems. But for multiple
response problems like in the present case, grey relational analysis is needed in conjunction
with Taguchi method to obtain the optimized condition. The Grey system theory was first
proposed by Deng in 1989 [17]. It is similar to fuzzy technique and is an effective
mathematical tool to deal with system analysis characterized by imprecise and incomplete
information. The theory is based on the degree of information known. If the system
information is unknown, it is called a black system; if the information is fully known, it is
called a white system. And a system with information known partially is called a grey
system. Deng [17] had also proposed grey relational analysis (GRA) in the grey theory that
was proved to be an accurate method for multiple attribute decision making problems. The
GRA method is based on the minimization of maximum distance from the ideal referential
alternative. The aim of GRA is to investigate the factors that affect the system. The method is
based on finding the relationships of both independent and interrelating data series. By
finding the GRA mathematically, the grey relational grade (GRG) can be used to evaluate the
relational level between referential series and each comparative series. Grey relational
analysis begins with the calculation of the grey relational generation in which the set of
experimental results are normalized in between zero and one. Then grey relational
coefficients are calculated from the normalized data to represent the correlation between the
desired and actual experimental data. The next step is to find the grey relational grade by
averaging the grey relational coefficients. The grey relational grade is treated as the overall

1310 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
response of the process instead of the multiple responses of corrosion potential and corrosion
current. Analysis of variance (ANOVA) [18] is performed with the grey relational grade in
order to find which of the parameters significantly affects the process performance. Finally
the optimal levels of process parameters are selected and confirmation test is employed to
verify the optimal combination of the process parameters
4. EXPERIMENTAL METHODS
4.1 Coating Procedure
Blocks (20 mm × 20 mm × 8 mm) of steel (AISI 1040) are used as substrates for the
deposition of electroless Ni-B coating. The blocks are carefully prepared by a sequence of
machining processes viz. shaping, parting and milling. Finally, the blocks are subjected to
surface grinding process so that all the substrates have nearly equal roughness (centre line
average value). It is important to note here that corrosion of Ni-P coatings is found to be
dependent on the smoothness of the coating [19] which again depend on the smoothness of
the substrate. Such behaviour is also suspected in case of Ni-B coatings [13] and hence to
remove the effect of substrate roughness on the final response, all the substrates need to be of
similar roughness.
Before coating the substrates are cleaned of any foreign particles and corrosion products.
Then the samples are cleaned with distilled water. The specimens after thorough cleaning are
given a pickling treatment with dilute (18 %) hydrochloric acid for one minute to remove any
surface layer formed like rust and other oxides. Finally, they are cleaned with distilled water
prior to coating. A large number of trial experiments were performed before deciding on the
bath composition with the ranges of the coating parameters. Three most important parameters
are varied and others are kept constant for coating deposition. The bath for electroless Ni–B
coatings has been prepared by mixing nickel chloride (NiCl
2
), sodium borohydride (NaBH
4
),
ethylenediamine (C
2
H
8
N
2
), sodium hydroxide (NaOH), lead nitrate (Pb(NO
3
)
2
) and distilled
water in appropriate sequence (Table 1). The pH of the bath was maintained around 12.5 by
adding required quantity of sodium hydroxide. The cleaned substrates are at first activated in
palladium chloride solution maintained at temperature of 55°C and then placed in the
electroless bath for a deposition time of two hours. The coating thickness is found to lie
around 30 microns as evident from the micrograph of the cross section of the coating (Fig. 1).
After deposition, the coated samples are taken out of the bath and cleaned using distilled
water. Then the samples are subjected to annealing at various temperatures (250°C, 350°C,
450°C) according to the OA, in a box furnace. After annealing, the samples are allowed to
cool down to the room temperature naturally.
Table 1: Bath constituents and deposition conditions
Parameters Ranges of parameters
Nickel chloride 15 – 25 g/l
Sodium borohydride 0.6 – 1.0 g/l
Ethylenediamine 59 g/l
Lead nitrate 0.0145 g/l
Sodium hydroxide 40 g/l
Bath temperature 85 – 95°C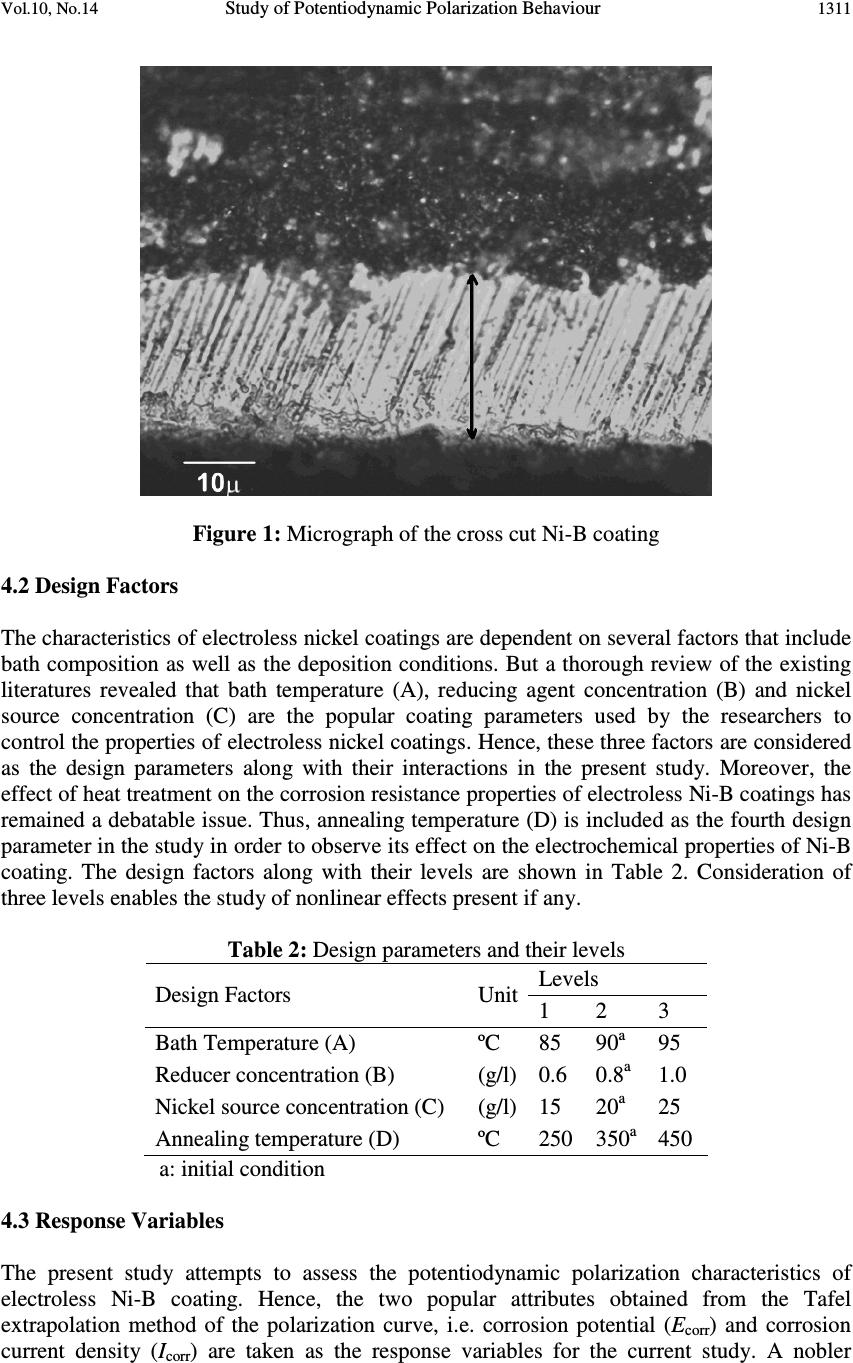

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1311
Figure 1: Micrograph of the cross cut Ni-B coating
4.2 Design Factors
The characteristics of electroless nickel coatings are dependent on several factors that include
bath composition as well as the deposition conditions. But a thorough review of the existing
literatures revealed that bath temperature (A), reducing agent concentration (B) and nickel
source concentration (C) are the popular coating parameters used by the researchers to
control the properties of electroless nickel coatings. Hence, these three factors are considered
as the design parameters along with their interactions in the present study. Moreover, the
effect of heat treatment on the corrosion resistance properties of electroless Ni-B coatings has
remained a debatable issue. Thus, annealing temperature (D) is included as the fourth design
parameter in the study in order to observe its effect on the electrochemical properties of Ni-B
coating. The design factors along with their levels are shown in Table 2. Consideration of
three levels enables the study of nonlinear effects present if any.
Table 2: Design parameters and their levels
Levels
Design Factors Unit
1 2 3
Bath Temperature (A) ºC 85 90
a
95
Reducer concentration (B) (g/l) 0.6 0.8
a
1.0
Nickel source concentration (C) (g/l) 15 20
a
25
Annealing temperature (D) ºC 250 350
a
450
a: initial condition
4.3 Response Variables
The present study attempts to assess the potentiodynamic polarization characteristics of
electroless Ni-B coating. Hence, the two popular attributes obtained from the Tafel
extrapolation method of the polarization curve, i.e. corrosion potential (E
corr
) and corrosion
current density (I
corr
) are taken as the response variables for the current study. A nobler

1312 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
(positive) E
corr
value and a lower I
corr
value indicate that a particular material has higher
corrosion resistance.
4.4 Design of Experiments
An experiment is a process that results in the collection of data. Usually, statistical
experiments are conducted in which researchers can manipulate the conditions of the
experiment and can control the factors that are irrelevant to the research objectives. Planning
an experiment properly is very important in order to ensure that the right type of data and a
sufficient sample size and power are available to answer the research questions of interest as
clearly and efficiently as possible. As mentioned earlier, Taguchi method uses an OA
(orthogonal array) to reduce the number of experiments for determining the optimal process
parameters. Orthogonal arrays allow one to compute the main and interaction effects via a
minimum number of experimental trials [15]. The choice of a suitable OA depends on the
number of design factors and their interactions considered. In the present case, an L
27
OA
which has 27 rows corresponding to the number of tests and 26 degrees of freedom (DOFs)
with 13 columns at three levels is chosen. The factors and their interactions are assigned to
the columns of the array according to the Triangular Table for 3-level OA [16]. The OA
together with the column assignments are shown in Table 3. Values in each cell of the main
parameter columns (A, B, C and D) in the array indicate their levels (1, 2 and 3). Again in
case of interactions, two columns are assigned to a single interaction and the two cell values
in a particular row indicate the levels of each of the factors involved in the interaction. The
unassigned columns in the OA are kept for the errors terms.
4.5 Potentiodynamic Tests
The potentiodynamic polarization tests are performed with a potentiostat (Gill AC) of ACM
Instruments, UK. A 3.5% sodium chloride solution is taken as the electrolyte and the tests are
conducted at an ambient temperature of about 25°C. The electrochemical cell consists of
three electrodes. The coated specimen forms the working electrode which is actually the
sample being interrogated. A saturated calomel electrode (SCE) forms the reference electrode
which provides a stable “reference” against which the applied potential may be accurately
measured. A platinum electrode serves as the counter electrode which provides the path for
the applied current into the solution. The design of the cell is such that only an area of 1 cm
2
of the coated surface is exposed to the electrolyte. A settling time of 15 min is assigned
before every experiment in order to stabilize the open circuit potential (OCP). The
potentiostat is controlled via a PC which also captures the polarization data. The polarization
plot is obtained from the dedicated software, which also possesses a special tool in order to
manually extrapolate the values of E
corr
(corrosion potential) and I
corr
(corrosion current
density) from the plot. As a fully developed linear portion was difficult to find, for an
accurate extrapolation, two thumb rules [20] are followed:
a) One of the branches of the polarization curve should exhibit Tafel (i.e. linear on semi-
logarithmic scale) over at least one decade of current density
.
b) The extrapolation should start at least 50-100 mV away from E
corr
.

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1313
4.6 Microstructure Characterization
Microstructure characterization becomes indispensable in a study involving corrosion which
largely depends on the microstructure of the material. Scanning electron microscopy (JEOL,
JSM-6360 and FEI, Quanta 200) is used to observe the surface morphology of the coating
before and after heat treatment. This is done in order to analyze the effect of heat treatment
on the Ni-B coatings. Energy dispersive X-ray analysis (EDAX Corporation) is made use of
in order to determine the composition of the coating in terms of the weight percentages of
nickel and boron. It has been demonstrated by previous studies [21] that the physical
properties of the deposited film are greatly influenced by the concentration of boron in the
film. This concentration in turn depends upon the amount of reducing agent added. Hence
EDX analysis is done on the coatings developed from the bath consisting of different
concentrations of sodium borohydride (reducing agent) in order to capture the range of boron
content in the coatings. The different precipitated phases before and after heat treatment are
detected by using X-ray diffraction analyzer (Rigaku, Ultima III).
Table 3: L
27
Orthogonal Array with design factors and interactions
Column numbers
Trial
No.
1
A
2
B
3
A×B
4
A×B
5
C
6
A×C
7
A×C
8
B×C
9
D
10
-
11
B×C
12
-
13
-
1
1
1
1
1
1
1
1
1
1
1
1
1
1
2 1 1 1 1 2 2 2 2 2 2 2 2 2
3 1 1 1 1 3 3 3 3 3 3 3 3 3
4 1 2 2 2 1 1 1 2 2 2 3 3 3
5 1 2 2 2 2 2 2 3 3 3 1 1 1
6 1 2 2 2 3 3 3 1 1 1 2 2 2
7 1 3 3 3 1 1 1 3 3 3 2 2 2
8 1 3 3 3 2 2 2 1 1 1 3 3 3
9 1 3 3 3 3 3 3 2 2 2 1 1 1
10 2 1 2 3 1 2 3 1 2 3 1 2 3
11 2 1 2 3 2 3 1 2 3 1 2 3 1
12 2 1 2 3 3 1 2 3 1 2 3 1 2
13 2 2 3 1 1 2 3 2 3 1 3 1 2
14 2 2 3 1 2 3 1 3 1 2 1 2 3
15 2 2 3 1 3 1 2 1 2 3 2 3 1
16 2 3 1 2 1 2 3 3 1 2 2 3 1
17 2 3 1 2 2 3 1 1 2 3 3 1 2
18 2 3 1 2 3 1 2 2 3 1 1 2 3
19 3 1 3 2 1 3 2 1 3 2 1 3 2
20 3 1 3 2 2 1 3 2 1 3 2 1 3
21 3 1 3 2 3 2 1 3 2 1 3 2 1
22 3 2 1 3 1 3 2 2 1 3 3 2 1
23 3 2 1 3 2 1 3 3 2 1 1 3 2
24 3 2 1 3 3 2 1 1 3 2 2 1 3
25 3 3 2 1 1 3 2 3 2 1 2 1 3
26 3 3 2 1 2 1 3 1 3 2 3 2 1
27 3 3 2 1 3 2 1 2 1 3 1 3 2

1314 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
5. RESULTS AND DISCUSSION
5.1 Grey Analysis
The responses (E
corr
and I
corr
) obtained from the potentiodynamic tests are given in Table 4.
For conversion of the multiple responses into a single response (grey relational grade) to be
handled by Taguchi technique requires the following set of calculations:
5.1.1 Grey relational generation
Grey relational generation involves the linear normalization of the experimental results (E
corr
and I
corr
) in the range between 0 and 1. The normalization can be done based on three
objectives which include (1) normalization by maximum value (lower-the-better), (2)
normalization by minimum value (higher-the-better) and (3) normalization by objective
value. The objective of the present study is to maximize the corrosion resistance of Ni-B
coatings. Now, from Table 4, it is seen that E
corr
is always negative. As a nobler E
corr
value
indicates that the material will have lesser tendency to corrode, the normalization is carried
out for E
corr
with higher-the-better criterion. Moreover, since a lower value of corrosion
current density indicates higher corrosion resistance, the normalization for I
corr
is carried out
with lower-the-better criterion. The normalization expressions for both are given as follows:
( )
)(min)(max
)(min)(
kyky
kyky
kx
ii
ii
i
−
−
= ; (higher-the-better) (1)
( )
)(min)(max
)()(max
kyky
kyky
kx
ii
ii
i
−
−
= ; (lower-the better) (2)
where
(
)
kx
i
is the value after grey relational generation while )(min ky
i
and )(max ky
i
are
respectively the smallest and largest values of )(ky
i
for the kth response; k being 1 (E
corr
) and
2 (I
corr
). The processed data after grey relational generation is given in Table 5. Larger
normalized results correspond to the better performance and the best normalized result should
be equal to 1.
5.1.2 Grey relational coefficient
Grey relational coefficients are calculated to express the relationship between the ideal (best
= 1) and the actual experimental results. The Grey relational coefficient
(
)
k
i
ξ
can be
calculated as:
( )( )
max0
maxmin
∆∆
∆
∆
ξ
rk
r
k
i
i
+
+
= (3)
where
=
i0
∆
||
(
)
(
)
kxkx
i
−
0
|| = difference of the absolute value between
(
)
kx
0
and
(
)
kx
i
,
min
∆
and
max
∆
are respectively the minimum and maximum values of the absolute
differences (
i0
∆
) of all comparing sequences and r is the distinguishing coefficient which is
used to adjust the difference of the relational coefficient, usually r
∈
[0,1] [17]. The

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1315
distinguishing coefficient weakens the effect of
max
∆
when it gets too big, enlarging the
different significance of the relational coefficient. The suggested value of the distinguishing
coefficient, r, is 0.5, due to the moderate distinguishing effects and good stability of
outcomes. Therefore, r is adopted as 0.5 for further analysis in the present case. The values of
i0
∆
and grey relational coefficients (with
Ψ
=0.5) are given in Table 5.
Table 4: Experimental results for corrosion potential and corrosion current density
Sl.
No.
E
corr
(mV vs.
SCE)
I
corr
(µA/cm
2
)
Sl.
No.
E
corr
(mV vs.
SCE)
I
corr
(µA/cm
2
)
1 -457.73 4.76 15 -340.54 1.08
2 -640.37 5.93 16 -319.15 3.06
3 -463.75 4.04 17 -309.87 1.60
4 -364.37 4.16 18 -315.07 1.01
5 -325.52 2.29 19 -331.66 1.36
6 -346.23 5.01 20 -342.32 1.53
7 -426.65 8.62 21 -303.64 2.33
8 -397.82 2.87 22 -328.41 1.56
9 -350.64 1.92 23 -306.05 0.64
10 -311.20 1.13 24 -275.31 0.11
11 -219.73 0.19 25 -377.45 1.79
12 -329.33 0.84 26 -290.71 0.89
13 -364.83 3.40 27 -348.52 0.76
14 -351.00 1.78
5.1.3 Generation of Grey relational grade
In the grey relational analysis, the grey relational grade is used to show the relationship
among the series. The overall multiple response characteristics evaluation is based on grey
relational grade which is calculated as follows:
( )
∑
=
=
n
k
ii
k
n
1
1
ξα (4)
where n = number of performance characteristics (2 in present case). The results of grey
relational grade are given in Table 6. Higher the grey relational grade, the closer is the
experimental value to the ideal normalized value. Thus, higher grey relational grade indicates
that the corresponding parameter combination is closer to the optimal.
5.1.4 Grey relational ordering
In relational analysis, the practical meaning of the numerical values of grey relational grades
between elements is not absolutely important, while the grey relational ordering between
them yields more subtle information. The combination yielding the highest grey relational
grade is assigned an order of 1 while the combination yielding the minimum grade is
assigned the lowest order. The ordering of the present grey grades is shown in Table 6.

1316 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
5.2 Analysis of Signal to Noise Ratio
Taguchi method uses S/N ratio to convert the experimental results into a value for the
evaluation characteristic in the optimum parameter analysis. In the present work, S/N ratio
analysis is done with grey relational grade as the performance index. As grey relational grade
is to be maximized, the S/N ratio is calculated using higher the better criterion and is given
by:
S/N =








−
∑
2
y
1
n
1
log10 (5)
Table 5: Grey relational analyses for corrosion potential and corrosion current density
Normalized data Values of ∆
0i
Grey relational coefficient
Exp.
No. E
corr
I
corr
E
corr
I
corr
E
corr
I
corr
1 0.434196 0.453584 0.565804 0.546416 0.469129 0.477821
2 0 0.316099 1 0.683901 0.333333 0.422333
3 0.419884 0.53819 0.580116 0.46181 0.462913 0.519853
4 0.656143 0.524089 0.343857 0.475911 0.592517 0.512342
5 0.748502 0.743831 0.251498 0.256169 0.665338 0.661228
6 0.699268 0.424207 0.300732 0.575793 0.624428 0.464773
7 0.508083 0 0.491917 1 0.504074 0.333333
8 0.576621 0.675676 0.423379 0.324324 0.54149 0.606557
9 0.688784 0.787309 0.311216 0.212691 0.616358 0.701566
10 0.782546 0.880141 0.217454 0.119859 0.696908 0.806635
11 1 0.990599 0 0.009401 1 0.981546
12 0.739445 0.914219 0.260555 0.085781 0.657414 0.853561
13 0.655049 0.613396 0.344951 0.386604 0.591751 0.56395
14 0.687928 0.80376 0.312072 0.19624 0.615709 0.718143
15 0.712795 0.886016 0.287205 0.113984 0.635158 0.814354
16 0.763646 0.653349 0.236354 0.346651 0.679021 0.590562
17 0.785707 0.824912 0.214293 0.175088 0.699993 0.740644
18 0.773345 0.894242 0.226655 0.105758 0.688085 0.825412
19 0.733905 0.853114 0.266095 0.146886 0.652661 0.772934
20 0.708563 0.833137 0.291437 0.166863 0.631762 0.74978
21 0.800518 0.73913 0.199482 0.26087 0.714815 0.657143
22 0.741632 0.829612 0.258368 0.170388 0.65931 0.745837
23 0.794789 0.93772 0.205211 0.06228 0.709008 0.889237
24 0.867868 1 0.132132 0 0.790974 1
25 0.625048 0.802585 0.374952 0.197415 0.57146 0.716933
26 0.831257 0.908343 0.168743 0.091657 0.747672 0.845084
27 0.693824 0.923619 0.306176 0.076381 0.620212 0.867482
where y is the observed data and n is the number of observations. The S/N ratio is preferred
to the traditional means as the former can capture variability within a trial condition. As the
experimental design is orthogonal, the separation of each coating parameters at different
levels is possible. For example, the mean of grey relational grade for factor A at levels 1, 2
and 3 can be calculated by taking the average of the grey relational grade for the experiments

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1317
1–9, 10–18 and 19–27, respectively. The mean of the grey relational grade for each level of
other coating parameters can be computed in the similar manner. The mean of the relational
grade for each level of the combining parameters is summarized in the multi-response
performance index table (Table 7). In addition, the total mean of the grey relational grade of
the twenty seven experiments is also calculated, as shown in Table 7. The response table also
contains ranks based on the delta values. The delta value is calculated by subtracting the
largest value from the lowest from among the values in each column. Basically, a design
factor with a large difference in the grey relational grade from one factor setting to another
indicates that the factor or design parameter is a significant contributor to the achievement of
the performance characteristic. From the response table it is found that parameter A is the
most significant factor in controlling the polarization characteristics of Ni-B coatings.
Table 6: Grey relational grade and its order
Exp. No.
Grey relational
grade Order Exp. No. Grey relational Order
1 0.4734 25 15 0.7247 9
2 0.3778 27 16 0.6347 19
3 0.4913 24 17 0.7203 10
4 0.5524 22 18 0.7567 5
5 0.6632 16 19 0.7127 11
6 0.5446 23 20 0.6907 13
7 0.4187 26 21 0.6859 14
8 0.5740 21 22 0.7025 12
9 0.6589 17 23 0.7991 3
10 0.7517 7 24 0.8954 2
11 0.9907 1 25 0.6441 18
12 0.7554 6 26 0.7963 4
13 0.5778 20 27 0.7438 8
14 0.6669 15
Table 7: Mean table for Grey relational grade
Level A B C D
1 0.5283 0.6589 0.6076 0.6429
2 0.7310 0.6808 0.6977 0.6573
3 0.7412 0.6609 0.6953 0.7004
Delta 0.2129 0.0219 0.0901 0.0574
Rank 1 4 2 3
Total mean grey relational grade = 0.6668
Fig. 2 shows the main effect plot of grey relational grade. The main effect plot gives the
optimal combination of coating parameters for maximum corrosion resistance. As the larger
the grey relation grade is, the closer will be the product quality to the ideal value. Hence, the
optimal combination of parameters is found to be A3B2C3D3. The main effects plot also
gives a rough idea about the relative significance of the parameters on the system response.
If the plot for a particular parameter has the highest inclination, then that parameter has the
most significance. Whereas the plot which is near horizontal has no significance. From Fig. 2,
it can be observed that parameter A has the most significance while parameter C is also quite

1318 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
significant. In the interaction effect plots (Fig. 3), the non-parallelism of the plots indicates
that some amount of significance exists between the two factors, whereas intersecting lines
are an indication of strong interaction. From Fig. 3, it can be seen that lines intersect in all the
plots. Hence, quite strong interaction is believed to be existent among all the factors as far as
the potentiodynamic polarization characteristics of electroless Ni-B coatings are concerned. It
may be noted that quality of Ni-B deposits are very much dependent on the ratio of
concentrations of nickel and borohydride ions in the bath. An improper balance between the
concentrations of nickel and borohydride can lead to poor and rough deposits [1]. The
optimal levels of nickel source (C3) and reducing agent (B2) obtained from the present study
may be helping in striking a proper balance between the two (nickel and borohydride ions)
for achieving smoother deposits which may be aiding to the corrosion resistance of the
coating. Moreover, bath temperature increases the deposition rate by accelerating the reaction
mechanism. Thus, the surface morphology of the coating is very much dependent on the bath
temperature, which controls the growth of the coating. Now, the present optimal level (A3) of
bath temperature may actually be helping in attaining such a morphology which is suitable
for resistant against corrosion.
Figure 2: Main effects plot for mean S/N ratio
5.3 Analysis of Variance
The analysis of variance (ANOVA) is employed in order to have a quantified idea about the
effect of the design parameters (A, B, C, D) and their interactions (A×B, A×C, B×C) on the
polarization characteristics of electroless Ni-B coating. The Taguchi experimental method
could not judge the effect of individual parameters on the entire process, thus the percentage
of contribution using ANOVA is used to compensate for this effect. ANOVA results for
overall grey relational grade of friction and wear response is obtained through Minitab [22]
and shown in Table 8. The ANOVA table also consists of F-values. By comparing the
evaluated F values with the tabulated ones, the significance of the factors and their
interactions can be readily understood. If the obtained F-value of a parameter or interaction is
greater than the tabulated one, then that particular parameter or interaction has a significant
influence over the process response. From Table 8, it can be observed that parameter A, i.e.
bath temperature has the most significant influence over the polarization characteristics at the
confidence level of 99% while parameter C (concentration of nickel source) is significant
only at a confidence level of 75%. In case of interactions, it is found that only the interaction
A×B is significant and at a confidence level of 90%.

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1319
(a)
(b)
(c)
Figure 3: Interaction effects plot for mean grade (a) A vs B, (b) A vs C and (c) B vs C

1320 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
Table 8: ANOVA table
Source DOF SS MS F %
contribution
A 2 0.259661 0.129831 17.92
b
52.93
B 2 0.002633 0.001316 0.18 0.54
C 2 0.047405 0.023703 3.27
d
9.66
D 2 0.016088 0.008044 1.11 3.28
A×B 4 0.095066 0.023767 3.28
c
19.37
A×C 4 0.006696 0.001674 0.23 1.36
B×C 4 0.019558 0.004889 0.67 3.98
Error 6 0.043463 0.007244
Total 26 0.490571
b
Significant at 99% confidence level (F
0.01,2,6
= 10.9)
c
Significant at 90% confidence level (F
0.10,4,6
= 3.18)
d
Significant at 75% confidence level (F
0.25,2,6
= 1.76)
5.4 Confirmation Test
Once the optimal level combination of the design parameters have been found out, the final
step is to verify if any improvement in the results actually occurs at the optimal condition
compared to the initial condition. Also, an estimated grey relational grade ( γ
ˆ
) is calculated at
the optimal condition with the help of the following expression:
( )
∑
=
−+=
o
i
m
i
m
1
ˆ
γγγγ
(6)
Table 9: Results of confirmation test
Optimal parameter
Initial
parameter Prediction Experimental
Level A2B2C2D2 A3B2C3D3 A3B2C3D3
E
corr
(mV
vs. SCE)
-381.55 -275.31
I
corr
(µA/cm
2
) 5.04 0.11
Grade 0.5142 0.7696 0.8954
Improvement of grey relational grade = 0.3812
where
m
γ
is the total mean grey relational grade,
i
γ is the mean grey relational grade at the
optimal level, and o is the number of the main design parameters that significantly affect the
polarization characteristics of electroless Ni-B coating. The comparison of the predicted grey
relational grade, experimental grey relational grade and the grey relational grade at the initial
condition is shown in Table 9. The mid-level combination of coating parameters is assumed
as the initial condition. From the table, it is found that the improvement of grey relational
grade at the optimal condition is 0.3812 which is about 57% of the mean grey relational
grade. This is considered to be a significant improvement. The polarization curves for the
coatings developed at initial condition and at optimal condition are shown in Fig. 4. As

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1321
expected, the polarization curves showed that Ni-B coatings do not exhibit any passive
behaviour.
Figure 4: Polarization curves for coatings developed at (1) Initial condition, (2) Optimal
condition
5.5 Study of Microstructure
The chemical composition of electroless coatings are analysed using one of the latest EDX
detectors without any Beryllium window, enabling the detection of light elements like boron
with considerable accuracy. The beryllium window if present absorbs all the soft X-rays
emanating from the lighter elements thereby preventing their detection. The EDX plots are
shown in Fig. 5 and boron content in terms of weight percentages is found to be in the range
of 5.72 - 7.46 while the remaining is mostly nickel.
The SEM micrographs of the coating surfaces in as-deposited and heat treated (at 250°C,
350°C and 450°C for one hour) conditions are shown in Fig. 6. The surface exhibits a
cauliflower like structure which strongly points towards the coating possessing a lubricious
behavior [8]. Surface of the Ni-B coatings appears to be dense and matte grey in colour with
low porosity. Also by careful observation, it can be noted that the Ni-B nodules are quite
deflated and flat in as deposited condition but gradually grow in size with increase in heat
treatment temperature giving rise to coarse grained structure.
The XRD analysis (Fig. 7) shows that the Ni-B film is almost amorphous in as-deposited
phase but turns crystalline with heat treatment. This is evident from the presence of
microcrystalline peaks in as-deposited phase whereas broad peaks of Ni, Ni
2
B and Ni
3
B are
found in samples heat treated at 450°C.

1322 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
(a)
(b)
Figure 5: EDX plots of Ni-B coatings (a) 0.6 g/l NaBH
4
, (b) 1.0 g/l NaBH
4
5.6 Corrosion Mechanism
Electroless Ni-B deposits demonstrate a moderate corrosion resistance in 3.5% sodium
chloride solution. Some of the corroded samples are observed under SEM in order to get a
rough idea about the corrosion process (Fig. 8). The effect of heat treatment on corrosion is
attempted to capture by observing samples annealed at different temperatures (250, 350 and
450 ºC). A quick view of the pictures reveals that the samples are quite affected by the
corrosion in saline environment. In almost every sample, localized cracks are found to be

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1323
(a) (b)
(c) (d)
Figure 6: SEM micrographs of the coating surfaces: (a) as-deposited, (b) annealed at 250ºC
(c) annealed at 350ºC and (d) annealed at 450ºC.
present which may be indicative of preferential dissolution at the boundaries of adjacent
grains and columns (23). Also black spots can be observed which are more prominent for
samples annealed at 250ºC (Fig. 8a) and 450ºC (Fig. 8c). These spots imply the occurrence of
localized corrosion on the coating surface due to the presence of chloride ions in the solution.
But since the coating does not display any passive behavior in the polarization curve, the
probability of pitting is quite less. Crobu et al [24] have observed a similar occurrence and
attributed the phenomenon to galvanic coupling due to composition heterogeneities in the
coating. The heterogeneity may be due to the inhomogeneous distribution of boron
throughout the coating providing areas of different corrosion potential on the surface, which
would have lead to the formation of minute active/passive corrosion cells.

1324 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
(a)
(b)
Figure 7: XRD patterns of electroless Ni–B deposit in (a) as-deposited and (b) annealed at
450ºC
6. CONCLUSION
The coating process parameters (bath temperature, reducing agent concentration, nickel
source concentration) together with the annealing temperature are optimized in order to
maximize the charge transfer resistance and minimize the double layer capacitance of
electroless Ni-B coatings. Grey relational analysis is successfully employed in conjunction
with Taguchi design of experiments to optimize this multiple response problem. The optimal

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1325
(a) (b)
(c)
Figure 8: SEM of the corroded coatings annealed at (a) 250ºC, (b) 350ºC and (c) 450ºC
combination of parameters is found to be A3B2C3D3 (highest level of bath temperature,
middle level of reducing agent concentration, highest level of nickel source concentration and
highest level of annealing temperature). Also through ANOVA, it is revealed that bath
temperature and concentration of nickel source has the maximum contribution in controlling
the corrosion behaviour of electroless Ni-B coating. Among the interactions, interaction
between A and B has the maximum contribution towards controlling the corrosion
characteristics of Ni-B coating. The coating surface resembles that of a cauliflower surface
under SEM. The coating also appears to be dense and light grey in colour. The XRD plots
showed that the electroless Ni-B coating is a mixture of amorphous and crystalline phase in
as deposited condition. But with heat treatment, the coating turns crystalline. This is
ascertained by the presence of Ni
2
B and Ni
3
B peaks in the XRD plot of Ni-B coating heat
treated at 450°C. The micrograph of the corroded surface of the coating reveals the presence
of cracks and black spots. The black spots are indicative of localized corrosion and can be
attributed to composition heterogeneity which gives rise to a phenomenon called galvanic
coupling.
ACKNOWLEDGEMENT
The research support provided by CSIR, India: (File No. 9/96(0621)2K10-EMR-I dated
05/03/2010) and partial support from DST-PURSE program is gratefully acknowledged.

1326 Suman Kalyan Das and Prasanta Sahoo Vol.10, No.14
REFERENCES
[1] Mallory, G.O. and Hadju, J.B. (1991), “Electroless Plating: Fundamentals and
Applications”, AESF, Orlando.
[2] Riedel, W. (1991), “Eletroless nickel plating. Stevenage”, Finishing Publications Ltd.,
Hertfordshire, UK.
[3] Agarwala, R.C. and Agarwala, V. (2003), “Electroless alloy/composite coatings: A
review”, Sadhana 28(3-4), pp. 475-493.
[4] Sahoo, P. and Pal, S.K. (2007), “Tribological performance optimization of electroless
Ni-P coatings using the Taguchi method and grey relational analysis”, Tribol Lett 28(2),
pp. 191-201.
[5] Sahoo, P. and Das, S.K. (2011), “Tribology of electroless nickel coatings - A review”,
Mater Des 32, pp. 1760-1775.
[6] Narayanan, T.S.N.S., Krishnaveni, K. and Seshadri, S.K. (2003), “Electroless Ni-P/Ni-
B duplex coatings: Preparation and evaluation of microhardness, wear and corrosion
resistance”, Mater Chem Phys 82(3), pp. 771-779.
[7] Kanta, A.F., Vitry, V. and Delaunois, F. (2009), “Wear and corrosion resistance
behaviours of autocatalytic electroless plating”, J Alloys Compd 486(1-2), pp. L21-23.
[8] Delaunois. F. and Lienard, P. (2002), “Heat treatments for electroless nickel-boron
plating on aluminium alloys”, Surf Coat Technol 160(2-3), pp. 239-248.
[9] Srinivasan, K.N., Meenakshi, R. and Santhi, A., Thangavelu, P.R. and John, S. (2010),
“Studies on development of electroless Ni-B bath for corrosion resistance and wear
resistance applications”, Surf Eng 26(3), pp. 153-158.
[10] Narayanan, T.S.N.S. and Seshadri, S.K. (2004), “Formation and characterization of
borohydride reduced electroless nickel deposits”, J Alloys Compd 365(1-2), pp. 197-
205.
[11] Dervos, C.T., Novakovic, J. and Vassiliou, P. (2004), “Vacuum heat treatment of
electroless Ni-B coatings”, Mater Lett 58(5), pp. 619-623.
[12] Anik, M., Korpe, E. and Sen, E. (2008), “Effect of coating bath composition on the
properties of electroless nickel-boron films”, Surf Coat Technol 202(9), pp. 1718-1727.
[13] Contreras, A., León, C., Jimenez, O., Sosa, E. and Pérez, R. (2006), “Electrochemical
behavior and microstructural characterization of 1026 Ni-B coated steel”, Appl Surf Sci
253(2), pp. 592-599.
[14] Taguchi, G. (1990), “Introduction to Quality Engineering”, Asian Productivity
Organization, Tokyo.
[15] Ross, P.J. (1996), “Taguchi Techniques for Quality Engineering”, 2nd edn., McGraw
Hill, New York.
[16] Roy, R.K. (1990), “A primer on the Taguchi method”, Society of Manufacturing
Engineers, Dearborn, Michigan.
[17] Deng, J. (1989), “Introduction to grey system”, Journal of Grey System 1(1), pp. 1-24.
[18] Montgomery, D.C. (2001), “Design and Analysis of Experiments”, Wiley, New York.
[19] Cheong, W.J., Luan, B.L. and Shoesmith, D.W. (2007), “Protective coating on Mg
AZ91D alloy - The effect of electroless nickel (EN) bath stabilizers on corrosion
behaviour of Ni-P deposit”, Corros Sci 49(4), pp. 1777-1798.
[20] Poorqasemi, E., Abootalebi, O., Peikari, M. and Haqdar, F. (2009), “Investigating
accuracy of the Tafel extrapolation method in HCl solutions”, Corros Sci 51, pp. 1043-
1054.
[21] Watanabe, T. and Tanabe, Y. (1976), “Formation and morphology of Ni-B amorphous
alloy deposited by electroless plating”, Materials Science and Engineering 23, pp. 97-
100.

Vol.10, No.14 Study of Potentiodynamic Polarization Behaviour 1327
[22] Minitab User Manual (2001), “Making data analysis easier”, MINITAB Inc., State
College, PA, USA.
[23] Kanta, A.F., Poelman, M., Vitry, V. and Delaunois, F. (2010), “Nickel-boron
electrochemical properties investigations”, J Alloys Compd 505, pp. 151-156.
[24] Crobu, M., Scorciapino, A., Elsener, B. and Rossi, A. (2008), “The corrosion resistance
of electroless deposited nano-crystalline Ni-P alloys”, Electrochim Acta 53(8), pp.
3364-3370.