Materials Sciences and Applicatio ns, 2010, 1, 59-65
doi:10.4236/msa.2010.12011 Published Online June 2010 (http://www.SciRP.org/journal/msa)
Conductivity Studies in Proton Irradiated
AgI-Ag2O-V2O5-TeO2 Super-Ionic Glass System
Poonam Sharma1, Dinesh Kumar Kanchan1*, Meenakshi Pant1, Karan Pal Singh2
1Department of Physics, Faculty of Science, M. S. University of Baroda, Vadodara, India; 2Department of Physics, Panjab University,
Chandigarh, India.
Received February 26th, 2010; revised May 6th, 2010; accepted May 8th, 2010.
ABSTRACT
The electrical properties of proton ion beam irradiated glass samples are carried out by impedance spectroscopy in the
frequency range from 10 Hz to 32 MHz. The ion beam of energy 3 MeV and fluence of of 1014 particles cm-2 was chosen
for irradiation. The conductivity of the super ionic glass samples increases after irradiation and other electrical
parameters like dielectric constant, dielectric loss and modulus of the proton irradiated glass samples as a function of
glass composition and temperature are observed to change. The dielectric constant and the dielectric losses are increased
after irradiation and the modulus parameters confirm the non-Debye nature for irradiated samples also.
Keywords: Proton-Irradiated Glass, DC Conductivity, Silver-Ion, Scaling, Modulus
1. Introduction
Among the ion-conducting materials, silver-ion conduct-
ing materials have been most widely investigated because
the silver-ion conducting materials usually show high ion
conductivities of more than 10-2 S/cm at room temperature
and have good stability against moisture and oxygen in air
[1-3]. Generally, in polymers, effects of ion beam irra-
diation at low and high dose have attracted much interest
and have been investigated. It has been reported, in lit-
erature, that the physical properties of polymeric films are
modified together with their chemical behaviors [4,5]. Ion
irradiation enables redistribution of pre-formed particles
and mixing of insulator-metal layer compounds to get
particulate composites [6-8]. Ionizing radiation like X-ray
or electron beams causes reduction of metal ions con-
tained in glass [9,10]. Ion irradiation causes liberation of
electrons, holes or displacement of lattice atoms or change
in network structure. When glasses are subjected to ion-
izing radiation, some of their physical and chemical
properties can be changed as a function of glass compo-
sitions [11-13]. The radiation effect in glasses may be due
to atomic displacement by momentum and energy transfer
or may be due to ionization or displacement damage. The
relative contribution of the net damage depends on the
type and energy of the radiation as well as on the total
dose [14]. Recent reports also show that the nature of the
defects produced can be modified as a function of irra-
diation temperature [15]. The electron beam irradiation in
Ag doped glass is also reported to create defects in the
matrix being rather effective in forming particles of uni-
form size, shape and arrangement [16]. It is also demon-
strated that proton-irradiation at room temperature in
simplified glass compositions induces an increase of the
glass polymerization and production of molecular oxygen
dissolved in the glasses linked to the migration and seg-
regation of alkaline ions[17-19]. The high-energy proton
irradiations may be used to simulate both ionization and
displacement damage caused by gamma and neutron ir-
radiation of quartz glasses, and so avoid the difficulties
involved with fission reactor irradiations [20]. Pro-
ton-beam irradiation also reported to induce defects which
are similar to hydrogen vacancies created from X-ray
irradiation, giving rise to an optical transition in the color
centers [21]. The silver super ionic materials find appli-
cations in low power devices viz., button type cells.
Nevertheless these cells are used at places of radiation in
different devices hence it is essential to study their con-
ducting behavior of such materials.
In this work, we have reported the results of conduc-
tivity and relaxation measurements performed on proton
ion irradiated AgI-Ag2O-V2O5-TeO2 super ionic glass
system.
2. Experimental
The glass samples of the composition xAgI-(95-x) [Ag2O:
2V2O5]-5TeO2, (40 ≤ x ≤ 65 in steps of 5) were prepared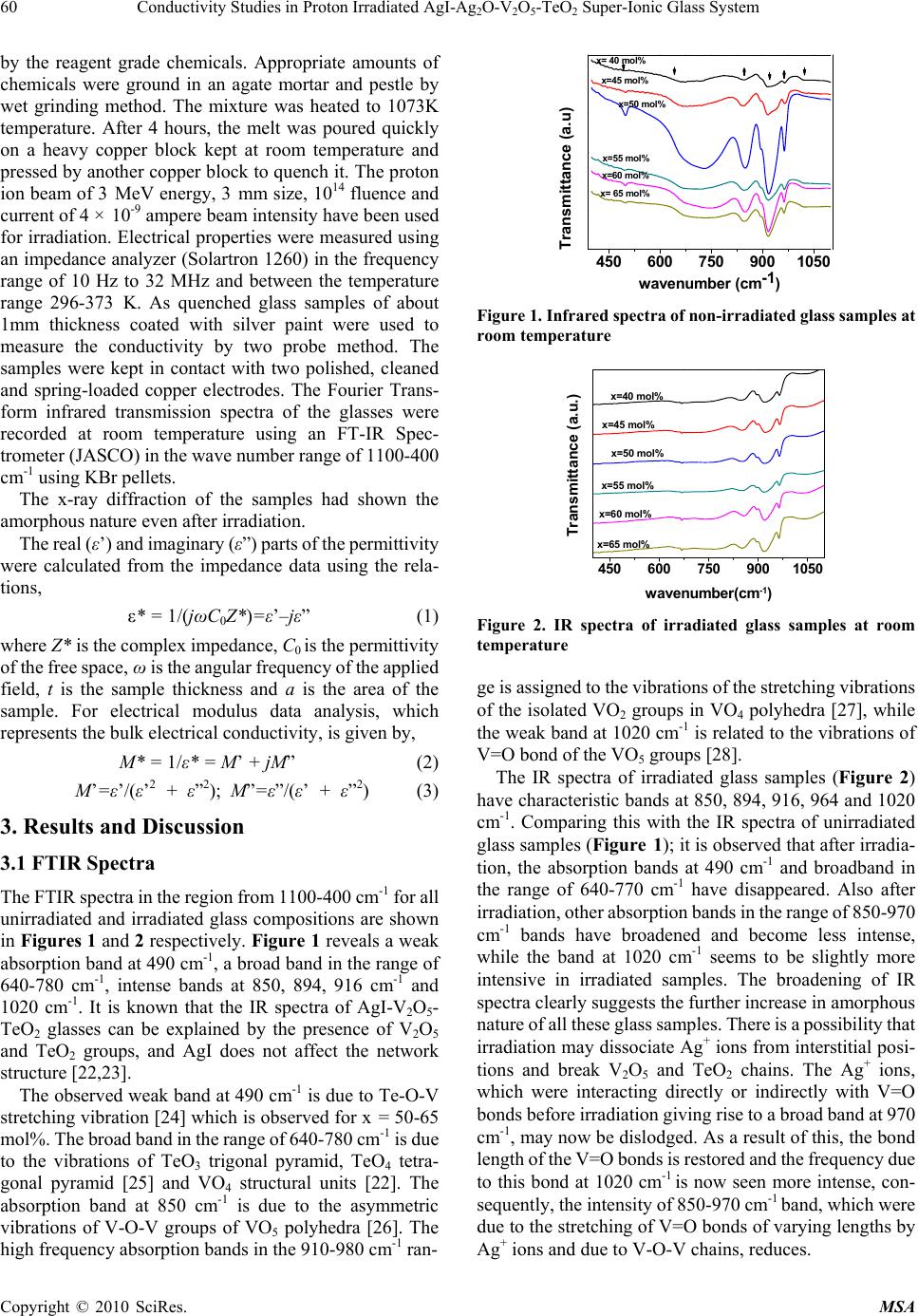

60 Conductivity Studies in Proton Irradiated AgI-AgO-V O-TeO Super-Ionic Glass System
22 52
by the reagent grade chemicals. Appropriate amounts of
chemicals were ground in an agate mortar and pestle by
wet grinding method. The mixture was heated to 1073K
temperature. After 4 hours, the melt was poured quickly
on a heavy copper block kept at room temperature and
pressed by another copper block to quench it. The proton
ion beam of 3 MeV energy, 3 mm size, 1014 fluence and
current of 4 × 10-9 ampere beam intensity have been used
for irradiation. Electrical properties were measured using
an impedance analyzer (Solartron 1260) in the frequency
range of 10 Hz to 32 MHz and between the temperature
range 296-373 K. As quenched glass samples of about
1mm thickness coated with silver paint were used to
measure the conductivity by two probe method. The
samples were kept in contact with two polished, cleaned
and spring-loaded copper electrodes. The Fourier Trans-
form infrared transmission spectra of the glasses were
recorded at room temperature using an FT-IR Spec-
trometer (JASCO) in the wave number range of 1100-400
cm-1 using KBr pellets.
The x-ray diffraction of the samples had shown the
amorphous nature even after irradiation.
The real (ε’) and imaginary (ε”) parts of the permittivity
were calculated from the impedance data using the rela-
tions,
ε* = 1/(jωC0Z*)=ε’–jε” (1)
where Z* is the complex impedance, C0 is the permittivity
of the free space, ω is the angular frequency of the applied
field, t is the sample thickness and a is the area of the
sample. For electrical modulus data analysis, which
represents the bulk electrical conductivity, is given by,
M* = 1/ε* = M’ + jM” (2)
M’=ε’/(ε’2 + ε”2); M”=ε”/(ε’ + ε”2) (3)
3. Results and Discussion
3.1 FTIR Spectra
The FTIR spectra in the region from 1100-400 cm-1 for all
unirradiated and irradiated glass compositions are shown
in Figures 1 and 2 respectively. Figure 1 reveals a weak
absorption band at 490 cm-1, a broad band in the range of
640-780 cm-1, intense bands at 850, 894, 916 cm-1 and
1020 cm-1. It is known that the IR spectra of AgI-V2O5-
TeO2 glasses can be explained by the presence of V2O5
and TeO2 groups, and AgI does not affect the network
structure [22,23].
The observed weak band at 490 cm-1 is due to Te-O-V
stretching vibration [24] which is observed for x = 50-65
mol%. The broad band in the range of 640-780 cm-1 is due
to the vibrations of TeO3 trigonal pyramid, TeO4 tetra-
gonal pyramid [25] and VO4 structural units [22]. The
absorption band at 850 cm-1 is due to the asymmetric
vibrations of V-O-V groups of VO5 polyhedra [26]. The
high frequency absorption bands in the 910-980 cm-1 ran-
450 600 750 9001050
wavenum ber (cm-1)
x= 40 mol%
x=45 mol%
x=50 mol%
x=55 mol%
x=60 mol%
x= 65 mol%
Transmitta nce (a.u)
Figure 1. Infrared spectra of non-irradiated glass samples at
room temperature
450 600 750 9001050
x=65 mol%
x=60 mol%
x=55 mol%
x=50 mol%
x=45 mol%
Transmittance (a.u.)
wavenumber(cm-1)
x=40 mol%
Figure 2. IR spectra of irradiated glass samples at room
temperature
ge is assigned to the vibrations of the stretching vibrations
of the isolated VO2 groups in VO4 polyhedra [27], while
the weak band at 1020 cm-1 is related to the vibrations of
V=O bond of the VO5 groups [28].
The IR spectra of irradiated glass samples (Figure 2)
have characteristic bands at 850, 894, 916, 964 and 1020
cm-1. Comparing this with the IR spectra of unirradiated
glass samples (Figure 1); it is observed that after irradia-
tion, the absorption bands at 490 cm-1 and broadband in
the range of 640-770 cm-1 have disappeared. Also after
irradiation, other absorption bands in the range of 850-970
cm-1 bands have broadened and become less intense,
while the band at 1020 cm-1 seems to be slightly more
intensive in irradiated samples. The broadening of IR
spectra clearly suggests the further increase in amorphous
nature of all these glass samples. There is a possibility that
irradiation may dissociate Ag+ ions from interstitial posi-
tions and break V2O5 and TeO2 chains. The Ag+ ions,
which were interacting directly or indirectly with V=O
bonds before irradiation giving rise to a broad band at 970
cm-1, may now be dislodged. As a result of this, the bond
length of the V=O bonds is restored and the frequency due
to this bond at 1020 cm-1 is now seen more intense, con-
sequently, the intensity of 850-970 cm-1 band, which were
due to the stretching of V=O bonds of varying lengths by
Ag+ ions and due to V-O-V chains, reduces.

Co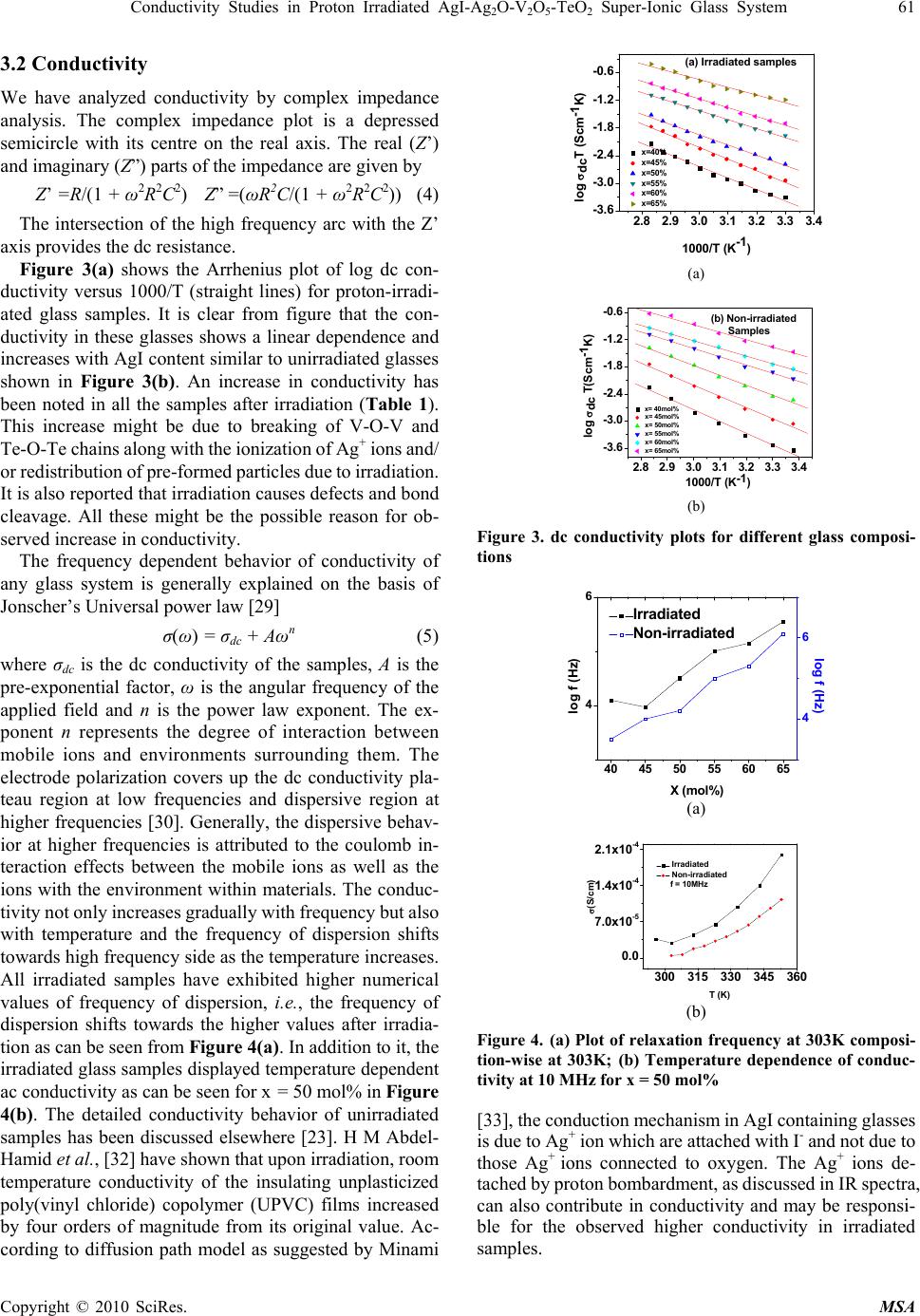nductivity Studies in Proton Irradiated AgI-AgO-V O-TeO Super-Ionic Glass System 61
22 52
3.2 Conductivity
We have analyzed conductivity by complex impedance
analysis. The complex impedance plot is a depressed
semicircle with its centre on the real axis. The real (Z’)
and imaginary (Z”) parts of the impedance are given by
Z’ =R/(1 + ω2R2C2) Z” =(ωR2C/(1 + ω2R2C2)) (4)
The intersection of the high frequency arc with the Z’
axis provides the dc resistance.
Figure 3(a) shows the Arrhenius plot of log dc con-
ductivity versus 1000/T (straight lines) for proton-irradi-
ated glass samples. It is clear from figure that the con-
ductivity in these glasses shows a linear dependence and
increases with AgI content similar to unirradiated glasses
shown in Figure 3(b). An increase in conductivity has
been noted in all the samples after irradiation (Table 1).
This increase might be due to breaking of V-O-V and
Te-O-Te chains along with the ionization of Ag+ ions and/
or redistribution of pre-formed particles due to irradiation.
It is also reported that irradiation causes defects and bond
cleavage. All these might be the possible reason for ob-
served increase in conductivity.
The frequency dependent behavior of conductivity of
any glass system is generally explained on the basis of
Jonscher’s Universal power law [29]
σ(ω) = σdc + Aωn (5)
where σdc is the dc conductivity of the samples, A is the
pre-exponential factor, ω is the angular frequency of the
applied field and n is the power law exponent. The ex-
ponent n represents the degree of interaction between
mobile ions and environments surrounding them. The
electrode polarization covers up the dc conductivity pla-
teau region at low frequencies and dispersive region at
higher frequencies [30]. Generally, the dispersive behav-
ior at higher frequencies is attributed to the coulomb in-
teraction effects between the mobile ions as well as the
ions with the environment within materials. The conduc-
tivity not only increases gradually with frequency but also
with temperature and the frequency of dispersion shifts
towards high frequency side as the temperature increases.
All irradiated samples have exhibited higher numerical
values of frequency of dispersion, i.e., the frequency of
dispersion shifts towards the higher values after irradia-
tion as can be seen from Figure 4(a). In addition to it, the
irradiated glass samples displayed temperature dependent
ac conductivity as can be seen for x = 50 mol% in Figure
4(b). The detailed conductivity behavior of unirradiated
samples has been discussed elsewhere [23]. H M Abdel-
Hamid et al., [32] have shown that upon irradiation, room
temperature conductivity of the insulating unplasticized
poly(vinyl chloride) copolymer (UPVC) films increased
by four orders of magnitude from its original value. Ac-
cording to diffusion path model as suggested by Minami
2.82.93.03.13.23.33.4
-3.6
-3.0
-2.4
-1.8
-1.2
-0.6
x=40%
x=45%
x=50%
x=55%
x=60%
x=65%
log dcT (Scm-1K)
1000/T (K-1)
(a) Irradiated samples
(a)
2.8 2.9 3.0 3.1 3.2 3.3 3.4
-3.6
-3.0
-2.4
-1.8
-1.2
-0.6
x= 40mol%
x= 45mol%
x= 50mol%
x= 55mol%
x= 60mol%
x= 65mol%
log dc T(Scm-1K)
1000/T (K-1)
(b) Non-irradiated
Samples
(b)
Figure 3. dc conductivity plots for different glass composi-
tions
40 45 50 55 60 65
4
6Irradiated
Non-i rr a di a t ed
X (mol% )
log f (Hz)
4
6
log f (Hz)
(a)
300 315 330 345 360
0.0
7.0x10-5
1.4x10-4
2.1x10-4
Irradiated
Non-irradiated
f = 10M Hz
(S/cm)
T (K)
(b)
Figure 4. (a) Plot of relaxation frequency at 303K composi-
tion-wise at 303K; (b) Temperature dependence of conduc-
tivity at 10 MHz for x = 50 mol%
[33], the conduction mechanism in AgI containing glasses
is due to Ag+ ion which are attached with I- and not due to
those Ag+ ions connected to oxygen. The Ag+ ions de-
tached by proton bombardment, as discussed in IR spectra,
can also contribute in conductivity and may be responsi-
ble for the observed higher conductivity in irradiated
samples.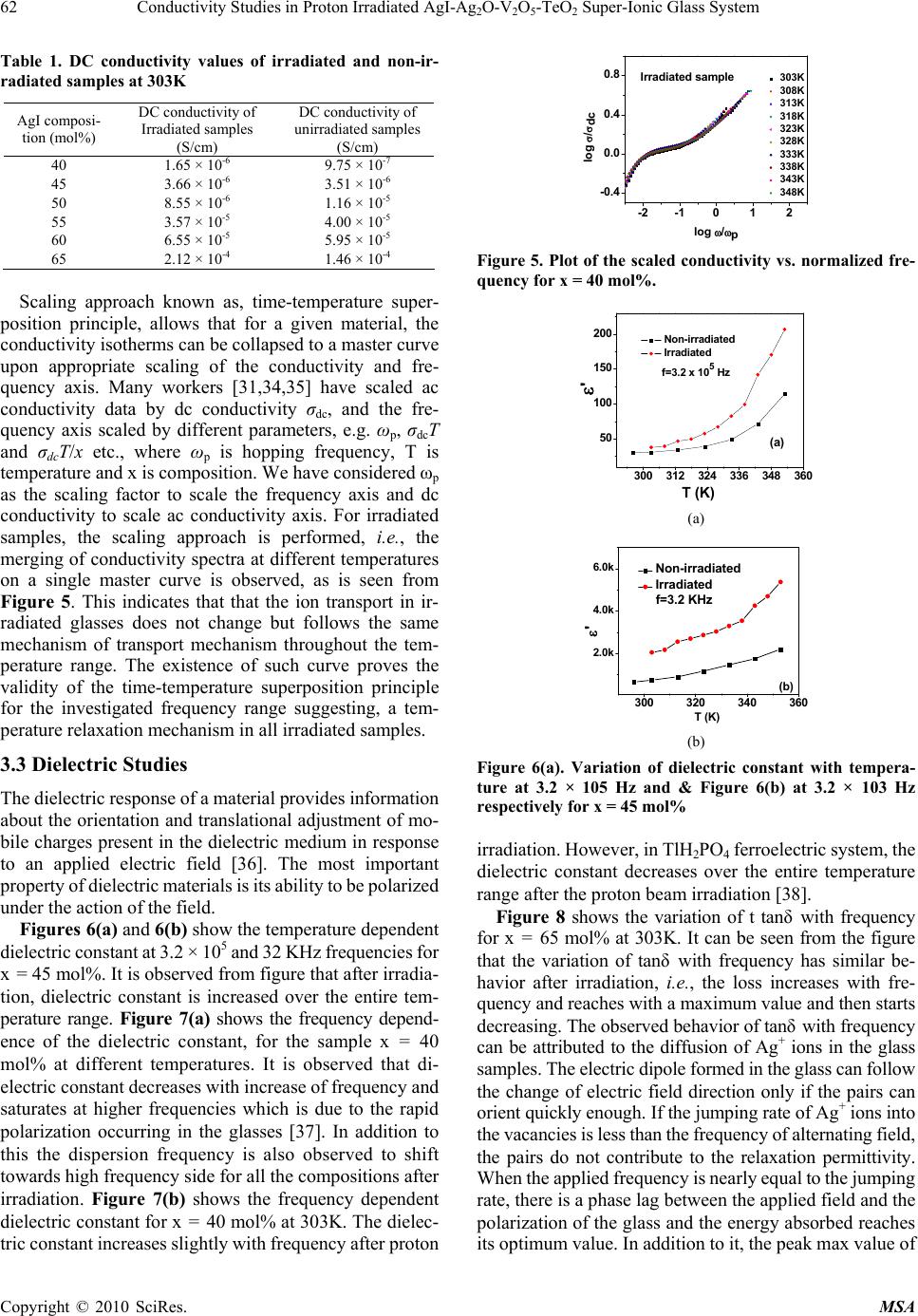

62 Conductivity Studies in Proton Irradiated AgI-AgO-V O-TeO Super-Ionic Glass System
22 52
Table 1. DC conductivity values of irradiated and non-ir-
radiated samples at 303K
AgI composi-
tion (mol%)
DC conductivity of
Irradiated samples
(S/cm)
DC conductivity of
unirradiated samples
(S/cm)
40 1.65 × 10-6 9.75 × 10-7
45 3.66 × 10-6 3.51 × 10-6
50 8.55 × 10-6 1.16 × 10-5
55 3.57 × 10-5 4.00 × 10-5
60 6.55 × 10-5 5.95 × 10-5
65 2.12 × 10-4 1.46 × 10-4
Scaling approach known as, time-temperature super-
position principle, allows that for a given material, the
conductivity isotherms can be collapsed to a master curve
upon appropriate scaling of the conductivity and fre-
quency axis. Many workers [31,34,35] have scaled ac
conductivity data by dc conductivity σdc, and the fre-
quency axis scaled by different parameters, e.g. ωp, σdcT
and σdcT/x etc., where ωp is hopping frequency, T is
temperature and x is composition. We have considered ωp
as the scaling factor to scale the frequency axis and dc
conductivity to scale ac conductivity axis. For irradiated
samples, the scaling approach is performed, i.e., the
merging of conductivity spectra at different temperatures
on a single master curve is observed, as is seen from
Figure 5. This indicates that that the ion transport in ir-
radiated glasses does not change but follows the same
mechanism of transport mechanism throughout the tem-
perature range. The existence of such curve proves the
validity of the time-temperature superposition principle
for the investigated frequency range suggesting, a tem-
perature relaxation mechanism in all irradiated samples.
3.3 Dielectric Studies
The dielectric response of a material provides information
about the orientation and translational adjustment of mo-
bile charges present in the dielectric medium in response
to an applied electric field [36]. The most important
property of dielectric materials is its ability to be polarized
under the action of the field.
Figures 6(a) and 6(b) show the temperature dependent
dielectric constant at 3.2 × 105 and 32 KHz frequencies for
x = 45 mol%. It is observed from figure that after irradia-
tion, dielectric constant is increased over the entire tem-
perature range. Figure 7(a) shows the frequency depend-
ence of the dielectric constant, for the sample x = 40
mol% at different temperatures. It is observed that di-
electric constant decreases with increase of frequency and
saturates at higher frequencies which is due to the rapid
polarization occurring in the glasses [37]. In addition to
this the dispersion frequency is also observed to shift
towards high frequency side for all the compositions after
irradiation. Figure 7(b) shows the frequency dependent
dielectric constant for x = 40 mol% at 303K. The dielec-
tric constant increases slightly with frequency after proton
-2 -1012
-0.4
0.0
0.4
0.8 303K
308K
313K
318K
323K
328K
333K
338K
343K
348K
log /dc
log /p
Irradiated sample
Figure 5. Plot of the scaled conductivity vs. normalized fre-
quency for x = 40 mol%.
300 312 324 336 348 360
50
100
150
200 Non-irradiated
Irradiated
f=3.2 x 105 Hz
'
T (K)
(a)
(a)
300 320 340 360
2.0k
4.0k
6.0k
Non-irradiated
Irradiated
f=3.2 KHz
'
T (K)
(b)
(b)
Figure 6(a). Variation of dielectric constant with tempera-
ture at 3.2 × 105 Hz and & Figure 6(b) at 3.2 × 103 Hz
respectively for x = 45 mol%
irradiation. However, in TlH2PO4 ferroelectric system, the
dielectric constant decreases over the entire temperature
range after the proton beam irradiation [38].
Figure 8 shows the variation of t tanδ with frequency
for x = 65 mol% at 303K. It can be seen from the figure
that the variation of tanδ with frequency has similar be-
havior after irradiation, i.e., the loss increases with fre-
quency and reaches with a maximum value and then starts
decreasing. The observed behavior of tanδ with frequency
can be attributed to the diffusion of Ag+ ions in the glass
samples. The electric dipole formed in the glass can follow
the change of electric field direction only if the pairs can
orient quickly enough. If the jumping rate of Ag+ ions into
the vacancies is less than the frequency of alternating field,
the pairs do not contribute to the relaxation permittivity.
When the applied frequency is nearly equal to the jumping
rate, there is a phase lag between the applied field and the
polarization of the glass and the energy absorbed reaches
its optimum value. In addition to it, the peak max value of

Co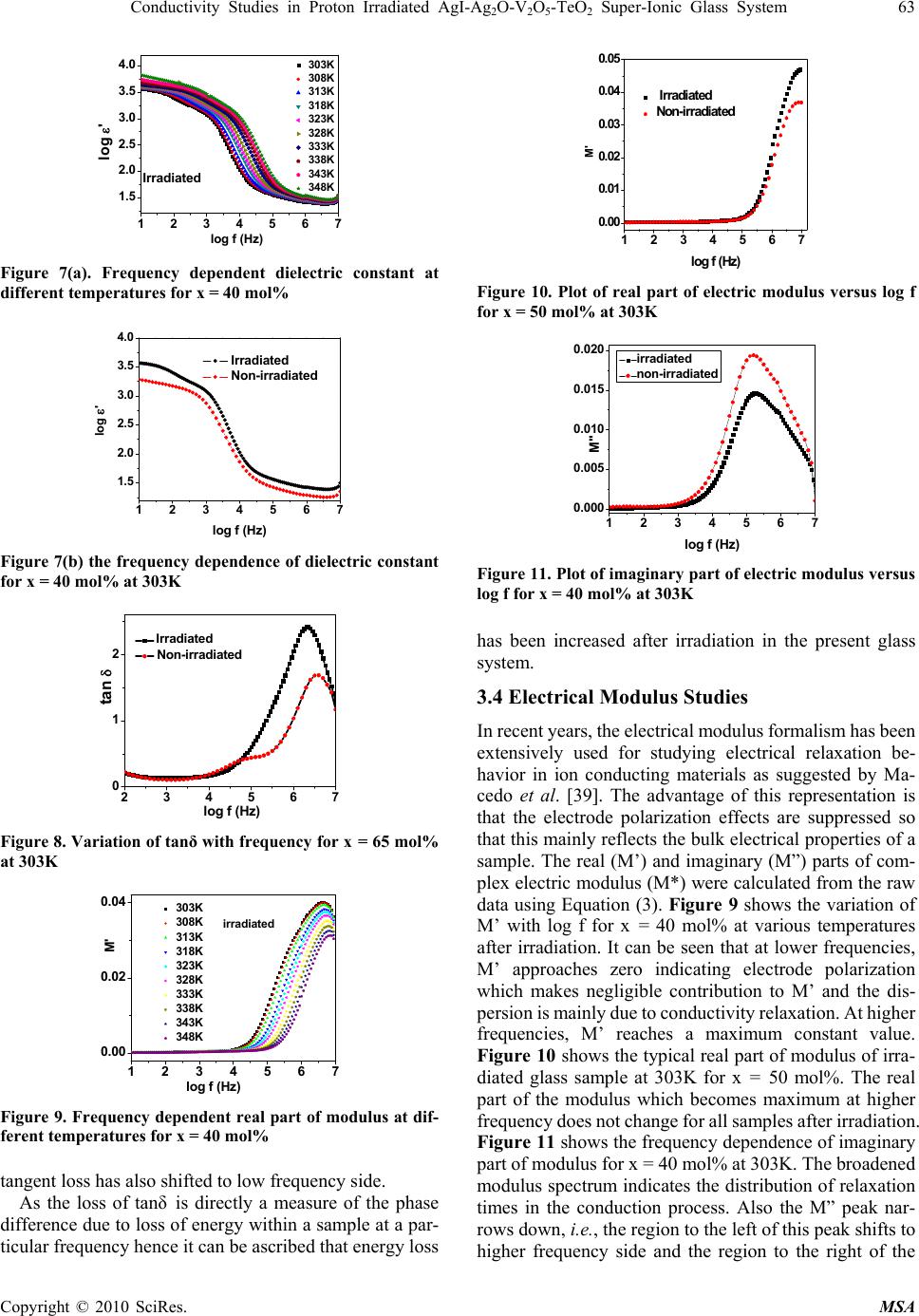nductivity Studies in Proton Irradiated AgI-AgO-V O-TeO Super-Ionic Glass System 63
22 52
1234567
1.5
2.0
2.5
3.0
3.5
4.0 303K
308K
313K
318K
323K
328K
333K
338K
343K
348K
log '
log f (Hz)
Irradiated
Figure 7(a). Frequency dependent dielectric constant at
different temperatures for x = 40 mol%
1234567
1.5
2.0
2.5
3.0
3.5
4.0
log '
log f (Hz)
Irradiated
Non-irradiated
Figure 7(b) the frequency dependence of dielectric constant
for x = 40 mol% at 303K
23456
0
1
2
7
tan 
log f (H z )
Irradiated
Non-irradiated
Figure 8. Variation of tanδ with frequency for x = 65 mol%
at 303K
1234567
0.00
0.02
0.04 303K
308K
313K
318K
323K
328K
333K
338K
343K
348K
M'
log f (Hz)
irradiated
Figure 9. Frequency dependent real part of modulus at dif-
ferent temperatures for x = 40 mol%
tangent loss has also shifted to low frequency side.
As the loss of tanδ is directly a measure of the phase
difference due to loss of energy within a sample at a par-
ticular frequency hence it can be ascribed that energy loss
1234567
0. 00
0. 01
0. 02
0. 03
0. 04
0. 0
5
Irradiated
Non-irradiated
M'
lo g f (H z)
Figure 10. Plot of real part of electric modulus versus log f
for x = 50 mol% at 303K
1234567
0.000
0.005
0.010
0.015
0.020 irradiated
non-irradiated
M"
log f (H z)
Figure 11. Plot of imaginary part of electric modulus versus
log f for x = 40 mol% at 303K
has been increased after irradiation in the present glass
system.
3.4 Electrical Modulus Studies
In recent years, the electrical modulus formalism has been
extensively used for studying electrical relaxation be-
havior in ion conducting materials as suggested by Ma-
cedo et al. [39]. The advantage of this representation is
that the electrode polarization effects are suppressed so
that this mainly reflects the bulk electrical properties of a
sample. The real (M’) and imaginary (M”) parts of com-
plex electric modulus (M*) were calculated from the raw
data using Equation (3). Figure 9 shows the variation of
M’ with log f for x = 40 mol% at various temperatures
after irradiation. It can be seen that at lower frequencies,
M’ approaches zero indicating electrode polarization
which makes negligible contribution to M’ and the dis-
persion is mainly due to conductivity relaxation. At higher
frequencies, M’ reaches a maximum constant value.
Figure 10 shows the typical real part of modulus of irra-
diated glass sample at 303K for x = 50 mol%. The real
part of the modulus which becomes maximum at higher
frequency does not change for all samples after irradiation.
Figure 11 shows the frequency dependence of imaginary
part of modulus for x = 40 mol% at 303K. The broadened
modulus spectrum indicates the distribution of relaxation
times in the conduction process. Also the M” peak nar-
rows down, i.e., the region to the left of this peak shifts to
higher frequency side and the region to the right of the

64 Conductivity Studies in Proton Irradiated AgI-AgO-V O-TeO Super-Ionic Glass System
22 52
peak shifts to lower frequency side after irradiation. The
peak maxima value of M’’ remains same for all compo-
sitions even after irradiation. The long tail at low fre-
quencies and the shape of the modulus spectra attribute
the non-Debye nature of samples after irradiation.
4. Conclusions
The proton ion beam irradiation on AgI-based super ionic
glass system shows that the dc and ac conductivity in-
crease after irradiation. Relaxation frequency further shifts
towards the higher frequency side and energy losses in-
creases after irradiation. The scaling of irradiated samples
follows the same relaxation process and confirms the
validity of the time-temperature superposition principle.
The dielectric constant and the dielectric losses are in-
creased after irradiation. The modulus spectra confirm the
non-Debye nature for irradiated and unirradiated samples.
Finally, it can be ascribed that proton irradiation causes
the structural defects by breaking the bridging oxygen or
oxygen displacement and/or cation displacement which
might be responsible for the observed changes in transport
properties of the irradiated super ionic glass system.
5. Acknowledgements
Authors DKK and PS, respectively thankfully acknowl-
edge the financial support by the DST, New Delhi, INDIA
via Grant No. SR/S2/CMP-40/2004 and UGC, New Delhi,
India for RFSMS fellowship.
REFERENCES
[1] S. Bhattacharya and A. Ghosh, “Relaxation Dynamics in
AgI-Doped Silver Vanadate Superionic Glasses,” Journal
of Chemical Physics, Vol. 123, No. 12, 2005, pp. 1-5.
[2] K. P. Padmsree, D. K. Kanchan, H. R. Panchal, A. M.
Awasthi and S. Bharadwaj, “Structural and Transport
Properties of CdI2 Doped Silver Ion Conducting System,”
Solid State Commun, Vol. 136, No. 2, October 2005, pp.
102-107.
[3] A. K. Arof, “Internal Resistance and Cathode Content in
Silver Borovanadate Batteries,” Journl of Power Sources,
Vol. 52, No. 1, November 1994, pp. 129-133.
[4] A. Singh, “Irradiation of Polymer Blends Containing a
Polyolefin,” Radiation Physics and Chemistry, Vol. 60,
No. 4-5, 2001, pp. 453-459.
[5] O. Puglisi, M. E. Fragala, K. G. Lynn, M. Petkov, et al.,
“Study of Ion Beam Induced Depolymerization Using
Positron Annihilation Techniques,” Nuclear Instruments
and Methods in Physics Research Section B: Beam
Interactions with Materials and Atoms, Vol. 175-177,
April 2001, pp. 605-609.
[6] J. C. Pivin, M. Sendova-Vassileva, M. Nikolaeva, D.
Dimova-Malinovska and A. Martucci, “Optical Extinction
Resonance of Au and Ag Clusters Formed by Ion
Irradiation in SiO2 and Al2O3,” Applied Physics A:
Materials Science and Processing, Vol. 75, No. 3, pp.
401-410.
[7] J. C. Pivin, “Mixing of Noble Metals in Oxides and
Formation of Colloids by Ion Irradiation,” Materials
Science and Engineering A, Vol. 293, No. 1-2, November
2000, pp. 30-38.
[8] J. C. Pivin, M. A. Garcia, J. Llopis, and H. Hofmeister,
“Interaction between Clusters in Ion Implanted and Ion
Beam Mixed SiO2: Ag Films,” Nuclear Instruments and
Methods in Physics Research Section B: Beam Interac-
tions with Materials and Atoms, Vol. 191, No. 1-4, May
2002, pp. 794-799.
[9] H. Hofmeister, S. Thiel, M. Dubiel and F. Schurig, “Syn-
thesis of Nanosized Silver Particles in Ion-Exchanged
Glass by Electron Beam Irradiation,” Applied Physics
Letter, Vol. 70, No. 13, 1997, pp. 1694-1696.
[10] S. Chen, T. Akai, K. Kadono and T. Yazawa, “Reversible
Control of Silver Nanoparticle Generation and Dis-
solution in Soda-Lime Silicate Glass through X-Ray Irra-
diation and Heat Treatment,” Applied Physic Letters, Vol.
79, No. 22, 2001, pp. 3687-3689.
[11] D. L. Griscom, “E.S.R. Studies of Radiation Damage and
Structure in Oxide Glasses not Containing Transition
Group Ions: A Contemporary Overview with Illustrations
from the Alkali Borate System,” Journal of Non-Crystalline
Solids, Vol. 13, No. 2, January 1974, pp. 251-285.
[12] R. A. B. Devine, “Macroscopic and Microscopic Effects
of Radiation in Amorphous SiO2,” Nuclear Instruments
and Methods in Physics Research Section B: Beam
Interactions with Materials and Atoms, Vol. 91, No. 1-4,
June 1994, pp. 378-390.
[13] V. N. Sandalov, M. I. Muminov and E. M. Ibragimova,
“Radiation-Induced Enhancement of Proton-Conductivity
in Porous Glass,” Materials Science and Engineering B,
Vol. 108, No. 1-2, April 2004, pp. 171-173.
[14] E. J. Friebele, “Optical Properties of Glasses,” American
Ceramic Society, Westerville, 1991.
[15] B. Boizot, G. Petite, D. Ghaleb and G. Calas, “Dose, Dose
Rate and Irradiation Temperature Effects in β-Irradiated
Simplified Nuclear Waste Glasses by EPR Spectroscopy,”
Journal of Non-Crystalline Solids, Vol. 283, No. 1-3, May
2001, pp. 179-185.
[16] H. Hofmeister, M. Dubiel, H. Graener and J. C. Pivin,
“Structural Characteristics of Metal Nanoparticles in
Glass upon Irradiation-Assisted Processing,” Radiation
Effects and Defects in Solids, Vol. 158, No. 1-6, 2003, pp.
49-54.
[17] B. Boizot, G. Petite, D. Ghaleb, B. Reynard and G. Calas,
“Radiation Induced Paramagnetic Centres in Nuclear
Glasses by EPR Spectroscopy,” Nuclear Instruments and
Methods in Physics Research Section B, Vol. 141, No.
1-4, 1998, pp. 580-585.
[18] B. Boizot, G. Petite, D. Ghaleb, B. Reynard and G. Calas,
“Raman study of β-irradiated glasses,” Journal of Non-
Crystalline Solids, Vol. 243, No. 2-3, February 1999, pp.
268-272.
[19] B. Boizot, G. Petite, D. Ghaleb, N. Pellerin, et al.,
“Migration and Segregation of Sodium under β-Irradiation

Conductivity Studies in Proton Irradiated AgI-Ag2O-V2O5-TeO2 Super-Ionic Glass System 65
in Nuclear Glasses,” Nuclear Instruments and Methods in
Physics Research Section B: Beam Interactions with
Materials and Atoms, Vol. 166-167, May 2000, pp.
500-504.
[20] B. Constantinescu, R. Bugoi, E. R. Hodgson, R. Vila and
P. Ioan, “Studies on Proton Irradiation-Induced Modifica-
tions of KU1 and KS-4V Quartz Glasses Ultraviolet Trans-
mission Properties,” Journal of Nuclear Materials, Vol.
367-370, August 2007, pp. 1048-1051.
[21] V. T. Kuanyshev, T. A. Belykh, et al., “Fundamental
Processes of Radiation Energy Storage in KDP (KH2PO4)
and ADP (NH4H2PO4) Crystals,” Radiation Measurements,
Vol. 33, No. 5, October 2001, pp. 503-507.
[22] A. K. Arof and S. Radhakrishna, “Electrical Properties of
Silver Vanadate Electrochemical Cells,” Journal of Alloys
and Compounds, Vol. 200, No. 1-2, October 1993, pp.
129-134.
[23] P. Sharma, D. K. Kanchan, M. Pant and K. P. Padmashree,
“Transport Properties of Super Ionic AgI-Ag2O-V2O5-TeO2
Glasses,” Indian Journal of Pure and Applied Physics,
Vol. 48, No. 1, 2010, pp. 39-46.
[24] A. A. Baghat, I. I. Shaltout and A. M. Abu-Elazm,
“Structural and thermal Properties of some Tellurite
Glasses,” Journal of Non-Crystalline Solids, Vol. 150, No.
1-3, November 1992, pp. 179-184.
[25] Y. Dimitriev, V. Dimitrov and M. Arnaudov, “IR Spectra
and Structures of Tellurite Glasses,” Journal of Materials
Science, Vol. 18, No. 5, May 1983, pp. 1353-1358.
[26] M. Pant, D. K. Kanchan and P. Sharma, “Characterization
and Transport Properties of 10BaO-xAg2O-(85-x)V2O5-
5TeO2 Glass System,” Communicated in Ionics, in Press.
[27] S. Sen and A. Ghosh, “Structure and Other Physical
Properties of Magnesium Vanadate Glasses,” Journal of
Non-Crystalline Solids, Vol. 258, No. 1-3, November
1999, pp. 29-33.
[28] E. F. Lambson, G. A. Saunders, B. Bridge and R. A. El-
Mallawany, “The Elastic Behaviour of TeO2 Glass under
Uniaxial and Hydrostatic Pressure,” Journal of Non-
Crystalline Solids, Vol. 69, No. 1, December 1984, pp.
117-133.
[29] A. K. Jonscher, “The ‘Universal’ Dielectric Response,”
Nature, Vol. 267, June 1977, pp. 673-679.
[30] D. K. Kanchan, K. P. Padmashree, H. R. Panchal and A.
R. Kulkarni, “Electrical Transport Studies on CdI2 Doped
Silver Oxysalt System,” Cermics International, Vol. 30,
No. 7, 2004, pp. 1655-1660.
[31] M. Pant, D. K. Kanchan, P. Sharma and M. S. Jayswal,
“Mixed Conductivity Studies in Silver Oxide Based
Barium Vanado—Tellurite Glasses,” Materials Science
and Engineering B, Vol. 149, No. 1, March 2008, pp.
18-25.
[32] H. M. Abdel-Hamid, R. M. Radwan and A. H. Ashour,
“Ion Beam Induced Changes in Electrical Resistivity of
Polymer Films: The Case of Unplasticized Poly (Vinyl
Chloride),” Journal of Physics D: Applied Physics, Vol.
35, No. 11, 2002, pp. 1183-1187.
[33] T. Minami, “Fast Ion Conducting Glasses,” Journal of
Non-Crystalline Solids, Vol. 73, No. 1-3, July 1984, pp.
273-284.
[34] A. Ghosh and A. Pan, “Scaling of the Conductivity
Spectra in Ionic Glasses: Dependence on the Structure,”
Physics Review Letters, Vol. 84, No. 10, March 2000, pp.
2188-2190.
[35] B. Roling, A. Happe, K. Funke and M. D. Ingram, “Carrier
Concentrations and Relaxation Spectroscopy: New Infor-
mation from Scaling Properties of Conductivity Spectra
in Ionically Conducting Glasses,” Physics Review Letters,
Vol. 78, No. 11, 1997, pp. 2160-2163.
[36] K. P. Padmashree and D. K. Kanchan, “Dielectric Studies
on CdI2 Doped Ag2O-V2O5-B2O3 System,” Materials
Chemistry Physics, Vol. 91, No. 2-3, June 2005, pp. 551-
557.
[37] D. L. Sidebottom, B. Roling and K. Funke, “Ionic
Conduction in Solids: Comparing Conductivity and Modulus
Representations with Regard to Scaling Properties,”
Physical Review B, Vol. 63, No. 2, pp. 1-7.
[38] S. H. Kim, K. W. Lee and C. E. Lee, “Dielectric
Properties of Proton-Irradiated TlH2PO4,” Journal of
Korean Physical Society, Vol. 48, No. 3, March 2006, pp.
433-436.
[39] P. B. Macedo, C. T. Moynihan and R. Bose, “The Role of
Ionic Diffusion in Polarization in Vitreous Ionic Condu-
ctors,” Physics and Chemistry Glasses, Vol. 13, 1972, pp.
171-179.