Journal of Minerals & Materials Characterization & Engineering, Vol. 10, No.3, pp.309-3 14, 2011
jmmce.org Printed in the USA. All rights reserved
309
Modeling Grain Structures of Some Carbon Steels using Voronoi Tesselation
O.O. Oluwole1* and A.L. Akinkunmi2
1Mechanical Engineering Dept., Univ. of Ibadan, Nigeria;
2Materials Science and Engineering Dept., Obafemi Awolowo Univ., Nigeria
* Corresponding Author: [e-mail]
ABSTRACT
Modeled grain structures of normalized carbon steels using voronoi tessellation is reported in
this work. Three stages of programming were used in modeling the microstructures. The first
stage was iteration of the voronoi cells in order to obtain equivalent grain size with experimental
specimens. In the second stage, the pearlite phase was introduced using the lever rule
represented by a plot of random points. The third layer was modeled to reveal the grain
boundaries of the carbon steels.
The values of the grain sizes of modeled microstructures showed good agreement with
experimental values. The study has shown that the microstructures can be modeled fairly
accurately thus enabling a fairly quick export of geometric models on to some other finite
element packages for analysis of stress - strain effect on microstructure and generally a stress-
microstructure response could be determined.
Key words: microstructure -modeling,voronoi tessellation, carbon steels
1. INTRODUCTION
Materials selection and design for durability, rests upon our understanding of phenomena
occurring at microstructural scales as well as at the scale of structural components [1-4]. The
goal of tailoring the material microstructure to control plastic deformation and related failure
processes are of great economic importance. I t then become s a necessity to find a way to predict
the effect of different levels of concentration of constitutional components on the mechanical
properties of alloys. The concept of Voronoi tessellation [5-8] has been extensively used in

310 O.O. Oluwole and A.L. Akinkunmi Vol.10, No.3
materials science, especially to model the geometrical features of random microstructures like
aggregates of grains in polycrystals, patterns of intergranular cracks and composites [9] .
2. METHODOLOGY
2.1 Experimentation
Steel rods of wt.% carbon compositions 0.23, 0.25, 0.28, 0.33 and 0.47% were cut into 1inch
lengths to ensure ease of handling during subsequent metallographic processes. The samples
were subjected to normalizing heat treatments using a CarboliteR electric furnace with
maximum operating temperature of 1700°C. They were then subjected to standard
metallographic analysis [10]. Nital was used for etching. Photomicrographs were obtained using
camera fitted optical metallurgical microscope. Heyn’s intercept method and ASTM E112 [11]
standard was used in obtaining grain size number.
2.2 Modeling
Three stages of programming were used in modeling the microstructures. The first stage
involved iteration of the voronoi cells in order to obtain equivalent grain size with experimental
specimens (Fig.1). In the second stage, the pearlite phase wa s introduced using the lever rule and
is represented by a plot of random points (Fig.2). Number of points were input to correspond
with the pearlite composition. The remaining spaces uncovered with the scattered points
represented the ferrite phase. The third layer was modeled to reveal the grain boundaries of the
carbon steels (Fig.3). Iteration of random tessellations continued until equivalent grain cells were
obtained by grain size calculations as expressed in section 2.1 above and comparing with
experimental results.
MATLABR 2007b [12,13] was used for the programming environment. The final result is a
model clearly showing the grain boundaries, the grain size, the grain shape, and the carbon
content represented by the ratio of ferrite to pearlite phases in the microstructure.
Fig 1:First Stage (Layer 1)

Vol.10, No.3 Modeling Grain Structures of Some Carbon Steels 311
311
Fig.2: Second Stage (Layer 2)
Fig.3:Third Stage (Layer 3)
3. RESULTS AND DISCUSSION
3.1 Results
Figures 4a, 5a, 6a, 7a and 8a are the photomicrographs of normalized carbon steels of %wt.
carbon composition of 0.23, 0.25, 0.28, 0.33 and 0.47%, respectively. Figures 4b, 5b, 6b, 7b and
8b are the modeled grains using voronoi tessellation. Their grain sizes are presented in Table 1.
Fig.4(a): Normalized 0.23% carbon Fig.4(b):Normalized 0.23% carbon
(Micrograph) X200 (Model)

312 O.O. Oluwole and A.L. Akinkunmi Vol.10, No.3
Fig.5(a): Normalized 0.25% carbon Fig.5(b): Normalized 0.25% carbon
(Micrograph) X200 (Model)
Figures 4b, 5b, 6b, 7b and 8b show the modeled micrographs of the five steel samples. It was
observed that the greater the number of iterations, within any confined space, the smaller the
grain size.
Fig.6(a): Normalized 0.28% carbon Fig.6(b): Normalized 0.28% carbon
(Micrograph)X200 (Model)
Fig. 7(a): Normalized 0.33% carbon Fig.7(b): Normalized 0.33% carbon
(Micrograph) X200 (Model)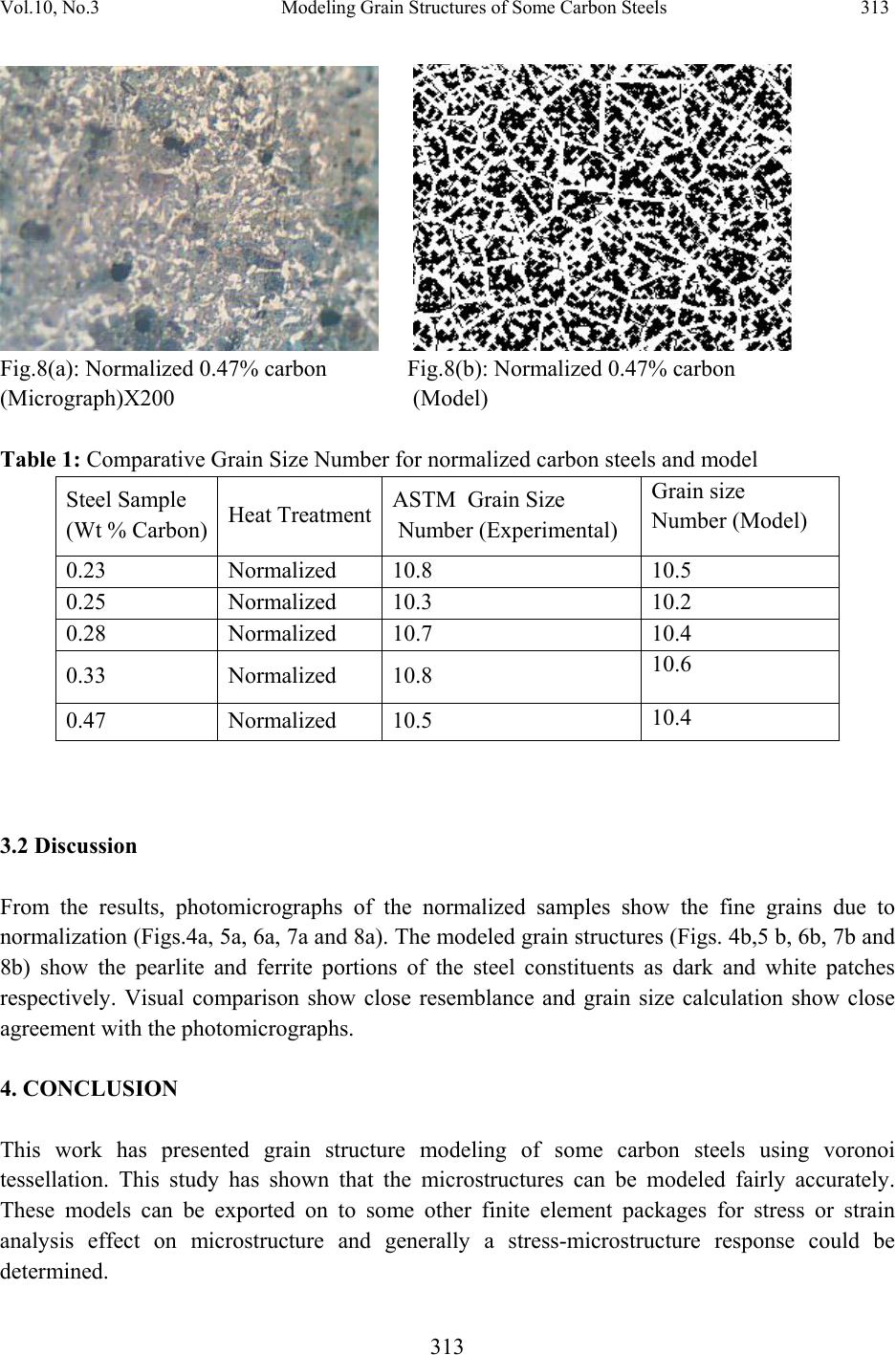

Vol.10, No.3 Modeling Grain Structures of Some Carbon Steels 313
313
Fig.8(a): Normalized 0.47% carbon Fig.8(b): Normalized 0.47% carbon
(Micrograph)X200 (Model)
Table 1: Comparative Grain Size Number for normalized carbon steels and model
Steel Sample
(Wt % Carbon) Heat TreatmentASTM Grain Size Number (Experimental)
Grain size
Number (Model)
0.23 Normalized 10.8 10.5
0.25 Normalized 10.3 10.2
0.28 Normalized 10.7 10.4
0.33 Normalized 10.8 10.6
0.47 Normalized 10.5 10.4
3.2 Discussion
From the results, photomicrographs of the normalized samples show the fine grains due to
normalization (Figs.4a, 5a, 6a, 7a and 8a). The modeled grain structures (Figs. 4b,5 b, 6b, 7b and
8b) show the pearlite and ferrite portions of the steel constituents as dark and white patches
respectively. Visual comparison show close resemblance and grain size calculation show close
agreement with the photomicrographs.
4. CONCLUSION
This work has presented grain structure modeling of some carbon steels using voronoi
tessellation. This study has shown that the microstructures can be modeled fairly accurately.
These models can be exported on to some other finite element packages for stress or strain
analysis effect on microstructure and generally a stress-microstructure response could be
determined.

314 O.O. Oluwole and A.L. Akinkunmi Vol.10, No.3
REFERENCES
[1] Reed-Hill, R.E., (1973) Physical Meta l lurgy Principles, 2nd ed., Van Nostrand
[2] Makinamuhendisi (2008) ‘Low Carbon steel’ http://www.makinamuhendisi.com /
mechanical / mechanical-engineering-archives/176-Low-Carbon-Steels-Mild-Steel.html
[3] Key-to-steel (2008) Hardenable Carbon Steels http://www.key-to-
steel.com/ViewArticle.asp?ID=90
[4] Wikepedia(2008) ‘Carbon Steels’ http://en.wikipedia.org/wiki/Carbon_steel
[5] JSTOR SIAM (1999 ) Generating Voronoi Tesselations’ JSTOR SIAM Review, 41( 4),
637-676.
[6] Arwade, S. R., 2000, Probabilistic Models for Aluminum Microstructures and
Intergranular Fracture Analysis, Masters Thesis, Cornell University.
[7] Sinum (2006)Lloyd algorithm for computing CVTs.
http://www.math.psu.edu/qdu/Res/Pre/dmj06sinum.pdf
[8] JSTOR(2008)‘Centroidal voronoi tessellations’.
[9] Qiang Du, Vance Faber and Max Gunzburger, (1999) Centroidal Voronoi Tessellations:
Applications and Algorithms, Society for Industrial and Applied Mathematics.
[10]Oluwole, O.O., D.T. Oloruntoba, and O. Awheme. (2008) "Effect of Zinc Plating of Low
Carbon Steel on Corrosion Resistance in Cocoa Fluid Environment". Materials and Design,
29(6):1266-1274.
[11]Singh.V (2005) ‘Physical Metallurgy’ Standard Publishers, New-Delhi,p.493-497
[12]Higham.D.J and N.J. Higham (2000) Matlab Guide SIAM Philadelphia.
[13]Mathwork (2004) Introduction: What is Matlab? Matlab Help File, Mathwork, Inc.USA