Journal of Minerals & Materials Characterization & Engineering, Vol. 9, No.10, pp.907-917, 2010
jmmce.org Printed in the USA. All rights reserved
907
The Development of Mathematical Model for the Prediction of Ageing Behaviour
for Al-Cu-Mg/Bagasse Ash Particulate Composites
V.S. Aigbodion*, S.B. Hassan, E.T. Dauda and R.A. Mohammed
Department of Metallurgical and Materials Engineering,
Ahmadu Bello University, Samaru, Zaria, Nigeria
*Corresponding Author: [e-mail]
ABSTRACT
The thermal ageing behaviour model of Al-Cu-Mg/Bagasse ash particulate composites with 2-
10wt% bagasse ash particles produced by double stir-casting method was developed in terms of
weight fraction of bagasse ash, ageing temperature and time. Hardness values measurement was
used in determining the ageing behaviour, after solution and age-hardened heat-treatment. The
experimental results demonstrate that the bagasse ash was the major parameter in the ageing
behaviour, followed by ageing temperature. The hardness values decreased as the ageing time
increases, interaction of weight of bagasse ash, ageing time and ageing temperature. Moreover,
the optimal combination of the testing parameters could be predicted. The predicted hardness
values were found to lie close to that of the experimentally observed ones. The developed
mathematical model can be employed for optimization of the process parameters of the ageing
behaviour of Al-Cu-Mg/Bagasse ash particulate composites with respect to har dness val ues.
Keywords: Al-Cu-Mg alloy, Ageing temperature and time, Analysis of variance, Bagasse Ash
and Linear regression
1. INTRODUCTION
The development of metal matrix composites (MMCs) is of great interest in industrial
applications for lighter materials with high specific strength, stiffness and heat resistance, they
form a new class of industrial materials [1]. In MMCs, aluminium-matrix composites (MMCs)
reinforced with discontinuous reinforcements are very attractive because they give the best
combination of strength, ductility and toughness and they can be processed by conventional

908 V.S. Aigbodion, S.B. Hassan, E.T. Dauda and R.A. Mohammed Vol.9, No.10
methods such as casting, rolling, forging, extrusion and as a final process, machining [1-3].
Recently, there has been an increasing interest in composites containing low density and low
cost reinforcements [4-5]. Among various discontinuous dispersions used bagasse ash has been
found to be one of the most inexpensive and low density reinforcement available in large quantities as
solid waste from the sugar processing mill [3, 6]. Hence, composites with bagasse ash as
reinforcement are likely to overcome the cost barrier for wide spread applications in automotive
and small engine applications. It is therefore expected that the incorporation of bagasse ash
particles in aluminium alloy will promote yet another use of this low-cost waste by-product and,
at the same time, have the potential for conserving energy- intensive aluminium and thereby,
reducing the cost of aluminium products [3, 6].
The age hardening characteristics of an alloy are generally modified by the introduction of
reinforcement. These modifications are due to the manufacturing process, the reactivity between
the reinforcement and the matrix, the size, morphology and volume fraction of the reinforcement.
Strength increment due to ageing is necessary in aluminium alloys because it helps to develop
acceptable mechanical properties [1].
The earlier works [7-11] concluded that the addition of discontinuous ceramic particles into
aluminium matrix resulted in the dislocation generation leading to different ageing kinetics
compared to monolithic alloys. Thus, there is a complexity involved in the ageing process of
composites when compared with that of unreinforced alloys [8]. Hence, an attempt has been
made in the present investigation to study and systematically record the effects of heat-treatment
parameters on hardness of Al-Cu-Mg/BAp composite. The age-hardening model of these
composites was developed based on the weight fraction of bagasse ash, ageing temperature and
time. Further more analysis of variance (ANOVA) is employed to investigate the testing
characteristics of the Al-Cu-Mg/BAp composites.
2. MATERIALS AND EXPERIMENTAL PROCEDURE
The Bagasse ash used in this study was characterized Composites used in this study were
A2009Al–Bagasse ash particles composites containing 2-10 Wt% Bagasse ash particles. The
samples were produced using the double stir casting method [1, 3] by keeping the percentage of
copper and magnesium constant (3.7%Cu and 1.4%Mg) according to the recommended standard
to produced alloy of type A2009 [3, 6] with 2-10Wt% Bagasse ash of particles size of 64µm. The
floating of the ash was avoid using the double stirring of the molten mixture. A control sample
without the Bagasse ash was also produced with this method. After casting, the specimen was
machined into hardness coupons for the purpose of determining the thermal ageing behaviour of
the produced composites.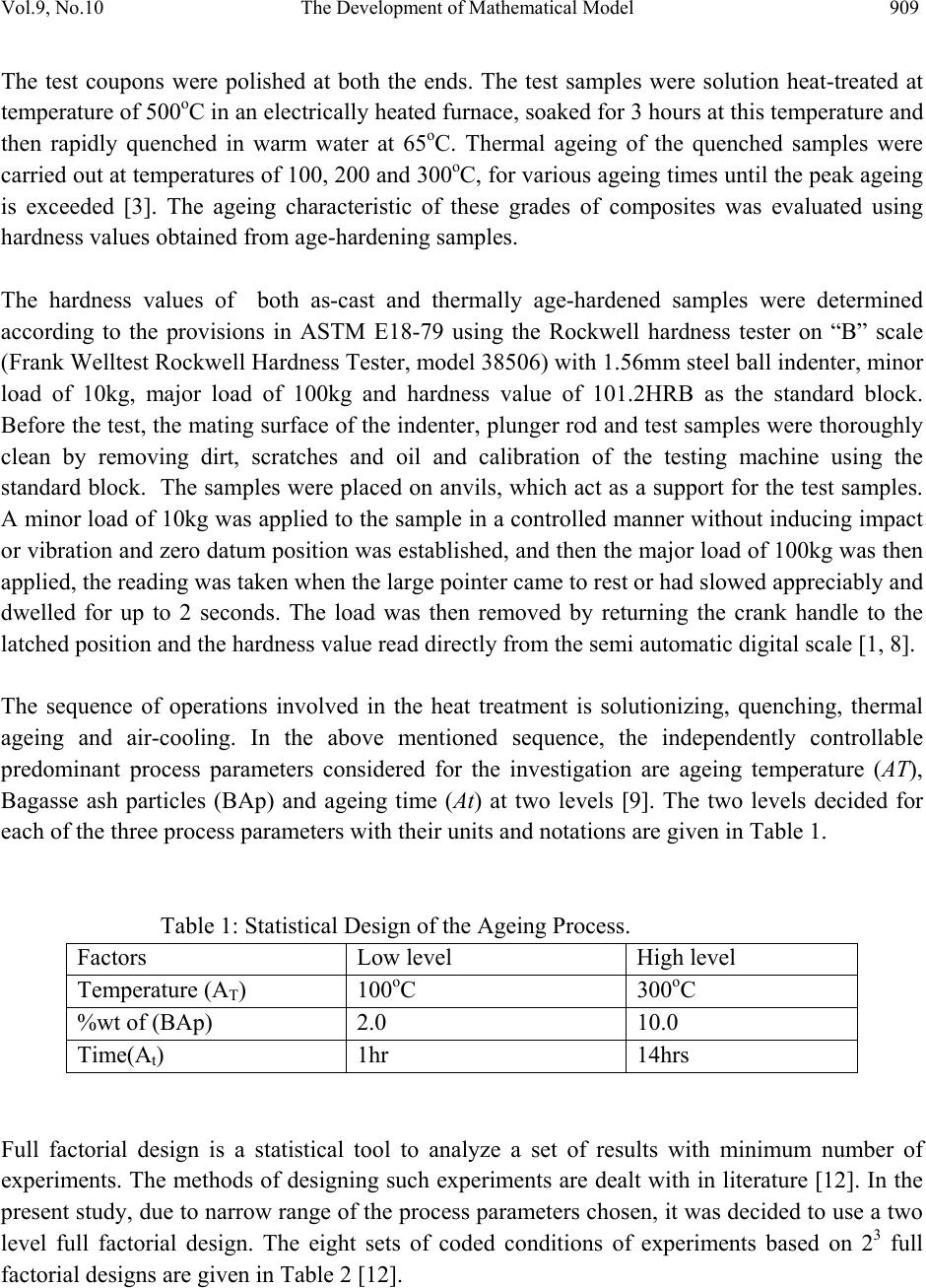

Vol.9, No.10 The Development of Mathematical Model 909
The test coupons were polished at both the ends. The test samples were solution heat-treated at
temperature of 500oC in an electrically heated furnace, soaked for 3 hours at this temperature and
then rapidly quenched in warm water at 65oC. Thermal ageing of the quenched samples were
carried out at temperatures of 100, 200 and 300oC, for various ageing times until the peak ageing
is exceeded [3]. The ageing characteristic of these grades of composites was evaluated using
hardness values obtained from age-hardening samples.
The hardness values of both as-cast and thermally age-hardened samples were determined
according to the provisions in ASTM E18-79 using the Rockwell hardness tester on “B” scale
(Frank Welltest Rockwell Hardness Tester, model 38506) with 1.56mm steel ball indenter, minor
load of 10kg, major load of 100kg and hardness value of 101.2HRB as the standard block.
Before the test, the mating surface of the indenter, plunger rod and test samples were thoroughly
clean by removing dirt, scratches and oil and calibration of the testing machine using the
standard block. The samples were placed on anvils, which act as a support for the test samples.
A minor load of 10kg was applied to the sample in a controlled manner without inducing impact
or vibration and zero datum position was established, and then the major load of 100kg was then
applied, the reading was taken when the large pointer came to rest or had slowed appreciably and
dwelled for up to 2 seconds. The load was then removed by returning the crank handle to the
latched position and the hardness value read directly from the semi automatic digital scale [1, 8].
The sequence of operations involved in the heat treatment is solutionizing, quenching, thermal
ageing and air-cooling. In the above mentioned sequence, the independently controllable
predominant process parameters considered for the investigation are ageing temperature (AT),
Bagasse ash particles (BAp) and ageing time (At) at two levels [9]. The two levels decided for
each of the three process parameters with their units and notations are given in Table 1.
Table 1: Statistical Design of the Ageing Process.
Factors Low level High level
Temperature (AT) 100oC 300oC
%wt of (BAp) 2.0 10.0
Time(At) 1hr 14hrs
Full factorial design is a statistical tool to analyze a set of results with minimum number of
experiments. The methods of designing such experiments are dealt with in literature [12]. In the
present study, due to narrow range of the process parameters chosen, it was decided to use a two
level full factorial design. The eight sets of coded conditions of experiments based on 23 full
factorial designs are given in Table 2 [12].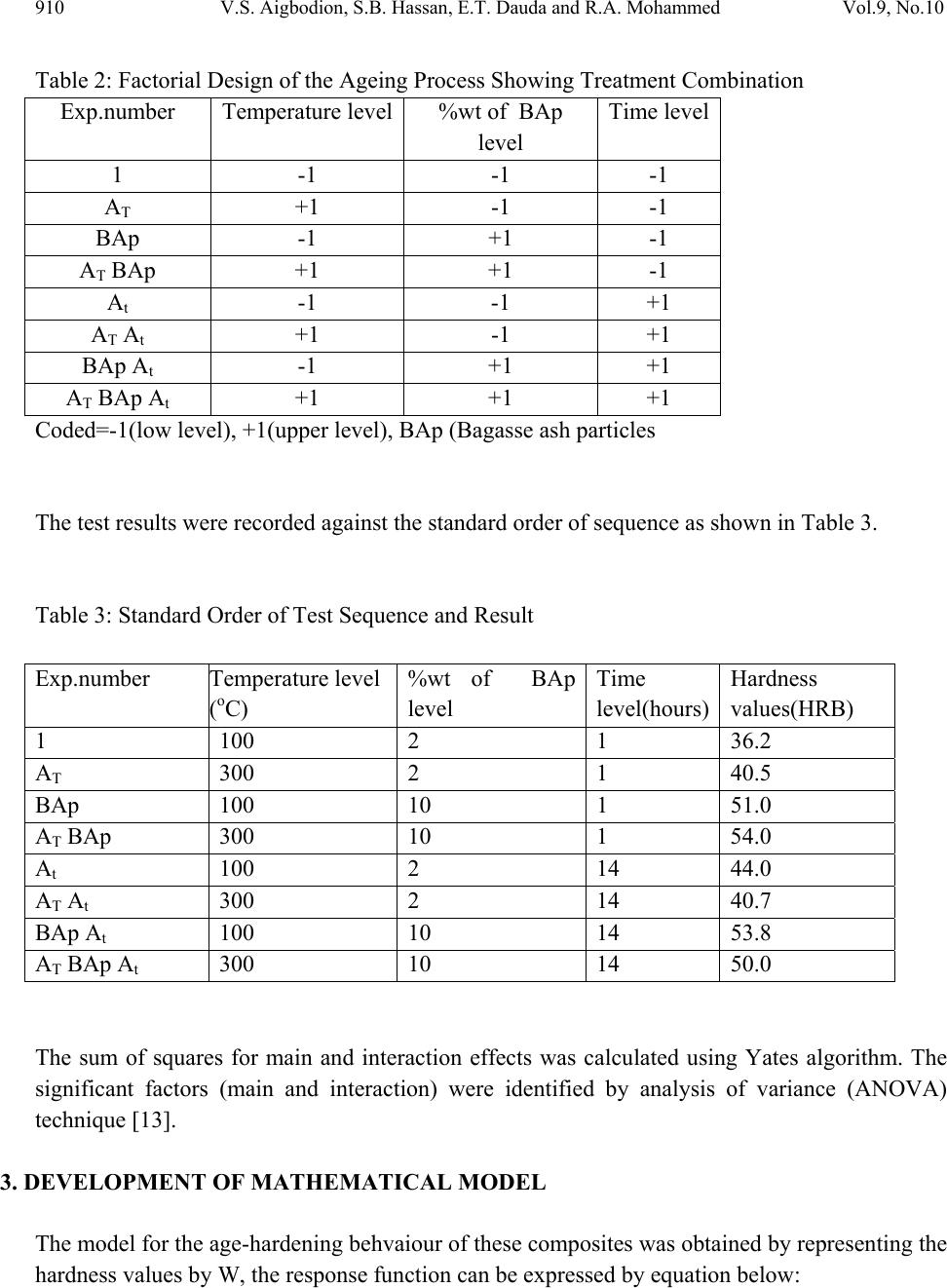

910 V.S. Aigbodion, S.B. Hassan, E.T. Dauda and R.A. Mohammed Vol.9, No.10
Table 2: Factorial Design of the Ageing Process Showing Treatment Combination
Exp.number Temperature level%wt of BAp
level
Time level
1 -1 -1 -1
AT +1 -1 -1
BAp -1 +1 -1
AT BAp +1 +1 -1
At -1 -1 +1
AT At +1 -1 +1
BAp At -1 +1 +1
AT BAp At +1 +1 +1
Coded=-1(low level), +1(upper level), BAp (Bagasse ash particles
The test results were recorded against the standard order of sequence as shown in Table 3.
Table 3: Standard Order of Test Sequence and Result
Exp.number Temperature level
(oC)
%wt of BAp
level
Time
level(hours)
Hardness
values(HRB)
1 100 2 1 36.2
AT 300 2 1 40.5
BAp 100 10 1 51.0
AT BAp 300 10 1 54.0
At 100 2 14 44.0
AT At 300 2 14 40.7
BAp At 100 10 14 53.8
AT BAp At 300 10 14 50.0
The sum of squares for main and interaction effects was calculated using Yates algorithm. The
significant factors (main and interaction) were identified by analysis of variance (ANOVA)
technique [13].
3. DEVELOPMENT OF MATHEMATICAL MODEL
The model for the age-hardening behvaiour of these composites was obtained by representing the
hardness values by W, the response function can be expressed by equation below:

Vol.9, No.10 The Development of Mathematical Model 911
W=ƒ (AT, BP, At) -------------------------------------------------------------------------- (1)
Where AT= Ageing temperature
BP= weight % bagasse ash particle
At =Ageing time
The model selected includes the effects of main variables first-order and second-order
interactions of all variables. Hence the general model is written as:
W=βo + β1AT+ β2BP+ β3At+ β4ATBP+ β5ATAt+ β6BPAt + β7ATBPAt---------------------(2)
Where βo is average response of W and β1, β2, β3, β4, β5, β6, β7 are coefficients associated with each
variable AT, BP, At and interaction are calculated using the linear regression method [13].
4. RESULTS AND DISCUSSION
The composition and properties of the bagasse ash used in this study is shown in Table 4.
Table 4. Composition and properties of Bagasse ash particles.
Constituent Formula Cliftonite,(C), Quartz (SiO2,), Moissanite(SiC), Titanium Oxide(Ti6O)
Density and phase 1.95g/cm3 and Solid
Refractoriness 1600oC
Appearance(color) Black-Odorless powder
Size 64µm
Hardness values 75.05 HRB
The results obtained for the ageing behaviour of these composites are presented in Figures 1-3.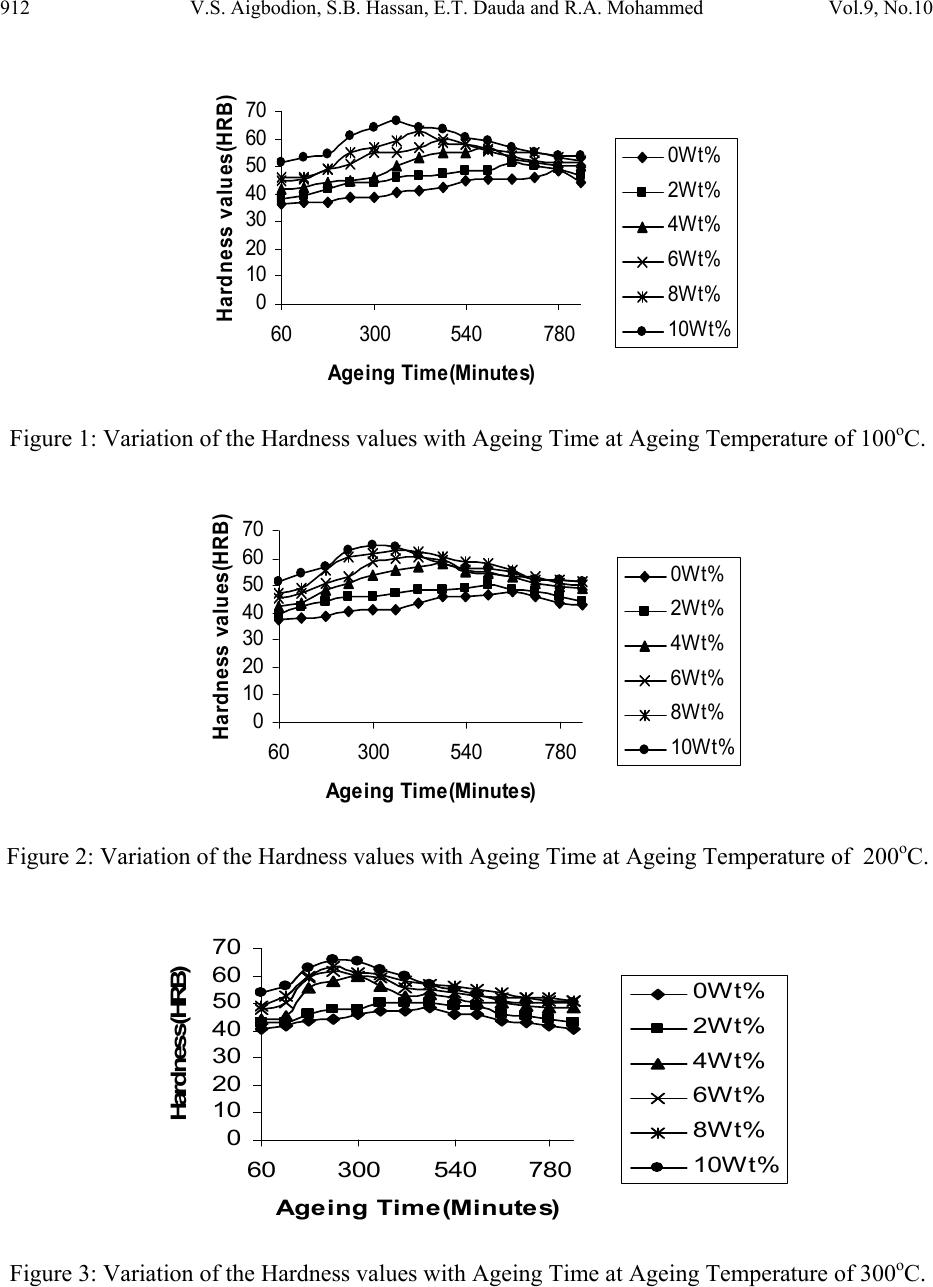

912 V.S. Aigbodion, S.B. Hassan, E.T. Dauda and R.A. Mohammed Vol.9, No.10
0
10
20
30
40
50
60
70
60300 540780
Age i ng Ti m e(Mi nute s)
Hardness valu es(HRB)
0Wt%
2Wt%
4Wt%
6Wt%
8Wt%
10Wt%
Figure 1: Variation of the Hardness values with Ageing Time at Ageing Temperature of 100oC.
0
10
20
30
40
50
60
70
60300 540780
Age i ng Ti m e(Min ute s)
Hardness values(HRB)
0Wt%
2Wt%
4Wt%
6Wt%
8Wt%
10Wt%
Figure 2: Variation of the Hardness values with Ageing Time at Ageing Temperature of 200oC.
0
10
20
30
40
50
60
70
60300540780
Age i ng Ti m e (Minute s)
Hardness(HRB)
0Wt%
2Wt%
4Wt%
6Wt%
8Wt%
10Wt%
Figure 3: Variation of the Hardness values with Ageing Time at Ageing Temperature of 300oC.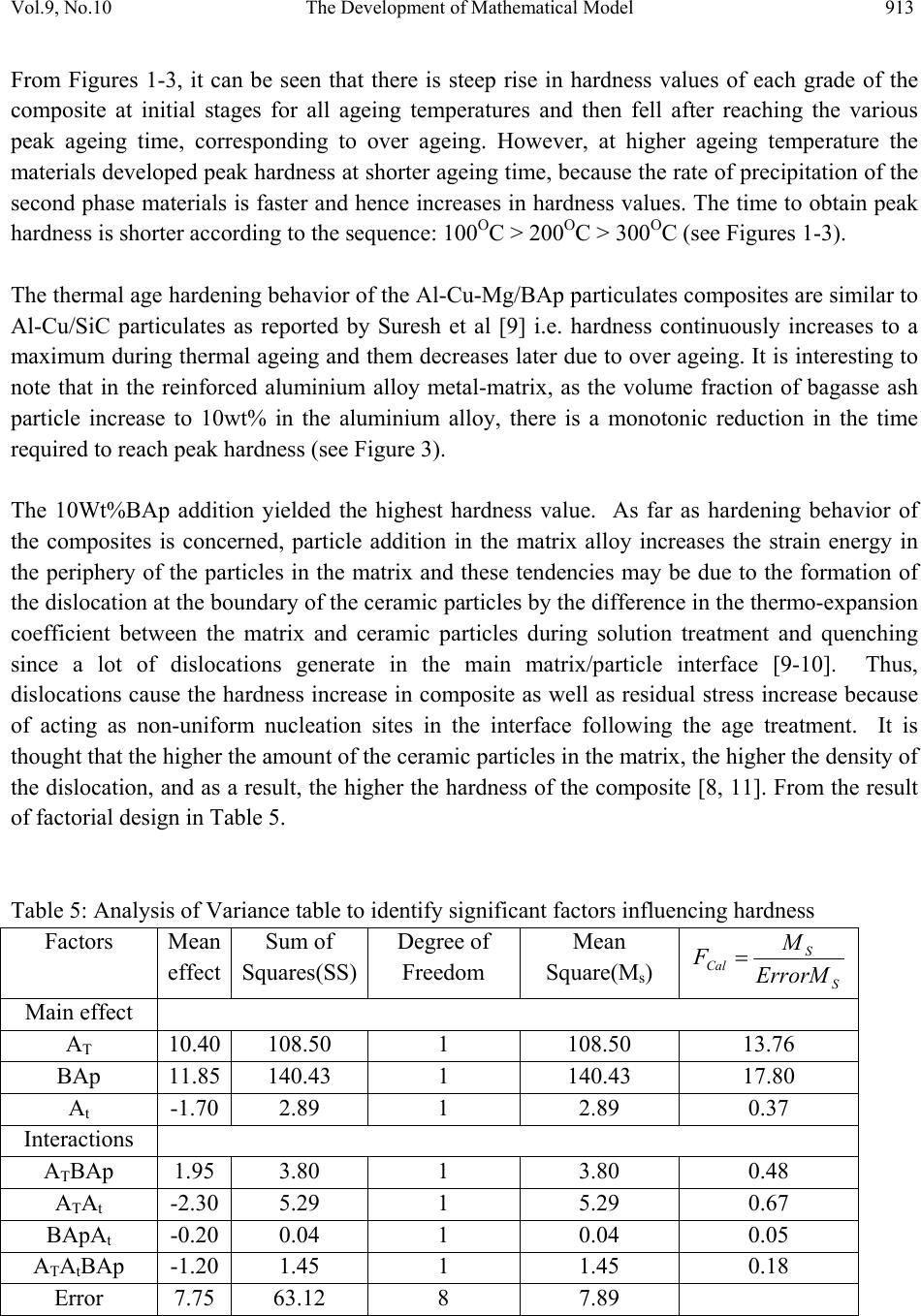

Vol.9, No.10 The Development of Mathematical Model 913
From Figures 1-3, it can be seen that there is steep rise in hardness values of each grade of the
composite at initial stages for all ageing temperatures and then fell after reaching the various
peak ageing time, corresponding to over ageing. However, at higher ageing temperature the
materials developed peak hardness at shorter ageing time, because the rate of precipitation of the
second phase materials is faster and hence increases in hardness values. The time to obtain peak
hardness is shorter according to the sequence: 100OC > 200OC > 300OC (see Figures 1-3).
The thermal age hardening behavior of the Al-Cu-Mg/BAp particulates composites are similar to
Al-Cu/SiC particulates as reported by Suresh et al [9] i.e. hardness continuously increases to a
maximum during thermal ageing and them decreases later due to over ageing. It is interesting to
note that in the reinforced aluminium alloy metal-matrix, as the volume fraction of bagasse ash
particle increase to 10wt% in the aluminium alloy, there is a monotonic reduction in the time
required to reach peak hardness (see Figure 3).
The 10Wt%BAp addition yielded the highest hardness value. As far as hardening behavior of
the composites is concerned, particle addition in the matrix alloy increases the strain energy in
the periphery of the particles in the matrix and these tendencies may be due to the formation of
the dislocation at the boundary of the ceramic particles by the difference in the thermo-expansion
coefficient between the matrix and ceramic particles during solution treatment and quenching
since a lot of dislocations generate in the main matrix/particle interface [9-10]. Thus,
dislocations cause the hardness increase in composite as well as residual stress increase because
of acting as non-uniform nucleation sites in the interface following the age treatment. It is
thought that the higher the amount of the ceramic particles in the matrix, the higher the density of
the dislocation, and as a result, the higher the hardness of the composite [8, 11]. From the result
of factorial design in Table 5.
Table 5: Analysis of Variance table to identify significant factors influencing hardness
Factors Mean
effect
Sum of
Squares(SS)
Degree of
Freedom
Mean
Square(Ms) S
S
Cal ErrorM
M
F
Main effect
AT 10.40 108.50 1 108.50 13.76
BAp 11.85 140.43 1 140.43 17.80
At -1.70 2.89 1 2.89 0.37
Interactions
ATBAp 1.95 3.80 1 3.80 0.48
ATAt -2.30 5.29 1 5.29 0.67
BApAt -0.20 0.04 1 0.04 0.05
ATAtBAp -1.20 1.45 1 1.45 0.18
Error 7.75 63.12 8 7.89

914 V.S. Aigbodion, S.B. Hassan, E.T. Dauda and R.A. Mohammed Vol.9, No.10
The Wt% of BAp appears to be the most important variable with main effect of 11.85HRB
followed by temperature with 10.48HRB and time -1.70HRB. The analysis shows that raising the
temperature from 100 to 300oC would result in increase in hardness values by 10.40HRB, while
allowing ageing to continue from 1 to 14hours would result in decreased hardness values by
1.7HRB and increasing the Wt% of BAp from 2 to 10 would result in increasing the hardness
values by 11.85HRB.
The estimated interactions between temperature and Wt% of BAp; temperature and time; Wt%
of BAp and time and then between the three factors, temperature, Wt% of BAp and time are
1.95HRB, -2.30HRB, -0.20HRB and -1.20HRB respectively. This means that raising
temperature and time would result in decrease in hardness values by 2.30HRB whereas raising
all the three factors would result in decrease in hardness values by 1.2HRB. The main reason for
the decreased in hardness values when time increase from 1 to 14hours is due to the fact that
after peak ageing time has been reached further ageing will not lead to any increases in hardness
as a result of over- ageing [8], this facts can be evident from the Figures 1-3.
The values of Fcalculated (F=Fishers distribution) are compared with Fcritical. F distribution
critical values for {1,8} degrees of freedom are above 5.32 for 95% and above 11.26 for 99%
confidence level [13]. Thus, from Table 5, it can be observed that only bagasse ash and
temperature have significant factor on hardness values, all others factor have no significant
effect. From this statistical analysis, %Wt of BAp and temperature is the most important
parameter in the ageing behaviour of Al-Cu-Mg/BAp particulate composites.
The model equation was obtained after calculating each of the coefficients of Eq. 2. The
developed model equation for the ageing behaviour of the composites can be expressed as:
W=48.55 + 3.63AT+ 4.57BP-1.42At -0.23ATBP -1.8ATAt -3.45BPAt -0.1ATBPAt ------(3)
Substituting the coded values of the variables for any experimental condition in Eq. 3, the
hardness values for the ageing behaviour of the composites can be calculated. Table 6 and Figure
4 show the predicted values along with the actual experimental values in different experimental
conditions.
It is evident from Table 5 and Figure 4 that the actual experimental values are in close proximity
with the predicted values. These facts suggested reasonably good reliability of the equation to
predict the ageing behaviour of the Al-Cu-Mg/BAp composites within the selected experimental
domains.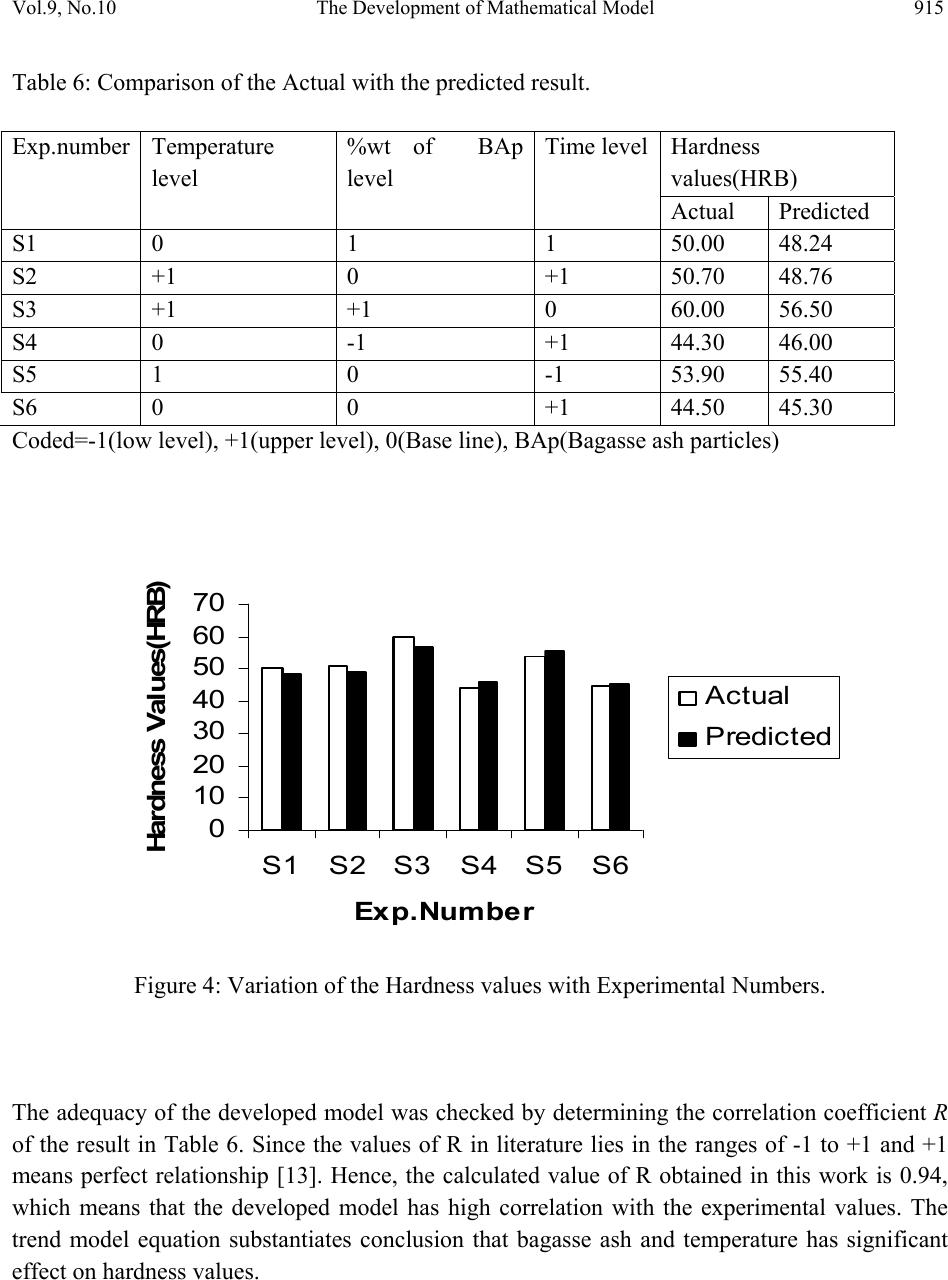

Vol.9, No.10 The Development of Mathematical Model 915
Table 6: Comparison of the Actual with the predicted result.
Exp.number Temperature
level
%wt of BAp
level
Time levelHardness
values(HRB)
Actual Predicted
S1 0 1 1 50.00 48.24
S2 +1 0 +1 50.70 48.76
S3 +1 +1 0 60.00 56.50
S4 0 -1 +1 44.30 46.00
S5 1 0 -1 53.90 55.40
S6 0 0 +1 44.50 45.30
Coded=-1(low level), +1(upper level), 0(Base line), BAp(Bagasse ash particles)
0
10
20
30
40
50
60
70
S1 S2S3 S4S5 S6
Exp.Numb er
Hardness Valu es(H RB)
Actual
Predicted
Figure 4: Variation of the Hardness values with Experimental Numbers.
The adequacy of the developed model was checked by determining the correlation coefficient R
of the result in Table 6. Since the values of R in literature lies in the ranges of -1 to +1 and +1
means perfect relationship [13]. Hence, the calculated value of R obtained in this work is 0.94,
which means that the developed model has high correlation with the experimental values. The
trend model equation substantiates conclusion that bagasse ash and temperature has significant
effect on hardness values.

916 V.S. Aigbodion, S.B. Hassan, E.T. Dauda and R.A. Mohammed Vol.9, No.10
5. CONCLUSIONS
Hardness tests were performed on smooth samples of Al-Cu-Mg/BAp composites subjected to
solution heat treatment and ageing schedule. The individual and interaction effects of the
parameters, viz ageing temperature, bagasse ash and aging time were studied. The conclusions
derived from this study are as follows:
1. At higher ageing temperature the composites developed peak hardness at shorter ageing
time.
2. As the volume fraction of bagasse ash particle increase to 10Wt% in the aluminium
alloy there is a monotonic reduction in the time required to reach peak hardness
3. The main and the interaction effects of significant combination of heat-treatment
parameters within the range of investigation of Al-Cu-Mg/BAp composite can be studied
emphatically by factorial experimentation technique.
4. The developed mathematical model can be used to predict the hardness values in terms of
heat-treatment process parameters obtained from any combinations within the ranges
studied.
5. The bagasse ash and temperature has the maximum influence on hardness values.
6. The results obtained from the statistical analysis are in good agreement with the
experimental findings for the bagasse ash, ageing time and ageing temperatures. It was
found that hardness increases with increasing weight fraction of bagasse ash in the alloy
and decreases with increasing ageing time.
7. The developed mathematical model can be employed for optimization of the process
parameters of Al-Cu-Mg/BAp composites with respect to hardness values.
REFERENCES
[1] V. S. Aigbodion and S. B. Hassan: Effect of Thermal Ageing Characteristics of Al-Si-Fe/SiC
Particulate Composite Synthesized By Double Stir Casting, The Journal of Engineering
Research(TJER), Vol. 7, No. 1, (2010) 53-61
[2] M. Sudarshan, K Surappa: Synthesis of fly ash particle reinforced A356 Al composites and
their characterization, Materials Science and Engineering A, 480(2008)117–124.
[3]. V.S. Aigbodion: Potential of using Bagasse ash particle in Metal Matrix Composite, PhD
Work, Department of Metallurgical and Materials Engineering, Ahmadu Bello University,
Samaru, Zaria, Nigeria (2010).
[4] J. Bienia, M. Walczak, B. Surowska., .J. Sobczaka: Microstructure and Corrosion Behaviour
of Aluminum Fly Ash Composites, Journal of Optoelectronics and Advanced Materials Vol.
5, No. 2(2003)493 – 502.
[5] V.Kevorkijan, B. Utar, M. Torkar, G. Hiarmetta: Preparation of Al Based Composite
Reinforced With Fine Dross Particles, ISSN 1318-0010 KZLTET(1998) 32(6)539.

Vol.9, No.10 The Development of Mathematical Model 917
[6] S. B. Hassan and V. S. Aigbodion: The study of the Microstructure and Interfacial Reaction
of Al-Cu-Mg/Bagasse Ash Particulate Composite, Journal of Alloy & Compounds, 491
(2010) 571–574.
[7] S. Valdez, B. Campillo, R. Pérez, L. Martínez, H. García: Synthesis and microstructural
characterization of Al–Mg alloy–SiC particle composite, Materials Letters 62(2008)2623–
2625.
[8] S.B. Hassan, O. Aponbiede and V.S. Aigbodion: Precipitation hardening characteristics of
Al- Si-Fe/SiC particulate composites Journal of alloys & compounds 466(2008), 268-272
[9] S. Suresh, T. Christman, Y. Sugimura, Accelerated aging in cast Al alloy—SiC particulate
Composite, Scr. Metall. 23 (1989) 1599– 1602.
[10] P. Appendino, C. Badini, F. Marino, A. Tomasi, 6061 Aluminium alloy—SiC particulate
Composite: a comparison between aging behaviour in T4 and T6 treatments, J. Mater. Sci.
Eng. A.135 (1991) 275–279.
[11] L. Salvo, M. Svery, Effect of reinforcement on age-hardening of cast 6061-AlSiC and 6061
Al-Al2O3 particulate composite, Metall. Trans. A 22A (1996) 2553–2653.
[12] Y. Sahin.: The prediction of wear resistance model for the metal matrix composites, Wear
258 (2005) 1717–1722.
[13] I. Miller, J.E. Freund: Probability and Statistics for Engineers, Prentice Hall India Ltd.,
India (2001), 125-140.