Journal of Minerals & Materials Characterization & Engineering, Vol. 8, No.10, pp.765-773, 2009
jmmce.org Printed in the USA. All rights reserved
765
Quadratic Model for Predicting the Hardness of Heat Affected Zone in Water
Cooled Cast Iron Weldment In Relation to Similarly Cooled Aluminum and
Mild Steel Weldments
C. I. Nwoye
Department of Materials and Metallurgical Engineering, Federal University of Technology,
P.M.B 1526, Owerri, Nigeria.
Contact: [e-mail]
ABSTRACT
Quadratic and linear models have been derived for predicting the heat-affected zone (HAZ)
hardness of water cooled cast iron weldment in relation to the combined and respective values of
the heat-affected zone hardness of aluminum and mild steel welded and cooled under the same
conditions. It was found that the validity of the model is rooted on the fractional expression;
γ/3.0749θ + γ/3.0749β + θ/3.0749β = 1. The respective deviations of the model-predicted heat-
affected zone hardness values of aluminum, cast iron and mild steel from the corresponding
experimental values were less than 0.01% which is quite insignificant, indicating reliability of
the model.
Keywords: Model, Hardness, Heat Affected Zone, Cast Iron Weldments, Aluminum, Mild Steel.
1. INTRODUCTION
Research reports [1, 2] have shown that there are several processes and methods of arc welding
including carbon-arc welding, atomic hydrogen welding, shielded metal arc welding, plasma arc
welding, electroslag welding. It has been demonstrated that arc welding involves the process
where by the heat generated by the electric arc is maintained in most cases between the
electrodes and the work piece [3]. The quantity of heat required for melting the base metal in the
vicinity of the arc and also the electrode is supplied by the arc. In arc welding, some of the
processes utilize consumable electrodes which serve to strike an arc onto the work pieces, and
they melt to provide the weld metal. In recent times, advancement has been made in such joining
processes as adhesives, mechanical fasteners, brazing soldering [4]. However, welding remains
the most important metal joining process.
It is generally believed that arc welding is the most widely used fusion welding process. It
produces smooth welding surfaces and utilizes both direct and alternating current. Oxidation is
minimal as weld metal is completely shielded from the atmosphere. The process is excellent
welding low carbon, medium carbon and alloy steels. The arc is quiet, discomfort from glare or

766 C. I. Nwoye Vol.8, No.10
fume is minimal, and is applicable in fabricating vessels, boilers and pipes, etc. Disadvantages of
the process include need for very high current for welding operations and formation of a crater in
the molten metal of the work piece arising from the pressure produced by the stream of ions
flowing from the cathode [2]. Electrodes are the elements of an arc lamp or furnace between
which an arc is struck. They are filler materials which a joining engineer should be able to match
with the parent material to avoid failure [1]. Uncoated electrodes produce an atmosphere of
oxygen and nitrogen, so that the oxides and nitrides formed may be in the weld metal, thus
impairing ductility and impact toughness in the weld. The situation is avoided by use of coated
electrodes, which contains slag and so form a fluid covering over the weld [2]. In this case,
stabilization of the arc is achieved by including materials which would produce ionization and
consequently may be wielded by the metallic arc process. In welding carbon and low carbon
steels, coated electrodes are used especially for low carbon steels but for alloy steels in which
martensite occurrence is likely on cooling and formation of hydrogen embrittlement expected,
the electrode coating must be free from hydrogen forming cellulose [5].
Cracking of weldment has been found [6] to be one of the reasons for low mechanical properties
such as hardness and impact strength in welded parts. Adjacent to the immediate welded area or
fusion zone is the heat affected zone [6]. The mechanical property of main importance in HAZ is
the hardness since it gives an indication of the degree of embrittlement there. Studies [7] have
shown that the heat affected zone hardness produced by any given welding operation depends on
the cooling rate experienced by the HAZ. Too rapid rate of cooling favours the formation of hard
and brittle martensite in all the sub zones of the HAZ or increases the martensite region in size
relative to the other regions. The presence of martensite in the HAZ results in a very high
hardness value for the heat affected zone. Slow cooling favours a better microstructure needed
for engineering applications. Also, the more rapid the quenching rate, the greater the HAZ
hardness.
Several literatures have reported studies carried out on different joining processes and methods,
but no emphasis has been placed on the derivation of models for prediction or evaluation of the
hardness of the heat affected zone (HAZ) in weldments cooled in different media; evaluation of
the hardness of HAZ cooled in a particular medium as a function of the hardness of HAZ from
the same material but cooled in different media. Researches carried out on HAZ; its cooling and
mechanical properties have not addressed the issue of predicting or evaluating the hardness of
the HAZ of a material cooled in a particular medium by simple substitution of the value of the
hardness of HAZ from the same material, but cooled in different media. The hardness of HAZ in
aluminum, cast iron and mild steel cooled in kerosine was found to be exactly the same as the
hardness value of the same materials cooled in groundnut oil [8]. This implies that
HG = HK (1)
Where
HG = Hardness of HAZ cooled in groundnut oil
HK = Hardness of HAZ cooled kerosine
It has been reported [8] that 8-10% less hardness than that from water occurs when kerosine or
groundnut oil is used as quenchant for HAZ. He discovered that quenching the HAZ with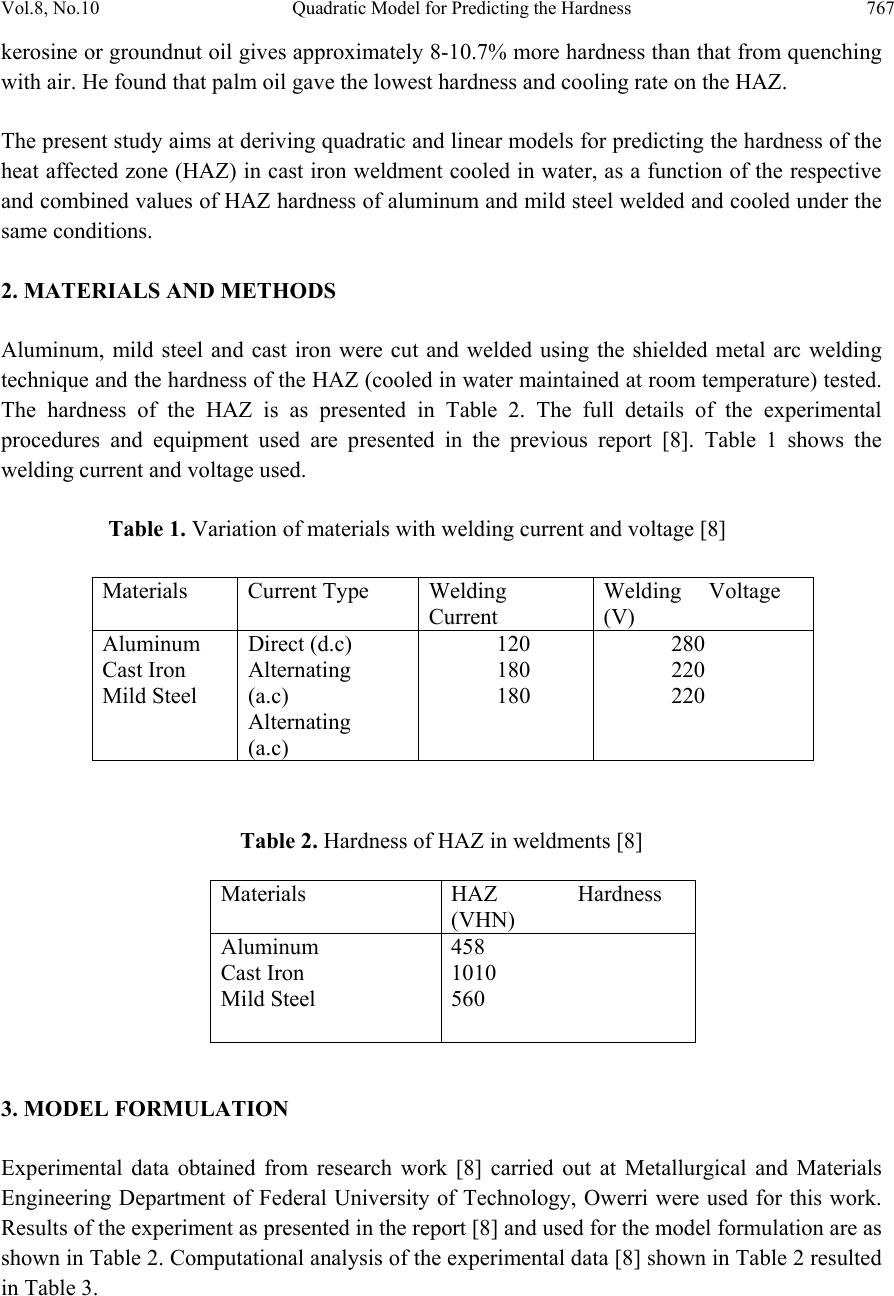

Vol.8, No.10 Quadratic Model for Predicting the Hardness 767
kerosine or groundnut oil gives approximately 8-10.7% more hardness than that from quenching
with air. He found that palm oil gave the lowest hardness and cooling rate on the HAZ.
The present study aims at deriving quadratic and linear models for predicting the hardness of the
heat affected zone (HAZ) in cast iron weldment cooled in water, as a function of the respective
and combined values of HAZ hardness of aluminum and mild steel welded and cooled under the
same conditions.
2. MATERIALS AND METHODS
Aluminum, mild steel and cast iron were cut and welded using the shielded metal arc welding
technique and the hardness of the HAZ (cooled in water maintained at room temperature) tested.
The hardness of the HAZ is as presented in Table 2. The full details of the experimental
procedures and equipment used are presented in the previous report [8]. Table 1 shows the
welding current and voltage used.
Table 1. Variation of materials with welding current and voltage [8]
Table 2. Hardness of HAZ in weldments [8]
3. MODEL FORMULATION
Experimental data obtained from research work [8] carried out at Metallurgical and Materials
Engineering Department of Federal University of Technology, Owerri were used for this work.
Results of the experiment as presented in the report [8] and used for the model formulation are as
shown in Table 2. Computational analysis of the experimental data [8] shown in Table 2 resulted
in Table 3.
Materials Current Type Welding
Current
Welding Voltage
(V)
Aluminum
Cast Iron
Mild Steel
Direct (d.c)
Alternating
(a.c)
Alternating
(a.c)
120
180
180
280
220
220
Materials HAZ Hardness
(VHN)
Aluminum
Cast Iron
Mild Steel
458
1010
560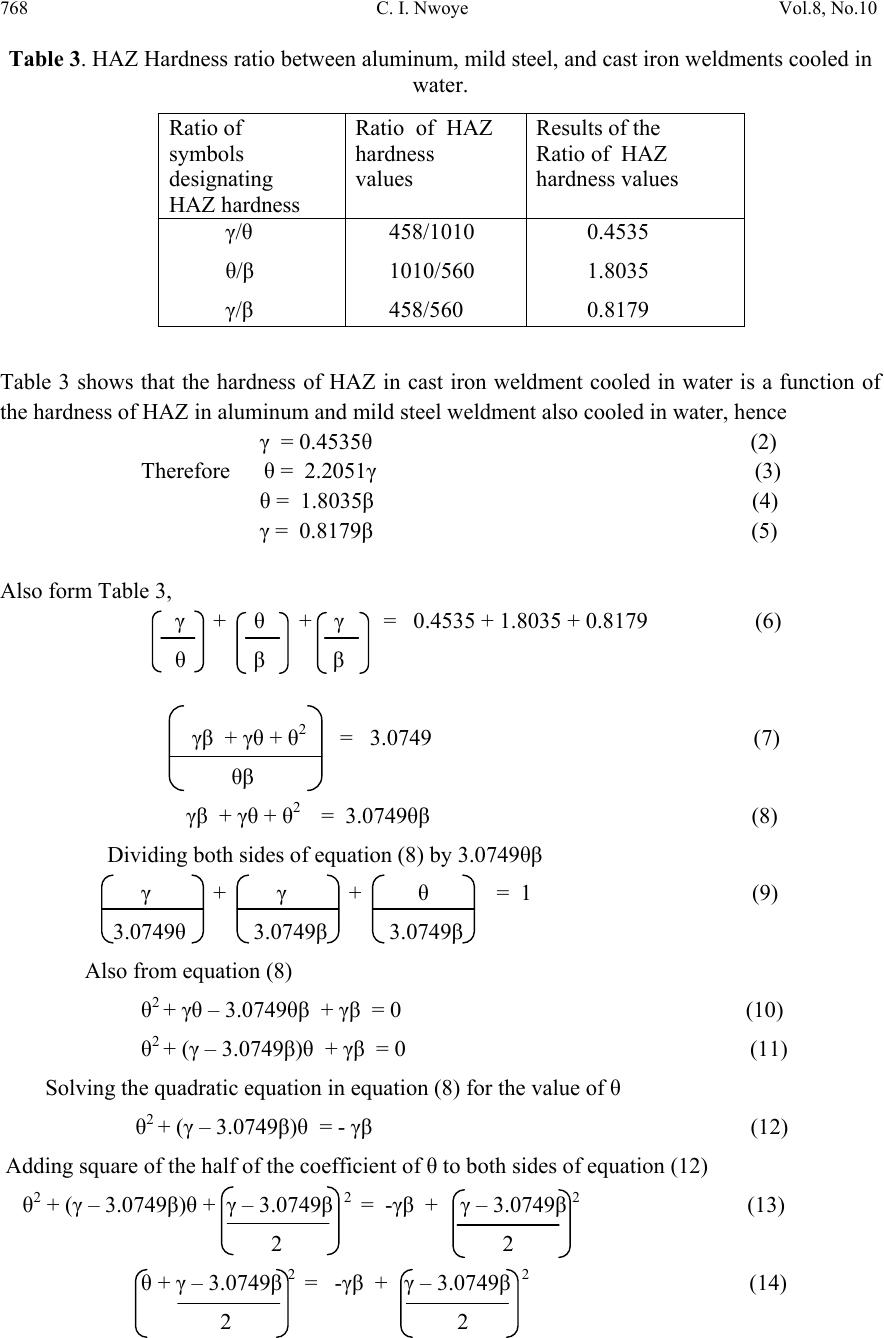

768 C. I. Nwoye Vol.8, No.10
Table 3. HAZ Hardness ratio between aluminum, mild steel, and cast iron weldments cooled in
water.
Table 3 shows that the hardness of HAZ in cast iron weldment cooled in water is a function of
the hardness of HAZ in aluminum and mild steel weldment also cooled in water, hence
γ = 0.4535θ (2)
Therefore θ = 2.2051γ (3)
θ = 1.8035β (4)
γ = 0.8179β (5)
Also form Table 3,
γ + θ + γ = 0.4535 + 1.8035 + 0.8179 (6)
θ β β
γβ + γθ + θ2 = 3.0749 (7)
θβ
γβ + γθ + θ2 = 3.0749θβ (8)
Dividing both sides of equation (8) by 3.0749θβ
γ + γ + θ = 1 (9)
3.0749θ 3.0749β 3.0749β
Also from equation (8)
θ2 + γθ – 3.0749θβ + γβ = 0 (10)
θ2 + (γ – 3.0749β)θ + γβ = 0 (11)
Solving the quadratic equation in equation (8) for the value of θ
θ2 + (γ – 3.0749β)θ = - γβ (12)
Adding square of the half of the coefficient of θ to both sides of equation (12)
θ2 + (γ – 3.0749β)θ + γ – 3.0749β 2 = -γβ + γ – 3.0749β 2 (13)
2 2
θ + γ – 3.0749β 2 = -γβ + γ – 3.0749β 2 (14)
2 2
Ratio of
symbols
designating
HAZ hardness
Ratio of HAZ
hardness
values
Results of the
Ratio of HAZ
hardness values
γ/θ
θ/β
γ/β
458/1010
1010/560
458/560
0.4535
1.8035
0.8179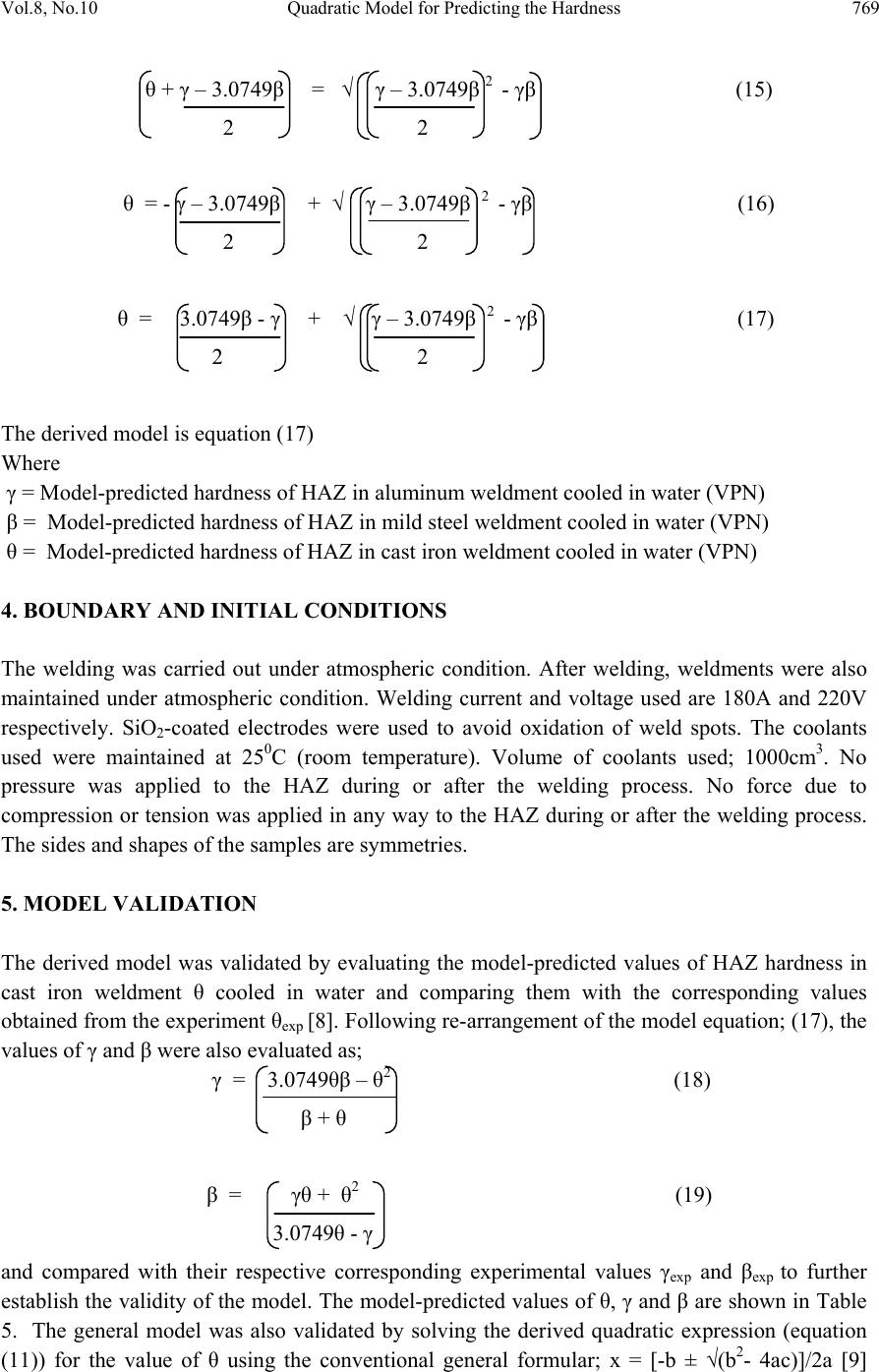

Vol.8, No.10 Quadratic Model for Predicting the Hardness 769
θ + γ – 3.0749β = √ γ – 3.0749β 2 - γβ (15)
2 2
θ = - γ – 3.0749β + √ γ – 3.0749β 2 - γβ (16)
2 2
θ = 3.0749β - γ + √ γ – 3.0749β 2 - γβ (17)
2 2
The derived model is equation (17)
Where
γ = Model-predicted hardness of HAZ in aluminum weldment cooled in water (VPN)
β = Model-predicted hardness of HAZ in mild steel weldment cooled in water (VPN)
θ = Model-predicted hardness of HAZ in cast iron weldment cooled in water (VPN)
4. BOUNDARY AND INITIAL CONDITIONS
The welding was carried out under atmospheric condition. After welding, weldments were also
maintained under atmospheric condition. Welding current and voltage used are 180A and 220V
respectively. SiO2-coated electrodes were used to avoid oxidation of weld spots. The coolants
used were maintained at 250C (room temperature). Volume of coolants used; 1000cm3. No
pressure was applied to the HAZ during or after the welding process. No force due to
compression or tension was applied in any way to the HAZ during or after the welding process.
The sides and shapes of the samples are symmetries.
5. MODEL VALIDATION
The derived model was validated by evaluating the model-predicted values of HAZ hardness in
cast iron weldment θ cooled in water and comparing them with the corresponding values
obtained from the experiment θexp [8]. Following re-arrangement of the model equation; (17), the
values of γ and β were also evaluated as;
γ = 3.0749θβ – θ2 (18)
β + θ
β = γθ + θ2 (19)
3.0749θ - γ
and compared with their respective corresponding experimental values γexp and βexp to further
establish the validity of the model. The model-predicted values of θ, γ and β are shown in Table
5. The general model was also validated by solving the derived quadratic expression (equation
(11)) for the value of θ using the conventional general formular; x = [-b ± √(b2- 4ac)]/2a [9]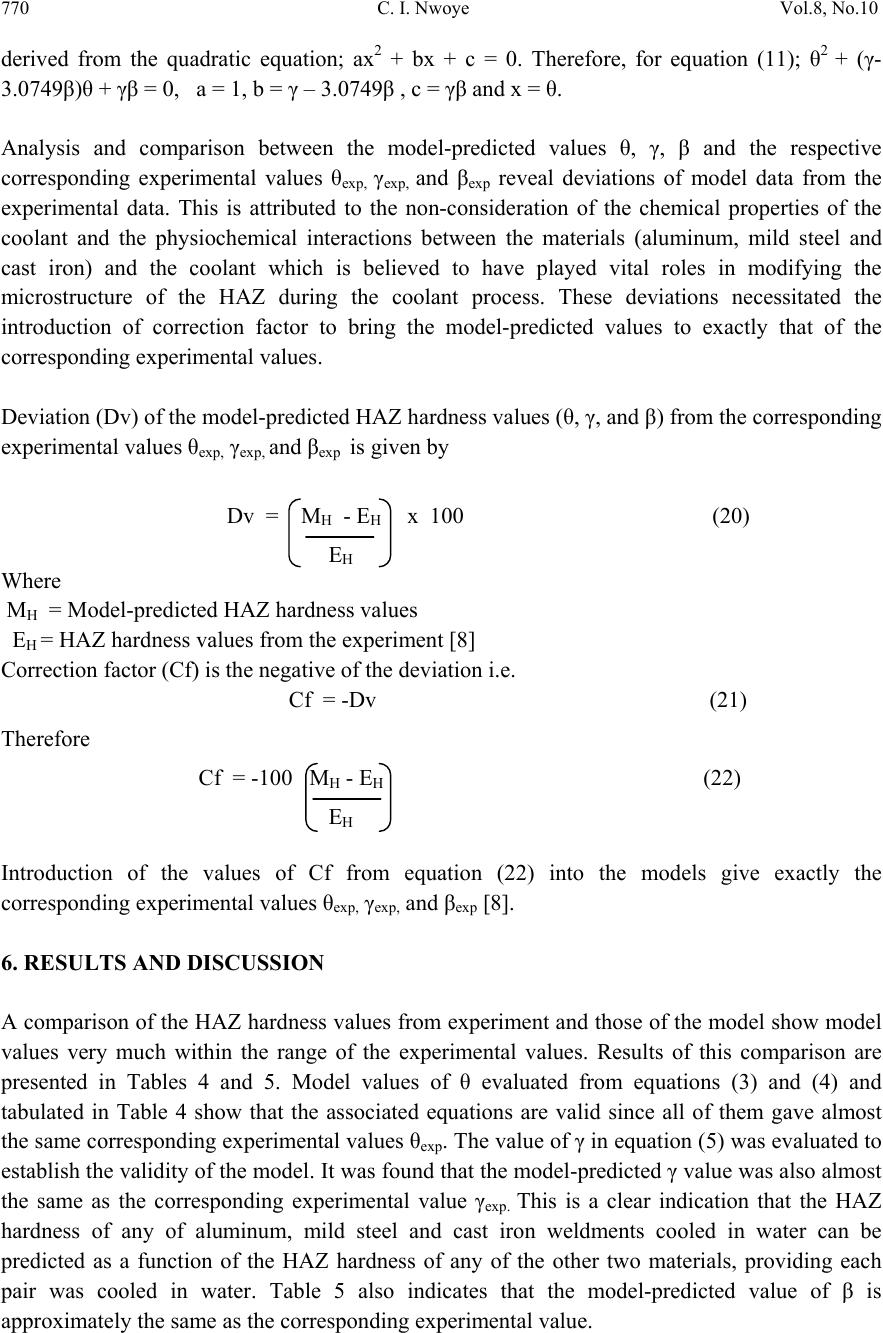

770 C. I. Nwoye Vol.8, No.10
derived from the quadratic equation; ax2 + bx + c = 0. Therefore, for equation (11); θ2 + (γ-
3.0749β)θ + γβ = 0, a = 1, b = γ – 3.0749β , c = γβ and x = θ.
Analysis and comparison between the model-predicted values θ, γ, β and the respective
corresponding experimental values θexp, γexp, and βexp reveal deviations of model data from the
experimental data. This is attributed to the non-consideration of the chemical properties of the
coolant and the physiochemical interactions between the materials (aluminum, mild steel and
cast iron) and the coolant which is believed to have played vital roles in modifying the
microstructure of the HAZ during the coolant process. These deviations necessitated the
introduction of correction factor to bring the model-predicted values to exactly that of the
corresponding experimental values.
Deviation (Dv) of the model-predicted HAZ hardness values (θ, γ, and β) from the corresponding
experimental values θexp, γexp, and βexp is given by
Dv = MH - EH x 100 (20)
EH
Where
MH = Model-predicted HAZ hardness values
EH = HAZ hardness values from the experiment [8]
Correction factor (Cf) is the negative of the deviation i.e.
Cf = -Dv (21)
Therefore
Cf = -100 MH - EH (22)
EH
Introduction of the values of Cf from equation (22) into the models give exactly the
corresponding experimental values θexp, γexp, and βexp [8].
6. RESULTS AND DISCUSSION
A comparison of the HAZ hardness values from experiment and those of the model show model
values very much within the range of the experimental values. Results of this comparison are
presented in Tables 4 and 5. Model values of θ evaluated from equations (3) and (4) and
tabulated in Table 4 show that the associated equations are valid since all of them gave almost
the same corresponding experimental values θexp. The value of γ in equation (5) was evaluated to
establish the validity of the model. It was found that the model-predicted γ value was also almost
the same as the corresponding experimental value γexp. This is a clear indication that the HAZ
hardness of any of aluminum, mild steel and cast iron weldments cooled in water can be
predicted as a function of the HAZ hardness of any of the other two materials, providing each
pair was cooled in water. Table 5 also indicates that the model-predicted value of β is
approximately the same as the corresponding experimental value.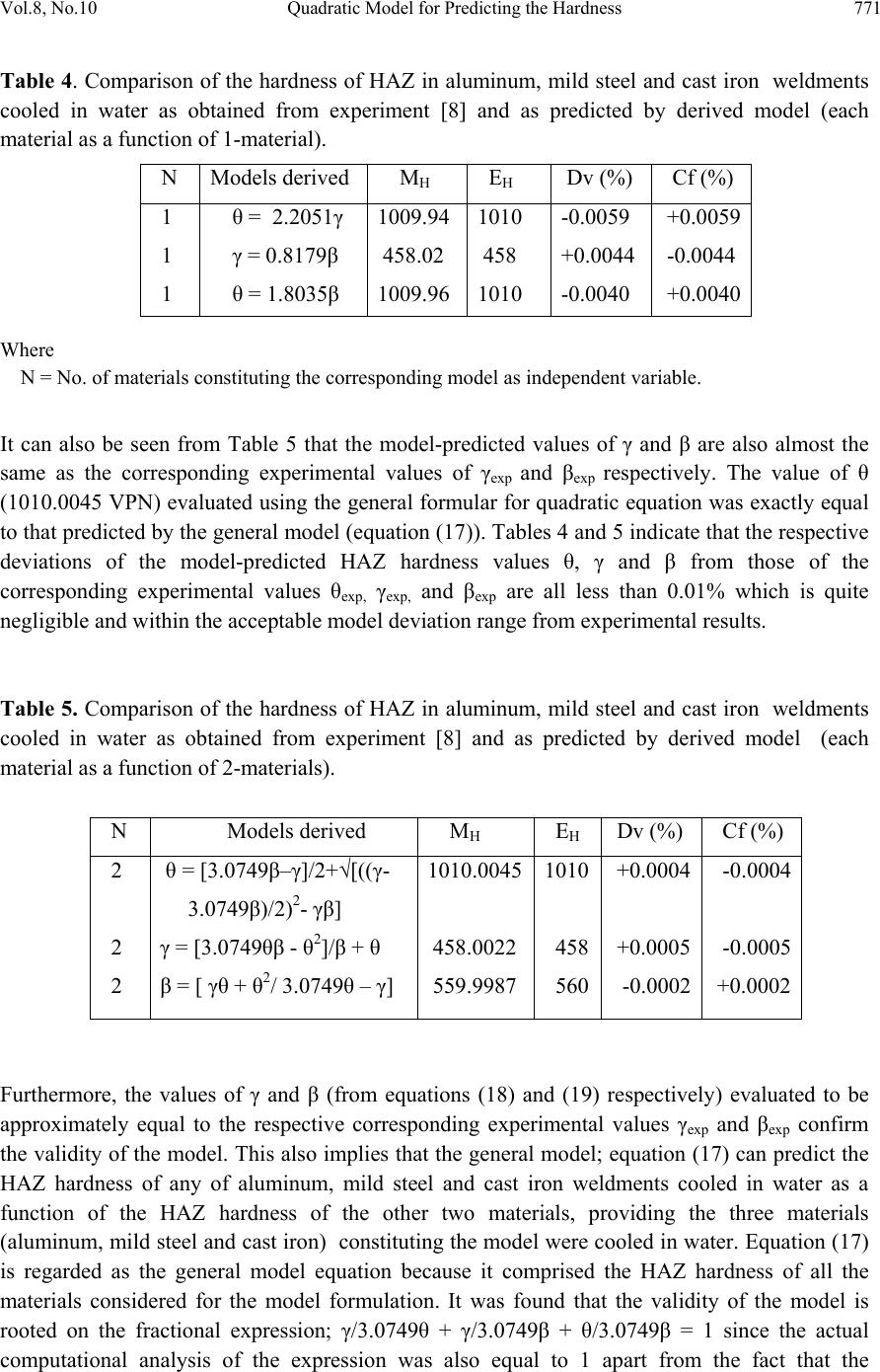

Vol.8, No.10 Quadratic Model for Predicting the Hardness 771
Table 4. Comparison of the hardness of HAZ in aluminum, mild steel and cast iron weldments
cooled in water as obtained from experiment [8] and as predicted by derived model (each
material as a function of 1-material).
Where
N = No. of materials constituting the corresponding model as independent variable.
It can also be seen from Table 5 that the model-predicted values of γ and β are also almost the
same as the corresponding experimental values of γexp and βexp respectively. The value of θ
(1010.0045 VPN) evaluated using the general formular for quadratic equation was exactly equal
to that predicted by the general model (equation (17)). Tables 4 and 5 indicate that the respective
deviations of the model-predicted HAZ hardness values θ, γ and β from those of the
corresponding experimental values θexp, γexp, and βexp are all less than 0.01% which is quite
negligible and within the acceptable model deviation range from experimental results.
Table 5. Comparison of the hardness of HAZ in aluminum, mild steel and cast iron weldments
cooled in water as obtained from experiment [8] and as predicted by derived model (each
material as a function of 2-materials).
Furthermore, the values of γ and β (from equations (18) and (19) respectively) evaluated to be
approximately equal to the respective corresponding experimental values γexp and βexp confirm
the validity of the model. This also implies that the general model; equation (17) can predict the
HAZ hardness of any of aluminum, mild steel and cast iron weldments cooled in water as a
function of the HAZ hardness of the other two materials, providing the three materials
(aluminum, mild steel and cast iron) constituting the model were cooled in water. Equation (17)
is regarded as the general model equation because it comprised the HAZ hardness of all the
materials considered for the model formulation. It was found that the validity of the model is
rooted on the fractional expression; γ/3.0749θ + γ/3.0749β + θ/3.0749β = 1 since the actual
computational analysis of the expression was also equal to 1 apart from the fact that the
N Models derived MH EH Dv (%) Cf (%)
1
1
1
θ = 2.2051γ
γ = 0.8179β
θ = 1.8035β
1009.94
458.02
1009.96
1010
458
1010
-0.0059
+0.0044
-0.0040
+0.0059
-0.0044
+0.0040
N Models derived MH EH Dv (%) Cf (%)
2
2
2
θ = [3.0749β–γ]/2+√[((γ-
3.0749β)/2)2- γβ]
γ = [3.0749θβ - θ2]/β + θ
β = [ γθ + θ2/ 3.0749θ – γ]
1010.0045
458.0022
559.9987
1010
458
560
+0.0004
+0.0005
-0.0002
-0.0004
-0.0005
+0.0002

772 C. I. Nwoye Vol.8, No.10
expression comprised the three metallic materials. Based on the foregoing, the models in
equations (3), (4) and (17) are valid and very useful for predicting HAZ hardness of aluminum,
mild steel and cast iron weldments cooled in water depending on the material of interest and the
given HAZ hardness values for the other materials. The general model (equation (17)) was also
found to give lesser magnitude of deviation from experimental HAZ hardness values and is
therefore preferred to other derived models (equations (3) and (4)). However, the latter models
will be much useful if the HAZ hardness is expected to be predicted in relation to just one
material which may be either aluminum, mild steel or cast iron.
7. CONCLUSION
The derived models; θ = 2.2051γ and θ = 1.8035β can predict the HAZ hardness of cast iron
weldment cooled in water as a function of the HAZ hardness of aluminum or mild steel welded
and cooled under the same conditions. Similarly, the general model; θ = [3.0749β – γ]/2 + √[((γ-
3.0749β)/2)2- γβ] can predict the HAZ hardness of cast iron weldment cooled in water as a
function of the HAZ hardness of both aluminum and mild steel welded and cooled under the
same conditions. Furthermore, re-arrangement of these models could be done to evaluate the
HAZ hardness of aluminum and mild steel respectively as in the case of cast iron. The validity of
the model was rooted on the fractional expression; γ/3.0749θ + γ/3.0749β + θ/3.0749β = 1 since
the actual computational analysis of the expression was also equal to 1. The respective deviations
of the model-predicted HAZ hardness values θ, γ, and β from the corresponding experimental
values θexp γexp and βexp was less 0.01% indicating the reliability and validity of the model.
ACKNOWLEDGEMENT
The author thanks the management of Federal University of Technology, Owerri for providing
the equipment used for this work.
REFERENCES
[1] Davies, A. C., (1993). The Science and Practice of Welding, 10th Edition, Cambridge
University
Press, p521.
[2] Higgins, R. A., (1994). Engineering Metallurgy, Arc Welding Processes, 2nd Edition, Edward
Arnold Publishers, London.p57.
[3] Lancaster, J. F., (1993). Metallurgy of Welding 5th Edition, London. Chapman and Hall
p389.
[4] Callister, W.D., (Jnr.) (1996). Material Science and Engineering. An introduction, 1st Edition,
Sydney John Wiley & Sons inc. p852.
[5] Higgins, R. A., (1977). Engineering Metallurgy Part II, 2nd Edition, London, ELBS, p466.
[6] Nnuka, E. E., Ovat, F. A., and Oseni, M. I., (2008). Effect of Electrode Types on the
Mechanical Properties and Structure of Welded Steel Joints. JMME Vol. 3, No. 1.
[7] Lancaster, J. F., (1987). Metallurgy of Welding: The Mechanical Properties of the Heat
Affected Zone, 4th Edition, Allen and University, London. p45.

Vol.8, No.10 Quadratic Model for Predicting the Hardness 773
[8] Nwoye, C. I. (2008). Comparative Studies of the Cooling Ability of Hydrocarbon Based
Media and their Effects on the Hardness of the Heat Affected Zone (HAZ) in Weldments,
JMME, Vol.3, No.1
[9] Stroud, K. A., (1986). Further Engineering Mathematics. 1st edition, Macmillan
Education, London. p47.