Applied Mathematics
Vol.3 No.12(2012), Article ID:25595,5 pages DOI:10.4236/am.2012.312270
Integral Inequalities of Hermite-Hadamard Type for r-Convex Functions
College of Mathematics, Inner Mongolia University for Nationalities, Tongliao, China
Email: hlx2980@163.com
Received September 17, 2012; revised October 17, 2012; accepted October 25, 2012
Keywords: Hermite-Hadamard Integral Inequality; r -Convex Function; Logarithmic Mean; Stolarsky Mean
ABSTRACT
The main aim of this present note is to establish three new Hermite-Hadamard type integral inequalities for r-convex functions. The three new Hermite-Hadamard type integral inequalities for r-convex functions improve the result of original one by Hölder’s integral inequality, Stolarsky mean and convexity of function.
1. Introduction
The inequalities
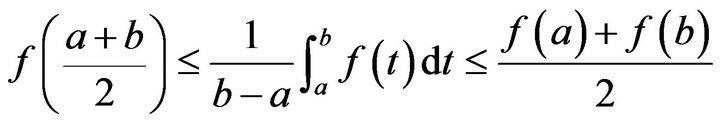 , (1.1)
, (1.1)
which discovered by C. Hermite and Hadamard for all convex functions 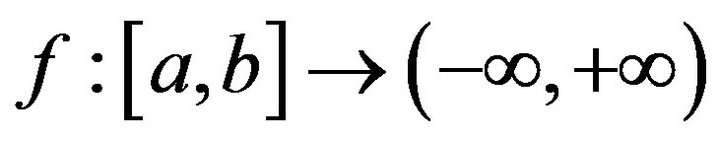 are known in the literature as Hermite-Hadamard inequalities.
are known in the literature as Hermite-Hadamard inequalities.
We note that Hermite-Hadamard inequalities may be regarded as a refinement of the concept of convexity and they follows easily from Jenson’s inequality. HermiteHadamard inequalities for convex functions has received renewed attention in recent years and a remarkable variety of refinements and generalizations have been found in [1-6].
Let 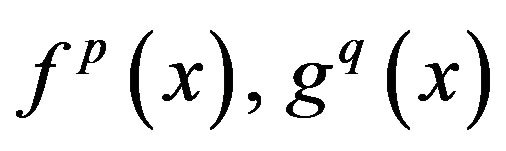 be integrable functions on
be integrable functions on
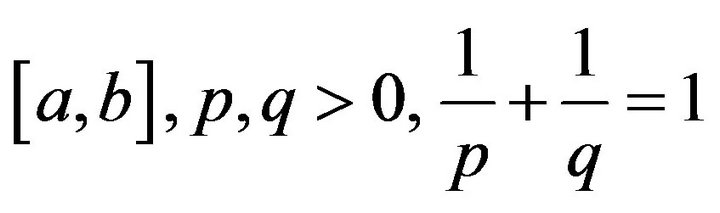 then the well known Hölder’s integral inequality is given as
then the well known Hölder’s integral inequality is given as
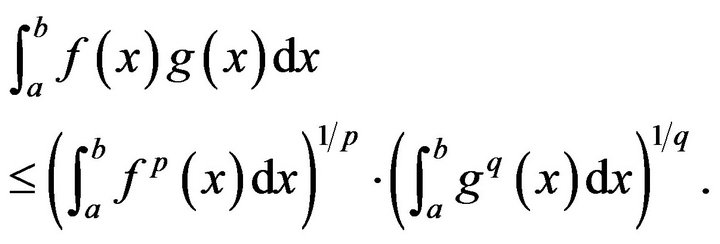 (1.2)
(1.2)
The following definition is well known in the literature.
Definition 1.1. Suppose
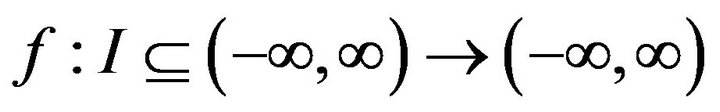 .
.
If following inequality holds
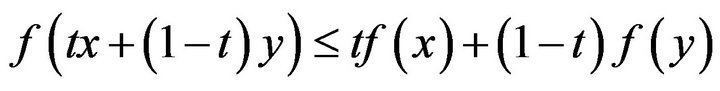 (1.3)
(1.3)
for any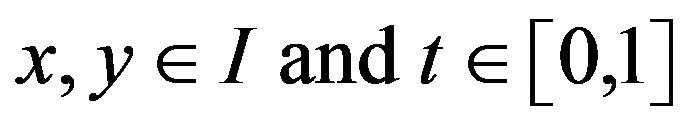 , then we say
, then we say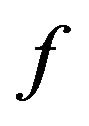 is convex function on
is convex function on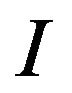 .
.
In [1], C. E. M. Pearce, J. Pecaric and V. Simic introduced the definition of 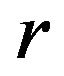 -convex function and studied the inequalities of Hermite-Hadamard type for
-convex function and studied the inequalities of Hermite-Hadamard type for 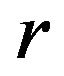 -convex functions.
-convex functions.
Definition 1.2. ([1]) A function
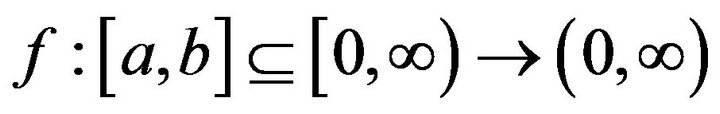
is said to be 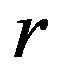 -convex function on
-convex function on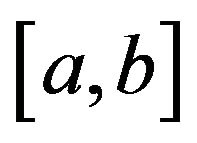 , if
, if
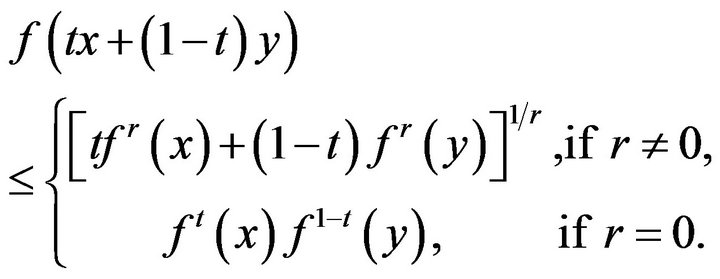 (1.4)
(1.4)
holds for any 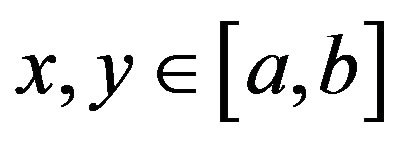 and
and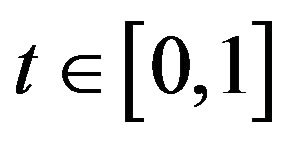 .
.
We have that 0-convex functions are simply log-convex functions and 1-convex functions are ordinary convex functions.
The integral power mean 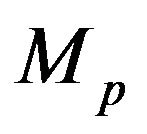 (see [2]) of a positive function
(see [2]) of a positive function 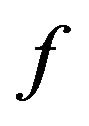 on
on  is a functional given by
is a functional given by
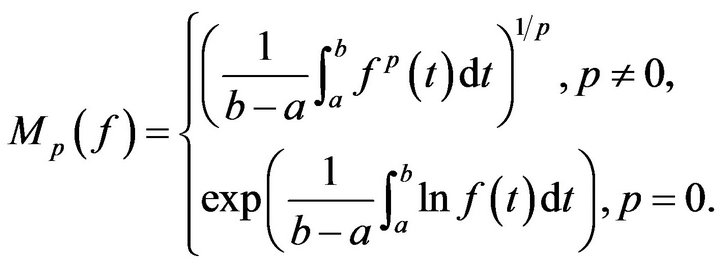 (1.5)
(1.5)
The Stolarsky mean 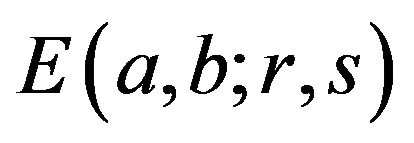 (see [7]) of two positive numbers
(see [7]) of two positive numbers 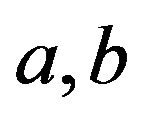 is given by
is given by
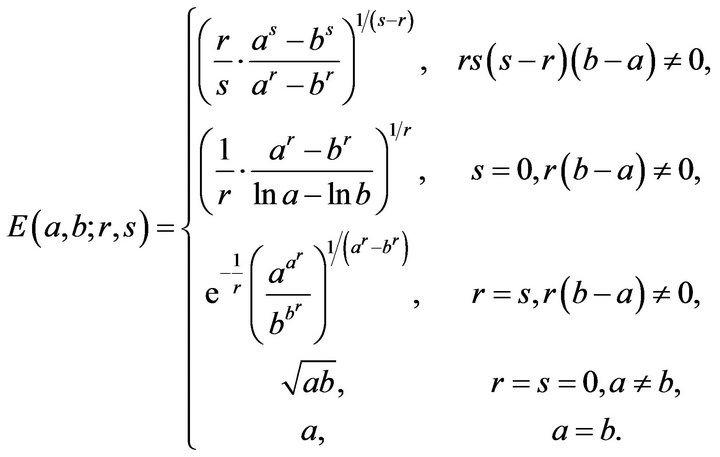 (1.6)
(1.6)
In [2], following theorem is given.
Theorem 1.1. ([2]) Let 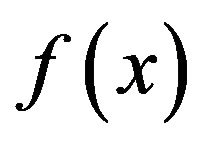 be a positive
be a positive 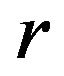 -convex function on
-convex function on 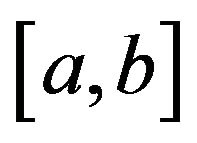 and
and 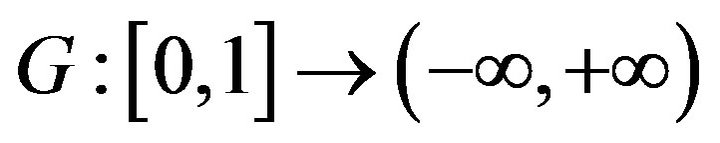 is defined by
is defined by
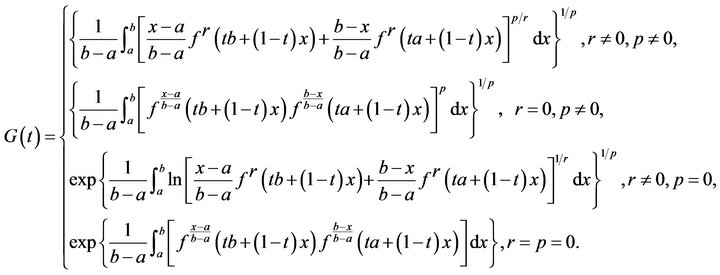 (1.7)
(1.7)
Then
(i) 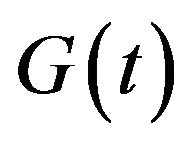 is monotonically increasing on
is monotonically increasing on ;
;
(ii)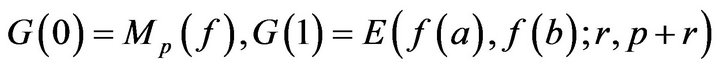 .
.
In [4], following theorems are given.
Theorem 1.2. ([3]) Let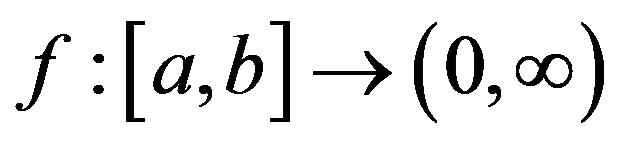 be
be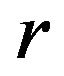 -convex function on
-convex function on 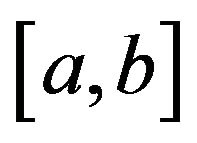 with
with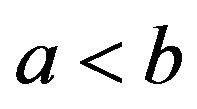 . Then the following inequality holds for
. Then the following inequality holds for 
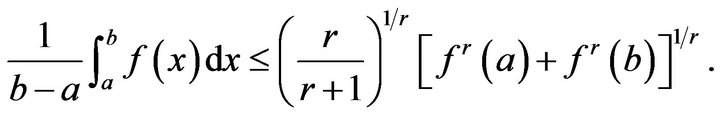
Theorem 1.3. ([3]) Let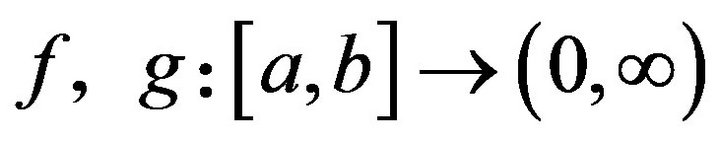 be
be 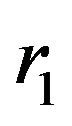 - convex and
- convex and ![]() -convex functions respectively on
-convex functions respectively on 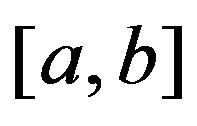 with
with 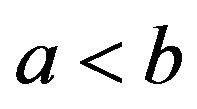 Then the following inequality holds for
Then the following inequality holds for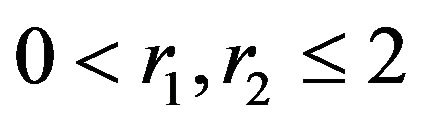 ,
,
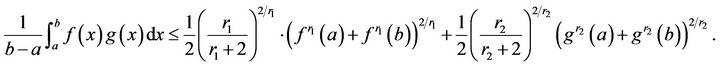
Theorem 1.4. ([3]) Let 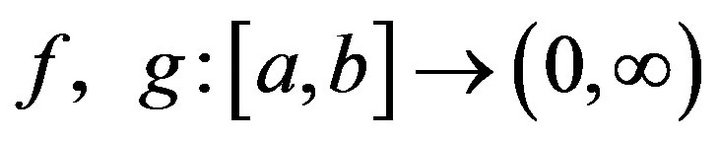 be
be 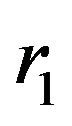 convex and
convex and ![]() -convex functions respectively on
-convex functions respectively on 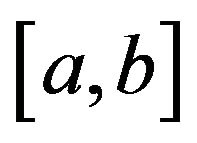 with
with  Then the following inequality holds
Then the following inequality holds
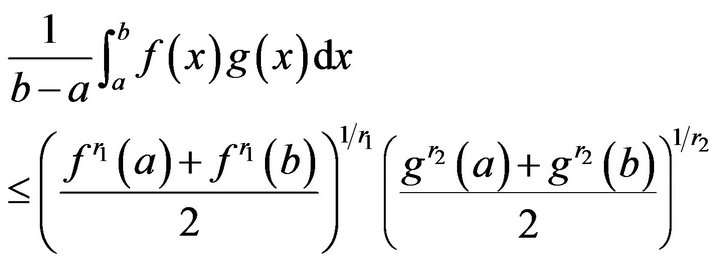
for 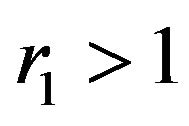 and
and 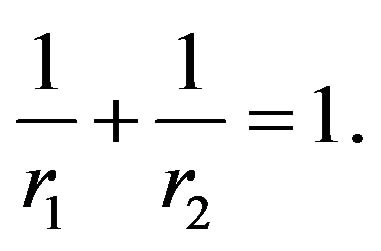
2. Main Results
In this paper we obtain some new Hermite-Hadamard type integral inequalities for 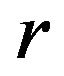 -convex functions and improve the results of Theorems 1.2-1.4.
-convex functions and improve the results of Theorems 1.2-1.4.
The following are extensions of Hermite-Hadamard type inequality:
Theorem 2.1. Let 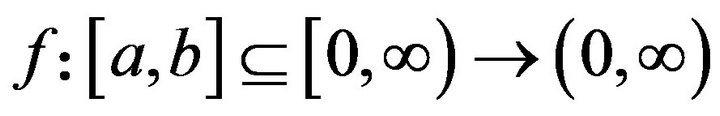 be
be 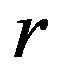 - convex function on
- convex function on 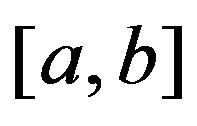 with
with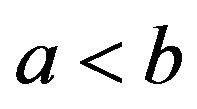 ,
,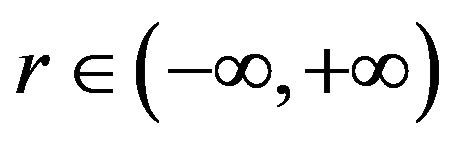 . Then
. Then
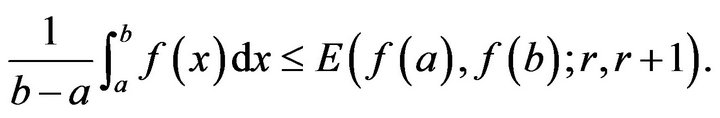 (2.1)
(2.1)
Proof. Let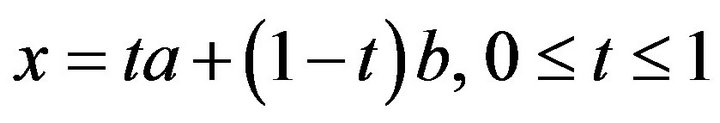 , then
, then
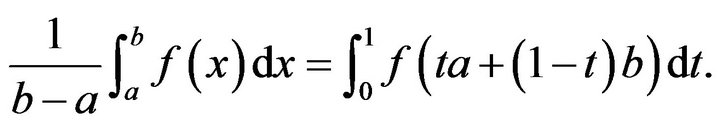
If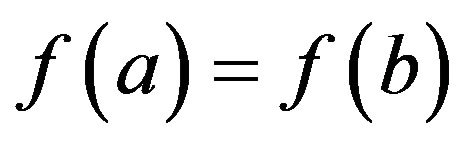 , by the
, by the 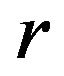 -convexity of
-convexity of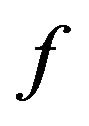 , we have
, we have
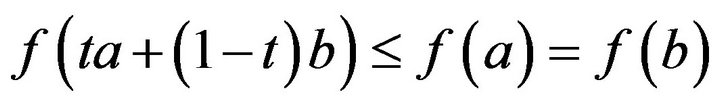
for any 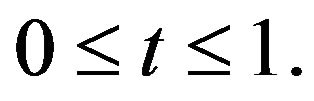 So the conclusion is valid.
So the conclusion is valid.
If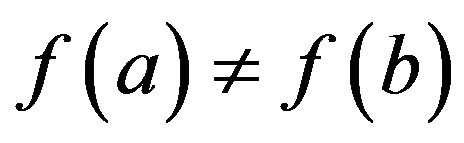 , we have to discuss three cases as following:
, we have to discuss three cases as following:
Case 1. If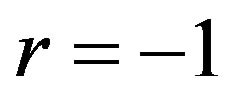 , we have
, we have
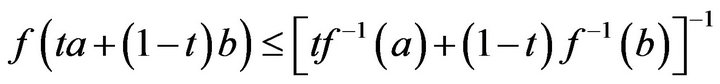
for any 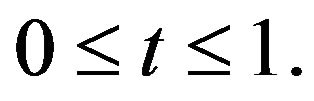 Hence, we obtain
Hence, we obtain
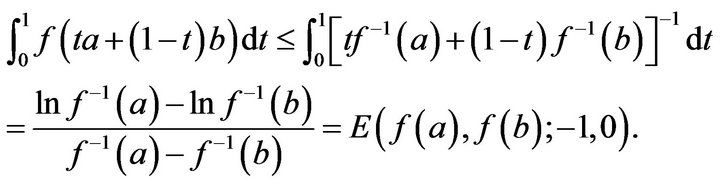
Case 2. If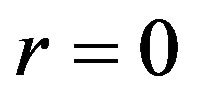 , we have
, we have
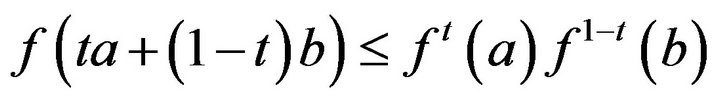
for any 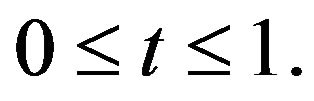 Hence, we obtain
Hence, we obtain

Case 3. If 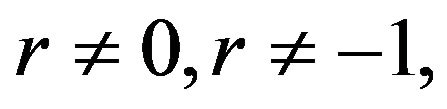 we have
we have
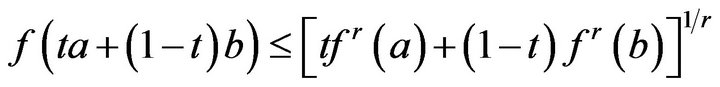
for any 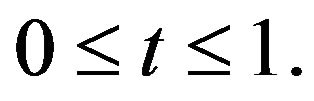 Hence, we get
Hence, we get
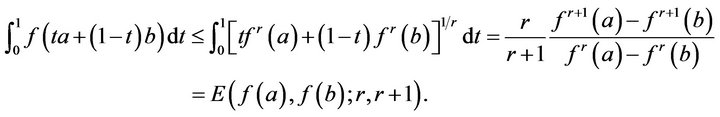
The proof of Theorem 2.1 is complete.
Corollary 2.1.1. If 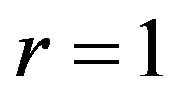 in Theorem 2.1, we have
in Theorem 2.1, we have
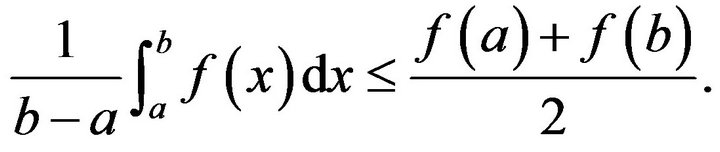 (2.2)
(2.2)
Theorem 2.2. Let
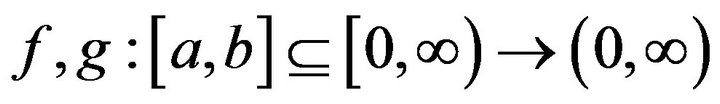
be 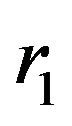 -convex and
-convex and ![]() -convex functions respectively on
-convex functions respectively on 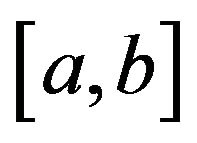 with
with 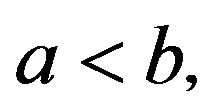
 . Then the following inequality holds
. Then the following inequality holds
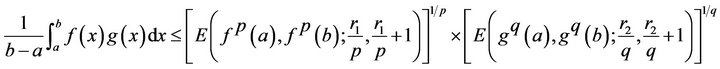 (2.3)
(2.3)
for any 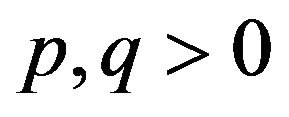 and
and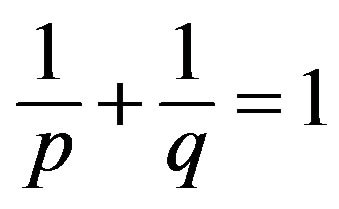 .
.
Proof. Let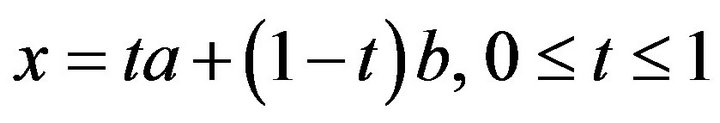 , then we have
, then we have
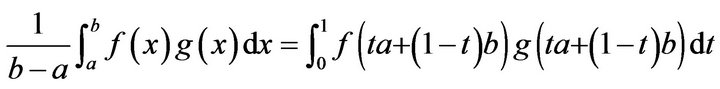 .
.
If 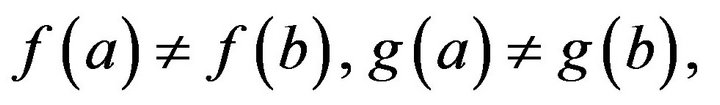 then 1) when
then 1) when  by the
by the  -convexity and
-convexity and ![]() -convexity of functions
-convexity of functions  respectively, we have
respectively, we have
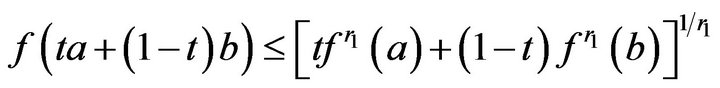
and
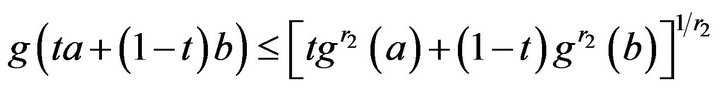
for any 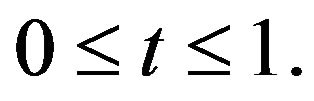 So we obtain
So we obtain
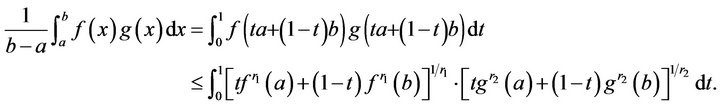
By the Hölder’s integral inequality and Theorem 2.1, we have
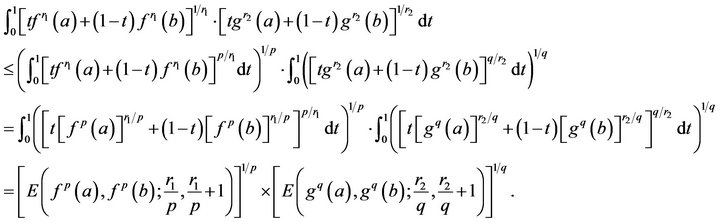
2) when 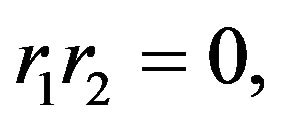 we just prove for
we just prove for 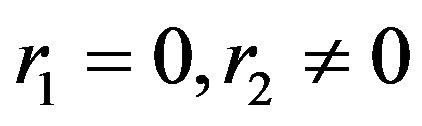 which is similar to
which is similar to 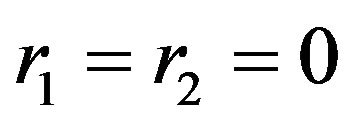 and
and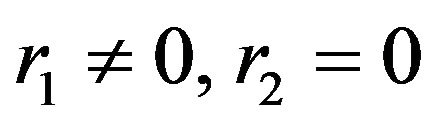 . By the Hölder’s integral inequality, Theorem 2.1 and
. By the Hölder’s integral inequality, Theorem 2.1 and 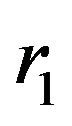 -convexity and
-convexity and ![]() -convexity of functions
-convexity of functions 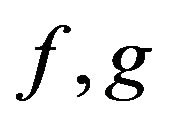 respectively, we have
respectively, we have
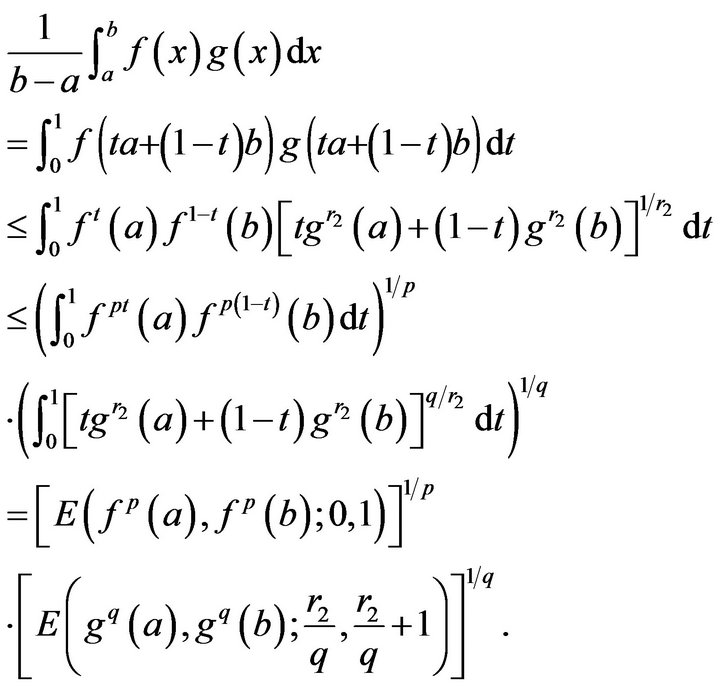
If 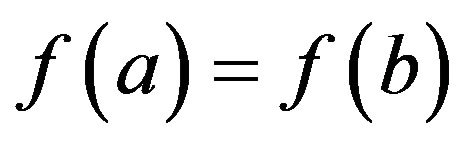 or
or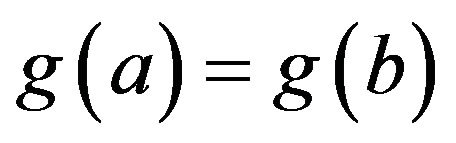 , by Theorem 2.1 we obtain the conclusion, which the proof of Theorem 2.2 is completed.
, by Theorem 2.1 we obtain the conclusion, which the proof of Theorem 2.2 is completed.
Corollary 2.2.1. Under the conditions of Theorem 2.2if 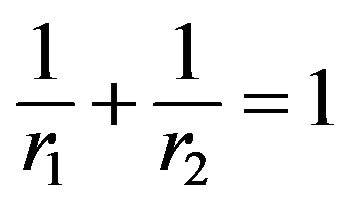 for any
for any , then we have
, then we have
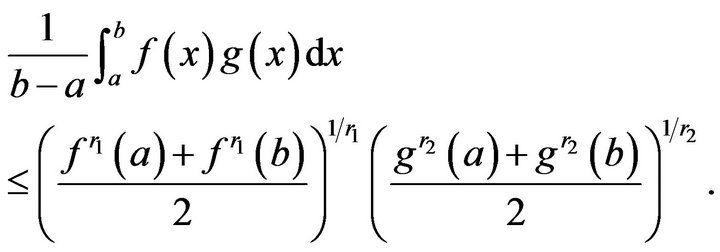 (2.4)
(2.4)
In particular, if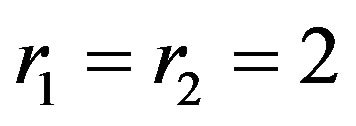 , then we have
, then we have
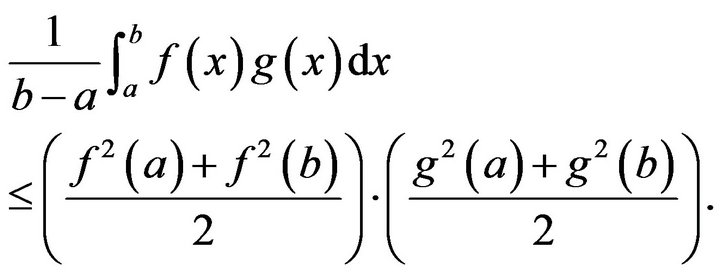
If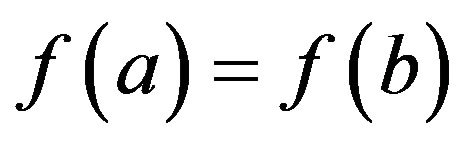 ,
, 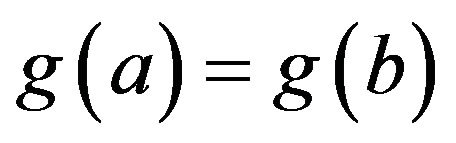 , we have
, we have
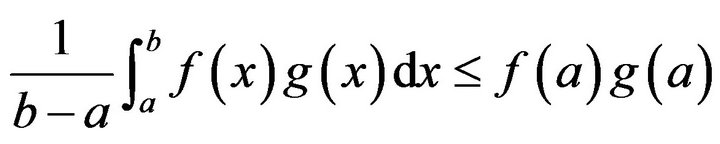
Corollary 2.2.2. Under conditions of Theorem 2.2, if 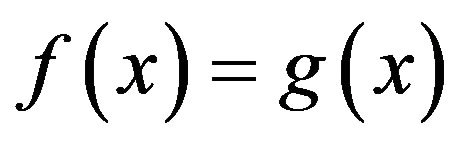 and
and 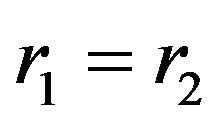 then we have
then we have
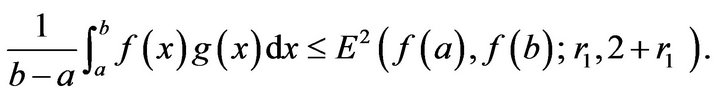 (2.5)
(2.5)
In particular, if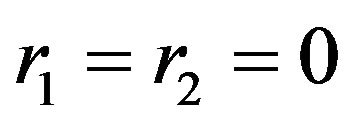 , then we have
, then we have
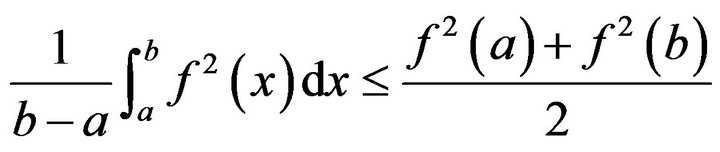
Theorem 2.3. Let 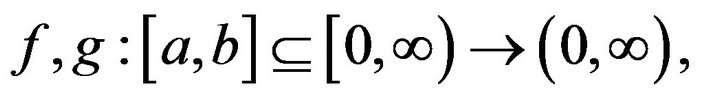
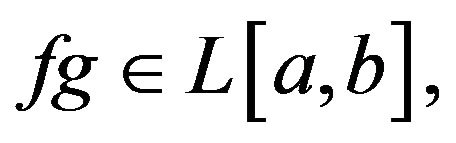
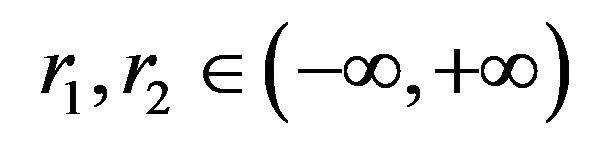 and
and 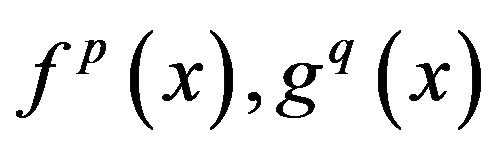 be
be 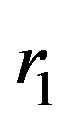 -convex and
-convex and ![]() -convex functions respectively on
-convex functions respectively on 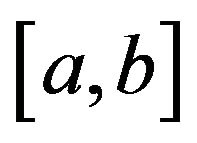 with
with 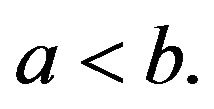 Then the following inequality holds
Then the following inequality holds
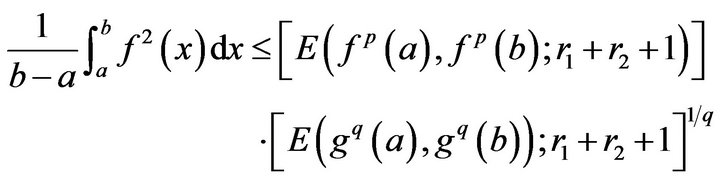 (2.6)
(2.6)
for any 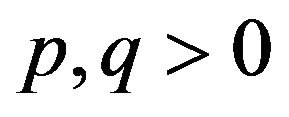 and
and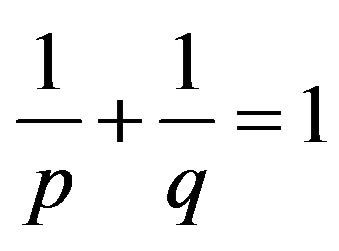 .
.
Proof. Let , then we have
, then we have
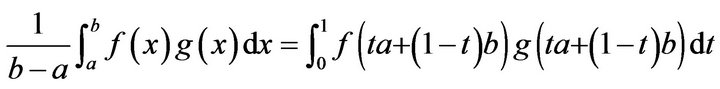 .
.
By the Hölder’s integral inequality, Theorem 2.1 and  -convexity and
-convexity and ![]() -convexity of function
-convexity of function  respectively, we have
respectively, we have
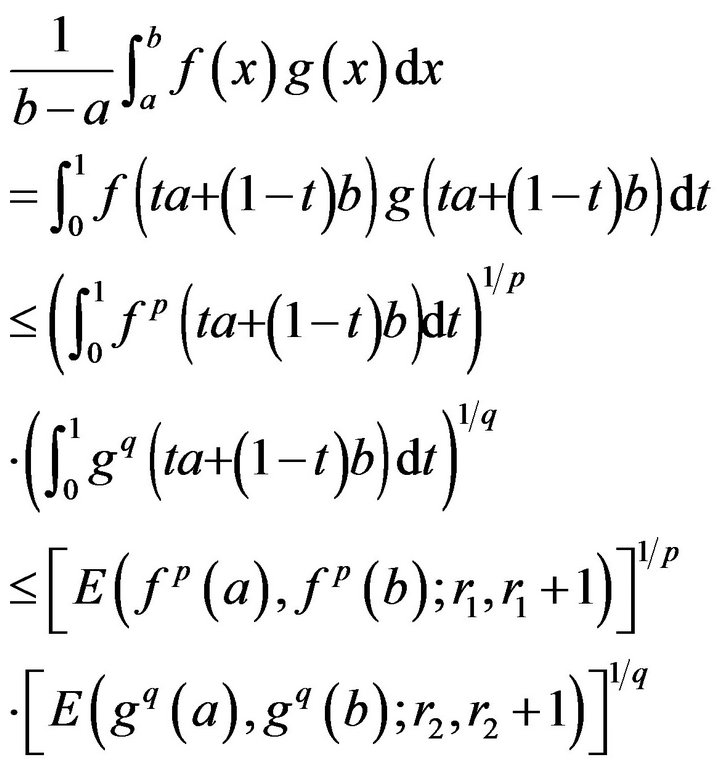
This completed the proof of Theorem 2.3.
Corollary 2.3.1. Under the conditions of Theorem 2.3if 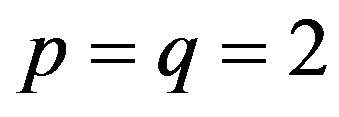 and
and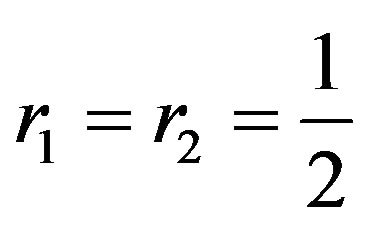 , then we have
, then we have
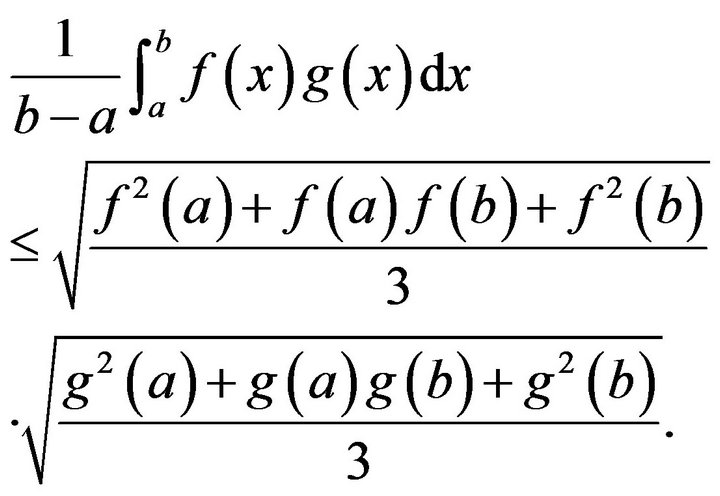 (2.7)
(2.7)
In particular, if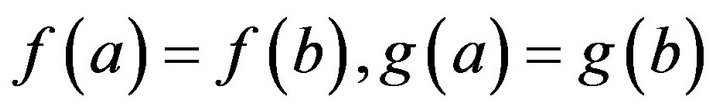 , we have
, we have
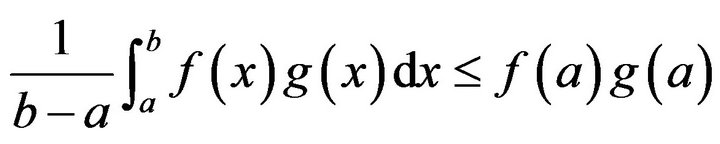
In this paper, we obtained three new Hermite-Hadamard type integral inequalities for 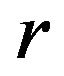 -convex functions, which improved the results of Theorems 1.2-1.4 by Hölder’s integral inequality, Stolarsky mean and convexity of function. The special case of new Hermite-Hadamard type integral inequalities is classical Hermite-Hadamard type integral inequality. So it improved the classical one.
-convex functions, which improved the results of Theorems 1.2-1.4 by Hölder’s integral inequality, Stolarsky mean and convexity of function. The special case of new Hermite-Hadamard type integral inequalities is classical Hermite-Hadamard type integral inequality. So it improved the classical one.
3. Acknowledgements
The first author was supported in part by the National Natural Science Foundation of China under Grant No. 11161033 and Inner Mongolia Natural Science of China under Grant No. 2010MS0119.
REFERENCES
- C. E. M. Pearce, J. Peccaric and V. Simic, “Stolarsky Means and Hadamard’s Inequality,” Journal of Mathematical Analysis and Applications, Vol. 220, No. 1, 1998, pp. 99-109. doi:10.1006/jmaa.1997.5822
- G.-S. Yang, “Refinements of Hadamard’s Inequality for r-Convex Functions,” Indian Journal of Pure and Applied Mathematics, Vol. 32, No. 10, 2001, pp. 1571-1579.
- N. P. N. Ngoc, N. V. Vinh and P. T. T. Hien, “Integral Inequalities of Hadamard Type for r-Convex Functions,” International Mathematical Forum, Vol. 4, No. 35, 2009, pp. 1723-1728.
- M. K. Bakula, M. E. Özdemir and J. Pečarić, “Hadamard Type Inequalities for m-Convex and (α−m)-Convex Functions,” Journal of Inequalities in Pure and Applied Mathematics, Vol. 9, No. 4, 2008, Article ID: 96.
- P. M. Gill, C. E. M. Pearce and J. Pečarić, “Hadamard’s Inequality for r-Convex Functions,” Journal of Mathematical Analysis and Applications, Vol. 215, No. 2, 1997, pp. 461-470. doi:10.1006/jmaa.1997.5645
- A. G. Azpeitia, “Convex Functions and the Hadamard Inequality,” Revista Colombiana de Matemáticas, Vol. 28, No. 1, 1994, pp. 7-12.
- K. B. Stolarsky, “Generalizations of the Logarithmic Mean,” Mathematics Magazine, Vol. 48, No. 2, 1975, pp. 87-92. doi:10.2307/2689825

