Received 10 March 2016; accepted 24 May 2016; published 27 May 2016

ABSTRACT

Groundwater provides one option to utilise renewable energy sources. The long-term groundwater energy potential for three building complexes, situated at latitude of 64°, was investigated by combining an energy demand simulation for the buildings with hydrogeological modelling. First, a reference year for the building energy demand was created. Secondly, groundwater flow requirements were calculated. The results of the previous stages were utilised in groundwater heat transport modelling in an environment where the natural temperature of groundwater was 4.9˚C. Finally, the long-term (50 years) groundwater energy potential was calculated. The groundwater maintained its heating potential during 50 years of operation. When both heating and cooling power were demanded, the long-term pumping rate of groundwater decreased by 60,000 m³/a. Energy utilisation created a cold groundwater plume downstream, in which the temperature decreased by 1 to 2.5˚C within a distance of 300 m from the site. Groundwater can provide a long- term energy source for large building complexes in the Nordic climate. Results indicate that groundwater could effectively be utilised until the temperature reaches approximately 4˚C. Accurate information on the building energy demand and hydrogeology is essential for successful operation.

Keywords:

Groundwater Energy, Building Energy Simulation, Numerical Modelling, Cold Region, Finland

1. Introduction

The ongoing economic crisis, dependence on imported energy and the need to tackle climate change have forced the EU to promote more efficient energy production. The Energy Efficiency Directive (2012/27/EU) endorses the application of innovative technology to increase the use of renewable energy systems (RES) in Europe. The need to adopt more RES in all EU countries is vital, as the EU has a commitment to reduce greenhouse gas emissions from 85% to 90% below 1990 levels by 2050 [1] . Finland, located in the north of Europe between the 60th and 70th degrees of latitude, is part of the EU and has set a target of 38% for the contribution of RES to gross overall energy consumption by 2020. In 2012, RES accounted for 35.1% of the overall energy consumption of Finland, 3.7% of which was produced by heat pumps [2] . One option to increase renewable energy production in Finland is to utilise groundwater reservoirs for energy production. Although groundwater has been widely used for decades as an RES, for instance in China [3] , Canada [4] and in Europe [5] , groundwater utilisation for energy purposes is still a new innovation in Finland, despite the locally significant groundwater energy potential [6] .

The main aquifers for water supply purposes are situated on shallow Quaternary eskers or ice-marginal end moraine complexes in Finland. The natural groundwater temperature is typically higher than the air temperature in cold regions [7] - [9] . This is due to the three reasons: firstly, in winter the snow cover functions as an insulator, preventing cold air conduction into the subsurface layers. Secondly, the change in the state of water releases latent heat into the soil when frost is formed and thirdly, frost acts as an insulator, reducing the flow of cold meltwater into deeper soil layers in early spring, when the melting of snow begins [10] - [12] . Finland’s mean air temperature was approximately 2.3˚C during the time period from 1981 to 2010 [13] and average groundwater temperatures varied from 3.0˚C in northern to 6.6˚C in southern parts of the country [14] - [16] . The reason for groundwater temperature variations is mainly climatological. Urbanisation has elevated groundwater temperatures by several degrees [7] [17] [18] , resulting in an increased heating capacity of groundwater in urbanised compared to rural areas [19] - [21] . Because of the groundwater low temperature, i.e. low enthalpy energy, it is essential to exploit heating energy from groundwater with heat pumps and/or heat exchangers. Cooling energy can also be exploited using a free cooling system, but groundwater needs to be pumped from the aquifer to the heat transfer system. Hence, groundwater energy utilisation systems, both for heating and cooling, require electricity or some other form of exterior power to work properly. Groundwater exploitation is highly regulated by the Water and Environment act in Finland. However, Finnish legislation has no binding operational limits for groundwater temperature variations during energy utilisation as exist, for example, in Germany [22] or in Switzerland [23] .

The typical technique, called an open loop energy system or open loop system [23] [24] , exploits groundwater from an aquifer by pumping it from and discharging it into the subsurface [23] [25] . A system where one abstraction well and one injection well have been constructed is called an open loop well-doublet scheme [3] [4] . Groundwater pumping and discharging for energy utilisation can be planned to work in two directions. Therefore, an abstraction well in summer can become an injection well in winter, meaning that cold groundwater pumped from an abstraction well in summer is used for cooling and hence returned to the injection well at a higher temperature. In winter, the system is reversed and warmer groundwater is utilised for heating purposes. This system can be called as aquifer thermal energy storage (ATES) [23] [24] [26] .

Groundwater energy utilisation requires site-specific hydrogeological knowledge and an ability to recognise the collective risks caused by variations in the groundwater temperature and level [24] [27] . Groundwater energy usage has to remain at a thermally sustainable level [4] to achieve both the environmental and economic operational requirements. Banks [3] presented a risk assessment approach to sustainable groundwater thermal use for cooling. Groundwater energy utilisation may also have positive environmental influences; in the Netherlands, an ATES system is used for the remediation of chemically contaminated groundwater [28] [29] . Using groundwater for heating, i.e. injecting cooled groundwater to the aquifer, may provide a solution to reduce the urbanisation impact, which raises groundwater temperatures. Replacing convectional oil heating systems with groundwater energy system will reduce the risk of oil leaks to the aquifer.

The long-term groundwater energy potential of aquifers situated in northern regions has remained undetermined. Neither has the latest information on building energy demands been taken into consideration in groundwater energy system design in Finland. The present study investigated whether groundwater with a natural temperature of 4.9˚C could allow thermally sustainable energy utilisation from a Quaternary aquifer for three large building complexes during an operational period of 50 years. The groundwater energy potential was investigated for: 1) an area with 20 detached houses; 2) area with 3 apartment buildings and 3) a shopping centre. The dimensioning of the groundwater pumping rate was defined by hourly-based information on building energy demand. This study combined the benefits of building energy simulations and hydrogeological modelling.

2. Area Description, Material and Methods

The project area, Karhinkangas, was chosen this study due to available geological and hydrological data and suitable location on the area of naturally cold groundwater temperature. Karhinkangas is located in western Finland, near the Gulf of Bothnia (Figure 1), approximately on the latitude of 64˚ passing through Central Finland. According to the Finnish Meteorological Institute, during the time period from 1981 to 2010, the mean annual air temperature was 3˚C to 4˚C, the mean yearly precipitation 450 mm to 550 mm and snow cover normally persisted for 135 to 140 days in winter.

2.1. Geology and Hydrogeology

The bedrock is dominated by plutonic and metamorphic rocks, including granites, gneisses and granodiorites, and is associated with the Svecofennian (1900 Ma) orogeny [30] . The bedrock is mainly covered by glacial and postglacial sediments [31] deposited during the Weichselian glacial stage and the Holocene. The Karhinkangas esker consists of glaciofluvial sand and gravel with a thickness of approximately 20 m [31] . The average natural groundwater temperature at a depth of approximately 15 m is 4.9˚C according two years of continuous temperature data recorded by the Geological Survey of Finland. Groundwater temperature measurements have been taken by data loggers from 18 monitoring wells.

2.2. Building Energy Simulations: Reference Year

The energy demand of three types of building was simulated. The net heating power for a detached house with an area of 134 m² and an apartment building with an area of 814 m² was simulated using the IDA Indoor Climate and Energy (IDA-ICE) 4.1 dynamic simulation tool, and the heating and cooling power demands of a shopping centre with area of 15,000 m² were simulated with the RIUSKA application. The simulation results were presented as the hourly-based power distribution during a one-year period. This period is named as the reference year, and describes the current Finnish climatic conditions according to Kalamees et al. [32] . The thermal insulation of buildings fulfils the minimum requirements of the Finnish Building Code, part C3 [33] . Power for household hot water heating, distribution losses of space heating and domestic hot water were not included in the net power simulations for houses or apartments. These additional energy demands were calculated based on the National Building code, parts D3 and D5 [34] [35] . These codes provide the total energy demand of additional systems, not the hourly-based power distribution. The additional energy demand was adjusted to the hourly power distribution with a four-phase calculation process. First, the hourly energy profiles of distribution losses from space heating and domestic hot water were produced using the same percentual energy distribution as the net power simulations. Secondly, the hourly profile of hot water heating power was produced, noting that hot water heating consumption is 14% less in June and July than in other months in Finland [36] . Thirdly, additional power distribution profiles were added to the net power profile, and finally, the heating power demand of one detached house was multiplied by 20 and that of one apartment building was multiplied by 3.

2.3. Groundwater Flow and Heat Transport Modelling

The groundwater flow model had previously been completed using the three-dimensional finite differences code MODFLOW [37] . Heat transport was simulated using the analogy between solute and heat transport. MT3DMS [38] was used in the simulations. The relationship between the solute transport equation and heat transport was derived by Thorne et al. [39] . The analogous heat transport equation is of the form:

(1)

where θ is the volumetric water content; v_i is the mean pore water velocity vector; q_s is a source or sink term; ρ_s is density of solid; ρ_w is density of water; c_s and c_w are specific heat capacity of the solid and water, respectively; T is the temperature; 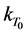is the effective thermal conductivity of the porous media; T_s is the temperature of the source; and D_ij is the dispersion tensor [39] . Thermal diffusion coefficient, D_m, is given as [39] :

(2)

Groundwater flow model and its calibration and validation were conducted in the previous study by Paalijärvi and Okkonen [40] . Some general information about the flow model is given here but the details of the flow model and its development can be found at that publication. The hydraulic conductivity varies between 1.2 ´ 10⁻⁶ - 2.3 ´ 10⁻³ m/s and groundwater recharge between 0 - 1.1 ´ 10⁻⁹ m/s (Figure 2) being 24,328 m³/d for the whole area (24.5 km²).

The heat transport focused on the southern part of the aquifer (Figure 2) and the area of interest was only a few hundreds of square meters, thus, so called regional to local model conversion was carried out [41] in order to ease the computational effort. From the regional model hydraulic conductivities, recharge rates, ground surface elevations and bedrock are interpolated to local model. The regional flow model was at first run with a different pumping scenarios (see last paragraph of the introduction) to get the groundwater level variations. These groundwater level variations were then applied in the local model as a time variant boundary conditions (Figure 3). Local model was then used in the heat transfer simulations. The maximum head change due to pumping (all scenarios included) at the east and west boundaries were only 0.005 meters. In the north and south no-flux boundary conditions was used. The local model was conceptualised in a rectangular area with a length of 800 m and a width of 400 m. The grid cell size in the local model was 5 m × 5 m (Figure 3). Initial condition was 4.9˚C at the whole domain. Heat content from the recharge and the east and south (time variant specified head) boundaries were 4.9˚C.

The minimum pumping well distance in the energy transport models was estimated using an analytical equation:

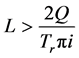(3)

where L is the distance between the abstraction and injection wells; Q is the groundwater pumping rate; T_r is aquifer transmissivity and i is hydraulic gradient [3] . A cold-water injection well was located downstream of the groundwater flow.

A daily time step was used and the total simulation time was 50 years. The average recharge was used, but the pumping rate was based on the power demand during the reference year and adjusted on a monthly basis. Thus, intra-annual changes were taken into account, but these changes were the same for the whole 50 years. In the MT3DMS simulations, a standard finite difference method with an upstream weighting scheme was used.

The soil thermal conductivity was calculated as the weighted mean thermal conductivity of the main minerals and water (soil pores are filled with water in the saturated zone). The mineralogy was determined at the laboratory of the Geological Survey of Finland, and the thermal conductivity of minerals was taken from Clauser and Huenges [42] . Bulk and fluid densities, specific heat capacities, porosity, thermal diffusion and dispersion coefficients were estimated based on literature values (see Table 1). Transverse and longitudinal dispersivities were 0.025.

2.4. Groundwater Flux, Heating and Cooling Power Calculations

The groundwater pumping requirements needed to achieve simulated heating and cooling power (reference year) were calculated on an hourly basis (8760 hours in a year) using the following equations [5] [19] :

Groundwater flux for heating:

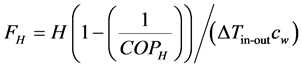(4)

Groundwater flux for cooling:

(5)

where F_H = flux of water (kg∙s⁻¹) for heating and F_C for cooling respectively; H is heating power (W) and C cooling power (W); ΔT_in-out = temperature difference between incoming and outgoing water in the heat pump / heat exchanger - temperature drop in heating mode and temperature rise in cooling mode (K); c_w = specific heat capacity of water (J∙kg⁻¹∙K⁻¹); COP_H = coefficient of performance of the heat pump for heating (dimensionless);

and COP_C = coefficient of performance of the heat exchanger system for cooling (dimensionless). The groundwater flux unit kg∙s⁻¹ was changed to l∙s⁻¹, as the change has no real effects on the results and l s⁻¹ is universally used to describe groundwater flow. In heating mode, groundwater utilisation down to a temperature of +1.4˚C was assumed, and ΔT_in-out was consequently 3.5˚C, because the initial groundwater temperature was 4.9˚C. Based on previous studies i.e. Andersson [48] and Cruickshanks and Adsett [49] and noting the Nordic location of Karhinkangas, a maximum groundwater return temperature of 12˚C was used for cooling calculations. Hence, ΔT_in-out for cooling was 7.1˚C.

The specific heat capacities of water were taken from Yaws [42] . A heat pump was used for heating and a heat exchanger for cooling applications. Based on the information presented by Allen et al., Bayer et al., Saner et al. [19] [50] [51] and the European Heat Pump Association, EHPA [52] , a COP_H of 3.5 and COP_C of 25 was used in this analysis. Based on the buildings energy simulation results (see paragraph 2.2) the heat pumps were dimensioned to achieve 60% of the peak design power requirement of a detached house and an apartment building and 50% of that for a shopping centre. Cooling power was dimensioned to achieve 100% from groundwater flux.

Finally, the long-term groundwater heating and cooling power capacity was calculated by solving H and C from equations 4 and 5 for each modelled month. Monthly variations in groundwater temperature in abstraction well(s) (ΔT_in) were taken from modelled groundwater temperature data to calculate the monthly differences of ΔT_in-out and finally, monthly variations of H and C. The groundwater flux (F_H and F_C) were kept in the level of reference year. The results of H and C were compared to the energy demands for the reference year to determine the long-term energy potential of groundwater.

3. Results

3.1. Building Energy and Groundwater Flow Demands: The Reference Year

The largest requirement for power and groundwater flow was for the shopping centre (Table 2). Table 2 also states that heat pumps were designed with a nominal heating power of 120 kW for the detached houses, 75 kW for the apartment buildings and 350 kW for the shopping center. The groundwater flow for the second peak (Table 2) is calculated from the peak hourly value and hence is not based on modelled energy consumption.

Groundwater flow requirements vary significantly between days, especially in the ATES system (Figure 4). The peak pumping rate occurs on 1st and 3rd August, when maximum cooling power is needed. For example, the maximum groundwater flow per hour is 20.98 m³/h for 20 detached houses and 121.08 m³/h for shopping centre (Table 2). The average pumping rate per day is 7.38 m³/d and the median 6.88 m³/d for 20 detached houses and 23.76 m³/h and 18.96 m³/h for shopping centre respectively. The largest groundwater demand for a day is 1572 m³, which is 6.5% of the modelled recharge value of the aquifer.

-not analysed; ^aPeak heating and cooling power denotes the peak heating/cooling power demands of the building; ^bHeat pump energy and peak heat pump power denote the heating energy and power respectively producible in a building by using a heat pump.

3.2. Groundwater Flow and Heat Transport Modelling

According to equation 3, the minimum distances between injection and abstraction wells were 36.5 m for the detached houses, 15.3 m for the apartment buildings and 88.3 m for the shopping centre. In modelling, a well separation of 40 m was used for the detached houses, 20 m for the apartment buildings and 125 m for the shopping centre (see Figure 3). The ground surface area of the shopping centre defined the well distance more than the calculated minimum distance.

The groundwater temperature decreased as groundwater was only used for heating in well-doublet scheme simulations (see Figure 5 and Figure 6). The thermal plume stretched to 300 m in approximately 30 months after pumping had started and groundwater temperatures achieved a steady state condition after approximately 2 years of operation. For example, the temperatures remained constant between 2.8˚C and 2.9˚C in the detached houses scenario and at 3.9˚C in the apartment buildings scenario at an observation point 300 m from the injection well (Figure 5 and Figure 6). The yearly temperature variation in the injection well was 0.15˚C in the detached house model and 0.5˚C in the apartment building model.

In the shopping centre scenario, the thermal plume is more mixed than the other two schemes, as groundwater is utilised both for heating and cooling (Figure 7 and Figure 8). Temperature variations reached a constant annual cycle after five years of operation on the cooling side and approximately after the first year on the heating side (Figure 7). In the steady state conditions, the maximum operating temperature is 3.2˚C and minimum 1.4˚C on the cooling side and the minimum is 5.7˚C and maximum 11.6˚C on the heating side respectively. At the observation point 300 meters downstream from the injection well, the temperature begins to decrease after 27 months of operation. The temperature reaches its minimum level of 2.2˚C after 60 months of operation and then increases to the constant level of 2.3˚C after approximately 100 months of operation (Figure 8).

The maximum changes in the groundwater level were 15.6 cm (heating side of the shopping centre) and a pumping cone occurred at a distance of only a few meters from the abstraction and injection wells.

3.3. Results of Energy Calculations

The groundwater temperature remained constant during pumping and hence had no effects on the heating power reservoir in the detached house and apartment building scenarios. In the shopping centre scenario, the peak months for heating and cooling power are January and August, respectively. Groundwater would provide over 20% more heating power in January and over 25% more cooling power in August when compared to the reference year (Figure 9). The groundwater flux has been retained at the level of the reference year; only ΔT_in-out has been changed according the modelled groundwater temperatures in Figure 9.

4. Discussion

4.1. Power and Groundwater Flux Design Authors and Affiliations

Specific information on the heating and cooling power demands of buildings provides a possibility to accurately design groundwater pumping and energy transfer systems. Accurate planning adjusts the size of the energy dis-

tribution system, e.g. the nominal power of the heat and water pump, which will optimize the building and electricity costs of the project. Table 2 indicates that dimensioning a heat pump to cover 50% to 60% of the peak design power of a building provides 97.5% to 98.5% of the total heating energy with the given groundwater flux values. Holopainen et al. [53] reported that the lowest lifecycle cost will be achieved if a heat pump is dimen-

sioned to cover 50% of the peak design power of apartment buildings in Finland. Rosen et al. [9] stated that in Sweden, the economically most suitable option is to dimension heat pumps to cover 50% to 60% of the peak design power of individual houses. Holopainen et al. [53] and Rosen et al. [9] made theirs calculation for a closed loop geoenergy system, i.e. a system where energy is exchanged from the ground to the fluid inside the heat exchanger pipes. Our calculation confirmed this assumption for an open-loop system in Nordic countries. As our results are comparable to previous studies in the Nordic climate [9] [53] , power calculations and hence groundwater pumping requirements are on a reliable level. The Nordic climate is characterised by significant air temperature differences between summer and winter. These temperature fluctuations cause changes in building energy demands and hence observed variations in the groundwater pumping rate. Even the ATES system cannot be designed for a relatively stable pumping scenario in the Nordic environment.

Groundwater temperature variations in abstraction and injection wells indicate that groundwater could effectively be utilised until the groundwater temperature reaches approximately 4˚C. At a temperature of 4˚C, groundwater would still maintain its energy potential, assuming that other calculation parameters used in this research remained constant. Even colder groundwater could be utilised, but the groundwater pumping demand would then significantly increase and the effectiveness of the system would decrease.

Hydrogeological circumstances provided suitable conditions for groundwater abstraction and injection in this study. At the aquifer scale energy utilisation had no effects on the groundwater level or the flow direction. These effects can be seen near the abstraction and injection wells resulting minor changes in local hydraulic gradient. This is due to the high hydraulic conductivity and relatively small groundwater circulation demands compared to the estimated natural recharge volume of the aquifer. The high hydraulic conductivity also allowed small distances between the abstraction and injection wells. The hydraulic conductivity of the Karhinkangas aquifer, 1.76 × 10⁻³ m/s, represents that of a typical Finnish sand and gravel aquifer, as the hydraulic conductivity of Finnish glaciofluvial aquifers is normally between 10⁻⁵ to 10⁻² m/s [43] [54] [55] . In ATES system (Figure 8) warm groundwater plume from the heating side collides with the cold plume on the cooling side, and part of the heating plume seem like to partially circulate around the cooling plume. The cooling plume in the upstream direction (to the east/southeast in Figure 8) is due to groundwater injection which rises groundwater level near injection well. A comparable upstream plume cannot be seen on the heating side of the ATES system in Figure 8. This is due to the larger heating than cooling demand of the building and hence the larger groundwater injection requirement on the cooling side.

The calculated groundwater temperature variations in the injection well were greater in the apartment building scenario than in the detached house scenario, even though the yearly groundwater pumping rate was 2.4 times higher for the detached houses. The reason may be that the distance between the abstraction and injection wells was only 20 m in the apartment building compared to 40 m in the detached house scenario.

Both the well-doublet scheme and the ATES system reduced groundwater temperatures and established a cold groundwater plume in the groundwater flow direction. The groundwater temperature decreased by approximately 1 to 2.5˚C from its natural temperature at a distance of 300 m from the site. The relatively high hydraulic conductivity, porosity and high water circulation rates allowed the thermal plume to spread over 300 m from the injection well. Due to the pumping rates, the apartment buildings had a less significant effect on the groundwater temperature than the detached houses or shopping centre. The observed temperature variations are due to the larger heating than cooling energy demand of buildings and are under the Swiss legislation temperature limit of 3˚C, which is the strictest legally specified numerical temperature fluctuation limit [23] . If the cooling and heating sides of the ATES system were opposite each other, a heated plume would appear. However, if the heating and cooling wells were switched, heated groundwater would flow downstream from the abstraction well and hence the groundwater would not provide enough heating power for buildings.

Comparing our results to those of Banks [3] and Ferguson and Woodbury [4] , who investigated the cooling effects of buildings on groundwater, it appears that the thermal effect of groundwater energy utilisation is less harmful when more heating than cooling power is needed in buildings. Groundwater cooling can reduce microbial growth in groundwater. In general, warm groundwater provides more a suitable environment for harmful thermophilic microbes such as faecal bacteria than cool groundwater [27] . Arola and Korkka-Niemi [20] reported elevated groundwater temperatures due to urbanisation in Finland. Groundwater energy utilisation in urbanised areas restores the elevated temperature and hence changes the thermal environment of aquifers towards natural conditions. Cool groundwater is also a benefit if groundwater is distributed to the communal water system. However, cooled groundwater can change the natural vegetation on groundwater discharges areas and may consequently form threat to endangered species which are protected by Directive 2006/118/EU in the EU. Remediation of light non-aqueous phase liquids (LNAP) chlorinated solvents from groundwater [29] and seawater intrusion to coastal aquifers [28] can be accelerated with temperature variations. Heating or cooling power can be extracted from flowing groundwater similarly with remediation, and hence increase the sustainability of groundwater use.

4.2. Energy Utilisation

In the apartment building and detached house scenarios, energy utilisation had no significant effects on the groundwater temperature in the abstraction well. Hence, the power requirements for 50 years of operation could be achieved with the groundwater flux of the reference year. Even though a cold groundwater plume was formed, thermal breakthrough was not observed in the abstraction well and hence the groundwater energy potential did not vary during operation. The National Building code, part D3 [34] , guides the use of passive cooling, e.g. solutions that prevent solar radiation, rather than active cooling systems for houses and apartments. Hence, cooling calculations were only performed for the shopping centre.

The results confirmed that the ATES system increased the groundwater energy potential compared to groundwater use for heating only. A similar discussion of the ATES system compared to a cooling scenario alone was presented by Banks [3] . In the first year of operation, approximately 400 MW of heating and 160 MW of cooling power could be distributed for external usage by the shopping centre. After five years of operation, when a steady state was achieved, approximately 450 MW of heating and 160 MW of cooling power could be distributed. Percentually more heating than cooling power could be distributed on a yearly scale. In monthly peak conditions, i.e. heating in January and cooling in August, more cooling than heating energy could be distributed from the shopping centre. This is due to the Nordic environment, which causes significant differences in heating and cooling power demands, particularly a major heating power demand in winter and a relatively short cooling period in summer. If heating and/or cooling power cannot be distributed for external use by the shopping centre, the groundwater abstraction requirements decrease significantly from the pumping demands of the reference year. In the first year of operation, approximately 52,700 m³ less groundwater, and after five years approximately 60,000 m³ less groundwater needs to be abstracted to meet the shopping centre’s reference year energy requirements.

5. Conclusions

Groundwater can form a thermally long-term renewable energy source for large buildings in areas of cold groundwater such as Finland. This research demonstrates that careful, interdisciplinary planning involving thermo-geologists and HVAC engineers can improve the sustainability and economic viability of geothermal energy utilization. Accurate planning reduces the environmental risks and the overall economics of energy system could be improved. It can be estimated that groundwater could be effectively utilized until the temperature of approximately 4˚C. This research also indicates that the long-term maximum energy potential of groundwater can be estimated when the natural groundwater temperature, geological and hydrogeological conditions of the aquifer and energy requirements of buildings are precisely known. The ATES system generates different thermal regimes for an aquifer and needs more detailed system planning than groundwater utilization for heating or cooling only.

Groundwater energy utilization reduced the groundwater temperatures in the groundwater flow direction. In the Nordic environment, the groundwater temperature decreases due to the significantly larger heating than cooling energy requirements. In glaciofluvial esker formations, the size of groundwater thermal plume is dependent on site-specific thermo- and hydro-geological factors and can extend a few hundreds of meters from injection wells. Groundwater energy utilization may also have environmentally beneficial side effects; in urbanized areas, energy use could reduce the groundwater temperature to its natural level, the remediation of LNAP and/or seawater contaminated groundwater could be accelerated with the use of a heat pump or heat exchanger and replacing conventional oil heating systems with groundwater energy utilization will reduce the soil and groundwater contamination risk. The methods and results of this study represent well the Finnish geological environment and are applicable to similar environments globally.

The accuracy of estimated groundwater abstraction requirements can be further improved by incorporating a climate change scenario, heat leakage from different building types to the soil and other artificial ground surface changes in thermo-geological modelling.

Acknowledgements

The authors wish to thank Kirsti Korkka-Niemi, Veli-Pekka Salonen, Pirjo Tuomi and Jukka Hellen for their support and advice and Roy Siddall for language revision. Special thanks go to colleagues at the Turku office of Golder Associates Oy.

This research was funded by Golder Associates Oy and the K.H. Renlund Foundation.

Cite this paper

Teppo Arola,Jarkko Okkonen,Juha Jokisalo, (2016) Groundwater Utilisation for Energy Production in the Nordic Environment: An Energy Simulation and Hydrogeological Modelling Approach. Journal of Water Resource and Protection,08,642-656. doi: 10.4236/jwarp.2016.86053

References