ABSTRACT

Acid dissociation constants, pKa, of 2-pyrrolaldehyde phenylsulphonyl hydrazone, 1, and 2-thiophenealdehyde phenylsulphonyl hydrazone, 2, have been determined spec- trophotometrically in ethanol-water media of various compositions over the temperature range 25˚C - 45˚C. The obtained results were used in the calculation of the enthalpy, ∆H^o, and the entropy, ∆S^o, of the ionization processes. The slight variations observed in the pKa values of the thiophene compound compared to the pyrrol analogue revealed that neither of the two hetero atoms in the pyrrol or thiophene rings, of the two compounds, is involved in a hydrogen bond chelation. This conclusion was also confirmed through measurements of the dipole moment, IR and NMR spectra.

Keywords:

Acid Dissociation Constant, Pyrrol, Thiophene

1. Introduction

Aldehyde phenylsulphonyl hydrazones of the type, Ar-CH=N-NH-SO₂Ph, were firstly prepared by Grammaticakis [1] . These compounds were only used as intermediates in the preparation of 5-arseno-sulphonylformazanes [2] and 2, 5-disubstituted tetrazoles [3] [4] . As such compounds showed pronounced acidity, it seemed interesting to prepare compounds 1 and 2 with Ar = pyrrol and thiophene rings, respectively, in order to investigate the effect of the nitrogen and sulpher hetero atoms, X, on the acidity of these derivatives and correlating their acid dissociation constants with the solvent, ethanol-water, composition and temperature , we can also investigate the possibility of metal chelation if the hetero atoms, X, are involved in hydrogen bond formation.

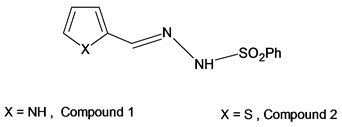
2. Experimental

2-pyrrolaldehye phenylsulphonyl hydrazone, 1, and 2-thiophenealdehyde phenylsulphonyl hydrazone, 2, were prepared by the general procedure described elsewhere [4] . Melting points were found to agree with those reported in the literature.

Measurements of PH were made by Hanna P^H meter, model H1 8417 equipped with combined glass electrode. The PH meter readings were converted to hydrogen ion concentration, [H⁺], by means of the Van Uitert relation [5] .

Electronic absorption spectra were measured on Perkin-Elmer Lambda 3 spectro- photometer.

For the spectrophotometric determination of the acid dissociation constant, PKa, an aliquot of the stock hydrazone solution was diluted with perchloric acid solution, absolute ethanol and water to give a definite overall ionic strength of 0.1 M and final hydrazone strength of 10 - 3 M and contain the required ethanol content of (20%, 40%, 60% and 80% v/v). The absorption spectra of each of these solutions were examined in terms of different PH values and at temperatures of (25˚C, 30˚C, 35˚C, 40˚C and 45˚C). Temperature was controlled by using a double jacket cell connected to an ultra thermostat adjusted to the required temperature (±0.1˚C). This thermostat is connected, in turn, to the spectrophotometric cell in order to verify the same temperature for both measurements.

Static dielectric constant of each solution was measured on a Dipolemeter DMO1 manufactured by WTW, Germany. Dielectric constantɛ, density, d, and refractive index, n_D, for hydrazone solutions were measured at 30˚C as described earlier [6] . The dipole moments, μ₂, in benzene was determined by the refractivity method employing the Debye Equation 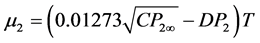(1)

(1)

where 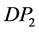is the molar deformation of the solute, obtained by extrapolating the measured molecular refraction for the sodium-D line to infinite wave length [7] ; and 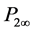is the molecular polarization of the solute at infinite dilution, taken as the average of that determined graphically and those calculated by Hedestrand’s [8] and Plait-Ba- neerjee’s [9] Equations.

IR spectra were determined for samples in KBr discs on a Pye Unicam SP3-300.

1H NMR spectra were measured in CDCl3 on a Varian Gimini 200 MHz NMR- Spectrophotometer.

3. Results and Discussion

The acid dissociation constants pKa’s of the analogues 1 and 2 were determined spectrophotometrically in 20%, 40%, 60% and 80% (v/v) ethanol-water mixture over the temperature range 25˚C to 45˚C. The pKa values were calculated from the absorbance, A, and P^H data, using the Equation (2).

(2)

Amax and Amin where are the absorbance values in basic and acidic media (PH = 10 and P^H = 1), respectively. The results obtained are given in Table 1. The values of the dielectric constants of the solvent mixtures, at the working temperature range, 25˚C - 45˚C are given in Table 2.

The data obtained show that at any given temperature, an increase of ethanol content in the solvent mixture resulted in an increase of the pKa values, as a consequence of decreasing the dielectric constant of the solvent mixture [10] . Also, for a given solvent mixture, increase of temperature resulted in a decrease of the pKa values.

From the data obtained, both the enthalpy, ∆H^o, and the entropy, ∆S^o, were calculated using the van’t Hoff Equations (3) and (4).

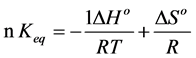(3)

(4)

The results are summarized in Table 3. It is clear that ∆H^o values are positive , whereas the entropy, ∆S^o, values are negative for all solvent mixtures indicating that the process of ionization is always endothermic whatever the ethyl-water content in the solvent mixture.

Harned et al. [11] investigated the temperature variation of the acidity of acetic acid in water and in aqueous dioxane, as well as the acidity of other acids in water [12] [13] . A parabolic relationship between pKa and temperature was found; and is given by the Equation (5).

(5)

where the temperature T, is expressed in degree centigrade, pKm is the minimum value of pKa, at temperature θ, and C is an arbitrary constant of the order, 5 × 10 − 2 degree.

When Equation (5) was applied to our experimental data, the values of pKm and θ were calculated and situated in Table 4. The data indicated that, increasing the ethanol content in the solvent mixture increases the value of θ while decreasing the pKm values. The increase of θ values with increasing the ethanol content being in agreement with the above finding that the ionization is opposed by increasing the ethanol content.

Comparison of the pKa values for the compounds 1 and 2 revealed that, replacement of the nitrogen hetero atom in compound 1 by the sulpher atom in compound 2 resulted in a slight increase in the pKa values whatever the working temperature and the solvent composition.

In general, compounds 1 and 2 can be represented by either of the following two structures, (i) and (ii).

According to the structure (i), it might be expected that the acidity is most likely dependent upon the strength of the intramolecular H-bond, X… H-N, in such a manner that, the stronger the bond the less will be the acidity of the compound. The electronegativity of the hetero atom, X, will affect the strength of the H-bond and hence, the acidity of the H-N group through an inductive effect; that is, the higher the electronegativity of the hetero atom, X, the higher is the acidity of the compound. Referring to the structure (i), the acidity is governed by the predominance of one of the above two effects. On the other hand, the structure (ii) suggests that the acidity is mainly governed by the electronegativity of the hetero atom, X. This means that the greater the electronegativity of, X, the greater will be the inductive effect and hence, the higher the acidity.

Based on the above agreements, structure (ii) seems to be more appropriate for compounds, 1 and 2, than structure (i), since the observed pKa values for the compound, 1, are less than those of compound 2. This conclusion was confirmed by dipole moment for the two compounds in benzene at 30˚C and comparing the results obtained with those calculated, using the method of bond moments [14] , for various possible conformations, Figure 1. The experimental results are given in Table 5, and those calculated for conformations (I-VIII) in Table 6. Comparing the experimental and calculated dipole moment values revealed that the conformation structures II-V, VII and VIII should be excluded, where the calculated dipole moment values are significantly different from the experimental values which are 4.87 and 4.77 D for compounds, 1, and 2, respectively. The calculated dipole moment values, 5.01 and 4.94 for

compounds, 1 and 2, respectively are close to the calculated value based on conformation VI.

The non-chelated structure (ii) was further confirmed by the observation that no metal complex could be detected spectrophotometrically with both compounds, 1 and 2, where, all trials made to prepare Fe III, Co II, Ni II and Cu II complexes were failed.

The IR and 1H NMR spectra of the two compounds were compared with that of ben- zaldehyde benzenesulphonylhydrazone, 3. The stretching frequencies, νNH, and the proton chemical shift, δNH, of the N-H proton of compounds, 1 - 3, are given in Table 7.

The close similarity of the stretching frequencies of NH bond and the chemical shift values of the three compounds (1 - 3), substantiate further our previous conclusion that compounds 1 and 2 exist in a non-chelated form.

4. Conclusion

Based on determined acid dissociation constant values, pKa, for the two compounds 1 and 2 and the electronegativity of the hetero atom, X, structure (ii), which is the non chelated form, seems to be more appropriate for these compounds. This was also confirmed through comparing the experimentally determined dipole moment values of the two compounds with the calculated values obtained of different conformation structures. Also, these compounds failed to form complexes with Fe III, Co II, Ni II and Cu II, metalions.

Cite this paper

Nessim, R.I., Albalaa, I.E. and Assi, M.A. (2016) Effect of Temperature and Solvent Composition on the Acid Dissociation Constants of 2-Pyrrol and 2-Thiophene Aldehyde Phenyl Sulphonyl Hy- drazones. Natural Science, 8, 442-449. http://dx.doi.org/10.4236/ns.2016.810046

References