Received 19 June 2016; accepted 14 August 2016; published 17 August 2016

ABSTRACT

In this paper, by means of constructing a special cone, we obtain a sufficient condition for the existence of positive solution to semipositone fractional differential equation.

Keywords:

Fractional Differential Equations, Boundary Value Problems, Positive Solution, Semipositone

1. Introduction

The aim of this paper is to investigate the existence of positive solutions to the semipositone fractional differential equation

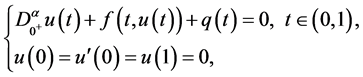(1)

where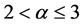, 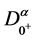is the standard Riemann-Liouville fractional derivative of order 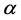which is defined as follows:

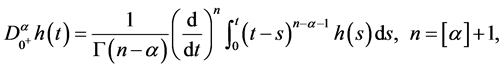
where 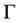denotes the Euler gamma function and 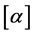denotes the integer part of number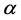, provided that the right side is pointwise defined on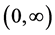, see [1] . Here, by a positive solution to the problem (1), we mean a function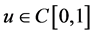, which is positive in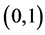, and satisfies (1).

Fractional differential equations have gained much importance and attention due to the fact that they have been proved to be valuable tools in the modelling of many phenomena in engineering and sciences such as physics, mechanics, economics and biology. In recent years, there exist a great deal of researches on the existence and/or uniqueness of solutions (or positive solutions) to boundary value problems for fractional-order differential equations. Sun [2] studied the existence of positive solutions for the following boundary value pro- blems:

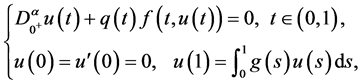
where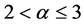, 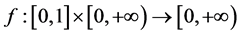is continuous and 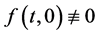on. But paper [2] did not give the results of the existence of positive solution when the nonlinearity can take negative value, i.e. semipositone problems.

The purpose of the present paper is to apply the method of varying translation together with the fixed point theorems in cone to discuss (1) without nonnegativity imposed on the nonlinearity. Meanwhile, we also allow the nonlinearity to have many finite singularities on.

2. Preliminaries and Lemmas

In this section, we present several lemmas that are useful to the proof of our main results. For the forthcoming analysis, we need the following assumptions:

(H₁) is continuous. For any, there exist constants

such that

(H₂) with and,

where

will be defined in the following text.

In [3] , the authors obtained the Green function associated with the problem (1). More precisely, the authors proved the following lemma.

Lemma 2.1 [3] . For any, the unique solution of the boundary value problem

(2)

is given by

(3)

where

(4)

Lemma 2.2 [4] . The Green function defined by (4) satisfies the inequality

(5)

here

Remark 2.1. A simple computation shows that there exists a constant such that

Remark 2.2 [5] . If satisfies (H₁), then for any is increasing on

and for any,

Lemma 2.3 [6] . Let X be a real Banach space, be a bounded open subset of X with and is a completely continuous operator, where P is a cone in X.

(i) Suppose that then.

(ii) Suppose that then.

Consider the Banach space with the usual supremum norm and define the

cone. Let, then is the unique solution

to (2) for. Now we first consider the singular nonlinear boundary value problem

(6)

where We have the following Lemma.

Lemma 2.4. If the singular nonlinear boundary value problem (2) has a positive solution such that for any. Then boundary value problem (1) has a positive solution.

Proof. In fact, if u is a positive solution to (6) such that for any. Let, then. Since is the unique solution to (2) for for any, we

have, which implies that. So

. Consequently is positive solution to (1). This complete the proof of Lemma 2.4.

For any, define an operator

(7)

Since for any fixed, we can choose such that. Note that

so by (H₁), we have

Consequently, for any, we have

(8)

Therefore, the operator T is well defined and

Lemma 2.5. Assume that (H₁), (H₂) hold. Then is a completely continuous operator.

Proof. For any, in view of (2) we conclude that

Whence, it follows from (8) that which implies

Next we show that is continuous. Suppose, and Then, there exists a constant such that. Since for any

, by Remark 2.2, we have

(9)

Thus, we have

and. It follows from the Lebesgue control convergence theorem that

which implies is continuous.

In what follows, we need to prove that is relatively compact.

Let be any bounded set. Then there exists a constant such that for any. Similarly as (9), for any we have

(10)

Consequently

(11)

Therefore is uniformly bounded.

Now we show that is equicontinuous on. For any, by (9), (11) and the Lebesgue control convergence theorem, and noticing the continuity of, we have

(12)

Thus, is equicontinuous on [0,1]. The Arezlà-Ascoli Theorem guarantees that is relatively compact set. Therefore is completely continuous operator.

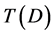
Lemma 2.6. Let then.

Proof. Assume that there exists such that Then and Thus we have

This contradiction shows that.

Lemma 2.7. There exists a constant such that, where

Proof. Choose constants and N such that

From Remark (2.2), there exists, such that

(13)

Let Obviously, Now we show that In

fact, otherwise, there exists such that By (2), for any we have

So

Consequently, That is This

contradiction shows that.

3. Main Results

Theorem 3.1. Suppose that (H₁), (H₂) hold. Then, the boundary value problems (1) has at least one positive solution, and exists a constant such that

Proof of Theorem 3.1. Applying Lemma 2.6 and Lemma 2.7 and the definition of the fixed point index, we have Thus T has a fixed point in with. Since, we have

Let It follows from Lemma (2.4) that is a positive solution to boundary value problem (1), and there exists a constant such that

Acknowledgements

We thank the Editor and the referee for their comments. This research was supported financially by the National Natural Science Foundation of China (11471187, 11571197), the Natural Science Foundation of Shandong Province of China (ZR2014AL004) and the Project of Shandong Province Higher Educational Science and Technology Program (J14LI08), the Project of Scientific and Technological of Qufu Normal University (XKJ201303).

Cite this paper

Xinsheng Du, (2016) Existence of Positive Solutions to Semipositone Fractional Differential Equations. Applied Mathematics,07,1484-1489. doi: 10.4236/am.2016.714127

References