Applied Mathematics
Vol.06 No.11(2015), Article ID:60340,10 pages
10.4236/am.2015.611161
The Harmonic Approximation in Heavy-Ion Reaction Study
Godwin Joseph Ibeh1*, Elijah Dika Mshelia2
1Department of Physics, Nigerian Defence Academy, Kaduna, Nigeria
2Department of Physics, University of Abuja, Abuja, Nigeria
Email: *gjibeh@nda.edu.ng
Copyright © 2015 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 15 August 2015; accepted 13 October 2015; published 16 October 2015
ABSTRACT
The derivation of the harmonic approximation of the Hamiltonian of a model of coupled three- dimensional harmonic oscillator is presented. It is shown how the splitting of the total Hamiltonian into the intrinsic and collective Hamiltonians leads to the description of the mechanism for energy dissipation in physical systems.
Keywords:
Harmonic Approximation, Energy Dissipation, Coupled Oscillators, Heavy-Ions, Dinuclear System, Cluster Model

1. Introduction
Investigation into the mechanism of energy dissipation in heavy-ion reactions has been carried out by different authors from different approaches, an example is the quantum dynamical model of Diaz-Torres, Hinde, Dasgupta, Milburn and Tostevin, which is based on the dissipative dynamics of open quantum systems in this model both deep-inelastic process and quantum tunneling were treated with a quantum mechanical coupled-channels approach [1] and [2] . For some review papers and other approaches to heavy-ion collisions studies, see the following refs. [3] -[11] .
Mshelia, Scheid and Greiner formulated a nuclear energy dissipation theory to account for energy dissipation that occurs in heavy-ion collisions [12] . This was described quantum mechanically as resulting from the coupling of collective degrees of freedom to intrinsic excitations. The formalism has been tested on several analytically solvable models of oscillators coupled to free motion in one-dimension [13] [14] . Recently, Ibeh and Mshelia presented a realistic but complex model for investigating the energy dissipation in physical systems [15] , which was an extension of the one-dimensional models of previous work [13] [14] . The results obtained showed a marked improvement to the previous models comparative to the exact results.
In this paper, we consider some of the salient features in the complex model of Ibeh and Mshelia which consists of three-dimensional coupled oscillators located at the corners of a tetrahedron, three oscillators at the corner of the triangular base representing intrinsic motion while the one at the apex represents the collective motion [15] . In Section 2, we present the derivation of the potential energy and the kinetic energy, leading to the equation of motion of the system. Section 3 deals with the quantization of the Hamiltonian of the dissipative system. In Section 4, the solution of the total and intrinsic Schrödinger equations is presented, while Section 5 consists of the method of determining the probability distribution function.
2. The Classical Hamiltonian
By the symmetry consideration of the arrangement of four particles in space Figure 1 gives the schematic of the vibrating system, consisting of three-dimensional coupled oscillators located at the corners of a tetrahedron- three oscillators at the corners of the triangular base representing intrinsic motion while one at the apex representing collective motion. All oscillators are coupled to each other elastically. Their equilibrium positions are as follows: the particle of mass M is at the point 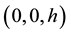 at the apex of the tetrahedron while the other
at the apex of the tetrahedron while the other
three particles are positioned at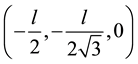 ,
, 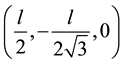 ,
, 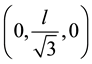 located at the corners of the triangular base.
located at the corners of the triangular base.
For harmonic vibration recall that in classical mechanics [16] -[19] , it is shown that near the equilibrium position the potential energy of the system may be developed in a Taylor series i.e.,
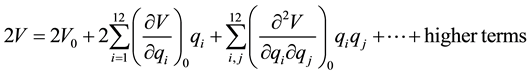 (1)
(1)
in this the only term of interest is the third term, which is sufficient for small amplitude of vibration, so that the harmonic potential energy is approximated to
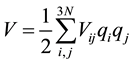 (2)
(2)
in which the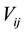 ’s are constants given by
’s are constants given by
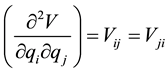 (3)
(3)
The quantities  form a symmetric matrix. Thus the potential energy of the system of Figure 1 about the equilibrium positions of the particles, becomes,
form a symmetric matrix. Thus the potential energy of the system of Figure 1 about the equilibrium positions of the particles, becomes,
 (4)
(4)
Figure 1. A system of three small mass coupled to each other and each coupled to a large mass.
where the constants, 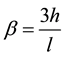 and
and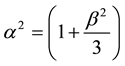 .
.
Similarly the quadratic kinetic energy of the system is
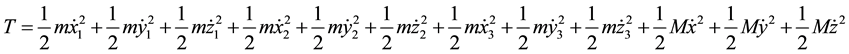 (5)
(5)
where, 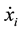 ,
, 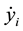 ,
, 



3. The Quantum Energy Dissipation
In Ibeh and Mshelia [15] the quantized Hamiltonian describing the dissipation of energy from the collective motion into intrinsic degrees of freedom is given as:

where the intrinsic and collective Hamiltonians are explicitly stated as:

and

The collective Hamiltonian is assumed to be that of a free particle with mass, M and described by coordinates

3.1. Normal Modes of Vibration
The total Hamiltonian in Equation (6) is given in terms of Equations (7) and (8) as

From the above consideration we observe that the kinetic energy matrix is diagonal while the potential energy matrix is non-diagonal due to the products









The normal frequencies are determined by the secular equation

where the coefficients 

Using the matrices of the kinetic and potential energies according to Equation (9), we obtain from Equation (10) the following twelve eigenfrequencies:

where, the constants appearing in the eigenfrequencies above are defined as follows:
Note the two double degenerate frequencies namely Ω1 = Ω3 and Ω2 = Ω4 and the two non-degenerate eigenfrequencies Ω5 and Ω6 describing the motion in which all the four particles vibrate about their common equilibrium configuration. The six eigenfrequencies: Ω7; Ω8; Ω9; Ω10; Ω11 and Ω12 which vanish are assumed to consists of the three zero eigenfrequencies Ω7, Ω8, Ω9, corresponding to the eigenmodes describing a uniform translational motion of the system as a whole, while the remaining three zero eigenfrequencies Ω10, Ω11 and Ω12 have no direct bearing on the theory of energy dissipation in this work. The corresponding transformations to normal coordinates are obtained as:

In terms of the normal coordinates the quantum mechanical total Hamiltonian is

3.2. The Solutions of the Schrödinger Equation for the Total and Intrinsic Hamiltonians
We now obtain solutions of the time-independent Schrödinger equation with the decoupled total Hamiltonian H given by Equation (13).

Since H describes a free translational motion of the centre of mass and the decoupled harmonic oscillators in the g1, g2, g3, g4, g5 and g6 degrees of freedom the eigenvalues and eigenfunctions are obtained as,

and

where, the quantum numbers are




The normalized, bound state, wave functions of the harmonic oscillators are written as

The quantity 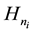



the normalization constant 

The total wave function in Equation (16) is normalized as follows:

The intrinsic Hamiltonian can be stated in terms of intrinsic coordinates defined as following

The resulting eigenvalue equation of the intrinsic Hamiltonian is

where 
Solving Equation (23) results in the eigenvalues

And the following set of eigenfrequencies and eigenfunctions:

where, the constants appearing in the eigenfrequencies above are defined as follows:
the normalized intrinsic oscillator eigenfunctions:

where, the intrinsic inverse oscillator lengths and normalization constants are respectively,


From Equation (26) the total intrinsic wave-function becomes

4. The Probability Density Functions
The fact that the intrinsic Hamiltonian eigenfunctions obtained form a complete set, by use of the completeness relation the total wave function 
thonormal set of oscillator functions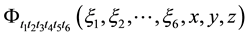


since the

On the other hand, the normalization of 

The relationship between the left hand and the right hand of Equations (30) and (31) is given by the transformation

When values are substituted the Jacobian is

Comparing Equations (31), (32) and (33) the normalization condition

Equation (34) gives a measure for the probability for intrinsic excitation from collective motion [15] . It should be noted that the derivation of Equation (34) demonstrates that the form of the collective amplitude, 
The collective amplitude is the expansion coefficient of the total wave function 


5. Conclusions
This work has shown that the harmonic approximation of the Hamiltonian of coupled oscillators leads to a Schrödinger equation which describes the coupling of collective degree of freedom, represented by free motion with intrinsic degrees of freedom, represented by three coupled oscillators. This model explains the mechanism for energy dissipation in a physical system, based on the coupling of intrinsic and collective degrees of freedom. The model can be extended to nuclear fission and heavy-ion reactions, where the collective degree of freedom is the relative coordinates of the two heavy-ions and the intrinsic degrees of freedom are the single-particle degrees of freedom [22] .
Furthermore, of current interest and one which is an extension of the above model is the cluster model consisting of a dinuclear system which is not easily solvable analytically because it includes other degrees of freedoms such as butterfly, belly-dancer-type motions, γ-and β-vibrations, etc., of individual nuclei, this model is based on the assumption that cluster-type shapes are produced in the mass asymmetry of nuclear molecules. Theoretical and experimental evidences exist that show that this model is capable of explaining many of the features of deformed heavy nuclei [26] - [38] . An example of such features is the resent work of Adamian, Antonenko and Lenske, in which the linear response theory was used to calculate the mass parameters for collective variables of the dinuclear systems formed in cold fusion reactions and found that the microscopic mass parameter in the neck is larger than the one obtained using the hydrodynamical model [39] .
Cite this paper
GodwinJoseph Ibeh,Elijah DikaMshelia, (2015) The Harmonic Approximation in Heavy-Ion Reaction Study. Applied Mathematics,06,1831-1841. doi: 10.4236/am.2015.611161
References
- 1. Diaz-Torres, A., Hinde, D.J., Dasgupta, M., Milburn, G.J. and Tostevin, J.A. (2008) Dissipative Quantum Dynamics in Low-Energy Collisions of Complex Nuclei. Physical Review C, 78, Article ID: 064604.
http://dx.doi.org/10.1103/physrevc.78.064604 - 2. Diaz-Torres, A., Hinde, D.J., Dasgupta, M., Milburn, G.J. and Tostevin, J.A. (2009) Coupled-Channels Approach for Dissipative Quantum Dynamics in Near-Barrier Collisions. International Conference on New Aspects of Heavy-Ion Barrier, Chicago.
http://dx.doi.org/10.1063/1.3108859 - 3. Swiatecki, W.J. and Bjфrnholm, S. (1972) Fission and Fusion Dynamics. Physics Reports, 4, 326-342.
http://dx.doi.org/10.1016/0370-1573(72)90003-8 - 4. Nörenberg, W. and Weidenmüller, H.A. (1976) Introduction to the Theory of Heavy-Ion Collisions. Lecture Notes in Physics 5, Springer-Verlag, Berlin.
- 5. Hasse, R.W. (1978) Approaches to Nuclear Friction. Reports on Progress in Physics, 41, 1027-1101.
http://dx.doi.org/10.1088/0034-4885/41/7/002 - 6. Weidenmüller, H.A. (1980) Transport Theories of Heavy-Ion Reactions. Progress in Particle and Nuclear Physics, 3, 49.
http://dx.doi.org/10.1016/0146-6410(80)90030-7 - 7. Mshelia, E.D. (1997) Nuclear Science and Technology in Human Progress. (Unpublished University Inaugural Lecture). Abubakar Tafawa Balewa University, Bauchi.
- 8. Aritomo, Y., Hagino, K., Nishio, K. and Chiba, S. (2012) Dynamical Approach to Heavy-Ion Induced Fission Using Actinide Target Nuclei at Energies around the Coulomb Barrier. Physical Review C, 85, Article ID: 044614.
http://dx.doi.org/10.1103/PhysRevC.85.044614 - 9. Liu, Z.H. and Bao, J.D. (2013) Possibility to Produce Element 120 in the 54Cr+248Cm Hot Fusion Reaction. Physical Review C, 87, Article ID: 0344616.
http://dx.doi.org/10.1103/PhysRevC.87.034616 - 10. Gontchar, I.I., Bhattacharya, R. and Chushnyakova, M.V. (2014) Quantitative Analysis of Precise Heavy-Ion Fusion Data at Above-Barrier Energies Using Skyrme-Hartree-Fock Nuclear Densities. Physical Review C, 89, Article ID: 034601.
http://dx.doi.org/10.1103/PhysRevC.89.034601 - 11. Wen, K., Sakata, F., Li, Z.-X., Wu, X., Zhang, Y. and Zhou, S. (2014) Energy Dependence of the Nucleus-Nucleus Potential and the Friction Parameter in Fusion Reactions. Physical Review C, 90, Article ID: 054613.
http://dx.doi.org/10.1103/PhysRevC.90.054613 - 12. Mshelia, E.D., Scheid, W. and Greiner, W. (1975) Theory of Energy Dissipation in Heavy-Ion Reactions. Il Nuovo Cimento A, 30, 589-608. http://dx.doi.org/10.1007/BF02730488
- 13. Mshelia, E.D., Haln, D. and Scheid, W. (1981) Energy Dissipation in a Model of Coupled Oscillators. Il Nuovo Cimento A, 61, 28-55.
http://dx.doi.org/10.1007/BF02776606 - 14. Mshelia, E.D. and Ngadda, Y.H. (1989) A Stimulation for Energy Dissipation in Nuclear Reactions. Journal of Physics G: Nuclear and Particle Physics, 15, 1281-1290.
http://dx.doi.org/10.1088/0954-3899/15/8/023 - 15. Ibeh, G.J. and Mshelia, E.D. (2014) Energy Dissipation in a Model of Coupled Three-Dimensional Harmonic Oscillator. Far East Journal of Mathematical Sciences, 88, 107-136.
- 16. Corben, H.C. and Stehle, P. (1960) Classical Mechanics. John Wiley, New York, 113-131, 364-372.
- 17. Wells, D.A. (1967) Theory and Problems of Lagrangian Dynamics. Schaum Publication, New York.
- 18. Marion, J.B. and Thornton, S.T. (1995) Classical Dynamics of Particles and Systems. 4th Edition, Saunders College Publications, New York.
- 19. Goldstein, H., Poole, C. and Safko, J. (2002) Classical Mechanics. 3rd Edition, Addison-Wesley, San Francisco, 238-258.
- 20. Wilson Jr., B., Decius, J.C. and Paul, C.C. (1980) Molecular Vibrations. Dover Publications, Inc., New York.
- 21. Desloge, E.A. (1982) Classical Mechanics. Volume II, Wiley-Interscience, New York, 665-707.
- 22. Mshelia, E.D. (1995) Method of Normal Coordinates in the Formulation of a System with Dissipation: The Harmonic Oscillator. Il Nuovo Cimento A, 108, 709-721.
http://dx.doi.org/10.1007/BF02813376 - 23. Schiff, L.I. (1987) Quantum Mechanics. 3rd Edition, McGraw-Hill, New York, 50-52.
- 24. Greiner, W. (1994) Quantum Mechanics: An Introduction. 3rd Edition, Springer-Verlag, New York, 97-105.
- 25. Courant, R. and Hilbert, D. (1953) Methods of Mathematical Physics. Interscience Publishers, New York, 424.
- 26. Bromley, D.A. (1978) Nuclear Molecules. Scientific American, 239, 58-68.
http://dx.doi.org/10.1038/scientificamerican1278-58 - 27. Volkov, V.V. (1978) Deep Inelastic Transfer Reaction—The New Type of Reactions between Complex Nuclei. Physics Reports, 44, 93-157.
http://dx.doi.org/10.1016/0370-1573(78)90200-4 - 28. Iachello, F. and Jackson, A.D. (1982) A Phenomenological Approach to α-Clustering in Heavy Nuclei. Physics Letters B, 108, 151-154.
http://dx.doi.org/10.1016/0370-2693(82)91162-5 - 29. Greiner, W., Park, J.Y. and Scheid, W. (1995) Nuclear Molecules. World Scientific, Singapore.
- 30. Buck, B., Merchant, A.C. and Perez, S.M. (1998) Systematic Study of Exotic Clustering in Even-Even Actinide Nuclei. Physical Review C, 58, 2049-2060.
http://dx.doi.org/10.1103/physrevc.58.2049 - 31. Buck, B., Merchant, A.C. and Perez, S.M. (1999) Cluster Structure and Gamma Transitions in Actinides. Physical Review C, 59, 750-754.
http://dx.doi.org/10.1103/PhysRevC.59.750 - 32. Adamian, G.G., Antonenko, N.V. and Scheid, W. (2000) Isotopic Dependence of Fusion Cross Sections in Reactions with Heavy Nuclei. Nuclear Physics A, 678, 24-38.
http://dx.doi.org/10.1016/s0375-9474(00)00317-1 - 33. Mshelia, E.D. and Scheid, W. (2004) Collective Dynamics of a Dinuclear System. The European Physical Journal A, 20, 251-254.
http://dx.doi.org/10.1140/epja/i2003-10102-7 - 34. Li, W., Nan, W., Fei, J., Hushan, X., Wei, Z., Li, Q.F., et al. (2006) Particle Transfer and Fusion Cross-Section for Super-Heavy Nuclei in Dinuclear System. Journal of Physics G, 32, 1143-1155.
http://dx.doi.org/10.1088/0954-3899/32/8/006 - 35. Kalandarov, S.A., Adamian, G.G., Antonenko, N.V. and Scheid, W. (2011) Role of Angular Momentum in the Production of Complex Fragments in Fusion and Quasifission Reactions. Physical Review C, 83, Article ID: 054611.
http://dx.doi.org/10.1103/PhysRevC.83.054611 - 36. Kalandarov, S.A., Adamian, G.G, Antonenko, N.V., Scheid, W., Heinz, S., Comas, V., Hofman, S., Khuyagbaatar, J., Ackermann, D., Heredia, J., HeBberger, F.P., Kindler, B., Lommel, B. and Mann, R. (2011) Emission of Cluster with Z > 2 from Excited Actinide Nuclei. Physical Review C, 84, Article ID: 054607.
http://dx.doi.org/10.1103/PhysRevC.84.054607 - 37. Kalandarov, S.A., Adamian, G.G., Antonenko, N.V., Scheid, W. and Wieleczko, J.P. (2011) Role of the Entrance Channel in the Production of Complex Fragments in Fusion-Fission and Quasifission Reactions in the Framework of the Dinuclear System Model. Physical Review C, 84, Article ID: 064601.
http://dx.doi.org/10.1103/PhysRevC.84.064601 - 38. Bansal, M., Sahila, C. and Rajk, G. (2012) Dynamical Cluster-Decay Model Using Various Formulations of a Proximity Potential for Compact Non-Coplanar Nuclei: Application to the 64Ni+100Mo Reaction. Physical Review C, 86, Article ID: 034604. http://dx.doi.org/10.1103/physrevc.86.034604
- 39. Adamian, G.G., Antonenko, N.V. and Lenske, H. (2015) Role of the Neck Degree of Freedom in Cold Fusion Reactions. Physical Review C, 91, Article ID: 054602. http://dx.doi.org/10.1103/PhysRevC.91.054602
NOTES
*Corresponding author.





