Received 15 November 2014; accepted 10 April 2015; published 17 April 2015

ABSTRACT

Bacteriophage MS2 is a viral particle whose symmetrical capsid consists of 180 copies of asymmetrical coat proteins with triangulation number T = 3. The mathematical theorems in this study show that the phage particles in three-dimension (3D) might be an icosahedron, a dodecahedron, or a pentakis dodecahedron. A particle with 180 coat protein subunits and T = 3 requires some geometrical adaptations to form a stable regular polyhedron, such as an icosahedron or a dodecahedron. However, with mathematical reasons electron micrographs of the phage MS2 show that 180 coat proteins are packed in an icosahedron. The mathematical analysis of electron micrographs in this study may be a useful tool for surveying the platonic solid structure of a phage or virus particle before performing 3D reconstruction.

Keywords:

MS2, Icosahedron, Mathematical Approach

1. Introduction

Many viruses and phages are icosahedral particles. However, spherical viruses may be polyhedral, forming dodecahedra or pentakis dodecahedra [1] . Bacteriophage MS2 is an icosahedral bacteriophage with a diameter of 27 - 34 nm [2] [3] .

When an icosahedron is transferred to a flat sheet [4] , its hexagon units consist of 6 regular triangles. A convex angle can be formed by 5 angles of regular triangles, but not 6 angles, which become a plane. The 20 triangular faces created by the vertices of 12 pentagonal cones and 30 edges can form an icosahedron.

The coat protein of MS2 forms a shell that protects the phage nucleic acid and acts as a translational repressor [5] . The tertiary structure of the coat proteins is asymmetrical. A particle with nucleotides packed with asymmetrical proteins requires a low free-energy to achieve a stable condition. Packing as a helix or a regular polyhedron is a way of getting a symmetrical solid with asymmetrical subunits. In icosahedral particles, proteins are packed on the faces and directed to the vertices and the particles become symmetrical [3] [6] . In the MS2 capsid, one triangle of the icosahedron contains 3 asymmetrical subunits [6] [7] . This study uses mathematical analysis to identify the reasonable Platonic solids for packing a symmetrical capsid with asymmetrical subunits. Results show that the MS2 particles with 180 coat proteins and triangulation number might form an icosahedron, a dodecahedron, or a pentakis dodecahedron.

The overall shape of MS2 is spherical, but is difficult to see the three-dimensional (3D) figure in electron microscopy (EM) two-dimensional (2D) images before 3D reconstruction. Therefore, this study also introduces a mathematical method to predict particle solid 3D figures with 2D EM images before performing 3D reconstruction.

2. Theory and Calculation

The 3D structure of MS2 should be an isohedron or a regular polyhedron if the particles need to pack the asymmetrical proteins to make a stable symmetrical structure. The capsid of phage MS2 contains 180 identical copies of a coat protein with a T = 3 isohedral (such as icosahedral) shell [3] [7] . MS2 particles may be constructed with 60 triangles, 45 quadrilaterals, 36 pentagons, or 30 hexagons. This study examines particle construction using the following theorems and mathematical analysis:

Theorem 1. If a polyhedron has 180 subunits, where 180/n-polygons have n sides, n should be a positive integer and equal or less than 5.

Proof. A polyhedron is constructed with n-side polygons, the polyhedron has 180/n faces and the number of edges should be

.

By the Euler theorem, , where V, F, and E denote the number of vertices, faces, and edges, respectively,

.

A vertex of solid angle needs at least trimer, then

.

Thus, the maximum of n is 5, where the polygons are pentagons, quadrilaterals, or triangles.

Therefore, a polyhedron with 180 structure protein subunits should not be constructed with 30 hexagons.

Theorem 2. If a polyhedron with 180 subunits is constructed with 36 pentagons, the polyhedron should not be an isohedron or a regular polyhedron.

Proof. If 36 pentagons can construct an isohedron or a regular polyhedron, the number of faces is 36. If two faces form dihedral angles, then the number of edges = 36 × 5 ÷ 2 = 90.

By the Euler theorem,

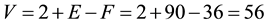
If vertices are constructed with x n-polymers and y m-polymers, where x, y, n, and m are positive numbers, and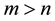, then.

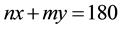Since, that is,

,

the maximum of n is 3.

Thus,.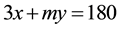

The shell of an isohedron or a regular polyhedron consists of m-polymer units, and y m-polymers consist of 36 faces.

That is

.

The solution of 3 simultaneous equations

,

,

,

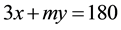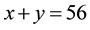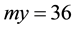is, ,.

This is a contradiction with positive integer m.

Therefore, this theorem suggests that 36 pentagons cannot form an isohedron or a regular polyhedron.

Theorem 3. If a polyhedron with 180 subunits is constructed with an odd number of quadrilaterals, the polyhedron should not be an isohedron or a regular polyhedron.

Proof. Suppose that an isohedron or a regular polyhedron can be constructed with an odd number, 2n + 1, of quadrilaterals.

The number of faces is then 2n + 1, and the number of edges.

By the Euler theorem,

.

The number of vertices is odd, which is a contradiction because an isohedron or a regular polyhedron is symmetrical, and the number of verticies should be even.

Thus, an odd number (such as 45) of quadrilaterals cannot form an isohedron or a regular polyhedron.

According to Theorems 1, 2, and 3, 45 quadrilaterals, 36 pentagons, or 30 hexagons (the polygons with more than 3 edges) cannot form an isohedron or a regular polyhedron with 180 subunits. Only 60 triangles can form a regular polyhedron for packing 180 subunits.

Lemma 4. If a convex solid angle is constructed with n equilateral triangles, n should be 3, 4, or 5.

Proof. In 3D models, a convex solid angle has at least by 3 faces.

For a solid angle, the total angle with 3 equilateral triangular angles is 180˚. The total angle with 4 equilateral triangular angles is 240˚. The total angle with 5 equilateral triangular angles is 300˚. The total angle with 6 equilateral triangular angles is 360˚, which is a cyclic angle.

For a convex solid angle constructed with n equilateral triangles, n should be 3, 4, or 5. The angle of a vertex equal to or greater than 360˚ is flat or concave.

Theorem 5. If a polyhedron is constructed with 60 identical equilateral triangles, the polyhedron is not an isohedron or a regular polyhedron.

Proof. If a polyhedron is constructed with 60 identical equilateral triangles, as in Figure 1, then vertex Q in Figure 1 consists of 6 identical equilateral triangles.

Lemma 4 shows that vertex Q is not a convex solid angle. Instead, vertex Q may belong to the following:

1) Vertex Q is a concave solid angle.

This hypothesis is not true because the polyhedron is convex.

2) Point Q is at the center of hexagon.

Vertex O and pentagon PQRST form a regular pentagonal cone. A dihedral angle forms between triangles OPQ and OQR on line. Thus, polygon is not a regular hexagon, and the hypothesis is not true.

3) Vertex Q locates on a dihedral angle.

Vertex and pentagon form a regular pentagonal cone. Line is on the dihedral angle between triangles and. On the other hand, a dihedral angle forms between triangles OPQ and OQR on line, as in (2). Therefore, two dihedral angles appear at vertex Q, and the hypothesis is not true.

Theorem 6. A polyhedron becomes an icosahedron when the solid angles of the hexamers of the polyhedron spread to be flat and the connecting lines between the vertices of 2 nearby pentagonal cones become the ridges of dihedral angles.

Proof. As Figure 1 shows that the distances between the vertices of any 2 pentagonal cones in Figure 2 are the same. Then,

and

Spreading and flattening the solid angles of the hexamers in the pentagonal cone forms dihedral angles on lines, , , , and. These lines become the edges of pentagonal cones, and vertex O has equilateral triangular faces. The vertices of 12 pentagonal cones have the same number of edges.

Therefore, a polyhedron becomes an icosahedron.

Theorem 7. A polyhedron becomes a dodecahedron when the solid angles of pentamers of the polyhedron spread out and become flat.

Proof. In Figure 3, for triangle OQR, triangle OQR becomes an isosceles triangle when lines and decrease. Because

and

Vertex Q becomes a convex solid angle and the polyhedron becomes a pentakis dodecahedron.

When the edge lines at vertex O decrease to allow the solid angle O to disappear and become flat, the pentagonal cone OPQRST flattens to become a pentagon PQRST.

Finally, the polyhedron becomes a dodecahedron.

3. Results and Discussion

3.1. Mathematical Theorems in Packing a Stable Particle

Theorems 1, 2, and 3 show that 45 quadrilaterals, 36 pentagons, or polygons with 6 or more edges cannot form an isohedron or a regular polyhedron with 180 subunits. Only 60 triangles can form a regular polyhedron for packing 180 subunits. In the MS2 particle, the way to pack 180 coat proteins is to form an isohedron or a regular polyhedron with 60 identical triangles (Lemma 4). The 3D polyhedron constructed with 60 identical equilateral triangles in Figure 1 is not an isohedron or a regular polyhedron (Theorem 5). That is, a polyhedron consisting of 60 identical equilateral triangles is not a convex isohedron. Therefore, a T = 3 MS2 phage with 180 coat protein subunits might become an icosahedron [3] only if the solid angles of hexamers in the polyhedron of 60 identical equilateral triangles spread to become flat and the connecting lines between the vertices of 2 nearby pentagonal cones become the ridges of dihedral angles (Theorem 6). Theorem 7 shows that the MS2 particle model may form a dodecahedron when the solid angles of pentamers spread out and become flat.

The 3D structure of MS2 [3] consists of symmetrical units that lie between 2 threefold axes and 1 fivefold axis. However, a particle with 180 coat protein subunits and T = 3 requires some geometrical adaptations to pack a stable regular polyhedron including an icosahedron or a dodecahedron (Theorem 6 and 7).

An array of hexamers is the basic unit for generating an icosahedron [4] . A hexagon consisting of 6 regular triangles cannot form a solid angle, but lies flat (Lemma 4). Removing a regular triangle from the triangles in the hexagon forms a pentagonal cone. The vertex of the pentagonal cone becomes one of the 12 solid angles of an icosahedron and the 20 triangular faces form part of hexagons [8] .

For MS2 capsids with the principal of quasi-equivalence [8] , the triangulation number in the hexagon net is 3, where h and k are nonnegative integers on the original hexagonal net, h and k cannot be zero simultaneously, and the capsid has 180 structure subunit proteins and 32 morphological units [8] . T values may be 1, 3, 4, 7, 9, 12, 13, 16, 19, 21, 25, etc [1] although T = 2 and 6 appear [9] [10] . When T > 1, the morphological unit appears to form pentamers or hexamers. In an assembly pathway, 5 dimers converge into a pentamer. Twelve pentamers are linked together with free dimers creating a complete particle [2] . According to Theorems 6 and 7, a T = 3 particle can form a regular polyhedron with geometrical degeneration. Although the numbers of subunit proteins vary, the particle morphology is quasi-equiva- lent.

This study hypothesizes that regular Platonic solids allow a single type of asymmetric subunit to assemble into a well-defined spherical structure [7] . The asymmetrical subunit contains 3 subunits, designated as A, B, and C, in an icosahedral particle of phage MS2. Pairwise interactions between the monomers form dimers. The capsid contains 2 types of dimers: one at the quasi-twofold axis composed of subunits A and B and the other at the icosahedral twofold axis consisting of 2 C subunits [7] . Therefore, the capsid is effectively constructed from 90 dimers [7] . The theorems above indicate that the T = 3 phage MS2 particles with 180 protein subunits may by icosahedral, dodecahedral, or pentakis dodecahedral (dual semiregular solid) particles. Therefore, further studies with EM images are necessary to determine if the Platonic solid of MS2 is an icosahedron or a dodecahedron.

3.2. Mathematical Reason with Electron Micrographs of MS2 Particles

With electron micrographs [11] , it is difficult to distinguish between regular hexagons and hexagon-like dodecagons in the projections of icosahedron. Both regular hexagons and regular decagons appear in EM images, though regular decagons appear spherical (Figure 4). However, unequilateral hexagons with large obtuse angles instead of narrow obtuse angles appear in EM images of MS2 in twofold views (Figure 5).

The 5 regular Platonic solids are tetrahedron, octahedron, cube, dodecahedron, and icosahedron. The icosahedron has a common symmetry with the dodecahedron, and the octahedron is similar to the cube [7] . Most studies recognize the bacteriophage MS2 as an icosahedral particle [1] -[3] [6] [12] [13] , though the MS2 coat protein mutant corresponds to T = 3 octahedral particles [9] . The main difference in the subunit packing between the octahedral and icosahedral arrangements is close to the fourfold and fivefold symmetry axes [7] .

The mathematical reason from Theorem 6 and 7 shows that the T = 3 phage MS2 particles may be icosahedral, dodecahedral, or pentakis dodecahedral particles. The projections of icosahedral particles in phage MS2 EM images exhibit unequilateral hexagons in twofold views, regular hexagons in threefold views, and regular decagons in fivefold views (Figure 4). The projections of dodecahedron are unequilateral hexagons in twofold views, hexagon-like dodecagons in threefold views, and regular decagons in fivefold views and asymmetrical views. The projections of pentakis dodecahedra are regular decagons in twofold, threefold, and fivefold views (Table 1). In fivefold views, icosahedra, dodecahedra, and pentakis dodecahedra models show the same projection as a

regular decagon. In threefold views, 3 models have different projections: regular hexagons form from icosahedra, hexagon-like dodecagons form from dodecahedra, and regular decagons form from pentakis dodecahedra. In twofold views, the projections of icosahedra and dodecahedra are unequilateral hexagons (Figure 6). In the unequilateral hexagons, 4 vertices have the same angles, and are unlike the other 2 obtuse angles. The 2 big obtuse angles in the unequilateral hexagons of the projections from icosahedra and dodecahedra are 138˚ and 116˚, respectively [14] . The obtuse angles of unequilateral hexagon projections from icosahedra are much larger than those from dodecahedra. EM images of the MS2 particles show the projections of icosahedral particles since the obtuse angles of unequilateral hexagons are 138˚. The T = 3 phage MS2 particles with 180 coat protein subunits [3] form icosahedra. This suggests that the solid angles of hexamers in the polyhedral particles may spread to become flat, and the connecting lines between the vertices of 2 nearby pentagonal cones become the ridges of dihedral angles during packing the coat protein subunits in MS2 (Theorem 6 and 7). A few viral particles are dodecahedra [4] [15] . In nature, spreading in the solid angles of hexamers in a polyhedron seems to be easier and more common than spreading of pentamers. Most viruses and phages form icosahedral particles instead of dodecahedral or pentakis dodecahedral particles.

3.3. Mathematical Analysis in Particle Electron Micrographs for 3D Reconstruction

Although the 3D structure reconstructed from the EM images shows the Platonic solids of the particles, the wrong order of 3D reconstruction might yield a false solid figure [16] . It is easy to recognize an icosahedron or a dodecahedron in the Platonic solid of a particle using EM images and mathematical analysis. According to a

primary survey of the Platonic solid of the particle, the 3D reconstruction can be performed confidently with a right symmetrical order [10] [14] . The mathematical analysis of EM images is a useful primary survey before performing the 3D reconstruction of a polyhedral particle.

4. Conclusion

In conclusion, a viral particle with 180 coat protein subunits and T = 3 requires some geometrical adaptation to form a stable regular polyhedron, such as an icosahedron or a dodecahedron. The mathematical analysis of MS2 particles reveals the EM projections of icosahedral particles. The MS2 particles are confirmed to be icosahedra.

Acknowledgements

We gratefully acknowledge Mr. Jaw-Fu Gau for his EM assistance.

References

NOTES

^*Corresponding author.