Applied Mathematics
Vol. 3 No. 6 (2012) , Article ID: 20074 , 6 pages DOI:10.4236/am.2012.36077
Existence of Solutions to a Generalized System*
Department of Mathematics, Bohai University, Jinzhou, China
Email: yalizhao2000@yahoo.com.cn, #xinglin251214@yeah.net
Received February 19, 2012; revised April 10, 2012; accepted April 17, 2012
Keywords: Generalized System; C-Strong Pseudomonotonicity; Brouwer’s Fixed Point Theorem; Hemicontinuous Mapping
ABSTRACT
In this paper, we introduce a generalized system (for short, GS) in real Banach spaces. Using Brouwer’s fixed point theorem, we establish some existence theorems for the generalized system without monotonicity. Further, we extend the concept of C-strong pseudomonotonicity and extend Minty’s lemma for the generalized system. And using the Minty lemma and KKM-Fan lemma, we establish an existence theorem for the generalized system with monotonicity in real reflexive Banach spaces. As the continuation of existing studies, our paper present a series of extended results based on existing corresponding results.
1. Introduction
Variational inequality theory has played a fundamental and important role in the study of a wide range of problems arising in physics, mechanics, differential equations, contact problems in elasticity, optimization, economics and engineering sciences, etc. As a useful and important branch of variational inequality theory, vector variational inequalities were initially introduced and considered by Giannessi [1] in a finite-dimensional space in 1980. Ever since then, vector variational inequalities have been extensively studied and generalized in infinite-dimensional spaces.
Very recently, Fang and Huang [2] studied some existence results for strong vector variational inequalities in Banach spaces. Long, Huang and Teo [3] established an existence theorem of solutions for a generalized strong vector quasi-equlilbrium problem by using KakutaniFan-Glicksberg fixed point theorem. Li and He [4] studied the existence of solutions for VVI with a single-valued function and a continuous selection theorem and obtained the existence theorem for the GVVI under the assumption of 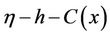 -pseudomonotonicity.
-pseudomonotonicity.
Motivated and inspired by research works mentioned above, in this paper, we consider a generalized system, which includes as special cases the strong vector variational inequalities [1,2] and problems so called equilibrium problems [5,6]. In order to derive the existence of solutions for the generalized system, Using Brouwer’s fixed point theorem, we obtain some existence results for the generalized system without monotonicity. Furthermore, by the concepts of -C-continuous, C-strong pseudomonotonicity and hemicontinuity, we extend Minty’s lemma, moreover, with the help of Minty’s lemma and KKM-Fan lemma, we establish an existence theorem for the generalized system with monotonicity in real reflexive Banach spaces. The results presented in this paper extend the corresponding results of [2,6], and Theorems 3.1-3.3 can be considered as a generalization of Theorems 2.1-2.3 in [6].
2. Preliminaries
Throughout the paper, otherwise special statement, let X and Y be two real Banach spaces, let 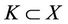 be a nonempty, closed and convex set, let
be a nonempty, closed and convex set, let 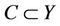 be a solid, pointed, closed and convex cone with apex at the origin. Let
be a solid, pointed, closed and convex cone with apex at the origin. Let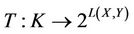 ,
,  be the space of all continuous linear mappings from X to Y. Let
be the space of all continuous linear mappings from X to Y. Let 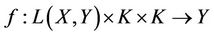 be a trifunction such that
be a trifunction such that 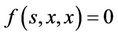 for all
for all 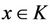 and
and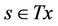 . Then we consider the following generalized systems (for short, GS): Find
. Then we consider the following generalized systems (for short, GS): Find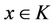 , for each
, for each  there exists
there exists  such that
such that
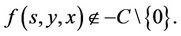 (1)
(1)
And find 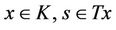 such that
such that
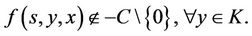 (2)
(2)
We know that a solution  is called a weak solution and a strong solution to GS(1) and GS(2), respectively. It is easy to see that a solution to GS(2) is a solution to GS(1), but in general the converse is not true. Noting
is called a weak solution and a strong solution to GS(1) and GS(2), respectively. It is easy to see that a solution to GS(2) is a solution to GS(1), but in general the converse is not true. Noting ,
,  , where
, where  is a single-valued mapping,
is a single-valued mapping, 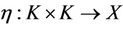 is a nonlinear mapping, then GS(1)and GS(2) both reduces to the following strong vector variational-like inequality problem (for short, SVVLIP): Find
is a nonlinear mapping, then GS(1)and GS(2) both reduces to the following strong vector variational-like inequality problem (for short, SVVLIP): Find  such that
such that
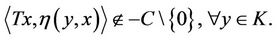 (3)
(3)
Now, we recall the following definitions.
Definition 2.1 [7]. Let K be a subset of a topological vector space X. A set-valued mapping 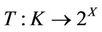 is called a KKM mapping if, for each nonempty finite subset
is called a KKM mapping if, for each nonempty finite subset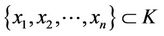 , we have
, we have 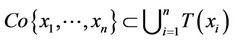 where CoA denotes the convex hull of the set A.
where CoA denotes the convex hull of the set A.
Definition 2.2. Let X and Y be topological vector spaces, let K be a nonempty, convex subset of X and let C be a convex cone in Y. A mapping 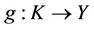 is said to be C-convex function if, for all
is said to be C-convex function if, for all  and
and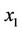 ,
,  ,
,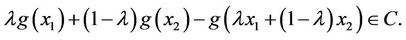
Remark 2.1. 1) If C contains (or is equal to,or is contained in) the non-negative orthant, then the C-convex function is called C-convex (or convex, or strictly Cconvex);
2) If C contains (or is equal to, or is contained in) the nonpositive orthant, then the C-convex function is called C-concave (or concave, or strictly C-concave).
Theorem 2.1. (Brouwer’s fixed point theorem [8]) Let K be a nonempty, compact and convex subset of a finite-dimensional space X and let  be a continuous mapping. Then, there exists
be a continuous mapping. Then, there exists 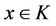 such that
such that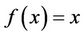 .
.
Theorem 2.2. (KKM-Fan Lemma [7]) Let K be a subset of a topological vector space X and let 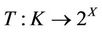 be a KKM mapping. If, for each
be a KKM mapping. If, for each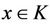 ,
,  is closed and for at least one
is closed and for at least one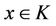 ,
, 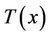 is compact, then
is compact, then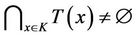 .
.
3. Main Results
In this section, we shall obtain the following existence theorems for GS(1).
Theorem 3.1. Let K be a nonempty, compact and convex subset of X and let the mapping  be a C-convex function in the second argument. Assume that, for every
be a C-convex function in the second argument. Assume that, for every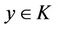 , the set
, the set  is open in K. Then, GS(1) has a solution.
is open in K. Then, GS(1) has a solution.
Proof. We proceed by contradiction. Assume that GS(1) admits no solution, then for each , there exists some
, there exists some 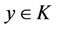 and for all
and for all  such that
such that
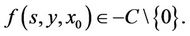 (4)
(4)
For every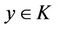 , define the set
, define the set 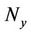 as follows:
as follows:
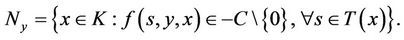 (5)
(5)
By given assumption, the set  is open in K and hence from (4), it follows that
is open in K and hence from (4), it follows that 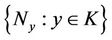 is an open cover of K. Since K is compact, there exists a finite set
is an open cover of K. Since K is compact, there exists a finite set 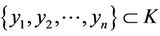 such that
such that . So there exists a continuous partition of unity
. So there exists a continuous partition of unity 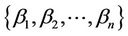 subordinate to
subordinate to  such that for all
such that for all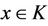 ,
, 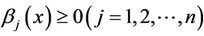 with
with 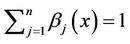 and
and  whenever
whenever ,
, 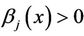 whenever
whenever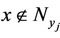 .
.
Define a mapping  by
by
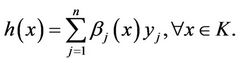 (6)
(6)
Since 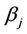 is continuous for each j, it follows from (6) that h is also continuous. Let
is continuous for each j, it follows from (6) that h is also continuous. Let . Then S is a simplex of a finite dimensional space and h maps S into S. By Theorem 1.1, there exists some
. Then S is a simplex of a finite dimensional space and h maps S into S. By Theorem 1.1, there exists some 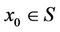 such that
such that .
.
Next define a mapping  by
by 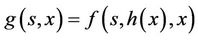 . Since f is a C-convex function in the second argument, by (6), we have
. Since f is a C-convex function in the second argument, by (6), we have
 (7)
(7)
For any given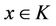 , let
, let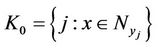 . Clearly,
. Clearly, 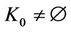 . since
. since 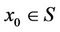 is a fixed point of h, it follows from (4) and (7) that for all
is a fixed point of h, it follows from (4) and (7) that for all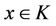 ,
,

That is, 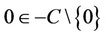 , a contradiction. Hence, GS(1) has a solution. This completes the proof.
, a contradiction. Hence, GS(1) has a solution. This completes the proof.
Example 3.1. Let ,
,  ,
,  ,
, 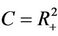 ,
,
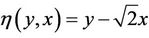 ,
, 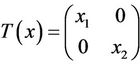 ,
, .
.

For all 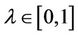 and
and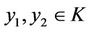 ,
,

i.e.,
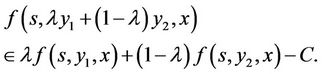
Then it is easy to see that f is a C-convex function in the second argument, and the
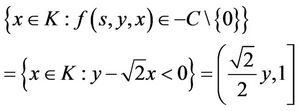
is open in K. Now we could say that all conditions of Theorem 2.1 hold. And also, the solutions set for GS(1)
in Theorem 2.1 is ,
,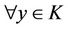 .
.
Theorem 3.2. Let K be a nonempty, closed and convex subset of X and let the mapping 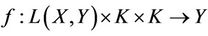 be a C-convex function in the second argument. Assume that:
be a C-convex function in the second argument. Assume that:
1) For every , the set
, the set
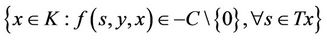 is open.
is open.
2) K is locally compact and there is an 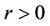 and
and ,
, 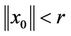 , such that, for all
, such that, for all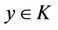 ,
, 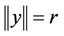 , there exists an
, there exists an 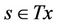 such that
such that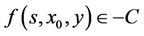 .
.
Then, GS(1) has a solution.
Proof. Let . Since K is locally compact,
. Since K is locally compact,  is compact; hence, it follows from Theorem 2.1 that there exists an
is compact; hence, it follows from Theorem 2.1 that there exists an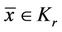 , for all
, for all 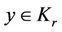 there exists
there exists 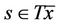 such that
such that
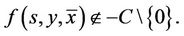 (7)
(7)
We claim that  is the desired solution of GS(1). Indeed:
is the desired solution of GS(1). Indeed:
1) If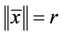 , by the assumption 2), there exists
, by the assumption 2), there exists 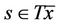 such that
such that
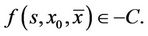 (8)
(8)
For any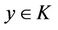 , choose
, choose 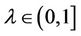 such that
such that  . Then, from (7), it follows that
. Then, from (7), it follows that . Since f is a C-convex function in the second argument, we have
. Since f is a C-convex function in the second argument, we have


Implying that
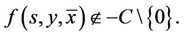
2) If , for any
, for any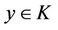 , choose
, choose 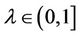 such that
such that . Then, it follows from (7) that
. Then, it follows from (7) that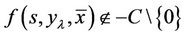 . Since f is a C-convex function in the second argument, we have
. Since f is a C-convex function in the second argument, we have
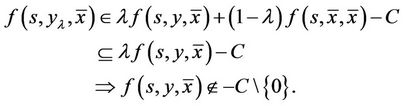
This completes the proof.
The following example shows that the assumption that the set ![]() is open in K, for every
is open in K, for every , is not trivial. For two similar examples for a vector-valued function, see [2,6].
, is not trivial. For two similar examples for a vector-valued function, see [2,6].
Example 3.2. Let ,
, 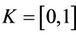 ,
,  ,
, 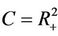 ,
,
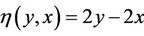 ,
, 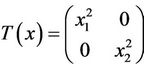 ,
, 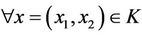 ,
,
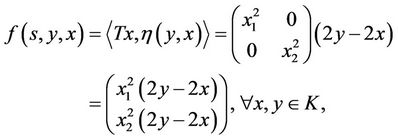
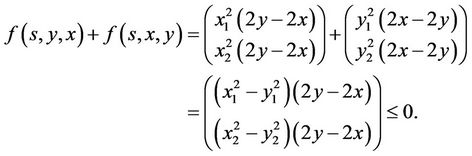
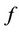 is continuous and monotone in the sense that
is continuous and monotone in the sense that 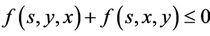 for all
for all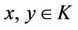 . Also, it is easy to see that, for each
. Also, it is easy to see that, for each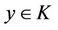 , the set
, the set 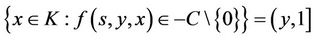 and hence is open in K.
and hence is open in K.
Next, we define following concepts which will be used in the sequel.
Definition 3.1. A mapping  is said to be C-strong pseudomonotone respect to
is said to be C-strong pseudomonotone respect to 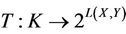 if, for any
if, for any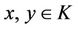 , there exists
, there exists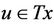 ,
, 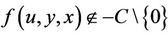 implies
implies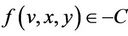 ,
,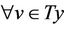 .
.
Remark 3.1. This definition generalizes corresponding definitions of [9,10].
Example 3.3. Let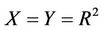 ,
,  ,
,  ,
,
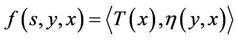 ,
, 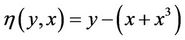 ,
,
 ,
,  ,
,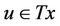 .
.
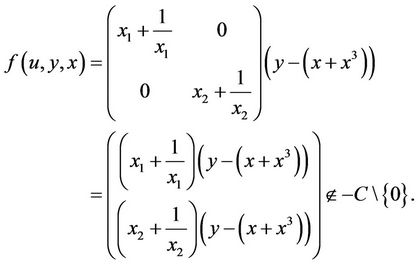
That implies that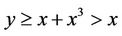 , it follows that
, it follows that
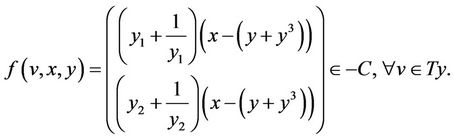
Hence, f is C-strong pseudomonotone. For a similar example for a vector-valued function, see [6].
Definition 3.2. A mapping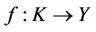 , is said to be −C-continuous at
, is said to be −C-continuous at  if, for each neighbourhood of nullelement in Y, there exists a neighbourhood of X, such that
if, for each neighbourhood of nullelement in Y, there exists a neighbourhood of X, such that
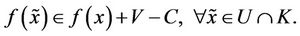
If f is −C-continuous at each point of K, then f is −C-continuous on K.
Definition 3.3. Let W, E be two topological spaces, a set-valued mapping  is upper semicontinuous at
is upper semicontinuous at 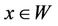 if, for each neighbourhood V of Tx, there exists a neighbourhood U of x, such that
if, for each neighbourhood V of Tx, there exists a neighbourhood U of x, such that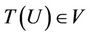 . If T is upper semicontinuous at each point of W, then T is upper semicontinuous on W.
. If T is upper semicontinuous at each point of W, then T is upper semicontinuous on W.
Lemma 3.1 [11]. Let W, E be two topological spaces, a set-valued mapping 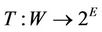 is compact valued, then T is said to be upper semicontinuous at
is compact valued, then T is said to be upper semicontinuous at 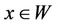 if and only if: for any net
if and only if: for any net ,
, 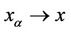 , and
, and ,
, 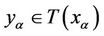 , there exists a setnet
, there exists a setnet  of
of , such that
, such that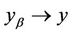 .
.
Now we prove the existence result for GS(2) with monotonicity. First, we prove the following Minty’s type lemma for GS(2).
Lemma 3.2. Let K be a nonempty closed and convex subset of X, Y be a real Banach space ordered by a nonempty closed convex pointed cone C with apex at the origin and . Let
. Let 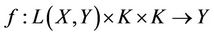 be a trifunction,
be a trifunction, 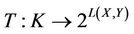 be a nonempty compact set-valued mapping. Suppose the following conditions hold:
be a nonempty compact set-valued mapping. Suppose the following conditions hold:
1)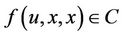 ,
,  ,
, ;
;
2)  is C-convex function in the second argument;
is C-convex function in the second argument;
3) T is upper semicontinous;
4)  is -C-continuous with respect to
is -C-continuous with respect to ,
, 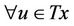 ,
,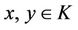 .
.
Then there exists  and
and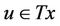 , such that
, such that
 (9)
(9)
if and only if there exists 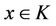 such that
such that
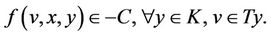 (10)
(10)
Proof. (9) 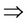 (10): It follows from the C-strong pseudomonotonicity of f respect to T.
(10): It follows from the C-strong pseudomonotonicity of f respect to T.
(10) 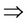 (9): Suppose that there exists
(9): Suppose that there exists 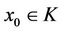 such that
such that
 (11)
(11)
If 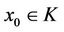 is not the solution of (9), that is to say,
is not the solution of (9), that is to say, 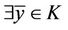 ,
, 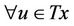 , we have
, we have
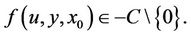 (12)
(12)
For any given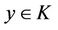 , we set
, we set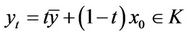 ,
,  , for each
, for each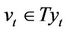 , it follows that
, it follows that
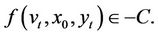 (13)
(13)
Since f is C-convex function in the second argument, we have
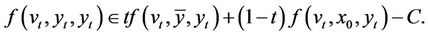 (14)
(14)
It follows from inclusions (13), (14) and condition 1), we obtain

Implying that
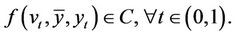 (15)
(15)
From T is upper semicontinous and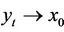 ,
, 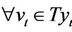 , as
, as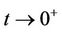 , we obtain that
, we obtain that 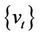 has a setnet (for simplicity, still denoted
has a setnet (for simplicity, still denoted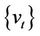 ) and there exists
) and there exists , such that
, such that .
.
If we could prove
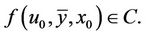 (16)
(16)
we will obtain a contradiction between (16) and (12), as C is convex pointed cone. That will complete the proof.
In fact, suppose on the contrary that
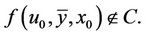
Since C is closed, we know that there exists a origin neighbourhood V in Y, such that
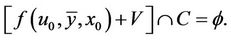
Since C is convex cone, it follows that
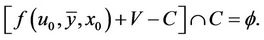 (17)
(17)
From 4), there exists neighbourhood  of
of 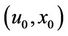 such that
such that
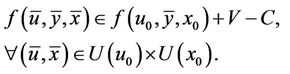 (18)
(18)
Since ,
, 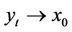 , as
, as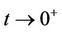 ,
, 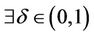 , such that
, such that

Thus from (18) we get
 (19)
(19)
it follows (17) and (19) that

which leads a contradiction to (15). This completes the proof.
Theorem 3.3. Let K be a nonempty, closed, bounded and convex subset of a real reflexive Banach space X and let the mapping  be a C-convex function in the second argument and C-strong pseudomonotone.
be a C-convex function in the second argument and C-strong pseudomonotone. 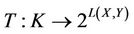 be a nonempty compact setvalued mapping. Then, GS(2) has a solution.
be a nonempty compact setvalued mapping. Then, GS(2) has a solution.
Proof. Define two set-valued mappings,  by
by

and
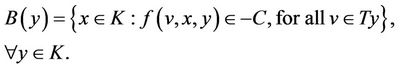
Clearly, 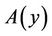 and
and 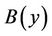 both are nonempty since
both are nonempty since 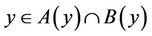 for all
for all .
.
We claim that A is KKM-mapping. If assertion were false, then there would exists 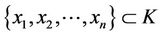 and
and ,
,  , with
, with  such that
such that
 . Hence for any
. Hence for any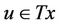 , we have
, we have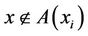 , for each i.
, for each i.
Implying that,
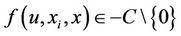 . Thus,
. Thus,
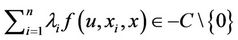 . Since f is a C-convex function in the second argument, we have
. Since f is a C-convex function in the second argument, we have

which is a contradiction. Hence A is a KKM-mapping.
Since f is C-strong pseudomonotone, it follows that 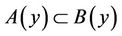 for all
for all , and hence B is also a KKM-mapping. By Lemma 2.1, we see that
, and hence B is also a KKM-mapping. By Lemma 2.1, we see that
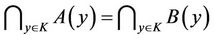 .
.
Next we claim that for each fixed ,
,  is bounded, convex and closed in K. Indeed,
is bounded, convex and closed in K. Indeed, 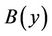 is bounded as
is bounded as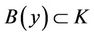 . Let
. Let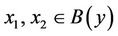 , then we have
, then we have

Since f is C-convex function in second argument, we have that, for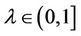 ,
,
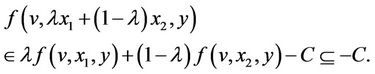
This implies that 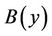 is convex for each fixed
is convex for each fixed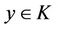 . The continuity of f in the second argument and closedness of -C give the closedness of
. The continuity of f in the second argument and closedness of -C give the closedness of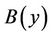 . We now equip X with that weak topology. Since
. We now equip X with that weak topology. Since  is closed, bounded and convex subset of the reflexive Banach space X, then it turns out to be weakly compact for all
is closed, bounded and convex subset of the reflexive Banach space X, then it turns out to be weakly compact for all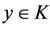 . Hence by Theorem 1.2, we have
. Hence by Theorem 1.2, we have . This implies that there exists
. This implies that there exists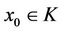 , such that
, such that

Therefore by Minty’s type Lemma 3.2, we conclude that there exists  and
and 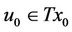 such that
such that
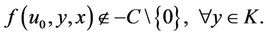
That is to say GS(2) has a solution. This completes the proof.
Remark 3.2. Let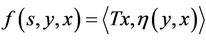 ,
, 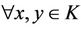 , where
, where 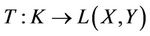 is a single-valued mapping,
is a single-valued mapping, 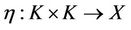 is a nonlinear mapping, then GS(1) and GS(2) both reduces to the SVVLIP(3). As applications, we have the following existence result for SVVLIP(3).
is a nonlinear mapping, then GS(1) and GS(2) both reduces to the SVVLIP(3). As applications, we have the following existence result for SVVLIP(3).
Corollary 3.1. Let K, X, T, 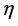 be as in Theorem 3.1 and Remark 3.2, let
be as in Theorem 3.1 and Remark 3.2, let 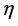 be affine in the first argument. For each
be affine in the first argument. For each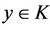 , the set
, the set 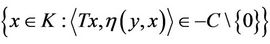 is open in K. Then the SVVLIP(3) has a solution.
is open in K. Then the SVVLIP(3) has a solution.
Corollary 3.2. Let K, X, T, 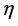 be as in Corollary 3.1. Assume that:
be as in Corollary 3.1. Assume that:
1) For every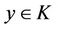 , the set
, the set
 is open.
is open.
2) K is locally compact and there is an  and
and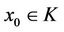 ,
, 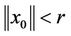 , such that for all
, such that for all ,
, 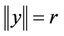 ,
, 
Then, the SVVLIP(3) has a solution.
REFERENCES
- F. Giannessi, “Theorem of Alternative, Quadratic Programs, and Complementarity Problems,” In: R. W. Cottle, F. Giannessi and J. L. Lions, Eds., Variational Inequality and Complementarity Problems, John Wiley and Sons, Chichester, 1980, pp. 151-186.
- Y.-P. Fang and N.-J. Huang, “Strong Vector Variational Inequalities in Banach Spaces,” Applied Mathematics Letters, Vol. 19, No. 4, 2006, pp. 362-368. doi:10.1016/j.aml.2005.06.008
- X. J. Long, N. J. Huang and K. L. Teo, “Existence and Stability of Solutions for Generalized Strong Vector Quasi-Equilibrium Problem,” Mathematical and Computer Modelling, Vol. 47, No. 3-4, 2008, pp. 445-451. doi:10.1016/j.mcm.2007.04.013
- J. Li and Z. Q. He, “Gap Functions and Existence of Solutions to Generalized Vector Variational Inequalities,” Applied Mathematics Letters, Vol. 18, 2005, pp. 989- 1000. doi:10.1016/j.aml.2004.06.029
- E. Blum and W. Oettli, “From Optimization and Variational Inequalities to Equilibrium Problems,” Math Students, Vol. 63, No. 1-4, 1994, pp. 123-145.
- K. R. Kazmi and S. A. Khan, “Existence of Solutions to a Generalized System,” Journal of Optimization Theory and Applications, Vol. 142, No. 2, 2009, pp. 355-361. doi:10.1007/s10957-009-9530-7
- K. Fan, “A Generalization of Tychonoff’s Fixed-Point Theorem,” Mathematische Annalen, Vol. 142, 1961, pp. 305-310. doi:10.1007/BF01353421
- L. E. J. Brouwer, “Zur Invarianz des n-Dimensional Gebietes,” Mathematische Annalen, Vol. 72, 1912, pp. 55-56. doi:10.1007/BF01456889
- Q. G. Liao and J. Y. Fu, “Systems of Generalized Vector Quasi-Equilibrium Problems with Pseudo-Monotone Mappings,” Journal of Nanchang University (Natural Science), Vol. 35, No. 1, 2011, pp. 12-15.
- F. Giannessi, “Vector Variational Inequalities and Vector Equilibria,” Mathematical Theoreies, Kluwer Academic Publishers, Berlin, 2000.
NOTES
*This work is supported by the Doctoral Initiating Foundation of Liaoning Province (20071097) and partially supported by the National Natural Science Foundation of China (61070242).
#Corresponding author.

