Energy and Power Engineering
Vol.5 No.9(2013), Article ID:39781,13 pages DOI:10.4236/epe.2013.59058
Towards Economic Single-Phase Motor
Faculty of Engineering Technology, Al-Balqa’ Applied University, Amman, Jordan
Email: Mahdism@hotmail.com
Copyright © 2013 Mahdi Alshamasin. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received September 6, 2013; revised October 6, 2013; accepted October 13, 2013
Keywords: Performance of capacitor-Run Motor; Main Phase; balanced operation; symmetry; connection circuit diagrams; control of balancing parameters; reactive elements; power factor; efficiency
ABSTRACT
Studying of operation balance in single-phase induction motors is an issue of interest due to the need for reducing the power consumption and increasing the motors’ life. The paper focuses on improving the motor performance by balancing the stator phase operation for the most common-used connection diagrams of single-phase capacitor-run induction motors (SPCRIMs) and three-phase induction motors (TPIMs) operating from single-phase supply (SPS). Therefore, a mathematical model is used to balance the motor operation by varying the frequency supply voltage. Characteristics of balancing parameters are investigated, various methods of motor balancing are presented and comparisons were done among these balancing methods.
1. Introduction
Economic single phase motors are required nowadays since a huge amount of power is consumed due to the wide using of these motors in the life fields such as: domestic, agricultural, industrial fields and so on [1-3].
By improving the performance of single-phase motors, farms, petroleum wells, homes and faraway workshops having only a single-phase line do not need to install expensive three-phase lines or resort to expensive inverters or diesel pumps. Also, in many applications, it may be necessary to use a three-phase induction motor on a single-phase supply system. For example, technical and economic advantages have been found to initially install a single wire earth Return (SWER) system for rural electrification in remote and hilly regions [4].
For single-phase capacitor-run induction motor (SPCRIM) and three-phase induction motor (TPIM) operating from single-phase supply (SPS), full-load current may have almost unity power factor which reduces the power company transformers and distribution losses. At balanced motor operation, the efficiency of single-phase motors may exceed 90 percent. Thus, single-phase motor performance can be improved and become competitive to that of three-phase motor on a three-phase line. Using the SPCRIMs is the best choice to compete the three-phase motors; whereas the run capacitor can improve the motor efficiency, the starting torque and the power factor. Also, using of additional reactive elements leads to robust motor balancing to ensure the excellent performance of the motor [5].
As a matter of fact, SPCRIM and TPIM fed from SPS suffer from heating due to the elliptical field caused by asymmetry of phase loads [6]. The non-uniform operation of stator phases of these motors is negatively reflected on the winding temperature, the power factor and the efficiency of the motor [7,8]. Therefore, the elimination of the asymmetric action is of a great theoretical and practical significance.
The conventional connection diagrams of SPCRIMs and TPIMs operating from SPS [9,10] using a constant capacitance value in a stator circuit supplied by constant frequency voltage, are not capable to provide balanced operation of stator phase in the whole range of motor slip [11]. This is due to the ellipticity of a rotating field, which takes a circular form only under certain conditions. In this case, balancing is possible only at a certain value of slip and load fluctuations will cause the unbalancing of the motor and produce heating in the motor windings [12]. Eliminating the asymmetry of phase loads is possible by using the following methods:
1) Using one value phase-shifting capacitor and regulating the frequency of the power supply.
2) Inserting of external reactive impedance into the circuit of the SPCRIM or TPIM fed from SPS. This is the most suitable method to provide the required values of phase currents and appropriate angles between them (rigorous symmetry).
3) Switching a number of stator winding turns and regulating the value of phase-shifting capacitance [13,14], which is regarded as the most economical method from the point of view of electrical energy utilization and motor heating. This paper develops a mathematical model to balance the motor operation by varying the frequency of supply voltage and investigating the characteristics of the balancing parameters. Also, the paper presents advanced review for the used methods of balancing, comparison among them through behavior investigation and limitations of each method for the most used connection diagrams of induction motors fed from single-phase supply in practical applications.
2. Balancing of the Motor Operation by Controlling the Supply Frequency
The produced field in the SPCRIM and TPIM operating from SPS can have a rectilinear, an elliptical or a circular form, depending on the reactance of the phase-shifting capacitor. Of course, the machine will have the best efficiency and power factor when the field has a circular form. Thus, phase currents are equal in magnitude and the phase angle between them is 90 electrical degrees at SPCRIMs or 120 electrical degrees at TPIM operating from SPS. The reactance can be controlled by varying the frequency of the supply voltage and under certain conditions at which negative sequence current  becomes zero the motor operation is balanced [15]. The capacitor reactance value that satisfies the first balancing condition can be calculated from the following relation:
becomes zero the motor operation is balanced [15]. The capacitor reactance value that satisfies the first balancing condition can be calculated from the following relation:
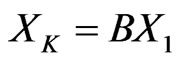 (1)
(1)
the second balancing condition is:
 (2)
(2)
where A and B are the balancing coefficients
2.1. Balancing Conditions of SPCRMs with Two Windings Connected in Parallel
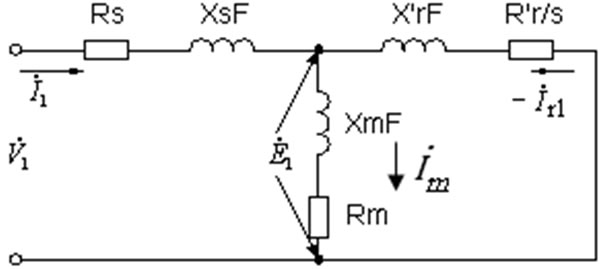
Circuit diagram of SPCRIM with two windings connected in parallel is shown in Figure 1. Utilizing the symmetrical components methods, the unbalanced motor variables can be decomposed into positive (forward) sequence and negative (backward) sequence components [16,17]. Figure 2 shows the equivalent circuit of these components [18].
According to the symmetrical components method, the phase currents can be written as [16,19]
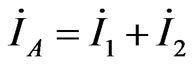 (3)
(3)
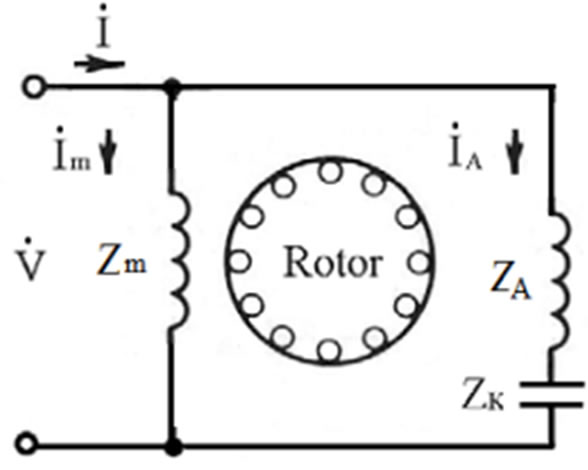
Figure 1. Circuit diagram of SPCRM with two windings connected in parallel.
 (a)
(a)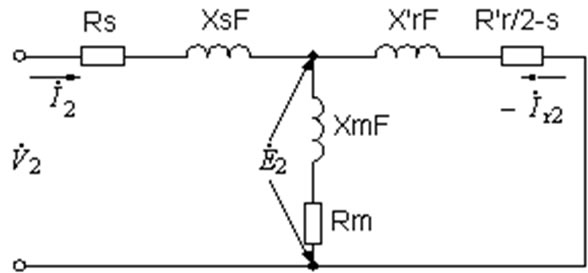 (b)
(b)
Figure 2. Per-phase equivalent circuit; (a) Positive sequence, (b) Negative sequence.
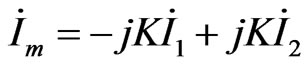 (4)
(4)
From Kirchhoff's Law, the voltages that model the SPCRM are
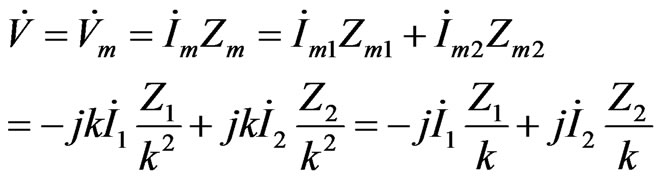 (5)
(5)
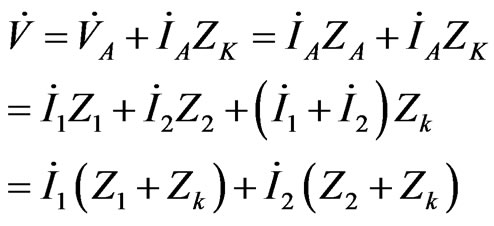 (6)
(6)
where
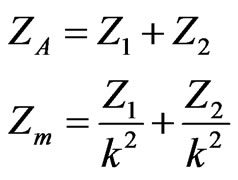
From equations (5) and (6), the balance equation (at which 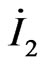 becomes zero) is
becomes zero) is
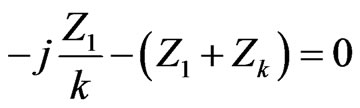 (7)
(7)
Substituting real and imaginary parts of the impedances then gives
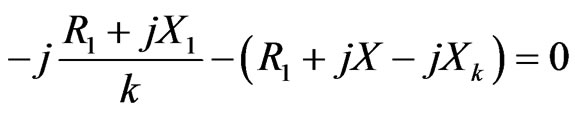 (8)
(8)
Solving this equation yields
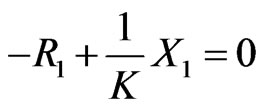 (9)
(9)
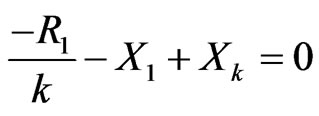 (10)
(10)
From equations (9) and (10), we get
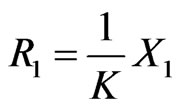
and
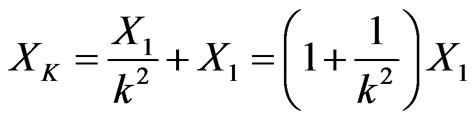
Thus, the balance coefficients are
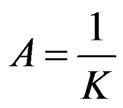
and

2.2. Balancing Conditions of Three-Phase Induction Motor Fed From Single-Phase Supply
The circuit diagram of TPIM connected in delta and operating from SPS is shown in Figure 3.
From Kirchhoff’s Laws, the voltages and currents are
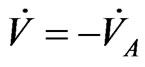 (11)
(11)
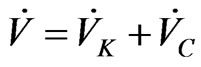 (12)
(12)
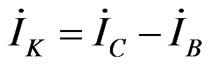 (13)
(13)
Substituting the symmetrical components for voltages and currents into equations (11) and (12) gives [20]:
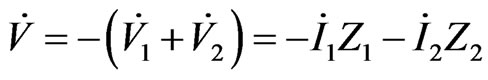 (14)
(14)
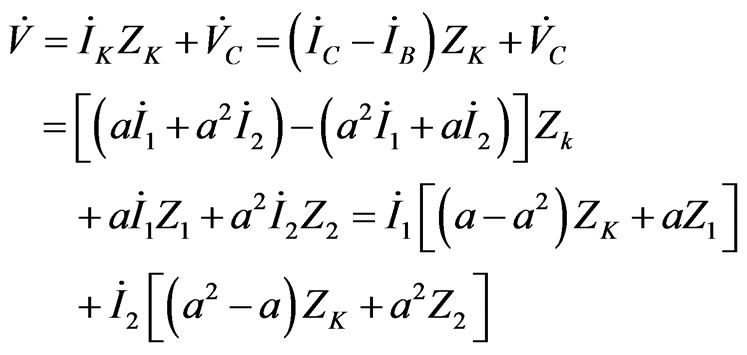 (15)
(15)
with a balanced condition
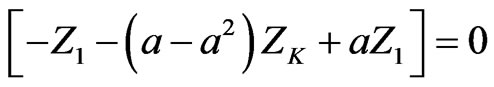 (16)
(16)
therefore,
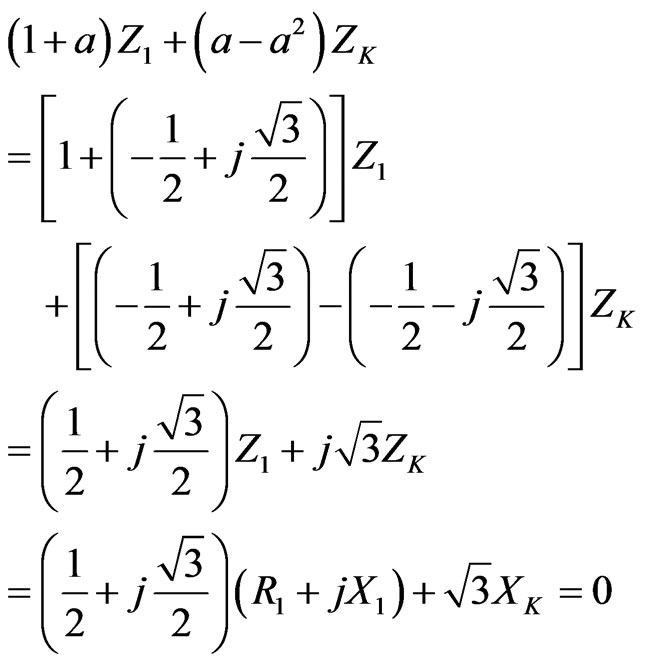 (17)
(17)
this implies
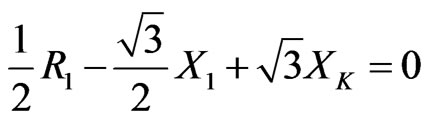 (18)
(18)
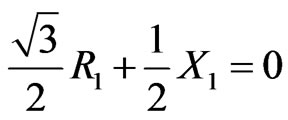 (19)
(19)
By solving equations (18) and (19), we get
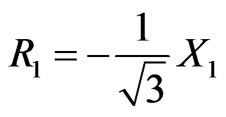
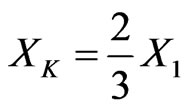
As a result, the balancing coefficients are
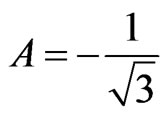 and
and 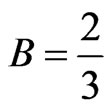
Using the same procedures of analysis, balancing coefficientsfor the least circuit diagrams of SPCRIM and TPIM fed from SPS can be derived. Balancing coefficients are found to be as in Table 1.
Further, when the frequency is kept constant, equation (2) is satisfied at certain value of slip. Varying of the slip (S) leads to variation of the stator currents while for certain values of the slip specifically S = Ssym, the stator currents will equal each other [21]. The phase-angle between the phase currents that required establishing the balance can be obtained by using shifting capacitor. In other words, for any slip (S) there is a certain frequency (fsym) at which motor will be balanced. In order to find
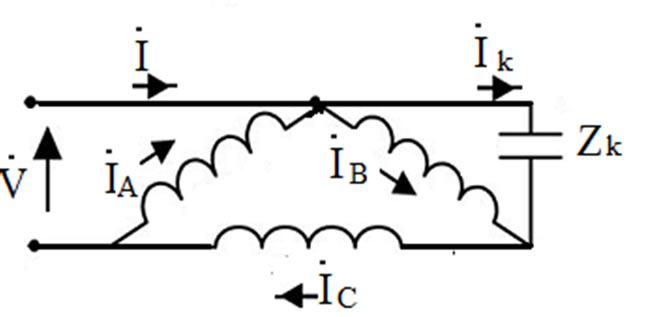
Figure 3. Circuit diagram of three-phase Δ -connected motor fed from single-phase supply.
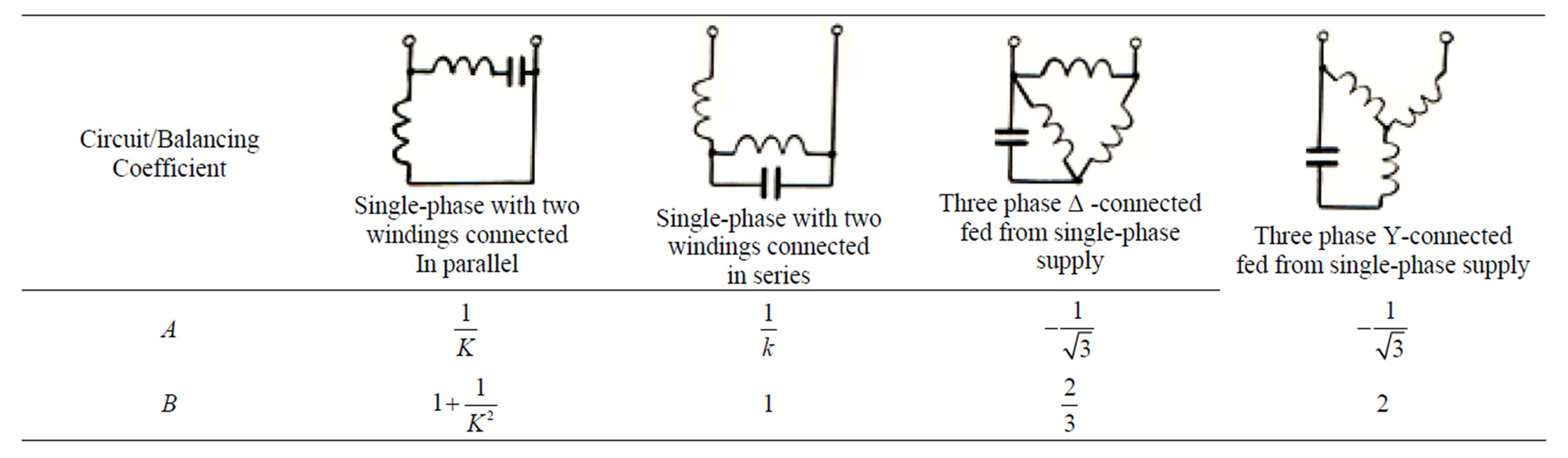
Table 1. Balancing coefficients for the common used connection circuits.
the frequency at which a balanced operation of the motor is achieved for different values of slip, the values of R1 and X1 must be found from the equivalent circuit of single phase motor in figure 2(a) as
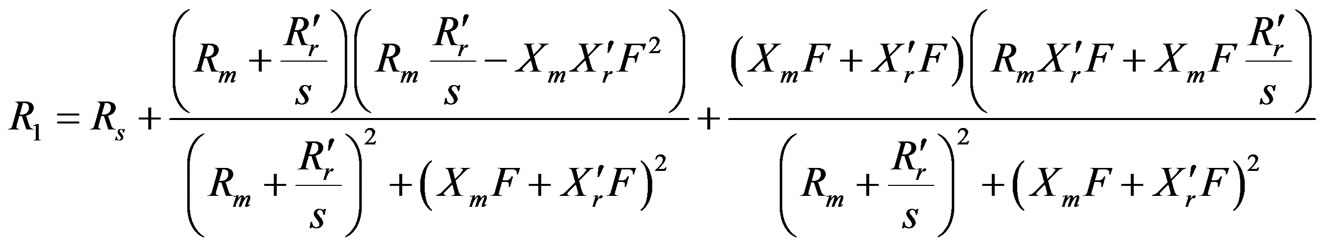 (20)
(20)
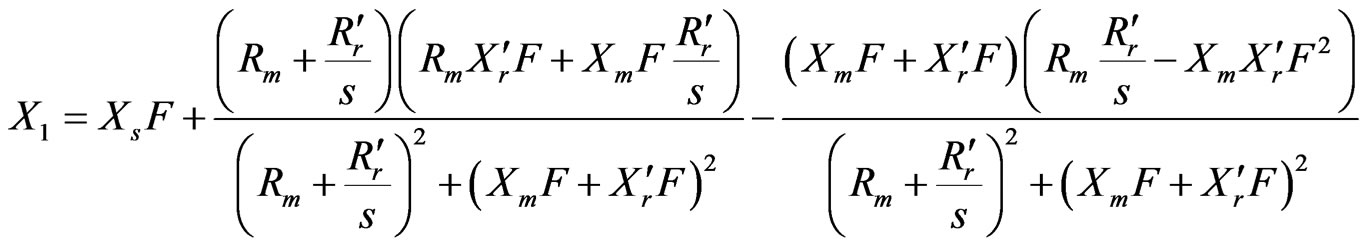 (21)
(21)
Substituting R1 and X1 from equation (20) and equation (21) into equation (2) and rearranging the obtained equation with neglecting of stator active resistance, the per-unit balancing frequency 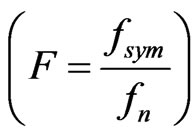 can be found as:
can be found as:
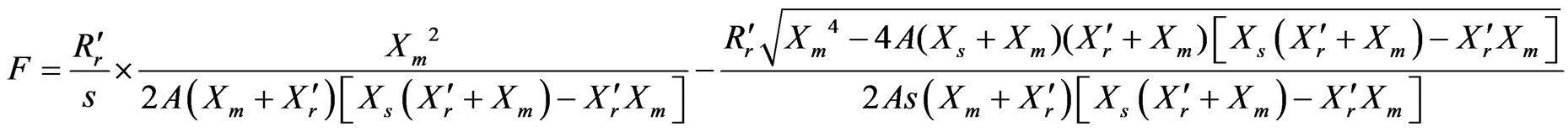 (22)
(22)
For low and medium power motors, one may consider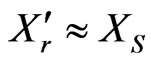 , and then the per-unit balancing frequency can be calculated as:
, and then the per-unit balancing frequency can be calculated as:
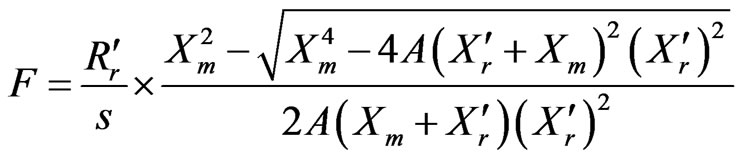 (23)
(23)
when the frequency value is given, the slip at which the motor operation is balanced can be derived as:
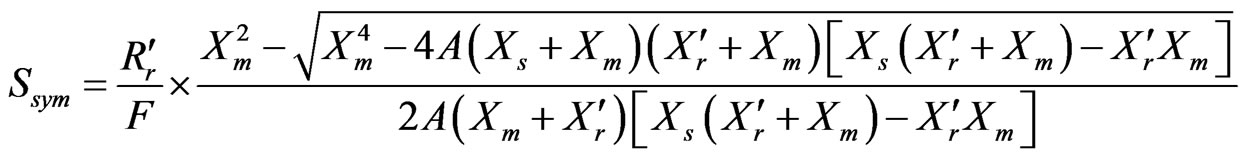 (24)
(24)
The critical slip (slip at maximum torque) is a function of frequency and can be calculated from the expression [22]
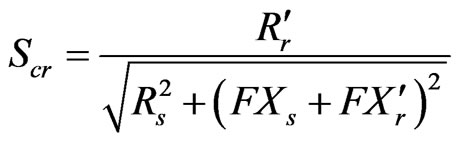 (25)
(25)
3. Balancing the SPCRIM by Inserting an Inductive Reactance into the Stator Circuit
The values of the balancing impedance (inductive and capacitive) for the most common connection diagrams of SPCRIMs and TPIMs fed from SPS can be determined by the following group of equations [5]:
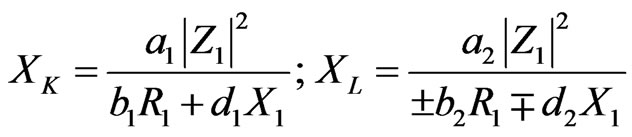 (26)
(26)
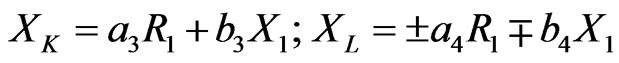 (27)
(27)
where, the coefficients of equations (26) and (27) can be obtained from Table 2.
4. Balancing the Motor Operation by Controlling the Capacitance Value
In this method, the frequency is constant and frequently is equal to the nominal frequency whereas the capacitance is varying to satisfy the balance operation when the load is changing. The balancing capacitor value can be controlled electronically [4,23].
Balancing of SPCRM with Two Windings Connected in Parallel
For the SPCRIM with two windings connected in parallel where the capacitor value is varying in respect with the load as in Figure 4, the balancing capacitance value can be calculated from the equations (5) and (6) by equating the absolute values of 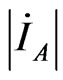 and
and 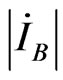 as [10,24].
as [10,24].
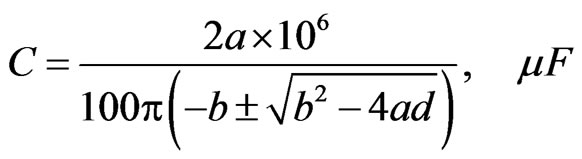 (28)
(28)
where
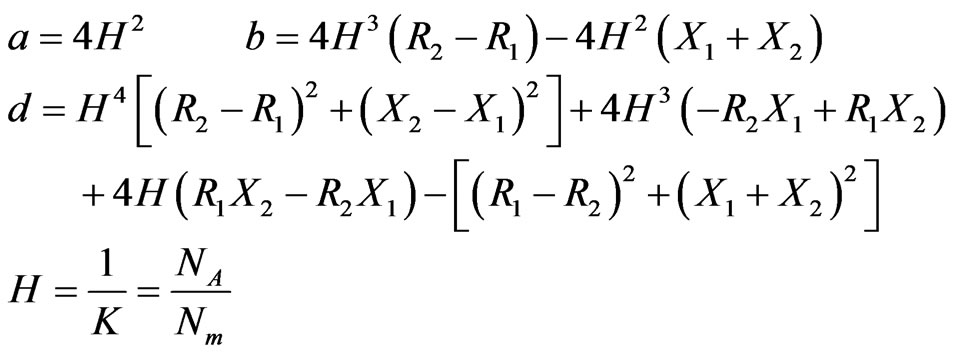
5. Simulation and Results
Curves of balancing parameters XK, Ssym and Scr versus frequency ,depending on the equations (1) , (24) and (25), were investigated by using labVIEW software for the SPCRIM and TIM operating from SPS with the following data:
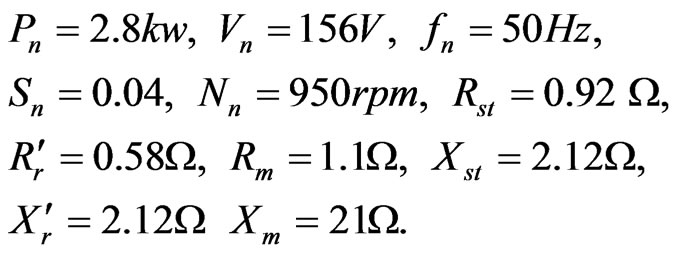
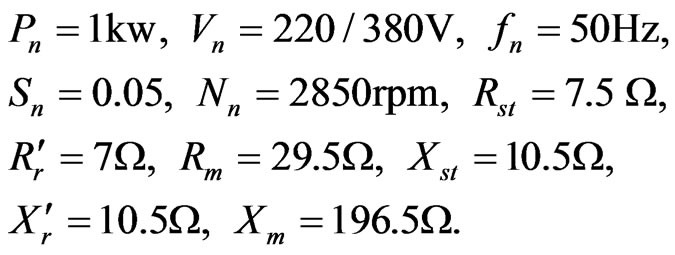
Figures 5-8 show the obtained curves for the most used connection diagrams.
It can be seen from these figures that Ssym is inversely proportional to the frequency, where its value at low frequencies approaches 1. This means that the motor can be started with a balanced status and this is considered a very important aspect in the intermittent periodically duty motors. However, at steady-state operation, the low frequency could cause a great loss in energy because of the high value of balancing slip, and this should be avoided. The doted curves give the variations of critical slip versus frequency. It should be noted that as long as Scr > Ssym, the motor will be stable and the stability will depend on the difference between Scr and Ssym where the greater the difference the more stable the motor. Therefore, the steady-state region is defined when f > 0.2 fn.
The impedance characteristics of the balancing elements are also built by using a labVIEW software.
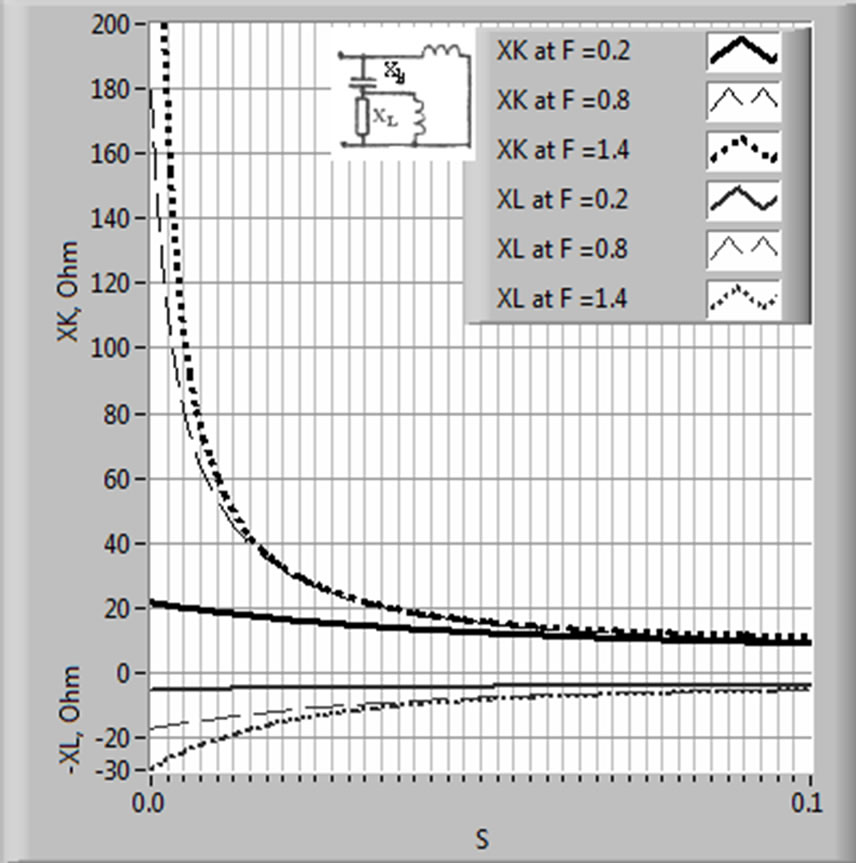
Figure 9 shows the relation between the balancing impedance and the slip at different frequencies for the above described motors with the attached connection diagrams:
The values of reactance are calculated by using equations (26) and (27) for Figures 9(a) and (b), respectively. Figure 9(a) shows that the inductive reactance XL is high at no-load condition, and decreases by increasing the load till it reaches a minimum value without crossing the X-axis (only inductive behavior. This is clear for high
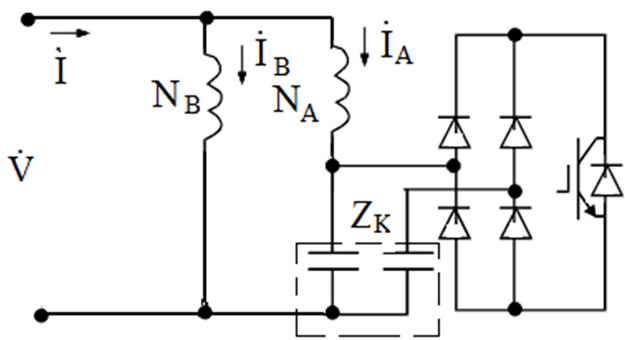
Figure 4. Single-phase induction motor with two windings connected in parallel and electronically controlled capacitor.
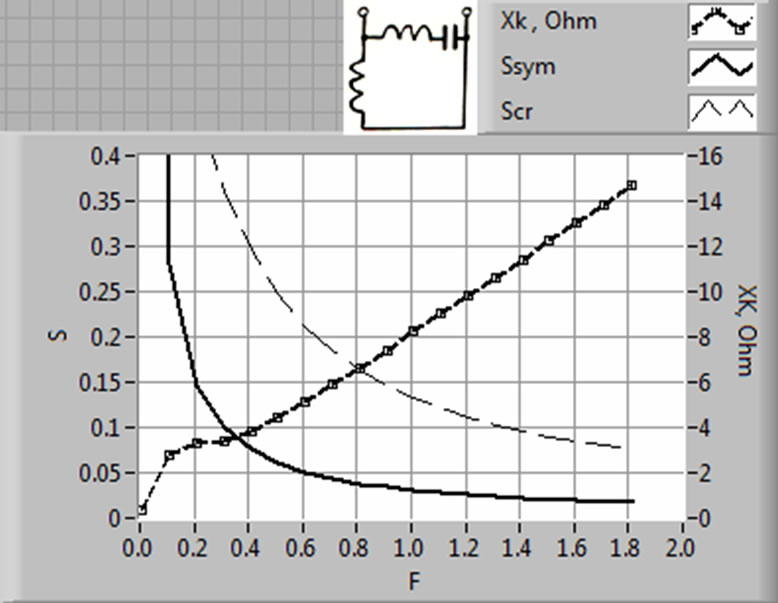
Figure 5. Balance of SPCRIM with two windings connected in parallel.
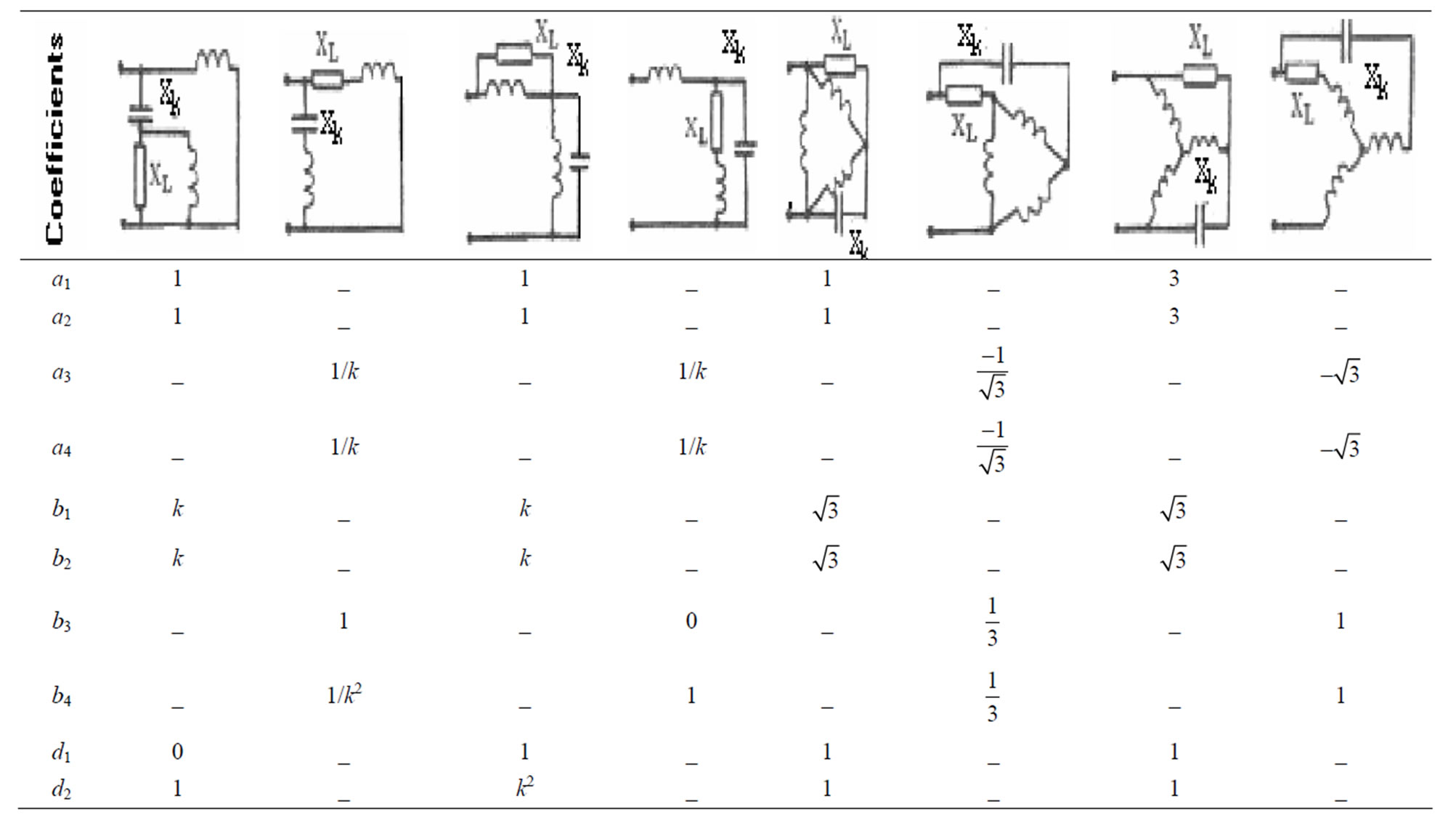
Table 2. Coefficients of balancing equations for common types of circuit diagrams.
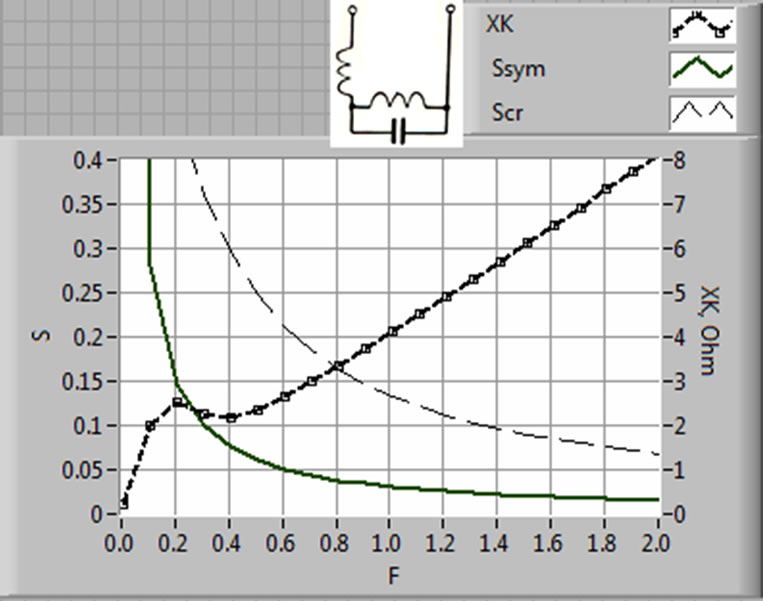
Figure 6. Balance of SPCRIM with two windings connected in series.
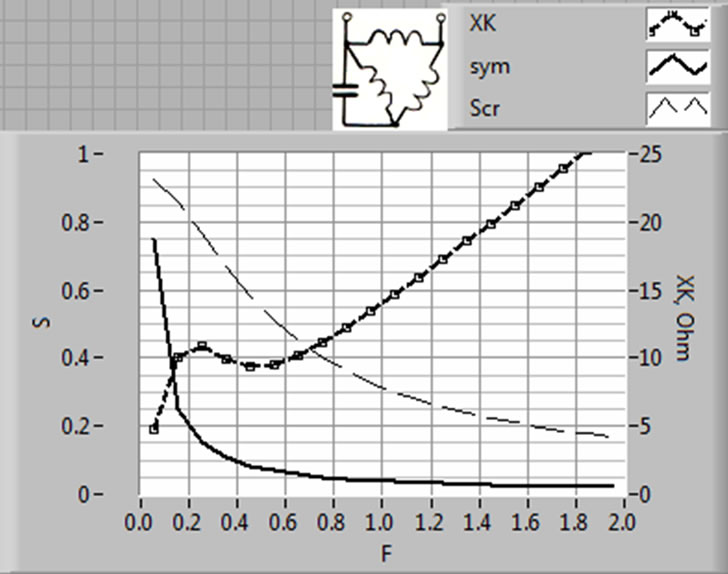
Figure 7. Balance of Δ—connected three-phase induction motor fed from single-phase supply.
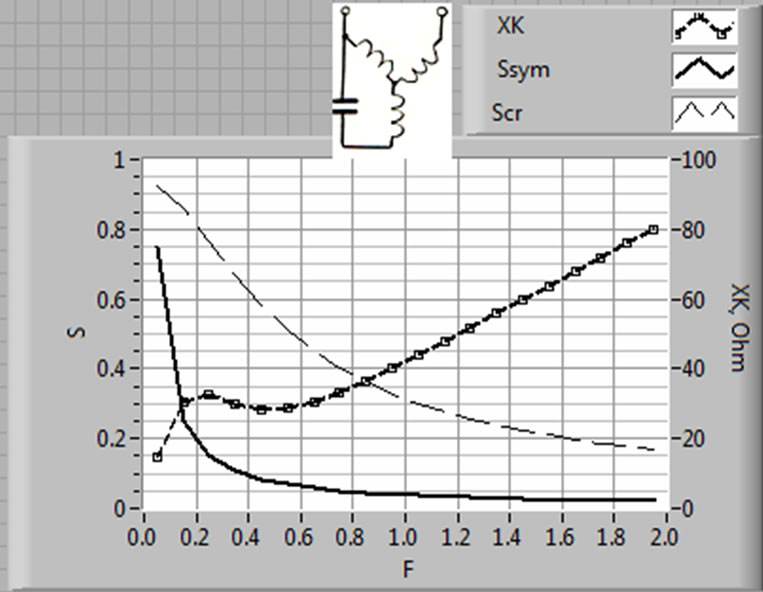
Figure 8. Balance of Ү—connected TPIM three-phase induction motor fed from single-phase supply.
frequencies of the supply voltages. The balancing capacitive reactance XK is high at no-load condition and decreases with increasing the load in the same way for all the frequencies of the supply voltages.
Figure 9(b) shows that both the balancing reactance XL and reactance XK have the same behavior. At first, they increase by increasing the load until they reach maximum values, then they begin to decrease again. Balancing inductive reactance XL will cross the X-axis (capacitive behavior) at the frequency f = 40 Hz (F = 0.8) and a high slip value. By increasing the supply voltage frequency, the crossing point of the XL with the X-axis will occur at lower values of the slip. It is clear that at high frequencies, the balanced operation will be achieved
 (a)
(a) (b)
(b)
Figure 9. Impedance characteristics; (a) auxiliary winding is shunting by inductive element, (b) Inductive element is inserted in series with the main winding.
by regulating the capacitance value only, in other words, both balancing elements should be capacitors.
The same inductive and capacitive behaviors are occurred for the least of connection diagrams, listed in the table 2, based on the group of equations (26) and (27).
The balancing capacitance characteristic was built using equation (28) also for the motor of power Pn = 2.8 kw as shown in Figure 10.
This figure shows that for constant frequency f = fn, the balancing capacitance value is proportional to the slip until specified value then the relation becomes nonlinear and the balancing capacitance almost hasn’t considerable change as slip increases over the critical slip. The bal-
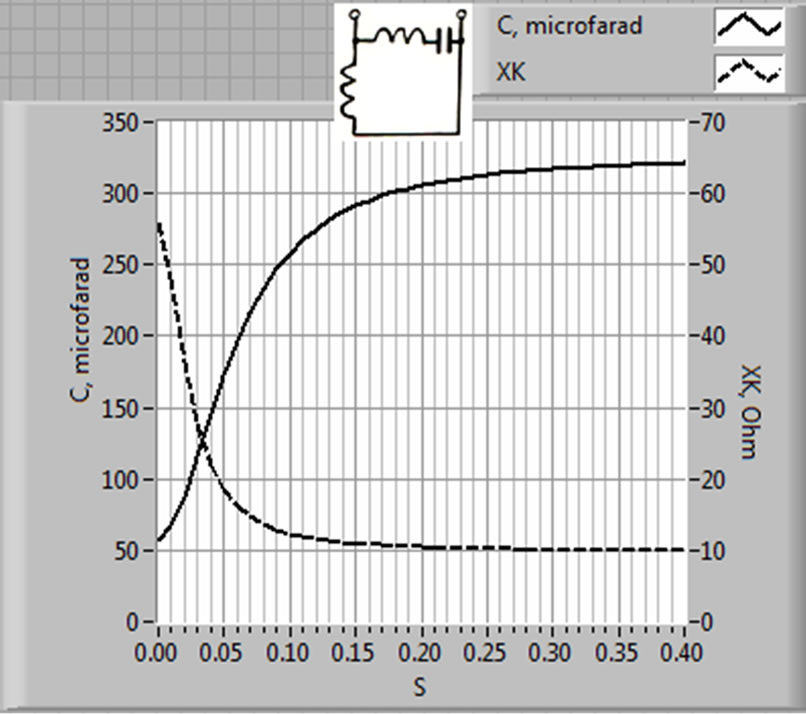
Figure 10. Balancing capacitance of single-phase capacitorrum induction motor versus slip.
ancing capacitance for the start condition is much larger than for the run condition. Although increasing the capacitance over the nominal value helps in balancing but it is accompanied by increasing the currents especially in the auxiliary winding .Therefore, this method is promising for the variations of load around the nominal value if the motor duty is continuous.
6. Conclusions
The study discusses the various methods to improve the performance of SPCRIMs and TPIMs operating from SPS. Single-phase induction motor is widely used in engineering practice and spends a lot of electricity each year. The promotion of induction motor’s efficiency has great significance for energy consumption, so the optimization design of single-phase induction motor is necessary. The mathematical model seems to be well.
The mathematical model is used to balance the motor operation by varying the frequency of supply voltage and investigating the characteristics of the balancing parameters. The proper selection of reactive element will improve the single-phase induction motor performance to compete the three-phase motor.
The advantages of the balancing method by changing the frequency are:
1) Wide range of speed control.
2) Soft speed control and improvement of starting characteristics.
3) This method can be used for various power rating motors with any stator circuit connection.
The disadvantages of this method are:
4) Steady state motor operation at low frequencies (F < 0.2) is forbidden due to the small maximum to the nominal torque ratio.
5) Expressions of balancing frequency, slip and capacitive reactance are awkward and have high orders.
Balancing by varying capacitance value of the condenser at constant frequency is the most economic especially if it is realized by electronic way but this method is not fair as the slip goes far from the nominal value.
For having robust balancing, in addition to the shifting phase capacitor, reactive element should be inserted into the stator circuit. This method will reduce the heat generated in the motor during the steady state operation mode for the full range of speed control. Thus, the benefits of this method include improved power factor, energy saving and elimination of the need for additional winding taps for speed variation.
According to the generalized calculation equations for balancing element impedance, the connection balancing diagrams could be grouped into two groups. For the first group of connection diagrams, the behavior of the balancing element is inductive over the whole slip regardless of the value of the voltage frequency. While for the second group of connection diagrams, the behavior of the balancing impedance XL will become capacitive depending on the load and the voltage frequency.
7. Acknowledgements
This work has been carried out during sabbatical leave granted to the author Mahdi Alshamasin from Al-Balqa’ Applied University (BAU)-Jordan during the academic year 2012/2013. I’d like to thank Al-Balqa’ Applied University for their support and Najran University-KSA for their logistic aid.
REFERENCES
- M. Chomat, “Adjustable-Speed Single-Phase IM Drive with Reduced Numbers of Switches,” IEEE Transactions on Industry Application, Vol. 39, No. 3, 2003, pp. 819- 826. http://dx.doi.org/10.1109/TIA.2003.811778
- K. Sundareswaran, N. Rajasekar and V. Sreedevi, “Performance Comparison of Capacitor-Run Induction Motors Supplied from AC Voltage Regulator and SPWM AC Chopper,” IEEE Transactions on Industrial Electronics, Vol. 53, No. 3, 2006, pp. 990-993. http://dx.doi.org/10.1109/TIE.2006.874256
- E. Muljadi, Y. Zhao, T. Liu and A. T. Lipo, “Adjustable ac Capacitor for a Single-Phase Induction Motor,” IEEE Transactions on Industry Applications, Vol. 29, No 3, 1993, pp. 479-485. http://dx.doi.org/10.1109/28.222415
- M. Alshamasin, “Control of Zero-Sequence Braking for a Three-Phase Induction Motor Operating from SinglePhase Supply with a Controlled Capacitor,” Journal of Applied Sciences, Vol. 12, No 24, 2012, pp. 2616-2620. http://dx.doi.org/10.3923/jas.2012.2616.2620
- M. S. Alshamasin, “Balancing the Operation of Stator Phases of the Capacitor-Run Single-Phase Induction Motors at Variable Frequencies,” Journal of Engineering, Vol. 14, No. 3, 2004, pp.173-180.
- S. K. Chowdhury, S. P. Chowdhury and K. Pal, “An Interactive Software for the Analysis of Thermal Characteristics of Capacitor-Run Single-Phase Induction Motors,” Electric Power Components and Systems, Vol. 29, No. 11, 2001, pp. 997-1011. http://dx.doi.org/10.1080/153250001753239211
- M. Alshamasin, “Optimization of the Performance of Single-Phase Capacitor-Run Motor,” American Journal of Applied Sciences, Vol. 6, No. 4, 2009, pp. 745-751. http://dx.doi.org/10.3844/ajassp.2009.745.751
- G. Singh, “A Research Survey of Induction Motor Operation with Non-Sinusoidal Supply Wave Forms,” Electric Power Systems Research, Vol. 75, No. 2-3, 2005, pp. 200-213. http://dx.doi.org/10.1016/j.epsr.2005.04.001
- T. A. Letenmaier, D. W. Novotny and T. A. Lipo, “Single-Phase Induction Motor with an Electronically Controlled Capacitor,” IEEE Transactions on Industry Applications, Vol. 27, No. 1, 1991, pp. 38-43. http://dx.doi.org/10.1109/28.67530
- N. M. Ocmanhodjaiev, M. S. Alshamasin and N. Laieb, “About Equilibrium of Stator-Load Currents of SinglePhase Capacitor Asynchronous Motors,” Problems of Informatics and Energy, No. 4, 1997, pp. 18-20.
- M. B. Senan, I. Aris and S. H. Hamad, “Development of Single Phase Induction Motor Adjustable Speed Control Using M68C11E-9 Microcontroller,” Journal of applied sciences, Vol. 5, No. 2, pp. 259-252.
- L. H. Liu, M. T. Lin and H. C. Wu, “A Single Phase Induction Motor Drive with Improved Performance,” Electric Power System Research, Vol. 47, No. 1, 1998, pp. 29-38. http://dx.doi.org/10.1016/S0378-7796(98)00036-4
- M. A. Abelhaleem, “Control of Single Phase Induction Motor Using Forced-Commutated Electronic Switches and Free-Wheeling Paths,” Electric Machines and Power Systems Journal, Vol. 27, No. 11, 1999, pp. 1201-1214. http://dx.doi.org/10.1080/073135699268669
- G. S. Ilango, K. Samidurai, M. Roykumar and K. Thanushkodi, “Energy Efficient Power Electronic Controller for a Cpacitor-Run Single-Phase Induction Motor,” Energy Conversion and Management, Vol. 50, No. 9, 2009, pp. 2152-2157. http://dx.doi.org/10.1016/j.enconman.2009.04.024
- S. Williamson and A. C. Smith, “A Unified Approach to the Analysis of Single-Phase Induction Motors,” IEEE Transactions on Industry Applications, Vol. 35, No. 4, 1999, pp. 837-843.
- S. D. Umans, “Steady-State, Lumped-Parameter Model for Capacitor-Run Single-Phase Induction Motors,” IEEE Transactions on Industry Applications, Vol. 32, No. 1, 1996, pp. 169-179. http://dx.doi.org/10.1109/28.485829
- M. Popescu, T. J. Miller, M. McGilp, G. Strappazzon, N. Traivillin and R. Santarossa, “Line-Start PermanentMagnet Motor: Single-Phase Starting Performance Analysis,” IEEE Transactions on Industry Applications, Vol. 39, No. 4, 2003, pp. 1021-1130. http://dx.doi.org/10.1109/TIA.2003.813745
- N. M. Osmanhodjaef, “Speed Control Methods of Capacitor-Run Single-Phase Induction Motors,” Energy, Moscow, 1980.
- M. Popescu, T. J. miller, M. McGilp, G. Strappazzon, N. Traivillin and R. Santarossa, “Asynchronous Performance Analysis of a Single-Phase Capacitor-Start, Capacitor-Run Permanent Magnet Motor,” IEEE Transactions on Energy Conversion, Vol. 20, No. 1, 2005, pp. 142-150. http://dx.doi.org/10.1109/TEC.2004.837307
- N. Ahmed, “Three-Phase Inductiom Motor Operating from Single-Phase Supply with an Electronically Controlled Capacitor,” Electric Power Systems Research, Vol. 73, No. 2, 2005, pp. 121-128. http://dx.doi.org/10.1016/j.epsr.2004.06.007
- E. S. Obe, T. Senjyu, M. U. Agu, L. U. Anih and O. Ojo, “Analysis of the Steady-State Components in a Balanced Single-Phase Synchronous Reluctance Motor with a Running Capacitor,” Electric Power Systems Research, Vol. 77, No. 3-4, 2007, pp. 295-302. http://dx.doi.org/10.1016/j.epsr.2006.03.004
- G. K. Dubey, “Fundamental of Electric Drives,” 2nd Edition, Narosa Publishing House, New Delhi, 2002.
- J. Faez, F. Kesebi and P. Pillay, “Design and Testing of an Integrated Electronically Controlled Capacitor for Integral and Fractional Horse Power Single Phase Induction Motor,” Energy Conversion and Management, Vol. 45, No. 18-19, 2004, pp. 2989-3001. http://dx.doi.org/10.1016/j.enconman.2004.01.008
- M. S. Alshamasin, “Improving the Performance of a Capacitor-Run Single-Phase Motor by Using a labVIEW Program,” Journal of Institute of Mathematics & Computer Sciences, Vol. 16, No. 1, 2005, pp. 137-148.
Nomenclature
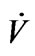 : supply voltage.
: supply voltage.
Rs, Xs: resistance and leakage reactance of the stator winding.
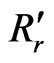 ,
,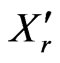 : resistance and leakage reactance of the rotor referred to the stator.
: resistance and leakage reactance of the rotor referred to the stator.
Rm, Xm: magnetizing resistance and magnetizing reactance.
XK: reactance of the capacitive element.
Nm, NA: turns numbers of the main and the auxiliary windings.
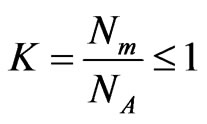 : turns ratio
: turns ratio
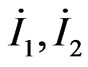 : forward, backward sequence currents.
: forward, backward sequence currents.
R1, X1: resistance and reactance of the forward sequence.
R2, X2: resistance and reactance of the backward sequence.
S: the slip of the motor.
F,f: per-unit and stator frequency of the motor.
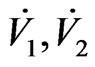 : forward, backward sequence voltage.
: forward, backward sequence voltage.

