Advances in Pure Mathematics
Vol.4 No.4(2014), Article ID:44632,5 pages DOI:10.4236/apm.2014.44016
Some Mappings on Operator Spaces
Guimei An
Department of Mathematics and LPMC, Nankai University, Tianjin, China
Email: angm@nankai.edu.cn
Copyright © 2014 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 7 February 2014; revised 7 March 2014; accepted 15 March 2014
ABSTRACT
We discuss two types of maps on operator spaces. Firstly, through example we show that there is an isometry on unit sphere of an operator space cannot be extended to be a complete isometry on the whole operator space. Secondly, we give a new characterization for complete isometry by the concept of approximate isometry.
Keywords:Unit Sphere, Approximate Isometry, Complete Isometry

1. Introduction
The theory of operator spaces is very recent. It was developed after Ruan’s thesis (1988) by Effros and Ruan and Blecher and Paulsen and Pisier.
Theorem (Ruan) in [1] suppose that 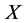 is a vector space, and that for each
is a vector space, and that for each 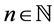 we are given a norm
we are given a norm 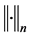 on
on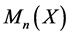 . Then
. Then 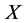 is completely isometrically linearly isomorphic to a subspace of
is completely isometrically linearly isomorphic to a subspace of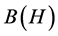 , for some Hilbert space
, for some Hilbert space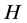 , if and only if conditions (R1) and (R2) above hold.
, if and only if conditions (R1) and (R2) above hold.
R1: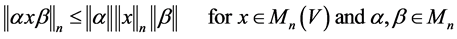 R2:
R2: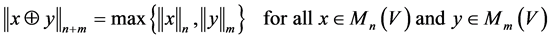 .
.
In this new category, the objects remain Banach spaces but the morphisms become the completely bounded maps. In fact, the completely bounded maps appeared in the early 1980s following Stinespring’s pioneering work (1955) and Arveson’s fundamental results (1969) on completely positive maps see [1] -[4] .
Definition 1 Let 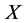 and
and 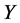 be operator spaces and that
be operator spaces and that 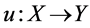 be a linear map. For a positive integer
be a linear map. For a positive integer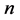 , we write
, we write 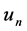 for the associated map
for the associated map 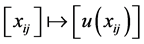 from
from 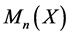 to
to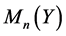 . This is often called the (nth) amplification of
. This is often called the (nth) amplification of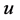 , and may also be thought of as the map
, and may also be thought of as the map 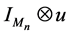 on
on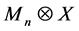 . A map
. A map 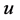 is completely bounded (in short c.b.) if
is completely bounded (in short c.b.) if
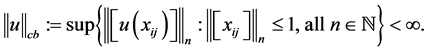
We denote by 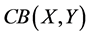 the Banach space of all c.b. maps from
the Banach space of all c.b. maps from  into
into 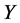 equipped with the c.b. norm. If
equipped with the c.b. norm. If 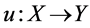 is c.b. map, we have
is c.b. map, we have
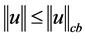
and
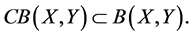
The notion of isomorphism is replaced by that of “complete isomorphism”. If 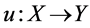 is a completely bounded linear bijection, and if its inverse is completely bounded too, then we say that
is a completely bounded linear bijection, and if its inverse is completely bounded too, then we say that 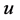 is complete isomorphism. In this case, we say that
is complete isomorphism. In this case, we say that 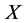 and
and 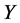 are completely isomorphic and we write “
are completely isomorphic and we write “ as operator spaces”. Similarly, if
as operator spaces”. Similarly, if 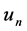 is an isometry for any
is an isometry for any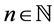 , then we say that
, then we say that  is complete isometry. We often identify two operator spaces
is complete isometry. We often identify two operator spaces 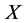 and
and 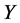 if they are completely isometrically isomorphic. In this case we often write “
if they are completely isometrically isomorphic. In this case we often write “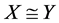 as operator spaces”.
as operator spaces”.
In 1932 [5] , Mazur and Ulam got the famous theorem that a surjective isometry between two real normed spaces must be linear up to translation, which started the study of the theory of isometry. Many researchers have tried to generalize it. In 1972 Mankiewicz [6] considered the extension problem for isometries and every surjective isometry between the open connected subsets of two normed spaces can be extended to a surjective affine isometry on the whole space. This result can be seen as local Mazur-Ulam Theorem. From this result, we get easily that a map’s property on unit ball of a normed space determines the relationship between distance preserving and linearity. In 1987, D.Tingley raised the following problem (the isometric extension problem) in [7] :
Problem. Suppose that 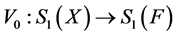 is a surjective isometric mapping, does there exist a linear isometric mapping
is a surjective isometric mapping, does there exist a linear isometric mapping  such that
such that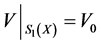 ?
?
D. Tingley, who is the first one to study the problem, gave some results in [7] under the condition that 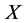 and
and  are finite dimensional spaces. More precisely, the answer can be formulated as follows: Suppose that
are finite dimensional spaces. More precisely, the answer can be formulated as follows: Suppose that 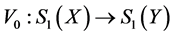 is a surjective isometric mapping, then
is a surjective isometric mapping, then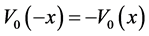 , i.e.
, i.e.  is an odd mapping. In the complex spaces, the answer to Tingleys problem is negative. For example, we take
is an odd mapping. In the complex spaces, the answer to Tingleys problem is negative. For example, we take 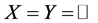 (complex plane) and
(complex plane) and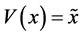 . Some affirmative results have been obtained between classical real Banach spaces, which had been shown in [8] -[12] . The recent development on this problem you can find in [13] . We ask can we discuss the isometric extension problem in operator space category? Through example, we give some results on it.
. Some affirmative results have been obtained between classical real Banach spaces, which had been shown in [8] -[12] . The recent development on this problem you can find in [13] . We ask can we discuss the isometric extension problem in operator space category? Through example, we give some results on it.
2. Some Examples about Isometric Extension Problem on Operator Spaces
Theorem Let 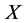 be
be 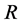 (resp.
(resp.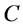 ,
, 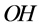 ,
, 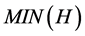 ,
, 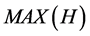 ,
, 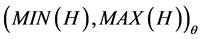 , etc.). Let
, etc.). Let 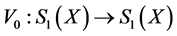 be a
be a 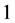 -Lip operator. Then
-Lip operator. Then 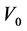 can be extended to a linearly complete isometry on
can be extended to a linearly complete isometry on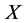 .
.
Proof. By [11] , 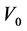 can be linearly extended to the whole Hilbert space. Since
can be linearly extended to the whole Hilbert space. Since  are homogeneous operator space, the the result can be got directly.
are homogeneous operator space, the the result can be got directly.
How about the result on non-homogeneous operator spaces?
Theorem There exists an isometry 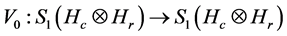 such that there is no complete isometry
such that there is no complete isometry 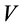 on the whole space
on the whole space  satisfying
satisfying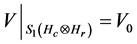 .
.
Proof. Let 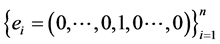 be the basis of
be the basis of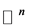 . To express conveniently, we denote
. To express conveniently, we denote 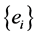
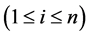 to be the basis of the n-dimensional column Hilbert operator space
to be the basis of the n-dimensional column Hilbert operator space 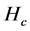 and
and 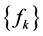
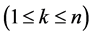 be the basis of the n-dimensional row Hilbert operator space
be the basis of the n-dimensional row Hilbert operator space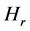 . Then
. Then 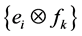 constructs a basis of
constructs a basis of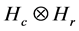 . Give the
. Give the 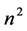 elements an order defined as
elements an order defined as
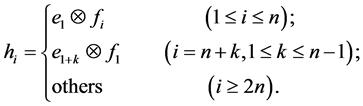
Let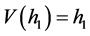 ,
, 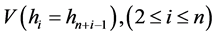 and
and 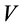 maps other elements
maps other elements 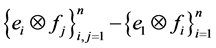 of the basis to
of the basis to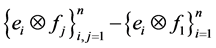 . So
. So 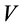 forms a permutation among the basis of
forms a permutation among the basis of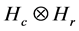 . By the isometric theory on Hilbert space,
. By the isometric theory on Hilbert space, 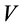 is an isometry on the Banach space
is an isometry on the Banach space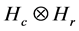 . Of course let
. Of course let 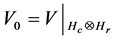 is an isometry between unit sphere of
is an isometry between unit sphere of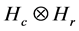 . But
. But 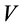 is not complete isometry on
is not complete isometry on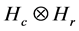 .
.
Indeed, for 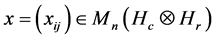 where
where
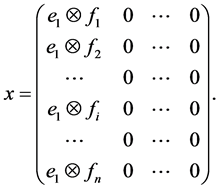
Then
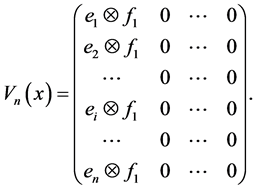
It is easy to calculate that 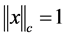 but
but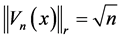 . So
. So 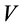 is not complete isometry.
is not complete isometry.
3. On Stability of (-Isometries of Operator Spaces
The study on the stability of ò-isometry is from Banach Space Theory.
Let  be two Banach spaces and
be two Banach spaces and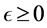 . A mapping
. A mapping 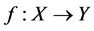 is said to be an ò-isometry provided
is said to be an ò-isometry provided
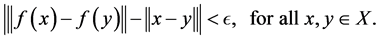
In 1945, Hyers and Ulam proposed the following question: whether for every surjective ò-isometry 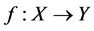 with
with 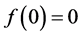 there exist a surjective linear isometry
there exist a surjective linear isometry 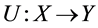 and
and  such that
such that 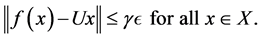 After many efforts of a number of mathematicians (see, for instance [14] -[19] ) Omladi
After many efforts of a number of mathematicians (see, for instance [14] -[19] ) Omladi 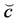 and
and 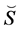 emrl gave the sharp estimate that
emrl gave the sharp estimate that 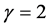 in 1995 (see [20] ).
in 1995 (see [20] ).
In this section, we ask whether we can discuss the the stability of ò-isometry in operator space category? We try to propose a question and give a little answer.
Firstly, we get the following result.
Theorem Let 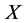 and
and 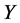 be operator spaces. If a mapping
be operator spaces. If a mapping 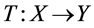 satisfies that for any
satisfies that for any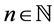 ,
,
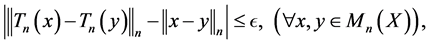
then 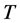 is an isometry.
is an isometry.
Proof. For any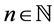 ,
, 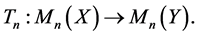 Let
Let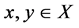 . Specially, we put
. Specially, we put
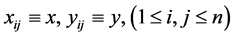 , then
, then 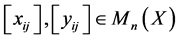 and we have
and we have
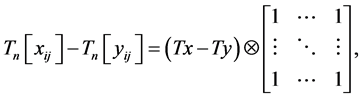
Since
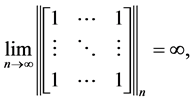
we get 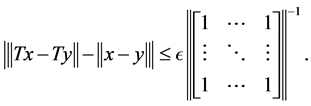
So 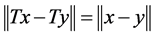 holds for any
holds for any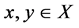 .
. 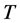 is an isometry.
is an isometry.
Acknowledgements
A part of this work was completed when I visited the University of Illinois, Urbana. I would like to thank Professor Zhong-Jin Ruan for the invitation and great help.
The author also expresses his appreciation to Dr. Liu Rui for many very helpful comments regarding the stability of ò-isometry in operator space category.
Funding
This work is supported by the NSFC grant 11101220, 11271199 and the Fundamental Research Funds for the Central Universities.
References
- Effros, E.G. and Ruan, Z-J. (2000) Operator Spaces. In: London Mathematical Society Monographs New Series 23, The Clarendon Press, Oxford University Press, New York.
- An, G., Lee, J.-J. and Ruan, Z.-J. (2010) On p-Approximation Properties for p-Operator Spaces. Journal of Functional Analysis, 259, 933-974. http://dx.doi.org/10.1016/j.jfa.2010.04.007
- Pisier, G. (1990) Completely Bounded Maps between Sets of Banach Space Operators. Indiana University Mathematics Journal, 39, 249-277. http://dx.doi.org/10.1512/iumj.1990.39.39014
- Pisier, G. (2003) An Introduction to the Theory of Operator Spaces. In: London Mathematical Society Lecture Note Series 294, Cambridge University Press, Cambridge.
- Mazur, S. and Ulam, S. (1932) Sur less transformations isométriques d’espaces vectoriels normés. Comptes Rendus de l’Académie des Sciences de Paris, 194, 946-948.
- Mankiewicz, P. (1972) On Extension of Isometries in Normed Linear Spaces. Bulletin de l Académie Polonaise des Sciences, Série des Sciences Mathématiques, Astronomiques, et Physiques, 20, 367-371.
- Tingley, D. (1987) Isometries of the Unit Sphere. Geometriae Dedicata, 22, 371-378. http://dx.doi.org/10.1007/BF00147942
- Ding, G.G. (2003) The Isometric Extension Problem in the Unit Spheres of
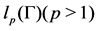 Type Spaces. Science in China, Series A, 46, 333-338.
Type Spaces. Science in China, Series A, 46, 333-338. - Ding, G.G. (2004) The Representation Theorem of onto Isometric Mappings between Two Unit Spheres of
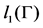 Type Spaces and the Application to Isometric Extension Problem. Acta Mathematica Sinica, English Series, 20, 1089- 1094. http://dx.doi.org/10.1007/s10114-004-0447-7
Type Spaces and the Application to Isometric Extension Problem. Acta Mathematica Sinica, English Series, 20, 1089- 1094. http://dx.doi.org/10.1007/s10114-004-0447-7 - Ding, G.G. (2004) The Representation of onto Isometric Mappings between Two Spheres of
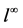 -Type Spaces and the Application on Isometric Extension Problem. Science in China Series A, 47, 722-729. http://dx.doi.org/10.1360/03ys0049
-Type Spaces and the Application on Isometric Extension Problem. Science in China Series A, 47, 722-729. http://dx.doi.org/10.1360/03ys0049 - Ding, G.G. (2002) The 1-Lipschitz Mapping between the Unit Spheres of Two Hilbert Spaces Can Be Extended to a Real Linear Isometry of the Whole Space. Science in China Series A, 45, 479-483. http://dx.doi.org/10.1007/BF02872336
- Fang, X.N. and Wang, J.H. (2006) Extension of Isometries between the Unit Spheres of Normed Space E and C(
 ), Acta Mathematica Sinica, English Series, 22, 1819-1824. http://dx.doi.org/10.1007/s10114-005-0725-z
), Acta Mathematica Sinica, English Series, 22, 1819-1824. http://dx.doi.org/10.1007/s10114-005-0725-z - Ding, G.G. (2009) On Isometric Extension Problem between Two Unit Spheres. Science in China Series A, 52, 2069- 2083. http://dx.doi.org/10.1007/s11425-009-0156-x
- Bourgin, D.G. (1946) Approximate Isometries. Bulletin of the American Mathematical Society, 52, 704-714. http://dx.doi.org/10.1090/S0002-9904-1946-08638-3
- Bourgin, D.G. (1975) Approximate on Finite Dimensional Banach Spaces. Transactions of the American Mathematical Society, 207, 309-328.
- Figiel, T. (1968) On Non Linear Isometric Embedding of Normed Linear Spaces. Bulletin de l Académie Polonaise des Sciences, Série des Sciences Mathématiques, Astronomiques, et Physiques, 16, 185-188.
- Gruber, P.M. (1978) Stability of Isometries. Transactions of the American Mathematical Society, 245, 263-277. http://dx.doi.org/10.1090/S0002-9947-1978-0511409-2
- Gevirtz, J. (1983) Stability of Isometries on Banach Spaces. Proceedings of the American Mathematical Society, 89, 633-636. http://dx.doi.org/10.1090/S0002-9939-1983-0718987-6
- Ulam, S.M. and Hyers, D.H. (1945) On Approximate Isometries. Bulletin of the American Mathematical Society, 51, 288-292.
- Omladic, M. and Semrl, P. (1995) On Non Linear Perturbations of Isometries. Mathematische Annalen, 303, 617-628. http://dx.doi.org/10.1007/BF01461008

