ABSTRACT

The aspiration to clarify and visualize Einstein’s interpretation of the notion of simultaneity and relativistic understanding of length and time interval measurements led to the development of a model in which light pulses were replaced by sound signals. The model revealed the essence of Einstein’s mathematical constructs and the mechanism for origination of relativistic effects. Moreover, its consistent application produced novel results in particular, constructing a relativity theory based on derivation of its fundamentals out of a thought experiment. Using this approach, the ex- perimentally observed independence of the speed of light from the motion of source and observer was a necessary consequence of the finiteness of propagation speed for all kinds of information. The paper provides a rationale for the logical framework formulated in article [1] , and describes the experimental device built to validate the predictions of the theory and the results obtained.

Keywords:

Principle of Relativity, Measurement Process, Lorentz Group

1. Introduction

When studying the basics of the theory of relativity, one might encounter a psychological roadblock: the theory comes into conflict with the so-called common sense.

The desire to make evident the very basis of relativistic ideas―Einstein’s interpretation of the notion of simultaneity―led to a thought experiment in which light pulses were substituted with sound signals. This model was chosen for didactic reasons, however, its consistent application produced fundamentally novel results.

In the proposed thought experiment, the clocks are synchronized by sound signals; the information on current events from one observer to another is transferred by sound only. This scheme, conceptually, should give a result similar to that which was obtained by Einstein, and the only difference being that in all formulas of the speed of light will be substituted with the speed of sound. However, this path brings us to a paradox; its resolution drives the development of a logical framework which not only clarifies Einstein’s mathematical constructs but also exposes the mechanism for origination of relativistic effects.

The use of thought experiments that measure the segment lengths and time intervals in inertial reference systems moving relative to each other allows us to construct a logically complete system. In this context the postulates of the theory of relativity are derived not as absolute mathematical principles but as experimental results (produced with currently achievable accuracy). Another consequence is the possibility for experimental verification of the theory’s predictions: the last section of this paper is dedicated to the description of an experimental apparatus built to validate such predictions, including the results of the experiments.

2. Mechanics of Moving Bodies

In constructing a scheme of clock synchronization and measuring lengths of segments with the help of sound signals in reference frames, which uniformly move relative to still air, there emerges an apparent paradox: as it is known, in Lorentz transformations, verifiable by experiment, the speed of light is included, although it is not present “instrumentally” in the scheme of measuring segment lengths and time terms: for this purpose the sound is used, but not light. Let us consider this idea in more detail.

The reference frame, wherein the air rests, will be called “Air preferred” (AP) reference frame. In accordance with the idea, which seems to be self-evident, in this reference frame the clock that are synchronous “by sound” (i.e., such clock to synchronize which, the sound signals are used), will be synchronous “by light” too. It is, however, a mistake. “Light preferred” (LP) reference frame should not a priori coincide with AP, just their difference becomes noticeable only at the relativistic level of speeds, and it is very small when moving with subsonic speeds.

Reasoning system leading to the paradox, constructed on the misconception about the notorious coincidence of AP and LP, is as follows. If in the middle of segment, located in AP, the light signals from events on its ends come simultaneously, the sound signals come simultaneously too (just sound signals later). In order to measure the length of a moving segment, we fix its beginning and end so as sound signals (and light) from fixation events would come in the middle of the segment simultaneously. At that, the expe-

rience shows, that to reduce the length of a moving segment, formula 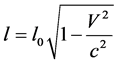is

true, where c is the speed of expressly light, but not of the sound. The moving reference frame itself does not take any part in the length measurement, the rate of its clock along with that where with exactly they were synchronized, does not play any part. It turns out that a kind of magic power as though distinguishes the speed of light, although the sound is “nothing the worse” in this situation. Moreover, the light from this measurement process can be eliminated at all, however reduction in the length, as an experience shows, will be “light”, but not “sound”. One gets the feeling of objective independence of the measurement process from those main “instrument” with the help of which this measurement is carried out―light signals. The speed of light appears in the final result, as though miraculously. However, there is no such thing as miracles―it is necessary to look for a mistake in reasoning.

Let us show the following: although AP and LP reference frames do not coincide, the difference between them cannot be detected with the help of sound signals and within the limits of the “sound accuracy”. For this purpose let us abstract from known constructs and consider the following scheme. Let there are two reference frames moving uniformly relative to AP with the speeds which are high in comparison with the speed of their movement relative to each other. Let us show that when measuring speeds of each other, observers in these reference frames obtain very close results. The same is true for results which will obtain each of these observers, measuring the speed of sound. Besides, since the clocks in each of these reference frames are synchronized with the help of sound signals, the speed of sound for each of observers within his frame will be the same in all directions. All this will allow described observers conclude that, firstly, the speed of sound in general is the same in all uniformly moving reference frames, and secondly, the measurement of the speed of one reference frame made from another, will give exactly the same result as the back measurement. Having added to the scheme the postulate of homogeneity and isotropy of the space, we obtain a sufficient basis for construction of all kinematics of Lorentz transformations (What will be done below).

In reality, at a sufficiently high measurement accuracy there is an asymmetry in both measurement of relative movement velocity of considered reference frames and measurement of the speed of sound, carried out in each of them. An observer in a frame moving relative to the air, measuring the speed of AP frame, will obtain less result than an observer in AP, who measures the speed of the first frame. When measuring the length of a moving object from AP itself, there will be no “length shortening”, as there will be no “time dilation” too.

With ideal measurement accuracy in frames, moving uniformly relative to AP and using the sound for clock synchronization it is possible, moving in the direction of that of the frames, wherein the speed relative to AP is less, to go out finally to the frame, which rests relative to the air―that is the AP itself.

Let us show that everything gets on in precisely this way.

“Absolute” (Abs) will be called an observer who is located in AP reference frame, which is motionless relative to the air, and uses light signals in order to receive the information. At that we assume that speeds of reference frames under study relatively to AP frame are small to an extent that “light” relativistic effects can be neglected. Each of two observers under our consideration rest in one of moving reference frames and uses only sound signals, which propagate in the still air in all directions with one and the same speed, equal to e.

From Abs viewpoint the picture is: at rest lengths of two segments are equal. Since Abs receives signals almost without delay (light delay is neglected), then with respect to him these segments will have equal lengths in the motion too. Segments move relative to the initial frame with speeds of V₁ and V₂, the observer is located in the middle of each segment (see Figure 1).

E frame “overtakes” F frame, at that the contact of A and A` points and B and B` points from Abs viewpoint occurs simultaneously. Abs observer sees: signal from B and B` point contact came to F observer earlier than the signal from A and A` contact for

.

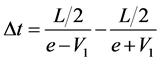This means that from the viewpoint of F observer (who uses the “sound” concept definition of simultaneity of distinct located events and, accordingly, synchronizes the clock with sound signals) A`B` segment in E frame is longer than AB segment in F frame.

Let us construct in F reference frame such ab segment with the centre at F point, that, from the F viewpoint, it was equal to A`B` segment in E frame: the sound signal flew out

of b point later, than from B point for the time of 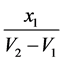and moved to the left relative to F observer with the speed. The signal from a point flew out earlier, than from A for the time and moved to the right with the speed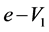. These sig-

nals came to the centre of ab segment―to F observer―at the same time, wherefrom he concludes, within the accepted axiomatic, that his ab segment is equal in the length to A`B` segment in the moving reference frame.

Let us find the length of ab segment, equal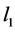. The signal from b point flew out later

than from a point for a time.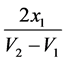

And, eventually:

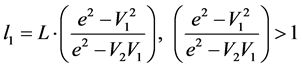(1)

Thus, when “sound” observation from F reference frame (“lagging”), takes place an increase in the length of the moving body along a line parallel to the speed of movement in comparison to its length at rest.

Similar reasoning for E observer. From Abs viewpoint: sound signal from B and B` point’ contact came to E point earlier than the signal from A and A` contact for

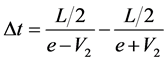. That is, from E viewpoint, AB segment in F frame is shorter than

A`B` segment in E frame. Let us construct in E frame a`b` segment with the centre at E point, the length of which, from E viewpoint, is equal to the length of AB segment in F

frame: the signal from b` flew out later, than from B`, for a time 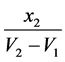and moved to the left relative to E observer with the speed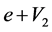. The signal from a` flew out earlier, than from A` for 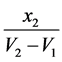and moved to the right with the speed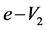. These signals came to E point simultaneously. Let us find the length of a`b` segment equal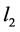. The signal from b` point flew out later, than from a` for a time.

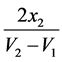Eventually:

(2)

In the observation from “overtaking” frame takes place a reduction in the segment length compared to its length at rest.

Let us view how the described picture looks by “eyes” of each E and F observers.

Picture from F observer viewpoint (see Figure 2):

From F viewpoint, lengths of ab and A`B` segments are equal―signals from their contact come to F point simultaneously. E observer flies to the right (towards b ‘signal

and moving away from A` signal), he sees the signal from B` earlier than from A`, therefore, in the “light simultaneity” logic from E viewpoint ab segment is shorter than A`B`.

Picture from E observer viewpoint (see Figure 3):

From E viewpoint, the lengths of a`b` and AB segments are equal: signals from their contact come to E point simultaneously. F observer flies towards the signal from A and moving away from the signal from B, he sees the signal from A earlier than from B, i.e. from F viewpoint a`b` segment is shorter than AB.

Let us calculate, what fold is one of the segments shorter than another in each of two considered cases.

From E viewpoint the length of ab segment (flying by with the speed―see Figure 3) is greater than the length of a`b` segment, located in E itself, in so much times, as many times the ab length is actually greater than AB length―at the rest. The length of a`b` at the rest is. “In actual fact” ? i.e. from the viewpoint of Abs observer, AB length is L.

We get:

So, from the viewpoint of E observer, the length of moving ab segment is less than the length of resting A`B` segment:;.

Similarly―“in the reverse direction”: from F viewpoint the length of a`b` segment (flying by with the speed, see Figure 2) is less than the length of ab segment, located in F itself, in so much times, as many times the a`b` length is actually less than A`B` length―at the rest.

We get:

As we see, the symmetry is observed in this case.

Combining two considered cases, we obtain the following. With respect to the transformation of moving segment length in comparison with its length at the rest there is the asymmetry (depending on whether the observer is in “overtaking” or “lagging” reference frame, the length either increases or decreases, at that it is possible to carry out

the direct experiment only at movement speeds, which are comparable with the speed of the synchronization object―in our case it is the sound―and with strict adherence to the principle of simultaneous fixation of the segment endpoints, what in practice is impossible); in contrast, in the case of comparing lengths of segments that are already in motion, with segments arranged in the laboratory reference frame, there is complete symmetry: if from the viewpoint of laboratory reference frame (“by sound”), some segment “B”, located in the moving frame, is equal to segment “A”, located in the laboratory reference frame, then from the viewpoint of the moving frame the segment “A” is shorter than the segment “B”, and the reduction factor is equal to

(3)

- this is true “in any direction”.

Quite similarly formulas are derived for relative speeds, time terms and speed of the sound. All these findings in detail the work are done in [1] .

For any kinematic measurements carried out in one of uniformly moving reference frames, if the speed of light, obtained in this reference frame, is used as a measurement unit (i.e., own reference standards of length and time), results will turn out to be the same, as in the other reference frame.

The derived formulas do not “prohibit” the existence of speed greater than speed of the sound. Just strictly following the selected procedure of clock synchronization and determination of the simultaneity of events, we find out that a moving body “cannot”, being accelerated, overcome the speed of sound, since, approaching it, it―from our point of view and according to our clock―moves with more and more lower acceleration, while from its own point of view (or rather, from the viewpoint of the instantaneously accompanying reference frame) its acceleration is constant. However, no physical phenomena (like, for instance, the growth of inertness with the increase of speed), is behind that, the whole thing is in the strict execution of the agreement on clock synchronization. One has only to substitute in this scheme the sound for the light, and the “sound barrier” will disappear, and the light barrier will take its place.

It is possible to speak in this context about the experimental verification of described regularities only in that case, if we are ready to consider such and only such physical phenomena, which by their very nature inherent in the information transmission rate, which coincides with the speed of our “sound”. In particular, it is the speed of light for electromagnetic phenomena.

The derived regularities are deprived of an attractive beauty, symmetry and simplicity, which have Einstein’s laws - measurements are linked to the selected reference frame, and therefore, the complete symmetry in this case is impossible. This is a fee for move away from the beauty of mathematical abstraction to physical regularities.

The aforesaid does not negate the need to show the manner in which the laws of nature very exactly match the mentioned abstraction―the system of axioms formulated by Einstein. Application of the obtained regularities allows accurate description of those phenomena, wherein the propagation of signals occurs precisely with the speed of light. It is also shown, that in the observed Universe not only electromagnetic, but also generally any information, that is directly or indirectly available for observation, will be transferred in a vacuum with one and the same speed.

So, let us abstract from the “absolute” Abs observer capable to obtain the “true” information with the help of light signals (which, when considering the “air sound universe” at actually available measurement accuracy of the universe have, in fact, the infinite speed). Let there is only the sound at the disposal of any observer―nothing faster does not exist. Clock synchronization is performed in such a world with sound signals. What are virtually observed “pictures” in this case?

With acceptable accuracy are measurable: the relative speed of bodies’ motion, the speed of sound, and the time term. Direct experimental comparison of “longitudinal” length of the physical body at rest with its length in motion would require unattainable measurement accuracy: at low speeds the difference in lengths itself is vanishingly small and at subsonic speeds time terms become immeasurably small, since the length of a real solid body, being accelerated to such speed, cannot be big enough. But the most difficult task is determining the speed of the laboratory reference frame relative to the still air.

Observers, operating in accepted conditions, when carrying out measurements of the sound speed and relative velocity of uniformly moving frames with available to them accuracy, will obtain, as it was shown, results carrying into the following axiomatic: indiscriminability of uniformly moving reference frames, i.e. the principle of relativity, applicable to any experiments, including experiments with sound; plus the principle of constancy of speed of the sound―it is obtained the same at measurements, regardless of the movement of a source or an observer (in short: “PR” and “CS”).

Within the framework of the proposed hypothetical axiomatic it is possible to lead the following theoretical reasoning.

Let us consider the following experiment:

The speed of sound from the viewpoint of E reference frame is e. By the E observer E, located in the centre of the motionless segment with the length L, at a speed u flies through the segment, ends of which come into contact with the ends of segment in E frame simultaneously (sound signals from contacts come to the segment centre at one and the same moment), i.e. from the viewpoint of E observer, the length of the moving segment is L too. All the data E observer received directly, without having any “external” information. Picture from the viewpoint of observer in E frame is (see Figure 4):

E observer reasons. F point moves to the left, i.e. towards the signal from the contact of left ends and moving away from the signal from the right. Signals, which came to E point simultaneously, will come to F point with mismatch in time. This mismatch, measured by E observer, is equal

(4)

From the fact that the signal from the left comes to F observer earlier, than the signal from the right (this fact is absolute), F observer himself, who also reasons in the framework of accepted axioms “PR and CS”, can make the only one conclusion: the segment in moving E frame is shorter than the segment in his frame, motionless. From the viewpoint of F reference frame, wherein the clock is synchronized by sound pulses, the picture looks like this (see Figure 5):

(To carry out all these reasoning, the real observer located in F frame is not needed - just enough is an objective fact of the time mismatch of signal’s coming in the middle of F frame, as to the rest E observer can reason theoretically).

Continuing to develop the logic on the basis of the hypothesis that all uniformly moving reference frames are equivalent, we must now find such transformations for segment lengths and time terms which in the framework of this axiomatic would consistently describe the existing experimental situation: the observer in one of frames sees that “resting” and “moving” segments are equal, and the observer in the other frame― that lengths of the same segments are different: the “moving” segment is shorter than the segment resting in his reference frame. Within such formulation, the problem has the unique solution: the segment which length at the rest is equal to L, as measured in the motion, has a smaller longitudinal length:

; (), where u―the speed of this segment movement, e―the speed of sound:

the length transformation function cannot depend on any other parameters. Our system of axioms include the regulation on uniformity and isotropy of the space (also experimentally confirmed), therefore the linear dependence is selected. Within the framework of “PR and CS” hypothesis, e and u values are identical, in whatever of two considered reference frames they would be measured.

Continuing the same logic, let us consider two events, which are single-placed in F reference frame. If between them, according to F clock, the time has passed then, according to E clock, between the same events has passed the larger time:

. (This is proved in the following way: events that are single-placed in F, leave on the segment, located in E frame, marks, the distance between which from F viewpoint is equal to. Since these marks limit the segment, motionless in E frame, then its length, measured by F observer, is less than that measured by E observer to fold. That is, its length in E frame is equal to. And since the movement speed of a point located in F frame, wherein these two events took place, measured by E, is equal u, therefore, the time term that this point spent to cover this segment is equal to.)

Applying this relation to the mismatch time between two events, single- placed in F reference frame, we get:

.

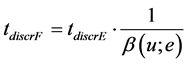
Further. From the viewpoint of F observer the segment in E frame is shorter than the segment in his frame for. At that, the length of E frame from the viewpoint of

F is equal to, i.e. the segment length in F frame from the viewpoint of F frame

itself is equal:

(5)

At the same time the segment length in F frame from the viewpoint of E frame is L, and the ratio of lengths “(the segment length in F from the viewpoint of F)/(the segment length in F from the viewpoint of E)”―that is. Substituting:

, wherefrom (6)

Thus, remaining within the framework of “PR and CS” hypothesis, we get Lorentz transformations for the longitudinal length of moving segment and time term which divides the events, single-placed in a moving reference frame.

As it was shown, in the reference frames, wherein the clock are synchronized by sound signals, another picture is virtually observed: for reference frame, moving relative to AP with the speed, there takes place the increase in the segment length, moving with the speed V₂, in comparison with its length at rest (formula (1)), in the inverse case there takes place the reduction of the length (formula (2)). However, a direct experimental verification of these relations at available measurement accuracy is impossible.

Further, the results of measurements of the relative movement speed of frames and the speed of sound do not contradict the hypothesis that all uniformly moving reference frames are equivalent, if these measurements are carried out in frames, the speed of which relative to AP is the order of one-thousandth of the speed of sound or less, and the measurement accuracy is not enough to notice the difference of the order of one-millionth. The very same speed of sound, as these experiments will show, is the maximum achievable speed for a material object, the observation over which is carried out with the help of sound signals and sensors, responsive to these signals. As for the material objects that are moving faster than sound (or accelerate from subsonic to supersonic speed right in the front of “eyes of the observer”), then their existence is theoretically not prohibited and if such objects in described “sound universe” would appear, then it would be possible to watch them there, but they would violate accepted in this world ideas about causal regularities.

The above considered experiment on comparison of lengths of moving and resting segments (contrary to comparing the length of one and the same segment in motion and at rest) gives completely symmetrical results at any measurement accuracy.

3. Experimental Procedure

Conclusions, to which lead the proposed logic in the field of electrodynamics, allow rather simple experimental verification. Such an experiment allows single-valued division of two theoretical approaches―axiomatic of SRT and our constructions, since results predicted by these theories are fundamentally different.

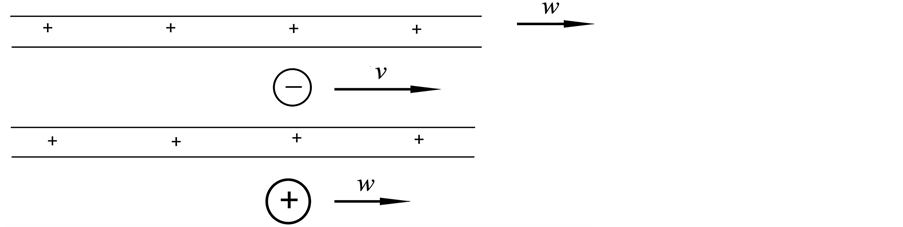
Let us consider the interaction of the current-carrying wire moving with the speed w relative to “LP” reference frame (“Light preferred” reference frame―the analogue of “Air preferred”), with the particle, that is motionless relative to the wire. At that, electrons in a wire move in the same direction relatively to “LP” with the speed. At the same time, “Abs” observer is located in “LP” frame (see Figure 6).

For the observer located in F frame (in designations of Figure 1), the length of the segment, located in E frame of drifting electrons as compared with the length of the same segment at rest will be increased in accordance with formula (1):

(7)

Accordingly, the charge density of moving electrons, measured by F observer, will decrease:

(8)

Here s―the charge density, measured at rest. Expression in the parentheses <1.

(Independence of the charge amount from the reference frame is the experimental fact).

The test charge is positive; moving electrons in the wire attract it, uncompensated ions of the conductor―push off with a force which is determined by the initial charge density:.

The force from ions is greater; the resultant force-repulsion: the wire with current at such arrangement of relative speeds should behave like the positive charged body. Summing up the effect of drifting electrons and ions, we obtain the specific charge of such “charged” filament―from the viewpoint of F observer:

(9)

The value represents a drift velocity of electrons in a conductor (measured by “Abs” observer), i.e. the repulsive force of the resting positive charge from the wire with a current is directly proportional to the current value. If the test charge and the wire with a current are located in “LP” frame, i.e., there is no interaction of the charge with the current, what we just observe in ordinary laboratory conditions (in “calm air” conditions).

So, if the proposed model corresponds to reality, then beyond “LP” the motionless positive charge must suffer a repulsion from the current-carrying conductor. Continuing the analogy with the air, it can be said: the experiment should be carried out so, that any protection from the “wind” is brought down to minimum.

(STR requires in this experiment―like in the all others - the complete symmetry: presence or absence of “wind protection” should in no way affect the results of the experiment).

Let us assume that “LP” reference frame is that, wherein the cosmic microwave background radiation is isotropic. Then the interaction of a motionless charge with a current will be maximum in the case, when the electron drift in the wire is co-directed with the movement of the Earth relative to cosmic microwave background, and will be zero, if the wire is perpendicular to this direction.

Before proceeding to the description of the experimental set-up, let us make a rough estimate of the expected force of interaction for realistically achievable values of the test charge, value of the current and distances. Let the speed w of the laboratory reference frame relative to “LP” is the order of 300 km/s, and electron drift speed in the wire

is the order of 5 mm/s. Then.

At a current of the order of 1,000 A the specific charge of drifting electrons per unit

length of the wire:. If the conductor represents a thin plate,

wherein the current density is the same everywhere, then on 25 cm long section the drifting charge of will be concentrated, that, at the plate width of 3.6 cm, gives the specific charge per area unit. When carrying out the experiment in conditions of “LP” frame (the presence of “wind protection”), the entire charge of moving electrons is compensated by the equal charge of wire ions. Under conditions, corresponding to Figure 6, uncompensated specific ion charge will be equal to, i.e. as estimated, the order of 10⁻⁷ C/m². Subject to described conditions (complete absence of obstacles for the “wind” and location of the current-carrying wire so that electrons in it would drift in the same direction, where the Earth is moving relative to cosmic microwave background), such plate with a current should interact with the test charge in the same way, as if it was charged up to the calculated charge density.

In the experimental set-up the plate 3.6 ´ 25 cm is used as a test charge, located on the torsion balance arm in parallel to the current-carrying plate and at a distance of the order of 1 mm from it. To both plates a positive potential 10⁴ V is applied with respect to the ground. Electrostatic repulsion of plates is compensated with the plate of large area, located on the other side from the torsion balance at the distance of 20 cm.

The charge on the movable plate is determined by its potential and capacity with respect to the ground. The target of the experiment is not measurement of plates interaction force (for this there would be required not only the highly sensitive equipment, but also such tough provision of installation withdrawal from under the “wind protection”, to reach which on the Earth’s surface is nonreal), but a qualitative assessment. Therefore, for the preliminary estimations of torsion balance sensitivity, the precise determination of the plate capacity will not be needed. Theoretical evaluation and rough measurement give the single result: the plate capacity is the order of 20 pF. For the voltage 10⁴ V it gives the charge value 2 × 10⁻⁷ Kl and specific charge of the order of 2 × 10⁻⁵ C/m².

After supply of the potential 10⁴ V to two parallel plates (whereupon the torsion balance are motionless, since the interaction is compensated with the third plate), the current of 1,000 A begins to flow, that should lead to appearance of a repulsion force― such as if at current-carrying plate have emerged an additional positive charge of 10⁻⁷ C/m². Calculated value of this force is the order of 10⁻³ N. However, such interaction force is achievable only in the complete absence of “wind protection” (in other words, in the Earth orbit and at strict co-directionality of plates with w vector). In the case of experimental set-up location on a hill, in the absence of monumental walls and robust enclosure, and at the possibly exact orientation of the current-carrying plate in the movement direction of the Earth relative to the cosmic microwave background, obtaining of plate’s interaction force can be expected, differing from the maximum force for not more than two orders of magnitude, i.e. such, which would correspond to the speed value w of the order of km/s units. In other words, the torsion balance, confidently responding to the interaction force of the order of 10⁻⁵ N, should be recognized as suitable for the test experiment.

In order to cause the attraction of plates with the specified force, it is possible to create a potential difference between them, thereby transforming them into plates of a plane capacitor. For two plain plates with the area of 90 cm², arranged in the air at a distance of 1 mm and being attracted with the force of 10⁻⁵ N, we obtain: if the torsion balance respond to a voltage of about 15 V, then their sensitivity is sufficient to verify the theory at the expected values w.

4. Experimental Set-Up

With due regard to the requirement of the “wind protection” minimization, the working part of the experimental set-up is assembled in a glass enclosure (the wall thickness of 4 mm). The source of current is a battery (12 V, 55 Ah), which can maintain in the current-carrying plate the constant current of the order of 1000 A for 20 seconds. To create the stable DC voltage of 10 kV the high-voltage source is used, powered by a battery of electrochemical cells. The entire installation is mobile and can be assembled outside the laboratory in the open area. This allows choosing for experiment carrying out a hill whereon it is possible to orient plates along a straight line, along which at the moment of the experiment the Earth moves relative to the isotropic space microwave background (the direction to the border of Leo and Crater constellations), at that between plates and outer space on this straight line there are no obstacles in both directions except the glass enclosure and the Earth’s atmosphere.

Figure 7 shows the torsion balance; the balance-beam of which is made of 0.5 mm thick foil-coated fibre-glass plastic and is suspended on a copper filament with diameter 0.5 mm. The balance beam is arranged parallel to the current-carrying plate (the strip of pure copper 250 × 36 × 0.5 mm, wires to a power supply attached on it with screws are seen), at that the П-shaped beam counterweight is located outside the current effective area. Inside the glass enclosure at its rear wall parallel to the counterweight the vertical conductive plate is located, connected, as well as both working plates, to a high voltage source. This plate serves for balancing the torsion balance; it can be moved along the bottom surface of the enclosure at enabled high voltage with the help of a vertical glass handle. The entire front wall of the glass enclosure is closed on the inside with the motionless plate, to which the high voltage is supplied too.

After pre-balancing torsion balance beam in the operating position (parallel to the

current-carrying plate at the distance of about 1 mm from it) the high voltage is switched on. Then, the fine balancing is performed with the help of the movable plate. Since the suspension of the torsion balance is made of annealed copper, the elastic properties of which are small, beam oscillations damp in a few seconds. Thereafter current-carrying plate is connected to the power supply for 20 seconds (in such period of time wires and plate are heated up to the maximum permissible temperature).

To evaluate the sensitivity of a torsion balance, the battery of electrochemical cells is temporarily switched into the electrical circuit between the high voltage source and the working plate (beam)―in such a way that after the fine balancing of scales at enabled high voltage between the balance beam and current-carrying plate it is possible to create a potential difference of 15 V. After battery switching the beam extreme point is attracted to the motionless plate till a touch (i.e., for 1 mm) in less than 8 seconds. This gives the reason to suppose the following: repulsion of the beam edge from the current-carrying plate (from the initial parallel position) for a distance of the order of 1 mm in about the same time would mean the appearance on the current-carrying plate of “excess charge”, which according to an order of magnitude corresponds to the calculated above.

During indoor tuning-up of the installation, the experiment gives the implicitly negative result: beforehand balanced torsion balance, when switching on of the current in the motionless plate, remain motionless in the course of 20 seconds. This is true for any orientation of plates in the cardinal directions.

Thus, the main task is the following: find out if the experiment result depends on the installation orientation, if it is assembled in an open area under the conditions, when outside the enclosure there are no visible obstacles on “Earth―the border of Leo and Crater constellations” straight line.

5. Experimental Results

The experiment was carried out in Leningrad region, in the area of Lake Hepoyarvi, on a dominating hill. The installation was assembled in an open area, subject to the condition observance of the lack of visible obstacles on the straight line, along which at the time of the experiment performance moved the Earth relative to the isotropic cosmic microwave background radiation. The current-carrying plate and the torsion balance beam at the current switching moment were parallel to this straight line with the accuracy ± of 5 degrees.

Three series of experiments in total were carried out within two weeks, by ten current switching in each series. Experiments were conducted at that time of the day, when the border of Leo and Crater constellations was located close to the horizon, which allowed directing horizontal plates at this point. The time term between separate experiments is in each series 5 - 7 minutes (the time necessary for cooling wires). At the beginning and end of each series the control experiment was performed: the current was switched on at the arrangement of the installation plates, perpendicular to the working position. In all these control experiments after switching on the current the beam remained motionless for 20 seconds.

In all the experiments at the working location of plates, the torsion balance beam after switching on the current was repulsed from the current-carrying plate. The time, in which the beam end moved away from the current-carrying plate for a distance of the order of 1 mm, was different and varied in the range 5 to 12 seconds. (With increasing the angle between installation plate direction and “Earth―the border of Leo and Crater constellations” straight line, the beam rotation rate decreased, and at the angle of about 30 degrees became indistinguishable).

Results of this experiment should be considered as preliminary. One cannot declare the single-value confirmation of effect, predicted by the theory. The experiments should be repeated many times in different geographical locations and at high altitudes (ideally in the Earth orbit). For transition from a qualitative result to the measurement of described interaction value (this will allow to calculate the value of laboratory speed relative to “light preferred” reference frame) a more sensitive experimental device will be required.

6. Conclusions

The theory of relativity has provided answers to the questions raised by experimental data. Classical mechanics leads to contradictions when attempting to explain stellar aberration by the Fizeau and Michelson-Morley experiments. This contradiction is eliminated by the postulate of the independence of the speed of light in vacuum from the movements of source and observer (see [2] - [15] ). Over the past century, the experimental data with high accuracy confirmed the correctness of STR, with the experiment by Ives and Stillwell [16] being crucial and the most compellent.

The article [1] and this paper have attempted to find a solution to the same problem, distinct from Einstein’s solution. The proposed solution is based particularly on the condition of existence of an original reference frame in which the speed of light is isotropic. The results obtained are consistent with the logic of the theory of relativity. This approach allowed for derivation of all kinematic and electro dynamic conclusions of STR, as well as for Mach’s principle for rotation (see [1] ).

It also demonstrates that the experimental discrepancies from STR predicted by this approach are so minute that they cannot be detected in the vast majority of laboratory experiments. Still, this discrepancy does exist, and its detection does not require high- precision measurements or expensive facilities. Such an experimental validation is proposed in this paper. Carrying out the experiment described here in a specialized laboratory setting as opposed to the “home-made” variant implemented so far would answer the question of whether the proposed logical framework is just an abstraction or a reflection of physical reality.

Acknowledgements

This work was supported by the Saint Petersburg Academic University of the Russian Academy of Sciences.

Cite this paper

Filippov, L. (2016) On a Heuristic Point of View Concerning the Mechanics and Electrodynamics of Moving Bodies. World Journal of Mechanics, 6, 305-322. http://dx.doi.org/10.4236/wjm.2016.69023

References