World Journal of Mechanics
Vol.06 No.08(2016), Article ID:69798,11 pages
10.4236/wjm.2016.68019
The Harmonic Oscillator with Random Damping in Non-Markovian Thermal Bath
N. J. Hassan1, A. Pourdarvish1, J. Sadeghi2
1Department of Statistics, Faculty of Mathematical Sciences, University of Mazandran, Babolsar, Iran
2Department of Physics, Faculty of Basic Science, University of Mazandaran, Babolsar, Iran

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 10 July 2016; accepted 14 August 2016; published 17 August 2016
ABSTRACT
In this paper, we define the harmonic oscillator with random damping in non-Markovian thermal bath. This model represents new version of the random oscillators. In this side, we derive the overdamped harmonic oscillator with multiplicative colored noise and translate it into the additive colored noise by changing the variables. The overdamped harmonic oscillator is stochastic differential equation driving by colored noise. We derive the change in the total entropy production (CTEP) of the model and calculate the mean and variance. We show the fluctuation theorem (FT) which is invalid at any order in the time correlation. The problem of the deriving of the CTEP is studied in two different examples of the harmonic potential. Finally, we give the conclusion and plan for future works.
Keywords:
Random Damping, Total Entropy, Non-Markovian Bath, Fluctuation Theorem, Additive Colored Noise

1. Introduction
In the 1980s, studies of linear and non linear oscillators were extended to the case of colored noise driving force. Many applications of the random damping in Markovian thermal bath include water waves influenced by turbulent wind field, the Ginzburg-Landau equation with a convective term, mean flow passing through a region under study, open flows of liquids, dendritic growth, chemical waves and motion of vortices [1] - [7] respectively. The effect of correlations in the random driving force on the stationary probability density and its moments were studied [8] - [12] . The exact formula is found for the first moment and the system of equations for second moments for harmonic oscillator with random mass [13] . The analytical expressions are derived for the sta- tionary probability density of the particle’s energy [14] . Both theoretical approaches were formulated in the context of the standard Langevin equation [15] [16] , where the friction force was proportional to the speed with a constant friction coefficient and additive Gaussian white noise. The non-equilibrium process efforts are com- monly formulated in the form of stochastic thermodynamic culminates into fluctuation relations connecting exten- sive thermodynamic variables such as work, free energy, and entropy [17] - [21] . The violation of the Markovian approximation of the environment leads to generation of additional entropy [21] . The ideas behind the entropy production are studied and some insights are given about relevance [22] . The statistical properties of stochastic entropy production associated with the non stationary transport of heat through system coupled to a time depen- dent nanisothermal heat bath [23] . When the harmonic oscillator with external noise in non-Markovian thermal bath, the cumulants of order two and three contain the natural effects of the non-Markovian bath through the noise correlation time, consequently the non Gaussian characteristic of the totel entropy change drives to a breakdown of the usual fluctuation theorems [24] . The purpose of this paper is discussing the change in total entropy production for the harmonic oscillator with random damping in non-Markovian thermal bath and stu- dying the fluctuation theorem (FT) at any order in time correlation when the harmonic potentials are represented the time dependent driving force or the time dependent dragging force where this force is arbitrary time depen- dent. We derive in this paper the stochastic differential equation (SDE) driving by the multiplicative colored noise and translate it into additive colored noise by changing variables from x to y. In this side, in order to compatible the additive colored noise system with potential, we change variables in harmonic potentials (time dependent driving force and time dependent dragging force). We calculate the mean, variance and the distri- bution function for the change in total entropy production in new formulas of the harmonic potentials. We show that in our model, the fluctuation theorem is invalid at any order in the noise correlation time. Finally, we pre- sent our conclusion and we give the future works. This paper can be divided by six sections. In Section 2, we define the new model based on the SDE driving by additive colored noise in the overdamped approximation, and we find the Fokker Planck equation which it is associated of the SDE. In Section 3, we change variables in Equation (1) from x into y, this represent first example. In this example, we find the change in total entropy, mean and variance. In Section 4, we change variables in Equation (2), this represent second example. In this example, we also compute the change in total entropy, mean and variance. In Section 5, we show that the FT is invalid at any order in the time correlation. Finally, we introduce the conclusions and future works.
2. Stochastic Differential Equation (SDE) Driving by Additive Colored Noise in Overdamed Approximation
In this section, we define the harmonic oscillator with random damping in non-Markovian thermal bath. We de- rive the stochastic differential equation (SDE) driving by the multiplicative colored noise and translate it into the additive colored noise by changing variables in overdamped approximation and its stochastic treatment. Our model can be defined as,
 (1)
(1)
where 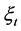 is Ornstein-Uhlenbeck noise (special type of colored noise),
is Ornstein-Uhlenbeck noise (special type of colored noise), 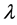 is friction constant,
is friction constant, 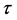 is corre- lation time,
is corre- lation time, 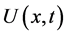 is the harmonic potential,
is the harmonic potential, 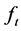 is arbitrary time depend force,
is arbitrary time depend force, 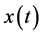 is particle’s position and
is particle’s position and  is the velocity. The
is the velocity. The 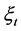 is Gaussian distribution with zero mean and correlation function is,
is Gaussian distribution with zero mean and correlation function is,
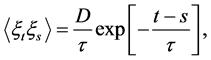 (2)
(2)
where 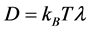 such that
such that 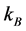 is Boltzmann constant and T is heat temperature. We assume that the following ,
is Boltzmann constant and T is heat temperature. We assume that the following ,
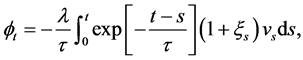 (3)
(3)
and read the Equation (1) as,
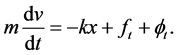 (4)
(4)
In overdamped approximation , the Equation (1) become,
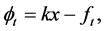 (5)
(5)
taking the time derivative of Equation (3) we get,

Substituting Equation (5) in (6), one can obtain,

let 


By using power series at first order in the noise

let 


cative colored noise. To translate Equation (9) from multiplicative colored noise into additive ,we must divided Equation (9) by

let,

then the translation [25] is defined as,

then the Equation (10) is,

Equation (13) represent SDE driving by additive colored noise. The Fokker Planck equation [25] is defined,

where the initial condition

we take the time derivative into above equation, we have,

Assume that

where 

To obtain the initial distribution function we must assume that 
mean (

where the initial distribution is exponential distribution. Then the marginal probability of the particle’s position is,

also the distribution of y is exponential distribution , and note that y and 
3. The First Example
In this section, we change variables in the time dependent driving force from x into y which is defined as

where 

Substituting Equation (22) in Equation (21), one can obtain,

Equation (23) represent first new formula of harmonic potential in y. The change in new harmonic potential can be defined as,

where we assume that

where the work can be computed [29] as,

The mean of the work is calculated as,

where the quantity 

Putting Equation (28) inside Equation (27), we get,

the variance of the work can be calculated as,

where the quantity 

Substituting Equation (31) inside Equation (30). one can obtain,

The change in the environment entropy 

The change in entropy of the system 

Now, we can find the CTEP 

where 


we must calculate the following quantities



where

and,

note here

before we find the variance of the CTEP, we make some the following assumptions, let 










we must calculate the following quantities






note here


and,

Putting Equation (32) and an above quantities’s values in Equation (41), one can obtain,

At zero order in time correlation

where 


and variance,

where 



4. The Second Example
In this section , we change variables in the time dependent driving force from x into y which is defined as

where 

Equation (52) represent second new formula of harmonic potential in y .

where we assume that

The mean of the work is calculated as,

and the variance of the work is,

We note that, the mean of the work in first and second example are different, while, the variance is equal. The change in the environment entropy 

where


The variance of the 

since the variance of the 

then we note, the variance of the change in entropy of the environment is same in tow examples, while the mean is different. Now, we can calculate 

The mean of the 

The variance of the 

At zero order in time correlation , the CTEP 

the mean is,

and variance is,

where 





ples are different. From Equations (59) and (60), we conclude that the variance of the CTEP is the same in two examples and at any order in time correlation and also we can not find any linear relation between the mean and variance of the CTEP at any order in time correlation , while ref. [24] is shown that the entropy variance is same in his two examples at first order in time correlation and it found the relation between the mean and variance of the CTEP at first order in time correlation.
5. Fluctuation Theorem
In this section, we show the fluctuation theorem (FT) is invalid at any order in time correlation whether the distribution function of the change in the total entropy production (CTEP) is Gaussian or non Gaussian. We study the distribution function of the CTEP with respect first example , because any example chosen no problem. We base on the relation between the moments and cumulants to find the distribution function of the CTEP which is defined as,

where 




from above equation, we find that the 




The other perspective, at any order in u, this perspective is studied in [24] , it show that the distribution function of the CTEP is non Gaussian and the FT is invalid as

Based on Equation (70), we note that ,at any order in time correlation, the FT in our work is invalid, while at zero order in time correlation, the FT in [24] becomes valid.
6. Conclusion and Future Work
In this letter, we defined the harmonic oscillator with random in non-Markovian thermal bath and we derived the SDE driving by multiplicative colored noise. By changing variables, we translated SDE from multiplicative colored noise into additive colored noise to become the calculations easier. Under the new formulas of the har- monic potential in the two examples, we derived the change in the total entropy production (CTEP) of the our model and calculated the mean and the variance. By comparing our results in the two examples, we found the variances of the CTEP are the same while the means are different. At zero order in the time correlation, in first example the mean of the CTEP equal zero while in other example the mean is nonzero, also we find the variances in the two examples are different. In the two examples we can not obtain on the linear relation be- tween the variance and the mean at any order in time correlation, while [24] it obtained this relation at zero order in the time correlation. The FT in our work is invalid at any order in the time correlation while in [24] the FT is valid at zero order in the time correlation. Finally , we will study the harmonic oscillator with random frequency in Markovian and non-Markovian thermal bath. This problem will be done in future.
Acknowledgements
We thanks Iraqi Ministry of Higher Education and Scientific Research, specifically Iraqi Cultural Relations and Scholarship Department and Cultural Attach in Tehran.
Cite this paper
N. J. Hassan,A. Pourdarvish,J. Sadeghi, (2016) The Harmonic Oscillator with Random Damping in Non-Markovian Thermal Bath. World Journal of Mechanics,06,238-248. doi: 10.4236/wjm.2016.68019
References
- 1. West, B. and Seshadri, V. (1981) Model of Gravity Wave Growth Due to Fluctuations in the Air-Sea Coupling Parameter. Journal of Geophysical Research, 86, 4293.
http://dx.doi.org/10.1029/JC086iC05p04293 - 2. Gitterman, M. (2003) Phase Transitions in Moving Systems. Physical Review E, 70, Article ID: 036116.
http://dx.doi.org/10.1103/PhysRevE.70.036116 - 3. Onuki, A. (1997) Phase Transitions of Fluids in Shear Flow. Journal of Physics: Condensed Matter, 9, 6119.
http://dx.doi.org/10.1088/0953-8984/9/29/001 - 4. Chomaz, J.M. and Couairon, A. (1999) Against the Wind. Physics of Fluids, 11, 2977.
http://dx.doi.org/10.1063/1.870157 - 5. Hestol, F. and Libchaber, A. (1985) Unidirectional Crystal Growth and Crystal Anisotropy. Physica Scripta, T9, 126.
- 6. Saul, A. and Showalter, K. (1985) Oscillations and Traveling Waves in Chemical Systems. In: Field, R.J. and Burger, M., Eds., Propagating Rraction-Diffusion Fronts, Wiley, New York, 419-440.
- 7. Gitterman, M., Ya Shapiro, B. and Shapiro, I. (2002) Phase Transitions in Vortex Matter Driven by Bias Current. Physical Review B, 65, Article ID: 174510.
http://dx.doi.org/10.1103/PhysRevB.65.174510 - 8. Fronzoni, L., Grigolini, P., Hanggi, P., Moss, F., Mannella, R. and McClintock, P.V.E. (1986) Bistable Oscillator Dynamics by Nonwhite Noise. Physical Review A, 33, 3320.
http://dx.doi.org/10.1103/PhysRevA.33.3320 - 9. Kosek-Dygas, M., Matkowsky, B. and Schuss, Z. (1988) Colored Noise in Dynamical Systems. SIAM Journal on Applied Mathematics, 48, 425.
http://dx.doi.org/10.1137/0148023 - 10. Mannella, R., McClintock, P.V.E. and Moss, F. (1987) Spectral Distribution of a Double-Well Duffing Oscillator Subject to a Random Force. Europhysics Letters, 4, 511. http://dx.doi.org/10.1209/0295-5075/4/5/001
- 11. Hanggi, P. and Jung, P. (1995) Colored Noise in Dynamical Systems. Advances in Chemical Physics, 89, 239.
http://dx.doi.org/10.1002/9780470141489.ch4 - 12. Gitterman, M. (2010) New Stochastic Equation for a Harmonic Oscillator: Brownian Motion with Adhesion. Journal of Physics: Conference Series, 248, Article ID: 012049.
http://dx.doi.org/10.1088/1742-6596/248/1/012049 - 13. Vicenc, M., Daniel, C. and Werner, H. (2013) Stationary Energy Probability Density of Oscillators Driven by a Random External Force. Physical Review E, 87, Article ID: 062132.
http://dx.doi.org/10.1103/PhysRevE.87.062132 - 14. Saha, A., Lahiri, S. and Jayannavar, A.M. (2009) Entropy Production Theorems and Some Consequences. Physical Review E, 80, Article ID: 011117.
http://dx.doi.org/10.1103/PhysRevE.80.011117 - 15. Jimnez-Aquino, J.I. (2010) Entropy Production Theorem for a Charged Particle in an Electromagnetic Field. Physical Review E, 82, Article ID: 051118.
http://dx.doi.org/10.1103/PhysRevE.82.051118 - 16. Seifert, U. (2008) Stochastic Thermodynamics: Principles and Perspectives. European Physical Journal B, 64, 423-431.
http://dx.doi.org/10.1140/epjb/e2008-00001-9 - 17. Seifert, U. (2005) Entropy Production along a Stochastic Trajectory and an Integral Fluctuation Theorem. Physical Review Letters, 95, Article ID: 040602.
http://dx.doi.org/10.1103/physrevlett.95.040602 - 18. Jarzynski, C. (1997) Equilibrium Free-Energy Differences from Nonequilibrium Measurements: A Master-Equation Approach. Physical Review E, 56, 5018-5035.
http://dx.doi.org/10.1103/PhysRevE.56.5018 - 19. Sagawa, T. and Ueda, M. (2010) Generalized Jarzynski Equality under Nonequilibrium Feedback Control. Physical Review Letters, 104, Article ID: 090602.
http://dx.doi.org/10.1103/physrevlett.104.090602 - 20. Crooks, G.E. (1999) Entropy Production Fluctuation Theorem and the Nonequilibrium Work Relation for Free Energy Differences. Physical Review E, 60, 2721-2726.
http://dx.doi.org/10.1103/PhysRevE.60.2721 - 21. Aki, K., Tapio, A.N. and Jukka, P. (2015) Entropy Production in a Non-Markovian Environment. Physical Review E, 92, Article ID: 012107.
- 22. Velasco, R.M., Scherer Garca-Coln, L. and Uribe, F.J. (2011) Entropy Production: Its Role in Non-Equilibrium Thermodynamics. Entropy, 13, 82-116.
http://dx.doi.org/10.3390/e13010082 - 23. Ford, J., Laker, P.L. and Charlesworth, J. (2015) Stochastic Entropy Production Arising from Nonstationary Thermal Transport. Physical Review E, 92, Article ID: 042108.
http://dx.doi.org/10.1103/physreve.92.042108 - 24. Jimnez-Aquino, J.I. and Velasco, R.M. (2014) The Entropy Production Distribution in Non-Markovian Thermal Baths. Entropy, 16, 1917-1930.
http://dx.doi.org/10.3390/e16041917 - 25. Hänggi, P. and Peter, J. (1995) Colored Noise Dynamic. John Wiley and Sons Inc., Hoboken.
- 26. Risken, H. (1984) The Fokker Planck Equation. Springer-Verlag, Berlin.
http://dx.doi.org/10.1007/978-3-642-96807-5 - 27. Seifert, U. (2012) Stochastic Thermodynamics, Fluctuation Theorems and Molecular Machines. Reports on Progress in Physics, 75, 12601.
http://dx.doi.org/10.1088/0034-4885/75/12/126001 - 28. Sekimoto, K. (1998) Langevin Equation and Thermodynamics. Progress of Theoretical Physics, 130, 17-27.
http://dx.doi.org/10.1143/PTPS.130.17 - 29. Jarzynski, C. (2011) Equalities and Inequalities: Irreversibility and the Second Law of Thermodynamics at the Nanoscale. Annual Review of Condensed Matter Physics, 2, 329-351.
http://dx.doi.org/10.1146/annurev-conmatphys-062910-140506

