World Journal of Condensed Matter Physics
Vol.08 No.03(2018), Article ID:86866,6 pages
10.4236/wjcmp.2018.83007
On the Isotope-Like Effect for High-Tc Superconductors in the Scenario of 2-Phonon Exchange Mechanism for Pairing
G. P. Malik1,2
1Present Address: B-208 Sushant Lok 1, Gurgaon, Haryana, India
2Formerly: Theory Group, School of Environmental Sciences, Jawaharlal Nehru University, New Delhi, India
Copyright © 2018 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: August 1, 2018; Accepted: August 21, 2018; Published: August 24, 2018
ABSTRACT
By generalizing the isotope effect for elemental superconductors (SCs) to the case of pairing in the 2-phonon exchange mechanism for composite SCs, we give here an explanation of the well-known increase in the critical temperature (Tc) of Bi2Sr2CaCu2O8 from 95 K to 110 K and of Bi2Sr2Ca2Cu3O10 from 105 to 115 - 125 K when Bi and Sr in these are replaced by Tl and Ba, respectively. On this basis, we also give the estimated Tcs of some hypothetical SCs, assuming that they may be fabricated by substitutions similar to Bi → Tl and Sr → Ba.
Keywords:
Isotope-Like Effect, 2-Phonon Exchange Mechanism, Bi- and Tl-Based High-Tc SCs, Suggestions for New Substitutions for Further Enhancement of Tc of the Bi-Based SCs

1. Introduction
In this note we deal with an explanation of why the replacements of Bi and Sr in Bi2Sr2CaCu2O8 and Bi2Sr2Ca2Cu3O10 by Tl and Ba, respectively, lead to an increase in the critical temperature Tc of the former from 95 K to 110 K and of the latter from 105 to 115 - 125 K. This is an important undertaking because it has the potential to act as a general guide about substituting one or the other element in a composite superconductor (SC) in order to enhance its Tc.
Empirically, it has been shown [1] that greater the Tc of an SC, greater is its critical current density j0, for which, theoretically, an expression has been derived in terms of the following five parameters [2] [3] : Debye temperature θ, the electronic specific heat constant γ, gram-atomic volume vg, Fermi energy EF, and a dimensionless construct 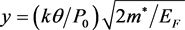 where k is the Boltzmann constant, P0 the critical momentum of Cooper pairs and m* the effective mass of an electron. Therefore, if theory could predict the values of these parameters after one or more substitutions are made in an SC, then we would have a handle on its j0 and Tc. Since, as of now, theory cannot perform this task, we must resort to an approach that relies on a property or properties that can be unequivocally determined, regardless of the number of elements that are replaced by others. Such a property is the mass of the SC.
where k is the Boltzmann constant, P0 the critical momentum of Cooper pairs and m* the effective mass of an electron. Therefore, if theory could predict the values of these parameters after one or more substitutions are made in an SC, then we would have a handle on its j0 and Tc. Since, as of now, theory cannot perform this task, we must resort to an approach that relies on a property or properties that can be unequivocally determined, regardless of the number of elements that are replaced by others. Such a property is the mass of the SC.
Above considerations lead us to recall the isotope effect
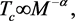 (1)
(1)
where M is the average mass of ions of an elemental SC. While BCS theory gives the value of α as 0.5, values significantly different from this have also been found for some elements, e.g., Mo, Os, and Ru, which are characterized by α = 0.33, 0.2, and 0, respectively. Hence, while (1) does not have the status of a law, it nonetheless helped in the formulation of BCS theory because it sheds light on the role of the ion lattice in the scenario of 1-phonon exchange mechanism (1 PEM) for pairing. Since the Tcs and gaps of all the SCs dealt with here have been explained in the framework of the generalized-BCS equations (GBCSEs) employing the 2-phonon exchange mechanism (2 PEM) [4] , we take up in the next Section the task of generalizing (1) for this case. Applications of the generalized equation are addressed in Section 3, where also given are the estimated values of Tcs of some members of the families of SCs represented by X2Y2CaCu2O8 and X2Y2Ca2Cu3O10 for different choices of X and Y. The final Section sums up our present study.
2. Isotope-Like Effect in the 2 PEM Scenario
GBCSE for the Tc of a composite SC in the 2 PEM scenario is [5]
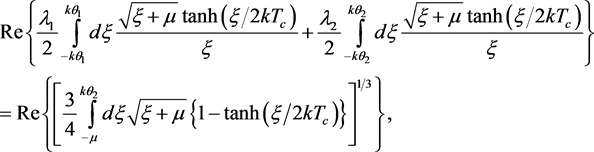 (2)
(2)
where chemical potential μ has been used interchangeably with EF, θ1 and θ2 are the Debye temperatures and λ1 and λ2 the interaction parameters of the ion-species responsible for pairing, and the operator Re ensures that the integrals yield real values even when ξ + μ < 0. When either of the λs is zero and μ >> kθ1 (or kθ2), (2) reduces to the usual BCS equation for the Tc of an elemental SC in the 1 PEM scenario. Since Tc of an SC in the 2 PEM scenario is due to the cooperative effect of two kinds of ions, the following generalization of (1) suggests itself naturally
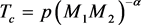 (3)
(3)
where p is the constant of proportionality and M1 and M2 are the masses of the ion-species that cause pairing. A discussion of (3) vis-à-vis (1) will be given below.
3. Applications of Equation (3)
3.1. Bi2Sr2CaCu2O8
Pairing in this SC may be caused by the cooperative effect of one or more of the following pairs of ions: Bi and Sr, Bi and Ca, and Sr and Ca. For each of these choices, guided by the values of α noted in Section 1, we calculate the value of p corresponding to Tc = 95 K, and α = 0.5, 0.4, 0.3, 0.2, and 0.1. With MBi = 208.98, MSr = 87.62, and MCa = 40.08 (amu), we then obtain the results given in Table 1.
3.2. Tl2Ba2CaCu2O8
We calculate Tc (Tl2Ba2CaCu2O8) via (3) for each of the 15 {α, p}-values in Table 1 by successively taking M1M2 as MTlMBa, MTlMCa, and MBaMCa. With MTl = 204.39 and MBa = 137.33 (amu), the resulting 45 values are given in Table 2.
It is seen from Table 1 and Table 2 that while α = 0.2 and p = 676.6 correspond to Tc (Bi2Sr2CaCu2O8) = 95 K in the 2PEM scenario involving predominantly the Bi and Sr ions, they lead to Tc (Tl2Ba2CaCu2O8) = 111.6. K when pairing is predominantly due to the Tl and Ca ions. Two other notable pairs of {α, p}-values in Table 1 are {0.3, 1427.7} and {0.1, 253.5} which yield, respectively, values of Tc (Tl2Ba2CaCu2O8) as 107.8 and 107.1 K (pairing via the Ba and Ca ions in each case); both of these Tcs are also close to the experimental values of 110 K.
Since Bi and Tl belong to the same period and Sr and Ba to the same group of the periodic table, it seems interesting to investigate the effects of further similar substitutions in light of the above results. We assume that it is feasible to obtain the compounds noted below from the parent compound Bi2Sr2CaCu2O8.
For α = 0.2 and p = 676.6 K amu2α, we obtain
1) Tc (Bi2Mg2CaCu2O8); via Bi + Mg) = 123 K = Tc (Tl2Mg2CaCu2O8); via Tl + Mg)
2) Tc (Bi2 (or Tl2) Mg2CaCu2O8); via Bi + Mg) = 171 K
Table 1. The values of p obtained by solving (3) corresponding to Tc = 95 K, α = 0.5, 0.4, 0.3, 0.2, and 0.1 and different choices of ions responsible for pairing in Bi2Sr2CaCu2O8.
Table 2. Tc (Tl2Ba2CaCu2O8) calculated via (3) for each pair of {α, p} values in Table 1 for different combinations of ions in the 2 PEM scenario.
3) Tc (Bi2Be2CaCu2O8); via Bi + Be) = 150 K = Tc (Tl2Be2CaCu2O8); via Tl + Be)
4) Tc (Bi2 or (Tl2) Be2CaCu2O8); via Be + Ca) = 208 K
3.3. Bi2Sr2Ca2Cu3O10 and Tl2Ba2Ca2Cu3O10
Following the same procedure as above, we can find 15 {α, p}-values, each of them corresponding to Tc (Bi2Sr2Ca2Cu3O10) = 105 K. Among these, the following four sets: {0.4, 3894.1; Bi + Ca}, {0.3, 1578.0; Bi + Ca}, {0.1, 280.2; Bi + Sr}, and {0.2, 747.7; Bi + Sr} also lead to Tc (Tl2Ba2Ca2Cu3O10) in the range 115 - 125 K; the Tc-values corresponding to the first three due to the (Ba + Ca) ions are 124.2, 119.1, and 118.4 K, respectively, and the fourth value is 123.3 K due to the (Tl + Ca) ions.
Some typical Tc-values corresponding to {α, p} = {0.4, 3894.1} when Sr in Bi2Sr2CaCu3O10 is replaced by Mg and Be, respectively, are 248.2 (due to Mg + Ca) and 190.8 K (due to Bi + Be).
4. Discussion and Conclusion
It was noted above that BCS theory gives the value of α in (1) as 0.5. This follows from two relations: (a) 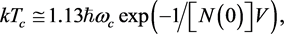 where ωc is Debye frequency of the ions, N(0) the density of states at the Fermi surface, and V the net attraction between electrons bound as pairs, and (b)
where ωc is Debye frequency of the ions, N(0) the density of states at the Fermi surface, and V the net attraction between electrons bound as pairs, and (b) 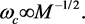 It is hence seen that α = 0.5 only if N(0) and V do not change when one isotope is replaced by another. This is a reasonable assumption for N(0) because it is a purely electronic property; not so for V which is determined jointly by the ions and the electrons. It is not surprising therefore that α = 0.5 holds only for a few so-called classic elemental SCs, e.g., Zn, Pb, and Hg and that most of the other SCs are characterized by a multitude of values―some of which were noted above. Since, unlike elemental SCs, we do not have an analytic expression for the Tc of a composite SC, we cannot derive for it a “blanket relation” such as α = 0.5. The value of α for such SCs is expected to differ from family-to-family and we believe to have indicated how it may be tested; besides, in the best-case scenario, it may prove to be useful in the current endeavor to reach room temperature Tcs.
It is hence seen that α = 0.5 only if N(0) and V do not change when one isotope is replaced by another. This is a reasonable assumption for N(0) because it is a purely electronic property; not so for V which is determined jointly by the ions and the electrons. It is not surprising therefore that α = 0.5 holds only for a few so-called classic elemental SCs, e.g., Zn, Pb, and Hg and that most of the other SCs are characterized by a multitude of values―some of which were noted above. Since, unlike elemental SCs, we do not have an analytic expression for the Tc of a composite SC, we cannot derive for it a “blanket relation” such as α = 0.5. The value of α for such SCs is expected to differ from family-to-family and we believe to have indicated how it may be tested; besides, in the best-case scenario, it may prove to be useful in the current endeavor to reach room temperature Tcs.
The applicability of the isotope effect via (1) has been investigated experimentally for the high-Tc SC YBa2Cu3O7 by replacing up to 75% of its O-16 by O-18 [4] . Since this did not have any effect on the Tc, it was concluded that α = 0 for this SC. We note in this context that scattering with the O ions is not the direct cause of pairing in any SC; what needs to be monitored is the change in Tc when one or more ions that are actually responsible for pairing are substituted. A remark about the vital role of CuO2 planes in an SC is in order since greater their number, greater is the Tc of the SC. We believe that the dual role of these planes is (a) to meet the stoichiometric requirements for the stability of the SC when new ion layers are added to it to provide additional channels for pairing and (b) to provide additional sites for pairs to reside on.
To conclude, by appealing to an isotope-like effect, we have given here an explanation of the known increase in the Tcs of Bi2Sr2CaCu2O8 and Bi2Sr2Ca2Cu3O10 when Bi and Sr in these are replaced by Tl and Ba, respectively. Based on this approach, we have given plausible values of Tcs of some hypothetical SCs that may be obtained from the parent SCs by one or more substitutions. Fabrication of these hypothetical SCs, e.g., Tl2Be2CaCu2O8 and ensuring that pairing in it occurs predominantly via the Be and the Ca ions (which lead to Tc = 208 K), is a problem that belongs to the realm of chemical engineering.
Acknowledgements
Author thanks Professor D. C Mattis for a critical reading of the manuscript and for encouragement.
Cite this paper
Malik, G.P. (2018) On the Isotope-Like Effect for High-Tc Superconductors in the Scenario of 2-Phonon Exchange Mechanism for Pairing. World Journal of Condensed Matter Physics, 8, 109-114. https://doi.org/10.4236/wjcmp.2018.83007
References
- 1. Semenov, A., et al. (2009) Optical and Transport Properties of Ultrathin NbN and Nanostructures. Physical Review B, 80, 054510. https://doi.org/10.1103/PhysRevB.80.054510
- 2. Malik, G.P. (2016) On the Role of Fermi Energy in Determining Properties of Superconductors: A Detailed Comparative Study of Two Elemental Superconductors (Sn and Pb), a Non-Cuprate (MgB2) and Three Cuptates (YBCO, Bi-2212 and Tl-2212). Journal of Superconductivity and Novel Magnetism, 29, 2755-2764. https://doi.org/10.1007/s10948-016-3637-5
- 3. Malik, G.P. (2018) Correction to: On the Role of Fermi Energy in Determining Properties of Superconductors: A Detailed Comparative Study of Two Elemental Superconductors (Sn and Pb), a Non-Cuprate (MgB2) and Three Cuptates (YBCO, Bi-2212 and Tl-2212). Journal of Superconductivity and Novel Magnetism, 31, 941-941. https://doi.org/10.1007/s10948-017-4520-8
- 4. Malik, G.P. (2016) Superconductivity: A New Approach Based on the Bethe-Salpeter Equation in the Mean-Field Approximation. In: Directions in Condensed Matter Physics, Vol. 21, World Scientific, Singapore. https://doi.org/10.1142/9868
- 5. Malik, G.P. (2018) On the s±-Wave Superconductivity in the Iron-Based Superconductors: A Perspective Based on a Detailed Study of Ba0.6K0.4Fe2AS2 via the Generalized-Bardeen-Cooper-Schrieffer Equations Incorporating Fermi Energy. Open Journal of Composite Materials, 7, 130-145. https://doi.org/10.4236/ojcm2017.73008


